Scalar Field Static Spherically Symmetric Solutions in Teleparallel F(T) Gravity
Abstract
1. Introduction
- Quintessence: This form describes a controlled accelerating universe expansion where energy conditions are always satisfied, i.e., [60,61,62,63,64,65,66,67,68,69,70,71,72]. This usual DE form has been significantly studied in the literature in recent decades for the fascination it provokes and the realism of the models.
- Phantom energy : This form can usually describe an uncontrolled universe expansion accelerating toward a Big Rip event (or singularity) [73,74,75,76,77,78,79,80,81]. The energy condition is violated, i.e., . But this DE form is fascinating because we can find new teleparallel solutions and physical models.
- Cosmological constant : This primary DE form is an intermediate limit between the quintessence and phantom DE states, where . A constant scalar field source added by a positive scalar potential will directly lead to this primary DE state. Note that a non-positive scalar potential (i.e., ) will not lead to a positive cosmological constant and/or a DE solution.
- Quintom models: This is a mixture of previous DE types, usually described by some double scalar field models [86,87,88,89,90,91]. This type of model is more complete to study and solve in general. Several types of models are in principle possible and these physical processes need further studies in the future.
2. Summary of Teleparallel Gravity and Field Equations
2.1. Summary of Teleparallel Field Equations
2.2. Static Spherically Symmetric Coframe and Spin-Connection Components
2.3. Static Scalar Field Energy-Momentum Source
2.4. Static Scalar Field Source Field Equations
3. Power-Law Scalar Field Solutions
- Ordinary matter limit ():By substituting Equation (25) into Equation (33), we find as :where is the characteristic equation solution defined from Equation (23). Equation (33) is a simple harmonic oscillator (SHO) potential. This last case is also valid for any coframe ansatz, not only the power-law case. Finally, Equation (18) becomes under this limit:Equation (35) leads to the ordinary matter (or dust) equivalent EoS under the limit (i.e., for any density of ordinary matter expression). This case describes an ordinary matter and/or a bosonic scalar field. For the current paper, we will consider this case as the ordinary matter limit because Equation (35) under the limit leads to .
- Quintessence: We must consider a value of higher than because of dark energy’s physical limit. Under the consideration of and from Equations (29), (32) and (35)’s respective results, we find the constraints to satisfy for the quintessence process asThe Equation (35) condition for leads to and this last case cannot lead to any DE scalar field. The constraint system of Equations (36)–(38) can yield, in principle, a significant number of possible solutions, because of terms inside each of the equations. Therefore, Equations (36)–(38) provide a minimal value for a characteristic equation solution and Equation (36) will lead to a maximal value for and p.
- Phantom energy: Because of in this type of model, we will find constraints from Equations (29) and (32) to satisfy:Equation (35), under the limit, cannot consistently lead to any phantom DE model. Once again, in the case of Equations (39) and (40), there are a significant number of possible solutions for and p. But the main point is that Equations (39) and (40) provide, respectively, a minimal and a maximal value for .
3.1. Power-Law Ansatz for
3.2. Power-Law Ansatz for
3.2.1. Flat Cosmological Case
- General ():
- : constant. Equation (51) will be a power-law like solution.
- Ordinary matter limit ():
- General ():
- : constant. Equation (54) will be a GR (TEGR-like) solution.
- Ordinary matter limit ():
3.2.2. General Cases
- (a)
- General ():
- (b)
- :where and .
- (c)
- Ordinary matter limit ():
- : Equation (47) will be
3.2.3. Cases
3.2.4. Cases
3.3. Other Ansatzes and Possible Comparisons with the Literature
4. Other Scalar Field Source Solutions
4.1. Exponential Scalar Field Solutions
- By combining Equations (98) and (99), we find in general that for positive and terms. The and classes of solutions found in Section 3.2.3 and Section 3.2.4 as the Section 3.2.1 and Section 3.2.2 are in principle all ideal candidates for describing quintessence processes.
- Phantom Energy: We only require that from Equation (96) asIn this case, we have primarily that the and ideally that term will be dominating for . Only the general solution obtained in Section 3.2.2 for positive values of a can be a candidate for phantom energy models with an exponential scalar field.
- Power-law ansatz with : We find the same solution form as Equation (43) in Section 3.1, but the potential will be Equation (95) with defined by Equation (41). Equation (95) becomes:where , , and .
- Power-law ansatz with : We find the same solution forms as in Section 3.2, but only the potential expressions change by replacing Equations (28), (31) and (34) by Equation (95) for each subcase treated in this section. Equation (95) will be for the simplest cases:
- (a)
- : The potential in Equation (51) iswhere and .
- (b)
- and : The expression in Equation (59) iswhere and .
- (c)
- (d)
- and : The expression in Equation (74) iswhere and .
- (e)
- and : The expression in Equation (78) iswhere and .
- (f)
- and : The expression in Equation (84) iswhere and .
- (g)
- and : The expression in Equation (88) iswhere and .
The other subcases of Section 3.2 can be computed by the same manner as in the previous simple examples.
4.2. Logarithmic Scalar Field Solutions
- By satisfying the Equation (112) criterion, we automatically satisfy Equation (113)’s requirement. To guarantee a -valued expression, it requires that in Equations (112) and (113) for a quintessence process. For the subcase, Equation (112)’s constraint simplifies as . Any Section 3.2 may lead in principle to quintessence solution.
- Phantom Energy: Equation (111) with requirement leads to the following constraint:For any positive and solution (or -valued), we need to satisfy the criterion. In a such case, only the Section 3.2.2 solutions may lead to the phantom energy models and any Section 3.2.3 and Section 3.2.4 cannot lead to this type of models (due to the negative values of a).
- Power-law ansatz with : As in Section 4.1, the solution is under the same form as Equation (43) in Section 3.1. The potential will be Equation (110) with defined by Equation (41) aswhere , , and .
- Power-law ansatz with : As in Section 4.1, we find the same solution forms than Section 3.2, but only the potential expressions change for the Equation (110) form for each subcases treated in this section. Equation (110) will be for the simplest cases:
- (a)
- : The potential in Equation (51) iswhere and .
- (b)
- and : The expression in Equation (59) iswhere and .
- (c)
- (d)
- and : The expression in Equation (74) iswhere and .
- (e)
- and : The expression in Equation (78) iswhere and .
- (f)
- and : The expression in Equation (84) iswhere and .
- (g)
- and : The expression in Equation (88) iswhere and .
As in Section 4.1, the other subcases of Section 3.2 can be computed in the same manner as in the current section examples.
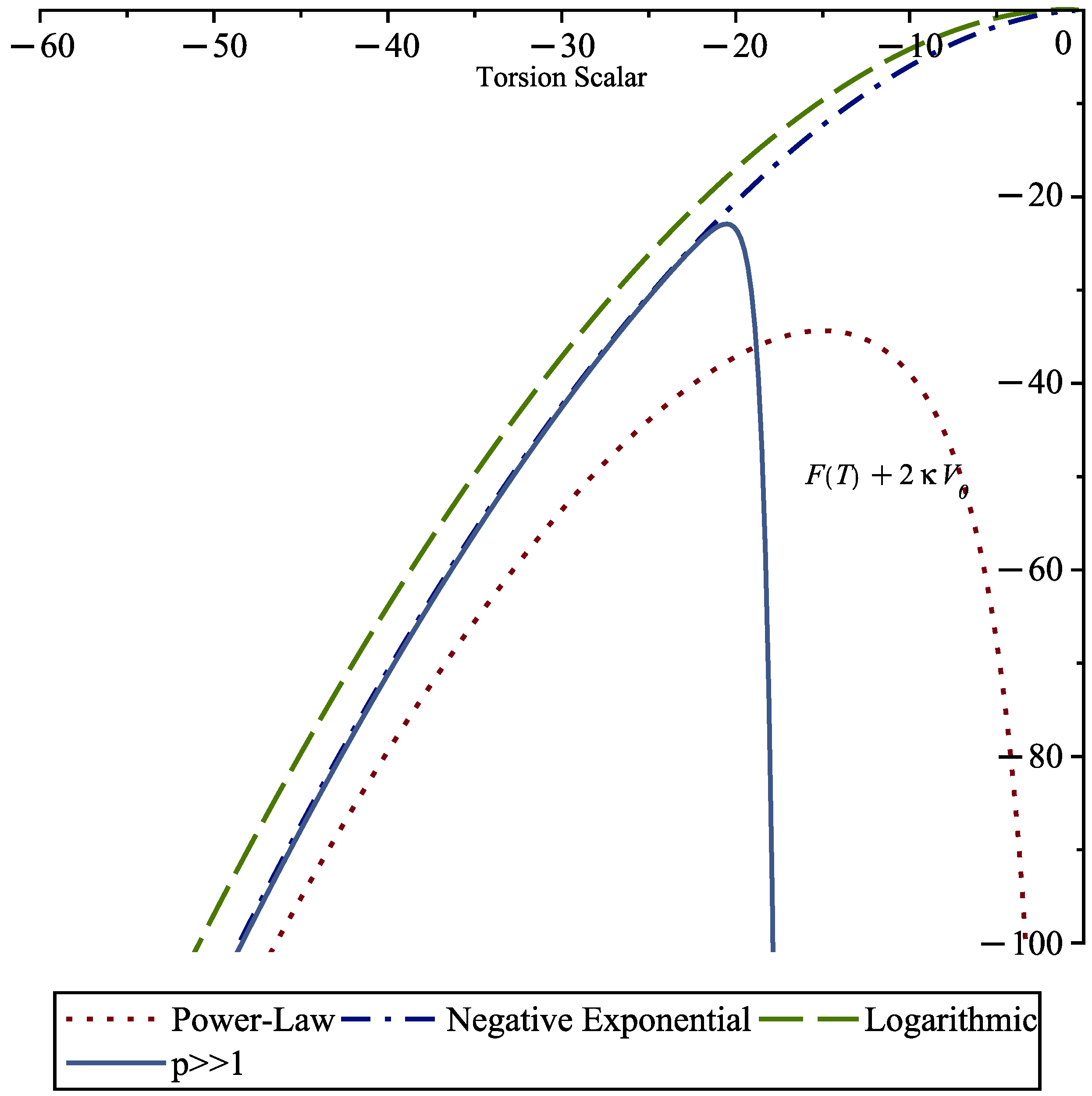
4.3. Graphical Comparisons and Summary of Main Results
Summary of Main Analytical Solutions
5. Concluding Remarks
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| AL | Alexandre Landry |
| DE | Dark Energy |
| EoS | Equation of State |
| GR | General Relativity |
| KV | Killing Vector |
| NS | Neutron star |
| TRW | Teleparallel Robertson–Walker |
| BH | Black Hole |
| DoF | Degree of Freedom |
| FE | Field Equation |
| KS | Kantowski–Sachs |
| NGR | New General Relativity |
| TEGR | Teleparallel Equivalent of General Relativity |
| WD | White Dwarf |
| Notation | |
| coordinate indices | |
| tangent space indices | |
| spacetime coordinates | |
| , , or | coframe expressions (tetrad for orthonormal frames) |
| , | spin-connection |
| gauge metric | |
| spacetime metric | |
| curvature tensor | |
| torsion tensor | |
| T | torsion scalar |
| superpotential | |
| teleparallel theory function of T | |
| , | derivatives with respect to (w.r.t) T |
| Einstein tensor | |
| , | conserved energy-momentum tensor |
| covariant derivative | |
| Lorentz Transformation | |
| hypermomentum | |
| scalar field | |
| radial coordinate derivative | |
Appendix A. Field Equation Components
Appendix A.1. General Components
Appendix A.2. A 3 =c 0 = Constant Power-Law Components
Appendix A.3. A 3 =r Power-Law Components
Appendix B. Tables of Section 3.2.3 and Section 3.2.4 Special Functions and Solutions
| b | ||
|---|---|---|
| N.A. | ||
| 1 | N.A. | |
| N.A. | ||
| 2 | ||
| N.A. | ||
| 3 | ||
| b | |
|---|---|
| 1 | |
| 2 | |
| 3 |
| b | ||
|---|---|---|
| 1 | ||
| N.A. | ||
| 2 | N.A. | |
| N.A. | ||
| 4 | ||
| N.A. | ||
| 6 | ||
| b | |
|---|---|
| 1 | |
| 2 | |
| 4 | |
| 6 | |
References
- Aldrovandi, R.; Pereira, J.G. Teleparallel Gravity: An Introduction; Springer: Dordrecht, The Netherlands, 2013. [Google Scholar]
- Bahamonde, S.; Dialektopoulos, K.; Escamilla-Rivera, C.; Farrugia, G.; Gakis, V.; Hendry, M.; Hohmann, M.; Said, J.L.; Mifsud, J.; Di Valentino, E. Teleparallel Gravity: From Theory to Cosmology. Rep. Prog. Phys. 2023, 86, 026901. [Google Scholar]
- Krssak, M.; van den Hoogen, R.; Pereira, J.; Boehmer, C.; Coley, A. Teleparallel Theories of Gravity: Illuminating a Fully Invariant Approach. Class. Quantum Gravity 2019, 36, 183001. [Google Scholar] [CrossRef]
- McNutt, D.D.; Coley, A.A.; van den Hoogen, R.J. A frame based approach to computing symmetries with non-trivial isotropy groups. J. Math. Phys. 2023, 64, 032503. [Google Scholar] [CrossRef]
- Coley, A.A.; van den Hoogen, R.J.; McNutt, D.D. Symmetry and Equivalence in Teleparallel Gravity. J. Math. Phys. 2020, 61, 072503. [Google Scholar] [CrossRef]
- Lucas, T.G.; Obukhov, Y.; Pereira, J.G. Regularizing role of teleparallelism. Phys. Rev. D 2009, 80, 064043. [Google Scholar] [CrossRef]
- Krssak, M.; Pereira, J.G. Spin Connection and Renormalization of Teleparallel Action. Eur. Phys. J. C 2015, 75, 519. [Google Scholar] [CrossRef]
- Chinea, F. Symmetries in tetrad theories. Class. Quantum Gravity 1988, 5, 135. [Google Scholar] [CrossRef]
- Estabrook, F.; Wahlquist, H. Moving frame formulations of 4-geometries having isometries. Class. Quantum Gravity 1996, 13, 1333. [Google Scholar] [CrossRef]
- Papadopoulos, G.; Grammenos, T. Locally homogeneous spaces, induced Killing vector fields and applications to Bianchi prototypes. J. Math. Phys. 2012, 53, 072502. [Google Scholar] [CrossRef]
- Olver, P. Equivalence, Invariants and Symmetry; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Ferraro, R.; Fiorini, F. Modified teleparallel gravity: Inflation without an inflation. Phys. Rev. D 2007, 75, 084031. [Google Scholar] [CrossRef]
- Ferraro, R.; Fiorini, F. On Born-Infeld Gravity in Weitzenbock spacetime. Phys. Rev. D 2008, 78, 124019. [Google Scholar] [CrossRef]
- Linder, E. Einstein’s Other Gravity and the Acceleration of the Universe. Phys. Rev. D 2010, 81, 127301, Erratum in Phys. Rev. D 2010, 82, 109902.. [Google Scholar] [CrossRef]
- Hayashi, K.; Shirafuji, T. New general relativity. Phys. Rev. D 1979, 19, 3524. [Google Scholar] [CrossRef]
- Jimenez, J.B.; Dialektopoulos, K.F. Non-Linear Obstructions for Consistent New General Relativity. J. Cosmol. Astropart. Phys. 2020, 2020, 018. [Google Scholar] [CrossRef]
- Bahamonde, S.; Blixt, D.; Dialektopoulos, K.F.; Hell, A. Revisiting Stability in New General Relativity. arXiv 2024, arXiv:2404.02972. [Google Scholar]
- Heisenberg, L. Review on f(Q) Gravity. arXiv 2023, arXiv:2311.05495. [Google Scholar] [CrossRef]
- Heisenberg, L.; Hohmann, M.; Kuhn, S. Cosmological teleparallel perturbations. arXiv 2023, arXiv:2311.05495. [Google Scholar] [CrossRef]
- Flathmann, K.; Hohmann, M. Parametrized post-Newtonian limit of generalized scalar-nonmetricity theories of gravity. Phys. Rev. D 2022, 105, 044002. [Google Scholar] [CrossRef]
- Hohmann, M. General covariant symmetric teleparallel cosmology. Phys. Rev. D 2021, 104, 124077. [Google Scholar] [CrossRef]
- Jimenez, J.B.; Heisenberg, L.; Koivisto, T.S. The Geometrical Trinity of Gravity. Universe 2019, 5, 173. [Google Scholar] [CrossRef]
- Nakayama, Y. Geometrical trinity of unimodular gravity. Class. Quantum Gravity 2023, 40, 125005. [Google Scholar] [CrossRef]
- Xu, Y.; Li, G.; Harko, T.; Liang, S.-D. f(Q,T) gravity. Eur. Phys. J. C 2019, 79, 708. [Google Scholar] [CrossRef]
- Maurya, D.C.; Yesmakhanova, K.; Myrzakulov, R.; Nugmanova, G. Myrzakulov, FLRW Cosmology in Myrzakulov F(R,Q) Gravity. arXiv 2024, arXiv:2403.11604. [Google Scholar]
- Maurya, D.C.; Myrzakulov, R. Exact Cosmology in Myrzakulov Gravity. arXiv 2024, arXiv:2402.02123. [Google Scholar]
- Harko, T.; Lobo, F.S.N.; Nojiri, S.; Odintsov, S.D. f(R,T) gravity. Phys. Rev. D 2011, 84, 024020. [Google Scholar] [CrossRef]
- Momeni, D.; Myrzakulov, R. Myrzakulov Gravity in Vielbein Formalism: A Study in Weitzenböck Spacetime. arXiv 2024, arXiv:2412.04524. [Google Scholar]
- Maurya, D.C.; Yesmakhanova, K.; Myrzakulov, R.; Nugmanova, G. Myrzakulov F(T,Q) gravity: Cosmological implications and constraints. Phys. Scr. 2024, 99, 10. [Google Scholar] [CrossRef]
- Maurya, D.C.; Yesmakhanova, K.; Myrzakulov, R.; Nugmanova, G. FLRW Cosmology in Metric-Affine F(R,Q) Gravity. Chin. Phys. C 2024, 48, 125101. [Google Scholar] [CrossRef]
- Maurya, D.C.; Myrzakulov, R. Transit cosmological models in Myrzakulov F(R,T) gravity theory. Eur. Phys. J. C 2024, 84, 534. [Google Scholar] [CrossRef]
- Mandal, S.; Myrzakulov, N.; Sahoo, P.K.; Myrzakulov, R. Cosmological bouncing scenarios in symmetric teleparallel gravity. Eur. Phys. J. Plus 2021, 136, 760. [Google Scholar] [CrossRef]
- Coley, A.A.; Landry, A.; van den Hoogen, R.J.; McNutt, D.D. Generalized Teleparallel de Sitter geometries. Eur. Phys. J. C 2023, 83, 977. [Google Scholar] [CrossRef]
- Coley, A.A.; Landry, A.; van den Hoogen, R.J.; McNutt, D.D. Spherically symmetric teleparallel geometries. Eur. Phys. J. C 2024, 84, 334. [Google Scholar] [CrossRef] [PubMed]
- Landry, A. Static spherically symmetric perfect fluid solutions in teleparallel F(T) gravity. Axioms 2024, 13, 333. [Google Scholar] [CrossRef]
- Landry, A. Kantowski-Sachs spherically symmetric solutions in teleparallel F(T) gravity. Symmetry 2024, 16, 953. [Google Scholar] [CrossRef]
- van den Hoogen, R.J.; Forance, H. Teleparallel Geometry with Spherical Symmetry: The diagonal and proper frames. J. Cosmol. Astrophys. 2024, 11, 033. [Google Scholar] [CrossRef]
- Landry, A. Scalar field Kantowski-Sachs spacetime solutions in teleparallel F(T) gravity. Universe 2025, 11, 26. [Google Scholar] [CrossRef]
- Landry, A. Scalar field sources Teleparallel Robertson-Walker F(T)-gravity solutions. Mathematics 2025, 13, 374. [Google Scholar] [CrossRef]
- Coley, A.A.; Landry, A.; Gholami, F. Teleparallel Robertson-Walker Geometries and Applications. Universe 2023, 9, 454. [Google Scholar] [CrossRef]
- Golovnev, A.; Guzman, M.-J. Approaches to spherically symmetric solutions in f(T)-gravity. Universe 2021, 7, 121. [Google Scholar] [CrossRef]
- Golovnev, A. Issues of Lorentz-invariance in f(T)-gravity and calculations for spherically symmetric solutions. Class. Quantum Gravity 2021, 38, 197001. [Google Scholar] [CrossRef]
- Golovnev, A.; Guzman, M.-J. Bianchi identities in f(T)-gravity: Paving the way to confrontation with astrophysics. Phys. Lett. B 2020, 810, 135806. [Google Scholar] [CrossRef]
- DeBenedictis, A.; Ilijić, S.; Sossich, M. On spherically symmetric vacuum solutions and horizons in covariant f(T) gravity theory. Phys. Rev. D 2022, 105, 084020. [Google Scholar] [CrossRef]
- Bahamonde, S.; Camci, U. Exact Spherically Symmetric Solutions in Modified Teleparallel gravity. Symmetry 2019, 11, 1462. [Google Scholar] [CrossRef]
- Awad, A.; Golovnev, A.; Guzman, M.-J.; El Hanafy, W. Revisiting diagonal tetrads: New Black Hole solutions in f(T)-gravity. Eur. Phys. J. C 2022, 82, 972. [Google Scholar] [CrossRef]
- Bahamonde, S.; Golovnev, A.; Guzmán, M.-J.; Said, J.L.; Pfeifer, C. Black Holes in f(T,B) Gravity: Exact and Perturbed Solutions. J. Cosmol. Astropart. Phys. 2022, 1, 037. [Google Scholar] [CrossRef]
- Bahamonde, S.; Faraji, S.; Hackmann, E.; Pfeifer, C. Thick accretion disk configurations in the Born-Infeld teleparallel gravity. Phys. Rev. D 2022, 106, 084046. [Google Scholar] [CrossRef]
- Nashed, G.G.L. Quadratic and cubic spherically symmetric black holes in the modified teleparallel equivalent of general relativity: Energy and thermodynamics. Class. Quantum Gravity 2021, 38, 125004. [Google Scholar] [CrossRef]
- Pfeifer, C.; Schuster, S. Static spherically symmetric black holes in weak f(T)-gravity. Universe 2021, 7, 153. [Google Scholar] [CrossRef]
- El Hanafy, W.; Nashed, G.G.L. Exact Teleparallel Gravity of Binary Black Holes. Astrophys. Space Sci. 2016, 361, 68. [Google Scholar] [CrossRef]
- Aftergood, J.; DeBenedictis, A. Matter Conditions for Regular Black Holes in f(T) Gravity. Phys. Rev. D 2014, 90, 124006. [Google Scholar] [CrossRef]
- Bahamonde, S.; Doneva, D.D.; Ducobu, L.; Pfeifer, C.; Yazadjiev, S.S. Spontaneous Scalarization of Black Holes in Gauss-Bonnet Teleparallel Gravity. Phys. Rev. D 2023, 107, 104013. [Google Scholar] [CrossRef]
- Bahamonde, S.; Ducobu, L.; Pfeifer, C. Scalarized Black Holes in Teleparallel Gravity. J. Cosmol. Astropart. Phys. 2022, 2022, 018. [Google Scholar] [CrossRef]
- Iorio, L.; Radicella, N.; Ruggiero, M.L. Constraining f(T) gravity in the Solar System. J. Cosmol. Astropart. Phys. 2015, 2015, 021. [Google Scholar] [CrossRef]
- Pradhan, S.; Bhar, P.; Mandal, S.; Sahoo, P.K.; Bamba, K. The Stability of Anisotropic Compact Stars Influenced by Dark Matter under Teleparallel Gravity: An Extended Gravitational Deformation Approach. Eur. Phys. J. C 2025, 85, 127. [Google Scholar] [CrossRef]
- Mohanty, D.; Ghosh, S.; Sahoo, P.K. Charged gravastar model in noncommutative geometry under f(T) gravity. Phys. Dark Universe 2025, 46, 101692. [Google Scholar] [CrossRef]
- Calza, M.; Sebastiani, L. A class of static spherically symmetric solutions in f(T)-gravity. Eur. Phys. J. C 2024, 84, 476. [Google Scholar] [CrossRef]
- Gholami, F.; Landry, A. Cosmological solutions in teleparallel F(T,B) gravity. Symmetry 2025, 17, 060. [Google Scholar] [CrossRef]
- Zlatev, I.; Wang, L.; Steinhardt, P. Quintessence, Cosmic Coincidence, and the Cosmological Constant. Phys. Rev. Lett. 1999, 82, 896. [Google Scholar] [CrossRef]
- Steinhardt, P.; Wang, L.; Zlatev, I. Cosmological tracking solutions. Phys. Rev. D 1999, 59, 123504. [Google Scholar] [CrossRef]
- Caldwell, R.R.; Dave, R.; Steinhardt, P. Cosmological Imprint of an Energy Component with General Equation of State. Phys. Rev. Lett. 1998, 80, 1582. [Google Scholar] [CrossRef]
- Carroll, S.M. Quintessence and the Rest of the World. Phys. Rev. Lett. 1998, 81, 3067. [Google Scholar] [CrossRef]
- Doran, M.; Lilley, M.; Schwindt, J.; Wetterich, C. Quintessence and the Separation of CMB Peaks. Astrophys. J. 2001, 559, 501. [Google Scholar] [CrossRef]
- Zeng, X.-X.; Chen, D.-Y.; Li, L.-F. Holographic thermalization and gravitational collapse in the spacetime dominated by quintessence dark energy. Phys. Rev. D 2015, 91, 046005. [Google Scholar] [CrossRef]
- Chakraborty, S.; Mishra, S.; Chakraborty, S. Dynamical system analysis of quintessence dark energy model. Int. J. Geom. Methods Mod. Phys. 2025, 22, 2450250. [Google Scholar] [CrossRef]
- Shlivko, D.; Steinhardt, P.J. Assessing observational constraints on dark energy. Phys. Lett. B 2024, 855, 138826. [Google Scholar] [CrossRef]
- Wetterich, C. Cosmology and the Fate of Dilatation Symmetry. Nucl. Phys. B 1988, 302, 668. [Google Scholar] [CrossRef]
- Ratra, B.; Peebles, P.J.E. Cosmological consequences of a rolling homogeneous scalar field. Phys. Rev. D 1988, 37, 3406. [Google Scholar] [CrossRef]
- Wolf, W.J.; Ferreira, P.G. Underdetermination of dark energy. Phys. Rev. D 2023, 108, 103519. [Google Scholar] [CrossRef]
- Wolf, W.J.; García-García, C.; Bartlett, D.J.; Ferreira, P.G. Scant evidence for thawing quintessence. Phys. Rev. D 2024, 110, 083528. [Google Scholar] [CrossRef]
- Wolf, W.J.; Ferreira, P.G.; García-García, C. Matching current observational constraints with nonminimally coupled dark energy. arXiv 2024, arXiv:2409.17019. [Google Scholar] [CrossRef]
- Chiba, T.; Okabe, T.; Yamaguchi, M. Kinetically Driven Quintessence. Phys. Rev. D 2000, 62, 023511. [Google Scholar] [CrossRef]
- Carroll, S.M.; Hoffman, M.; Trodden, M. Can the dark energy equation-of-state parameter w be less than ™1? Phys. Rev. D 2003, 68, 023509. [Google Scholar] [CrossRef]
- Caldwell, R.R. A phantom menace? Cosmological consequences of a dark energy component with super-negative equation of state. Phys. Lett. B 2002, 545, 23. [Google Scholar] [CrossRef]
- Farnes, J.S. A Unifying Theory of Dark Energy and Dark Matter: Negative Masses and Matter Creation within a Modified ΛCDM Framework. Astron. Astrophys. 2018, 620, A92. [Google Scholar] [CrossRef]
- Baum, L.; Frampton, P.H. Turnaround in Cyclic Cosmology. Phys. Rev. Lett. 2007, 98, 071301. [Google Scholar] [CrossRef]
- Hu, W. Crossing the Phantom Divide: Dark Energy Internal Degrees of Freedom. Phys. Rev. D 2005, 71, 047301. [Google Scholar] [CrossRef]
- Karimzadeh, S.; Shojaee, R. Phantom-Like Behavior in Modified Teleparallel Gravity. Adv. High Energy Phys. 2019, 2019, 4026856. [Google Scholar] [CrossRef]
- Pati, L.; Kadam, S.A.; Tripathy, S.K.; Mishra, B. Rip cosmological models in extended symmetric teleparallel gravity. Phys. Dark Universe 2022, 35, 100925. [Google Scholar] [CrossRef]
- Kucukakca, Y.; Akbarieh, A.R.; Ashrafi, S. Exact solutions in teleparallel dark energy model. Chin. J. Phys. 2023, 82, 47. [Google Scholar] [CrossRef]
- Duchaniya, L.K.; Gandhi, K.; Mishra, B. Attractor behavior of f(T) modified gravity and the cosmic acceleration. Phys. Dark Universe 2024, 44, 101464. [Google Scholar] [CrossRef]
- Paliathanasis, A.; Leon, G. f(T,B) gravity in a Friedmann-Lemaitre-Robertson-Walker universe with nonzero spatial curvature. Math. Methods Appl. Sci. 2022, 46, 3905–3922. [Google Scholar] [CrossRef]
- Kofinas, G.; Leon, G.; Saridakis, E.N. Dynamical behavior in f(T,TG) cosmology. Class. Quantum Gravity 2014, 31, 175011. [Google Scholar] [CrossRef]
- Leon, G.; Paliathanasis, A. Anisotropic spacetimes in f(T,B) theory III: LRS Bianchi III Universe. Eur. Phys. J. Plus 2022, 137, 927. [Google Scholar] [CrossRef]
- Cai, Y.-F.; Saridakis, E.N.; Setare, M.R.; Xia, J.-Q. Quintom Cosmology: Theoretical implications and observations. Phys. Rep. 2010, 493, 1–60. [Google Scholar] [CrossRef]
- Guo, Z.-K.; Piao, Y.-S.; Zhang, X.; Zhang, Y.-Z. Cosmological evolution of a quintom model of dark energy. Phys. Lett. B 2005, 608, 177. [Google Scholar] [CrossRef]
- Feng, B.; Li, M.; Piao, Y.-S.; Zhang, X. Oscillating quintom and the recurrent universe. Phys. Lett. B 2006, 634, 101. [Google Scholar] [CrossRef]
- Mishra, S.; Chakraborty, S. Dynamical system analysis of quintom dark energy model. Eur. Phys. J. C 2018, 78, 917. [Google Scholar] [CrossRef]
- Tot, J.; Coley, A.A.; Yildrim, B.; Leon, G. The dynamics of scalar-field Quintom cosmological models. Phys. Dark Universe 2023, 39, 101155. [Google Scholar] [CrossRef]
- Bahamonde, S.; Marciu, M.; Rudra, P. Generalised teleparallel quintom dark energy non-minimally coupled with the scalar torsion and a boundary term. J. Cosmol. Astropart. Phys. 2018, 04, 056. [Google Scholar] [CrossRef]
- Iosifidis, D. Cosmological Hyperfluids, Torsion and Non-metricity. Eur. Phys. J. C 2020, 80, 1042. [Google Scholar] [CrossRef]
- Heisenberg, L.; Hohmann, M.; Kuhn, S. Homogeneous and isotropic cosmology in general teleparallel gravity. Eur. Phys. J. C 2023, 83, 315. [Google Scholar] [CrossRef]
- Heisenberg, L.; Hohmann, M. Gauge-invariant cosmological perturbations in general teleparallel gravity. Eur. Phys. J. C 2024, 84, 462. [Google Scholar] [CrossRef]
- Sharif, M.; Majeed, B. Teleparallel Killing Vectors of Spherically Symmetric Spacetimes. Commun. Theor. Phys. 2009, 52, 435. [Google Scholar] [CrossRef]
- Hohmann, M.; Jarv, L.; Krssak, M.; Pfeifer, C. Modified teleparallel theories of gravity in symmetric spacetimes. Phys. Rev. D 2019, 100, 084002. [Google Scholar] [CrossRef]
- Pfeifer, C. A quick guide to spacetime symmetry and symmetric solutions in teleparallel gravity. arXiv 2022, arXiv:2201.04691. [Google Scholar]
- Hohmann, M. Scalar-torsion theories of gravity III: Analogue of scalar-tensor gravity and conformal invariants. Phys. Rev. D 2018, 98, 064004. [Google Scholar] [CrossRef]
- Hawking, S.W.; Ellis, G.F.R. The Large Scale Structure of Space-Time; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Coley, A.A. Dynamical Systems and Cosmology; Kluwer Academic: Dordrecht, The Netherlands, 2003. [Google Scholar]
- Bohmer, C.G.; d’Alfonso del Sordo, A. Cosmological fluids with boundary term couplings. Gen. Relativ. Gravit. 2024, 56, 75. [Google Scholar] [CrossRef]
- Sharif, M.; Rani, S. Generalized teleparallel gravity via some scalar field dark energy models. Astrophys. Space Sci. 2013, 345, 217. [Google Scholar] [CrossRef]
- Gonzalez, P.A.; Papantonopoulos, E.; Robledo, J.; Vasquez, Y. Nonlinear Scalarization of Schwarzschild Black Hole in Scalar-Torsion Teleparallel Gravity. Phys. Rev. D 2025, 111, 044064. [Google Scholar] [CrossRef]
- Jusufi, K.; Capozziello, S.; Bahamonde, S.; Jamil, M. Testing Born-Infeld f(T) teleparallel gravity through Sgr A* observations. Eur. Phys. J. C 2022, 82, 1018. [Google Scholar] [CrossRef]
- Landry, A.; van den Hoogen, R.J. Teleparallel Minkowski Spacetime with Perturbative Approach for Teleparallel Theories on the Proper Frame. Universe 2013, 9, 232. [Google Scholar] [CrossRef]



| a | 0 | |||
| b | 0 | 0, , | 0, , , , 3 | 0, , , , , , |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Landry, A. Scalar Field Static Spherically Symmetric Solutions in Teleparallel F(T) Gravity. Mathematics 2025, 13, 1003. https://doi.org/10.3390/math13061003
Landry A. Scalar Field Static Spherically Symmetric Solutions in Teleparallel F(T) Gravity. Mathematics. 2025; 13(6):1003. https://doi.org/10.3390/math13061003
Chicago/Turabian StyleLandry, Alexandre. 2025. "Scalar Field Static Spherically Symmetric Solutions in Teleparallel F(T) Gravity" Mathematics 13, no. 6: 1003. https://doi.org/10.3390/math13061003
APA StyleLandry, A. (2025). Scalar Field Static Spherically Symmetric Solutions in Teleparallel F(T) Gravity. Mathematics, 13(6), 1003. https://doi.org/10.3390/math13061003






