Nonlinear Dynamical Analysis and New Solutions of the Space-Fractional Stochastic Davey–Stewartson Equations for Nonlinear Water Waves
Abstract
1. Introduction
2. Mathematical Analysis
3. Analysis of Bifurcation
- (a)
- (b)
- When (), O is a maximum of the potential function (18) and is therefore a saddle point, while are minima and thus serve as center points. Conversely, when (), O becomes a minimum of the potential function (18), making it a center, whereas are maxima and therefore saddle points. See Figure 1c,d for visualization. The corresponding phase portraits are shown in Figure 3.
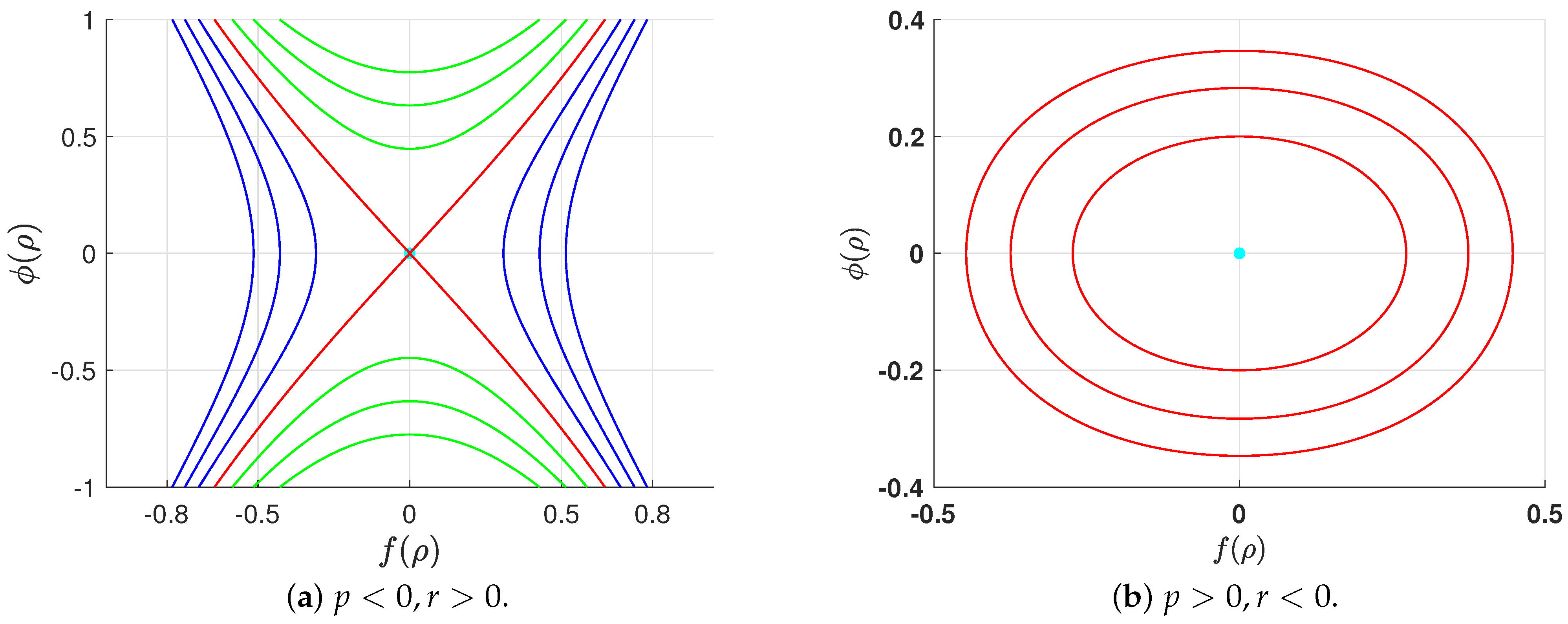
Phase Portrait Description
- (a)
- (b)
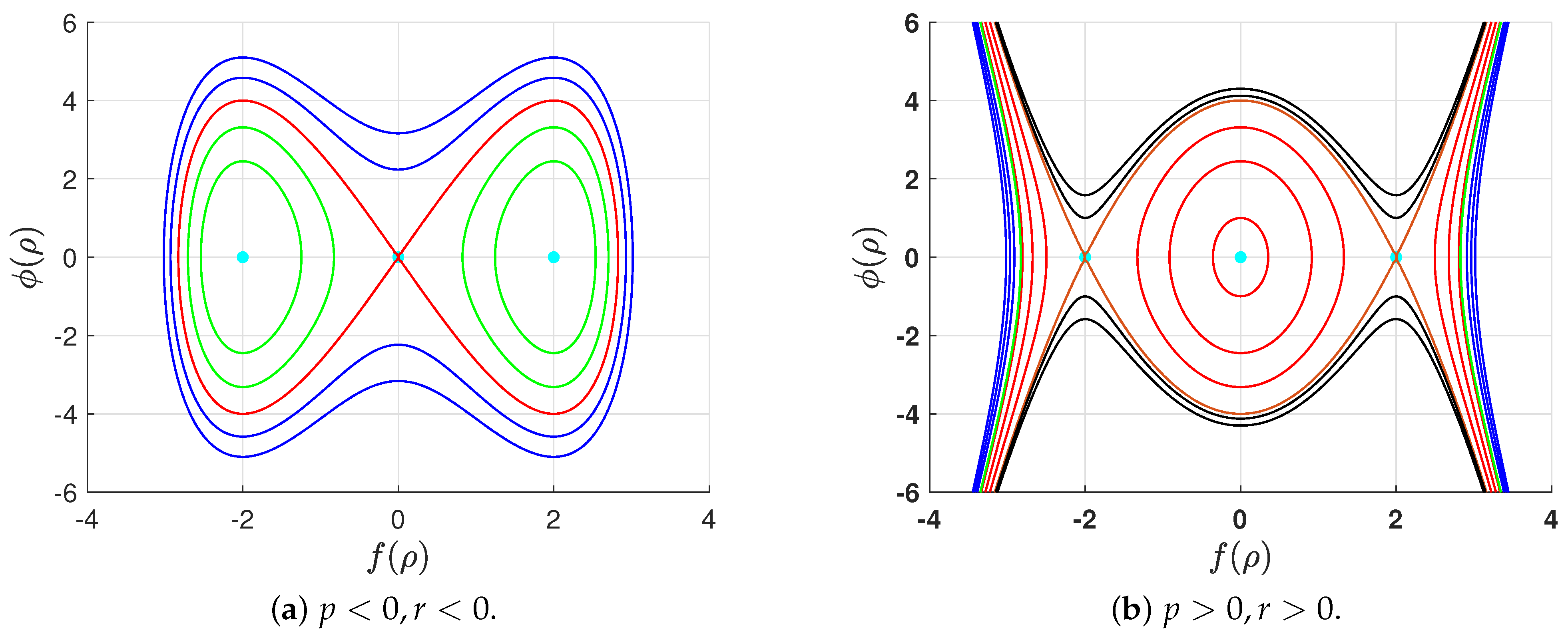
- (c)
- For , the system (15) has three equilibrium points, with O being a saddle point and center points. All phase plane orbits are bounded for all values of the parameter , as shown in Figure 3a. The system (15) has a homoclinic orbit in red, two families of periodic orbits in green, and a family of super periodic orbits in blue for , , and , respectively.
- (d)
- When , there are three equilibrium points for the system (15), with O being a center point and saddle points, as displayed by Figure 3b. If , the system has a heteroclinic orbit, shown in brown, connecting the two saddle points , along with its unbounded extension. For , there are three families of orbits in red: one periodic family located inside the heteroclinic orbit, and two unbounded families located outside the heteroclinic orbit. The system has two families of unbounded orbits in black when in addition to a green orbit when . For , there are two families of unbounded orbits in blue.
4. Solutions
- (a)
- When , the polynomial (20) has two real roots, , and two purely imaginary roots, . Thus, it takes the form . The interval of real solutions for is . By taking and integrating both sides of Equation (19), we obtainwhere is a Jacobi elliptic function [45]. Thus, the solution of Equation (2) becomeswhere is the incomplete elliptic integral of the second kind, defined aswhere u and k represent the amplitude and elliptic modulus, respectively, with for real applications. For further details on Jacobi elliptic functions, see, e.g., [45]. Solution (24) represents a novel solution to Equation (2).
- (b)
- For , we construct different types of solutions based on the various values of .
- i.
- For positive values of , the system (15) exhibits a family of super periodic orbits, shown in blue in Figure 3a. A single orbit from this family intersects the axis at two points. Therefore, the polynomial (20) has two real roots, , and two purely imaginary roots, , and thus has the form . By taking , and integrating both sides of Equation (19), we obtainwhere denotes the Jacobi elliptic function [45]. Employing Equations (4) and (11), a solution to Equation (2) is found in the formThis expression gives a new solution for Equation (2).
- ii.
- At the zero level of the parameter , there is a homoclinic orbit in red for the system (15), as displayed by Figure 3a. This indicates that the polynomial has a double root at the origin and two simple roots at . Thus, we have . Taking and integrating both sides of Equation (19), we obtainEquation (29) provides a novel solution to Equation (2).
- iii.
- At a fixed value for the parameter , the system has two families of periodic orbits, as shown in green in Figure 3a. In this case, the polynomial (20) has four real roots, and , where , and, hence, it has the form . The function is real when . Let . Integration of both sides of Equation (19) provideswhere is a Jacobi elliptic function [45]. A new solution to Equation (2) is found by utilizing both Equations (4) and (11). Therefore, we have
- (c)
- When , the solutions of Equation (2) depend on the values of .
- i.
- For , any orbit of the system (15) intersects the axis at four points, as shown in red in Figure 3b. This means that the polynomial (20) has four real roots, and . Therefore, it can be written as , where . The function is real and bounded in the interval . By taking and integrating both sides of Equation (19), we obtain
- ii.
- On the level , the system (15) has a heteroclinic orbit, as shown in brown in Figure 3b. This indicates that the polynomial has two double roots which are the coordinates for the saddle points . Hence, takes the form . The function is real if . Taking and integrating both sides of Equation (19), we obtain
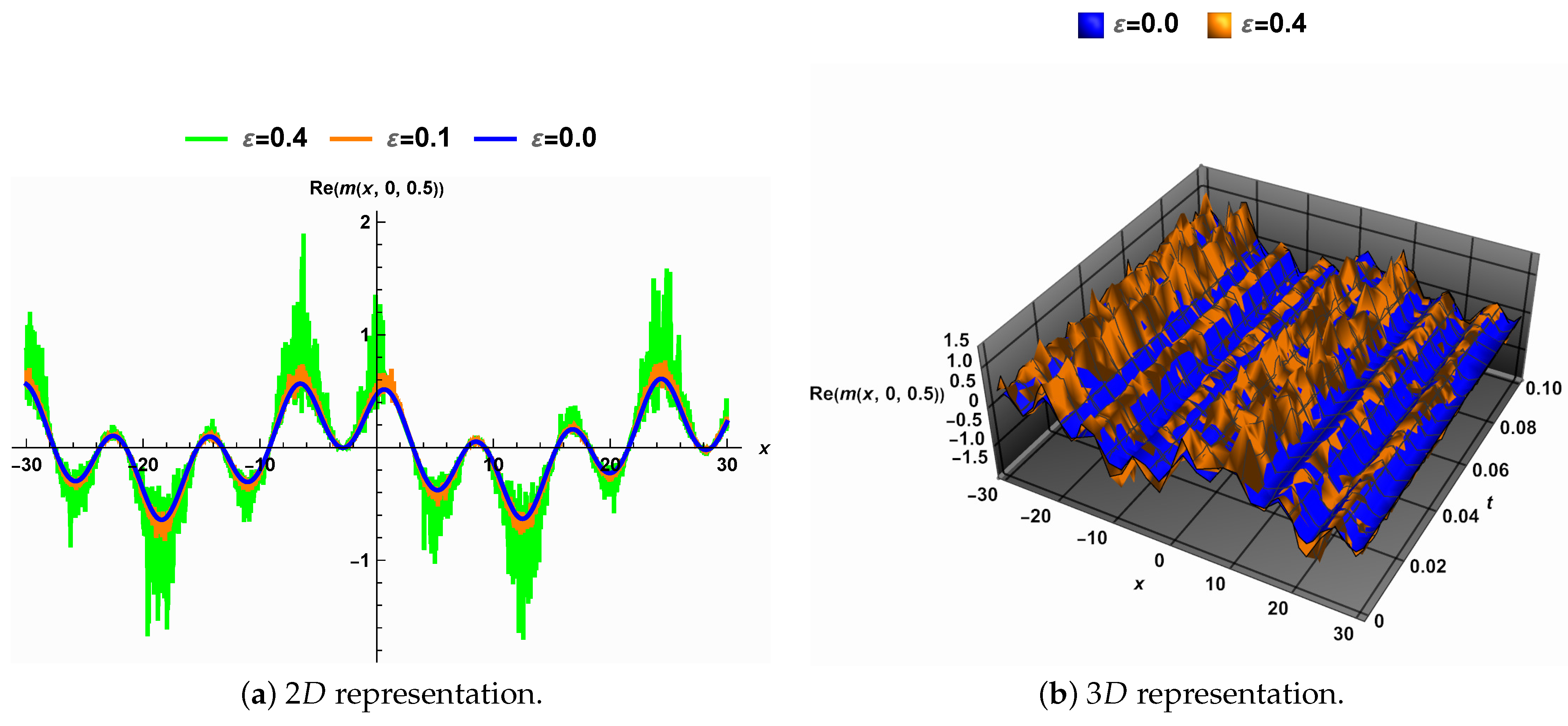
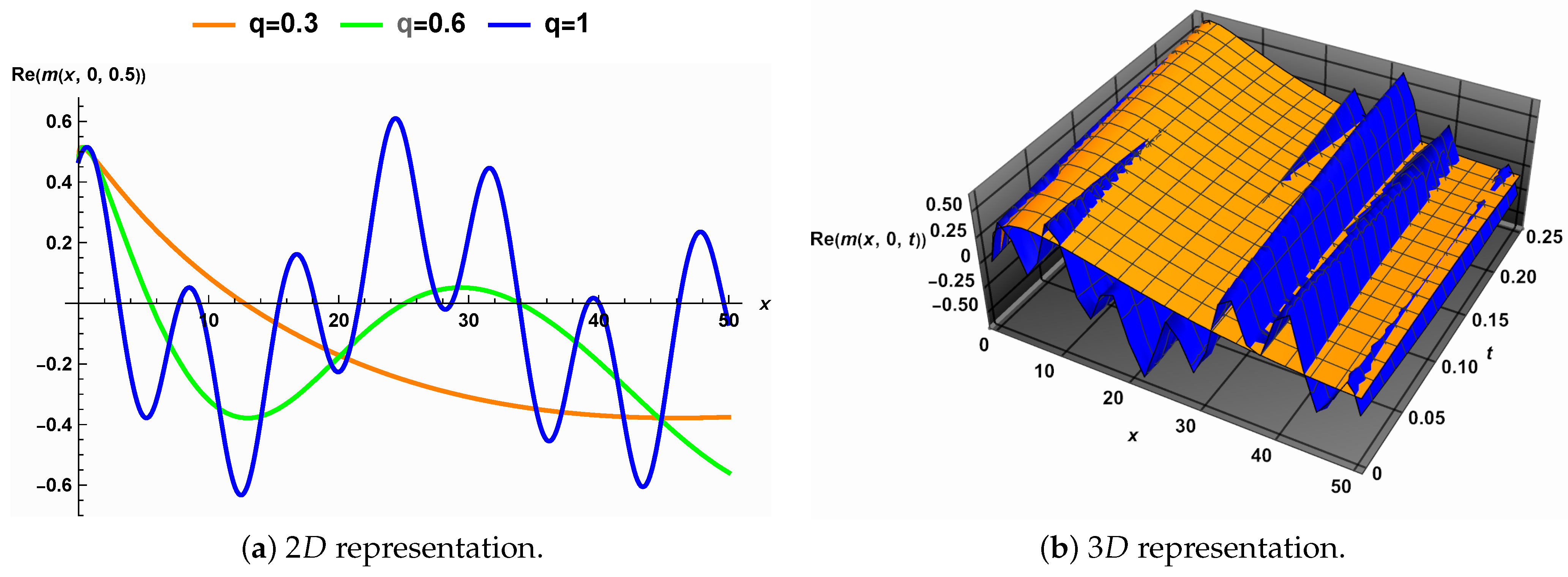
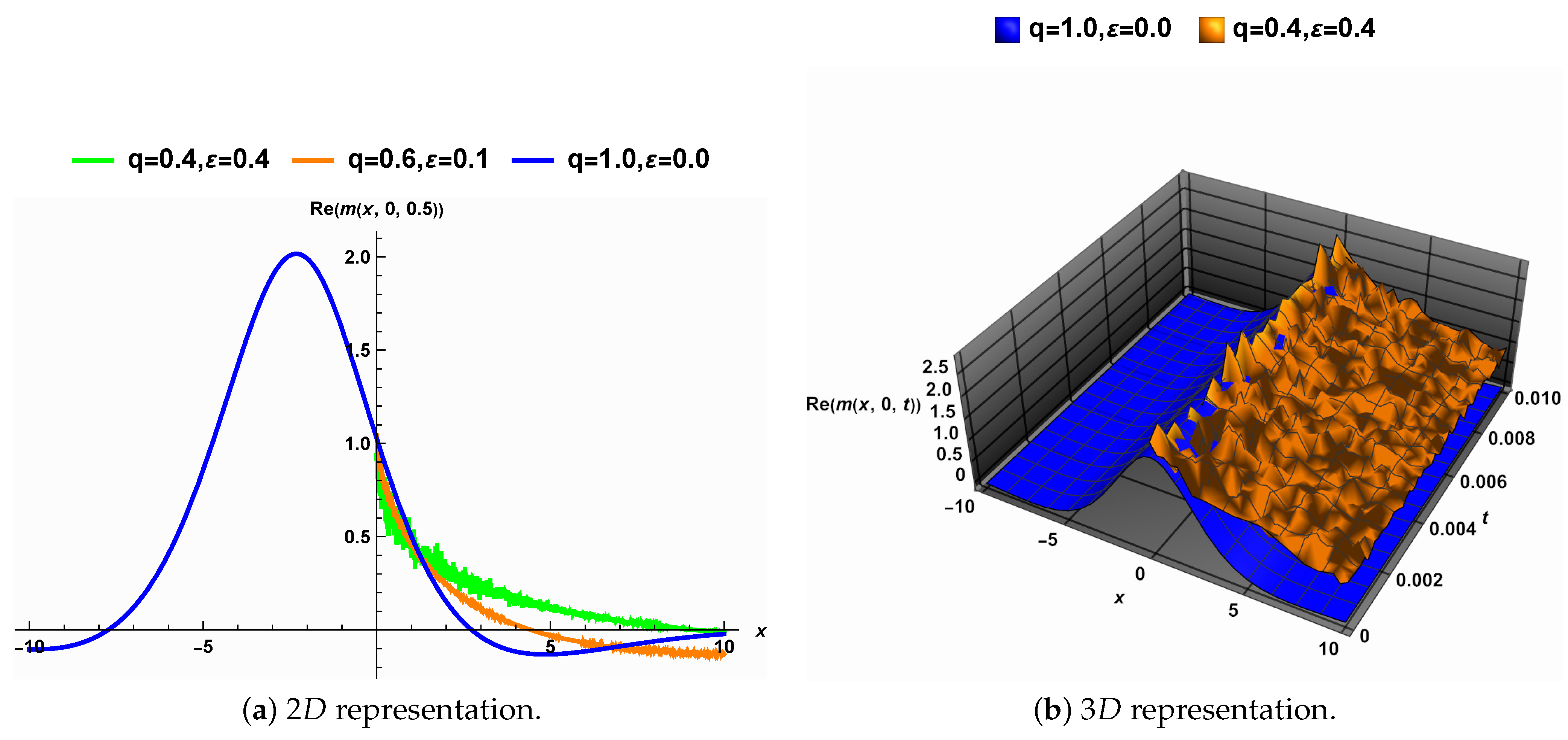
5. Physical Interpretation
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Riemann–Liouville Fractional Derivative Modified by Jumarie and the Standard Wiener Process
- (a)
- , where .
- (b)
- for any differentiable function g at x, in the sense of Jumarie’s modified Riemann–Liouville form.
- (a)
- (Initial Condition):;
- (b)
- (Continuity): is a continuous function for ;
- (c)
- (Independent Increments):For , and are independent;
- (d)
- (Independent Increments):
Appendix B. Hamiltonian Concepts
References
- Davey, A.; Stewartson, K. On three-dimensional packets of surface waves. Proc. R. Soc. London. A. Math. Phys. Sci. 1974, 338, 101–110. [Google Scholar]
- Freeman, N.; Davey, A. On the evolution of packets of long surface waves. Proc. R. Soc. London. A. Math. Phys. Sci. 1975, 344, 427–433. [Google Scholar]
- Zhou, Z.; Ma, W.X.; Zhou, R. Finite-dimensional integrable systems associated with the Davey-Stewartson I equation. Nonlinearity 2001, 14, 701. [Google Scholar] [CrossRef]
- Zedan, H.A.; Monaquel, S.J. The sine-cosine method for the Davey-Stewartson equations. Appl. Math. E-Notes 2010, 10, 103–111. [Google Scholar]
- Ebadi, G.; Biswas, A. The G′/G method and 1-soliton solution of the Davey–Stewartson equation. Math. Comput. Model. 2011, 53, 694–698. [Google Scholar] [CrossRef]
- Abdelaziz, M.A.; Moussa, A.; Alrahal, D. Exact Solutions for the Nonlinear (2+1)-Dimensional Davey-Stewartson Equation Using the Generalized (G′G)-Expansion Method. J. Math. Res. 2014, 6, 91–99. [Google Scholar] [CrossRef][Green Version]
- Aghdaei, M.F.; Manafian, J. Optical soliton wave solutions to the resonant Davey–Stewartson system. Opt. Quantum Electron. 2016, 48, 1–33. [Google Scholar] [CrossRef]
- Gaballah, M.; El-Shiekh, R.M.; Akinyemi, L.; Rezazadeh, H. Novel periodic and optical soliton solutions for Davey–Stewartson system by generalized Jacobi elliptic expansion method. Int. J. Nonlinear Sci. Numer. Simul. 2024, 24, 2889–2897. [Google Scholar] [CrossRef]
- Mirzazadeh, M. Soliton solutions of Davey–Stewartson equation by trial equation method and ansatz approach. Nonlinear Dyn. 2015, 82, 1775–1780. [Google Scholar] [CrossRef]
- Jafari, H.; Sooraki, A.; Talebi, Y.; Biswas, A. The first integral method and traveling wave solutions to Davey–Stewartson equation. Nonlinear Anal. Model. Control 2012, 17, 182–193. [Google Scholar] [CrossRef]
- Gao, X.Y. Hetero-Bäcklund transformation, bilinear forms and multi-solitons for a (2+1)-dimensional generalized modified dispersive water-wave system for the shallow water. Chin. J. Phys. 2024, 92, 1233–1239. [Google Scholar] [CrossRef]
- Gao, X.Y. Symbolic computation on a (2+1)-dimensional generalized nonlinear evolution system in fluid dynamics, plasma physics, nonlinear optics and quantum mechanics. Qual. Theory Dyn. Syst. 2024, 23, 202. [Google Scholar] [CrossRef]
- Gao, X.Y. In an ocean or a river: Bilinear auto-Bäcklund transformations and similarity reductions on an extended time-dependent (3+1)-dimensional shallow water wave equation. China Ocean Eng. 2025. [Google Scholar] [CrossRef]
- Shan, H.W.; Tian, B.; Cheng, C.D.; Gao, X.T.; Chen, Y.Q.; Liu, H.D. N-soliton and other analytic solutions for a (3+1)-dimensional Korteweg–de Vries–Calogero–Bogoyavlenskii–Schiff equation with the time-dependent coefficients for the shallow water waves. Qual. Theory Dyn. Syst. 2024, 23, 267. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Iqbal, N.; Botmart, T. Additive noise effects on the stabilization of fractional-space diffusion equation solutions. Mathematics 2022, 10, 130. [Google Scholar] [CrossRef]
- Yuste, S.B.; Lindenberg, K. Subdiffusion-limited A+ A reactions. Phys. Rev. Lett. 2001, 87, 118301. [Google Scholar] [CrossRef] [PubMed]
- Mohammed, W.W. Fast-diffusion limit for reaction–diffusion equations with degenerate multiplicative and additive noise. J. Dyn. Differ. Equa. 2021, 33, 577–592. [Google Scholar] [CrossRef]
- Peter, O.J.; Oguntolu, F.A.; Ojo, M.M.; Olayinka Oyeniyi, A.; Jan, R.; Khan, I. Fractional order mathematical model of monkeypox transmission dynamics. Phys. Scr. 2022, 97, 084005. [Google Scholar] [CrossRef]
- Weinan, E.; Li, X.; Vanden-Eijnden, E. Some Recent Progress in Multiscale Modeling. In Proceedings of the Multiscale Modelling and Simulation, Lecture Notes in Computational Science and Engineering; Springer: Berlin/Heidelberg, Germany, 2004; Volume 39, pp. 3–21. [Google Scholar]
- Franzke, C.L.; O’Kane, T.J.; Berner, J.; Williams, P.D.; Lucarini, V. Stochastic climate theory and modeling. Wiley Interdiscip. Rev. Clim. Chang. 2015, 6, 63–78. [Google Scholar] [CrossRef]
- Jan, R.; Boulaaras, S. Analysis of fractional-order dynamics of dengue infection with non-linear incidence functions. Trans. Inst. Meas. Control 2022, 44, 2630–2641. [Google Scholar] [CrossRef]
- Boulaaras, S.; Jan, R.; Khan, A.; Ahsan, M. Dynamical analysis of the transmission of dengue fever via Caputo-Fabrizio fractional derivative. Chaos Solitons Fractals X 2022, 8, 100072. [Google Scholar] [CrossRef]
- Elbrolosy, M.; Alhamud, M.; Elmandouh, A. Analytical solutions to the fractional stochastic (3+1) equation of fluids with gas bubbles using an extended auxiliary function method. Alex. Eng. J. 2024, 92, 254–266. [Google Scholar] [CrossRef]
- Almutairi, B.; Al Nuwairan, M.; Aldhafeeri, A. Exploring the Exact Solution of the Space-Fractional Stochastic Regularized Long Wave Equation: A Bifurcation Approach. Fractal Fract. 2024, 8, 298. [Google Scholar] [CrossRef]
- Elbrolosy, M. Qualitative analysis and new exact solutions for the extended space-fractional stochastic (3+1)-dimensional Zakharov-Kuznetsov equation. Phys. Scr. 2024, 99, 075225. [Google Scholar] [CrossRef]
- Horsthemke, W.; Lefever, R. Noise-Induced Transitions in Physics, Chemistry, and Biology; Springer: Berlin/Heidelberg, Germany, 1984. [Google Scholar]
- Oksendal, B. Stochastic Differential Equations: An Introduction with Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Platen, E.; Bruti-Liberati, N. Numerical Solution of Stochastic Differential Equations with Jumps in Finance; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Mohammed, W.W.; Al-Askar, F.M.; El-Morshedy, M. Impacts of Brownian motion and fractional derivative on the solutions of the stochastic fractional Davey-Stewartson equations. Demonstr. Math. 2023, 56, 20220233. [Google Scholar] [CrossRef]
- Qi, J.; Cui, Q.; Bai, L.; Sun, Y. Investigating exact solutions, sensitivity, and chaotic behavior of multi-fractional order stochastic Davey–Sewartson equations for hydrodynamics research applications. Chaos, Solitons Fractals 2024, 180, 114491. [Google Scholar] [CrossRef]
- Tariq, M.M.; Riaz, M.B.; Kozubek, T.; Aziz-ur Rehman, M. Exploring chaos and stability: Dynamic insights into the stochastic Davey-Stewartson system through advanced sensitivity analysis. Model. Earth Syst. Environ. 2025, 11, 83. [Google Scholar] [CrossRef]
- Al-Askar, F.M.; Cesarano, C.; Mohammed, W.W. Multiplicative Brownian motion stabilizes the exact stochastic solutions of the Davey–Stewartson equations. Symmetry 2022, 14, 2176. [Google Scholar] [CrossRef]
- Iqbal, M.S.; Inc, M. Optical Soliton solutions for stochastic Davey–Stewartson equation under the effect of noise. Opt. Quantum Electron. 2024, 56, 1148. [Google Scholar] [CrossRef]
- Liu, C.; Li, Z. Multiplicative brownian motion stabilizes traveling wave solutions and dynamical behavior analysis of the stochastic Davey–Stewartson equations. Results Phys. 2023, 53, 106941. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Saha, A.; Banerjee, S. Dynamical Systems and Nonlinear Waves in Plasmas; CRC Press: Boca Raton, FL, USA, 2021; p. x+207. [Google Scholar]
- Goldstein, H. Classical Mechanics Addison-Wesley Series in Physics; Addison-Wesley: Reading, MA, USA, 1980. [Google Scholar]
- Nemytskii, V.V. Qualitative Theory of Differential Equations; Princeton University Press: Princeton, NJ, USA, 2015; Volume 2083. [Google Scholar]
- Elbrolosy, M.E.; Elmandouh, A.A. Construction of new traveling wave solutions for the (2+1) dimensional extended Kadomtsev-Petviashvili equation. J. Appl. Anal. Comput. 2022, 12, 533–550. [Google Scholar] [CrossRef] [PubMed]
- Elbrolosy, M.; Elmandouh, A. Dynamical behaviour of conformable time-fractional coupled Konno-Oono equation in magnetic field. Math. Probl. Eng. 2022, 2022, 3157217. [Google Scholar] [CrossRef]
- Yang, L.; Hou, X.; Zeng, Z. A complete discrimination system for polynomials. Sci. China Ser. E 1996, 39, 628–646. [Google Scholar]
- Elbrolosy, M.; Elmandouh, A. Bifurcation and new traveling wave solutions for (2+1)-dimensional nonlinear Nizhnik–Novikov–Veselov dynamical equation. Eur. Phys. J. Plus 2020, 135, 533. [Google Scholar] [CrossRef]
- Elbrolosy, M.; Elmandouh, A. Dynamical behaviour of nondissipative double dispersive microstrain wave in the microstructured solids. Eur. Phys. J. Plus 2021, 136, 955. [Google Scholar] [CrossRef]
- El-Dessoky, M.; Elmandouh, A. Qualitative analysis and wave propagation for Konopelchenko-Dubrovsky equation. Alex. Eng. J. 2023, 67, 525–535. [Google Scholar] [CrossRef]
- Byrd, P.F.; Friedman, M.D. Handbook of Elliptic Integrals for Engineers and Scientists, 2nd ed.; Die Grundlehren der mathematischen Wissenschaften, Band 67; Springer: New York, NY, USA; Berlin/Heidelberg, Germany, 1971; p. xvi+358. [Google Scholar]
- Jumarie, G. Modified Riemann-Liouville derivative and fractional Taylor series of nondifferentiable functions further results. Comput. Math. Appl. 2006, 51, 1367–1376. [Google Scholar] [CrossRef]
- Gantmacher, F. Lectures in Analytical Mechanics; Mir: Moscow, Russia, 1970. [Google Scholar]
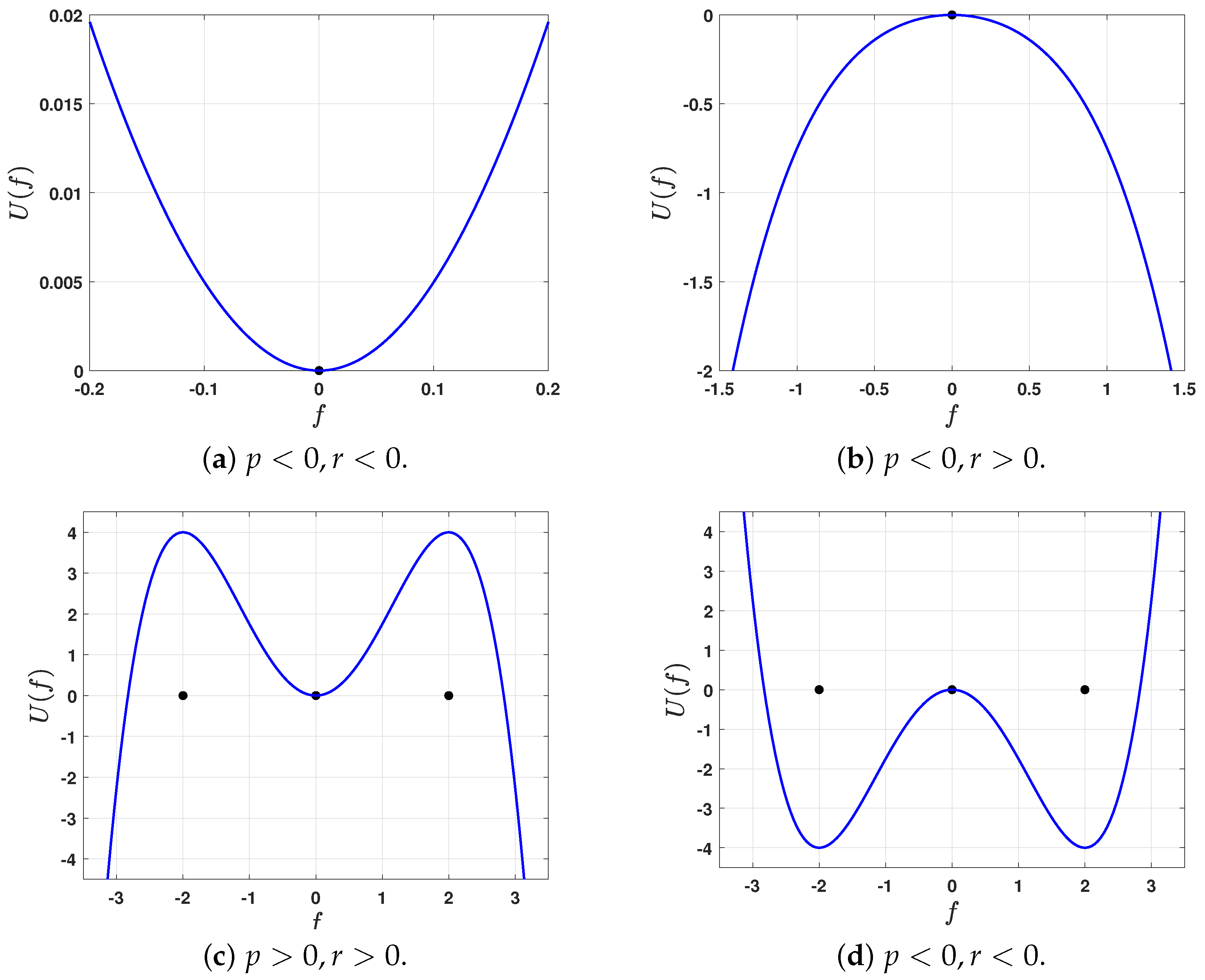



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elmandouh, A.; Al Nuwairan, M.; El-Dessoky, M.M. Nonlinear Dynamical Analysis and New Solutions of the Space-Fractional Stochastic Davey–Stewartson Equations for Nonlinear Water Waves. Mathematics 2025, 13, 692. https://doi.org/10.3390/math13050692
Elmandouh A, Al Nuwairan M, El-Dessoky MM. Nonlinear Dynamical Analysis and New Solutions of the Space-Fractional Stochastic Davey–Stewartson Equations for Nonlinear Water Waves. Mathematics. 2025; 13(5):692. https://doi.org/10.3390/math13050692
Chicago/Turabian StyleElmandouh, Adel, Muneerah Al Nuwairan, and M. M. El-Dessoky. 2025. "Nonlinear Dynamical Analysis and New Solutions of the Space-Fractional Stochastic Davey–Stewartson Equations for Nonlinear Water Waves" Mathematics 13, no. 5: 692. https://doi.org/10.3390/math13050692
APA StyleElmandouh, A., Al Nuwairan, M., & El-Dessoky, M. M. (2025). Nonlinear Dynamical Analysis and New Solutions of the Space-Fractional Stochastic Davey–Stewartson Equations for Nonlinear Water Waves. Mathematics, 13(5), 692. https://doi.org/10.3390/math13050692





