On the Martínez–Kaabar Fractal–Fractional Reduced Pukhov Differential Transformation and Its Applications

Abstract

This paper addresses the extension of the Martinez–Kaabar fractal–fractional calculus (simply expressed as MK calculus) to the context of reduced differential transformation, with applications to the solution of some partial differential equations. Since this differential transformation is derived from the Taylor series expansion of real-valued functions of several variables, it is necessary to develop this theory in the context of such functions. Firstly, classical elements of the analysis of functions of several real variables are introduced, such as the concept of partial derivative and Clairaut’s theorem, in terms of the MK partial $\alpha ,\gamma$-derivative. Next, we establish the fractal–fractional (FrFr) Taylor formula with Lagrange residue and discuss a sufficient condition for a function of class $C_{\alpha ,\gamma }^{\infty }$ on an open and bounded set $D\subset R^2$ to be expanded into a convergent infinite series, the so-called FrFr Taylor series. The theoretical study is completed by defining the FrFr reduced differential transformation and establishing its fundamental properties, which will allow the construction of the FrFr reduced Pukhov differential transformation method (FrFrRPDTM). Based on the previous results, this new technique is applied to solve interesting non-integer order linear and non-linear partial differential equations that incorporate the fractal effect. Finally, the results show that the FrFrRPDTM represents a simple instrument that provides a direct, efficient, and effective solution to problems involving this class of partial differential equations.

1. Introduction

In the late 1970s, the differential transformation method was first introduced by Pukhov [1] via Taylor transformations through various research works by Pukhov, which were written in the Russian language. To ensure accurate naming and to avoid confusion with other studies that have shown other authors who initially proposed this method other than G. E. Pukhov, this method is named as the Pukhov differential transformation method (PDTM) in this work. This is a semi-analytical technique to solve various types of differential equations. PDTM constitutes an iterative procedure to obtain analytical solutions of differential equations with the help of Taylor transformations to reduce the size of the computational work while providing an accurate solution in series form. Recently, PDTM has been recently employed in studying the dynamics of a non-Newtonian fluid model in squeezing flows [2]. PDTM has been applied to analytically investigate the Moore–Gibson–Thompson thermoelastic model [3], the SARS-CoV-2 model [4], and the buckling analysis of functionally graded thick sandwich beams with porous ceramic core [5].

In addition, the main advantage of this method is that it can be directly applied to nonlinear ordinary differential equations without requiring linearization, discretization, or perturbation. For all these reasons, the development and application of PDTM have focused on the interest of many researchers. Therefore, this technique has been successfully used to search for the approximate analytical solution of problems involving differential equations of the Lane-Emdem type [6]. This technique has also been extended to the context of partial differential equations. Thus, through the so-called two-dimensional Pukhov differential transformation method (TDPDTM), important partial differential equations such as the hyperbolic Telegraph equation or the Newell–Whitehead–Segel equation, among others, have been solved [7,8,9]. The main drawback of using TDPDTM to solve partial differential equations is the complexity of the calculations that its application entails in many cases. To overcome this drawback, the so-called reduced Pukhov differential transformation method (RPDTM), is introduced, which has as its main advantage the fact that its application to this type of equation provides an analytical approximation, in many cases an exact solution, in a rapidly convergent sequence with elegantly computed terms. Thus, using RPDTM, important partial differential equations such as the generalized KDV equation, the Klein–Gordon equation, the nonlinear reaction–diffusion–convection equation, the linear and nonlinear Goursat equations, the wave equation, the Burger’s equation, among other, are solved efficiently and with performance [10,11,12,13,14,15].

Fractional calculus is a generalized and extended branch of mathematical analysis that has been the subject of numerous research investigations in the last decade. Fractional calculus is classically based on a non-local conception of fractional derivatives, such as the well-known Riemann–Liouville (R-L) and Caputo (Cp) derivatives that have been widely applied to the resolution of various research problems. More information on these well-known derivatives and the formulation of the RPDTM in the context of fractional partial differential equations can be found in [16,17,18,19,20].

In the last decades, several notions of non-integer local derivatives based on incremental ratios have emerged, among which the conformal derivative introduced in [21] stands out. This formulation of derivative presents clear disadvantages in relation to the definition of Cp for certain functions, as discussed in [22]. Recently, to face this challenge, in [23], a new generalization of the non-integer local derivative was introduced, the so-called Abu–Shady–Kaabar derivative (ASKD), which provides analytical solutions for fractional differential equations in a simple way, which are compatible with those obtained by R-L and Cp.

In [24], a new notion of differentiation was proposed that combines the concept of fractional differentiation in the Cp sense and the concept of fractal differentiation proposed in [25,26]. This formulation of the fractal–fractional derivative (FrFrD) includes the properties of fractal geometry, heterogeneity, and memory effect in its system.

Recently, in [27], a new local generalization of the fractal–fractional derivative (FrFrD), called the Martinez-Kaabar (MK) fractal–fractional derivative, has been established. The fractal effects in MK derivative are introduced implicitly because the term $t^{2-\alpha -\gamma }$ in MK derivative represents a nonlinear scaling factor via $\alpha$ and $\gamma$, and hence, this will create a system that is similar to fractals in its behaviour that can exhibit the properties of self-similarity and scaling laws. In addition, the term $M\left(\alpha ,\gamma ,\lambda \right)$ in MK derivative involves Gamma functions, which are very common in the theory of fractional calculus and fractal geometry, which can help study the behaviours of the dynamics of complex systems.

It should be noted that this new formulation has the property that when applied to some elementary functions, results that are compatible with those obtained by the FrFrD in the Cp sense with the power law introduced in [21], are obtained. Furthermore, this novel theory of calculus, the so-called MK calculus theory, has been developed in several directions, such as the establishment of the fundamental elements of classical mathematical analysis, the field of integral transformations, and the solution of important integral equations in this context (see [28,29]). Complex fractal–fractional chaotic systems have been recently solved and analyzed via the sixth-order Adomian decomposition method [30].

Based on all these previous investigations, in this work, we set as our main objective the extension of the MK calculus theory to the RPDTM and its application to the solution of some linear and nonlinear partial differential equations. Thus, we formulate the fractal–fractional RPDTM (FrFrRPDTM), which, as in the classical case, its application to linear and nonlinear MK partial differential equations provides an analytical approximation, in many cases, an exact solution, in an efficient and effective way. In addition, for MK nonlinear partial differential equations, this new method presents the main advantage that it can be applied directly to these equations without requiring linearization, discretization, or perturbation. Next, we describe the structure of our study:

(i)

Since the concept of RPDTM is derived from the Taylor series expansion of real-valued functions of several real variables, we first state some important notions and results of MK calculus theory in the field of these functions. Thus, we define MK partial $\alpha ,\gamma$-derivative at a point and establish the notion of class $C_{\alpha ,\gamma }^p$ function on a certain open set $D\subset R^n$. In the case of real-valued functions of two real variables, we prove the classical Clairaut theorem in the sense of MK partial $\alpha ,\gamma$-derivative, which we extend to functions of class $C_{\alpha ,\gamma }^p$ on a given open set $D\subset R^2$. Next, we state FrFr Taylor’s formula in the sense of MK partial $\alpha ,\gamma$-derivative and the Lagrange form of the remainder. Finally, a sufficient condition for a class function $C_{\alpha ,\gamma }^{\infty }$ of a bounded open set $D\subset R^2$ to expand into a convergent infinite series, the so-called FrFr Taylor series, is discussed.

(ii)

Next, the notion of fractal–fractional RPDTM (FrFrRPDTM) is defined and its main properties are established. In this way, the foundations are laid for the construction of the so-called fractal–fractional PDTM (FrFrPDTM).

(iii)

Finally, the application of this new technique to find the approximate analytical solution of some interesting MK linear and non-linear partial differential equations is illustrated.

2. Preliminaries

In this section, we recall fundamental concepts and results of the MK calculus theory, which will be necessary for the developments that we will present throughout the following sections of this work. We begin with the definition of MK derivative of order $\alpha$ introduced in [27].

Definition 1. 

For function $f:\left[0,\infty \right.)\to R$, the MK derivative of order $0<\alpha \le 1$, of $f$ at $t>0$ is written as:

$${}^{MK}D^{\alpha ,\gamma }f\left(t\right)=\underset{\epsilon \to 0}{lim}{\displaystyle \frac{f\left(t+\epsilon M\left(\alpha ,\gamma ,\lambda \right)t^{2-\alpha -\gamma }\right)-f\left(t\right)}{\epsilon }},$$

(1)

where $M\left(\alpha ,\gamma ,\lambda \right)={\displaystyle \frac{\Gamma \left(\lambda -\gamma +1\right)}{\gamma \Gamma \left(\lambda -\alpha -\gamma +2\right)}}$ with $0<\gamma \le 1$ and $\lambda >-1.$

If $f$ is MK $\alpha ,\gamma$-differentiable in some $\left(0,a\right)$, $a>0$, and $\underset{t\to 0^{+}}{lim}{}^{MK}D^{\alpha ,\gamma }f\left(t\right)$ exists, then it is expressed as:

$${}^{MK}D^{\alpha ,\gamma }f\left(0\right)=\underset{t\to 0^{+}}{\lim }{}^{MK}D^{\alpha ,\gamma }f\left(t\right),$$

(2)

Remark 1. 

It is interesting to highlight some special cases of Definition 3:

(i) 

If $\alpha =\gamma =1$, then Equation (1) becomes the classical definition of a derivative.

(ii) 

If $\gamma =1$, then Equation (1) becomes the definition of the Abu–Shady–Kaabar fractional derivative of order $\alpha ,$ proposed in [23].

(iii) 

If $\gamma =1$, then Equation (1) can be written as:

$${}^{GEF}D^{\alpha ,\gamma }f\left(t\right)={M\left(\alpha ,1,\lambda \right)T}_{\alpha }f\left(t\right)$$

where $T_{\alpha }$ is the conformable derivative of order $\alpha ,$ introduced in [21].

(iv) 

If $\alpha =1$, then Equation (1) becomes the definition of the fractal derivative of order $\gamma ,$ introduced in [25,26].

Theorem 1. 

Let $0<\alpha ,\gamma \le 1,$ and let $f$ be MK $\alpha ,\gamma$-differentiable at a point $t>0$. If, additionally, $f$ is a differentiable function, then

$${}^{MK}D^{\alpha ,\gamma }f\left(t\right)={M\left(\alpha ,\gamma ,\lambda \right)t}^{2-\alpha -\gamma }{\displaystyle \frac{df(t)}{dt}},$$

(3)

where $M\left(\alpha ,\gamma ,\lambda \right)={\displaystyle \frac{\Gamma \left(\lambda -\gamma +1\right)}{\gamma \Gamma \left(\lambda -\alpha -\gamma +2\right)}}$ with $\lambda >-1$.

Remark 2. 

Consider a function $f\left(t\right)=t^{\lambda }$, $\lambda >-1$. Using Theorem 1, we get:

$${}^{MK}D^{\alpha ,\gamma }\left(t^{\lambda }\right)={M\left(\alpha ,\gamma ,\lambda \right)t}^{2-\alpha -\gamma }\lambda t^{\lambda -1}={\displaystyle \frac{\lambda \Gamma \left(\lambda -\gamma +1\right)}{\gamma \Gamma \left(\lambda -\alpha -\gamma +2\right)}}{t}^{\lambda -\alpha -\gamma +1},$$

(4)

Remark 3. 

From the above remark, in [27], it is proved that the MK derivative constitutes a local type of formulation of the FrFrD, which has the property that when applied to some elementary functions it provides results compatible with those obtained by the FrFrD in the Cp sense with the power law introduced in [24] .

Using Definition 1, we get [27]:

Theorem 2. 

Assume that $0<\alpha , \gamma \le 1,$ $\lambda >-1$ and let $f,g$ be MK $\alpha ,\gamma$-differentiable at a point $t>0$. Then, we have:

(i) 

${}^{MK}D^{\alpha ,\gamma }\left(af+bg\right)(t)=a {}^{MK}D^{\alpha ,\gamma }f(t)+b {}^{MK}D^{\alpha ,\gamma }g(t)$, $\forall a,b\in R$.

(ii) 

${}^{MK}D^{\alpha ,\gamma }\left(\mu \right)=0,$ $\forall$constant functions $f\left(t\right)=\mu$.

(iii) 

${}^{MK}D^{\alpha ,\gamma }\left(fg\right)(t)=f\left(t\right){}^{MK}D^{\alpha ,\gamma }g(t)+g\left(t\right){}^{MK}D^{\alpha ,\gamma }f(t)$.

(iv) 

${}^{MK}D^{\alpha ,\gamma }\left({\displaystyle \frac{f}{g}}\right)(t)={\displaystyle \frac{g\left(t\right){}^{MK}{D}^{\alpha ,\gamma }f(t)-f\left(t\right){}^{MK}{D}^{\alpha ,\gamma }g(t)}{{[g(t)]}^2}}$.

Now, we recall the extension of an essential result of classical mathematical analysis, the chain rule, to the context of MK calculus [27].

Theorem 3 (Chain Rule). 

Let $0<\alpha ,\gamma \le 1,$ $\lambda >-1$, $g$ MK $\alpha ,\gamma$-differentiable at $t>0$ and $f$ is differentiable at $g\left(t\right)$, then

$${}^{MK}D^{\alpha ,\gamma }\left(f ⃘g\right)\left(t\right)=f’\left(g\left(t\right)\right){}^{MK}D^{\alpha ,\gamma }g\left(t\right),$$

(5)

Remark 4. 

According to the above, the MK derivative of order $\alpha$ of the following elementary functions is obtained as:

(i) 

${}^{MK}D^{\alpha ,\gamma }\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}\right)=1,$

(ii) 

${}^{MK}D^{\alpha ,\gamma }\left(e^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}}\right)=e^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}},$

(iii) 

${}^{MK}D^{\alpha ,\gamma }\left(sin\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}\right)\right)=cos\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}\right),$

(iv) 

${}^{MK}D^{\alpha ,\gamma }\left(cos\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}\right)\right)=-sin\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}\right),$

In [27], the classical mean value theorems have also been formulated in the sense of the MK derivative.

Theorem 4 (Roll’s theorem for MK $\mathit{\alpha },\mathit{\gamma }$-differentiable functions). 

Assume that $a>0,$ $0<\alpha ,\gamma \le 1$, $\lambda >-1$ and $f:[a,b]\to R$ be a given function that satisfies:

(i) 

$f$ is continuous on $\left[a,b\right]$,

(ii) 

$f$ is MK $\alpha ,\gamma$ -differentiable on $\left(a,b\right)$,

(iii) 

$f\left(a\right)=f\left(b\right)$.

Then, there exists $c\in \left(a,b\right),$ such that ${}^{MK}D^{\alpha ,\gamma }f\left(c\right)=0.$

Theorem 5 (Mean value theorem for MK $\mathit{\alpha },\mathit{\gamma }$-differentiable functions). 

Suppose that $a>0,$ $0<\alpha ,\gamma \le 1$, and $f:[a,b]\to R$ is a given function that satisfies:

(i) 

$f$ is continuous on $\left[a,b\right]$,

(ii) 

$f$ is MK $\alpha ,\gamma$ -differentiable on $\left(a,b\right)$,

Then, there exists $c\in \left(a,b\right),$ such that ${}^{MK}D^{\alpha ,\gamma }f\left(c\right)={\displaystyle \frac{f\left(b\right)-f\left(a\right)}{\frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}\left(b^{\alpha +\gamma -1}-a^{\alpha +\gamma -1}\right)}}.$

Theorem 6 (Modified mean value theorem for MK $\mathit{\alpha },\mathit{\gamma }$-differentiable functions). 

Suppose that $a>0,$ $0<\alpha ,\gamma \le 1$, and $f:[a,b]\to R$ is a given function that satisfies:

(i) 

$f$ is continuous on $\left[a,b\right],$

(ii) 

$f$ is MK $\alpha ,\gamma$-differentiable on $\left(a,b\right),$

Then, there exists $c\in \left(a,b\right),$ such that ${\displaystyle \frac{{}^{MK}D^{\alpha ,\gamma }f\left(c\right)}{\frac{\gamma \Gamma \left(2-\alpha \right)}{\gamma \Gamma \left(3-\alpha -\gamma \right)}{c}^{2-\alpha -\gamma }}}={\displaystyle \frac{f\left(b\right)-f\left(a\right)}{b-a}}.$

In the end, the MK $\alpha ,\gamma$-integral of a function $f$ starting at $a\ge 0$ is recalled as [27]:

Definition 2. 

${}^{MK}I_{\alpha ,\gamma }^a\left(f\right)(t)={\displaystyle \frac{1}{M\left(\alpha ,\gamma ,\lambda \right)}}{\int }_a^t{\displaystyle \frac{f(x)}{x^{2-\alpha -\gamma }}}·dx$, where this integral is basically the usual Riemann improper integral,  $M\left(\alpha ,\gamma ,\lambda \right)={\displaystyle \frac{\Gamma \left(\lambda -\gamma +1\right)}{\gamma \Gamma \left(\lambda -\alpha -\gamma +2\right)}}, 0<\alpha ,\gamma \le 1$, and  $\lambda >-1.$

From Definition 2, we get the following results:

Theorem 7. 

${}^{MK}D^{\alpha ,\gamma }{}^{MK}I_{\alpha ,\gamma }^a\left(f\right)\left(t\right)=f(t)$, for $t\ge a$, where $f$ is any continuous function in the domain of ${}^{MK}I_{\alpha ,\gamma }^a$.

Theorem 8. 

Let $a>0$, $0<\alpha ,\gamma \le 1$, $\lambda >-1$, and $f$ be a continuous real-valued function on the interval $\left[a,b\right]$. Let $G$ be any real-valued function with the property ${}^{MK}D^{\alpha ,\gamma }G\left(t\right)=f\left(t\right)$ for all $t\in \left[a,b\right]$. Then

$${}^{MK}I_{\alpha ,\gamma }^a\left(f\right)\left(b\right)=G\left(b\right)-G\left(a\right),$$

(6)

3. Some Elements of MK Calculus of Real-Valued Functions of Several Real Variables

In this section, we introduce some important notions and results from the theory of MK calculus in the field of real-valued functions of several real variables. Thus, we define the concept of MK partial $\alpha ,\gamma$-derivative at a point and establish the notion of class $C_{\alpha ,\gamma }^p$ function on a certain open set $D\subset R^n$. In the context of real-valued functions of two real variables, we formulate the classical Clairaut theorem in the sense of MK partial $\alpha ,\gamma$-derivative, which we extend to class $C_{\alpha ,\gamma }^p$ functions on a certain open set $D\subset R^2$. Next, we establish the FrFr Taylor formula in the sense of the MK partial $\alpha ,\gamma$-derivative and propose the Lagrange form of the remainder. Finally, we establish sufficient conditions that a class $C_{\alpha ,\gamma }^{\infty }$ function on an open bounded set $D\subset R^2$ can be expanded into a convergent infinite series, known as the FrFr Taylor series.

Definition 3. 

Let $f$ be a real-valued function with $n$ variables $x_1,\dots ,x_n$ and the MK partial derivative of order $0<\alpha \le 1$ in $x_i$ at a point $a=\left(a_1,\dots ,a_n\right)$ where $a_i>0$, is defined as follows

$${}^{MK}D_{x_i}^{\alpha ,\gamma }f\left(a_1,\dots ,a_n\right)=\underset{\epsilon \to 0}{lim}{\displaystyle \frac{f\left(a_1,\dots , a_i+\epsilon M\left(\alpha ,\gamma ,\lambda \right)a_i^{2-\alpha -\gamma },{\dots ,a}_n\right)-f\left(a_1,\dots ,a_n\right)}{\epsilon }}, i=\mathrm{1,2},..,n,$$

(7)

where $M\left(\alpha ,\gamma ,\lambda \right)={\displaystyle \frac{\Gamma \left(\lambda -\gamma +1\right)}{\gamma \Gamma \left(\lambda -\alpha -\gamma +2\right)}}$ with $0<\gamma \le 1$ and $\lambda >-1.$

Remark 5. 

Let $0<\alpha ,\gamma \le 1$, and $f$ be a real-valued function with $n$ variables defined on an open set $D\subset R^n$, such that for all $\left(x_1,\dots ,x_n\right)\in D$, each $x_i>0$. The function $f$ is said to be $C_{\alpha ,\gamma }^p(D,R)$ if all its MK partial derivatives of order $\le p$ exist and are continuous on $D$.

Remark 6. 

In our study, we are going to consider real-valued functions of two real variables defined on an open set $D\subset R^2$, such that for all $\left(x,y\right)\in D$, each $x,y>0$. In this case, Definition 4 at a point $\left(a,b\right)$ can be written as:

$${}^{MK}D_x^{\alpha ,\gamma }f\left(a,b\right)=\underset{h\to 0}{lim}{\displaystyle \frac{f\left(a+\epsilon M\left(\alpha ,\gamma ,\lambda \right)a^{2-\alpha -\gamma },b\right)-f\left(a,b\right)}{h}},$$

(8)

and

$${}^{MK}D_y^{\alpha ,\gamma }f\left(a,b\right)=\underset{k\to 0}{lim}{\displaystyle \frac{f\left(a,b+\epsilon M\left(\alpha ,\gamma ,\lambda \right)b^{2-\alpha -\gamma }\right)-f\left(a,b\right)}{k}},$$

(9)

where $M\left(\alpha ,\gamma ,\lambda \right)={\displaystyle \frac{\Gamma \left(\lambda -\gamma +1\right)}{\gamma \Gamma \left(\lambda -\alpha -\gamma +2\right)}}$ with $0<\gamma \le 1$ and $\lambda >-1.$

Next, we formulate the classical Clairaut theorem in terms of the MK partial derivatives of real-valued functions of two real variables.

Theorem 9. 

Let $0<\alpha ,\gamma \le 1.$ Assume that $f(x,y)$ is the function for which ${}^{MK}D_x^{\alpha ,\gamma }\left({}^{MK}D_y^{\alpha ,\gamma }f\left(x,y\right)\right)$ and ${}^{MK}D_y^{\alpha ,\gamma }\left({}^{MK}D_x^{\alpha ,\gamma }f\left(x,y\right)\right)$ exist and are continuous on an open set $D\subset R^2$, such that for all $\left(x,y\right)\in D$, each $x,y>0$. Then

$${}^{MK}D_y^{\alpha ,\gamma }\left({}^{MK}D_x^{\alpha ,\gamma }f\left(x,y\right)\right)={}^{MK}D_x^{\alpha ,\gamma }\left({}^{MK}D_y^{\alpha ,\gamma }f\left(x,y\right)\right), \forall \left(x,y\right)\in D,$$

(10)

Proof. 

Let us consider the following expression:

$$\begin{array}{ll}S\left(h,k\right)& =f\left(a+h{\displaystyle \frac{\Gamma \left(2-\alpha \right)}{\gamma \Gamma \left(3-\alpha -\gamma \right)}}{a}^{2-\alpha -\gamma },b+k{\displaystyle \frac{\Gamma \left(2-\alpha \right)}{\gamma \Gamma \left(3-\alpha -\gamma \right)}}{b}^{2-\alpha -\gamma }\right)\\ & -f\left(a+h{\displaystyle \frac{\Gamma \left(2-\alpha \right)}{\gamma \Gamma \left(3-\alpha -\gamma \right)}}{a}^{2-\alpha -\gamma },b\right)\\ & -f\left(a,b+k{\displaystyle \frac{\Gamma \left(2-\alpha \right)}{\gamma \Gamma \left(3-\alpha -\gamma \right)}}{b}^{2-\alpha -\gamma }\right)+f\left(a,b\right),\end{array}$$

where $\left(a,b\right)\in D$.

By fixing $a$ and $k$ and defining

$$g\left(x\right)=f\left(x,b+k{\displaystyle \frac{\Gamma \left(2-\alpha \right)}{\gamma \Gamma \left(3-\alpha -\gamma \right)}}{b}^{2-\alpha -\gamma }\right)-f\left(x,b\right),$$

In this case, we write:

$$S\left(h,k\right)=g\left(a+h{\displaystyle \frac{\Gamma \left(2-\alpha \right)}{\gamma \Gamma \left(3-\alpha -\gamma \right)}}{a}^{2-\alpha -\gamma }\right)-g\left(a\right),$$

Then, by Theorem 6,

$$S\left(h,k\right)=h{\left({\displaystyle \frac{a}{c}}\right)}^{2-\alpha -\gamma }\left[{}^{MK}D_x^{\alpha ,\gamma }f\left(c,b+k{\displaystyle \frac{\Gamma \left(2-\alpha \right)}{\gamma \Gamma \left(3-\alpha -\gamma \right)}}{b}^{2-\alpha -\gamma }\right)-{}^{MK}D_x^{\alpha ,\gamma }f\left(c,b\right)\right],$$

for some point $c$ between $a$ and $a+h{\displaystyle \frac{\Gamma \left(2-\alpha \right)}{\gamma \Gamma \left(3-\alpha -\gamma \right)}}{a}^{2-\alpha -\gamma }$.

By applying Theorem 6 again, there exists a point $c’$ between $b$ and $b+k{\displaystyle \frac{\Gamma \left(2-\alpha \right)}{\gamma \Gamma \left(3-\alpha -\gamma \right)}}{b}^{2-\alpha -\gamma }$ such that:

$$S\left(h,k\right)=hk{\left({\displaystyle \frac{ab}{cc’}}\right)}^{2-\alpha -\gamma }{}^{MK}D_y^{\alpha ,\gamma }\left({}^{MK}D_x^{\alpha ,\gamma }f\left(c,c’\right)\right),$$

Since ${}^{MK}D_y^{\alpha ,\gamma }\left({}^{MK}D_x^{\alpha ,\gamma }f\left(x,y\right)\right)$ is continuous, it follows that:

$${}^{MK}D_y^{\alpha ,\gamma }\left({}^{MK}D_x^{\alpha ,\gamma }f\left(a,b\right)\right)=\underset{\left(h,k\right)\to \left(\mathrm{0,0}\right)}{\lim }{\displaystyle \frac{S\left(h,k\right)}{hk}},$$

Since $S\left(h,k\right)$ is symmetric in $h$ and $k$, it can be shown analogously that ${}^{MK}D_x^{\alpha ,\gamma }\left({}^{MK}D_y^{\alpha ,\gamma }f\left(a,b\right)\right)$ is given by the limit formula above, which proves the result. □

Remark 7. 

As in classical analysis, if $f$ is a real function of two real variables of class $C_{\alpha ,\gamma }^p$ on an open set $D\subset R^2$, such that for all $\left(x,y\right)\in D$, each $x,y>0$, then the value of any of the $p^{th}$ MK mixed partial $\alpha ,\gamma$-derivatives of $f$ at each point $\left(x,y\right)\in D$, does not depend on the order in which the $p$ variables are involved in the derivation.

In all the concepts, results, and developments that follow, we assume the following condition for $\alpha$ and $\gamma$ (parameters): $0<\alpha ,\gamma \le 1$, with $\alpha +\gamma -1>0$.

Definition 4. 

Let $0<\alpha ,\gamma \le 1, with \alpha +\gamma -1>0. Let f:D\to R$ be real-valued function of two real variables defined on an open set $D\subset R^2$, such that for all $\left(x,y\right)\in D$, each $x,y>0$. Let $\left(x_0,y_0\right)$ and $\left(x,y\right)$ be two points in $D$ such that the line segment $\left[\left(x_0,y_0\right),\left(x,y\right)\right]\subset D$. If $f$ is of class $C_{\alpha ,\gamma }^{n+1}$ on $\left[\left(x_0,y_0\right),\left(x,y\right)\right]$, the FrFr Taylor’s formula of order $n$ is given by:

$$\begin{array}{ll}f\left(x,y\right)=f\left(x_0,y_0\right)& +{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{\displaystyle \frac{1}{1!}}\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)f\left(x_0,y_0\right)\\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }\right)}^{\left(2\right)}f\left(x_0,y_0\right)\\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^3{\displaystyle \frac{1}{3!}}{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(3\right)}f\left(x_0,y_0\right)+\dots \\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^n{\displaystyle \frac{1}{n!}}{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(n\right)}f\left(x_0,y_0\right)+R_n^{\alpha ,\gamma }\left(x,y\right),\end{array}$$

(11)

where

$$\begin{array}{ll}{P}_n^{\alpha ,\gamma }\left(x,y\right)=f\left(x_0,y_0\right)& +{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{\displaystyle \frac{1}{1!}}\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)f\left(x_0,y_0\right)\\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(2\right)}f\left(x_0,y_0\right)\\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^3{\displaystyle \frac{1}{3!}}{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(3\right)}f\left(x_0,y_0\right)+\dots \\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^n{\displaystyle \frac{1}{n!}},\end{array}$$

(12)

is called FrFr Taylor’s polynomial of order $n$ for the function $f$ at the point $\left(x_0,y_0\right)$, and $R_n^{\alpha ,\gamma }\left(x,y\right)$ is the $n^{th}$ remainder term in FrFr Taylor’s formula.

Remark 8. 

In the above equations, the power $p^{th}$ of the operator $\left(x-x_0\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y-y_0\right){}^{MK}D_y^{\alpha ,\gamma }$ is given by:

$$\begin{gathered} {\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(p\right)}f \\ =\sum _{i=0}^p\left(\begin{array}{c}p\\ i\end{array}\right){\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right)}^{p-i}{\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right)}^i{{}_{\left(p\right)}{}^{MK}D}_{x^{p-i}{y}^i}^{\alpha ,\gamma }f\left(x_0,y_0\right), \end{gathered}$$

Here, ${{}_{\left(p\right)}{}^{MK}D}_{x^{p-i}{y}^i}^{\alpha ,\gamma }f\left(x,y\right)$ means the application of the MK partial $\alpha ,\gamma$-derivative  $p$ times ($p-i$ times with respect to variable $x$ and $i$ times with respect to variable $y$).

Remark 9. 

Under the same conditions of Definition 4, the FrFr form of the Lagrange remainder can be written as:

$$R_n^{\alpha ,\gamma }\left(x,y\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^{n+1}{\displaystyle \frac{1}{\left(n+1\right)!}}{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(n+1\right)}f\left(c,c’\right),$$

(13)

for some point $\left(c,c’\right)$  interior to the line segment  $\left[\left(x_0,y_0\right),\left(x,y\right)\right]$.

Remark 10. 

Also, under the same conditions as in Definition 4, if $f$ is of class $C_{\alpha ,\gamma }^{\infty }$ on an open set $D\subset R^2$, such that for all $\left(x,y\right)\in D$, each $x,y>0$, then for any point $\left(x,y\right)\in D$, the FrFr Taylor formula (11) can be applied to the function $f$ on the line segment $\left[\left(x_0,y_0\right),\left(x,y\right)\right]$ for all $n\in N$, such that there exists $\left(c_{n+1},c_{n+1}^{,}\right)$ interior to the line segment $\left[\left(x_0,y_0\right),\left(x,y\right)\right]$ (which depends on $n$) such that:

$$\begin{array}{ll}f\left(x,y\right)=f\left(x_0,y_0\right)& +{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{\displaystyle \frac{1}{1!}}\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)f\left(x_0,y_0\right)\\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(2\right)}f\left(x_0,y_0\right)\\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^3{\displaystyle \frac{1}{3!}}{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(3\right)}f\left(x_0,y_0\right)+\dots \\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^n{\displaystyle \frac{1}{n!}}{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(n\right)}f\left(x_0,y_0\right)+R_n^{\alpha ,\gamma }\left(x,y\right),\end{array}$$

(14)

where $R_n^{\alpha ,\gamma }\left(x,y\right)$ is given by Equation (13), valid for all $n\in N$.

If, in addition, it is verified that $\underset{n\to \infty }{\mathit{lim}}{R}_n^{\alpha ,\gamma }\left(x,y\right)=0, \forall \left(x,y\right)\in D$, then f is said to be $\alpha ,\gamma$ -analytic at the point $\left(x_0,y_0\right)$. In this assumption, taking the limit as $n\to \infty$ in both members of Equation (14), the FrFr Taylor series of f around the point $\left(x_0,y_0\right)$ is obtained, which is given by:

$$\begin{gathered} f\left(x,y\right)=f\left(x,y\right)+\sum _{n=1}^{\infty }{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^n{\displaystyle \frac{1}{n!}}{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma } \\ +\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(n\right)}f\left(x_0,y_0\right), \end{gathered}$$

(15)

Next, we establish sufficient conditions for a function $f$ to be $\alpha ,\gamma$-analytic at a point $\left(x_0,y_0\right)$.

Theorem 9. 

$Let f:D\to R$ be real-valued function of two real variables defined on an open bounded set $D\subset R^2$, such that for all $\left(x,y\right)\in D$, each $x,y>0$. Let $\left(x_0,y_0\right)\in D$. If $f$ is of class $C_{\alpha ,\gamma }^{\infty }$ on $D$ and if there exists a constant $K>0$ such that:

$$\left|{{}_{\left(n\right)}{}^{MK}D}_{x^{n-i}{y}^i}^{\alpha ,\gamma }f\left(x,y\right)\right|\le K^n,\forall n\in N,\forall \left(x,y\right)\in D,$$

then f is $\alpha ,\gamma$-analytic at point $\left(x_0,y_0\right)$.

Proof. 

Let $\rho =diamD=sup\left\{d_2\left(x,y\right):x,y\in D\right\}$, where $d_2$ is the Euclidean metric on $R^2$. In that case, for all $\left(x,y\right)\in D$ we have that $\left|x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right|\le \rho$, $\left|y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right|\le \rho$. Note also that the intermediate point $\left(c_{n+1},c_{n+1}^{,}\right)$ of the $n^{th}$ remainder of FrFr Taylor’s formula at point $\left(x_0,y_0\right)$ belongs to the bounded open set $D$. Therefore, if $\left(x,y\right)\in D$ and $n\in N$, for that $n^{th}$ remainder, we have:

$$\begin{gathered} \left|R_n^{\alpha ,\gamma }\left(x,y\right)\right|={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^{n+1}{\displaystyle \frac{1}{\left(n+1\right)!}}\left|{\left(\left(x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right){}^{MK}D_x^{\alpha ,\gamma }+\left(y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right){}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(n+1\right)}f\left(c_{n+1},c_{n+1}^{,}\right)\right|\le \\ {\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^{n+1}{\displaystyle \frac{1}{\left(n+1\right)!}}{\sum }_{i=0}^{n+1}\left(\begin{array}{c}n+1\\ i\end{array}\right){\left|x^{\alpha +\gamma -1}-x_0^{\alpha +\gamma -1}\right|}^{n+1-i}{\left|y^{\alpha +\gamma -1}-y_0^{\alpha +\gamma -1}\right|}^i\left|{{}_{\left(n+1\right)}{}^{MK}D}_{x^{n+1-i}{y}^i}^{\alpha ,\gamma }f\left(c_{n+1},c_{n+1}^{,}\right)\right|<{\displaystyle \frac{H^{n+1}}{\left(n+1\right)!}}, \end{gathered}$$

where $H=2\rho \left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)K$.

Finally, taking limits as $n\to \infty$, it follows that $\underset{n\to \infty }{\lim }{R}_n^{\alpha ,\gamma }\left(x,y\right)=0, \forall \left(x,y\right)\in D$, as we wished to prove. □

Remark 11. 

In the special case where $\left(x_0,y_0\right)=\left(\mathrm{0,0}\right)$, FrFr Taylor’s formula given by Equation (14) takes the form:

$$\begin{array}{ll}f\left(x,y\right)& =f\left(\mathrm{0,0}\right)+{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{\displaystyle \frac{1}{1!}}\left(x^{\alpha +\gamma -1}{}^{MK}D_x^{\alpha ,\gamma }+y^{\alpha +\gamma -1}{}^{MK}D_y^{\alpha ,\gamma }\right)f\left(\mathrm{0,0}\right)\\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}{\left(x^{\alpha +\gamma -1}{}^{MK}D_x^{\alpha ,\gamma }+y^{\alpha +\gamma -1}{}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(2\right)}f\left(\mathrm{0,0}\right)\\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^3{\displaystyle \frac{1}{3!}}{\left(x^{\alpha +\gamma -1}{}^{MK}D_x^{\alpha ,\gamma }+y^{\alpha +\gamma -1}{}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(3\right)}f\left(\mathrm{0,0}\right)+\dots \\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^n{\displaystyle \frac{1}{n!}}{\left(x^{\alpha +\gamma -1}{}^{MK}D_x^{\alpha ,\gamma }+y^{\alpha +\gamma -1}{}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(n\right)}f\left(\mathrm{0,0}\right)\\ & +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^{n+1}{\displaystyle \frac{1}{\left(n+1\right)!}}{\left(x^{\alpha +\gamma -1}{}^{MK}D_x^{\alpha ,\gamma }+y^{\alpha +\gamma -1}{}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(n+1\right)}f\left(c,c’\right),\end{array}$$

(16)

where $\left(c,c’\right)$ is between $\left(0, 0\right)$ and $\left(x,y\right)$. Equation (16) is called FrFr Maclaurin’s formula. Furthermore, the FrFr Maclaurin series of $f$ in powers of $x^{\alpha +\gamma -1}{y}^{\alpha +\gamma -1}$ is:

$$f\left(x,y\right)=f\left(\mathrm{0,0}\right)+\sum _{n=1}^{\infty }{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^n{\displaystyle \frac{1}{n!}}{\left(x^{\alpha +\gamma -1}{}^{MK}D_x^{\alpha ,\gamma }+y^{\alpha +\gamma -1}{}^{MK}D_y^{\alpha ,\gamma }\right)}^{\left(n\right)}f\left(\mathrm{0,0}\right),$$

(17)

4. Fractal–Fractional Reduced Pukhov Differential Transformation (FrFrRPDT)

In this section, the concept of FrFrRPDT is introduced. From this definition, its main properties are established and proven.

Definition 5. 

Assume $f\left(x,t\right)$ is a class $C_{\alpha ,\gamma }^{\infty }$ function with respect to time $t$ and space $x$ for some $0<\alpha ,\gamma \le 1$, with $\alpha +\gamma -1>0$, on a certain domain of $R^2$, the FrFrRPDT of $f\left(x,t\right)$ is defined as:

$$F_k^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{}_{\left(k\right)}{}^{MK}D_t^{\alpha ,\gamma }f\left(x,t\right)\right]}_{t=0},$$

(18)

where ${{}_{\left(k\right)}{}^{MK}D}_t^{\alpha ,\gamma }f\left(x,t\right)$ means the application of the MK partial $\alpha ,\gamma$-derivative  $k$ times with respect to variable $t$.

Remark 12. 

The inverse FrFrRPDT of $F_k^{\alpha ,\gamma }\left(x\right)$ is defined as:

$$f\left(x,t\right)=\sum _{k=0}^{\infty }{F}_k^{\alpha ,\gamma }\left(x\right) t^{k\left(\alpha +\gamma -1\right)},$$

(19)

By substituting Equation (18) in Equation (19), we get:

$$f\left(x,t\right)=\sum _{k=0}^{\infty }{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{t^{k\left(\alpha +\gamma -1\right)}}{k!}}{\left[{{}_{\left(k\right)}{}^{MK}D}_t^{\alpha ,\gamma }f\left(x,t\right)\right]}_{t=0},$$

The above equation shows that the concept of FrFrRPDT originated from the power series expansion.

Next, the main properties of the FrFrRPDT are established using Equations (18) and (19).

Theorem 10. 

If $f\left(x,t\right)=\lambda g\left(x,t\right)+\mu h\left(x,t\right)$, then $F_k^{\alpha ,\gamma }\left(x\right)=\lambda G_k^{\alpha ,\gamma }\left(x\right)+\mu H_k^{\alpha ,\gamma }\left(x\right)$, where $\lambda$ and $\mu$ are real constants.

Proof. 

Using Definition 5, our result follows directly. □

To establish the following result, we need to introduce the classical Leibnitz rule for the $n^{th}$ derivative of a product of two functions, in the context of MK calculus.

Lemma 1. 

If the functions  $f$ and $g$ have MK $\alpha ,\gamma$-derivatives up to order $n$ inclusive in an open set $X\subset R$, then the function $fg$ has the $n^{th}$ MK $\alpha ,\gamma$-derivative, which is given by:

$${}^{MK}D_{\left(n\right)}^{\alpha ,\gamma }\left(fg\right)\left(t\right)=\sum _{l=0}^n\left(\begin{array}{c}n\\ l\end{array}\right){}^{MK}D_{\left(l\right)}^{\alpha ,\gamma }f\left(t\right){}^{MK}D_{\left(n-l\right)}^{\alpha ,\gamma }g\left(t\right),$$

Proof. 

Proceeding inductively the result follows easily. □

Theorem 11. 

If $f\left(x,t\right)=g\left(x,t\right)h\left(x,t\right)$, then $F_k^{\alpha ,\gamma }\left(x\right)={\sum }_{l=0}^k{F}_l^{\alpha ,\gamma }\left(x\right)H_{k-l}^{\alpha ,\gamma }\left(x\right)$.

Proof. 

From Lemma 1, the FrFrRPDT of $f\left(x,t\right)$ is given by:

$$\begin{array}{c}{F}_k^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{}_{\left(k\right)}{}^{MK}D_t^{\alpha ,\gamma }f\left(x,t\right)\right]}_{t=0}\\ ={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{\displaystyle \sum _{l=0}^k}\left(\begin{array}{c}k\\ l\end{array}\right){{}_{\left(l\right)}{}^{MK}D}_t^{\alpha ,\gamma }g\left(x,t\right){{}_{\left(k-l\right)}{}^{MK}D}_t^{\alpha ,\gamma }h\left(x,t\right)\right]}_{t=0}\\ ={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{\displaystyle \sum _{l=0}^k}{\displaystyle \frac{k!}{l!\left(k-l\right)!}}{{}_{\left(l\right)}{}^{MK}D}_t^{\alpha ,\gamma }g\left(x,t\right){{}_{\left(k-l\right)}{}^{MK}D}_t^{\alpha ,\gamma }h\left(x,t\right)\right]}_{t=0}\\ ={\displaystyle \sum _{l=0}^K}{G}_l^{\alpha ,\gamma }\left(x\right)H_{k-l}^{\alpha ,\gamma }\left(x\right),\end{array}$$

□

Theorem 12. 

If $f\left(x,t\right)=x^{m\left(\alpha +\gamma -1\right)}{t}^{n\left(\alpha +\gamma -1\right)}$, with $m,n\in N$, then

$$F_k^{\alpha ,\gamma }\left(x\right)=x^{m\left(\alpha +\gamma -1\right)}\delta \left(k-n\right)=\left\{\begin{array}{cc}1& k=n\\ 0& k\ne n\end{array}\right.,$$

Proof. 

Let $f\left(x,t\right)$ be the original function, then the FrFrRPDT of $f\left(x,t\right)$ is given by:

$$\begin{array}{l}{F}_k^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{}_{\left(k\right)}{}^{MK}D_t^{\alpha ,\gamma }f\left(x,t\right)\right]}_{t=0}\\ ={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{}_{\left(k\right)}{}^{MK}D_t^{\alpha ,\gamma }\left(x^{m\left(\alpha +\gamma -1\right)}{t}^{n\left(\alpha +\gamma -1\right)}\right)\right]}_{t=0}\\ =x^{m\left(\alpha +\gamma -1\right)}\delta \left(k-n\right),\end{array}$$

□

Theorem 13. 

If $f\left(x,t\right)=x^{m\left(\alpha +\gamma -1\right)}{t}^{n\left(\alpha +\gamma -1\right)}g\left(x,t\right)$, with $m,n\in N$, then

$$F_k^{\alpha ,\gamma }\left(x\right)=x^{m\left(\alpha +\gamma -1\right)}{G}_{k-n}^{\alpha ,\gamma }\left(x\right),$$

Proof. 

Let $f\left(x,t\right)$ be the original function, then the FrFrRPDT of $f\left(x,t\right)$ is given by:

$$\begin{array}{l}{F}_k^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{}_{\left(k\right)}{}^{MK}D_t^{\alpha ,\gamma }f\left(x,t\right)\right]}_{t=0}\\ ={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{}_{\left(k\right)}{}^{MK}D_t^{\alpha ,\gamma }\left(x^{m\left(\alpha +\gamma -1\right)}{t}^{n\left(\alpha +\gamma -1\right)}g\left(x,t\right)\right)\right]}_{t=0}\\ =x^{m\left(\alpha +\gamma -1\right)}{\displaystyle \sum _{l=0}^k}\delta \left(l-n\right)G_{k-l}^{\alpha ,\gamma }\left(x\right)=x^{m\left(\alpha +\gamma -1\right)}{G}_{k-n}^{\alpha ,\gamma }\left(x\right),\end{array}$$

□

Theorem 14. 

If $f\left(x,t\right)={{}_{\left(r\right)}{}^{MK}D}_t^{\alpha ,\gamma }g\left(x,t\right)$, then

$$F_k^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}{\gamma }}\right)}^r\left(k+1\right)\left(k+2\right)\dots \left(k+r\right)G_{k+r}^{\alpha ,\gamma }\left(x\right),$$

Proof. 

Assume that $f\left(x,t\right)$ is the original function, then the FrFr Pukhov differential transformation (FrFrPDT) of $f\left(x,t\right)$ is given by:

$$\begin{array}{c}{F}_k^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{}_{\left(k\right)}{}^{MK}D_t^{\alpha ,\gamma }f\left(x,t\right)\right]}_{t=0}\\ ={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{}_{\left(k\right)}{}^{MK}D_t^{\alpha ,\gamma }\left({{}_{\left(r\right)}{}^{MK}D}_t^{\alpha ,\gamma }g\left(x,t\right)\right)\right]}_{t=0}\\ ={\left({\displaystyle \frac{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}{\gamma }}\right)}^r{\displaystyle \frac{\left(k+r\right)!}{k!}}{\left[{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^{k+r}{\displaystyle \frac{1}{\left(k+r\right)!}} {}_{\left(k+n\right)}{}^{MK}D_t^{\alpha ,\gamma }g\left(x,t\right)\right]}_{t=0}\\ ={\left({\displaystyle \frac{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}{\gamma }}\right)}^r\left(k+1\right)\left(k+2\right)\dots \left(k+r\right)G_{k+r}^{\alpha ,\gamma }\left(x\right),\end{array}$$

□

Theorem 15. 

If $f\left(x,t\right)={{}_{\left(1\right)}{}^{MK}D}_x^{\alpha ,\gamma }g\left(x,t\right)$, then

$$F_k^{\alpha ,\gamma }\left(x\right)={{}_{\left(1\right)}{}^{MK}D}_x^{\alpha ,\gamma }\left(G_k^{\alpha ,\gamma }\left(x\right)\right),$$

Proof. 

Let $f\left(x,t\right)$ be the original function, then the FrFrPDT of $f\left(x,t\right)$ is given by:

$$\begin{array}{l}{F}_k^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{}_{\left(k\right)}{}^{MK}D_t^{\alpha ,\gamma }f\left(x,t\right)\right]}_{t=0}\\ ={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{\left[{}_{\left(k\right)}{}^{MK}D_t^{\alpha ,\gamma }\left({{}_{\left(1\right)}{}^{MK}D}_x^{\alpha ,\gamma }g\left(x,t\right)\right)\right]}_{t=0}\\ ={{}_{\left(1\right)}{}^{MK}D}_x^{\alpha ,\gamma }{G}_k^{\alpha ,\gamma }\left(x\right),\end{array}$$

□

5. Numerical Applications

In the previous section, we developed the theory of FrFrRPDT, which is the basis of the technique that we are going to apply to find approximate analytical solutions of some interesting MK partial differential equations. It is well known that partial differential equations play an important role in the modelling of many problems in natural sciences and engineering and that is why we apply FrFrRPDTM to some examples of initial value problems involving MK linear and nonlinear partial differential equations. Among the examples that we show, it is worth highlighting the problem associated with the Klein–Gordon equation formulated in this context, which from the classical point of view plays a very important role in mathematical physics in fields such as plasma physics, fluid dynamics, and chemical kinetics [11]. In addition, we also include another interesting example concerning the Goursat partial differential equation that arises in linear and nonlinear partial differential equations with mixed derivatives in the study of wave phenomena [13].

Example 1. 

Consider the initial value problem involving MK nonlinear partial differential equation in $\alpha ,\gamma$ as follows:

$${}_{\left(1\right)}{}^{MK}D_t^{\alpha ,\gamma }y\left(x,t\right)-y\left(x,t\right){}_{\left(2\right)}{}^{MK}D_t^{\alpha ,\gamma }y\left(x,t\right)-{\left({}_{\left(1\right)}{}^{MK}D_x^{\alpha ,\gamma }y\left(x,t\right)\right)}^2-y\left(x,t\right)=0,$$

(20)

with the initial condition:

$$y\left(x,0\right)=x^{{\displaystyle \frac{\alpha +\gamma -1}{2}}},$$

By using the fundamental properties of FrFrRPDTM, the following recurrence equation is obtained:

$$\begin{gathered} Y_{k+1}^{\alpha ,\gamma }\left(x\right)={\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{\displaystyle \frac{1}{\left(k+1\right)}}\left[\sum _{l=0}^k{Y}_l^{\alpha ,\gamma }\left(x\right){}_{\left(2\right)}{}^{MK}D_x^{\alpha ,\gamma }{Y}_{k-l}^{\alpha ,\gamma }\left(x\right) \\ +\sum _{l=0}^k{{{}_{\left(1\right)}{}^{MK}D}_x^{\alpha ,\gamma }Y}_l^{\alpha ,\gamma }\left(x\right){{}_{\left(1\right)}{}^{MK}D}_x^{\alpha ,\gamma }{Y}_{k-l}^{\alpha ,\gamma }\left(x\right)+Y_k^{\alpha ,\gamma }\left(x\right)\right], \end{gathered}$$

From the initial condition $y\left(x,0\right)=x^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}$ we have $Y_0^{\alpha ,\gamma }\left(x\right)=x^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}$, and from the above recurrence equation, it follows:

$$\begin{gathered} Y_1^{\alpha ,\gamma }\left(x\right)={\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}, \\ {Y}_2^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}{x}^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}, \\ {Y}_3^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^3{\displaystyle \frac{1}{3!}}{x}^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}, \\ \dots , \\ {Y}_n^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^n{\displaystyle \frac{1}{n!}}{x}^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}, \\ \dots \end{gathered}$$

Therefore, we can easily write the closed form of the solution as:

$$\begin{array}{c}y\left(x,t\right)={\displaystyle \sum _{k=0}^{\infty }}{Y}_k^{\alpha ,\gamma }\left(x\right)t^{k\left(\alpha +\gamma -1\right)}\\ =x^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}+{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}{t}^{\alpha +\gamma -1}\\ +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}{x}^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}{t}^{2\left(\alpha +\gamma -1\right)}\\ +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^3{\displaystyle \frac{1}{3!}}{x}^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}{t}^{3\left(\alpha +\gamma -1\right)}+\dots \\ =x^{{\displaystyle \frac{\alpha +\gamma -1}{2}}}{e}^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}}.\end{array}$$

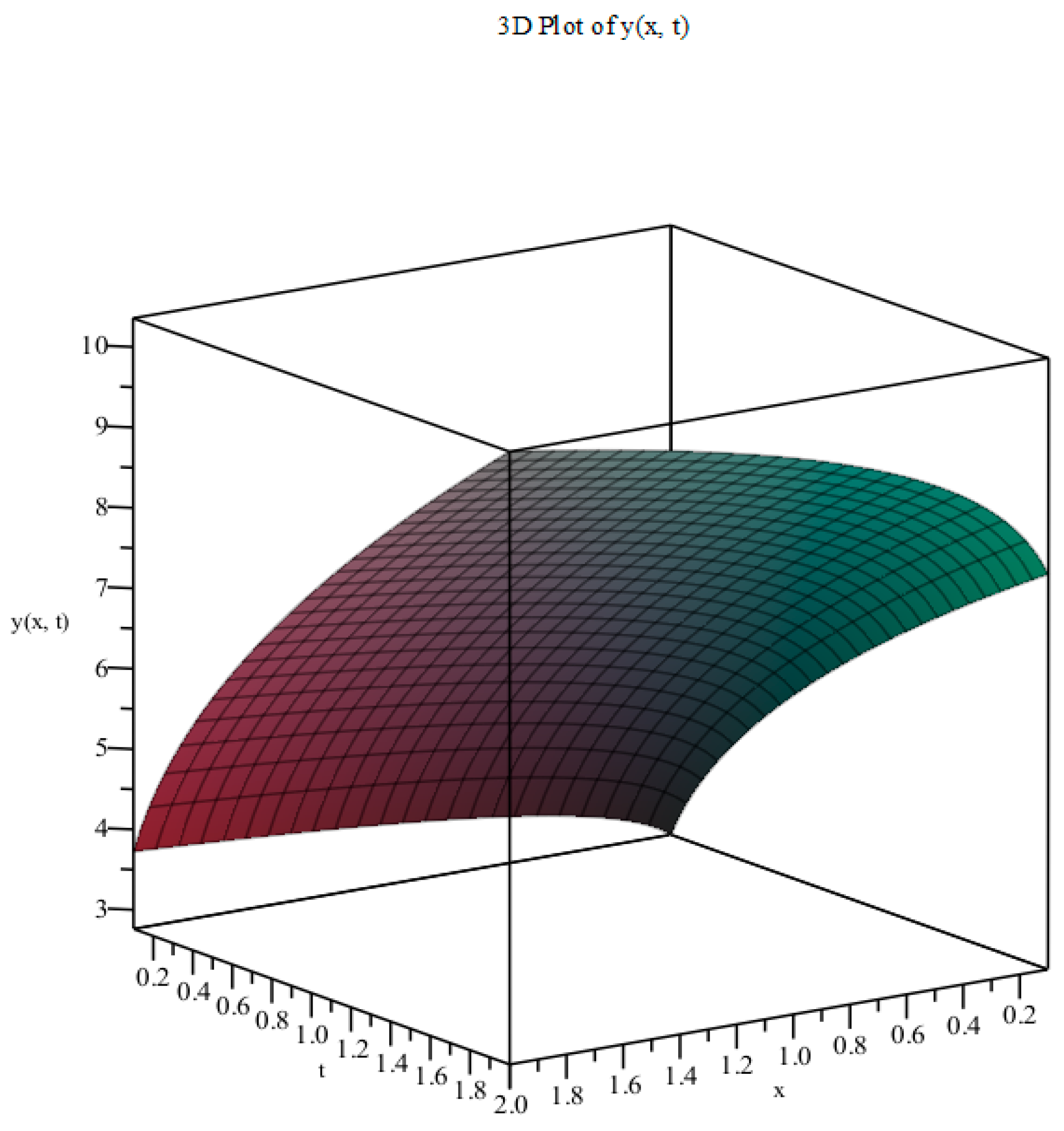
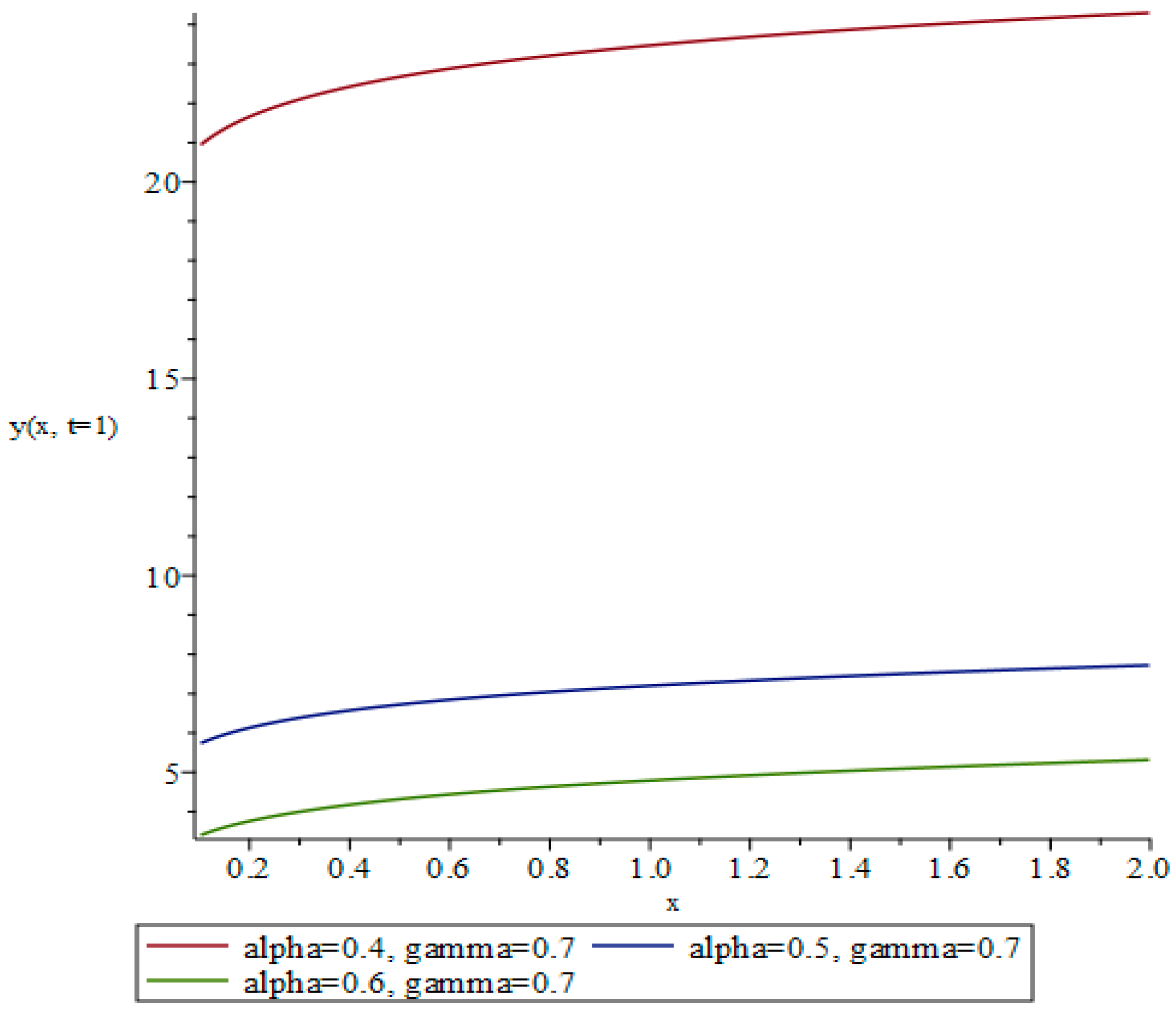
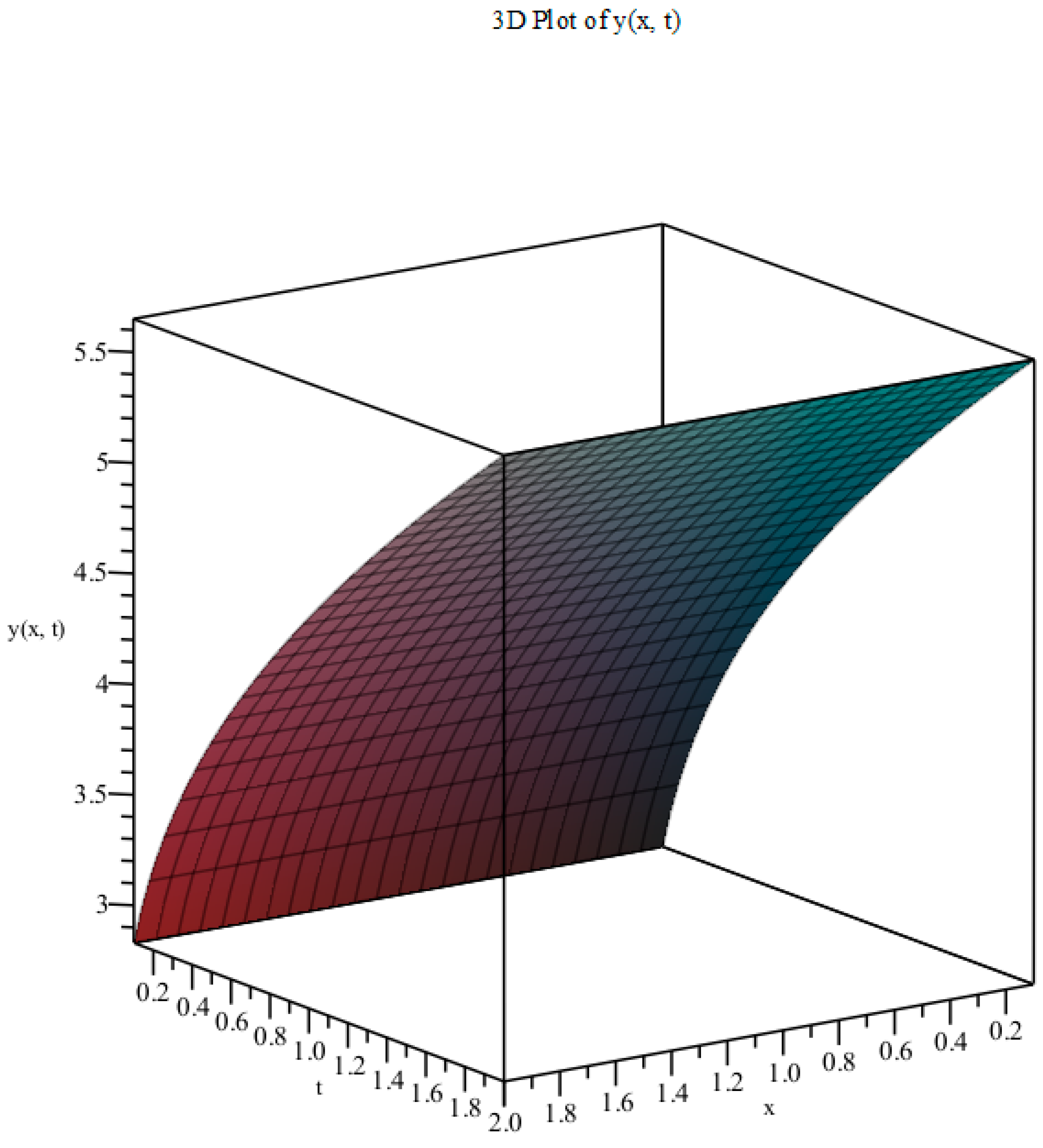
The solution in Example 1 has been plotted in both 3-D (Figure 1) and 2-D (Figure 2) using MAPLE for various parameters’ values where ($0<\alpha ,\gamma \le 1$) and ($\alpha +\gamma -1>0$).

Example 2. 

Consider the following MK nonlinear partial differential equation in $\alpha ,\gamma$

$$\begin{array}{ll}{{}_{\left(1\right)}{}^{MK}D}_t^{\alpha ,\gamma }y\left(x,t\right)& ={}_{\left(2\right)}{}^{MK}D_t^{\alpha ,\gamma }y\left(x,t\right)+y\left(x,t\right)+{y\left(x,t\right)}^2\\ & -2\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)x^{\alpha +\gamma -1}{t}^{\alpha +\gamma -1}\\ & -4{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{x}^{2\left(\alpha +\gamma -1\right)}{t}^{2\left(\alpha +\gamma -1\right)},\\ & 0<x\le \pi ,t>0\end{array}$$

(21)

with the initial conditions:

$$y\left(x,0\right)=0,{}_{\left(1\right)}{}^{MK}D_t^{\alpha ,\gamma }y\left(x,0\right)=2x^{\alpha +\gamma -1},$$

By using the fundamental properties of FrFrRPDTM, the following recurrence equation is obtained:

$$\begin{array}{ll}{Y}_{k+2}^{\alpha ,\gamma }\left(x\right)& ={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{\left(k+1\right)\left(k+2\right)}}[{{}_{\left(2\right)}{}^{MK}D}_x^{\alpha ,\gamma }{Y}_k^{\alpha ,\gamma }\left(x\right)+Y_k^{\alpha ,\gamma }\left(x\right)\\ & +{\displaystyle \sum _{l=0}^k}{Y}_l^{\alpha ,\gamma }\left(x\right)Y_{k-l}^{\alpha ,\gamma }\left(x\right)-2{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)x}^{\alpha +\gamma -1}\delta \left(k-1\right)\\ & -4{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{x}^{2\left(\alpha +\gamma -1\right)}\delta \left(k-2\right)],\end{array}$$

From the initial conditions: $y\left(0\right)=0,{{}_{\left(1\right)}{}^{MK}D}_t^{\alpha ,\gamma }y\left(x,0\right)=2x^{\alpha +\gamma -1}$, we have: $Y_0^{\alpha ,\gamma }\left(x\right)=0,Y_1^{\alpha ,\gamma }\left(x\right)=2{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)x}^{\alpha +\gamma -1}$, and from the above recurrence equation, it follows:

$$Y_k^{\alpha ,\gamma }\left(x\right)=0,k=\mathrm{2,3},\dots ,$$

By using the inverse FrFrRPDT, we obtain the solution in a closed form by:

$$y\left(x,t\right)=2\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)x^{\alpha +\gamma -1}{t}^{\alpha +\gamma -1}.$$

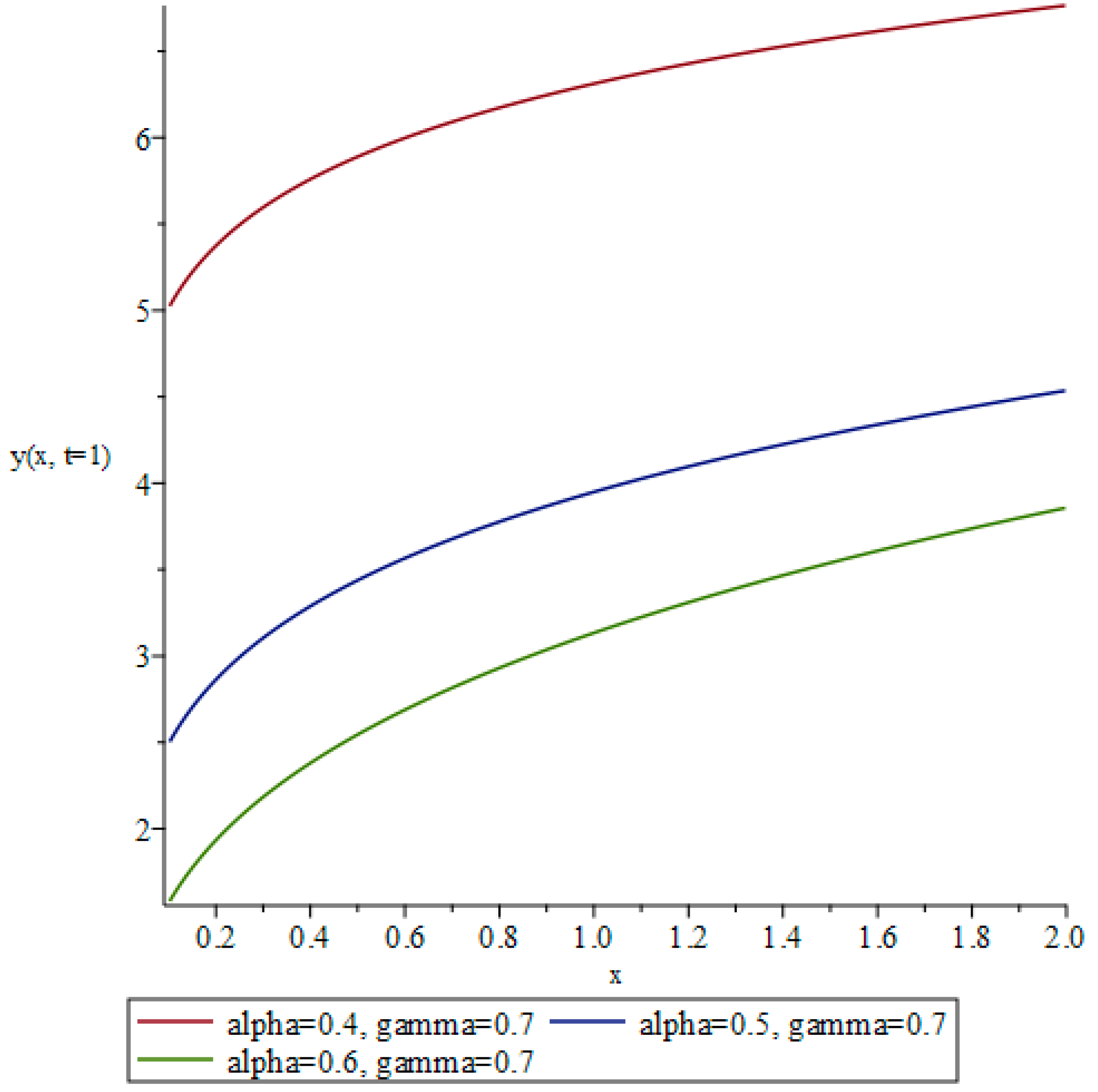
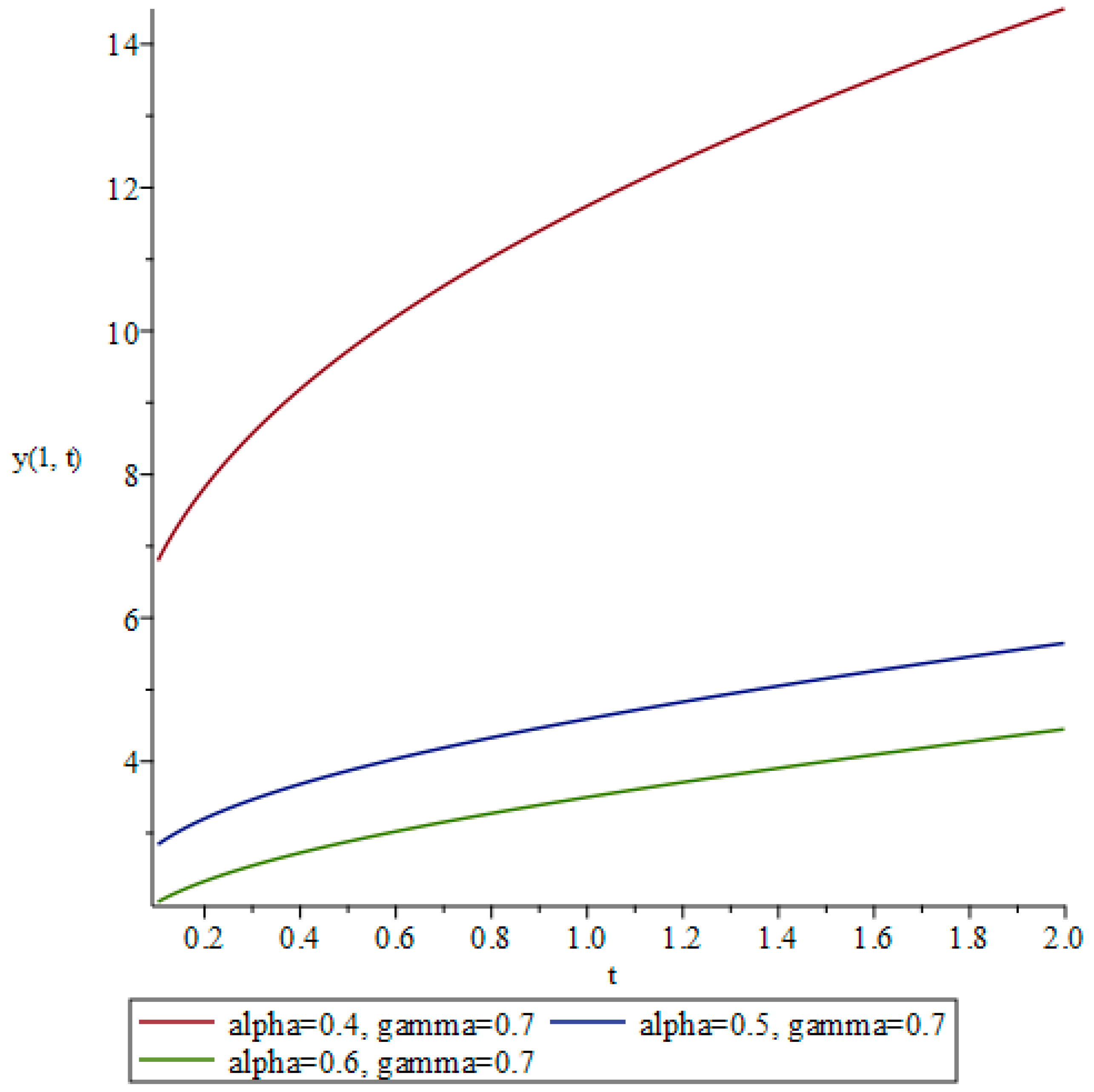
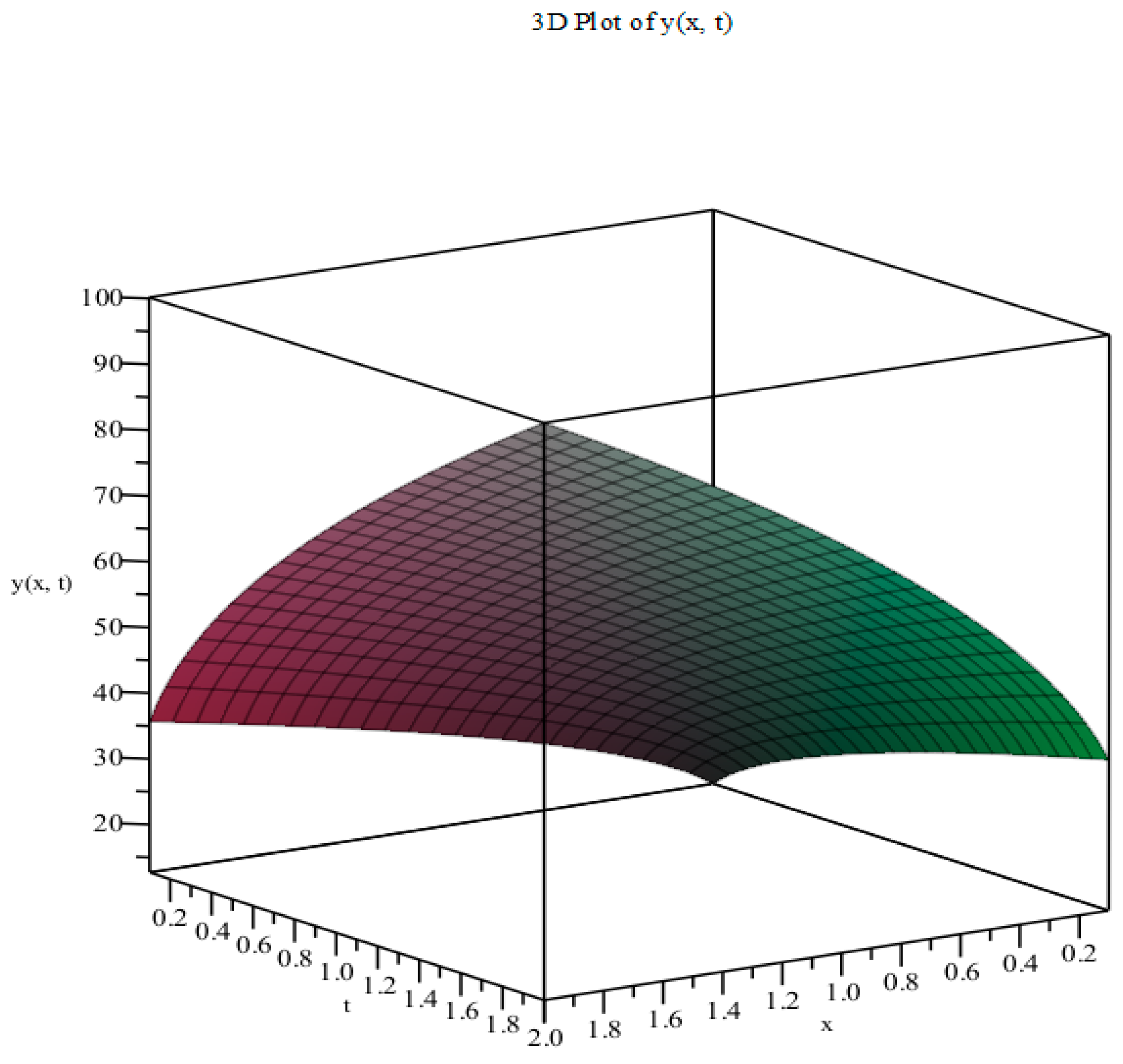
The solution in Example 2 has been plotted in both 3-D (Figure 3) and 2-D (Figure 4) using MAPLE for various parameters’ values where ($0<\alpha ,\gamma \le 1$) and ($\alpha +\gamma -1>0$).

Example 3. 

Consider the initial value problem involving MK homogeneous Klein–Gordon in $\alpha ,\gamma$ as follows:

$${{}_{\left(2\right)}{}^{MK}D}_t^{\alpha ,\gamma }y\left(x,t\right)-{}_{\left(2\right)}{}^{MK}D_t^{\alpha ,\gamma }y\left(x,t\right)-y\left(x,t\right)=0,$$

(22)

with the initial conditions:

$$y\left(x,0\right)=1+sin\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}\right),{}_{\left(1\right)}{}^{MK}D_t^{\alpha ,\gamma }y\left(x,0\right)=0,$$

By using the fundamental properties of FrFrRPDTM, the following recurrence equation is obtained:

$$Y_{k+2}^{\alpha ,\gamma }\left(x\right)=-{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{\left(k+1\right)\left(k+2\right)}}\left[{{}_{\left(2\right)}{}^{MK}D}_x^{\alpha ,\gamma }{Y}_k^{\alpha ,\gamma }\left(x\right)+Y_k^{\alpha ,\gamma }\left(x\right)\right],$$

From the initial conditions: $y\left(x,0\right)=1+sin\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}\right),{{}_{\left(1\right)}{}^{MK}D}_t^{\alpha ,\gamma }y\left(x,0\right)=0,$ we have: $Y_0^{\alpha ,\gamma }\left(x\right)=1+sin\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}\right),Y_1^{\alpha ,\gamma }\left(x\right)=0$, and from the above recurrence equation, it follows:

$$\begin{gathered} Y_2^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}, \\ {Y}_3^{\alpha ,\gamma }\left(x\right)=0, \\ {Y}_4^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^4{\displaystyle \frac{1}{4!}}, \\ {Y}_5^{\alpha ,\gamma }\left(x\right)=0, \\ {Y}_6^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^6{\displaystyle \frac{1}{6!}}, \\ {Y}_7^{\alpha ,\gamma }\left(x\right)=0, \\ \dots , \end{gathered}$$

Therefore, we can easily write the closed form of the solution as:

$$\begin{array}{c}y\left(t\right)={\displaystyle \sum _{k=0}^{\infty }}{Y}_k^{\alpha ,\gamma }\left(x\right)t^{k\left(\alpha +\gamma -1\right)}\\ =1+sin\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}\right)+{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}{t}^{2\left(\alpha +\gamma -1\right)}\\ +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^4{\displaystyle \frac{1}{4!}}{t}^{4\left(\alpha +\gamma -1\right)}+{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^6{\displaystyle \frac{1}{6!}}{t}^{6\left(\alpha +\gamma -1\right)}+\dots \\ =sin\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}\right)+cosh\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}\right),\end{array}$$

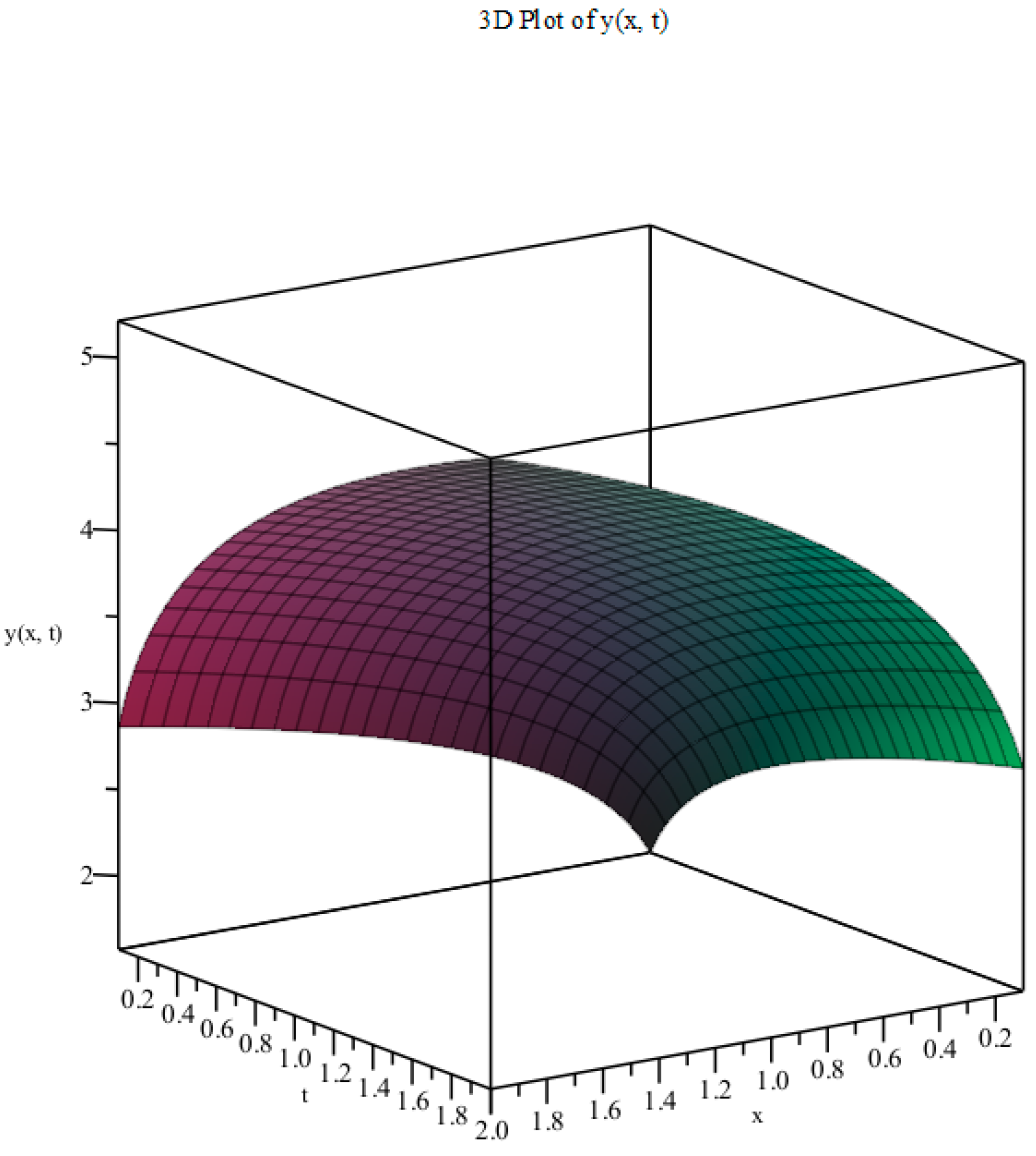
The solution in Example 3 has been plotted in both 3-D (Figure 5) and 2-D (Figure 6) using MAPLE for various parameters’ values where ($0<\alpha ,\gamma \le 1$) and ($\alpha +\gamma -1>0$).

Example 4. 

Consider the problem involving MK inhomogeneous Goursat equation in $\alpha ,\gamma$ as follows:

$${{}_{\left(2\right)}{}^{MK}D}_{x,t}^{\alpha ,\gamma }y\left(x,t\right)=y\left(x,t\right)+4x^{\alpha +\gamma -1}{t}^{\alpha +\gamma -1}-x^{2\left(\alpha +\gamma -1\right)}{t}^{2\left(\alpha +\gamma -1\right)},$$

(23)

with the initial conditions:

$$y\left(x,0\right)=e^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}},y\left(0,t\right)=e^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{\alpha +\gamma -1}},y\left(\mathrm{0,0}\right)=1,$$

Taking FrFrRPDTM in the above equation gives:

$$\begin{array}{cc}\hfill \left(k+1\right)& {{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{{}_{\left(1\right)}{}^{MK}D}_x^{\alpha ,\gamma }Y}_{k+1}^{\alpha ,\gamma }\left(x\right)\hfill \\ & =Y_k^{\alpha ,\gamma }\left(x\right)+4x^{\alpha +\gamma -1}\delta \left(k-1\right)\hfill \\ & -x^{2\left(\alpha +\gamma -1\right)}\delta \left(k-2\right),\hfill \end{array}$$

(24)

$$Y_0^{\alpha ,\gamma }\left(x\right)=e^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}},$$

(25)

By substituting Equation (25) into Equation (24) and using the recurrence relation, we have:

$$\begin{gathered} Y_1^{\alpha ,\gamma }\left(x\right)={\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{e}^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}}, \\ {Y}_2^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}{e}^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}}+{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{x}^{2\left(\alpha +\gamma -1\right)}, \\ {Y}_3^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^3{\displaystyle \frac{1}{3!}}{e}^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}}, \\ {Y}_5^{\alpha ,\gamma }\left(x\right)=0, \\ {Y}_4^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^4{\displaystyle \frac{1}{4!}}{e}^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}}, \\ \dots \end{gathered}$$

and so on.

In general, we have: $Y_k^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^k{\displaystyle \frac{1}{k!}}{e}^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}}$ except $Y_2^{\alpha ,\gamma }\left(x\right)={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}{e}^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}}+{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{x}^{2\left(\alpha +\gamma -1\right)}$.

Therefore, we can easily write the closed form of the solution as:

$$\begin{array}{c}y\left(t\right)={\displaystyle \sum _{k=0}^{\infty }}{Y}_k^{\alpha ,\gamma }\left(x\right)t^{k\left(\alpha +\gamma -1\right)}\\ ={\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{x}^{2\left(\alpha +\gamma -1\right)}{t}^{2\left(\alpha +\gamma -1\right)}\\ +e^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{x}^{\alpha +\gamma -1}}(1+{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}{t}^{k\left(\alpha +\gamma -1\right)}\\ +{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^2{\displaystyle \frac{1}{2!}}{t}^{2\left(\alpha +\gamma -1\right)}+{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^3{\displaystyle \frac{1}{3!}}{t}^{3\left(\alpha +\gamma -1\right)}+\dots )\\ ={{\left({\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\right)}^{2}x}^{2\left(\alpha +\gamma -1\right)}{t}^{2\left(\alpha +\gamma -1\right)}+e^{{\displaystyle \frac{\gamma }{\left(\alpha +\gamma -1\right)\Gamma \left(\alpha \right)}}\left(x^{\alpha +\gamma -1}+t^{\alpha +\gamma -1}\right)}.\end{array}$$

The solution in Example 4 has been plotted in both 3-D (Figure 7) and 2-D (Figure 8) using MAPLE for various parameters’ values where ($0<\alpha ,\gamma \le 1$) and ($\alpha +\gamma -1>0$).

6. Conclusions

In this paper, a new extension of the recently introduced theory of MK calculus to the important field of partial differential equations has been addressed. This study has been approached by applying the well-known reduced differential transformation, which is derived from the Taylor series expansion of a real-valued function of several real variables, which in this paper, we formulate in the sense of the MK derivative. The establishment of this new concept and its fundamental properties requires the development in the context of MK calculus of essential notions and results of classical mathematical analysis of functions of several real variables. Thus, we have first introduced basic elements such as the concept of the MK partial $\alpha ,\gamma$-derivative at a point and the notion of class function $C_{\alpha ,\gamma }^p$ on a certain open set $D\subset R^n$. Furthermore, in the context of real functions of two real variables, we have formulated the classical Clairaut theorem in the sense of the MK $\alpha ,\gamma$-partial derivative, which we have extended to functions of class $C_{\alpha ,\gamma }^p$ on a given open set $D\subset R^2$. Next, we have established the Taylor formula FrFr with Lagrange residue in the sense of the MK partial $\alpha ,\gamma$-derivative. Finally, we have discussed a sufficient condition for a function of class $C_{\alpha ,\gamma }^{\infty }$ on a bounded open set $D\subset R^2$ to be expanded into a convergent infinite series, the so-called Taylor series FrFr. This theoretical study has been completed by defining the FrFrRPDT, and establishing its fundamental properties, which will allow the construction of the FrFrRPDTM. The use of this new technique has been illustrated with several interesting examples of linear and nonlinear MK partial differential equations, among which the Klein–Gordon equation and the Goursat equation stand out. In conclusion, the results obtained show that the proposed technique, by including the MK derivative operator, provides a simple and efficient mathematical tool to model various problems and solve various classes of differential equations, both linear and nonlinear. Finally, we would like to point out that the study carried out can be continued in several future directions, such as completing the theory of FrFrPDTM for some nonlinear functions, applying this technique to the resolution of problems involving MK integrodifferential equations, or deepening the theory of MK calculus for real-valued functions of several real variables.