Mathematical Calculations for the Design of Elliptical Isolated Foundations with Optimal Cost
Abstract
1. Introduction
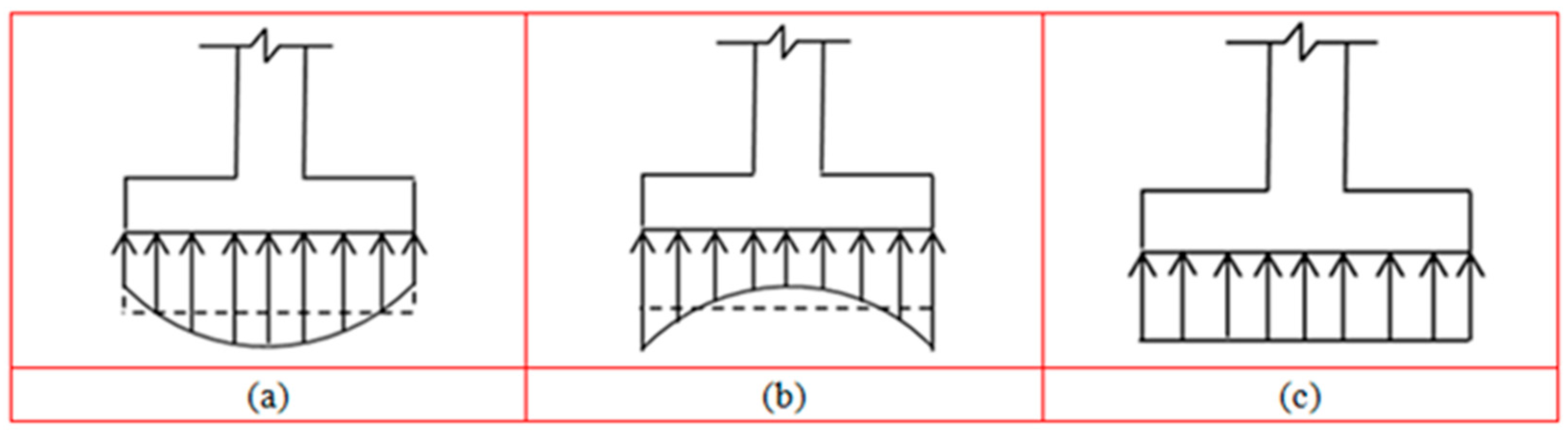
2. Formulation of the Model
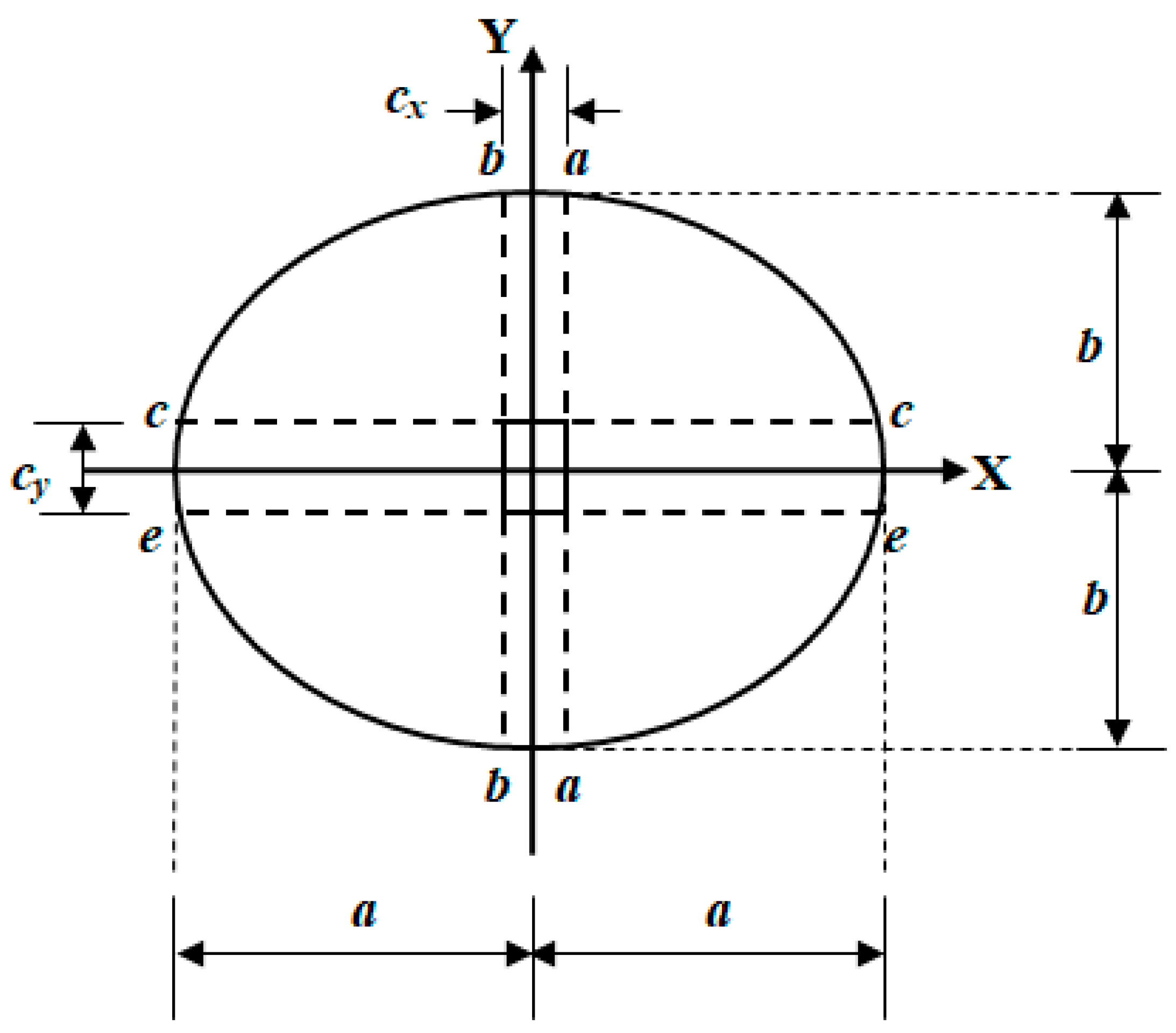
2.1. Minimum Surface for an Elliptical Isolated Footing
2.2. Minimum Cost for an Elliptical Isolated Footing
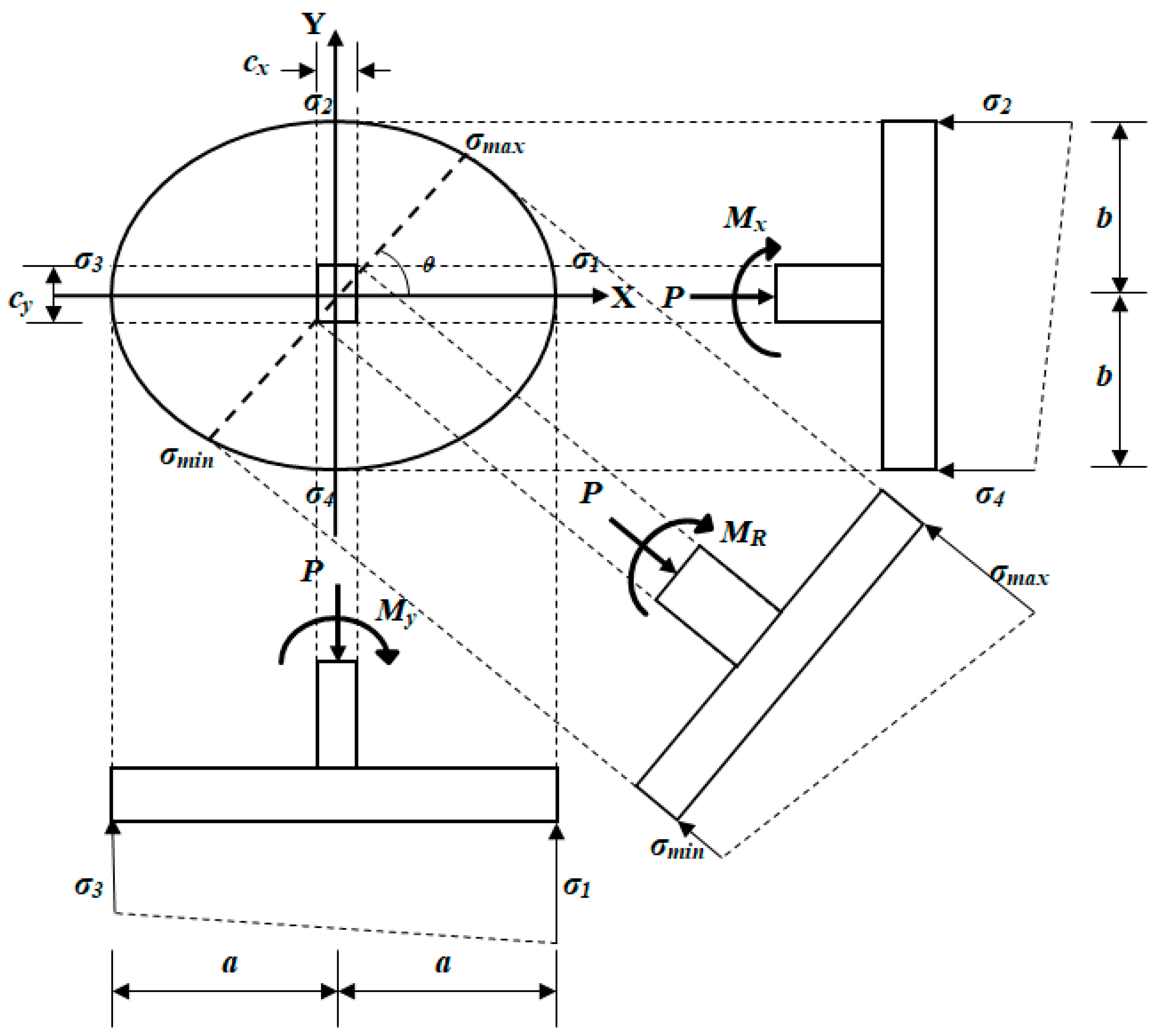
2.2.1. Moments
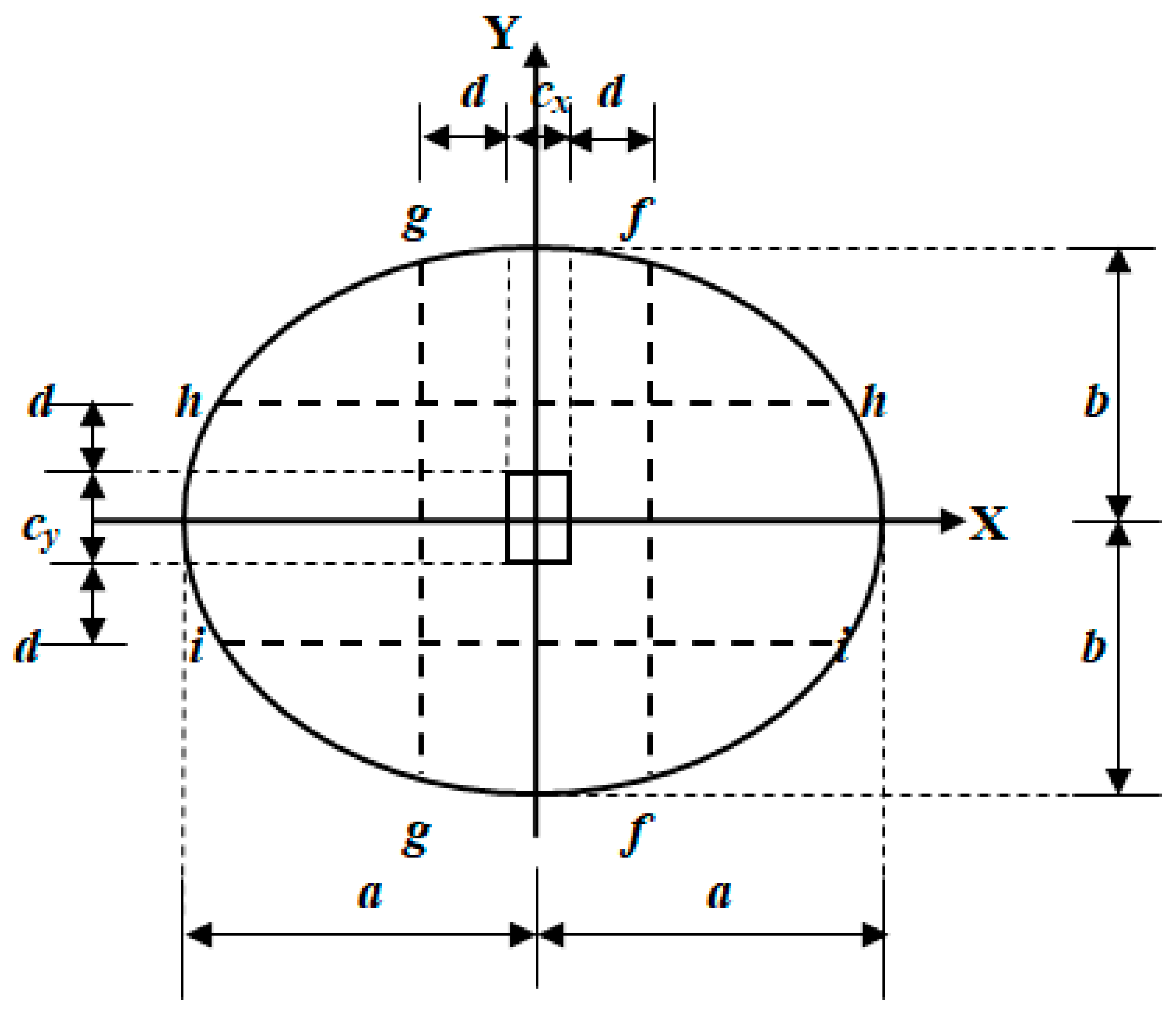
2.2.2. Bending Shear
2.2.3. Punching Shear
2.2.4. Objective Function
2.2.5. Constraint Functions
3. Numerical Examples
4. Results
- Substituting a = D/2, b = D/2 into Equation (12) gives σu(x, y), and substituting ex = 0, ey = 0 into Equation (8) of the reference [47] gives σu(x, y); both are equal.
- Substituting a = D/2, b = D/2 into Equation (14) gives Mua, and substituting ex = 0, ey = 0 into Equation (14) of the reference [47] gives Muc, Mua = Muc (both are parallel to the Y axis).
- Substituting a = D/2, b = D/2 into Equation (16) gives Mub, and substituting ex = 0, ey = 0 into Equation (16) of the reference [47] gives Mue, Mub = Mue (both are parallel to the Y axis).
- Substituting a = D/2, b = D/2 into Equation (18) gives Muc, and substituting ex = 0, ey = 0 into Equation (10) of the reference [47] gives Mua, Muc = Mua (both are parallel to the X axis).
- Substituting a = D/2, b = D/2 into Equation (20) gives Mue, and substituting ex = 0, ey = 0 into Equation (12) of the reference [47] gives Mub, Mue = Mub (both are parallel to the X axis).
- Substituting a = D/2, b = D/2 into Equation (21) gives Vuf, and substituting ex = 0, ey = 0 into Equation (22) of the reference [47] gives Vubh, Vuf = Vubh (both are parallel to the Y axis).
- Substituting a = D/2, b = D/2 into Equation (23) gives Vug, and substituting ex = 0, ey = 0 into Equation (24) of the reference [47] gives Vubi, Vug = Vubi (both are parallel to the Y axis).
- Substituting a = D/2, b = D/2 into Equation (25) gives Vuh, and substituting ex = 0, ey = 0 into Equation (18) of the reference [47] gives Vubf, Vuh = Vubf (both are parallel to the X axis).
- Substituting a = D/2, b = D/2 into Equation (27) gives Vui, and substituting ex = 0, ey = 0 into Equation (20) of the reference [47] gives Vubg, Vui = Vubg (both are parallel to the X axis).
- Substituting a = D/2, b = D/2 into Equation (29) gives Vup, and substituting ex = 0, ey = 0, x1 = cx/2 + d/2, x2 = −cx/2 − d/2, y1 = cy/2 + d/2, y2 = −cy/2 − d/2 into Equation (26) of the reference [47] gives Vup, both are equal.
- Integrating Equation (13) from zero to “a” and obtaining moments at the free end gives Mua = 0.
- Integrating Equation (15) from “−a” to “a” and obtaining moments at the free end gives Mub = 0.
- Integrating Equation (13) from zero to “a” and obtaining moments at the center of footing gives Mua = −2Pua/3π − Myu/2. Integrating Equation (15) from “−a” to zero and obtaining moments at the center of footing gives Mub = 2Pua/3π + Myu/2. Therefore, Mua and Mub are the same, but in the opposite sense. Thus, continuity is guaranteed.
- Integrating Equation (17) from zero to “b” and obtaining moments at the free end gives Muc = 0.
- Integrating Equation (19) from “−b” to “b” and obtaining moments at the free end gives Mue = 0.
- Integrating Equation (17) from zero to “b” and obtaining moments at the center of footing gives Muc = −2Pub/3π − Mxu/2. Integrating Equation (19) from “−b” to zero and obtaining moments at the center of footing gives Mue = 2Pub/3π + Mxu/2. Therefore, Muc and Mue are the same, but in the opposite sense. Thus, continuity is guaranteed.
- Integrating Equation (21) from zero to “a” and obtaining the bending shear at the free end gives Vuf = 0.
- Integrating Equation (23) from “−a” to “a” and obtaining the bending shear at the free end gives Vug = 0.
- Integrating Equation (21) from zero to “a” and obtaining the bending shear at the center of footing gives Vuf = −Pu/2 − 8Myu/3aπ. Integrating Equation (23) from “−a” to zero and obtaining the bending shear at the center of footing gives Vug = Pu/2 + 8Myu/3aπ. Therefore, Vuf and Vug are the same, but in the opposite sense. Thus, continuity is guaranteed.
- Integrating Equation (25) from zero to “b” and obtaining the bending shear at the free end gives Vuh = 0.
- Integrating Equation (27) from “−b” to “b” and obtaining the bending shear at the free end gives Vui = 0.
- Integrating Equation (25) from zero to “b” and obtaining the bending shear at the center of footing gives Vuh = −Pu/2 − 8Mxu/3bπ. Integrating Equation (27) from “−b” to zero and obtaining the bending shear at the center of footing gives Vui = Pu/2 + 8Mxu/3bπ. Therefore, Vuh and Vui are the same, but in the opposite sense. Thus, continuity is guaranteed.
- Integrating Equation (29) from zero to “a” in the X direction and from zero to “b” in the Y direction to obtain the punching shear at the free ends of the footing results in zero.
5. Conclusions
- (1)
- There is no literature on the subject of elliptical footings since the closest are circular footings; therefore, it is an innovative topic.
- (2)
- The moments, bending shears, and punching shear are verified by equilibrium (see Section 4).
- (3)
- The new model for elliptical footings can be applied to other building standards, taking into account the moments, bending shears, and punching shear that must be resisted.
- (4)
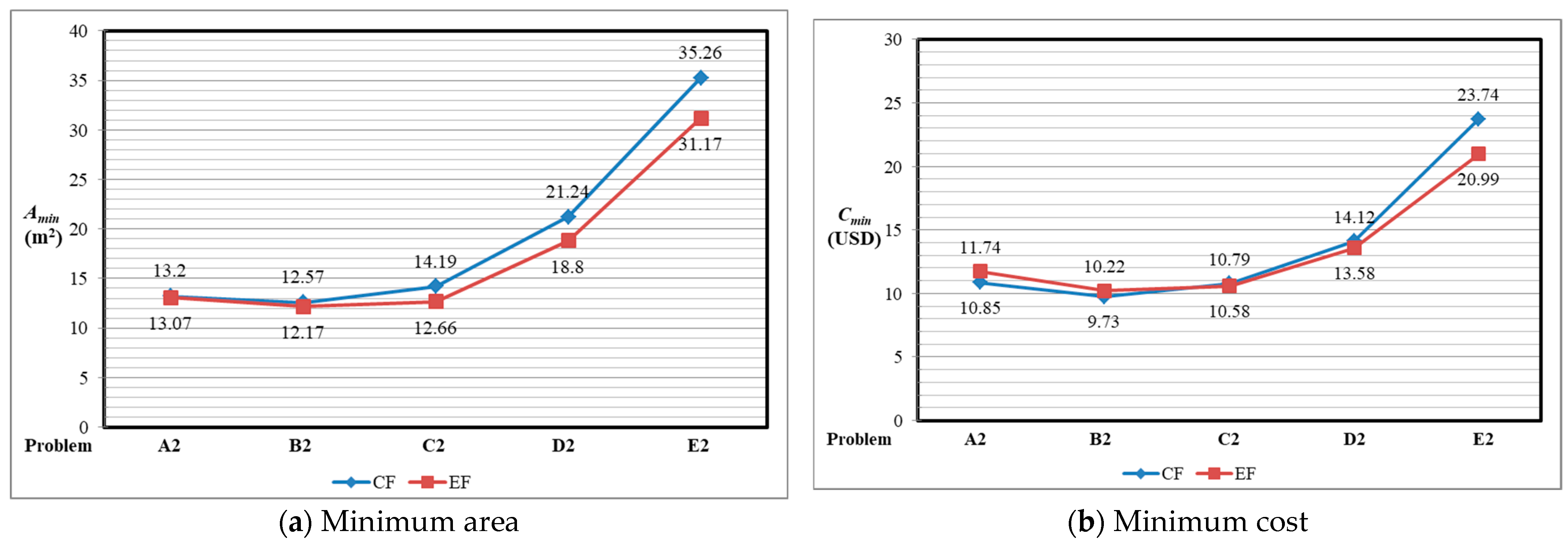
- When the factored moment about the Y axis “Myu” is reduced, the minimum area “Amin” and the minimum cost “Cmin” are reduced (see Table 2).
- (5)
- (6)
- The minimum area is smaller for elliptical footings than for circular footings in all problems (see Figure 7a).
- (7)
- (8)
- (9)
- The elliptical footing model presents a saving of 11.60% in the minimum area and 11.58% in the minimum cost for E2 compared to the circular footing model (see Figure 7).
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A


References
- Bowles, J.E. Foundation Analysis and Design; McGraw-Hill: New York, NY, USA, 2001. [Google Scholar]
- Shahin, M.A.; Cheung, E.M. Stochastic design charts for bearing capacity of strip footings. Geomech. Eng. 2011, 3, 153–167. [Google Scholar] [CrossRef]
- Dixit, M.S.; Patil, K.A. Experimental estimate of Nγ values and corresponding settlements for square footings on finite layer of sand. Geomech. Eng. 2013, 5, 363–377. [Google Scholar] [CrossRef]
- ErzÍn, Y.; Gul, T.O. The use of neural networks for the prediction of the settlement of pad footings on cohesionless soils based on standard penetration test. Geomech. Eng. 2013, 5, 541–564. [Google Scholar] [CrossRef]
- Colmenares, J.E.; Kang, S.-R.; Shin, Y.-J.; Shin, J.-H. Ultimate bearing capacity of conical shell foundations. Struct. Eng. Mech. 2014, 52, 507–523. [Google Scholar] [CrossRef]
- Cure, E.; Sadoglu, E.; Turker, E.; Uzuner, B.A. Decrease trends of ultimate loads of eccentrically loaded model strip footings close to a slope. Geomech. Eng. 2014, 6, 469–485. [Google Scholar] [CrossRef]
- Fattah, M.Y.; Yousif, M.A.; Al-Tameemi, S.M.K. Effect of pile group geometry on bearing capacity of piled raft foundations. Struct. Eng. Mech. 2015, 54, 829–853. [Google Scholar] [CrossRef]
- Uncuoğlu, E. The bearing capacity of square footings on a sand layer overlying clay. Geomech. Eng. 2015, 9, 287–311. [Google Scholar] [CrossRef]
- Anil, Ö.; Akbaş, S.O.; BabagĪray, S.; Gel, A.C.; Durucan, C. Experimental and finite element analyses of footings of varying shapes on sand. Geomech. Eng. 2017, 12, 223–238. [Google Scholar] [CrossRef]
- Khatri, V.N.; Debbarma, S.P.; Dutta, R.K.; Mohanty, B. Pressure-settlement behavior of square and rectangular skirted footings resting on sand. Geomech. Eng. 2017, 12, 689–705. [Google Scholar] [CrossRef]
- Mohebkhah, A. Bearing capacity of strip footings on a stone masonry trench in clay. Geomech. Eng. 2017, 13, 255–267. [Google Scholar] [CrossRef]
- Zhang, W.-X.; Wu, H.; Hwang, H.-J.; Zhang, J.-Y.; Chen, B.; Yi, W.-J. Bearing behavior of reinforced concrete column-isolated footing substructures. Eng. Struct. 2019, 200, 109744. [Google Scholar] [CrossRef]
- Turedi, Y.; Emirler, B.; Ornek, M.; Yildiz, A. Determination of the bearing capacity of model ring footings: Experimental and numerical investigations. Geomech. Eng. 2019, 18, 29–39. [Google Scholar] [CrossRef]
- Gnananandarao, T.; Khatri, V.N.; Dutta, R.K. Bearing capacity and settlement prediction of multi-edge skirted footings resting on sand. Ing. Investig. 2020, 40, 9–21. [Google Scholar] [CrossRef]
- Gör, M. Analyzing the bearing capacity of shallow foundations on two-layered soil using two novel cosmology-based optimization techniques. Smart Struct. Syst. 2022, 29, 513–522. [Google Scholar] [CrossRef]
- Hu, D.; Hu, Y.; Hu, R.; Tan, Z.; Ni, P.; Chen, Y.; Xiang, X.; Li, Y.; Liu, J. Machine Learning–Finite Element Mesh Optimization-Based Modeling and Prediction of Excavation-Induced Shield Tunnel Ground Settlement. Int. J. Comput. Methods 2024, 22, 2450066. [Google Scholar] [CrossRef]
- Yin, Q.; Xin, T.; Zhenggang, H.; Minghua, H. Measurement and Analysis of Deformation of Underlying Tunnel Induced by Foundation Pit Excavation. Adv. Civil Eng. 2023, 1, 8897139. [Google Scholar] [CrossRef]
- Li, Z.-Q.; Nie, L.; Yiguo Xue, Y.; Li, W.; Fan, K. Model Testing on the Processes, Characteristics, and Mechanism of Water Inrush Induced by Karst Caves Ahead and Alongside a Tunnel. Rock Mech. Rock Eng. 2025, 58, 5363–5380. [Google Scholar] [CrossRef]
- Zhang, J.; Li, Y.; Zhang, C. Pounding induced overturning resistance of FPB-isolated structures considering soil-structure-interactions. Soil Dyn. Earthq. Eng. 2024, 177, 108416. [Google Scholar] [CrossRef]
- Ren, S.-P.; Chen, X.; Ren, Z.-L.; Cheng, P.; Liu, Y. Large-deformation modelling of earthquake-triggered landslides considering non-uniform soils with a stratigraphic dip. Comput. Geotech. 2023, 159, 105492. [Google Scholar] [CrossRef]
- Liu, Y.; Chen, X.; Hu, M. Three-dimensional large deformation modeling of landslides in spatially variable and strain-softening soils subjected to seismic loads. Can. Geotech. J. 2023, 60, 426–437. [Google Scholar] [CrossRef]
- Chaabani, W.; Remadna, M.S.; Abu-Farsakh, M. Numerical Modeling of the Ultimate Bearing Capacity of Strip Footings on Reinforced Sand Layer Overlying Clay with Voids. Infrastructures 2023, 8, 3. [Google Scholar] [CrossRef]
- Vitone, D.M.A.; Valsangkar, A.J. Stresses from loads over rectangular areas. J. Geotech. Geoenviron. 1986, 112, 961–964. [Google Scholar] [CrossRef]
- Michalowski, R.L. Upper-bound load estimates on square and rectangular footings. Géotechnique 2001, 51, 787–798. [Google Scholar] [CrossRef]
- Özmen, G. Determination of base stresses in rectangular footings under biaxial bending. Teknik Dergi Digest 2011, 22, 1519–1535. Available online: https://dergipark.org.tr/tr/download/article-file/136568 (accessed on 1 May 2025).
- Aydogdu, I. New Iterative method to Calculate Base Stress of Footings under Biaxial Bending. Int. J. Eng. Appl. Sci. 2016, 8, 40–48. [Google Scholar] [CrossRef][Green Version]
- Girgin, K. Simplified formulations for the determination of rotational spring constants in rigid spread footings resting on tensionless soil. JCEM 2017, 23, 464–474. [Google Scholar] [CrossRef]
- Al-Gahtani, H.J.; Adekunle, S.K. A boundary-type approach for the computation of vertical stresses in soil due to arbitrarily shaped foundations. World J. Eng. 2019, 16, 419–426. [Google Scholar] [CrossRef]
- Rawat, S.; Mittal, R.K.; Muthukumar, G. Isolated Rectangular Footings under Biaxial Bending: A Critical Appraisal and Simplified Analysis Methodology. Pract. Period. Struct. Des. Const. 2020, 25, 04020011. [Google Scholar] [CrossRef]
- López-Machado, N.A.; Perez, G.; Castro, C.; Perez, J.C.V.; López-Machado, L.J.; Alviar-Malabet, J.D.; Romero-Romero, C.A.; Guerrero-Cuasapaz, D.P.; Montesinos-Machado, V.V. A Structural Design Comparison Between Two Reinforced Concrete Regular 6-Level Buildings using Soil-Structure Interaction in Linear Range. Ing. Investig. 2022, 42, e86819. [Google Scholar] [CrossRef]
- Lezgy-Nazargah, M.; Mamazizi, A.; Khosravi, H. Analysis of shallow footings rested on tensionless foundations using a mixed finite element model. Struct. Eng. Mech. 2022, 81, 379–394. [Google Scholar] [CrossRef]
- Teng, W.C. Foundation Design; Prentice-Hall Inc.: New Delhi, India, 1979. [Google Scholar]
- Highter, W.H.; Anders, J.C. Dimensioning footings subjected to eccentric loads. J. Geotech. Geoenviron. 1985, 111, 659–665. [Google Scholar] [CrossRef]
- Galvis, F.A.; Smith-Pardo, P.J. Axial load biaxial moment interaction (PMM) diagrams for shallow foundations: Design aids, experimental verification, and examples. Eng. Struct. 2020, 213, 110582. [Google Scholar] [CrossRef]
- Rodriguez-Gutierrez, J.A.; Aristizabal-Ochoa, J.D. Rigid spread footings resting on soil subjected to axial load and biaxial bending. I: Simplified analytical method. Int. J. Geomech. 2013, 13, 109–119. [Google Scholar] [CrossRef]
- Rodriguez-Gutierrez, J.A.; Aristizabal-Ochoa, J.D. Rigid spread footings resting on soil subjected to axial load and biaxial bending. II: Design aids. Int. J. Geomech. 2013, 13, 120–131. [Google Scholar] [CrossRef]
- Maheshwari, P.; Khatri, S. Influence of inclusion of geosynthetic layer on response of combined footings on stone column reinforced earth beds. Geomech. Eng. 2012, 4, 263–279. [Google Scholar] [CrossRef]
- Konapure, C.G.; Vivek, B. Analysis of Combined rectangular footing by Winkler’s Model and Finite Element Method. Int. JEIT 2013, 3, 128–132. Available online: https://www.ijeit.com/Vol%203/Issue%205/IJEIT1412201311_21.pdf (accessed on 1 January 2020).
- Vivek, B.; Arkal, L.S.; Bandgar, R.V.; Kalekhan, F.A.S. Comparative Study on Conventional and Simplified Elastic Analysis of Rectangular Combined Footing. Int. J. Res. Eng. Technol. 2014, 3, 422–427. Available online: https://ijret.org/volumes/2014v03/i04/IJRET20140304076.pdf (accessed on 1 May 2025).
- Ravi Kumar Reddy, C.; Satish Kumar, M.; Kondala Rao, M.; Gopika, N. Numerical Analysis of Rectangular Combined Footings Resting on Soil for Contact Pressure. Int. J. Civil Engi. Technol. 2018, 9, 1425–1431. Available online: http://iaeme.com/Home/issue/IJCIET?Volume=9&Issue=9 (accessed on 31 January 2025).
- Kashani, A.R.; Camp, C.V.; Akhani, M.; Ebrahimi, S. Optimum design of combined footings using swarm intelligence-based algorithms. Adv. Eng. Soft. 2022, 169, 103140. [Google Scholar] [CrossRef]
- Al-Douri, E.M.F. Optimum design of trapezoidal combined footings. Tikrit J. Eng. Sci. 2007, 14, 85–115. [Google Scholar] [CrossRef]
- Luévanos-Rojas, A. Optimization for trapezoidal combined footings: Optimal design. Adv. Concr. Constr. Int. J. 2023, 16, 21–34. [Google Scholar] [CrossRef]
- Moreno-Landeros, V.M.; Luévanos-Rojas, A.; Santiago-Hurtado, G.; López-León, L.D.; Olguin-Coca, F.J.; López-León, A.L.; Landa-Gómez, A.E. Optimal Cost Design of RC T-Shaped Combined Footings. Buildings 2024, 14, 3688. [Google Scholar] [CrossRef]
- Aishwarya, K.M.; Balaji, N.C. Analysis and design of eccentrically loaded corner combined footing for rectangular columns. In Proceedings of the International Conference on Advances in Sustainable Construction Materials, Guntur, India, 18–19 March 2022. [Google Scholar] [CrossRef]
- Luévanos-Rojas, A.; Santiago-Hurtado, G.; Moreno-Landeros, V.M.; Olguin-Coca, F.J.; López-León, L.D.; Diaz-Gurrola, E.R. Mathematical Modeling of the Optimal Cost for the Design of Strap Combined Footings. Mathematics 2024, 12, 294. [Google Scholar] [CrossRef]
- Luévanos-Rojas, A.; Moreno-Landeros, V.M.; Santiago-Hurtado, G.; Olguin-Coca, F.J.; López-León, L.D.; Baltazar-Zamora, M.A.; Diaz-Gurrola, E.R. Mathematical Modeling for the Optimal Cost Design of Circular Isolated Footings with Eccentric Column. Mathematics 2024, 12, 733. [Google Scholar] [CrossRef]
- Elhanash, M.; Elsherif, A.K.; Aref, A.; Nagy, N.M. New analytical method for optimum design of circular and elliptical footings. Adv. Comput. Des. 2025, 10, 151–167. [Google Scholar]
- Das, B.M.; Sordo-Zabay, E.; Arrioja-Juárez, R. Principios de Ingeniería de Cimentaciones; Cengage Learning Latín América: Mexico City, Mexico, 2006. [Google Scholar]
- McCormac, J.C.; Brown, R.H. Design of Reinforced Concrete; John Wiley & Sons, Inc.: Mexico City, Mexico, 2014. [Google Scholar]
- ACI 318-19; Building Code Requirements for Structural Concrete and Commentary. American Concrete Institute: Farmington Hills, MI, USA, 2019.
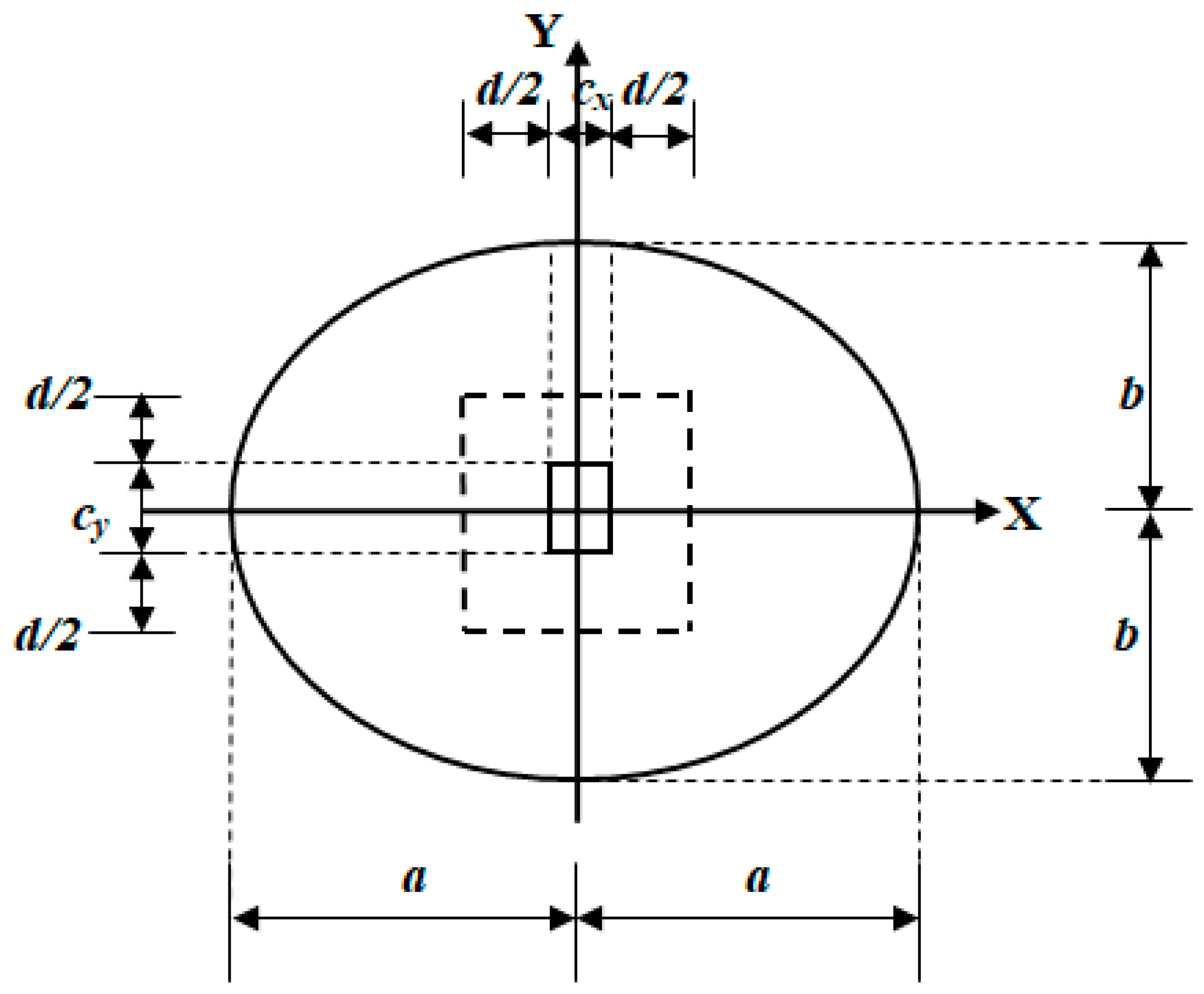

| Example | PD (kN) | PL (kN) | P (kN) | MxD (kN-m) | MxL (kN-m) | Mx (kN-m) | MyD (kN-m) | MyL (kN-m) | My (kN-m) |
|---|---|---|---|---|---|---|---|---|---|
| A.1 | 600 | 500 | 1100 | 300 | 200 | 500 | 500 | 400 | 900 |
| B.1 | 600 | 500 | 1100 | 300 | 200 | 500 | 400 | 300 | 700 |
| C.1 | 600 | 500 | 1100 | 300 | 200 | 500 | 300 | 200 | 500 |
| D.1 | 600 | 500 | 1100 | 300 | 200 | 500 | 200 | 100 | 300 |
| E.1 | 600 | 500 | 1100 | 300 | 200 | 500 | 100 | 50 | 150 |
| Problem | a (m) | b (m) | σmax (kN/m2) | σmin (kN/m2) | Amin (m2) | Pu (kN) | Mxu (kN-m) | Myu (kN-m) | Asx (cm2) | Asy (cm2) | sx (cm) | sy (cm) | d (cm) | ρx | ρy | Cmin (USD) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A.1 | 4.60 | 2.60 | 58.48 | 0.07 | 37.57 | 1520 | 680 | 1240 | 148.05 | 114.82 | 17.77 | 40.49 | 37.50 | 0.00761 | 0.00333 | 31.11Cc |
| B.1 | 3.60 | 2.60 | 74.61 | 0.21 | 29.41 | 1520 | 680 | 960 | 109.52 | 89.66 | 24.03 | 40.60 | 37.50 | 0.00563 | 0.00333 | 22.39Cc |
| C.1 | 2.60 | 2.60 | 103.02 | 0.57 | 21.24 | 1520 | 680 | 680 | 73.07 | 73.07 | 35.94 | 35.94 | 37.50 | 0.00376 | 0.00376 | 14.88Cc |
| D.1 | 1.55 | 2.60 | 173.09 | 0.68 | 12.66 | 1520 | 680 | 400 | 73.03 | 71.37 | 35.82 | 21.95 | 42.50 | 0.00333 | 0.00535 | 10.58Cc |
| E.1 | 1.25 | 2.60 | 196.54 | 18.93 | 10.21 | 1520 | 680 | 200 | 81.14 | 58.55 | 32.05 | 21.56 | 47.50 | 0.00333 | 0.00495 | 9.34Cc |
| Example | PD (kN) | PL (kN) | P (kN) | MxD (kN-m) | MxL (kN-m) | Mx (kN-m) | MyD (kN-m) | MyL (kN-m) | My (kN-m) |
|---|---|---|---|---|---|---|---|---|---|
| A.2 | 800 | 700 | 1500 | 300 | 200 | 500 | 200 | 100 | 300 |
| B.2 | 700 | 600 | 1300 | 300 | 200 | 500 | 200 | 100 | 300 |
| C.2 | 600 | 500 | 1100 | 300 | 200 | 500 | 200 | 100 | 300 |
| D.2 | 500 | 400 | 900 | 300 | 200 | 500 | 200 | 100 | 300 |
| E.2 | 400 | 300 | 700 | 300 | 200 | 500 | 200 | 100 | 300 |
| Problem | a (m) | b (m) | σmax (kN/m2) | σmin (kN/m2) | Amin (m2) | Pu (kN) | Mxu (kN-m) | Myu (kN-m) | Asx (cm2) | Asy (cm2) | sx (cm) | sy (cm) | d (cm) | ρx | ρy | Cmin (USD) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A.2 | 1.60 | 2.60 | 196.98 | 32.57 | 13.07 | 2080 | 680 | 400 | 81.62 | 73.58 | 32.05 | 14.51 | 47.50 | 0.00333 | 0.00486 | 11.74Cc |
| B.2 | 1.55 | 2.50 | 198.24 | 15.34 | 12.17 | 1800 | 680 | 400 | 70.24 | 71.73 | 35.80 | 21.84 | 42.50 | 0.00333 | 0.00546 | 10.22Cc |
| C.2 | 1.55 | 2.60 | 173.09 | 0.68 | 12.66 | 1520 | 680 | 400 | 73.03 | 71.37 | 35.82 | 21.95 | 42.50 | 0.00333 | 0.00535 | 10.58Cc |
| D.2 | 1.90 | 3.15 | 95.50 | 0.24 | 18.80 | 1240 | 680 | 400 | 67.89 | 90.29 | 46.75 | 21.28 | 32.50 | 0.00333 | 0.00733 | 13.58Cc |
| E.2 | 2.45 | 4.05 | 44.77 | 0.14 | 31.17 | 960 | 680 | 400 | 87.81 | 91.11 | 46.60 | 27.21 | 32.50 | 0.00335 | 0.00573 | 20.99Cc |
| Problem | D (m) | σmax (kN/m2) | σmin (kN/m2) | Amin (m2) | Pu (kN) | Mxu (kN-m) | Myu (kN-m) | Asx (cm2) | Asy (cm2) | sx (cm) | sy (cm) | d (cm) | ρx | ρy | Cmin (USD) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A.2 | 4.10 | 199.79 | 27.44 | 13.20 | 2080 | 680 | 400 | 64.54 | 64.54 | 32.21 | 32.21 | 47.50 | 0.00333 | 0.00333 | 10.85Cc |
| B.2 | 4.00 | 196.25 | 10.65 | 12.57 | 1800 | 680 | 400 | 56.33 | 63.52 | 35.82 | 31.77 | 42.50 | 0.00333 | 0.00376 | 9.73Cc |
| C.2 | 4.25 | 154.91 | 0.17 | 14.19 | 1520 | 680 | 400 | 59.87 | 63.86 | 35.82 | 33.58 | 42.50 | 0.00333 | 0.00355 | 10.79Cc |
| D.2 | 5.20 | 84.62 | 0.14 | 21.24 | 1240 | 680 | 400 | 64.69 | 64.69 | 40.60 | 40.60 | 37.50 | 0.00333 | 0.00333 | 14.12Cc |
| E.2 | 6.70 | 39.60 | 0.11 | 35.26 | 960 | 680 | 400 | 83.56 | 83.55 | 40.59 | 40.60 | 37.50 | 0.00333 | 0.00333 | 23.74Cc |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Diaz-Gurrola, E.R.; Luévanos-Rojas, A.; Santiago-Hurtado, G.; Moreno-Landeros, V.M.; Landa-Gómez, A.E. Mathematical Calculations for the Design of Elliptical Isolated Foundations with Optimal Cost. Mathematics 2025, 13, 1777. https://doi.org/10.3390/math13111777
Diaz-Gurrola ER, Luévanos-Rojas A, Santiago-Hurtado G, Moreno-Landeros VM, Landa-Gómez AE. Mathematical Calculations for the Design of Elliptical Isolated Foundations with Optimal Cost. Mathematics. 2025; 13(11):1777. https://doi.org/10.3390/math13111777
Chicago/Turabian StyleDiaz-Gurrola, Eyran Roberto, Arnulfo Luévanos-Rojas, Griselda Santiago-Hurtado, Victor Manuel Moreno-Landeros, and Aldo Emelio Landa-Gómez. 2025. "Mathematical Calculations for the Design of Elliptical Isolated Foundations with Optimal Cost" Mathematics 13, no. 11: 1777. https://doi.org/10.3390/math13111777
APA StyleDiaz-Gurrola, E. R., Luévanos-Rojas, A., Santiago-Hurtado, G., Moreno-Landeros, V. M., & Landa-Gómez, A. E. (2025). Mathematical Calculations for the Design of Elliptical Isolated Foundations with Optimal Cost. Mathematics, 13(11), 1777. https://doi.org/10.3390/math13111777






