Optimal Control Strategies for Dengue and Malaria Co-Infection Disease Model
Abstract
1. Introduction
2. Model of Dengue–Malaria Co-Infection
- All the theoretical results that were rigorously established and validated in the study referenced as [17] are applicable to and hold true for our analysis. This demonstrates the alignment of our work with the prior theoretical framework and ensures the robustness of our assumptions and methods.
- In this study, we consider three control strategies that vary over time, which are as follows: the application of mosquito spray, denoted as ; the practice of self-precautionary measures, represented by ; and the implementation of a treatment rate for infected individuals, denoted as . These time-dependent controls are designed to mitigate the spread of the diseases under consideration.
- It is assumed that the treatment rate is uniform and identical for all individuals infected with dengue, malaria, or a co-infection of both diseases. This simplifies the model and reflects a generalized approach to medical intervention.
- Apart from the time-dependent controls, all other parameters used in this study are considered constant. This assumption allows us to focus on the dynamic effects of the controls while maintaining a manageable complexity in the model.
3. Optimal Control of Dengue–Malaria Co-Infection
3.1. Objective Functional
3.2. Hamiltonian and Necessary Optimality Condition
4. Results and Discussion
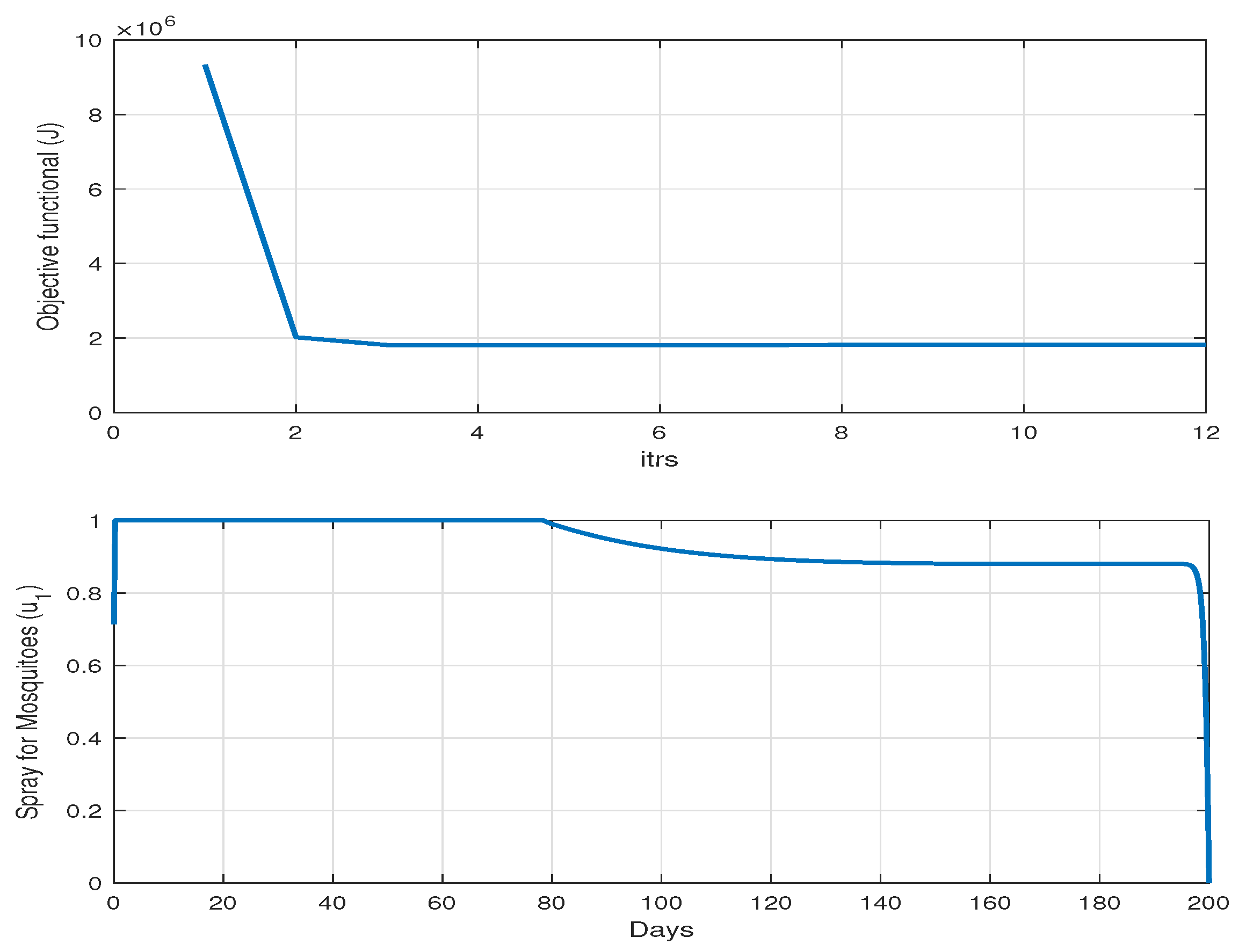
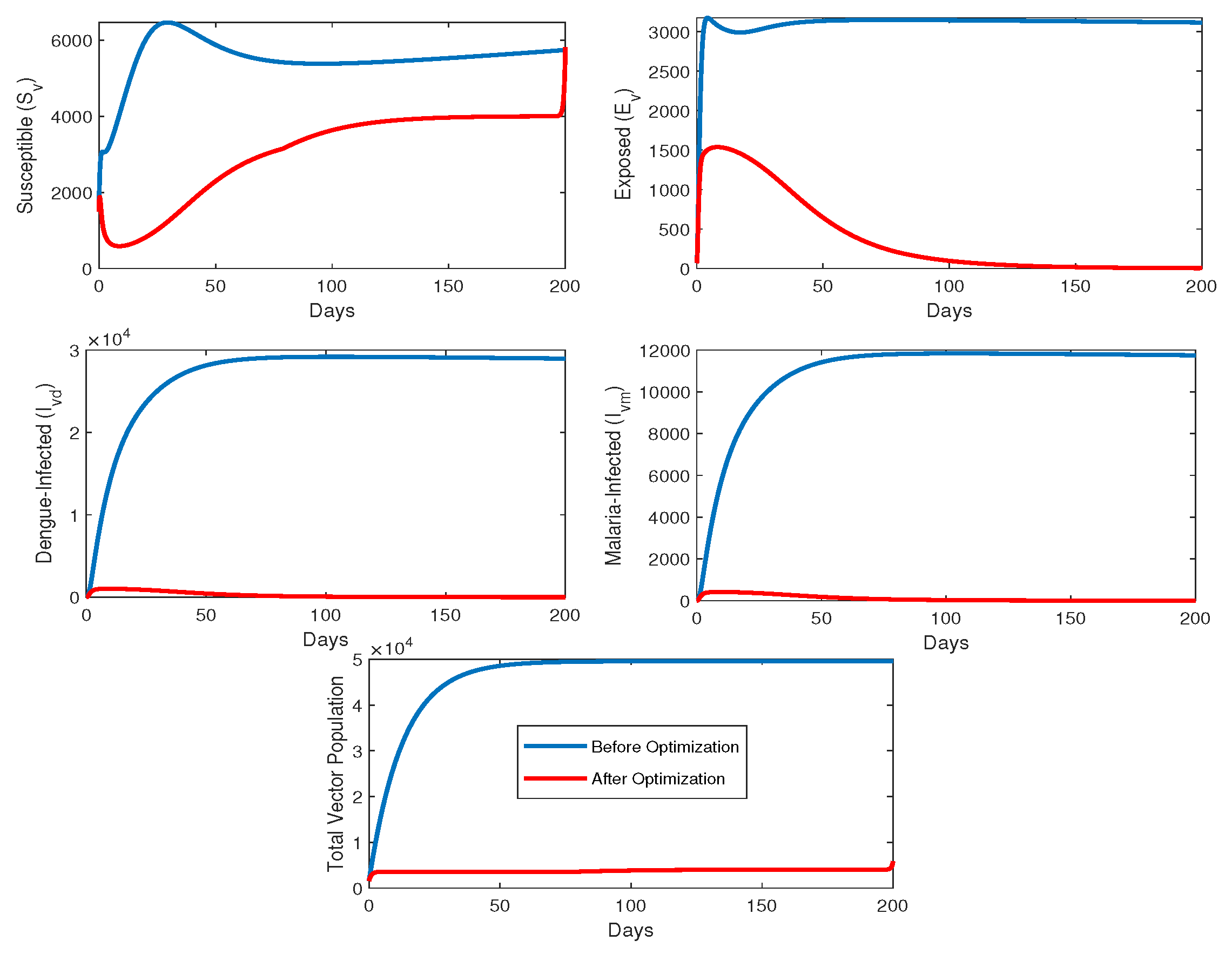
4.1. Spray for Mosquitoes, , as the Unique Control Law
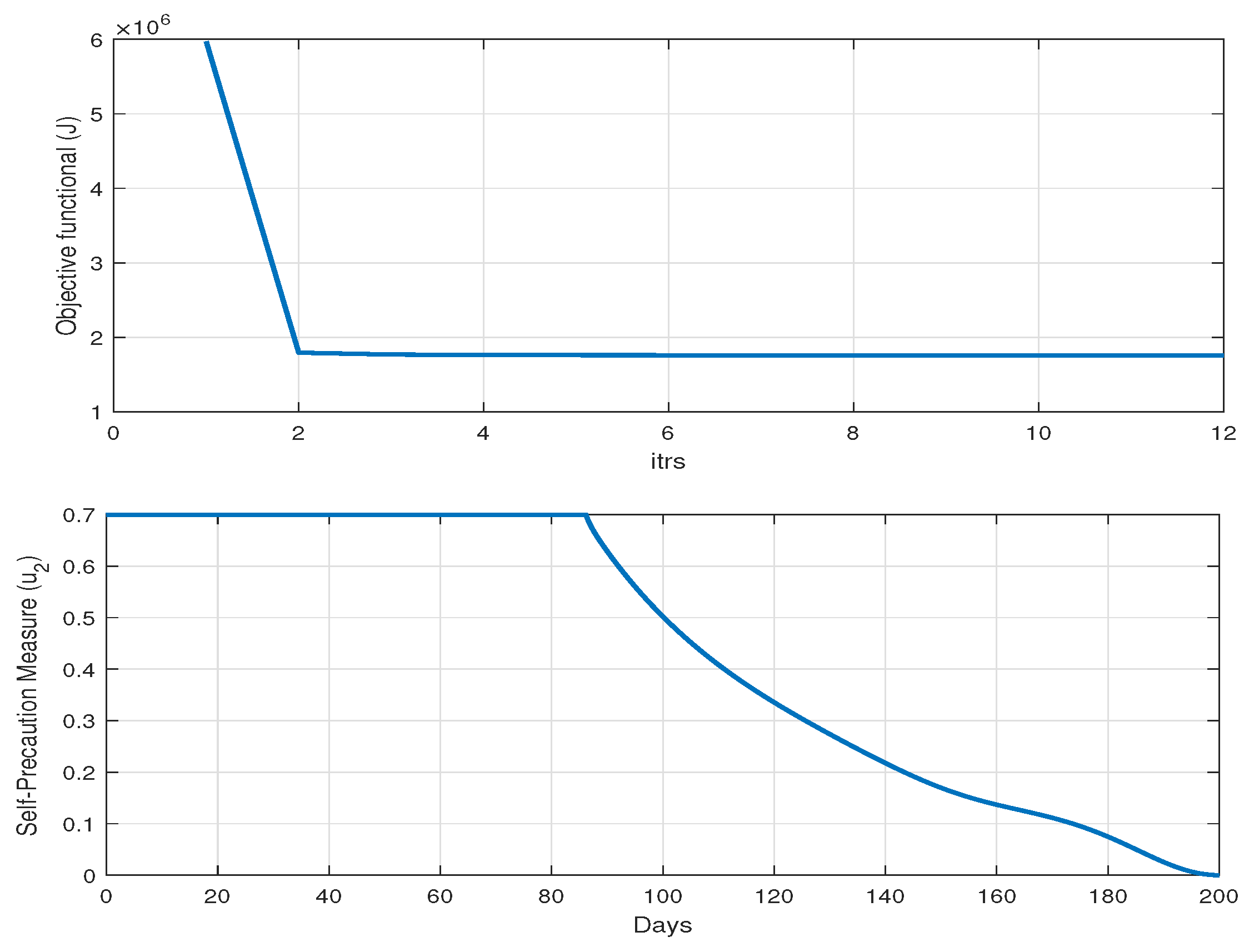
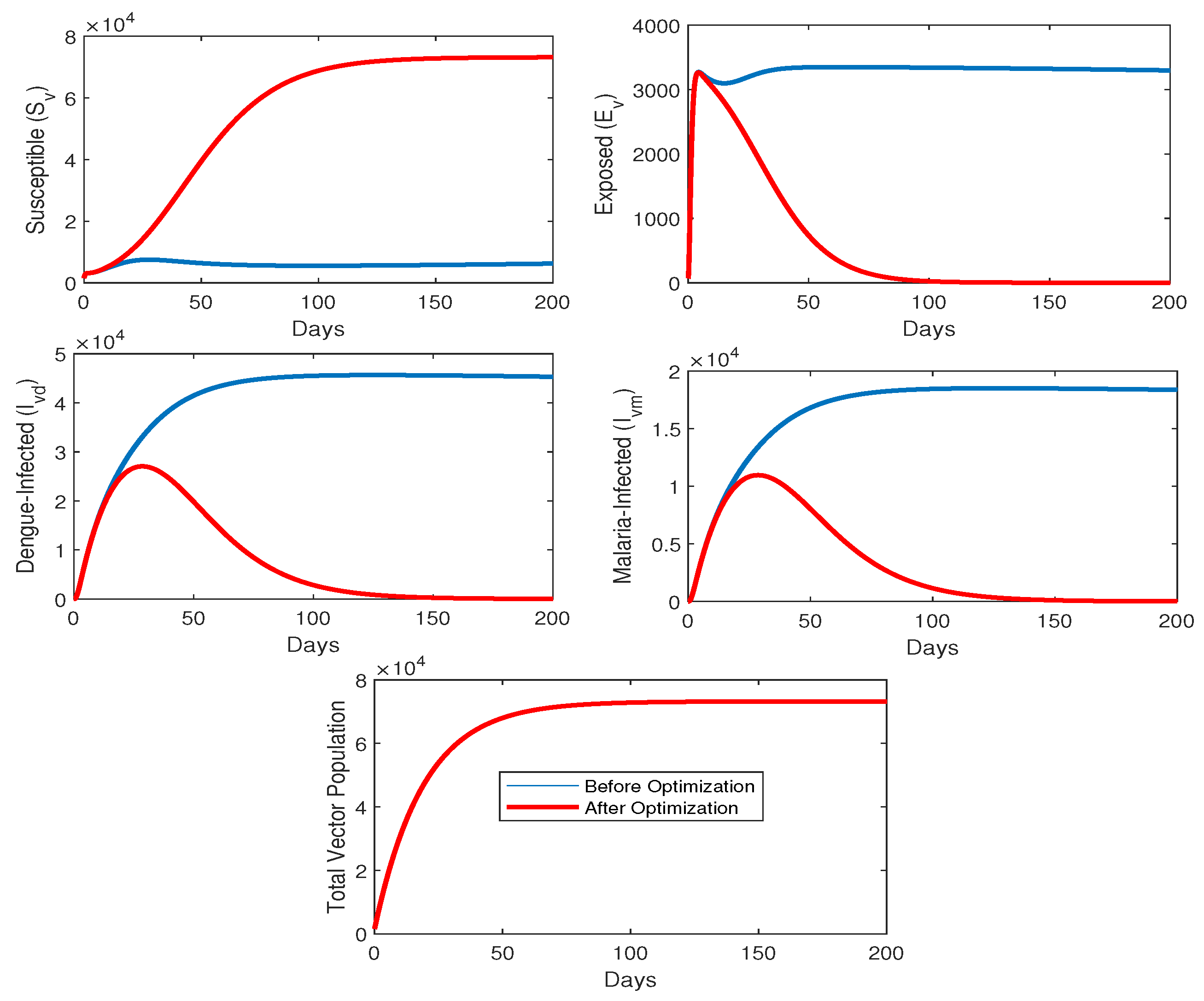
4.2. Self-Precaution Measures, , as the Unique Control Law
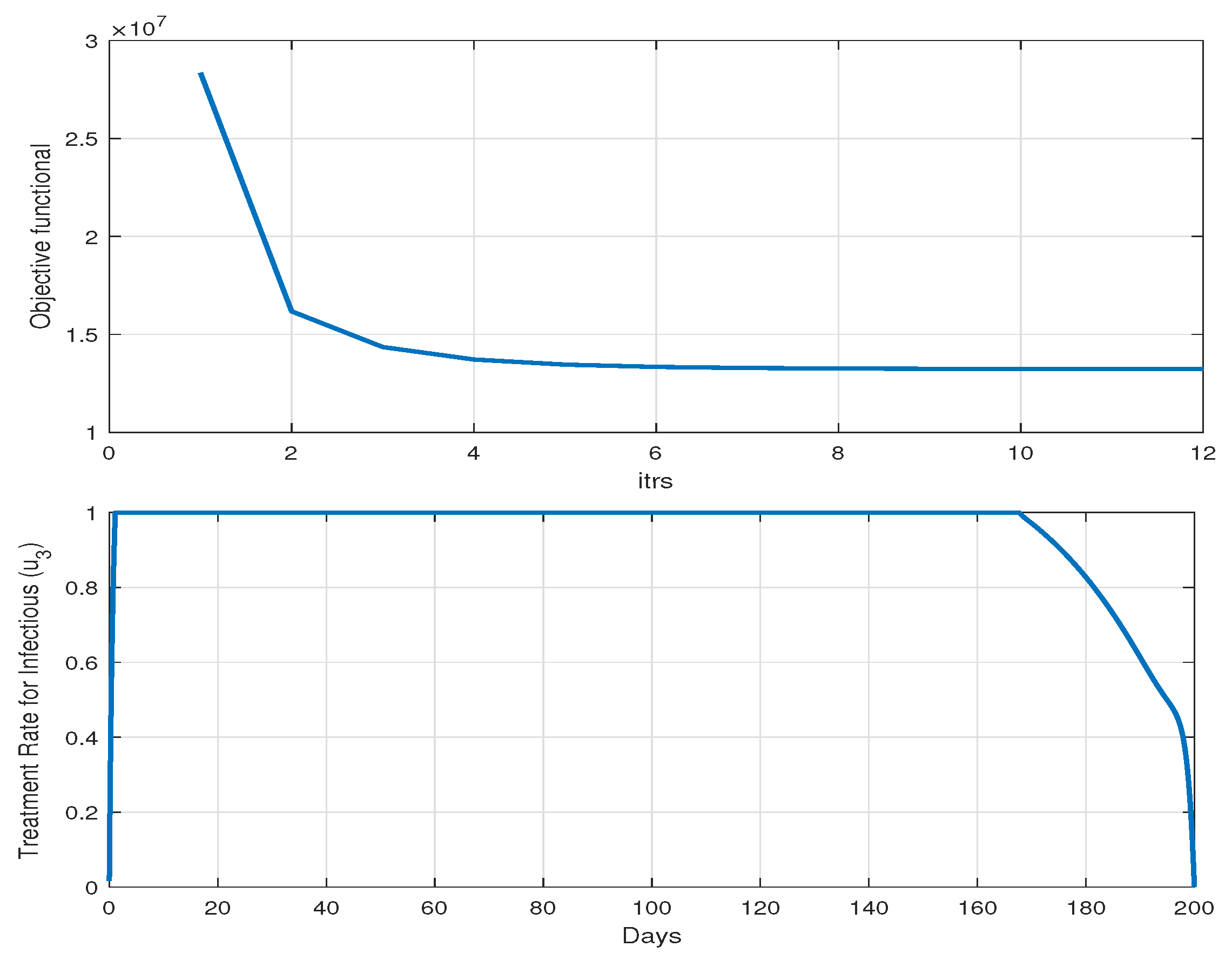
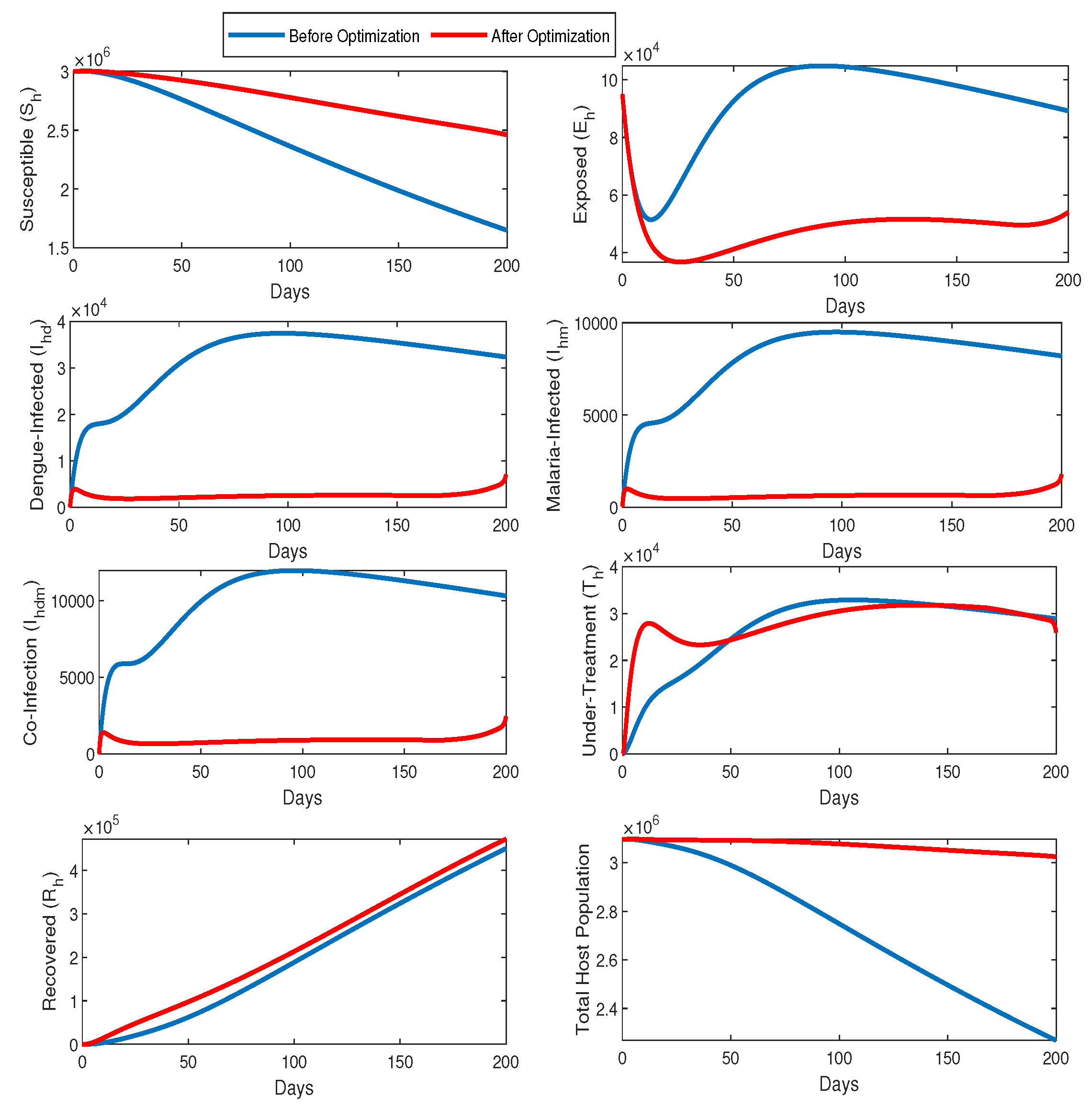
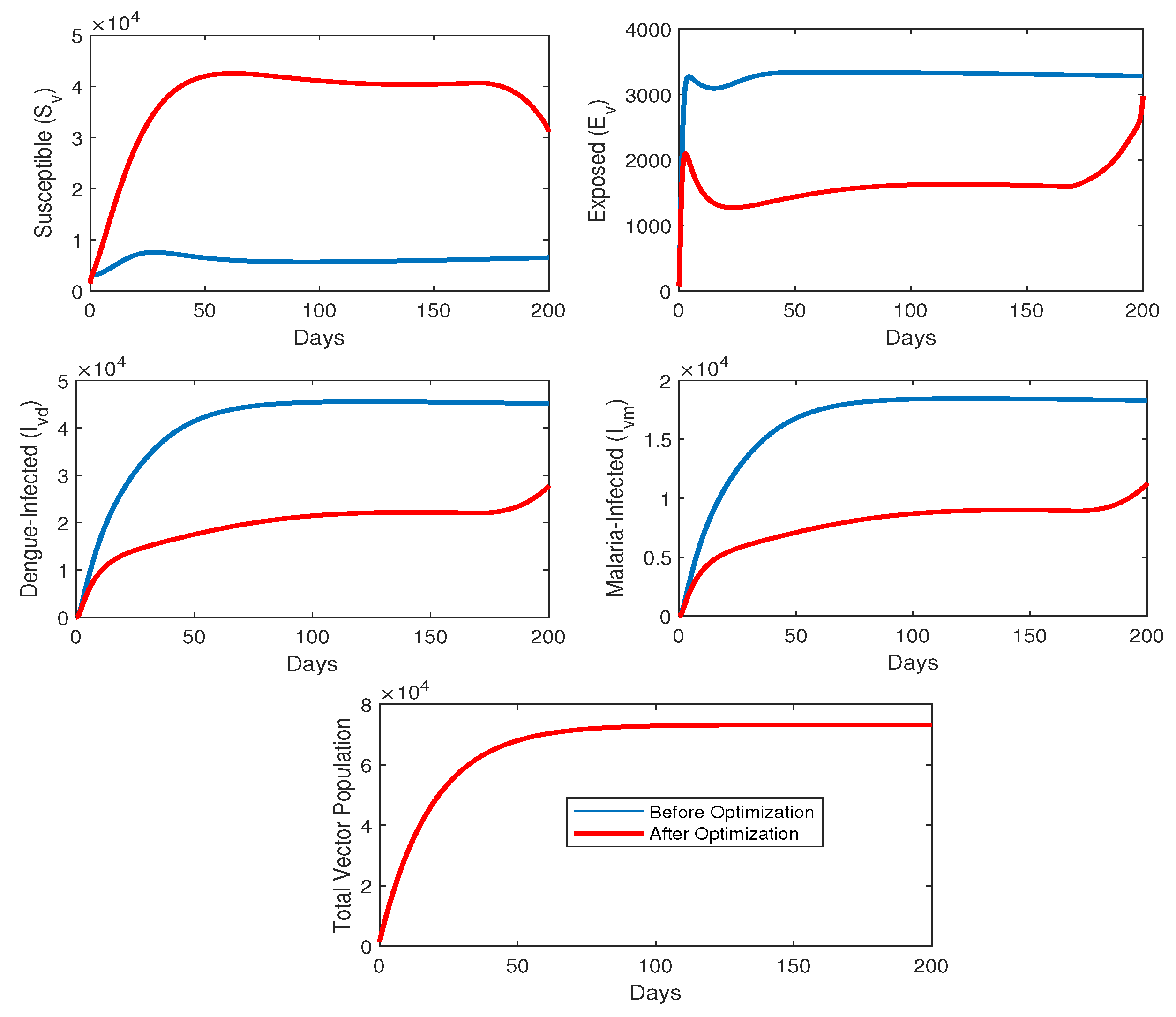
4.3. Pharmaceutical Therapy, , as the Unique Control Law
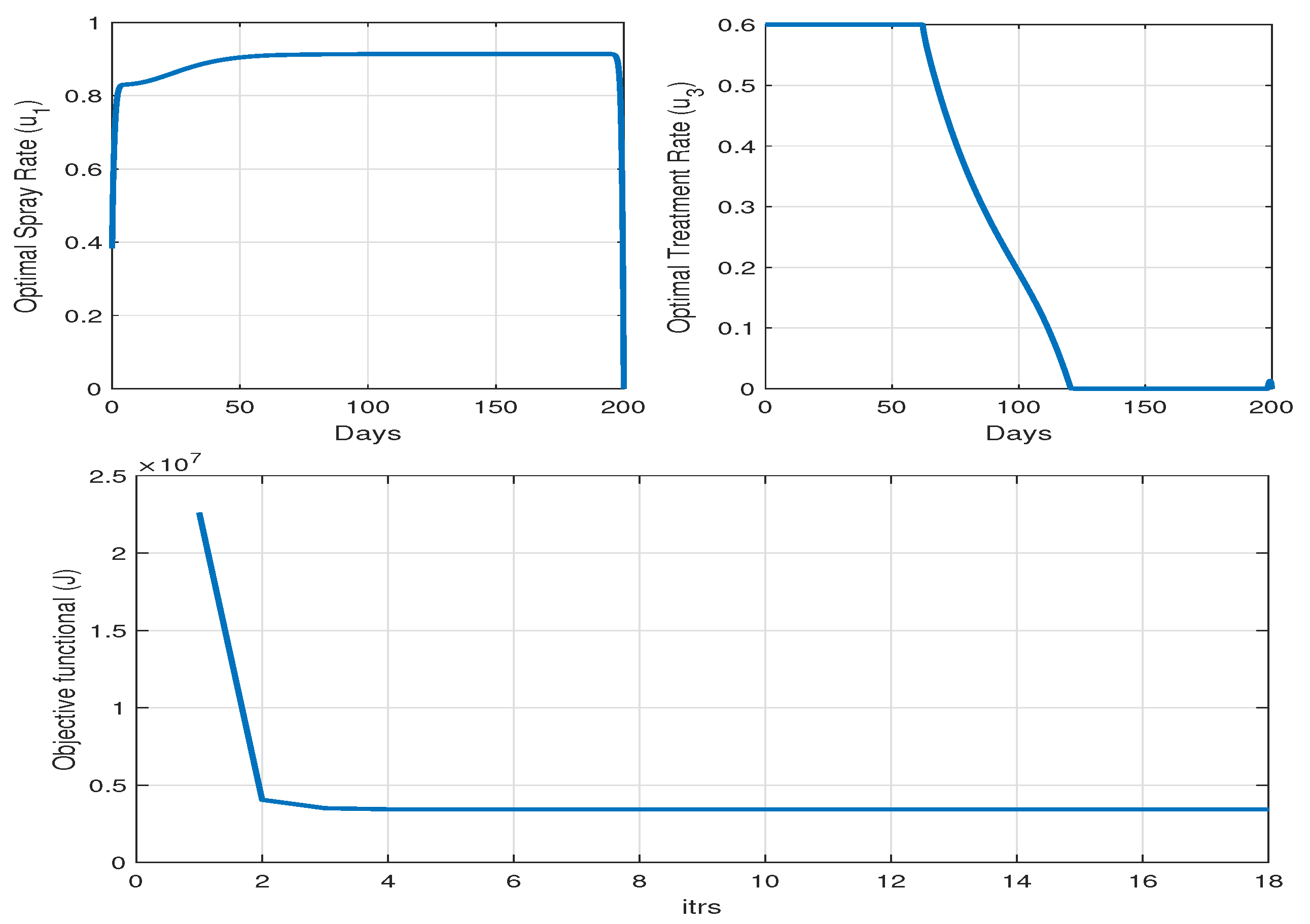
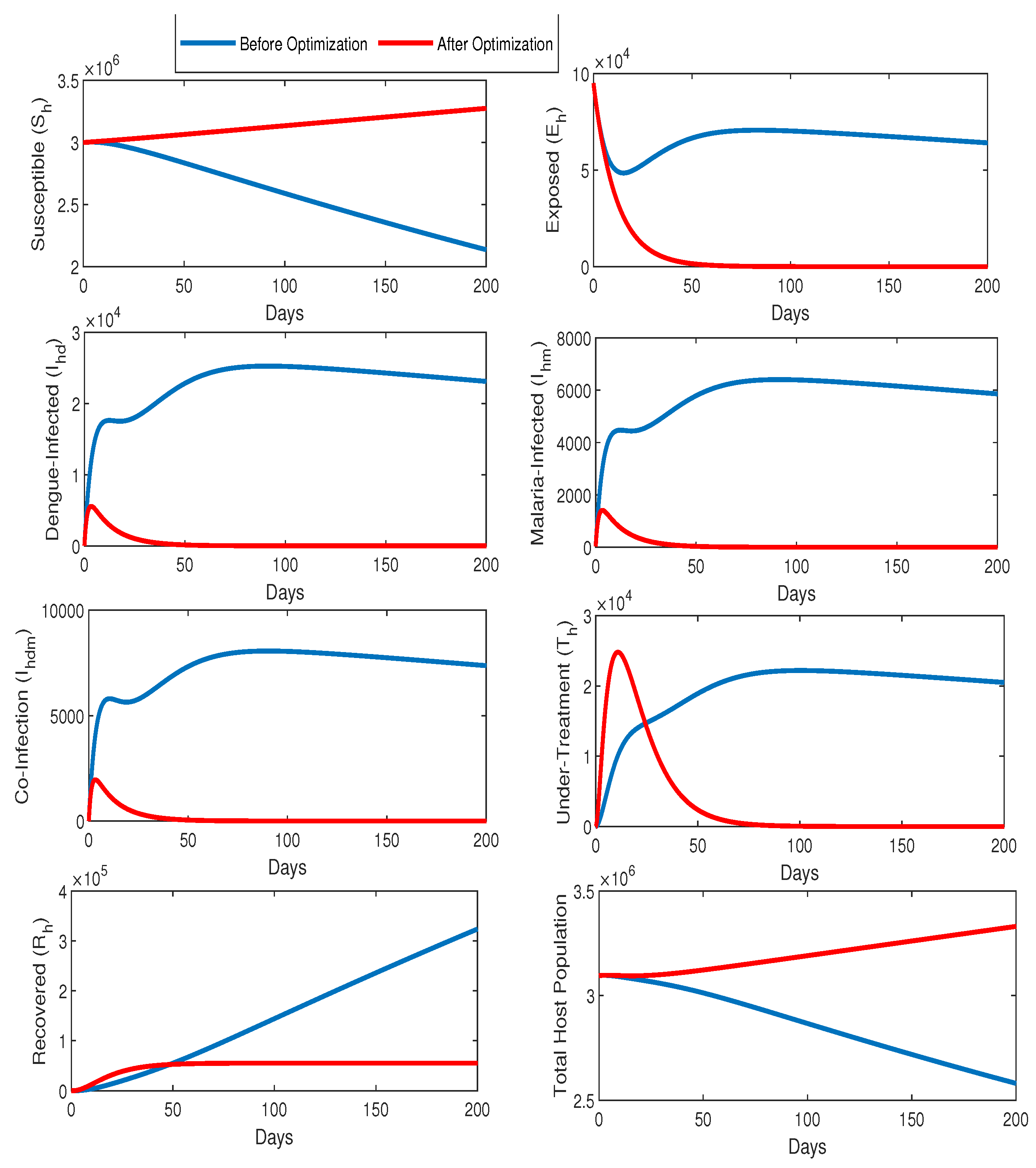
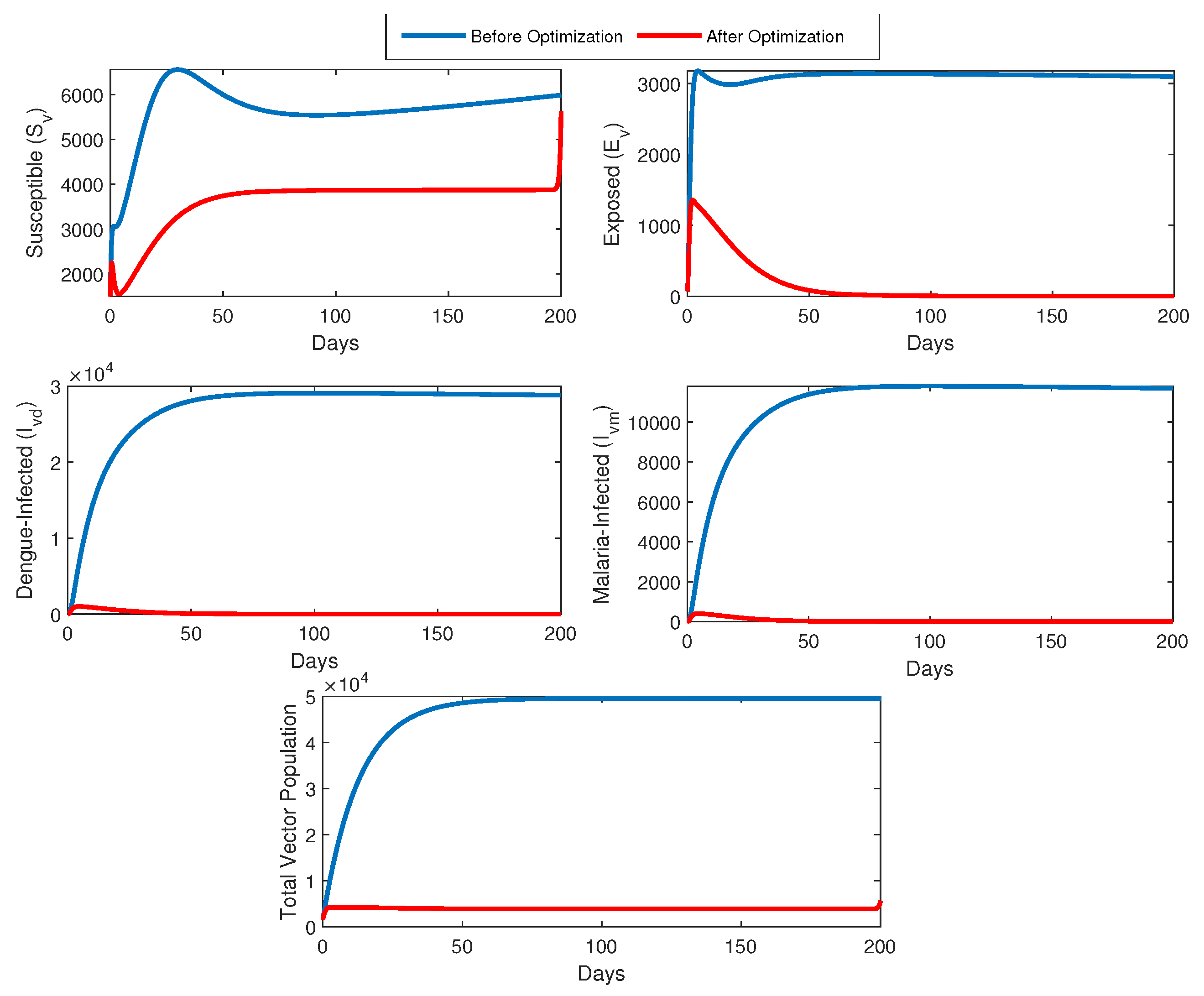
4.4. Combined Effects of Spray, , and Pharmaceutical Treatment,
5. Conclusions
Declaration of Generative AI and AI-Assisted Technologies in the Writing Process
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Khan, M.A. Dengue infection modeling and its optimal control analysis in East Java, Indonesia. Heliyon 2021, 7, e06023. [Google Scholar] [CrossRef]
- World Health Organisation. Malaria. 2021. Available online: https://www.who.int/news-room/fact-sheets/detail/malaria (accessed on 9 March 2023).
- Bonyah, E.; Khan, M.A.; Okosun, K.O.; Gomez-Aguilar, J.F. On the co-infection of dengue fever and Zika virus. Optim. Control Appl. Methods 2019, 40, 394–421. [Google Scholar] [CrossRef]
- CDC Report. Available online: https://www.cdc.gov/dengue/signs-symptoms/?CDC_AAref_Val=https://www.cdc.gov/dengue/symptoms/index.html (accessed on 20 September 2021).
- Wang, J.; Zhao, H. Threshold dynamics and regional optimal control of a malaria model with spatial heterogeneity and ivermectin therapy. Appl. Math. Model. 2024, 125, 591–624. [Google Scholar] [CrossRef]
- Wang, Y.; Chitnis, N.; Fairbanks, E.L. Optimizing malaria vector control in the Greater Mekong Subregion: A systematic review and mathematical modelling study to identify desirable intervention characteristics. Parasites Vectors 2024, 17, 162. [Google Scholar] [CrossRef] [PubMed]
- Duffy, P.E.; Gorres, J.P.; Healy, S.A.; Fried, M. Malaria vaccines: A new era of prevention and control. Nat. Rev. Microbiol. 2024, 22, 756–772. [Google Scholar] [CrossRef]
- Pongsumpun, P. Mathematical model of dengue disease with the incubation period of virus. World Acad. Sci. Eng. Technol. 2008, 44, 328–332. [Google Scholar]
- Adom-Konadu, A.; Yankson, E.; Naandam, S.M.; Dwomoh, D. A Mathematical Model for Effective Control and Possible Eradication of Malaria. Hindawi J. Math. 2022, 2022, 6165581. [Google Scholar] [CrossRef]
- Traor, B.; Sangar, B.; Traor, S. A Mathematical Model of Malaria Transmission with Structured Vector Population and Seasonality. Hindawi J. Appl. Math. 2017, 2017, 6754097. [Google Scholar] [CrossRef]
- Al Basir, F.; Abraha, T. Mathematical Modelling and Optimal Control of Malaria Using Awareness-Based Interventions. Mathematics 2023, 11, 1687. [Google Scholar] [CrossRef]
- Selvaretnam, A.A.; Sahu, P.S.; Sahu, M.; Ambu, S. A review of concurrent infections of malaria and dengue in Asia. Asian Pac. J. Trop. 2016, 6, 633–638. [Google Scholar] [CrossRef]
- Wiwanitkit, V. Concurrent malaria and dengue infection: A brief summary and comment. Asian Pac. J. Trop. Biomed. 2011, 1, 326–327. [Google Scholar] [CrossRef] [PubMed]
- Centers for Disease Control and Prevention. Biology. Atlanta: Centers for Disease Control and Prevention. 2015. Available online: https://www.cdc.gov/nchs/data/hus/hus15.pdf (accessed on 26 February 2016).
- Gautam, A.; Aryal, U.; Bhari, S.; Pradhan, S.; Bhattarai, U.; Mishra, A.; Sharma, S.K. Dengue and malaria coinfection: The first case report in Nepal. Oxf. Med. Case Rep. 2022, 2022, omac022. [Google Scholar] [CrossRef] [PubMed] [PubMed Central]
- Sheoran, N.; Satia, M.H. Stability Analysis of Co-Infection of Malaria-Dengue. In Mathematical Models of Infectious Diseases and Social Issues; IGI Global: Hershey, PA, USA, 2020. [Google Scholar] [CrossRef]
- Butt, A.I.K.; Imran, M.; McKinney, B.A.; Batool, S.; Aftab, H. Mathematical and Stability Analysis of Dengue-Malaria Co-Infection with Disease Control Strategies. Mathematics 2023, 11, 4600. [Google Scholar] [CrossRef]
- Hanif, A.; Butt, A.I.K.; Ismaeel, T. Fractional optimal control analysis of Covid-19 and dengue fever co-infection model with Atangana-Baleanu derivative. AIMS Math. 2024, 9, 5171–5203. [Google Scholar] [CrossRef]
- Tchoumi, S.Y.; Diagne, M.L.; Rwezaura, H.; Tchuenche, J.M. Malaria and COVID-19 co-dynamics: A mathematical model and optimal control. Appl. Math. Model. 2021, 99, 294–327. [Google Scholar] [CrossRef] [PubMed]
- Butt, A.I.K.; Chamaleen, D.B.D.; Batool, S.; Nuwairan, M.A. A new design and analysis of optimal control problems arising from COVID-19 outbreak. Math. Meth. Appl. Sci. 2023, 46, 16957–16982. [Google Scholar] [CrossRef]
- Abidemi, A.; Peter, O.J. An optimal control model for dengue dynamics with asymptomatic, isolation, and vigilant compartments. Decis. Anal. J. 2024, 10, 100413. [Google Scholar] [CrossRef]
- Butt, A.I.K.; Imran, M.; Aslam, J.; Batool, S. Computational analysis of control of hepatitis B virus disease through vaccination and treatment strategies. PLoS ONE 2023, 18, e0288024. [Google Scholar] [CrossRef] [PubMed]
- Borri, A.; Palumbo, P.; Papa, F.; Possieri, C. Optimal design of lock-down and reopening policies for early-stage epidemics through SIR-D model. Annu. Rev. Control 2021, 51, 511–524. [Google Scholar] [CrossRef]
- Borri, A.; Palumbo, P.; Papa, F.; Possieri, C. Optimizing restrictions in epidemics via piecewise time-varying SIRD models: Application to the COVID-19 Italian emergency. Eur. J. Control 2024, 75, 100902. [Google Scholar] [CrossRef]
- Pontryagin, L.S.; Boltyanskii, V.G. The Mathematical Theory of Optimal Processes; Golden and Breach Science Publishers: New York, NY, USA, 1986. [Google Scholar]
- Lenhart, S.; Workman, J.T. Optimal Control Applied to Biological Models; Chapman & Hall/CRC: Boca Raton, FL, USA, 2007. [Google Scholar]
- Abdulrashid, I.; Ghazzai, H.; Han, X.; Massoud, Y. Optimal control treatment analysis for the predator-prey chemotherapy model. In Proceedings of the 2019 31st International Conference on Microelectronics (ICM), Cairo, Egypt, 15–18 December 2019; pp. 296–299. [Google Scholar]
- Ren, Y.; Xue, Y. Modeling and optimal control of COVID-19 and malaria co-infection based on vaccination. Math. Model. Control 2024, 4, 316–335. [Google Scholar] [CrossRef]
- ELmojtaba, I.M.; Al-Maqrashi, K.; Al-Musalhi, F.; Al-Salti, N. Optimal control and cost effectiveness analysis of a Zika-Malaria co-infection model. Partial. Differ. Eq. Appl. Math. 2024, 11, 100754. [Google Scholar] [CrossRef]
- Boulaaras, S.; Yavuz, M.; Alrashedi, Y.; Bahram, S.; Jan, R. Modeling the co-dynamics of vector-borne infections with the application of optimal control theory. Discret. Contin. Dyn. Syst. 2024. [Google Scholar] [CrossRef]


| Parameter | Description | Value | Source |
|---|---|---|---|
| Recruitment rate of mosquito | day−1 | [1,17] | |
| Natural death rate of mosquito | day−1 | [17] | |
| Interaction rate of and | day−1 | [1,17] | |
| Interaction rate of and | day−1 | [9,17] | |
| Interaction rate of and | day−1 | [17] | |
| Translation from to | day−1 | [1,17] | |
| Translation from to | day−1 | [9,17] | |
| Recruitment rate of humans | day−1 | [1,17] | |
| Natural death rate of humans | day−1 | [1] | |
| Interaction rate of and | day−1 | [1,17] | |
| Interaction rate of and | day−1 | [9,17] | |
| Translation from to | day−1 | [1,17] | |
| Translation from to | day−1 | [9,17] | |
| Translation from to | day−1 | [17] | |
| Recovery rate of | day−1 | [1,17] | |
| Dengue induced mortality of | day−1 | [17] | |
| Malaria induced mortality of | day−1 | [17] | |
| Dengue–malaria induced mortality of | day−1 | [17] | |
| Disease induced mortality of | day−1 | [17] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Imran, M.; McKinney, B.A.; Butt, A.I.K.; Palumbo, P.; Batool, S.; Aftab, H. Optimal Control Strategies for Dengue and Malaria Co-Infection Disease Model. Mathematics 2025, 13, 43. https://doi.org/10.3390/math13010043
Imran M, McKinney BA, Butt AIK, Palumbo P, Batool S, Aftab H. Optimal Control Strategies for Dengue and Malaria Co-Infection Disease Model. Mathematics. 2025; 13(1):43. https://doi.org/10.3390/math13010043
Chicago/Turabian StyleImran, Muhammad, Brett Allen McKinney, Azhar Iqbal Kashif Butt, Pasquale Palumbo, Saira Batool, and Hassan Aftab. 2025. "Optimal Control Strategies for Dengue and Malaria Co-Infection Disease Model" Mathematics 13, no. 1: 43. https://doi.org/10.3390/math13010043
APA StyleImran, M., McKinney, B. A., Butt, A. I. K., Palumbo, P., Batool, S., & Aftab, H. (2025). Optimal Control Strategies for Dengue and Malaria Co-Infection Disease Model. Mathematics, 13(1), 43. https://doi.org/10.3390/math13010043







