Solutions to the Schrödinger Equation: Nonlocal Terms and Geometric Constraints
Abstract
1. Introduction
2. Schrödinger Equation
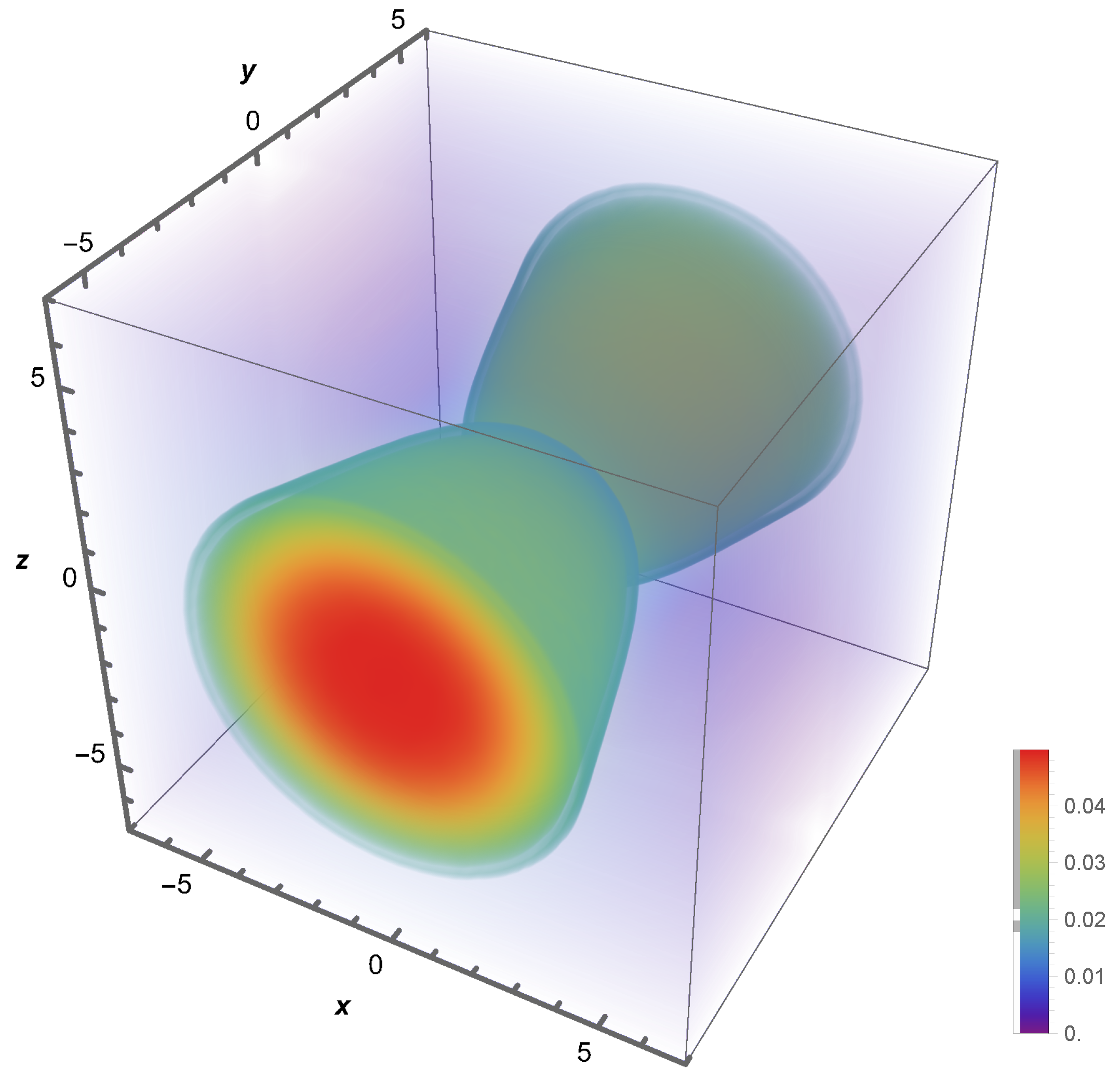
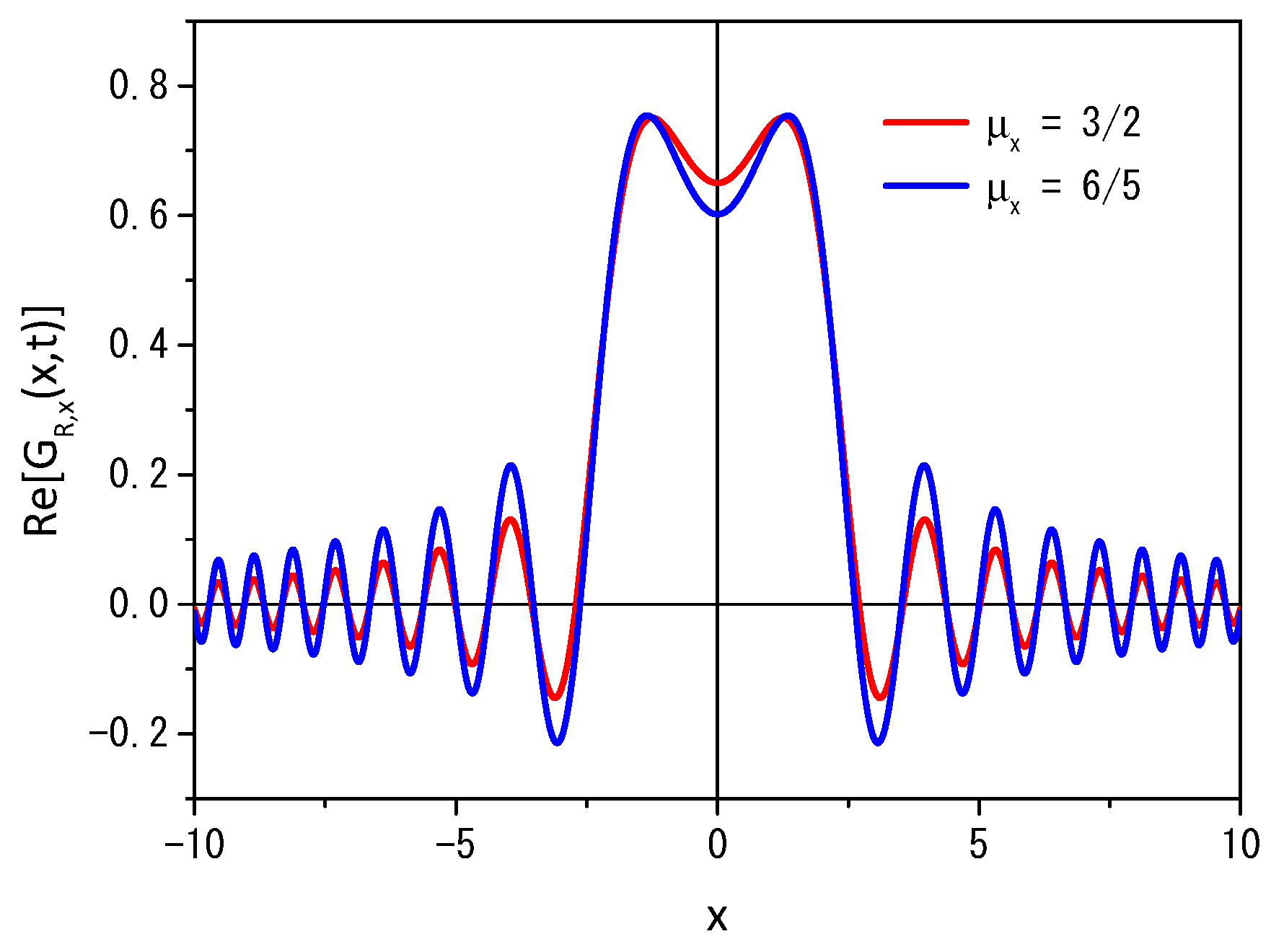
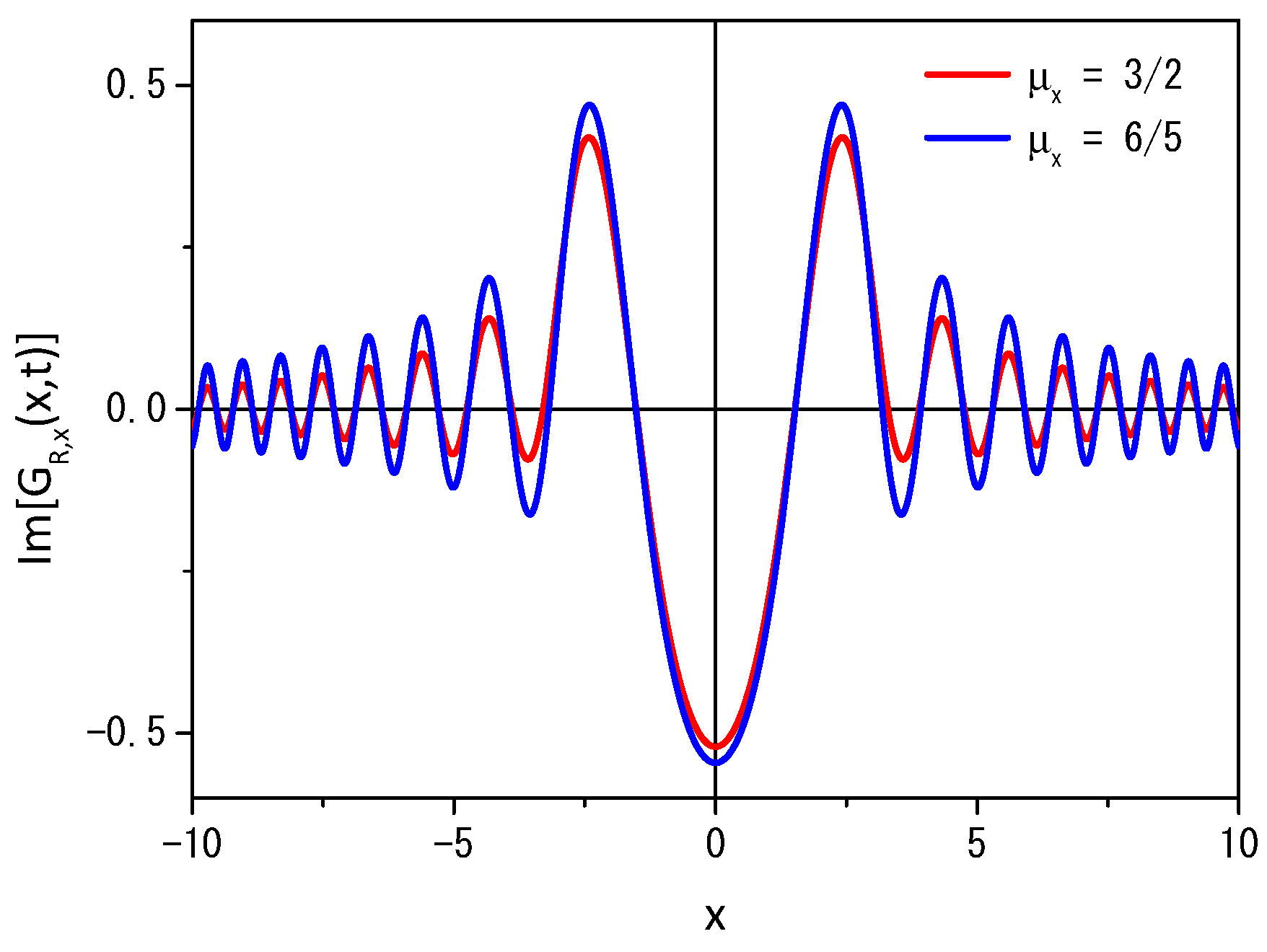
3. Reduced Green Functions
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Schneider, W.R.; Wyss, W. Fractional diffusion and wave equations. J. Math. Phys. 1989, 30, 134–144. [Google Scholar] [CrossRef]
- Metzler, R.; Klafter, J. The random walk’s guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2000, 339, 1–77. [Google Scholar] [CrossRef]
- Sokolov, I.M. Models of anomalous diffusion in crowded environments. Soft Matter 2012, 8, 9043–9052. [Google Scholar] [CrossRef]
- Chechkin, A.V.; Gorenflo, R.; Sokolov, I.M. Fractional Fokker-Planck equation for ultraslow anomalous diffusion. Phys. Rev. E 2002, 66, 046129. [Google Scholar] [CrossRef] [PubMed]
- Barkai, E. Fractional Fokker-Planck equation, solution, and application. Phys. Rev. E 2001, 63, 046118. [Google Scholar] [CrossRef]
- Gorenflo, R.; Mainardi, F.; Vivoli, A. Continuous-time random walk and parametric subordination in fractional diffusion. Chaos Solitons Fractals 2007, 34, 87–103. [Google Scholar] [CrossRef]
- Hilfer, R. Applications of Fractional Calculus in Physics; World Scientific: Singapore, 2000. [Google Scholar]
- Gorenflo, R.; Mainardi, F. Random walk models for space-fractional diffusion processes. Fract. Calc. Appl. Anal. 1998, 1, 167–191. [Google Scholar]
- Compte, A. Stochastic foundations of fractional dynamics. Phys. Rev. E 1996, 53, 4191. [Google Scholar] [CrossRef]
- Bazhlekova, E.; Bazhlekov, I. Subordination approach to space-time fractional diffusion. Mathematics 2019, 7, 415. [Google Scholar] [CrossRef]
- Sabzikar, F.; Meerschaert, M.M.; Chen, J. Tempered fractional calculus. J. Comput. Phys. 2015, 293, 14–28. [Google Scholar] [CrossRef] [PubMed]
- Tarasov, V.E. Generalized memory: Fractional calculus approach. Fractal Fract. 2018, 2, 23. [Google Scholar] [CrossRef]
- Tatom, F.B. The relationship between fractional calculus and fractals. Fractals 1995, 3, 217–229. [Google Scholar] [CrossRef]
- Magin, R. Fractional calculus in bioengineering, part 1. Crit. Rev. Biomed. Eng. 2004, 32. [Google Scholar] [CrossRef]
- Sibatov, R.T. Fractal generalization of the Scher–Montroll model for anomalous transit-time dispersion in disordered solids. Mathematics 2020, 8, 1991. [Google Scholar] [CrossRef]
- Iomin, A.; Méndez, V.; Horsthemke, W. Fractional Dynamics in Comb-like Structures; World Scientific: Singapore, 2018. [Google Scholar]
- Iomin, A.; Méndez, V.; Horsthemke, W. Comb model: Non-Markovian versus Markovian. Fractal Fract. 2019, 3, 54. [Google Scholar] [CrossRef]
- Iomin, A. Non-Markovian quantum mechanics on comb. Chaos Interdiscip. J. Nonlinear Sci. 2024, 34, 093135. [Google Scholar] [CrossRef]
- Petreska, I.; de Castro, A.S.M.; Sandev, T.; Lenzi, E.K. The time-dependent Schrödinger equation in three dimensions under geometric constraints. J. Math. Phys. 2019, 60, 032101. [Google Scholar] [CrossRef]
- Sokolov, I.M.; Klafter, J. From diffusion to anomalous diffusion: A century after Einstein’s Brownian motion. Chaos Interdiscip. J. Nonlinear Sci. 2005, 15, 026103. [Google Scholar] [CrossRef] [PubMed]
- Kochubei, A.N. General fractional calculus, evolution equations, and renewal processes. Integral Equ. Oper. Theory 2011, 71, 583–600. [Google Scholar] [CrossRef]
- Sandev, T.; Petreska, I.; Lenzi, E.K. Time-dependent Schrödinger-like equation with nonlocal term. J. Math. Phys. 2014, 55, 092105. [Google Scholar] [CrossRef]
- Luchko, Y.; Yamamoto, M. The general fractional derivative and related fractional differential equations. Mathematics 2020, 8, 2115. [Google Scholar] [CrossRef]
- Luchko, Y. Fractional differential equations with the general fractional derivatives of arbitrary order in the Riemann–Liouville sense. Mathematics 2022, 10, 849. [Google Scholar] [CrossRef]
- Laskin, N. Fractional Quantum Mechanics; World Scientific Publishing Company: Singapore, 2018. [Google Scholar]
- Laskin, N. Fractional Schrödinger equation. Phys. Rev. E 2002, 66, 056108. [Google Scholar] [CrossRef] [PubMed]
- Luchko, Y. Fractional Schrödinger equation for a particle moving in a potential well. J. Math. Phys. 2013, 54. [Google Scholar] [CrossRef]
- Liemert, A.; Kienle, A. Fractional Schrödinger equation in the presence of the linear potential. Mathematics 2016, 4, 31. [Google Scholar] [CrossRef]
- Sibatov, R.T.; Sun, H. Tempered fractional equations for quantum transport in mesoscopic one-dimensional systems with fractal disorder. Fractal Fract. 2019, 3, 47. [Google Scholar] [CrossRef]
- Liu, S.; Xu, X.; Xie, R.; Zhang, G.; Li, B. Anomalous heat conduction and anomalous diffusion in low dimensional nanoscale systems. Eur. Phys. J. B 2012, 85, 337. [Google Scholar] [CrossRef]
- Livi, R. Anomalous transport in low-dimensional systems: A pedagogical overview. Phys. A Stat. Mech. Its Appl. 2023, 631, 127779. [Google Scholar] [CrossRef]
- Afek, G.; Davidson, N.; Kessler, D.A.; Barkai, E. Colloquium: Anomalous statistics of laser-cooled atoms in dissipative optical lattices. Rev. Mod. Phys. 2023, 95, 031003. [Google Scholar] [CrossRef]
- Marcuzzi, M.; Levi, E.; Li, W.; Garrahan, J.P.; Olmos, B.; Lesanovsky, I. Non-equilibrium universality in the dynamics of dissipative cold atomic gases. New J. Phys. 2015, 17, 072003. [Google Scholar] [CrossRef]
- Ponomarev, A.V.; Denisov, S.; Hänggi, P. Lévy distribution in many-particle quantum systems. Phys. Rev. A—At. Mol. Opt. Phys. 2010, 81, 043615. [Google Scholar] [CrossRef]
- Lenzi, E.K.; de Oliveira, B.F.; da Silva, L.R.; Evangelista, L.R. Solutions for a Schrödinger equation with a nonlocal term. J. Math. Phys. 2008, 49, 032108. [Google Scholar] [CrossRef]
- Lenzi, E.K.; Evangelista, L.R.; Zola, R.S.; Petreska, I.; Sandev, T. Fractional Schrödinger equation and anomalous relaxation: Nonlocal terms and delta potentials. Mod. Phys. Lett. A 2021, 36, 2140004. [Google Scholar] [CrossRef]
- Wang, L.; Jin, F.F. Boundary output feedback stabilization of the linearized Schrödinger equation with nonlocal term. Int. J. Control. Autom. Syst. 2021, 19, 1528–1538. [Google Scholar] [CrossRef]
- Jiang, X.; Qi, H.; Xu, M. Exact solutions of fractional Schrödinger-like equation with a nonlocal term. J. Math. Phys. 2011, 52, 042105. [Google Scholar] [CrossRef]
- Jeng, M.; Xu, S.L.Y.; Hawkins, E.; Schwarz, J. On the nonlocality of the fractional Schrödinger equation. J. Math. Phys. 2010, 51, 062102. [Google Scholar] [CrossRef]
- Modanese, G. Time in quantum mechanics and the local non-conservation of the probability current. Mathematics 2018, 6, 155. [Google Scholar] [CrossRef]
- Petreska, I.; Sandev, T.; Lenzi, E.K. Comb-like geometric constraints leading to emergence of the time-fractional Schrödinger equation. Mod. Phys. Lett. A 2021, 36, 2130005. [Google Scholar] [CrossRef]
- Duffy, D. Green’s Functions with Applications; Applied Mathematics; CRC Press: Boca Raton, FL, USA, 2001. [Google Scholar]
- Mathai, A.M.; Saxena, R.K.; Haubold, H.J. The H-function: Theory and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Mathai, A.; Haubold, H.J. Mittag-Leffler Functions and Fractional Calculus; Springer: Berlin/Heidelberg, Germany, 2008; pp. 79–134. [Google Scholar]
- Uchaikin, V.V.; Sibatov, R.T. Fractional Kinetics in Solids: Anomalous Charge Transport in Semiconductors, Dielectrics and Nanosystems; World Scientific: Singapore, 2012. [Google Scholar]
- Gorenflo, R.; Kilbas, A.A.; Mainardi, F.; Rogosin, S.V. Mittag-Leffler Functions, Related Topics and Applications; Springer: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Wang, W.; Barkai, E. Fractional advection diffusion asymmetry equation, derivation, solution and application. J. Phys. A Math. Theor. 2024, 57, 035203. [Google Scholar] [CrossRef]
- Jiang, D.; Hong, Y.; Wang, W. Simulation of the continuous time random walk using subordination schemes. Phys. Rev. E 2024, 110, 034113. [Google Scholar] [CrossRef] [PubMed]
- Suleiman, K.; Zhang, X.; Wang, E.; Liu, S.; Zheng, L. Anomalous diffusion in branched elliptical structure. Chin. Phys. B 2023, 32, 010202. [Google Scholar] [CrossRef]
- Carvalho, A.; Trevisanutto, P.E.; Taioli, S.; Neto, A.C. Computational methods for 2D materials modelling. Rep. Prog. Phys. 2021, 84, 106501. [Google Scholar] [CrossRef] [PubMed]
- Rudenko, A.N.; Katsnelson, M.I. Anisotropic effects in two-dimensional materials. 2D Mater. 2024, 11, 042002. [Google Scholar] [CrossRef]
- Shen, H.; Chen, L.; Ferrari, L.; Lin, M.H.; Mortensen, N.A.; Gwo, S.; Liu, Z. Optical observation of plasmonic nonlocal effects in a 2D superlattice of ultrasmall gold nanoparticles. Nano Lett. 2017, 17, 2234–2239. [Google Scholar] [CrossRef] [PubMed]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Petreska, I.; Trajanovski, P.; Sandev, T.; Rocha, J.A.M.A.; de Castro, A.S.M.; Lenzi, E.K. Solutions to the Schrödinger Equation: Nonlocal Terms and Geometric Constraints. Mathematics 2025, 13, 137. https://doi.org/10.3390/math13010137
Petreska I, Trajanovski P, Sandev T, Rocha JAMA, de Castro ASM, Lenzi EK. Solutions to the Schrödinger Equation: Nonlocal Terms and Geometric Constraints. Mathematics. 2025; 13(1):137. https://doi.org/10.3390/math13010137
Chicago/Turabian StylePetreska, Irina, Pece Trajanovski, Trifce Sandev, Jonathan A. M. Almeida Rocha, Antonio Sérgio Magalhães de Castro, and Ervin K. Lenzi. 2025. "Solutions to the Schrödinger Equation: Nonlocal Terms and Geometric Constraints" Mathematics 13, no. 1: 137. https://doi.org/10.3390/math13010137
APA StylePetreska, I., Trajanovski, P., Sandev, T., Rocha, J. A. M. A., de Castro, A. S. M., & Lenzi, E. K. (2025). Solutions to the Schrödinger Equation: Nonlocal Terms and Geometric Constraints. Mathematics, 13(1), 137. https://doi.org/10.3390/math13010137







