A Sustainable Supply Chain Model with Low Carbon Emissions for Deteriorating Imperfect-Quality Items under Learning Fuzzy Theory
Abstract
1. Introduction
1.1. Literature Study Based on EOQ, Carbon Emissions, and Supply Chain Management (SCM)
1.2. Literature Study Regarding Imperfect-Quality-Based Inventory Model
1.3. Literature Study Regarding Imperfect-Quality-and Carbon-Emissions-Based Inventory Model
1.4. Literature Study Regarding Fuzzy-Environment-and Learning-Concept-Based Inventory Models
1.5. Research Gap and Proposed Study
1.6. Our Contribution and Novelty of the Proposed Model
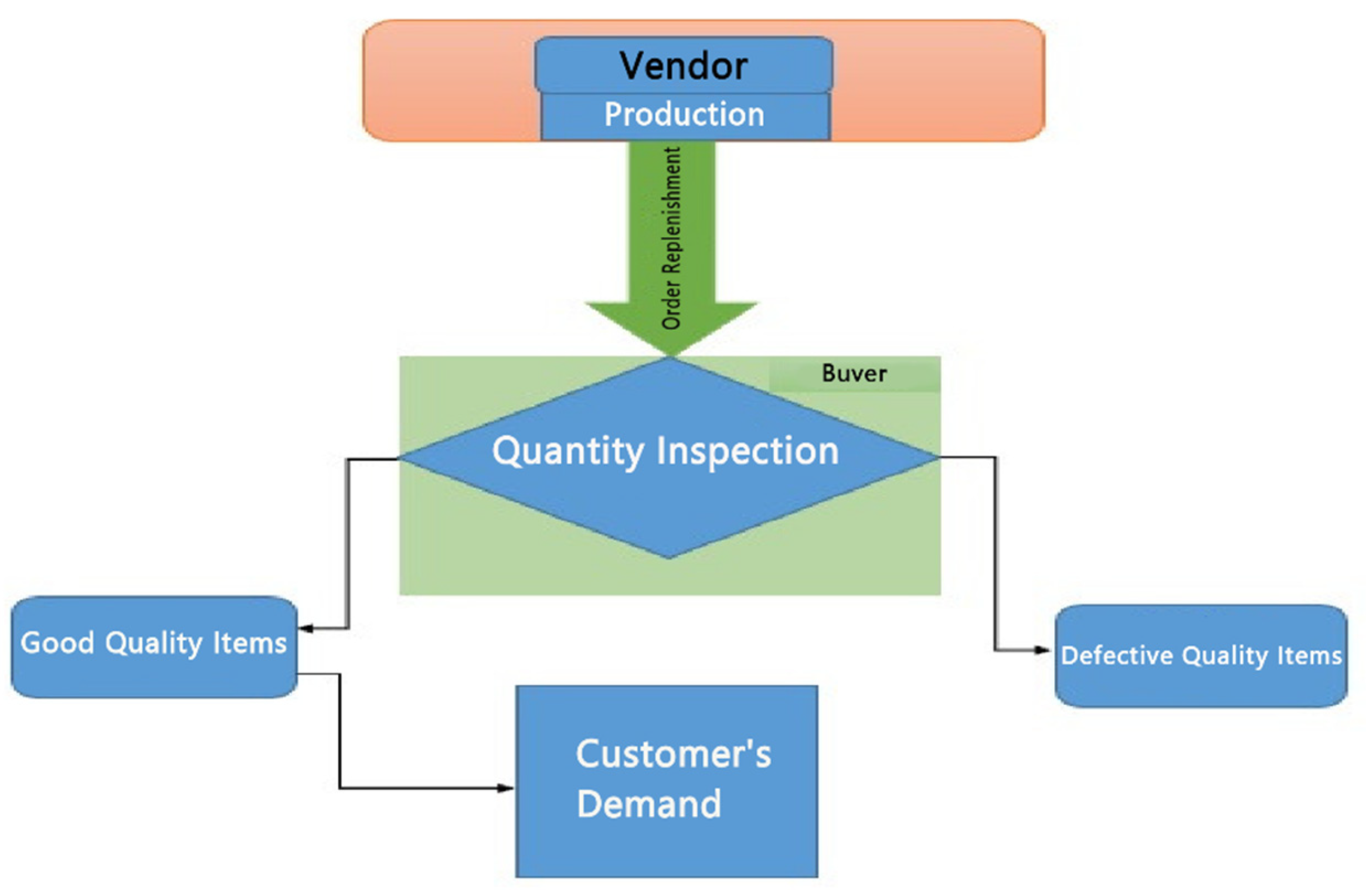
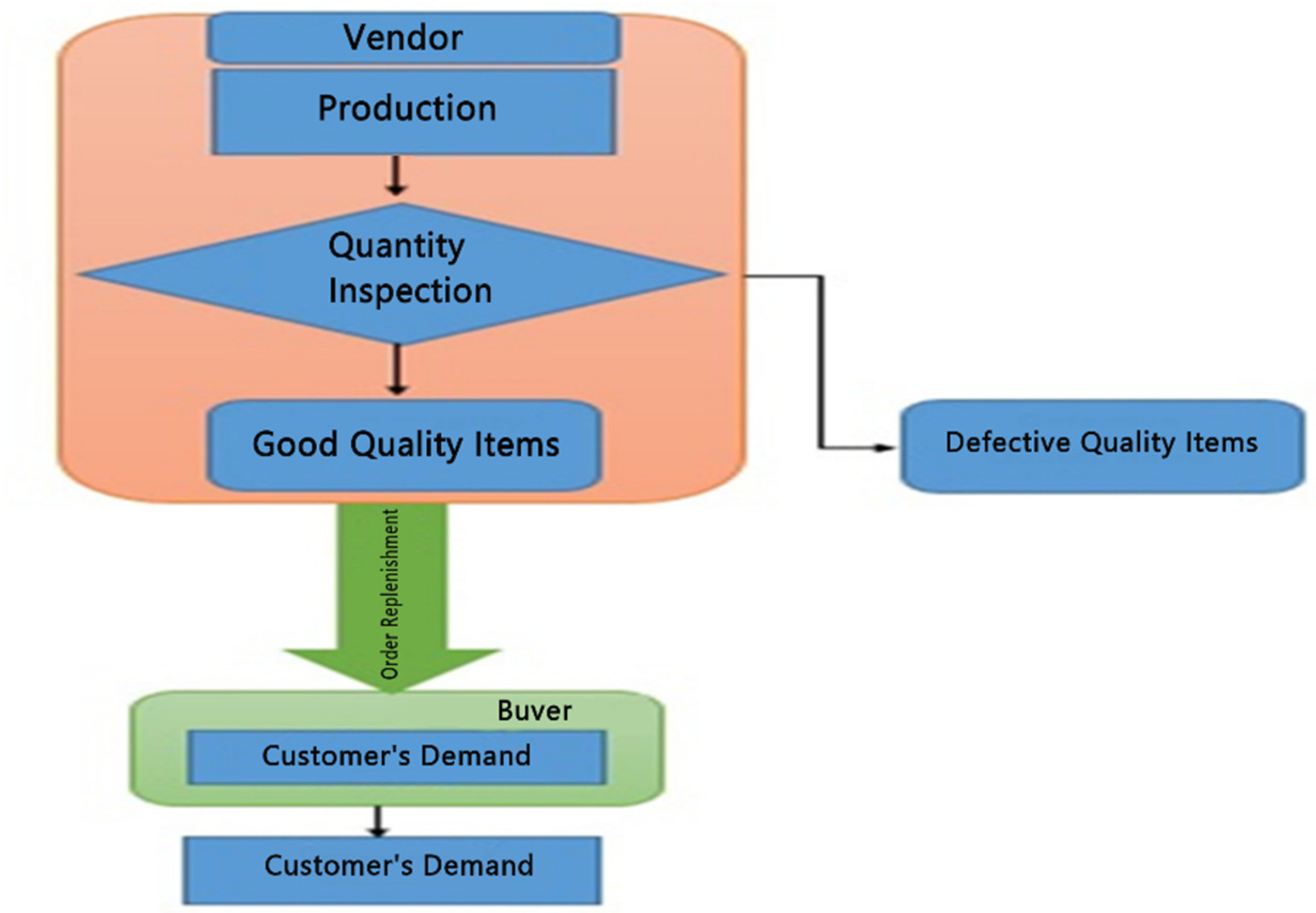
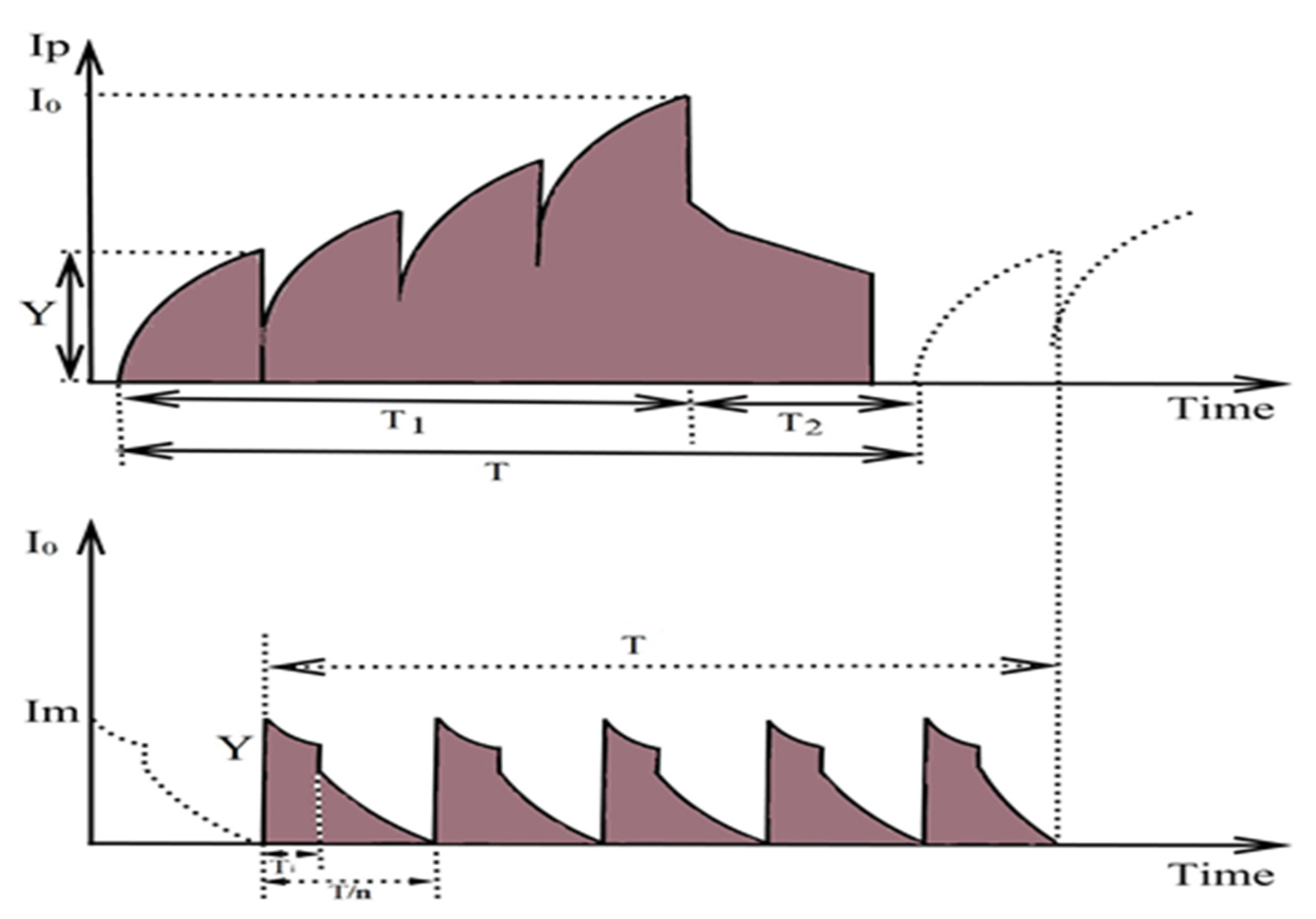
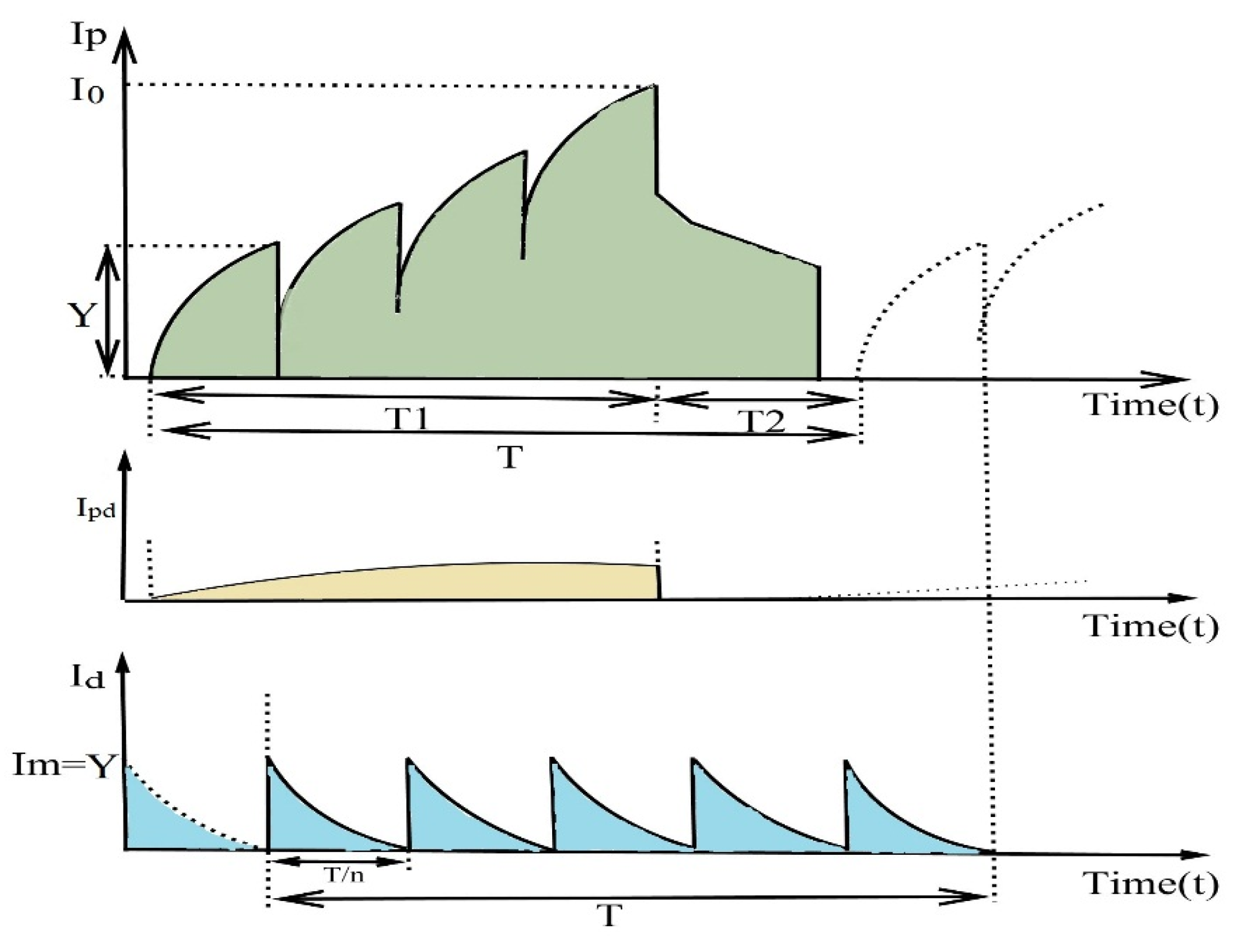
2. Problem Definition, Assumption, and Notations
2.1. Problem Definition
- What is the optimal shipment during the supply chain with a learning effect under a fuzzy environment for deteriorating items?
- What is the optimal cycle time during the supply chain with a learning effect under a fuzzy environment for deteriorating items?
- What is the minimum fuzzy inventory cost during the supply chain with a learning effect under a fuzzy environment for imperfect deteriorating items?
2.2. Assumptions
- This scenario is based on the single-setup multiple-deliveries (SSMD) policy.
- In this scenario, we considered the buyer’s demand rate to be imprecise in nature and follow the triangular fuzzy number.
- The fuzzy learning effect involves the lower and upper deviation of the demand rate.
- The production rate of the product is known and constant from the manufacturer side and the manufacturer prepares units of product in each production cycle to minimize the set time and system inventory cost, and also delivers the product in an equal lot size of the product at a fixed time interval [50].
- The replenishment of the product is instantaneous.
- The deterioration rate of the product is fixed and equal for the manufacturer and retailer.
- In model one, the retailer inspects whole lot with a fixed screening rate and the manufacturer also inspects the whole lot in the same manner as the retailer to guarantee the best facility.
- The percentage of imperfect-quality items has a uniform distribution, where .
- The defective items are sold at a discounted price without any extra cost, and products are always available during the quality inspection as .
- The fixed inspection cost per cycle is constant, whether performed by the buyer or the manufacturer.
- The concept of the carbon units/emissions of the carbon comes from the storage of inventory, burning of fuel, consumption of electricity, and transportation of the inventory products.
- The concept of shortages is not allowed in this model.
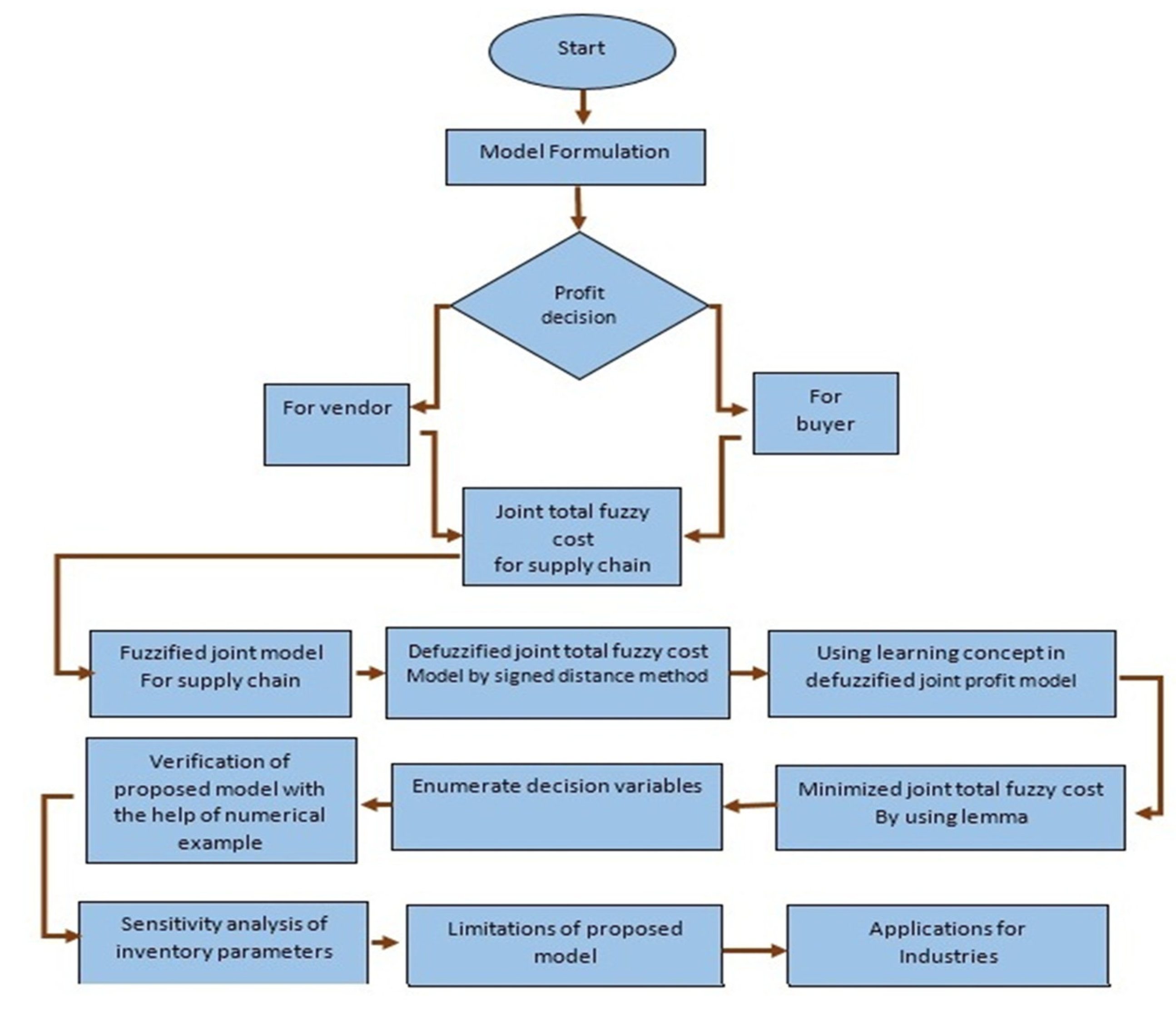
3. Model Development
3.1. Development of Model 1 When the Retailer Inspects Whole Lot
3.2. Manufacturer Strategy under Cost and Carbon Emissions
4. Formulation of the Proposed Model under Fuzzy Environment
4.1. Formulation of the Proposed Model with Fuzzy Environment under Learning Effect
4.2. Methodology and Solution Method
5. Model Development with Manufacturer Inspection
5.1. Retailer Cost and Emission
5.2. Manufacturer Cost and Emission
5.3. Proposed Model under Fuzzy Environment
5.4. Methodology and Solution Method
5.5. Numerical Example for Model 1 and 2
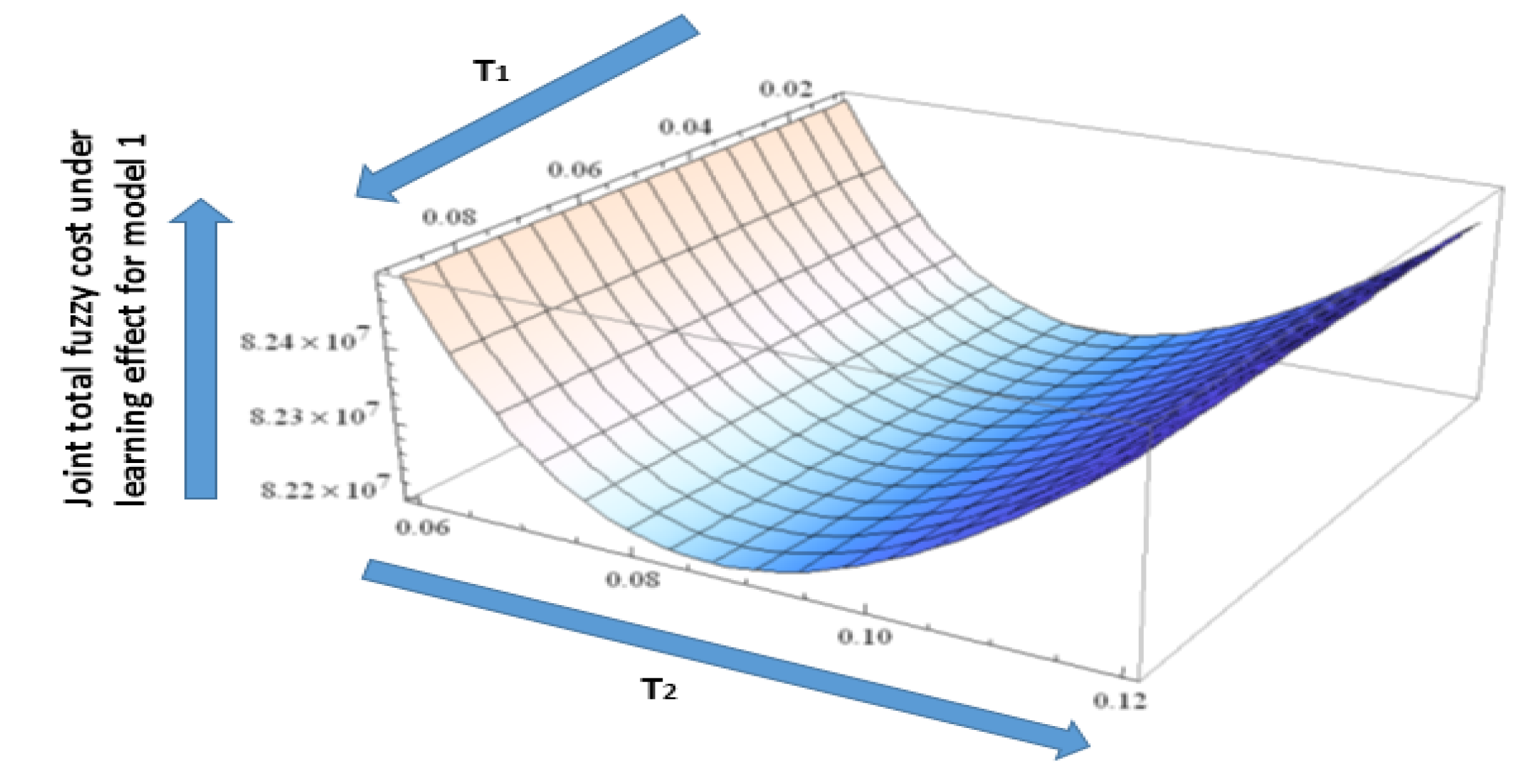
5.5.1. Numerical Example and Convexity of the Joint Total Fuzzy Cost for Model 1
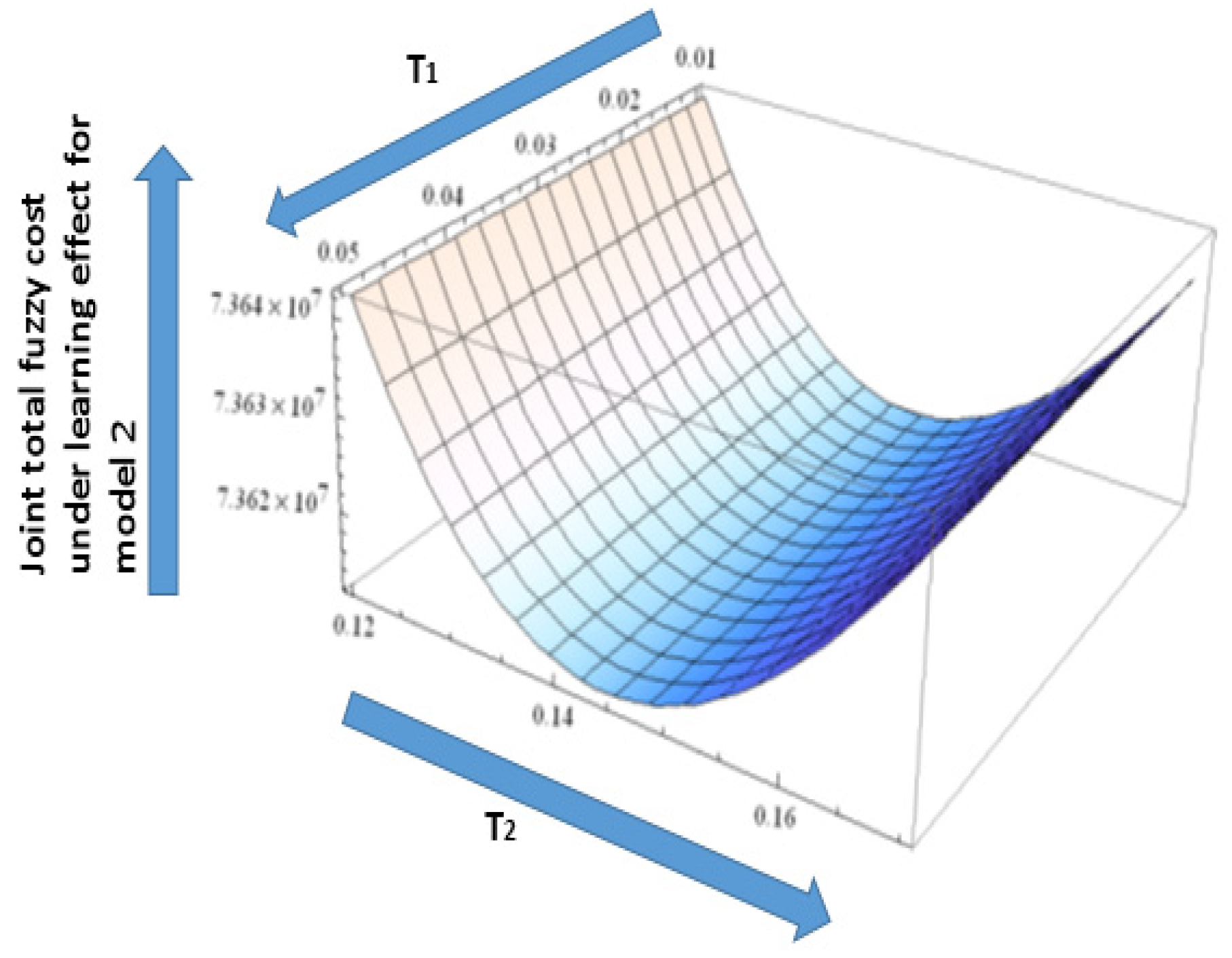
5.5.2. Numerical Example and Convexity of the Joint Total Fuzzy Cost for the Model 2
6. Sensitivity Analysis
6.1. Observation and Managerial Insights
- ➢
- By using Table 4, we concluded that from model1 and model 2, if the production rate of items increases then the number of shipments is fixed and the cycle time for the supply chain decreases, but the supply chain’s total fuzzy cost increases. This means that the production rate affects the cycle time and total fuzzy cost for the supply chain.
- ➢
- By using Table 5, we concluded that, from model 1 and model 2, if the fuzzy demand rate of items increases, then the number of shipments is fixed and the cycle time for the supply chain decreases, but the supply chain’s total fuzzy cost increases. This means that the fuzzy demand rate affects the cycle time and total fuzzy cost for the supply chain. It means that the fuzzy demand rate is a more sensitive parameter in the supply chain.
- ➢
- By using Table 6, we concluded that, from model 1 and model 2, if the values of learning rate increase, then the values of the shipments and cycle time increase, but the supply chain’s total fuzzy cost decreases up to when the learning rate moves from 0.150 to 0.154, but when the values of the learning rate increase after 0.154, then the shipments, cycle time, and supply chain’s total cost are fixed in position. This means that the learning rate affects the shipments, cycle time, and total fuzzy cost in the supply chain. Therefore, the learning effect is more effective for the decisions of the cycle time, number of shipments, and total fuzzy cost for model 1 and model 2, and also makes suggestions for the decision maker during the supply chain or ordering policies, etc. The learning fuzzy theory designs the numeric shape of the decision variables for the supply chain that we discuss in the model, like the cycle time, number of shipments, and lot size for model 1 and model 2.
- ➢
- By using Table 7 and Table 8, we concluded that, from model 1 and model 2, if the values of the unit screening cost and variable screening cost increase, then the values of the shipments and cycle time are fixed, but the supply chain’s total fuzzy cost increases. This means that the learning rate affects only the total fuzzy cost, but shipments, cycle time, and total fuzzy cost for the supply chain remain constant. This means that the shipment is independent from the inspection cost and the inspection process does not affect the shipment.
- ➢
- By using Table 9, we concluded that, from model 1 and model 2, if the deterioration rate increases, then the values of the shipment remain constant and the cycle time and supply chain’s total fuzzy cost increase.
- ➢
- By using Table 10, we concluded that, from model 1 and model 2, if the expected percentage defective increases, then the values of the shipment remain constant and the cycle time decreases, but the supply chain’s total fuzzy cost increases. It is also a sensitive parameter regarding cycle time and total fuzzy cost.
- ➢
- By using Table 11, we concluded that, from model 1 and model 2, if the carbon taxation increases, then the values of the shipment and cycle time remain constant, but the supply chain’s total fuzzy cost increases.
- ➢
- By using Table 12, we concluded that, from model 1 and model 2, if the manufacturer’s fixed transportation cost increases, then initially, the shipment and cycle time remain constant, but the supply chain’s total fuzzy cost increases. However, if the values of the manufacturer’s fixed transportation cost increase then the cycle time increases, but the shipments and total fuzzy cost decrease. Model 1 includes the transportation cost because the retailer pays the delivery charges when they receive the ordered lot and this cost saves the profit from the customer.
- ➢
- By using Table 13, we concluded that, from model 1 and model 2, if the manufacturer’s variable transportation cost increases, then initially, the shipments and cycle time remain constant, but the supply chain’s total fuzzy cost increases. However, if the values of the manufacturer’s variable transportation cost increase, then the cycle time increases but the shipments and total fuzzy cost decrease.
- ➢
- By using Table 14, we concluded from that, model 1 and model 2, if the product weight increases, then initially, the shipments and cycle time remain constant, but the supply chain’s total fuzzy cost increases.
- ➢
- By using Table 15, we concluded that, from model1 and model 2, if the average vehicle fuel consumption when there is no loading increases, then initially, the shipments and cycle time remain constant, but the supply chain’s total fuzzy cost increases.
- ➢
- By using Table 16, we concluded that, from model 1 and model 2, if the average additional fuel consumption with the loading of the items increases, then initially, the shipments and cycle time remain constant, but the supply chain’s total fuzzy cost increases.
6.2. Comparison of Proposed Model with Existing Model
7. Conclusions
7.1. Application and Future Scope of Proposed Model
7.2. Limitation of Proposed Model
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Notations
| The rate of the demand (unit per year); | |
| The rate of the fuzzy demand (unit per year); | |
| Lower deviation of the fuzzy demand (unit per year); | |
| Upper deviation of the fuzzy demand (unit per year) | |
| The rate of the production of the deteriorating items (unit per year); | |
| Manufacturer’s production period of the product in each cycle (year); | |
| The quantity for the production (units); | |
| Defective percentage items in each delivered lot; | |
| The rate of the deterioration of the product ; | |
| Screening rate of quality (unit per year); | |
| Fixed inspection rate for the quality (USD per year); | |
| The cost for unit screening items (USD per year); | |
| Ordering cost for the retailer (USD per shipment); | |
| Holding cost for the retailer (USD per unit per year); | |
| Deterioration cost for the retailer (unit per year); | |
| Set up cost for the manufacturer (USD per shipment); | |
| Holding cost for the manufacturer (USD per unit per year); | |
| Deterioration cost for the manufacturer (unit per year); | |
| Fixed transportation cost for the manufacturer (unit per delivery); | |
| Fuel cost for manufacturer’s change able transportation cost (USD per liter); | |
| Distance covered from the seller to buyer (Km); | |
| The weight of the product; | |
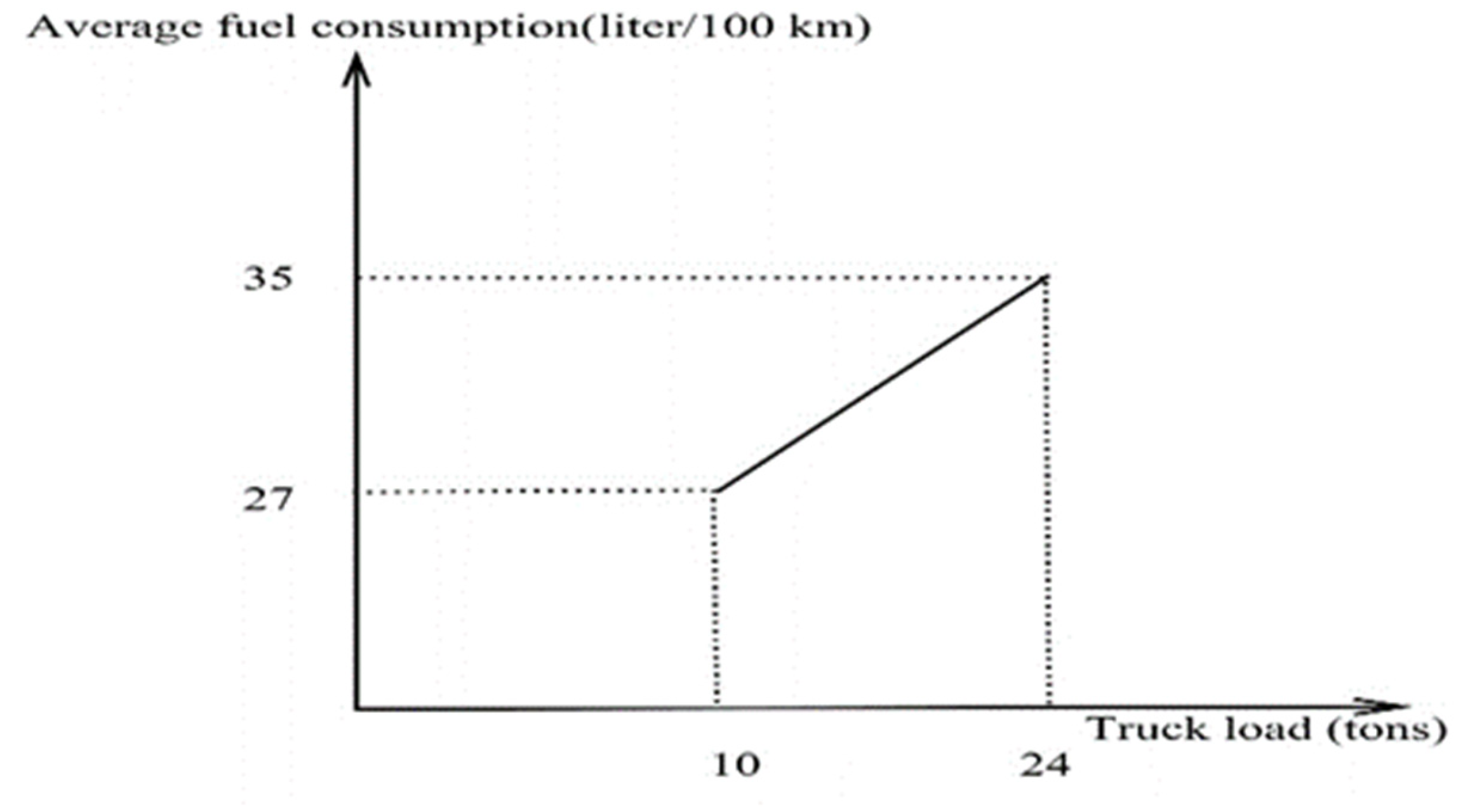
| The average vehicle fuel consumption when does not use any fuel (liter per Km); | |
| Extra or average additional fuel consumption per ton of load (liter per kilometer per ton); | |
| The tax for the carbon emissions (USD per ton CO2); | |
| Average carbon emissions due to burning of fuel (ton CO2 per liter); | |
| Average carbon emissions due to electricity generation (ton CO2 per kWh); | |
| Carbon emission cost due to transportation (USD per kilometer); | |
| Carbon emission cost per unit item due to average additional transportation (USD per unit per Km; | |
| Average carbon emissions from the warehouses of per unit item (kWh per unit per year); | |
| Carbon emission cost due to warehouse of unit per item (USD per unit per year); | |
| Cycle time (year); | |
| No production period for manufacturer; | |
| Inspection time period for each delivery for retailer; | |
| Cycle time per delivery for the retailer (year); | |
| Inventory level for the manufacturer at time ; | |
| Inventory level for the manufacturer due to defective items at time ; | |
| Inventory level for the retailer at time | |
| Retailer’s total expected cost; | |
| Manufacturer’s total expected cost; | |
| Integrated total inventory cost; | |
| Integrated total fuzzy cost; | |
| Integrated total fuzzy cost under learning effect; | |
| Retailer’s total expected cost under carbon emissions; | |
| Manufacturer’s total expected cost under carbon emissions; | |
| Integrated total expected cost under carbon emissions; | |
| Integrated total expected fuzzy cost under carbon emissions; | |
| Integrated total expected cost under carbon emissions and learning in fuzzy; | |
| Lot size for the supply chain (variable decision); | |
| Number of shipment (variable decision); |
References
- Glock, C.H. The joint economic lot size problem: A review. Int. J. Prod. Econ. 2012, 135, 671–686. [Google Scholar] [CrossRef]
- Luo, Z.; Gunasekaran, A.; Dubey, R.; Childe, S.J.; Papadopoulos, T. Antecedents of low carbon emissions supply chains. Int. J. Clim. Chang. Strateg. Manag. 2017, 9, 707–727. [Google Scholar] [CrossRef]
- Das, C.; Jharkharia, S. Low carbon supply chain: A state-of-the-art literature review. J. Manuf. Technol. Manag. 2018, 29, 398–428. [Google Scholar] [CrossRef]
- Kazemi, N.; Abdul-Rashid, S.H.; Ghazilla, R.A.R.; Shekarian, E.; Zanoni, S. Economic order quantity models for items with imperfect quality and emission considerations. Int. J. Syst. Sci. Oper. Logist. 2018, 5, 99–115. [Google Scholar] [CrossRef]
- Sarkar, B.; Ahmed, W.; Choi, S.B.; Tayyab, M. Sustainable inventory management for environmental impact through partial backordering and multi-trade-credit period. Sustainability 2018, 10, 4761. [Google Scholar] [CrossRef]
- Taleizadeh, A.A.; Soleymanfar, V.R.; Govindan, K. Sustainable economic production quantity models for inventory system with shortage. J. Clean. Prod. 2018, 174, 1011–1020. [Google Scholar] [CrossRef]
- Sarkar, B.; Ganguly, B.; Sarkar, M.; Pareek, S. Effect of variable transportation and carbon emission in a three-echelon supply chain model. Transp. Res. Part E Logist. Transp. Rev. 2016, 91, 112–128. [Google Scholar] [CrossRef]
- Sarkar, B.; Ahmed, W.; Kim, N. Joint effects of variable carbon emission cost and multi-delay-in-payments under single-setup-multiple-delivery policy in a global sustainable supply chain. J. Clean. Prod. 2018, 185, 421–445. [Google Scholar] [CrossRef]
- Daryanto, Y.; Wee, H.M. Single vendor-buyer integrated inventory model for deteriorating items considering carbon emission. In Proceedings of the 8th International Conference on Industrial Engineering and Operations Management (IEOM), Bandung, Indonesia, 6–8 March 2018; pp. 544–555. [Google Scholar]
- Wahab, M.I.M.; Mamun, S.M.H.; Ongkunarak, P. EOQ models for a coordinated two-level international supply chain considering imperfect items and environmental impact. Int. J. Prod. Econ. 2011, 134, 151–158. [Google Scholar] [CrossRef]
- Jauhari, W.A.; Pamuji, A.S.; Rosyidi, C.N. Cooperative inventory model for vendor-buyer system with unequal-sized shipment, defective items and carbon emission cost. Int. J. Logist. Syst. Manag. 2014, 19, 163–186. [Google Scholar] [CrossRef]
- Sarkar, B.; Saren, S.; Sarkar, M.; Seo, Y.W. A Stackelberg game approach in an integrated inventory model with carbon-emission and setup cost reduction. Sustainability 2016, 8, 1244. [Google Scholar] [CrossRef]
- Jauhari, W.A. A collaborative inventory model for vendor-buyer system with stochastic demand, defective items and carbon emission cost. Int. J. Logist. Syst. Manag. 2018, 29, 241–269. [Google Scholar]
- Gautam, P.; Khanna, A. An imperfect production inventory model with setup cost reduction and carbon emission for an integrated supply chain. Uncertain Supply Chain Manag. 2018, 6, 271–286. [Google Scholar] [CrossRef]
- Tiwari, S.; Daryanto, Y.; Wee, H.M. Sustainable inventory management with deteriorating and imperfect quality items considering carbon emissions. J. Clean. Prod. 2018, 192, 281–292. [Google Scholar] [CrossRef]
- Rosenblatt, M.J.; Lee, H.L. Economic production cycles with imperfect production processes. IIE Trans. 1986, 18, 48–55. [Google Scholar] [CrossRef]
- Porteus, E.L. Optimal lot sizing, process quality improvement and setup cost reduction. Oper. Res. 1986, 34, 137–144. [Google Scholar] [CrossRef]
- Salameh, M.K.; Jaber, M.Y. Economic production quantity model for items with imperfect quality. Int. J. Prod. Econ. 2000, 64, 59–64. [Google Scholar] [CrossRef]
- Huang, C.K. An integrated vendor-buyer cooperative inventory model for items with imperfect quality. Prod. Plan. Control 2002, 13, 355–361. [Google Scholar] [CrossRef]
- Goyal, S.K.; Huang, C.K.; Chen, K.C. A simple integrated production policy of an imperfect item for vendor and buyer. Prod. Plan. Control 2003, 14, 596–602. [Google Scholar] [CrossRef]
- Wee, H.M.; Yu, J.C.P.; Wang, K.J. An integrated production-inventory model for deteriorating items with imperfect quality and shortage backordering considerations. In Proceedings of the International Conference on Computational Science and Its Applications (ICCSA), Glasgow, UK, 8–11 May 2006; Springer: Berlin/Heidelberg, Germany, 2006; pp. 885–897. [Google Scholar]
- Lee, S.; Kim, D. An optimal policy for a single vendor single-buyer integrated production-distribution model with both deteriorating and defective items. Int. J. Prod. Econ. 2014, 147, 161–170. [Google Scholar] [CrossRef]
- Bazan, E.; Jaber, M.Y.; Zanoni, S.; Zavanella, L.E. Vendor managed inventory (VMI) with consignment stock (CS) agreement for a two-level supply chain with an imperfect production process with/without restoration interruptions. Int. J. Prod. Econ. 2014, 157, 289–301. [Google Scholar] [CrossRef]
- Sarkar, B.; Shaw, B.K.; Kim, T.; Sarkar, M.; Shin, D. An integrated inventory model with variable transportation cost, two-stage inspection, and defective items. J. Ind. Manag. Optim. 2017, 13, 1975–1990. [Google Scholar] [CrossRef]
- Yu, H.F.; Hsu, W.K. An integrated inventory model with immediate return for defective items under unequal-sized shipments. J. Ind. Prod. Eng. 2017, 34, 70–77. [Google Scholar] [CrossRef]
- Benjaafar, S.; Li, Y.; Daskin, M. Carbon footprint and the management of supply chains: Insights from simple models. IEEE Trans. Autom. Sci. Eng. 2013, 10, 99–116. [Google Scholar] [CrossRef]
- Fahimnia, B.; Sarkis, J.; Dehghanian, F.; Banihashemi, N.; Rahman, S. The impact of carbon pricing on a closed-loop supply chain: An Australian case study. J. Clean. Prod. 2013, 59, 210–225. [Google Scholar] [CrossRef]
- Bozorgi, A.; Pazour, J.; Nazzal, D. A new inventory model for cold items that considers costs and emissions. Int. J. Prod. Econ. 2014, 155, 114–125. [Google Scholar] [CrossRef]
- Bozorgi, A. Multi-product inventory model for cold items with cost and emission consideration. Int. J. Prod. Econ. 2016, 176, 123–142. [Google Scholar] [CrossRef]
- Ruidas, S.; Seikh, M.R.; Nayak, P.K. A production inventory model with interval-valued carbon emission parameters under price-sensitive demand. Compu. Ind. Eng. 2021, 154, 107154. [Google Scholar] [CrossRef]
- Ghosh, A.; Jha, J.K.; Sarmah, S.P. Optimizing a two-echelon serial supply chain with different carbon policies. Int. J. Sustain. Eng. 2016, 9, 363–377. [Google Scholar] [CrossRef]
- Toptal, A.; Çetinkaya, B. How supply chain coordination affects the environment: A carbon footprint perspective. Ann. Oper. Res. 2017, 250, 487–519. [Google Scholar] [CrossRef]
- Bouchery, Y.; Ghaffari, A.; Jemai, Z.; Tan, T. Impact of coordination on cost and carbon emissions for a two-echelon serial economic order quantity problem. Eur. J. Oper. Res. 2017, 260, 520–533. [Google Scholar] [CrossRef]
- Dwicahyani, A.R.; Jauhari, W.A.; Rosyidi, C.N.; Laksono, P.W. Inventory decisions in a two-echelon system with remanufacturing, carbon emission, and energy effects. Cogent Eng. 2017, 4, 1379628. [Google Scholar] [CrossRef]
- Li, J.; Su, Q.; Ma, L. Production and transportation outsourcing decisions in the supply chain under single and multiple carbon policies. J. Clean. Prod. 2017, 141, 1109–1122. [Google Scholar] [CrossRef]
- Wangsa, I.D. Greenhouse gas penalty and incentive policies for a joint economic lot size model with industrial and transport emissions. Int. J. Ind. Eng. Comput. 2017, 8, 453–480. [Google Scholar]
- Anvar, S.H.; Sadegheih, A.; Zad, M.A.V. Carbon emission management for greening supply chains at the operational level. Environ. Eng. Manag. J. 2018, 17, 1337–1347. [Google Scholar] [CrossRef]
- Hariga, M.; Babekian, S.; Bahroun, Z. Operational and environmental decisions for a two-stage supply chain under vendor managed consignment inventory partnership. Int. J. Prod. Res. 2018, 57, 3642–3662. [Google Scholar] [CrossRef]
- Ji, S.; Zhao, D.; Peng, X. Joint decisions on emission reduction and inventory replenishment with overconfidence and low-carbon preference. Sustainability 2018, 10, 1119. [Google Scholar] [CrossRef]
- Wang, S.; Ye, B. A comparison between just-in-time and economic order quantity models with carbon emissions. J. Clean. Prod. 2018, 187, 662–671. [Google Scholar] [CrossRef]
- Ghosh, A.; Sarmah, S.P.; Jha, J.K. Collaborative model for a two-echelon supply chain with uncertain demand under carbon tax policy. Sadhana 2018, 43, 144. [Google Scholar] [CrossRef]
- Ma, X.; Ho, W.; Ji, P.; Talluri, S. Coordinated pricing analysis with the carbon tax scheme in a supply chain. Decis. Sci. 2018, 49, 863–900. [Google Scholar] [CrossRef]
- Darom, N.A.; Hishamuddin, H.; Ramli, R.; Nopiah, Z.M. An inventory model of supply chain disruption recovery with safety stock and carbon emission consideration. J. Clean. Prod. 2018, 197, 1011–1021. [Google Scholar] [CrossRef]
- Huang, H.; He, Y.; Li, D. Pricing and inventory decisions in the food supply chain with production disruption and controllable deterioration. J. Clean. Prod. 2018, 180, 280–296. [Google Scholar] [CrossRef]
- Daryanto, Y.; Wee, H.M.; Astanti, R.D. Three-echelon supply chain model considering carbon emission and item deterioration. Transp. Res. Part E Logist. Transp. Rev. 2019, 122, 368–383. [Google Scholar] [CrossRef]
- Kundu, S.; Chakrabarti, T. A fuzzy rough integrated multi-stage supply chain inventory model with carbon emissions under inflation and time-value of money. Int. J. Math. Oper. Res. 2019, 14, 123–145. [Google Scholar] [CrossRef]
- Shalke, P.N.; Paydar, M.M.; Hajiaghaei-Keshteli, M. Sustainable supplier selection and order allocation through quantity discounts. Int. J. Manag. Sci. Eng. Manag. 2018, 13, 20–32. [Google Scholar]
- Moheb-Alizadeh, H.; Handfield, R. An integrated chance-constrained stochastic model for efficient and sustainable supplier selection and order allocation. Int. J. Prod. Res. 2018, 56, 6890–6916. [Google Scholar] [CrossRef]
- Moheb-Alizadeh, H.; Handfield, R. Sustainable supplier selection and order allocation: A novel multi-objective programming model with a hybrid solution approach. Comput. Ind. Eng. 2019, 129, 192–209. [Google Scholar] [CrossRef]
- Jaggi, C.K.; Sharma, A.; Mittal, M. A fuzzy inventory model for deteriorating items with initial inspection and allowable shortage under the condition of permissible delay in payment. Int. J. Invent. Control Manag. 2012, 2, 167–200. [Google Scholar] [CrossRef]
- Jaggi, C.K.; Pareek, S.; Sharma, A. A Fuzzy Inventory Model for Weibull Deteriorating Items with Price-Dependent Demand and Shortages under Permissible Delay in Payment. Inter. J. Appl. Indus. Eng. 2012, 1, 53–79. [Google Scholar] [CrossRef][Green Version]
- Jaggi, C.K.; Sharma, A.; Jain, R. EOQ model with permissible delay in payments under fuzzy environment. In Analytical Approaches to Strategic Decision-Making: Interdisciplinary Considerations; IGI Global: Hershey, PA, USA, 2014; pp. 281–296. [Google Scholar]
- Rout, C.; Kumar, R.S.; Paul, A.; Chakraborty, D.; Goswami, A. Designing a single-vendor and multiple-buyers’ integrated production inventory model for interval type-2 fuzzy demand and fuzzy rule based deterioration. RAIRO-Oper. Res. 2021, 55, 3715–3742. [Google Scholar] [CrossRef]
- Patro, R.; Acharya, M.; Nayak, M.M.; Patnaik, S. A fuzzy EOQ model for deteriorating items with imperfect quality using proportionate discount under learning effects. Int. J. Manag. Decis. Mak. 2018, 17, 171–198. [Google Scholar] [CrossRef]
- Bhavani, G.D.; Meidute-Kavaliauskiene, I.; Mahapatra, G.S.; Činčikaitė, R.A. Sustainable Green Inventory System with Novel Eco-Friendly Demand Incorporating Partial Backlogging under Fuzziness. Sustainability 2022, 14, 9155. [Google Scholar] [CrossRef]
- Wright, T.P. Factors affecting the cost of airplanes. J. Aeronaut. Sci. 1936, 3, 122–128. [Google Scholar] [CrossRef]
- Jayaswal, M.K.; Mittal, M.; Alamri, O.A.; Khan, F.A. Learning EOQ Model with Trade-Credit Financing Policy for Imperfect Quality Items under Cloudy Fuzzy Environment. Mathematics 2022, 10, 246. [Google Scholar] [CrossRef]
- Jayaswal, M.K.; Mittal, M.; Sangal, I.; Tripathi, J. Fuzzy-Based EOQ Model with Credit Financing and Backorders Under Human Learning. Int. J. Fuzzy Syst. Appl. 2021, 10, 14–36. [Google Scholar] [CrossRef]
- Jayaswal, M.K.; Mittal, M. Impact of Learning on the Inventory Model of Deteriorating Imperfect Quality Items with Inflation and Credit Financing under Fuzzy Environment. Int. J. Fuzzy Syst. Appl. 2022, 11, 1–36. [Google Scholar] [CrossRef]
- Alamri, O.A.; Jayaswal, M.K.; Mittal, M. A Supply Chain Model with Learning Effect and Credit Financing Policy for Imperfect Quality Items under Fuzzy Environment. Axioms 2023, 12, 260. [Google Scholar] [CrossRef]
- Alamri, O.A. Sustainable Supply Chain Model for Defective Growing Items (Fishery) with Trade Credit Policy and Fuzzy Learning Effect. Axioms 2023, 12, 436. [Google Scholar] [CrossRef]
- Alsaedi, B.S.; Alamri, O.A.; Jayaswal, M.K.; Mittal, M. A sustainable green supply chain model with carbon emissions for defective items under learning in a fuzzy environment. Mathematics 2023, 11, 301. [Google Scholar] [CrossRef]
- Giri, B.K.; Roy, S.K. Fuzzy-random robust flexible programming on sustainable closed-loop renewable energy supply chain. Appl. Energy 2024, 363, 123044. [Google Scholar] [CrossRef]
- Hariga, M.; As’ad, R.; Shamayleh, A. Integrated economic and environmental models for a multi stage cold supply chain under carbon tax regulation. J. Clean. Prod. 2017, 166, 1357–1371. [Google Scholar] [CrossRef]
- Wangsa, I.D.; Wee, H.M. An integrated vendor-buyer inventory model with transportation cost and stochastic demand. Int. J. Syst. Sci. Oper. Logist. 2018, 5, 295–309. [Google Scholar]
- Rahman, M.N.A.; Leuveano, R.A.C.; bin Jafar, F.A.; Saleh, C.; Deros, B.M.; Mahmood, W.M.F.W.; Mahmood, W.H.W. Incorporating logistic costs into a single vendor-buyer JELS model. Appl. Math. Model. 2016, 40, 10809–10819. [Google Scholar] [CrossRef]
- Nie, L.; Xu, X.; Zhan, D. Incorporating transportation costs into JIT lot splitting decisions for coordinated supply chains. J. Adv. Manuf. Syst. 2006, 5, 111–121. [Google Scholar] [CrossRef]
- Yang, P.C.; Wee, H.M. Economic ordering policy of deteriorated item for vendor and buyer: An integrated approach. Prod. Plan. Control 2000, 11, 474–480. [Google Scholar] [CrossRef]
- Ji, Y.; Li, Y.; Wijekoon, C. Robust two-stage minimum asymmetric cost consensus models under uncertainty circumstances. Inf. Sci. 2024, 120279. [Google Scholar] [CrossRef]
- Ji, Y.; Ma, Y. The robust maximum expert consensus model with risk aversion. Inf. Fusion 2023, 99, 101866. [Google Scholar] [CrossRef]



| Author | Supply Chain | Vender’s Inspection | Buyer’s Inspection | Carbon Emissions | Deteriorating Items | Imperfect Items and Inspection | Learning in Inspection | Learning in Fuzzy Environment |
|---|---|---|---|---|---|---|---|---|
| Wright [56] | ✔ | |||||||
| Salameh and Jaber [18] | ✔ | |||||||
| Tiwari et al. [15] | ✔ | ✔ | ✔ | ✔ | ||||
| Bazan et al. [23] | ✔ | ✔ | ||||||
| Wangsa [36] | ✔ | ✔ | ||||||
| Daryantoet al. [45] | ✔ | ✔ | ✔ | ✔ | ||||
| Jaggi et al. [50] | ✔ | |||||||
| Patro et al. [54] | ✔ | ✔ | ✔ | |||||
| Jayaswal et al. [59] | ✔ | ✔ | ✔ | |||||
| Almari [61] | ✔ | ✔ | ||||||
| Alsaedi et al. [62] | ✔ | ✔ | ✔ | ✔ | ||||
| Giri and Roy [63] | ✔ | Nice contribution in fuzzy environment and did not work with learning | ||||||
| Proposed model | ✔ | ✔ | ✔ | ✔ | ✔ | ✔ | ✔ | ✔ |
| Inventory Parameters | Values with Unit | Inventory Parameters | Values with Unit |
|---|---|---|---|
| 2,000,000 units per year | USD 2000 per order | ||
| 50,000 units per year | USD 100,000 per setup | ||
| 1,725,000 unit per year | USD 60 per unit per year | ||
| USD 500 per delivery | USD 40 per unit per year | ||
| USD 0.5 per unit | USD 600 per unit | ||
| 0.10 | USD 400 per unit | ||
| USD 1000 per delivery | 100 km | ||
| USD 0.75 per liter | 0.01 ton per unit | ||
| 1.44 kwh per hour unit per year | 27 L per 100 km | ||
| USD 75 per ton | 0.57 L per 100 km per ton truck load | ||
| ton C per liter | 0 | ||
| ton C per kwh | 0.04 with | ||
| 4 |
| Model 1 | Model 2 | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| 3 | USD | 5 | USD | ||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 2,000,000 | 3 | 5 | USD | |||||||
| 2,100,000 | 3 | 0.11006 | 5 | 0.17385 | USD | |||||
| 2,200,000 | 3 | 0.10774 | 5 | 0.1721 | USD | |||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 5 | USD | ||||||||
| 5 | 0.11023 | 7 | 0.17305 | USD | ||||||
| 5 | 0.10674 | 7 | 0.1715 | USD | ||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.10189 | 2 | 0.17206 | USD | ||||||
| 2 | 0.10619 | 3 | 0.17407 | USD | ||||||
| 3 | 5 | USD | ||||||||
| 0.153 | 3 | 5 | USD | |||||||
| 0.154 | 3 | 5 | USD | |||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 0.10374 | 5 | 0.17077 | USD | ||||||
| 3 | 0.10671 | 5 | 0.17465 | USD | ||||||
| 3 | 5 | USD | ||||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 5 | USD | ||||||||
| 3 | 5 | USD | ||||||||
| 3 | 5 | USD | ||||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 0.11295 | 5 | 0.18206 | USD | ||||||
| 3 | 0.11270 | 5 | 0.18075 | USD | ||||||
| 3 | 5 | USD | ||||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 5 | USD | ||||||||
| 3 | 0.11200 | 5 | 0.17435 | USD | ||||||
| 3 | 0.11118 | 5 | 0.17257 | USD | ||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 5 | USD | ||||||||
| 3 | 5 | USD | ||||||||
| 3 | 5 | USD | ||||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 5 | USD | ||||||||
| 3 | 5 | USD | ||||||||
| 3 | 5 | USD | ||||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 5 | USD | ||||||||
| 3 | 4 | USD | ||||||||
| 2 | 3 | USD | ||||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 5 | USD | ||||||||
| 3 | 4 | USD | ||||||||
| 2 | 3 | USD | ||||||||
| Input Parameter | Model I | Model II | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3 | 5 | USD | ||||||||
| 3 | 5 | USD | ||||||||
| 3 | 5 | USD | ||||||||
| Input Parameter | Model I | Model II | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.0057 | 3 | 5 | USD | ||||||||
| 0.0058 | 3 | 5 | USD | ||||||||
| 0.0059 | 3 | 5 | USD | ||||||||
| Author’s Name | Double Screening Process | Learning Fuzzy Thery | Lot Size | Cycle Time as a Decision Variable | Shipment as a Decision Variable | Total Profit/Total Cost under EOQ/EPQ/Supply Chain |
|---|---|---|---|---|---|---|
| Salsmeh and Jaber [18] | Not calculated | Not calculated | 1439 units | Not calculated | Not calculated | USD 1,212,235 |
| Yu et al. [25] | Not calculated | Not calculated | 1288 units | Not calculated | Not calculated | USD 1,212,148 |
| Jaggi et al. [52] | Not calculated | Not calculated | 1642 units | Not calculated | Not calculated | USD 347,086 |
| Patro et al. [54] | Not calculated | Not calculated | 1117 units | Not calculated | Not calculated | USD 1,273,420 |
| Alamri et al. [60] | Not calculated | Not calculated | 48225 units | Not calculated | Not calculated | USD 1,662,440 |
| Our paper under supply chain for model 1 | Yes | Yes | 6198 units | 0.11248 Year | Calculated, 3 | Total inventory fuzzy cost for the supply chain USD 8,220,400 |
| Our paper under supply chain for model 2 | Yes | Yes | 5987 units | 0.17627 year | Calculated, 5 | Total inventory fuzzy cost for the supply chain USD 7,361,220 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alsaedi, B.S.O.; Ahelali, M.H. A Sustainable Supply Chain Model with Low Carbon Emissions for Deteriorating Imperfect-Quality Items under Learning Fuzzy Theory. Mathematics 2024, 12, 1237. https://doi.org/10.3390/math12081237
Alsaedi BSO, Ahelali MH. A Sustainable Supply Chain Model with Low Carbon Emissions for Deteriorating Imperfect-Quality Items under Learning Fuzzy Theory. Mathematics. 2024; 12(8):1237. https://doi.org/10.3390/math12081237
Chicago/Turabian StyleAlsaedi, Basim S. O., and Marwan H. Ahelali. 2024. "A Sustainable Supply Chain Model with Low Carbon Emissions for Deteriorating Imperfect-Quality Items under Learning Fuzzy Theory" Mathematics 12, no. 8: 1237. https://doi.org/10.3390/math12081237
APA StyleAlsaedi, B. S. O., & Ahelali, M. H. (2024). A Sustainable Supply Chain Model with Low Carbon Emissions for Deteriorating Imperfect-Quality Items under Learning Fuzzy Theory. Mathematics, 12(8), 1237. https://doi.org/10.3390/math12081237







