Stationary Distribution of Stochastic Age-Dependent Population–Toxicant Model with Markov Switching
Abstract
1. Introduction
- A stochastic age-dependent population–toxicant model with Markov switching is established. The ergodicity of the invariant measure for this model is obtained, applying stochastic techniques such as Gronwall inequality, Young inequality, and so on.
- Under certain suitable conditions, the explicit EM semi-discrete method is used for the time variables, and the convergence of the numerical invariant measure is analyzed.
2. Model and Preliminaries
2.1. Model Formulation
2.2. Preliminaries
- Let be a complete probability space with as the natural filtration generated by Brownian motion (), which means augmented with all P-null sets of .
- stands for the expectation corresponding to .
- C denotes a positive constant whose value may change in different occurrences.
- , where represents generalized partial derivatives, and is a Sobolev space.
- denotes the duality product between V and , and is the scalar product in H.
3. Invariant Measure
3.1. Invariant Measure of Exact Solution
3.2. Numerical Invariant Measure
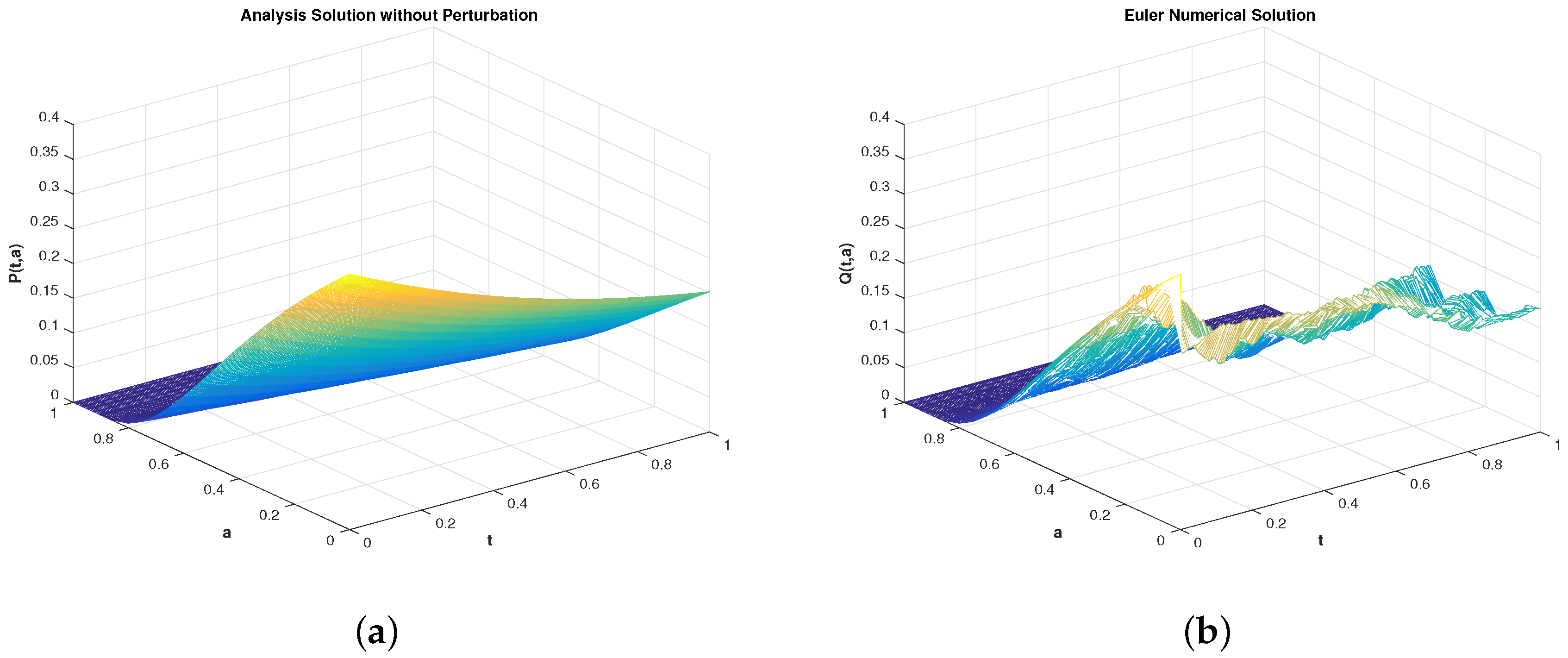
4. Numerical Example
5. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Srinivasu, P.D.N. Control of environmental pollution to conserve a population. Nonlinear Anal. Real World Appl. 2002, 3, 397–411. [Google Scholar] [CrossRef]
- Amani, A.Z. Studies on the population dynamics of some common weeds under the stress of environmental pollution. J. Dent. 1983, 41, 195–206. [Google Scholar]
- Agarwal, M.; Devi, S. The effect of environmental tax on the survival of biological species in a polluted environment: A mathematical model. Nonlinear Anal. Model. Control 2010, 3, 271–286. [Google Scholar] [CrossRef]
- Hallam, T.G.; Clark, C.E.; Lassiter, R.R. Effects of toxicants on populations: A qualitative approach I. Equilibrium environmental exposure. Ecol. Model. 1983, 18, 291–304. [Google Scholar] [CrossRef]
- Hallam, T.G.; Clar, C.E.; Jordan, G.S. Effects of toxicant on population: A qualitative approach II. First Order Kinetics. J. Math. Biol. 1983, 109, 411–429. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Ma, Z. The threshold of survival for system of two species in a polluted environment. J. Math. Biol. 1991, 30, 49–61. [Google Scholar] [PubMed]
- Feng, Y.; Wang, K. The survival analysis for single-species system in a polluted environment. Ann. Differ. Equ. 2006, 22, 154–159. [Google Scholar]
- Hallam, T.G.; Zhien, M. Persistence in Population models with demographic fluctuations. J. Math. Biol. 1986, 24, 327–339. [Google Scholar] [CrossRef] [PubMed]
- Jinxiao, P.; Zhen, J.; Zhien, M. Thresholds of survival for an n-dimensional Volterra mutualistic system in a polluted environment. J. Math. Anal. Appl. 2000, 252, 519–531. [Google Scholar] [CrossRef][Green Version]
- Mukherjee, D. Persistence and global stability of a population in a polluted environment with delay. J. Biol. Syst. 2008, 10, 225–232. [Google Scholar] [CrossRef]
- He, J.; Wang, K. The survival analysis for a population in a polluted environment. Nonlinear Anal. Real World Appl. 2009, 10, 1555–1571. [Google Scholar] [CrossRef]
- Li, R.; Leung, P.; Pang, W. Convergence of numerical solutions to stochastic age-dependent population equations with Markovian switching. J. Comput. Appl. Math. 2009, 233, 1046–1055. [Google Scholar] [CrossRef]
- Ma, W.; Zhang, Q. Convergence of the Semi-implicit Euler method for stochastic age-dependent population equations with Markovian switching. In Proceedings of the Information Computing and Applications, Tangshan, China, 15–18 October 2010; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Rathinasamy, A. Split-step θ-methods for stochastic age-dependent population equations with Markovian switching. Nonlinear Anal. Real World Appl. 2012, 13, 1334–1345. [Google Scholar] [CrossRef]
- Liu, M.; Wang, K. Persistence and extinction of a stochastic single-species model under regime switching in a polluted environment II. J. Theor. Biol. 2010, 267, 283–291. [Google Scholar] [CrossRef]
- Yin, G.; Mao, X.; Yin, K. Numerical approximation of invariant measures for hybrid diffusion systems. IEEE Trans. Autom. Control 2005, 50, 934–946. [Google Scholar] [CrossRef]
- Bao, J.; Yin, G.; Yuan, C. Ergodicity for functional stochastic differential equations and applications. Nonlinear Anal. Theory Methods Appl. 2014, 98, 66–82. [Google Scholar] [CrossRef]
- Ruttanaprommarin, N.; Sabir, Z.; Núñez, R.A.S.; Az-Zobi, E.; Weera, W.; Botmart, T.; Zamart, C. A stochastic framework for solving the prey-predator delay differential model of Holling type-III. Comput. Mater. Contin. 2023, 3, 5915–5930. [Google Scholar] [CrossRef]
- Mao, X.; Yuan, C.; Yin, G. Numerical method for stationary distribution of stochastic differential equations with Markovian switching. J. Comput. Appl. Math. 2005, 174, 1–27. [Google Scholar] [CrossRef]
- Yu, X.; Yuan, S.; Zhang, T. Persistence and ergodicity of a stochastic single species model with Allee effect under regime switching. Commun. Nonlinear Sci. Numer. Simul. 2018, 59, 359–374. [Google Scholar] [CrossRef]
- Vassiliou, P.C.G. Non-Homogeneous Markov Chains and Systems. Theory and Applications; Chapman and Hall: London, UK; CRC Press: Boca Raton, FL, USA, 2023. [Google Scholar]
- Yuan, C.; Mao, X. Stationary distributions of Euler-Maruyama-type stochastic difference equations with Markovian switching and their convergence. J. Differ. Equ. Appl. 2005, 11, 29–48. [Google Scholar] [CrossRef]
- Bao, J.; Shao, J.; Yuan, C. Approximation of invariant measures for Regime-switching Diffusions. Potential Anal. 2016, 44, 707–727. [Google Scholar] [CrossRef]
- Zhao, Y.; Yuan, S.; Zhang, Q. Numerical solution of a fuzzy stochastic single-species age-structure model in a polluted environment. Appl. Math. Comput. 2015, 260, 385–396. [Google Scholar] [CrossRef]
- Wu, R.; Zou, X.; Wang, K. Dynamics of logistic systems driven by Lévy noise under regime switching. Electron. J. Differ. Equ. 2014, 2014, 1–16. [Google Scholar]
- Du, Y.; Ye, M.; Zhang, Q. A positivity-preserving numerical algorithm for stochastic age-dependent population system with Lévy noise in a polluted environment. Comput. Math. Appl. Int. J. 2022, 125, 51–79. [Google Scholar] [CrossRef]
- Mao, X.; Yuan, C. Stochastic Differential Equations with Markovian Switching; Imperial College Press: London, UK, 2006. [Google Scholar]
- Luo, Z.; Fan, X. Optimal control for an age-dependent competitive species model in a polluted environment. Appl. Math. Comput. 2014, 228, 91–101. [Google Scholar] [CrossRef]
- Yang, H.; Li, X. Explicit approximations for nonlinear switching diffusion systems in finite and infinite horizons. J. Differ. Equ. 2018, 265, 2921–2967. [Google Scholar] [CrossRef]
- Fu, X. On invariant measures and the asymptotic behavior of a stochastic delayed SIRS epidemic model. Phys. A 2019, 523, 1008–1023. [Google Scholar] [CrossRef]
- Mao, X. Stochastic Differential Equations and Applications, 2nd ed.; Horwood Publishing Limited: Chichester, UK, 2008. [Google Scholar]
- Anderson, W.J. Continuous-Time Markov Chains; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Massey, F. The kolmogorov-smirnov test for goodness of fit. J. Am. Stat. Assoc. 1951, 46, 68–78. [Google Scholar] [CrossRef]


| Parameter | Biological Meaning |
|---|---|
| The density of the population | |
| The depuration rate of the toxicant | |
| Toxic substances in organisms | |
| Toxic substances in the environment | |
| The net organismal excretion rate of the toxicant | |
| The mortality rate function of the population | |
| The fertility rate function of the population | |
| The total loss rate of the toxicant from the environment | |
| The net organismal uptake rate of the toxicant from the environment | |
| The diffusion coefficient dependent on a, t, and | |
| The total density of the population at time t | |
| The exogenous total toxicant input into the environment at time t |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, Y.; Wang, Z. Stationary Distribution of Stochastic Age-Dependent Population–Toxicant Model with Markov Switching. Mathematics 2024, 12, 1212. https://doi.org/10.3390/math12081212
Du Y, Wang Z. Stationary Distribution of Stochastic Age-Dependent Population–Toxicant Model with Markov Switching. Mathematics. 2024; 12(8):1212. https://doi.org/10.3390/math12081212
Chicago/Turabian StyleDu, Yanyan, and Zong Wang. 2024. "Stationary Distribution of Stochastic Age-Dependent Population–Toxicant Model with Markov Switching" Mathematics 12, no. 8: 1212. https://doi.org/10.3390/math12081212
APA StyleDu, Y., & Wang, Z. (2024). Stationary Distribution of Stochastic Age-Dependent Population–Toxicant Model with Markov Switching. Mathematics, 12(8), 1212. https://doi.org/10.3390/math12081212







