Pallet Loading Problem: A Case Study in the Automotive Industry Applying a Simplified Mathematical Model
Abstract
1. Introduction
2. Literature Review
2.1. Logistics and Operational Research
2.2. Pallet Optimization Problems
3. Development
3.1. Company Framework
3.2. Optimization Model
- Typology: 100 m3;
- Payload: 25 tons;
- Capacity: 33 Europallets;
- Inner capacity: 13.6 m long and 2.45 m wide.
- The use of mixed pallets is allowed, i.e., pallets with different products and, consequently, with packages of different sizes.
- A package can have six different orientations when placed on a pallet, as it can be rotated around all the X, Y, and Z axes. However, only two are considered due to the type of product transported.
- The maximum pallet volume is 1.152 m3 (maximum truck height of 3 m, overlapping two pallets, a height of 1.5 m per pallet, and includes the height of the euro pallet (0.144 m) with a margin for the handling of the parts inside the truck; a useful height of 1.2 m is assumed).
- A package cannot coincide (overlap) in the same space as another, and each package must be contained entirely within the pallet, with its sides parallel to the sides of the pallet.
- The proportion of the number of packages of a given size to the total number of packages on a complete pallet should be as close as possible to the customer’s specifications (proportion ratio).
- The adopted model only covers the case in which the packages must be placed on the pallet in a single orientation. However, in this case, the packages can rotate, and both orientations should be considered so that it is possible to adopt the model and allow the packages to have different orientations, and therefore be positioned on the pallet with more than one orientation.
- Moreover, it is decided to consider a type of package with a different orientation as a different package. The developed algorithm differs from what is already found in the literature due to its simplicity of use, reliability in application, as well as flexibility in introducing constraints.
3.2.1. Formulation of the Problem According to Beasley [20]
3.2.2. Formulation of the Problem According to Tsai et al. [21]
4. Results and Discussion
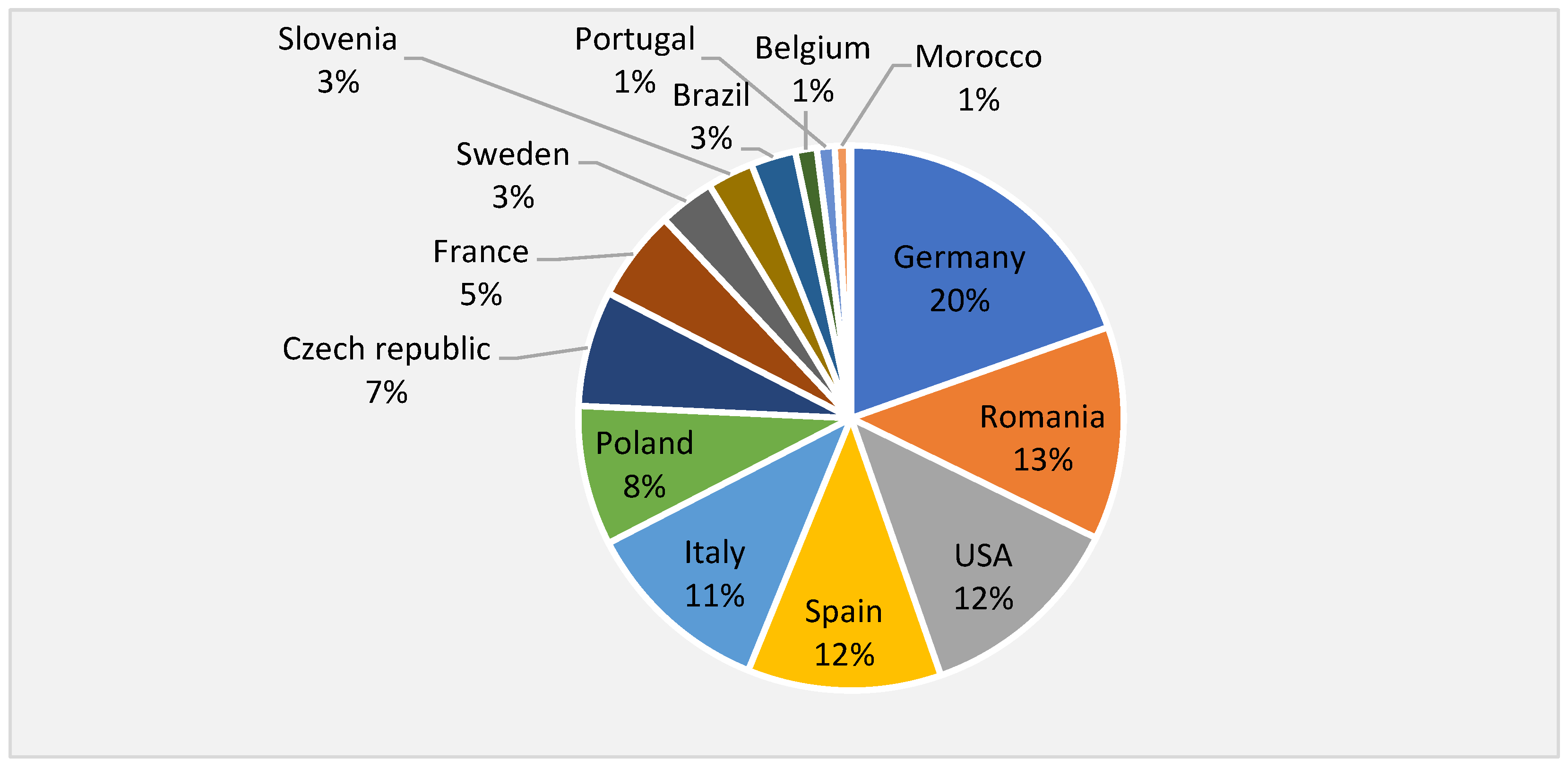
4.1. Characterization of Normal Transport
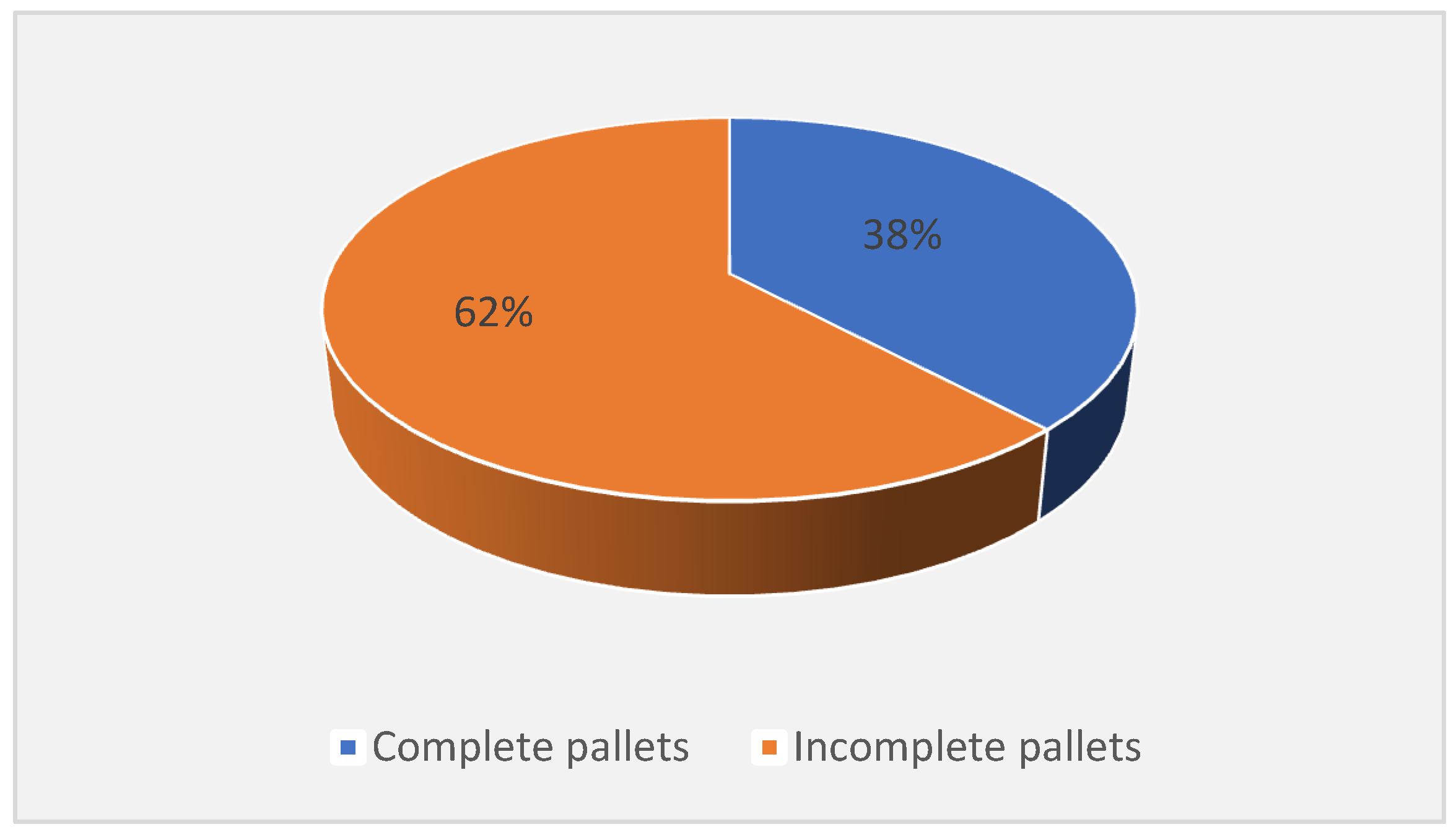
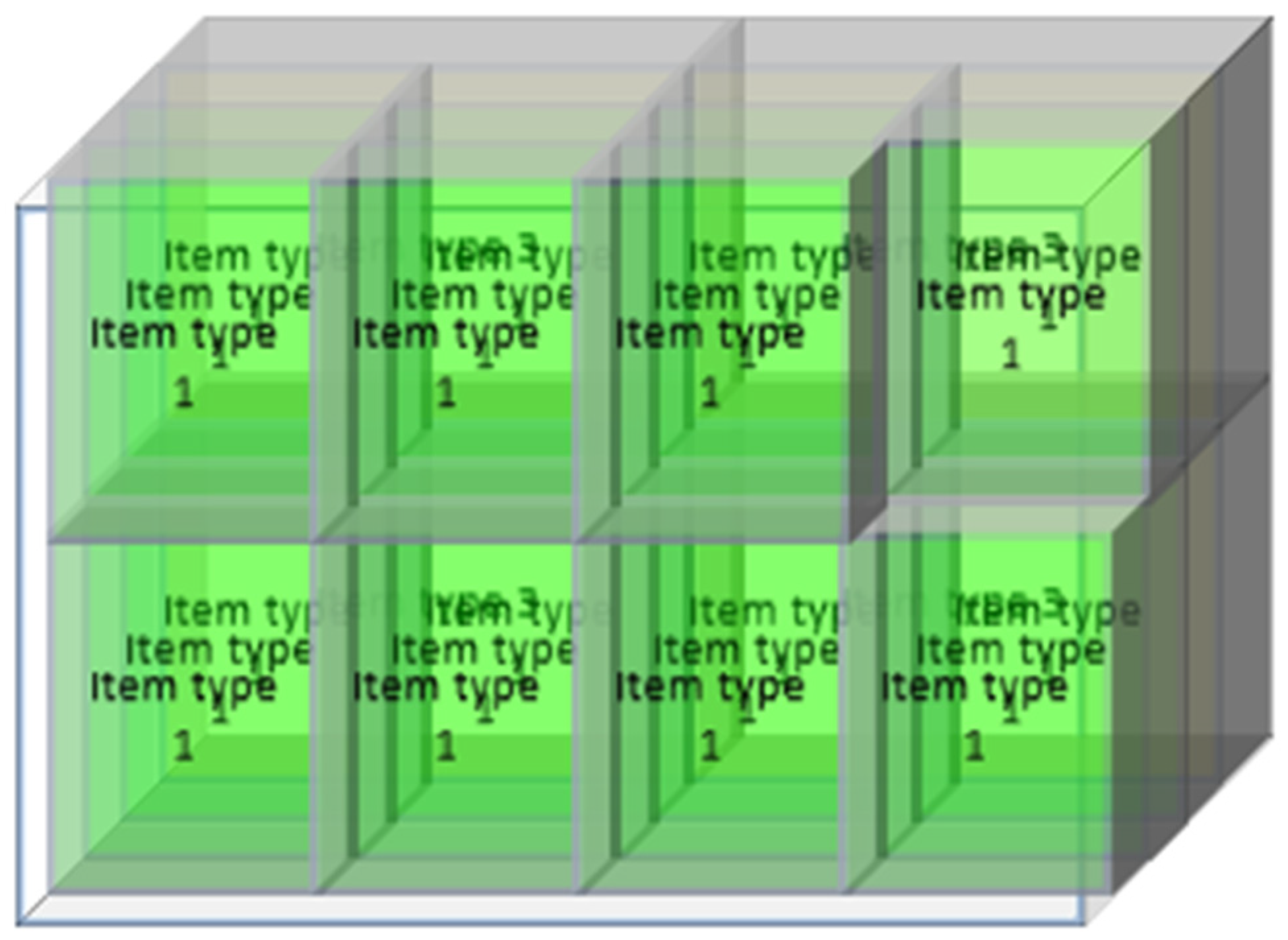
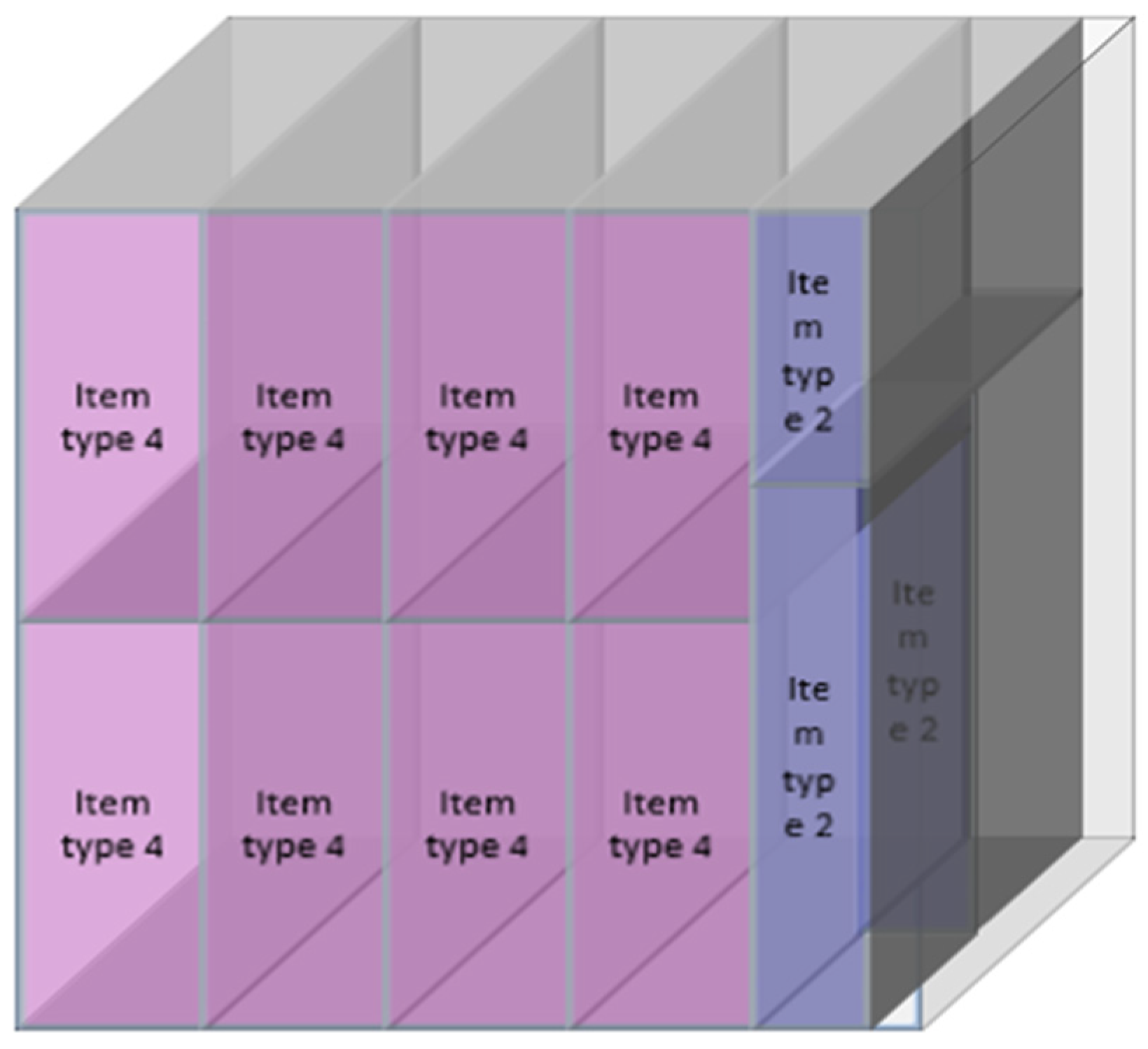
4.2. Pallet Optimization
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yingfei, Y.; Mengze, Z.; Zeyu, L.; Ki-Hyung, B.; Andriandafiarisoa Ralison Ny Avotra, A.; Nawaz, A. Green Logistics Performance and Infrastructure on Service Trade and Environment-Measuring Firm’s Performance and Service Quality. J. King Saud Univ. Sci. 2022, 34, 101683. [Google Scholar] [CrossRef]
- Tan, B.Q.; Wang, F.; Liu, J.; Kang, K.; Costa, F. A Blockchain-Based Framework for Green Logistics in Supply Chains. Sustainability 2020, 12, 4656. [Google Scholar] [CrossRef]
- Lowenberg-DeBoer, J.; Huang, I.Y.; Grigoriadis, V.; Blackmore, S. Economics of Robots and Automation in Field Crop Production. Precis. Agric. 2020, 21, 278–299. [Google Scholar] [CrossRef]
- Ballou, R.H. Business Logistics/Supply Chain Management; Bookman: Clifton, NJ, USA, 2006. [Google Scholar]
- Stevenson, W.J. Operations Management, 11th ed.; McGrawHill: New York, NY, USA, 2009. [Google Scholar]
- Rushton, A.; Croucher, P.; Baker, P. The Handbook of Logistics and Distribution Management, 3rd ed.; Kogan Page: London, UK, 2006. [Google Scholar]
- Chopra, S.; Meindl, P. Supply Chain Management: Strategy, Planning, and Operation, 5th ed.; Pearson: London, UK, 2012. [Google Scholar]
- Li, L. Managing Supply Chain and Logistics; World Scientific: Singapore, 2014. [Google Scholar] [CrossRef]
- Bozart, C.C.; Handfield, R.B. Introduction to Operations and Supply Chain Management, 4th ed.; Pearson: Clifton, NJ, USA, 2015. [Google Scholar]
- Hugos, M. Essentials of Supply Chain Management; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2003. [Google Scholar]
- Machline, C. Cinco Décadas de Logística Empresarial e Administração Da Cadeia de Suprimentos No Brasil. Rev. Adm. Empresas 2011, 51, 227–231. [Google Scholar] [CrossRef]
- Anca, V. Logistics and Supply Chain Management: An Overview. Stud. Bus. Econ. 2019, 14, 209–215. [Google Scholar] [CrossRef]
- Freitas, A.M.; Silva, F.J.G.; Ferreira, L.P.; Sá, J.C.; Pereira, M.T.; Pereira, J. Improving Efficiency in a Hybrid Warehouse: A Case Study. Procedia Manuf. 2019, 38, 1074–1084. [Google Scholar] [CrossRef]
- Garza-Reyes, J.A.; Forero, J.S.B.; Kumar, V.; Villarreal, B.; Cedillo-Campos, M.G.; Rocha-Lona, L. Improving Road Transport Operations Using Lean Thinking. Procedia Manuf. 2017, 11, 1900–1907. [Google Scholar] [CrossRef]
- Blumenfeld, D. Operations Research Calculations Handbook, 2nd ed.; CRC Press Taylor and Francis Group: New York, NY, USA, 2009. [Google Scholar]
- Rardin, R.L. Optimization in Operations Research, 2nd ed.; Pearson: Clifton, NJ, USA, 2017. [Google Scholar]
- Murthy, P.R. Operations Research, 2nd ed.; New Age International Publishers: New Delhi, India, 2007. [Google Scholar]
- Ramalhete, M.; Guerreiro, J.; Magalhães, A. Programação Linear I; McGrawHill: Lisboa, Portugal, 1995. [Google Scholar]
- Hill, M.M.; dos Santos, M.M. Investigação Operacional—Programação Linear, 3rd ed.; Edições Sílabo: Lisboa, Portugal, 2015. [Google Scholar]
- Beasley, J.E. An Exact Two-Dimensional Non-Guillotine Cutting Tree Search Procedure. Oper. Res. 1985, 33, 49–64. [Google Scholar] [CrossRef]
- Tsai, R.D.; Malstom, E.M.; Kuo, W.A.Y. Three Dimensional Palletization of Mixed Box Sizes. IIE Trans. 1993, 25, 64–75. [Google Scholar] [CrossRef]
- Morabito, R.; Morales, S. A Simple and Effective Recursive Procedure for the Manufacturer’s Pallet Loading Problem. J. Oper. Res. Soc. 1998, 49, 819–828. [Google Scholar] [CrossRef]
- Letchford, A.N.; Amaral, A. Analysis of Upper Bounds for the Pallet Loading Problem. Eur. J. Oper. Res. 2001, 132, 582–593. [Google Scholar] [CrossRef]
- Wäscher, G.; Haußner, H.; Schumann, H. An Improved Typology of Cutting and Packing Problems. Eur. J. Oper. Res. 2007, 183, 1109–1130. [Google Scholar] [CrossRef]
- Martins, G.H.A.; Dell, R.F. Solving the Pallet Loading Problem. Eur. J. Oper. Res. 2008, 184, 429–440. [Google Scholar] [CrossRef]
- Zhu, W.; Fu, Y.; Zhou, Y. 3D Dynamic Heterogeneous Robotic Palletization Problem. Eur. J. Oper. Res. 2024, in press. [CrossRef]
- Alvarez-Valdes, R.; Parreño, F.; Tamarit, J.M. A Branch-and-Cut Algorithm for the Pallet Loading Problem. Comput. Oper. Res. 2005, 32, 3007–3029. [Google Scholar] [CrossRef]
- Lu, Y.; Cha, J. A Fast Algorithm for Identifying Minimum Size Instances of the Equivalence Classes of the Pallet Loading Problem. Eur. J. Oper. Res. 2014, 237, 794–801. [Google Scholar] [CrossRef]
- Sheng, L.; Hongxia, Z.; Xisong, D.; Changjian, C. A Heuristic Algorithm for Container Loading of Pallets with Infill Boxes. Eur. J. Oper. Res. 2016, 252, 728–736. [Google Scholar] [CrossRef]
- Singh, M.; Almasarwah, N.; Süer, G. A Two-Phase Algorithm to Solve a 3-Dimensional Pallet Loading Problem. Procedia Manuf. 2019, 39, 1474–1481. [Google Scholar] [CrossRef]
- Terno, J.; Scheithauer, G.; Sommerweiß, U.; Riehme, J. An Efficient Approach for the Multi-Pallet Loading Problem. Eur. J. Oper. Res. 2000, 123, 372–381. [Google Scholar] [CrossRef]
- Ahn, S.; Park, C.; Yoon, K. An Improved Best-First Branch and Bound Algorithm for the Pallet-Loading Problem Using a Staircase Structure. Expert Syst. Appl. 2015, 42, 7676–7683. [Google Scholar] [CrossRef]
- Chan, F.T.S.; Bhagwat, R.; Kumar, N.; Tiwari, M.K.; Lam, P. Development of a Decision Support System for Air-Cargo Pallets Loading Problem: A Case Study. Expert Syst. Appl. 2006, 31, 472–485. [Google Scholar] [CrossRef]
- Arun Prasad, S.; Krishnakumar, P. Higher Order Block Heuristics for 2D Pallet Loading Problems with Multiple Box Inputs. Mater. Today Proc. 2021, 46, 4625–4633. [Google Scholar] [CrossRef]
- Ribeiro, G.M.; Lorena, L.A.N. Lagrangean Relaxation with Clusters and Column Generation for the Manufacturer’s Pallet Loading Problem. Comput. Oper. Res. 2007, 34, 2695–2708. [Google Scholar] [CrossRef]
- Gzara, F.; Elhedhli, S.; Yildiz, B.C. The Pallet Loading Problem: Three-Dimensional Bin Packing with Practical Constraints. Eur. J. Oper. Res. 2020, 287, 1062–1074. [Google Scholar] [CrossRef]
- Mascarenhas, W.F. Two Aspects of the Pallet Loading Problem. Electron. Notes Discret. Math. 2005, 19, 381–387. [Google Scholar] [CrossRef]
- Alonso, M.T.; Alvarez-Valdes, R.; Iori, M.; Parreño, F. Mathematical Models for Multi Container Loading Problems with Practical Constraints. Comput. Ind. Eng. 2019, 127, 722–733. [Google Scholar] [CrossRef]
- Kurpel, D.V.; Scarpin, C.T.; Pécora Junior, J.E.; Schenekemberg, C.M.; Coelho, L.C. The Exact Solutions of Several Types of Container Loading Problems. Eur. J. Oper. Res. 2020, 284, 87–107. [Google Scholar] [CrossRef]
- Silva, F.G.; Gouveia, R.M. Cleaner Production—Toward a Better Future; Springer Nature: Cham, Switzerland, 2020. [Google Scholar]
- Ronzoni, M.; Accorsi, R.; Guidani, B.; Manzini, R. Economic and Environmental Optimization of Packaging Containers Choice in Food Catering Supply Chain. Transp. Res. Procedia 2022, 67, 163–171. [Google Scholar] [CrossRef]
- Resat, H.G.; Unsal, B. A Novel Multi-Objective Optimization Approach for Sustainable Supply Chain: A Case Study in Packaging Industry. Sustain. Prod. Consum. 2019, 20, 29–39. [Google Scholar] [CrossRef]
- Vitale, I.; Dondo, R.G.; González, M.; Cóccola, M.E. Modelling and Optimization of Material Flows in the Wood Pellet Supply Chain. Appl. Energy 2022, 313, 118776. [Google Scholar] [CrossRef]
- Sharifi, E.; Fang, L.; Amin, S.H. A Novel Two-Stage Multi-Objective Optimization Model for Sustainable Soybean Supply Chain Design under Uncertainty. Sustain. Prod. Consum. 2023, 40, 297–317. [Google Scholar] [CrossRef]
- Jiao, G.; Huang, M.; Song, Y.; Li, H.; Wang, X. Container Loading Problem Based on Robotic Loader System: An Optimization Approach. Expert Syst. Appl. 2024, 236, 121222. [Google Scholar] [CrossRef]


| Author | Specific Problem | Solution |
|---|---|---|
| Zhu et al. [26] | Manual handling of heterogeneous carton boxes | The work proposes an automated robotic packaging system, with the limitation of dynamic heterogeneous robotic palletizing (DHRP). Thus, an algorithm was developed that would calculate the collision-free trajectories of the robotic arm, as well as guarantee online decision-making. The authors adopted the Monte Carlo simulation strategy. It was possible to verify that the algorithm was functional with effective decisions regarding box packaging, as well as selecting the most appropriate configuration. |
| Alvarez-Valdes et al. [27] | PLP with up to 100 boxes | Aiming at optimization with up to 100 boxes, the authors developed a branch-and-cut algorithm, in which they adapted Beasley’s 0–1 formulation to the PLP. It was possible to approach 40,609 equivalences based on the algorithm. |
| Lu et al. [28] | Minimum size instance (MSI) | The authors presented an algorithm that identified the minimum size instance (MSI), which was equivalent to the pallet loading problem (PLP), since in both cases, the equivalence indicates that the proportions of their items are within a range open of real numbers. Furthermore, the explored method can also be useful for transforming a non-integer PLP instance into an integer MSI. The resulting algorithm is simpler when compared to previous algorithms and has decreased the time complexity by two polynomial orders. |
| Sheng et al. [29] | Container loading problem at furniture factories | In this work, the authors aimed to maximize the volume of products that was placed in containers, and these products, before being organized in the containers, are arranged on pallets. Pallets must be complete before shipping. Thus, a heuristic algorithm was developed, which encompasses a tree search sub-algorithm, which aims to organize the pallets in the container, and a greedy sub-algorithm, which aims to fill smaller spaces. |
| Singh et al. [30] | Three-dimensional PLP while considering humidity and storage time | In this work, a two-phase algorithm was proposed: (1) boxes must fill the pallet in its entirety, and (2) calculation of horizontal layers that must be placed on the pallet based on maximum height, maximum weight, and dynamic compression resistance. The result was viable by maximizing the number of boxes per pallet. |
| Terno et al. [31] | Multi-pallet loading problem | In this work, the authors developed a heuristic through the three-dimensional (3D) solution approach, using the two-dimensional (2D) loading strategy, which considers loading in layers. Computational analyses were carried out to confirm the method. |
| Ahn et al. [32] | Maximize the number of identical boxes | In this work, an algorithm was proposed that generated a ladder structure, which proved to be highly efficient through demonstrated computational results, since the layout generated through the ladder structure removes patterns that are not necessary, as well as defining a limit for standards. |
| Chan et al. [33] | Air-cargo loading problem (ACLP) | In this work, a decision support system (DSS) was used, which consisted of two phases: (1) linear programming (LP) model to define the limits of both cost and maximum load, and (2) a heuristic that generates the loading configuration plan and confirms whether it is the most appropriate. A total of 54 different classes were analyzed from a total of 671 cargo boxes, which shows the high efficiency of the method in its full complexity. |
| Prasad et al. [34] | Maximum allowable number of input box types is 4 | As the restriction of this PLP is the maximum number of input boxes, the authors focused on how the block should be configured using non-guillotine block heuristics. In addition, higher-order block heuristics (more than four non-guillotine cuts—second order) with input from single box to multiple boxes were considered. Thus, the possibility of obtaining an arrangement of patterns with more than four different types of boxes was verified. On the other hand, in the second order, no differentiated solution was found. |
| Ribeiro et al. [35] | Lagrangian relaxation with clusters | In this work, the authors considered the manufacturer’s pallet loading problem (MPLP), approaching this problem through a new Lagrangian relaxation with clusters, in which it was possible to verify with computational tests that the approach is successful and can reach the optimum point. |
| Martins et al. [25] | PLP while maximizing the number of boxes placed on a rectangular pallet | In the work in question, new limits, heuristics, and an algorithm for the PLP were presented. Based on the area ratio and considering the set of all instances, 3,080,730 classes were possible. Through the G5-heuristic, the ideal solutions were found for 3,073,724, another three heuristics found another 54 instances, and with the developed algorithm, the remaining ones were found. |
| Gzara et al. [36] | Three-dimensional bin packing problem (3DBPP) | In their work, the authors addressed a three-dimensional packaging problem (3DBPP), considering vertical support, load support, planogram sequencing and weight limits, through a layered column generation method, second-order cone programming, and graphical representation to track load distribution. The computational tests resulted in a high quality of the methodology developed. |
| Mascarenhas [37] | Classical combinatorial optimization problem | Focusing on new ideas for PLP, the author used elementary number theory and duality limits to propose an algorithm capable of solving the standard and classic pallet loading problem. |
| Project | Customer | Pallet Dimension [mm] | Package Dimension [mm] |
|---|---|---|---|
| B1 (23XX520XL1) | 12226 | 1200 × 800 × 800 | 600 × 400 × 200 |
| 12259 | 1200 × 800 × 800 | 600 × 400 × 200 | |
| 1200 × 800 × 1000 | 600 × 400 × 200 | ||
| 1200 × 1000 × 1000 | 600 × 400 × 200 | ||
| 1000 × 190 × 200 | |||
| A1 (KIEV408XL1) | 14701 | 800 × 600 × 240 | 400 × 300 × 120 |
| 1200 × 800 × 900 | 800 × 400 × 150 | ||
| 1200 × 800 × 750 | 600 × 400 × 150 | ||
| 800 × 400 × 150 | |||
| 1200 × 800 × 735 | 800 × 600 × 245 | ||
| 1200 × 800 × 600 | 600 × 400 × 150 | ||
| 400 × 300 × 120 | |||
| 1200 × 800 × 1000 | 1200 × 190 × 200 |
| Indices | → Index for different types of package; p, s → Index for positions on the X-axis; q, t → Index for positions on the Y-axis; r, u → Index for positions on the Z-axis. |
| Parameters | S → A set of n packages to be considered; m → Total number of package types; Vi → Volume of package i; Qi → Maximum number of replicas of the box i that can be packed; (li, wi, hi) → Dimensions of package i; (L, W, H) → Pallet dimensions; aipqrstu → It is a binary function that takes the value 1 to indicate that the position (s, t, u) is occupied by the package of type i when the lower-left–front corner is positioned in the position (p, q, r) in the pallet. It takes the value 0 if that position is not occupied by the package. |
| Decision Variable |
| Indices | i, j → Index for packages. |
| Parameters | m → Total number of packages available; Vk → Volume of package k; (li, wi, hi) → Dimension of package i; (L, W, H) → Maximum pallet dimensions; (X°, Y°, Z°) → Position of the lower-front–left corner of the pallet in space. Note: These values must be large enough so that packages that do not fit on the pallet fit on the fictitious pallet (X°, Y°, Z°). |
| Decision Variable | Pk is a binary decision variable associated with the k-th package in the set S (xi, yi, zi) → Variables indicating the position of the lower-front–left corner of package i to the X, Y, and Z axes, i = 1, …, m. |
| Pallet Designation | Available Pallets Dimensions [mm] | Package Designation | Available Package Dimensions | Weekly Search |
|---|---|---|---|---|
| Pallet 1 | 800 × 600 × 1200 | Box A or Item type 1 | 400 × 300 × 120 | 24 |
| Pallet 2 | 1200 × 800 × 1200 | Box B or Item type 2 | 800 × 400 × 150 | 19 |
| Box C or Item type 3 | 600 × 400 × 150 | 20 | ||
| Box D or Item type 4 | 800 × 600 × 245 | 18 | ||
| Box F or Item type 5 | 1200 × 190 × 200 | 12 |
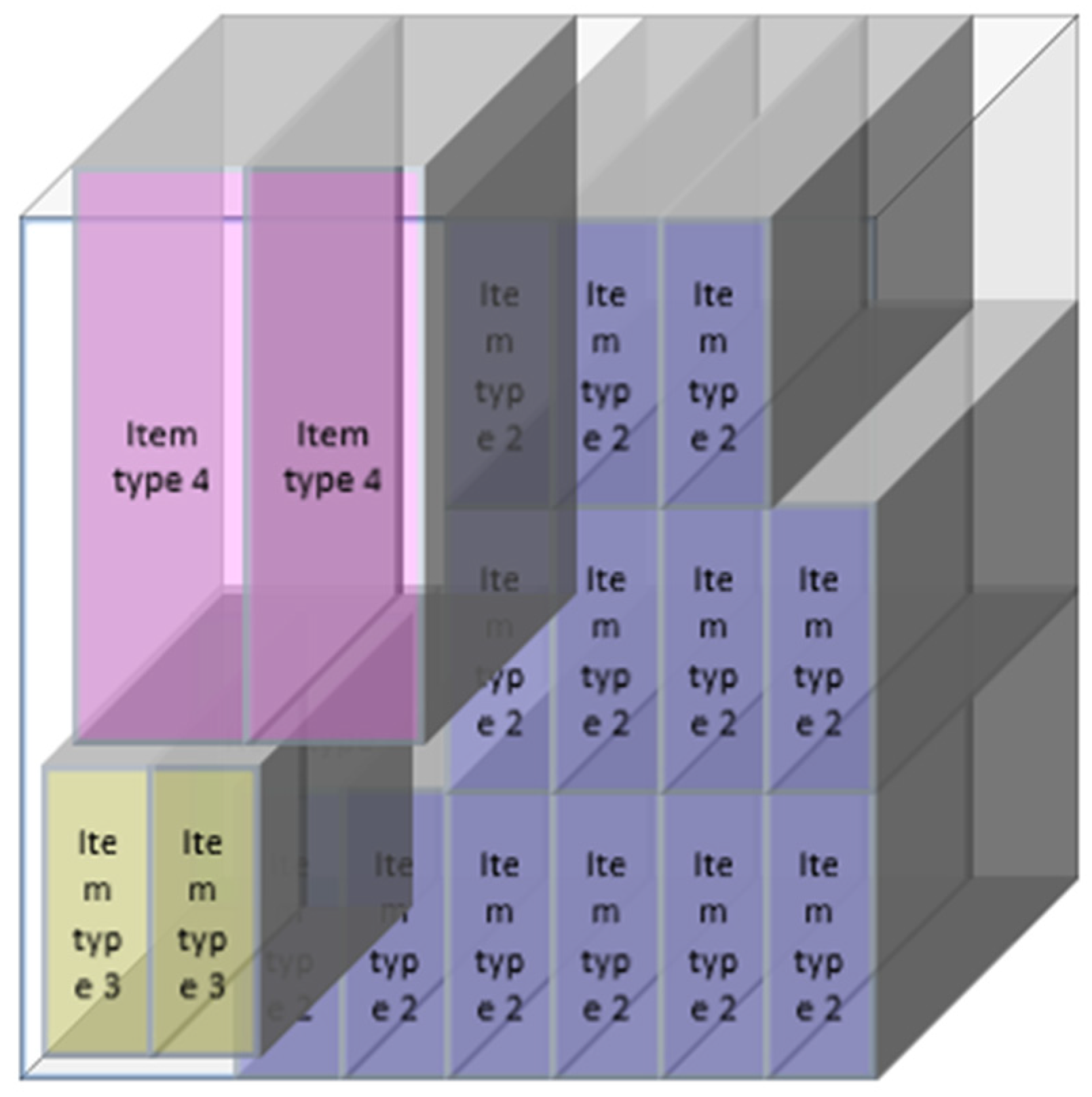
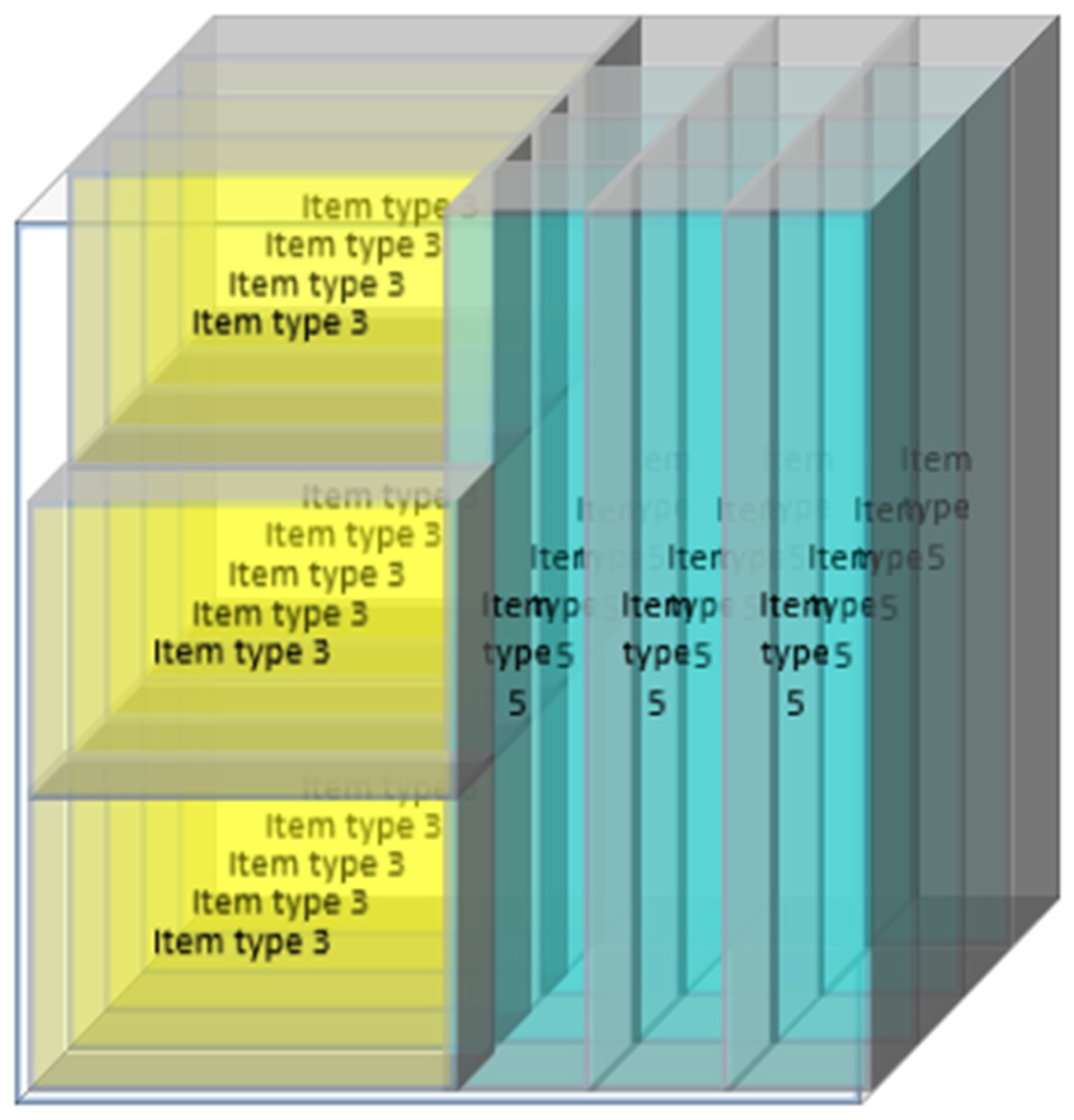
| Quantity of Pallets | Type of Pallets | Type of Box | Quantity of Packages |
|---|---|---|---|
| 1 | Pallet 1 | Package A | 23 |
| Package C | 4 | ||
| 2 | Pallet 2 | Package B | 3 |
| Package D | 8 | ||
| 1 | Pallet 2 | Package C | 14 |
| Package E | 12 | ||
| 1 | Pallet 2 | Package A | 1 |
| Package B | 13 | ||
| Package C | 2 | ||
| Package D | 2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sebbe, N.P.V.; Silva, F.J.G.; Barreiras, A.M.S.; Pinto, I.M.; Sales-Contini, R.C.M.; Ferreira, L.P.; Machado, A.B.M. Pallet Loading Problem: A Case Study in the Automotive Industry Applying a Simplified Mathematical Model. Mathematics 2024, 12, 984. https://doi.org/10.3390/math12070984
Sebbe NPV, Silva FJG, Barreiras AMS, Pinto IM, Sales-Contini RCM, Ferreira LP, Machado ABM. Pallet Loading Problem: A Case Study in the Automotive Industry Applying a Simplified Mathematical Model. Mathematics. 2024; 12(7):984. https://doi.org/10.3390/math12070984
Chicago/Turabian StyleSebbe, Naiara P. V., Francisco J. G. Silva, Alcinda M. S. Barreiras, Isabel M. Pinto, Rita C. M. Sales-Contini, Luis P. Ferreira, and Ana B. M. Machado. 2024. "Pallet Loading Problem: A Case Study in the Automotive Industry Applying a Simplified Mathematical Model" Mathematics 12, no. 7: 984. https://doi.org/10.3390/math12070984
APA StyleSebbe, N. P. V., Silva, F. J. G., Barreiras, A. M. S., Pinto, I. M., Sales-Contini, R. C. M., Ferreira, L. P., & Machado, A. B. M. (2024). Pallet Loading Problem: A Case Study in the Automotive Industry Applying a Simplified Mathematical Model. Mathematics, 12(7), 984. https://doi.org/10.3390/math12070984











