Numerical Investigation of Double-Diffusive Convection in an Irregular Porous Cavity Subjected to Inclined Magnetic Field Using Finite Element Method
Abstract
1. Introduction
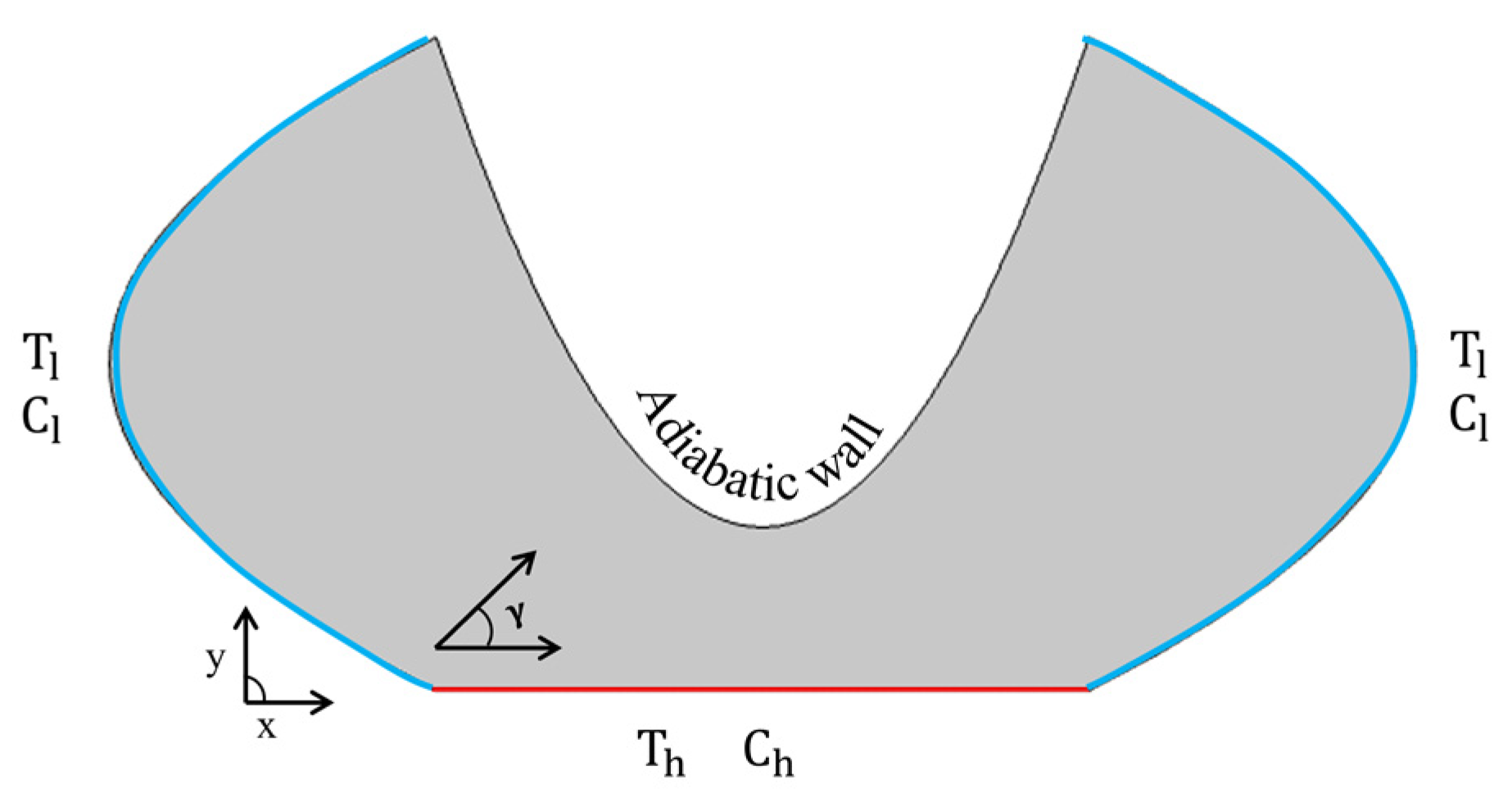
2. Mathematical Formulation
Governing Equations
| Dimensionless parameter | Definition |
| Rayleigh number | |
| Lewis number | |
| Prandtl number | |
| Darcy number | |
| Hartmann number | |
| Temperature | |
| Fluid pressure | |
| Velocity |
| Cold wall | , | |
| Hot wall | , | |
| Remaining wall | . |
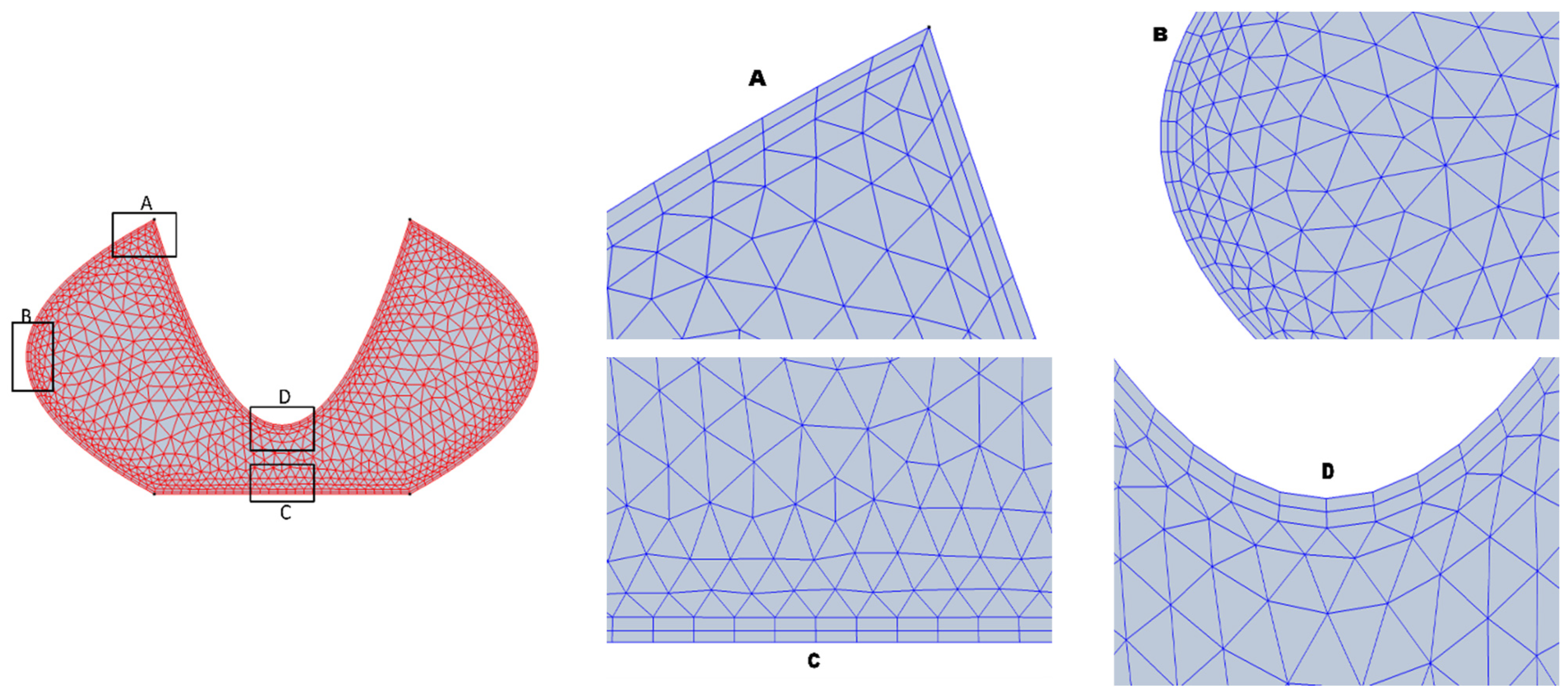
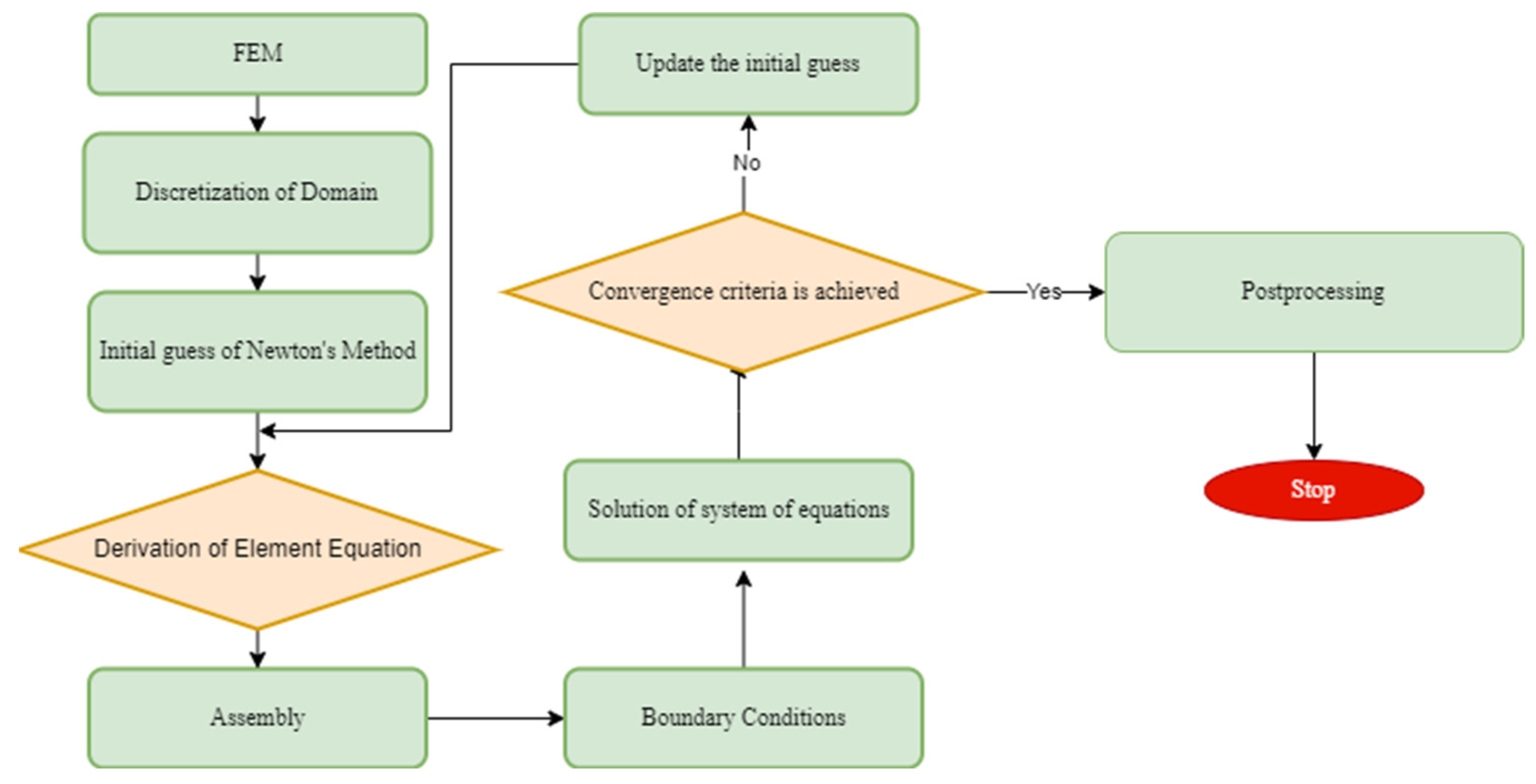
3. Numerical Methodology
Grid Convergence
4. Result and Discussions
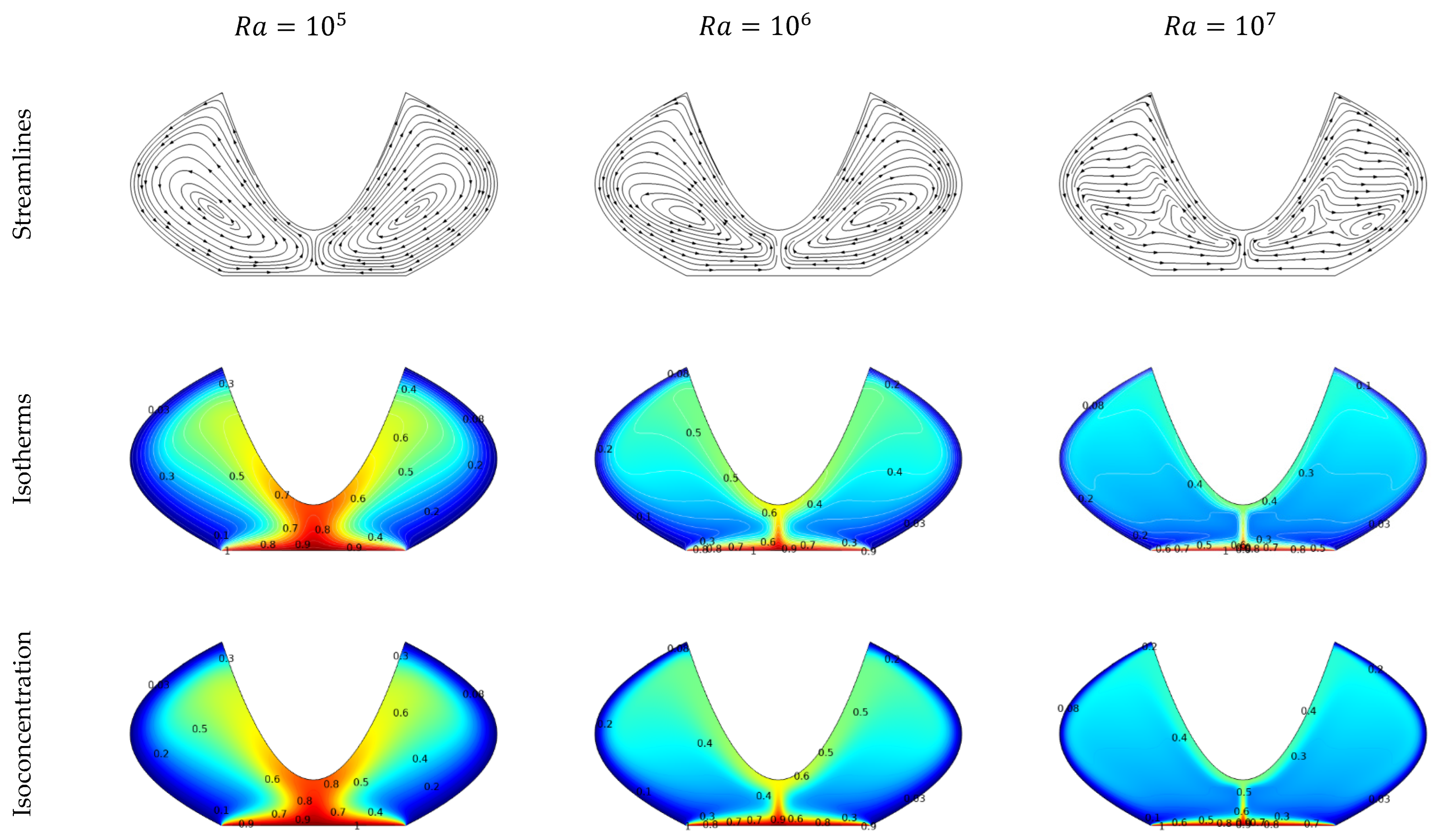
4.1. Impact of Rayleigh’s Number
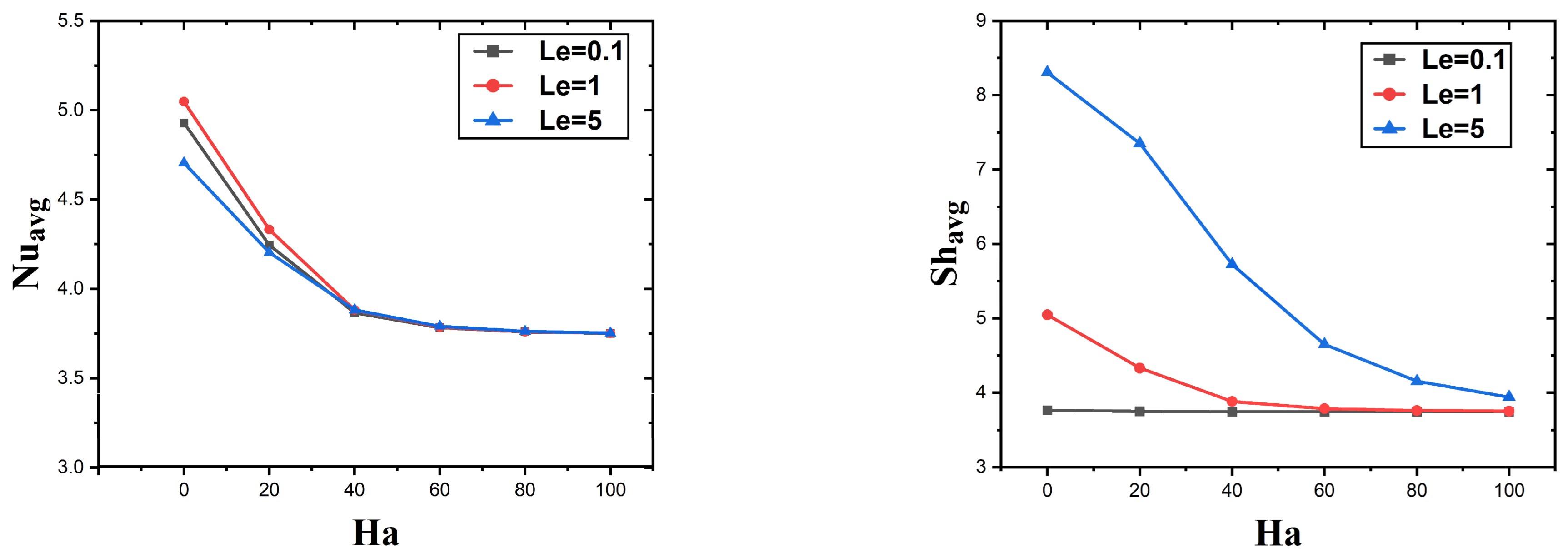
4.2. Impact of Lewis’s Number
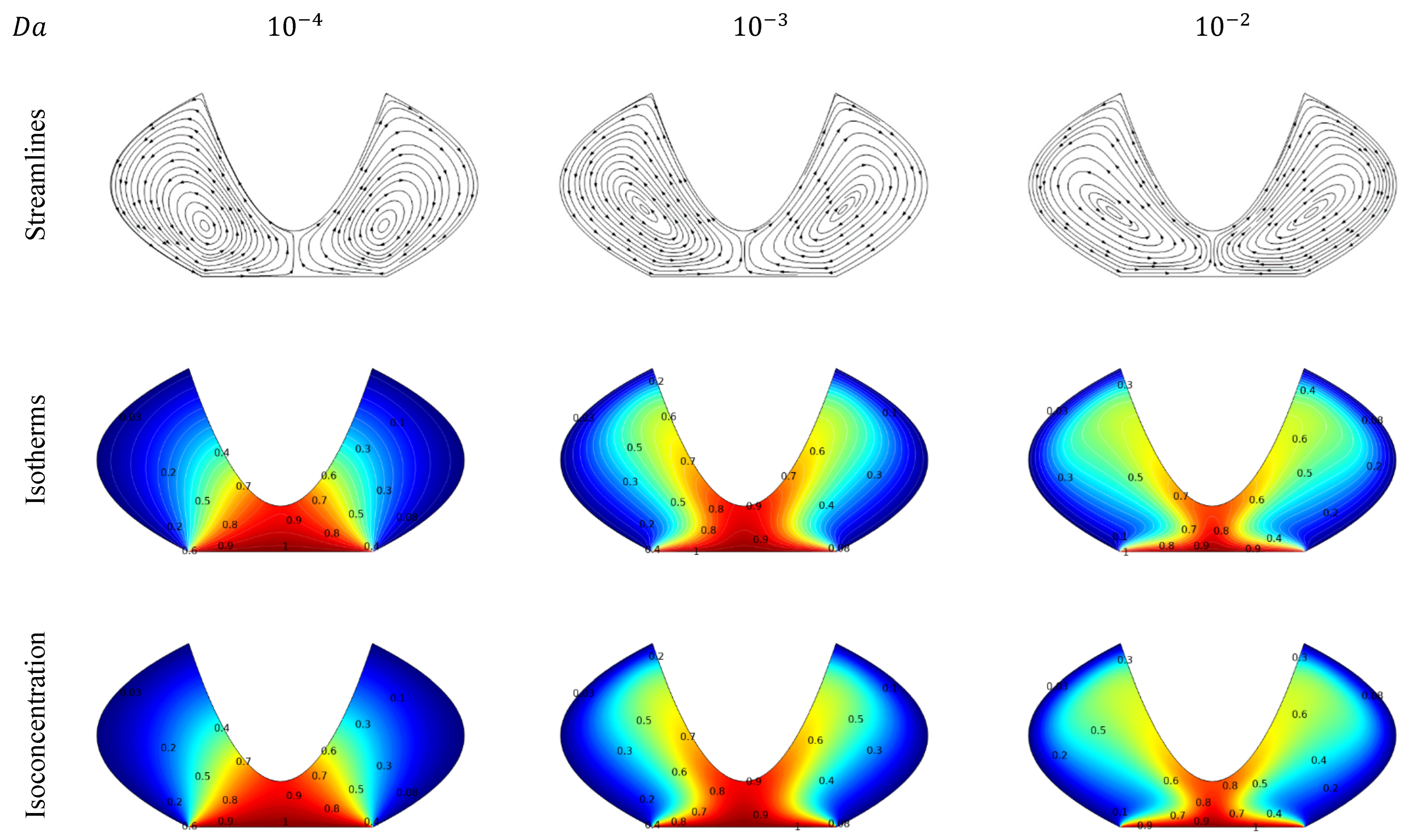
4.3. Impact of Darcy’s Number
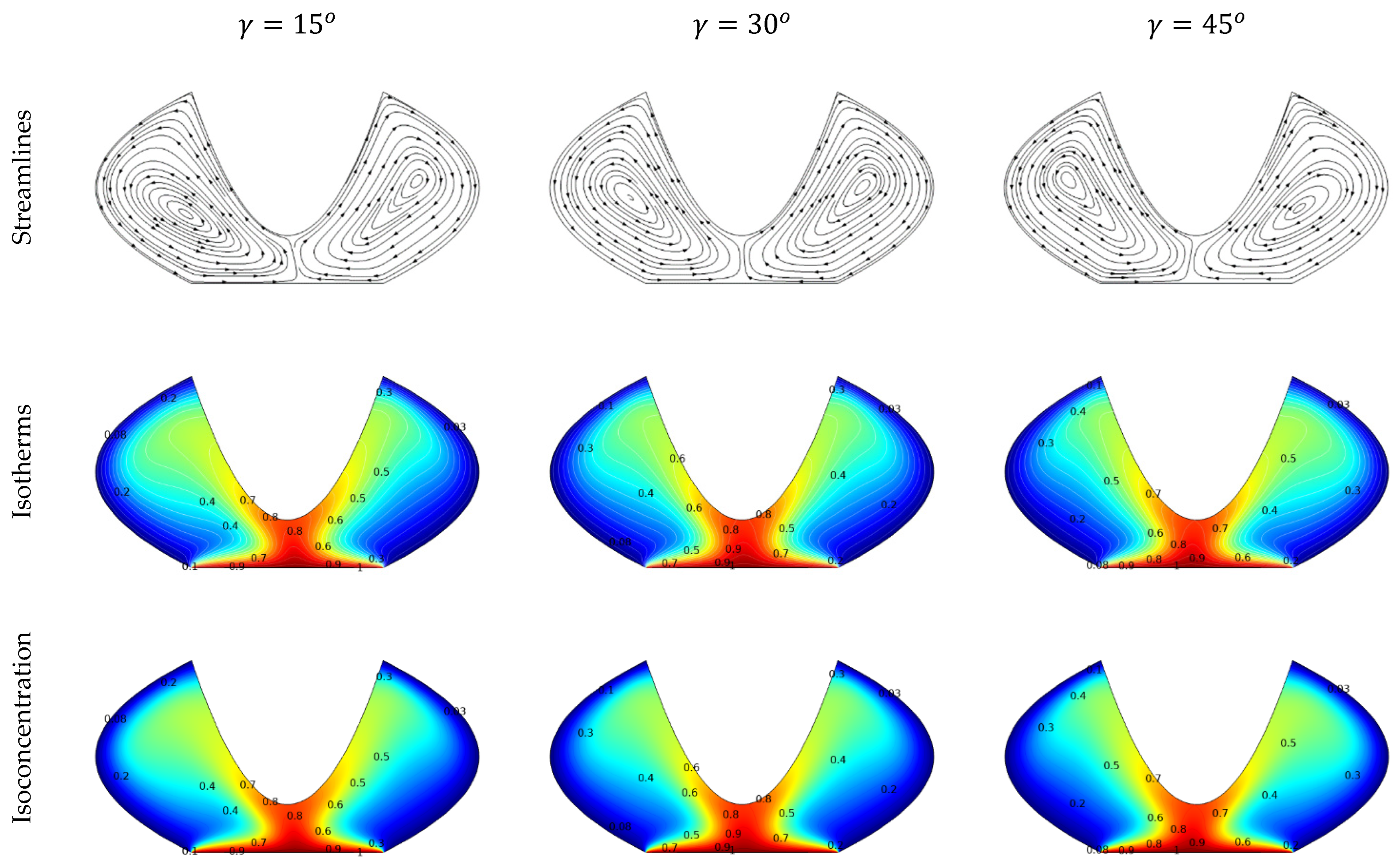
4.4. Impact of Inclined Angle
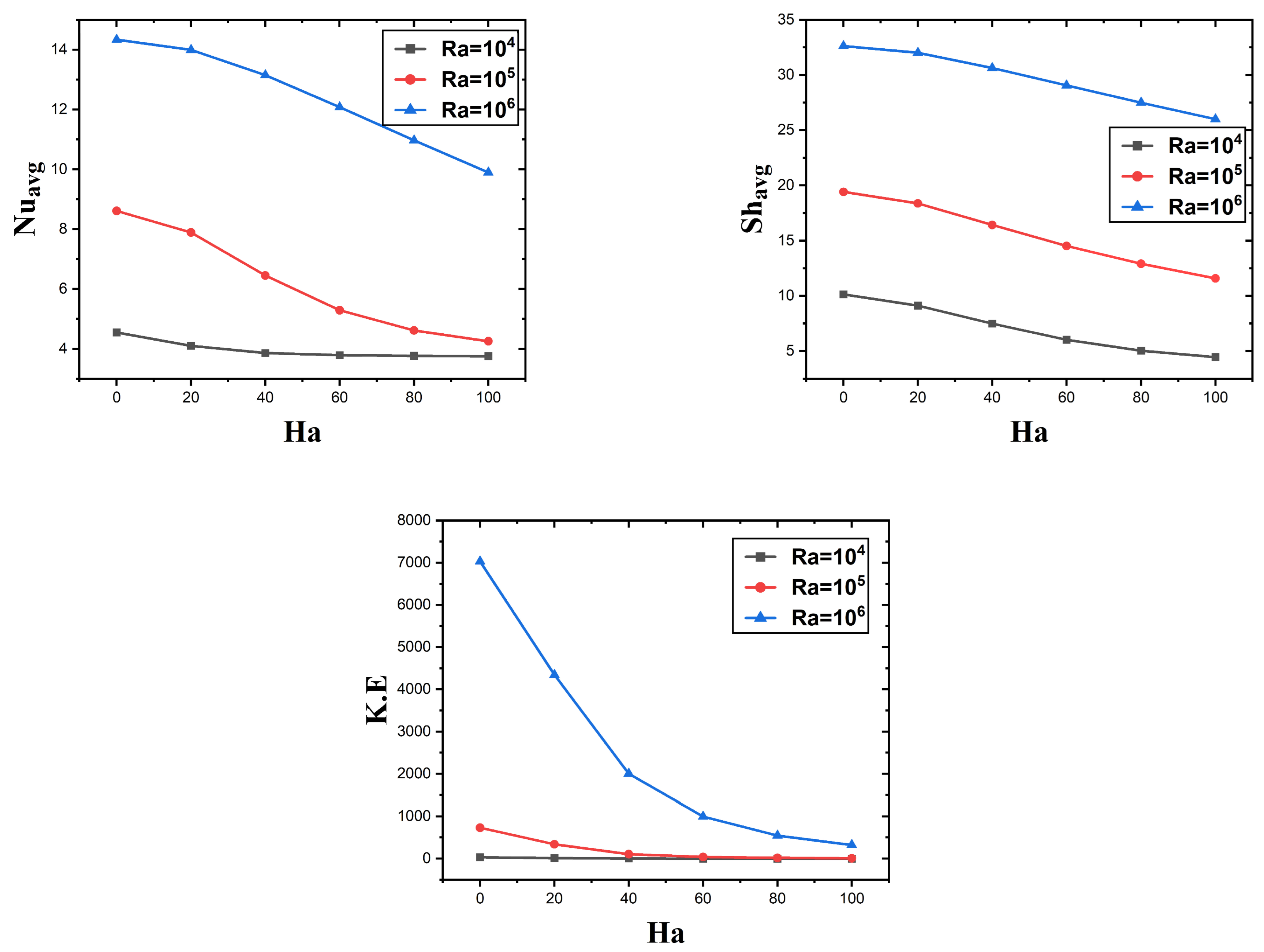
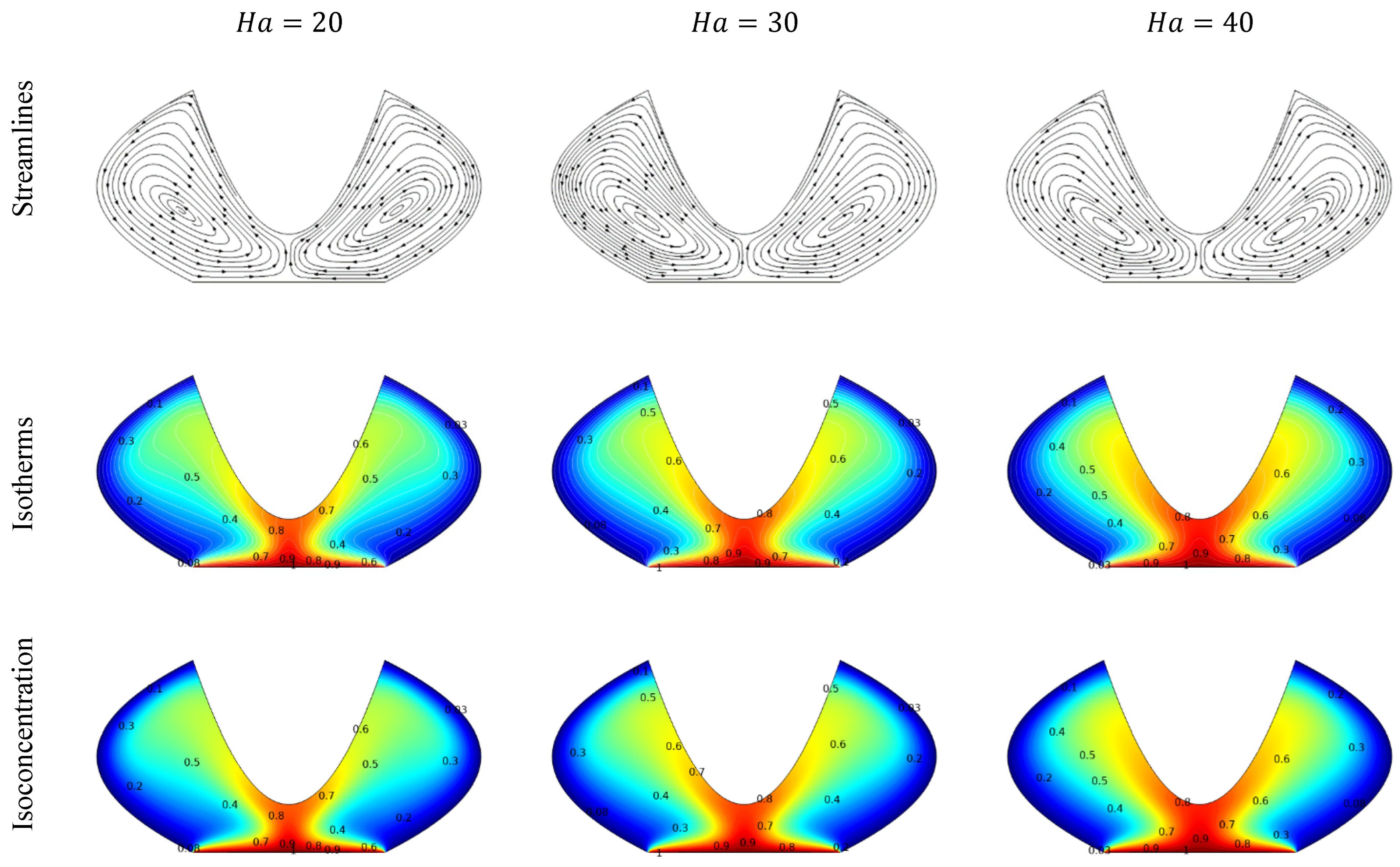
4.5. Impact of Hartmann’s Number
5. Conclusions
- The heat transfer rate, mass transfer rate, and total kinetic energy increase with the rise in the Rayleigh number.
- In a specific range, , when the Lewis number rises, the mean Nusselt number and total energy decrease, but the mean Sherwood number increases.
- As the Rayleigh number increases, the isotherms and isoconcentration lines become more distorted, the concentration and temperature gradients become steeper. With the formation of plumes and vortices, the flow becomes more turbulent and complex.
- The findings indicate that the velocity of fluid flow decreases because of porous media flow resistance increasing the Darcy number. The velocity lines of the fluid become more densely packed, resulting in a more laminar flow.
- , , and K.E. increase when the Darcy number rises.
- The flow pattern within the cavity can be described by a series of counterclockwise convection vortices, and temperatures rise on hot walls with the increases in the inclined angle.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Magnetic field strength (Tesla) | N | Buoyancy ratio | |
| Magnetic field (Tesla) | Number of elements | ||
| Specific heat | Fluid pressure | ||
| Concentration | Prandtl’s number | ||
| Da | Darcy’s number | Rayleigh’s number | |
| Degree of freedom | Sherwood’s number (local) | ||
| Gravity (m/s) | Temperature (K) | ||
| Hartmann’s number | , | Velocity (x and y axis) | |
| K.E | Total kinetic energy (J) | , | x and y coordinate (m) |
| Lewis’s number | Angle (inclination) (rad) | ||
| Kinematic viscosity | Fluid density | ||
| Nusselt number (local) | Temperature |
References
- Turner, J. Double-diffusive phenomena. Annu. Rev. Fluid Mech. 1974, 6, 37–54. [Google Scholar] [CrossRef]
- Schmitt, R.W. Double diffusion in oceanography. Annu. Rev. Fluid Mech. 1994, 26, 255–285. [Google Scholar] [CrossRef]
- Ha, S.H.; Kim, G.T. Heat transfer study of double diffusive natural convection in a two-dimensional enclosure at different aspect ratios and thermal Grashof number during the physical vapor transport of mercurous bromide (Hg2Br2): Part I. Heat transfer. J. Korean Cryst. Growth Cryst. Technol. 2022, 32, 16–24. [Google Scholar]
- Kelley, D.; Fernando, H.; Gargett, A.; Tanny, J.; Özsoy, E. The diffusive regime of double-diffusive convection. Prog. Oceanogr. 2003, 56, 461–481. [Google Scholar] [CrossRef]
- Gireesha, B.; Archana, M.; Prasannakumara, B.; Gorla, R.R.; Makinde, O.D. MHD three dimensional double diffusive flow of Casson nanofluid with buoyancy forces and nonlinear thermal radiation over a stretching surface. Int. J. Numer. Methods Heat Fluid Flow 2017, 27, 2858–2878. [Google Scholar] [CrossRef]
- Serrano-Arellano, J.; Gijón-Rivera, M.; Riesco-Ávila, J.; Elizalde-Blancas, F. Numerical study of the double diffusive convection phenomena in a closed cavity with internal CO2 point sources. Int. J. Heat Mass Transf. 2014, 71, 664–674. [Google Scholar] [CrossRef]
- VijayaVenkataRaman, S.; Iniyan, S.; Goic, R. A review of solar drying technologies. Renew. Sustain. Energy Rev. 2012, 16, 2652–2670. [Google Scholar] [CrossRef]
- Alao, F.; Fagbade, A.; Falodun, B. Effects of thermal radiation, Soret and Dufour on an unsteady heat and mass transfer flow of a chemically reacting fluid past a semi-infinite vertical plate with viscous dissipation. J. Niger. Math. Soc. 2016, 35, 142–158. [Google Scholar] [CrossRef]
- Esfe, M.H.; Arani, A.A.A.; Yan, W.-M.; Ehteram, H.; Aghaie, A.; Afrand, M. Natural convection in a trapezoidal enclosure filled with carbon nanotube–EG–water nanofluid. Int. J. Heat Mass Transf. 2016, 92, 76–82. [Google Scholar] [CrossRef]
- Khan, Z.H.; Khan, W.A.; Haq, R.; Usman, M.; Hamid, M. Effects of volume fraction on water-based carbon nanotubes flow in a right-angle trapezoidal cavity: FEM based analysis. Int. Commun. Heat Mass Transf. 2020, 116, 104640. [Google Scholar] [CrossRef]
- Samadifar, M.; Toghraie, D. Numerical simulation of heat transfer enhancement in a plate-fin heat exchanger using a new type of vortex generators. Appl. Therm. Eng. 2018, 133, 671–681. [Google Scholar] [CrossRef]
- Haq, R.U.; Shah, S.S.; Algehyne, E.A. Thermal drift and force convection analysis of nanofluid due to partially heated triangular fins in a porous circular enclosure. Phys. Scr. 2021, 96, 065701. [Google Scholar] [CrossRef]
- Soomro, F.A.; Haq, R.U.; Algehyne, E.A.; Tlili, I. Thermal performance due to magnetohydrodynamics mixed convection flow in a triangular cavity with circular obstacle. J. Energy Storage 2020, 31, 101702. [Google Scholar] [CrossRef]
- Mohammadi, M.; Nassab, S.G. Double-diffusive convection flow with Soret and Dufour effects in an irregular geometry in the presence of thermal radiation. Int. Commun. Heat Mass Transf. 2022, 134, 106026. [Google Scholar] [CrossRef]
- Haq, R.U.; Soomro, F.A.; Wang, X.; Tlili, I. Partially heated lid-driven flow in a hexagonal cavity with inner circular obstacle via FEM. Int. Commun. Heat Mass Transf. 2020, 117, 104732. [Google Scholar] [CrossRef]
- Izadi, S.; Armaghani, T.; Ghasemiasl, R.; Chamkha, A.J.; Molana, M. A comprehensive review on mixed convection of nanofluids in various shapes of enclosures. Powder Technol. 2019, 343, 880–907. [Google Scholar] [CrossRef]
- Bejan, A.; Khair, K.R. Heat and mass transfer by natural convection in a porous medium. Int. J. Heat Mass Transf. 1985, 28, 909–918. [Google Scholar] [CrossRef]
- Goyeau, B.; Songbe, J.-P.; Gobin, D. Numerical study of double-diffusive natural convection in a porous cavity using the Darcy-Brinkman formulation. Int. J. Heat Mass Transf. 1996, 39, 1363–1378. [Google Scholar] [CrossRef]
- Mondal, S.; Sibanda, P. Effects of buoyancy ratio on unsteady double-diffusive natural convection in a cavity filled with porous medium with non-uniform boundary conditions. Int. J. Heat Mass Transf. 2015, 85, 401–413. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Al-Mudhaf, A. Double-diffusive natural convection in inclined porous cavities with various aspect ratios and temperature-dependent heat source or sink. Heat Mass Transf. 2008, 44, 679–693. [Google Scholar] [CrossRef]
- He, B.; Lu, S.; Gao, D.; Chen, W.; Lin, F. Lattice Boltzmann simulation of double diffusive natural convection in heterogeneously porous media of a fluid with temperature-dependent viscosity. Chin. J. Phys. 2020, 63, 186–200. [Google Scholar] [CrossRef]
- Vijaybabu, T. Influence of porous circular cylinder on MHD double-diffusive natural convection and entropy generation. Int. J. Mech. Sci. 2021, 206, 106625. [Google Scholar] [CrossRef]
- Astanina, M.S.; Sheremet, M.A. Numerical study of natural convection of fluid with temperature-dependent viscosity inside a porous cube under non-uniform heating using local thermal non-equilibrium approach. Int. J. Thermofluids 2023, 17, 100266. [Google Scholar] [CrossRef]
- Rashid, U.; Shahzad, H.; Lu, D.; Wang, X.; Majeed, A.H. Non-Newtonian MHD double diffusive natural convection flow and heat transfer in a crown enclosure. Case Stud. Therm. Eng. 2023, 41, 102541. [Google Scholar] [CrossRef]
- Rahman, M.; Saidur, R.; Rahim, N. Conjugated effect of joule heating and magneto-hydrodynamic on double-diffusive mixed convection in a horizontal channel with an open cavity. Int. J. Heat Mass Transf. 2011, 54, 3201–3213. [Google Scholar] [CrossRef]
- Teamah, M.A. Numerical simulation of double diffusive natural convection in rectangular enclosure in the presences of magnetic field and heat source. Int. J. Therm. Sci. 2008, 47, 237–248. [Google Scholar] [CrossRef]
- Kumar, V.; Murthy, S.K.; Kumar, B.R. Influence of MHD forces on Bejan’s heatlines and masslines in a doubly stratified fluid saturated Darcy porous enclosure in the presence of Soret and Dufour effects—A numerical study. Int. J. Heat Mass Transf. 2018, 117, 1041–1062. [Google Scholar] [CrossRef]
- Bayareh, M.; Baghoolizadeh, M. An overview of the magnetic field effect on heat transfer and entropy generation in cavities: Application of the second law of thermodynamics and artificial intelligence. Int. Commun. Heat Mass Transf. 2024, 151, 107238. [Google Scholar] [CrossRef]
- Seyyedi, S.M.; Dogonchi, A.; Hashemi-Tilehnoee, M.; Waqas, M.; Ganji, D. Investigation of entropy generation in a square inclined cavity using control volume finite element method with aided quadratic Lagrange interpolation functions. Int. Commun. Heat Mass Transf. 2020, 110, 104398. [Google Scholar] [CrossRef]
- Ali, M.M.; Alim, M.; Ahmed, S.S. Magnetohydrodynamic mixed convection flow in a hexagonal enclosure. J. Procedia Eng. 2017, 194, 479–486. [Google Scholar] [CrossRef]
- Haq, R.U.; Soomro, F.A.; Mekkaoui, T.; Al-Mdallal, Q.M. MHD natural convection flow enclosure in a corrugated cavity filled with a porous medium. Int. J. Heat Mass Transf. 2018, 121, 1168–1178. [Google Scholar] [CrossRef]
- Hussain, S.; Shoeibi, S.; Armaghani, T. Impact of magnetic field and entropy generation of Casson fluid on double diffusive natural convection in staggered cavity. Int. Commun. Heat Mass Transf. 2021, 127, 105520. [Google Scholar] [CrossRef]
- Hamid, M.; Usman, M.; Khan, Z.; Haq, R.; Wang, W. Heat transfer and flow analysis of Casson fluid enclosed in a partially heated trapezoidal cavity. Int. Commun. Heat Mass Transf. 2019, 108, 104284. [Google Scholar] [CrossRef]
- Goodarzi, M.; Safaei, M.; Karimipour, A.; Hooman, K.; Dahari, M.; Kazi, S.; Sadeghinezhad, E. Comparison of the finite volume and lattice Boltzmann methods for solving natural convection heat transfer problems inside cavities and enclosures. Abstr. Appl. Anal. 2014, 2014, 762184. [Google Scholar] [CrossRef]
- Hussain, S.; Schieweck, F.; Turek, S. Efficient Newton-multigrid solution techniques for higher order space–time Galerkin discretizations of incompressible flow. Appl. Numer. Math. 2014, 83, 51–71. [Google Scholar] [CrossRef]
- Retsinis, E.; Papanicolaou, P. A Comparison Between Finite Volume and Finite Difference Method for Lid Driven Cavity Flow. In Proceedings of the 10th GRACM International Congress on Computational Mechanics, Virtual, 5–7 July 2021; pp. 5–7. [Google Scholar]
- Shahzad, H.; Ain, Q.U.; Pasha, A.A.; Irshad, K.; Shah, I.A.; Ghaffari, A.; Hafeez, M.B.; Krawczuk, M. Double-diffusive natural convection energy transfer in magnetically influenced Casson fluid flow in trapezoidal enclosure with fillets. Int. Commun. Heat Mass Transf. 2022, 137, 106236. [Google Scholar] [CrossRef]
- Basak, T.; Roy, S.; Paul, T.; Pop, I. Natural convection in a square cavity filled with a porous medium: Effects of various thermal boundary conditions. Int. J. Heat Mass Transf. 2006, 49, 1430–1441. [Google Scholar] [CrossRef]
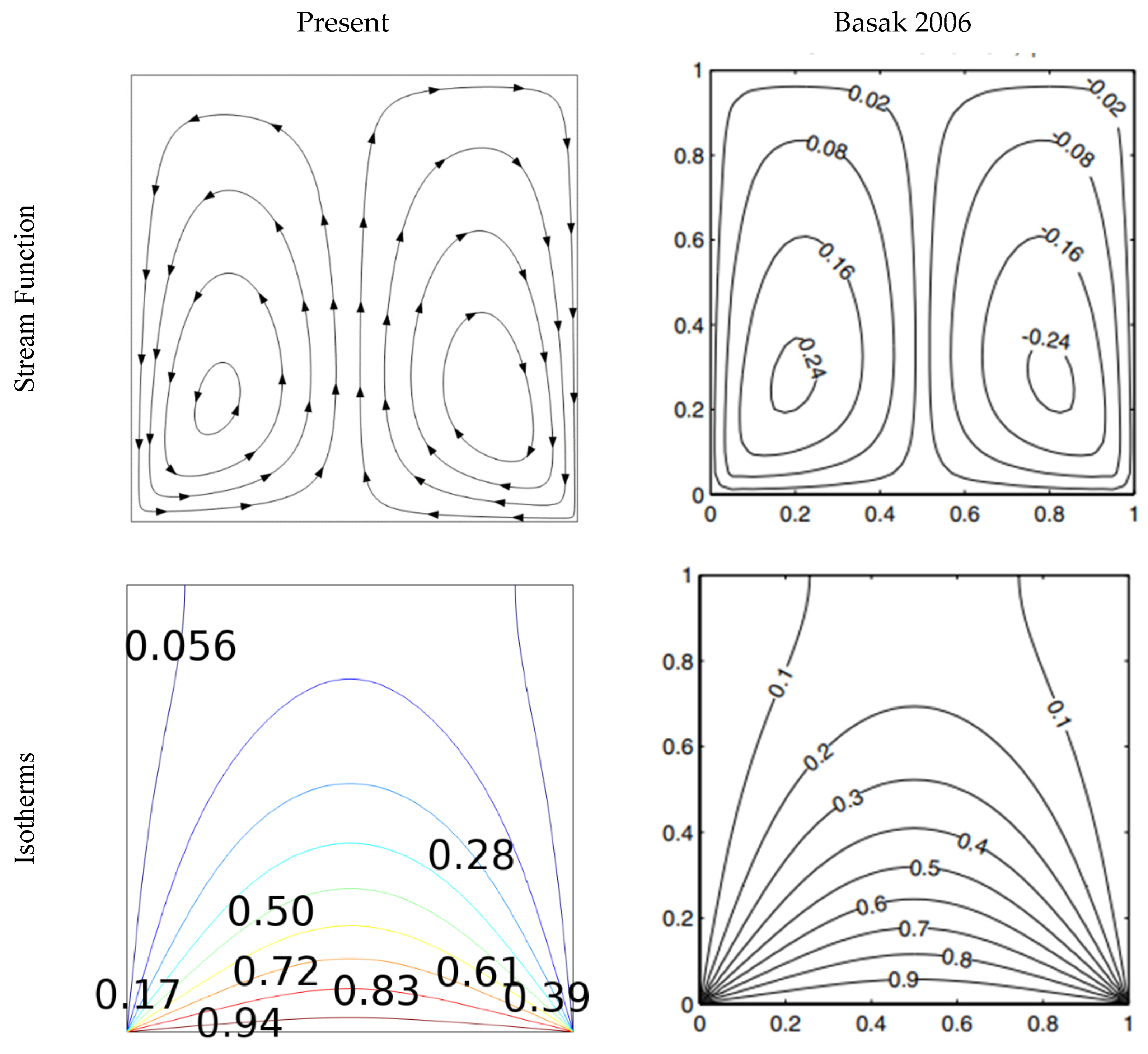


| [37] | [32] | Present | |
|---|---|---|---|
| 0.1 | 2.39023 | 2.38986 | 2.2821 |
| 1 | 3.81168 | 3.81194 | 3.7710 |
| 5 | 4.27511 | 4.27587 | 4.2651 |
| 10 | 4.35470 | 4.35556 | 4.3120 |
| Grid | NEL | DOFs | Error [%] | |
|---|---|---|---|---|
| Fine | 3352 | 32076 | 7.31940 | 7.69 |
| Finer | 9516 | 88550 | 7.88235 | 5.91 |
| Extrafine | 24908 | 226590 | 8.37816 | 0.003 |
| Extremely Fine | 34284 | 306286 | 8.37847 |
| 1 | 0.02 | 25 | 9.9430 | 9.9441 | 523.8414 | ||
| 0.1 | - | - | - | - | 9.9034 | 5.2336 | 647.8792 |
| 5 | - | - | - | - | 9.0698 | 15.6499 | 294.8795 |
| 10 | - | - | - | - | 8.7665 | 19.0674 | 253.2713 |
| - | - | - | - | 9.3401 | 9.3413 | 439.5714 | |
| - | - | - | - | 9.3477 | 9.3488 | 471.8329 | |
| - | - | - | - | 9.9732 | 9.9744 | 612.1477 | |
| - | - | - | - | 4.9731 | 4.9741 | 0.0700 | |
| - | - | - | - | 5.3781 | 5.3791 | 10.6999 | |
| - | - | - | - | 16.4802 | 16.4821 | 6513.5956 | |
| - | - | - | 0.0002 | - | 5.3800 | 5.3810 | 9.2370 |
| - | - | - | 0.002 | - | 8.5885 | 5.5896 | 229.3243 |
| - | - | - | 0.2 | - | 10.1091 | 10.1103 | 587.1623 |
| - | - | - | - | 0 | 10.8920 | 10.8923 | 1366.3958 |
| - | - | - | - | 50 | 8.1211 | 8.1222 | 134.800 |
| - | - | - | - | 75 | 6.6340 | 6.6351 | 38.6461 |
| - | - | - | - | 100 | 5.7814 | 5.7824 | 13.1207 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chuhan, I.S.; Li, J.; Ahmed, M.S.; Samuilik, I.; Aslam, M.A.; Manan, M.A. Numerical Investigation of Double-Diffusive Convection in an Irregular Porous Cavity Subjected to Inclined Magnetic Field Using Finite Element Method. Mathematics 2024, 12, 808. https://doi.org/10.3390/math12060808
Chuhan IS, Li J, Ahmed MS, Samuilik I, Aslam MA, Manan MA. Numerical Investigation of Double-Diffusive Convection in an Irregular Porous Cavity Subjected to Inclined Magnetic Field Using Finite Element Method. Mathematics. 2024; 12(6):808. https://doi.org/10.3390/math12060808
Chicago/Turabian StyleChuhan, Imran Shabir, Jing Li, Muhammad Shafiq Ahmed, Inna Samuilik, Muhammad Aqib Aslam, and Malik Abdul Manan. 2024. "Numerical Investigation of Double-Diffusive Convection in an Irregular Porous Cavity Subjected to Inclined Magnetic Field Using Finite Element Method" Mathematics 12, no. 6: 808. https://doi.org/10.3390/math12060808
APA StyleChuhan, I. S., Li, J., Ahmed, M. S., Samuilik, I., Aslam, M. A., & Manan, M. A. (2024). Numerical Investigation of Double-Diffusive Convection in an Irregular Porous Cavity Subjected to Inclined Magnetic Field Using Finite Element Method. Mathematics, 12(6), 808. https://doi.org/10.3390/math12060808







