Fuzzy Fractional Caputo Derivative of Susceptible-Infectious- Removed Epidemic Model for Childhood Diseases
Abstract
1. Introduction
- As a first attempt, in this paper, a fuzzy fractional Caputo derivatives approach subject to a SIR dynamic is developed for childhood dieases.
- The fixed point theorem of Schauder and Banach is used to demonstrate the existence and uniqueness of the solution to the addressed model.
- Perform the numerical simulations by using fuzzy Laplace and inverse Laplace transform based on the Adomian decomposition method.
- Numerical results shows the validity and effectiveness of the tracking performance of the proposed fuzzy fractional SIR dynamic.
2. Preliminaries
- should be bounded, increasing function, right continuous at 0 and left continuous over .
- should be bounded, decreasing over , right continuous at 0.
- should be less than or equal to .
- 1.
- for all ;
- 2.
- for all ;
- 3.
- for all ;
- 4.
- is a complete metric space.
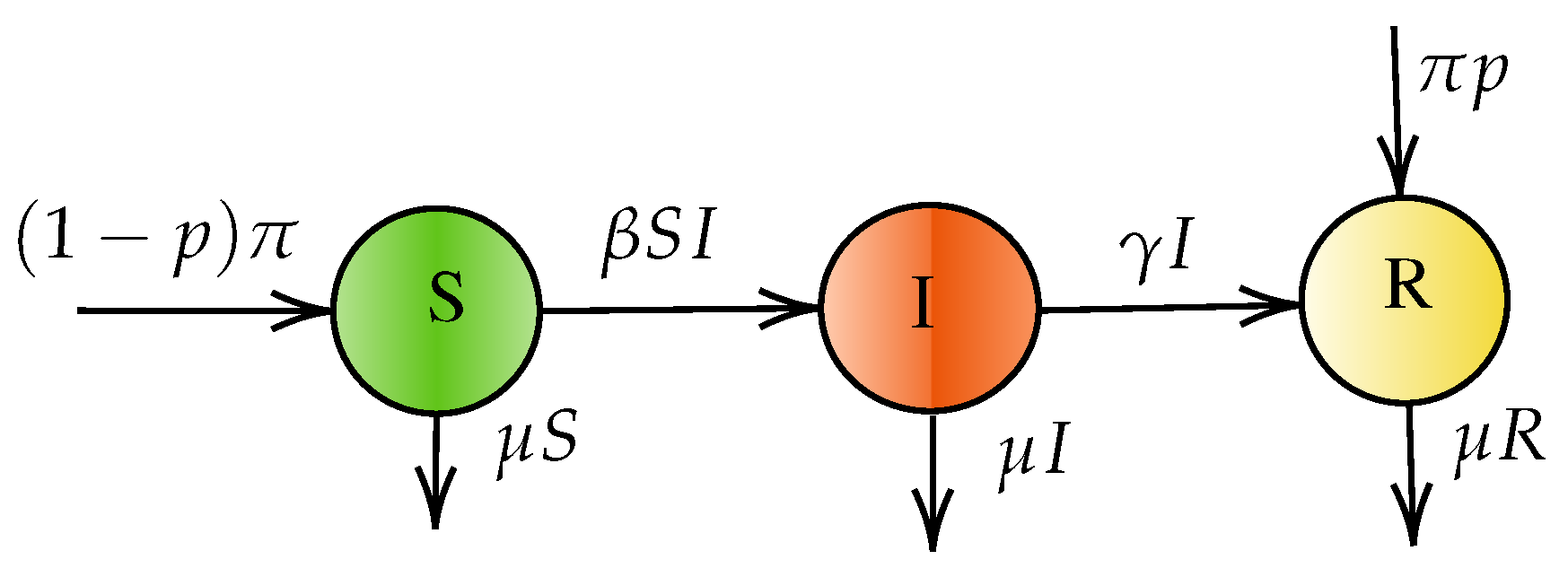
3. Formulation of Fuzzy Fractional SIR Model
4. Existence and Uniqueness Results
- There exists a constant and
- There exists constant such that for each we have
5. Method Description
6. Numerical Simulation Results and Discussions
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| SIR | Susceptible-Infectious-Removed |
| WHO | World Health Organization |
| DEs | Differential Equations |
| FODEs | Fractional Order Differential Equations |
| ML | Mittag–Leffler |
| LT | Laplace Transform. |
References
- Martcheva, M. An Introduction to Mathematical Epidemiology; Springer: Berlin/Heidelberg, Germany, 2015; Volume 61. [Google Scholar]
- Haq, F.; Shahzad, M.; Muhammad, S.; Wahab, H.A.; ur Rahman, G. Numerical analysis of fractional order epidemic model of childhood diseases. Discret. Dyn. Nat. Soc. 2017, 2017, 1–7. [Google Scholar] [CrossRef]
- Ahmad, A.; Farman, M.; Ahmad, M.; Raza, N.; Abdullah, M. Dynamical behavior of SIR epidemic model with non-integer time fractional derivatives: A mathematical analysis. Int. J. Adv. Appl. Sci 2018, 5, 123–129. [Google Scholar] [CrossRef]
- Kumar, R.; Kumar, S. A new fractional modelling on susceptible-infected-recovered equations with constant vaccination rate. Nonlinear Eng. 2014, 3, 11–19. [Google Scholar] [CrossRef]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations, 1st ed.; Springer: Berlin/Heidelberg, Germany, 1993. [Google Scholar]
- Kilbas, A.; Srivastava, H.; Trujillo, J. Theory and Applications of Fractional Differential Equations. In Number v. 13 in North-Holland Mathematics Studies; Elsevier Science: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Baleanu, D.; Diethelm, K.; Scalas, E.; Trujillo, J. Fractional Calculus: Models And Numerical Methods; Series On Complexity, Nonlinearity and Chaos; World Scientific Publishing Company: Singapore, 2012. [Google Scholar]
- Angstmann, C.N.; Henry, B.I.; McGann, A.V. A fractional-order infectivity and recovery SIR model. Fractal Fract. 2017, 1, 11. [Google Scholar] [CrossRef]
- Mouaouine, A.; Boukhouima, A.; Hattaf, K.; Yousfi, N. A fractional order SIR epidemic model with nonlinear incidence rate. Adv. Differ. Equ. 2018, 2018, 1–9. [Google Scholar] [CrossRef]
- Hasan, S.; Al-Zoubi, A.; Freihet, A.; Al-Smadi, M.; Momani, S. Solution of fractional SIR epidemic model using residual power series method. Appl. Math. Inf. Sci. 2019, 13, 153–161. [Google Scholar] [CrossRef]
- Srivastava, H.; Günerhan, H. Analytical and approximate solutions of fractional-order susceptible-infected-recovered epidemic model of childhood disease. Math. Methods Appl. Sci. 2019, 42, 935–941. [Google Scholar] [CrossRef]
- Suganya, S.; Parthiban, V. A mathematical review on Caputo fractional derivative models for COVID-19. AIP Conf. Proc. 2023, 2852, 110003. [Google Scholar]
- Gao, W.; Veeresha, P.; Prakasha, D.; Baskonus, H.M.; Yel, G. New approach for the model describing the deathly disease in pregnant women using Mittag–Leffler function. Chaos Solitons Fractals 2020, 134, 109696. [Google Scholar] [CrossRef]
- Baleanu, D.; Fernandez, A.; Akgül, A. On a fractional operator combining proportional and classical differintegrals. Mathematics 2020, 8, 360. [Google Scholar] [CrossRef]
- Asjad, M.I.; Aleem, M.; Ahmadian, A.; Salahshour, S.; Ferrara, M. New trends of fractional modeling and heat and mass transfer investigation of (SWCNTs and MWCNTs)-CMC based nanofluids flow over inclined plate with generalized boundary conditions. Chin. J. Phys. 2020, 66, 497–516. [Google Scholar] [CrossRef]
- Agarwal, R.P.; Lakshmikantham, V.; Nieto, J.J. On the concept of solution for fractional differential equations with uncertainty. Nonlinear Anal. Theory Methods Appl. 2010, 72, 2859–2862. [Google Scholar] [CrossRef]
- Park, J.Y.; Han, H.K. Existence and uniqueness theorem for a solution of fuzzy Volterra integral equations. Fuzzy Sets Syst. 1999, 105, 481–488. [Google Scholar] [CrossRef]
- Perfilieva, I. Fuzzy transforms: Theory and applications. Fuzzy Sets Syst. 2006, 157, 993–1023. [Google Scholar] [CrossRef]
- Salahshour, S.; Allahviranloo, T.; Abbasbandy, S. Solving fuzzy fractional differential equations by fuzzy Laplace transforms. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 1372–1381. [Google Scholar] [CrossRef]
- Kaleva, O. Fuzzy differential equations. Fuzzy Sets Syst. 1987, 24, 301–317. [Google Scholar] [CrossRef]
- Arshad, S.; Lupulescu, V. Fractional differential equation with the fuzzy initial condition. Electron. J. Differ. Equ. (EJDE) 2011, 2011, 34. [Google Scholar]
- Allahviranloo, T.; Ahmadi, M.B. Fuzzy Laplace Transforms. Soft Comput. 2010, 14, 235–243. [Google Scholar] [CrossRef]
- Lupulescu, V. Fractional calculus for interval-valued functions. Fuzzy Sets Syst. 2015, 265, 63–85. [Google Scholar] [CrossRef]
- Benchohra, M.; Cabada, A.; Seba, D. An existence result for nonlinear fractional differential equations on Banach spaces. Bound. Value Probl. 2009, 2009, 628916. [Google Scholar] [CrossRef]
- Belmekki, M.; Nieto, J.; Rodriguez-Lopez, R. Existence of periodic solution for a nonlinear fractional differential equation. Bound. Value Probl. 2009, 2009, 324561. [Google Scholar] [CrossRef]
- Ali, N.; Khan, R. Existence of positive solution to a class of fractional differential equations with three point boundary conditions. Math. Sci. Lett 2016, 5, 291–296. [Google Scholar] [CrossRef]
- Khan, R.A.; Shah, K. Existence and uniqueness of solutions to fractional order multi-point boundary value problems. Commun. Appl. Anal 2015, 19, 515–525. [Google Scholar]
- Lakshmikantham, V.; Leela, S. Nagumo-type uniqueness result for fractional differential equations. Nonlinear Anal. 2009, 7, 2886–2889. [Google Scholar] [CrossRef]
- Lakshmikantham, V.; Vatsala, A.S. Basic theory of fractional differential equations. Nonlinear Anal. Theory Methods Appl. 2008, 69, 2677–2682. [Google Scholar] [CrossRef]
- Allahviranloo, T.; Salahshour, S.; Abbasbandy, S. Explicit solutions of fractional differential equations with uncertainty. Soft Comput. 2012, 16, 297–302. [Google Scholar] [CrossRef]


| Parameters | Representation | Values |
|---|---|---|
| Natural death rate | 0.4 | |
| Birth rate | 0.4 | |
| p | Fraction of the vaccinated population at birth | 0.9 |
| Contact rate of susceptible individual | 0.8 | |
| Infected individuals recover at a rate | 0.03 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Subramanian, S.; Kumaran, A.; Ravichandran, S.; Venugopal, P.; Dhahri, S.; Ramasamy, K. Fuzzy Fractional Caputo Derivative of Susceptible-Infectious- Removed Epidemic Model for Childhood Diseases. Mathematics 2024, 12, 466. https://doi.org/10.3390/math12030466
Subramanian S, Kumaran A, Ravichandran S, Venugopal P, Dhahri S, Ramasamy K. Fuzzy Fractional Caputo Derivative of Susceptible-Infectious- Removed Epidemic Model for Childhood Diseases. Mathematics. 2024; 12(3):466. https://doi.org/10.3390/math12030466
Chicago/Turabian StyleSubramanian, Suganya, Agilan Kumaran, Srilekha Ravichandran, Parthiban Venugopal, Slim Dhahri, and Kavikumar Ramasamy. 2024. "Fuzzy Fractional Caputo Derivative of Susceptible-Infectious- Removed Epidemic Model for Childhood Diseases" Mathematics 12, no. 3: 466. https://doi.org/10.3390/math12030466
APA StyleSubramanian, S., Kumaran, A., Ravichandran, S., Venugopal, P., Dhahri, S., & Ramasamy, K. (2024). Fuzzy Fractional Caputo Derivative of Susceptible-Infectious- Removed Epidemic Model for Childhood Diseases. Mathematics, 12(3), 466. https://doi.org/10.3390/math12030466








