Fixed-Time Command-Filtered Control for Nonlinear Systems with Mismatched Disturbances
Abstract
1. Introduction
System Description
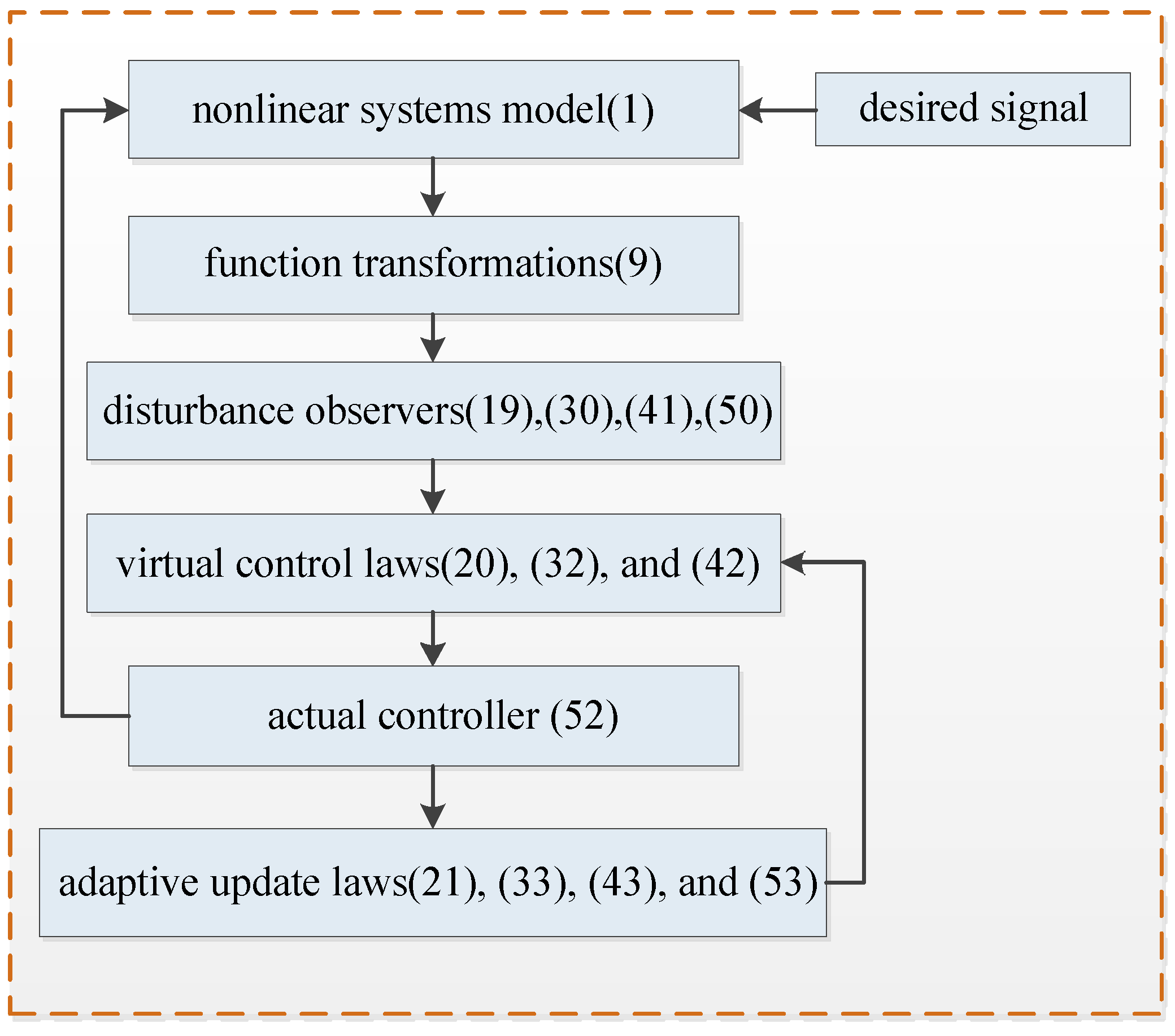
2. Main Results
2.1. Fixed-Time Fuzzy Control Law Design
2.2. Stability Analysis
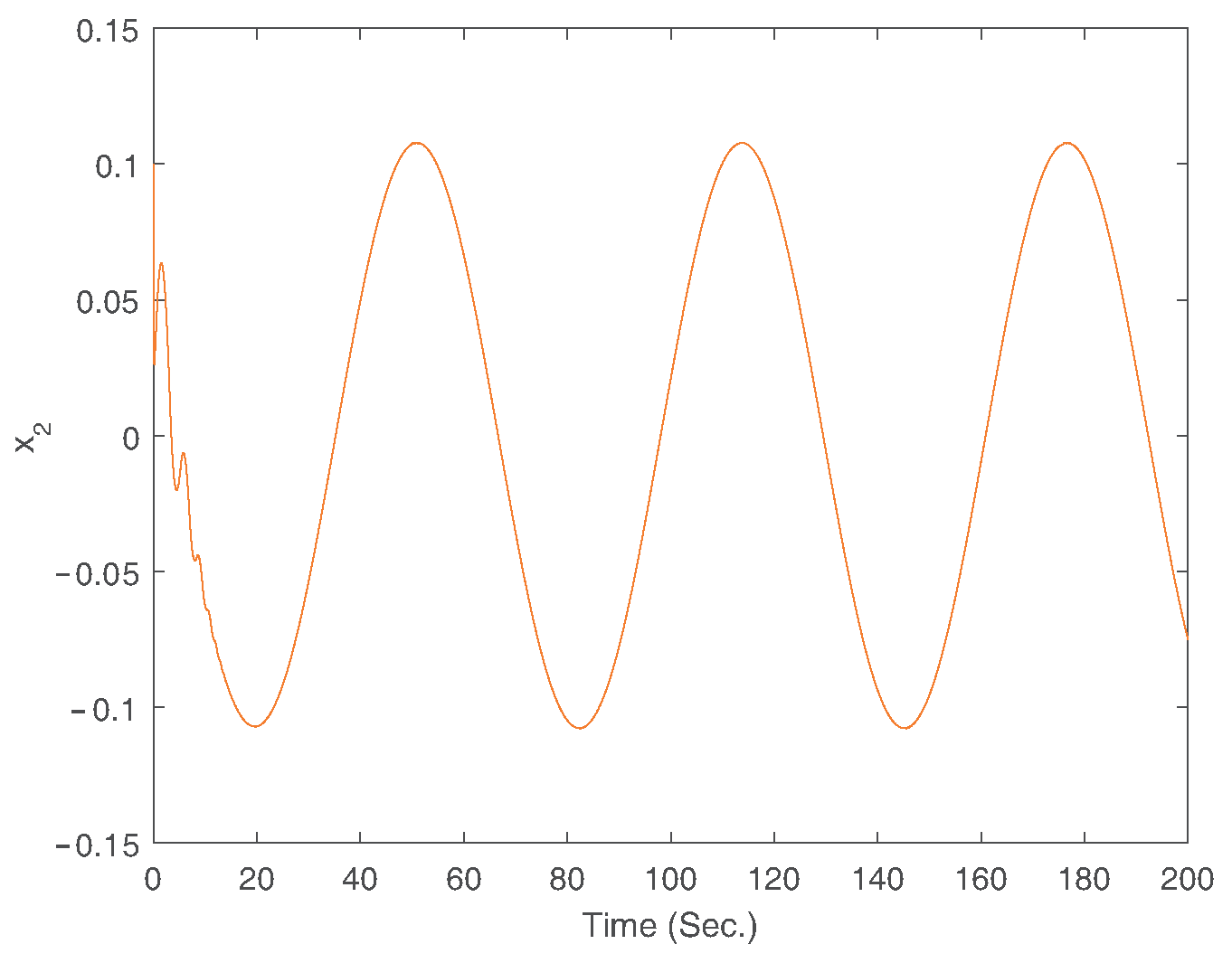
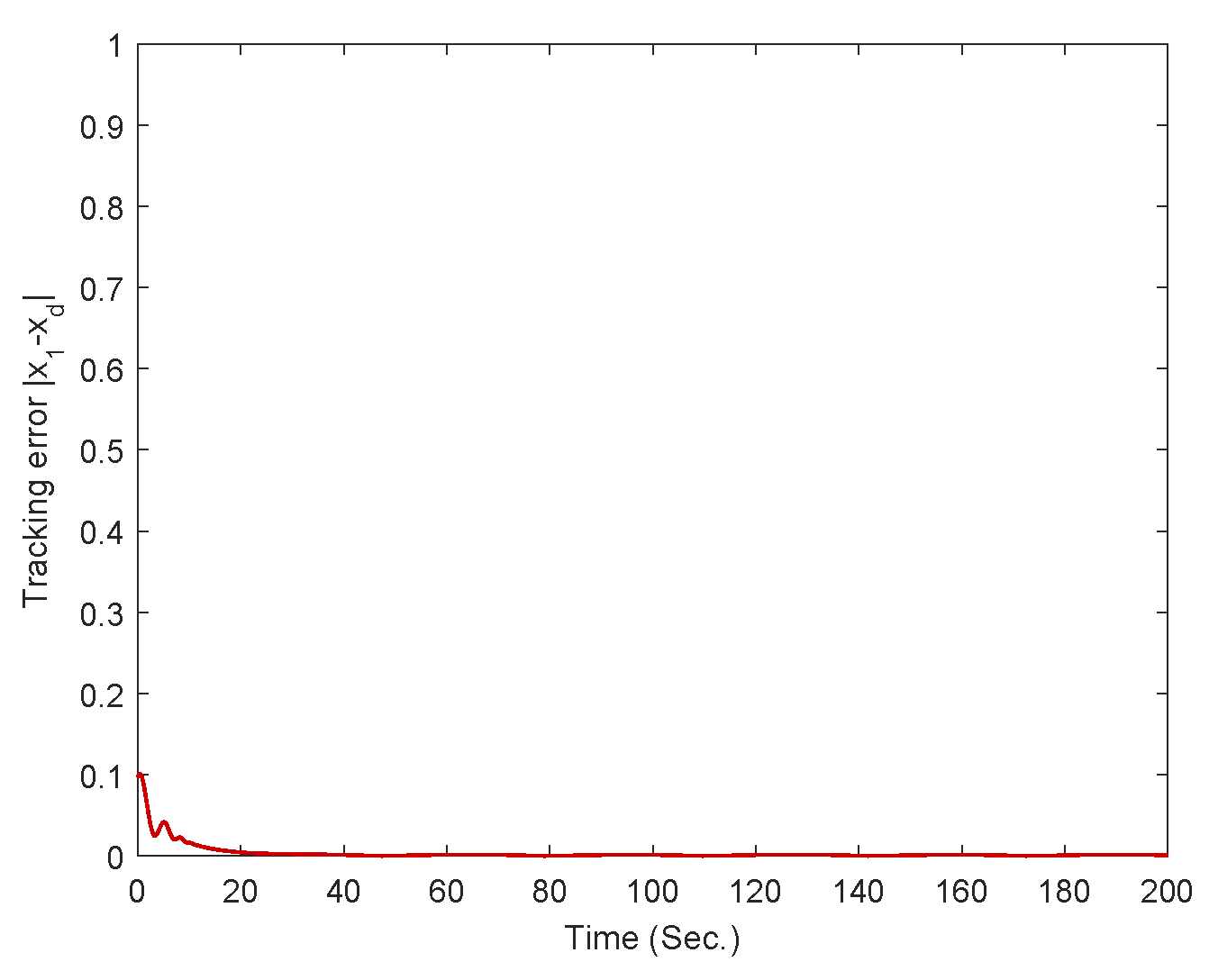
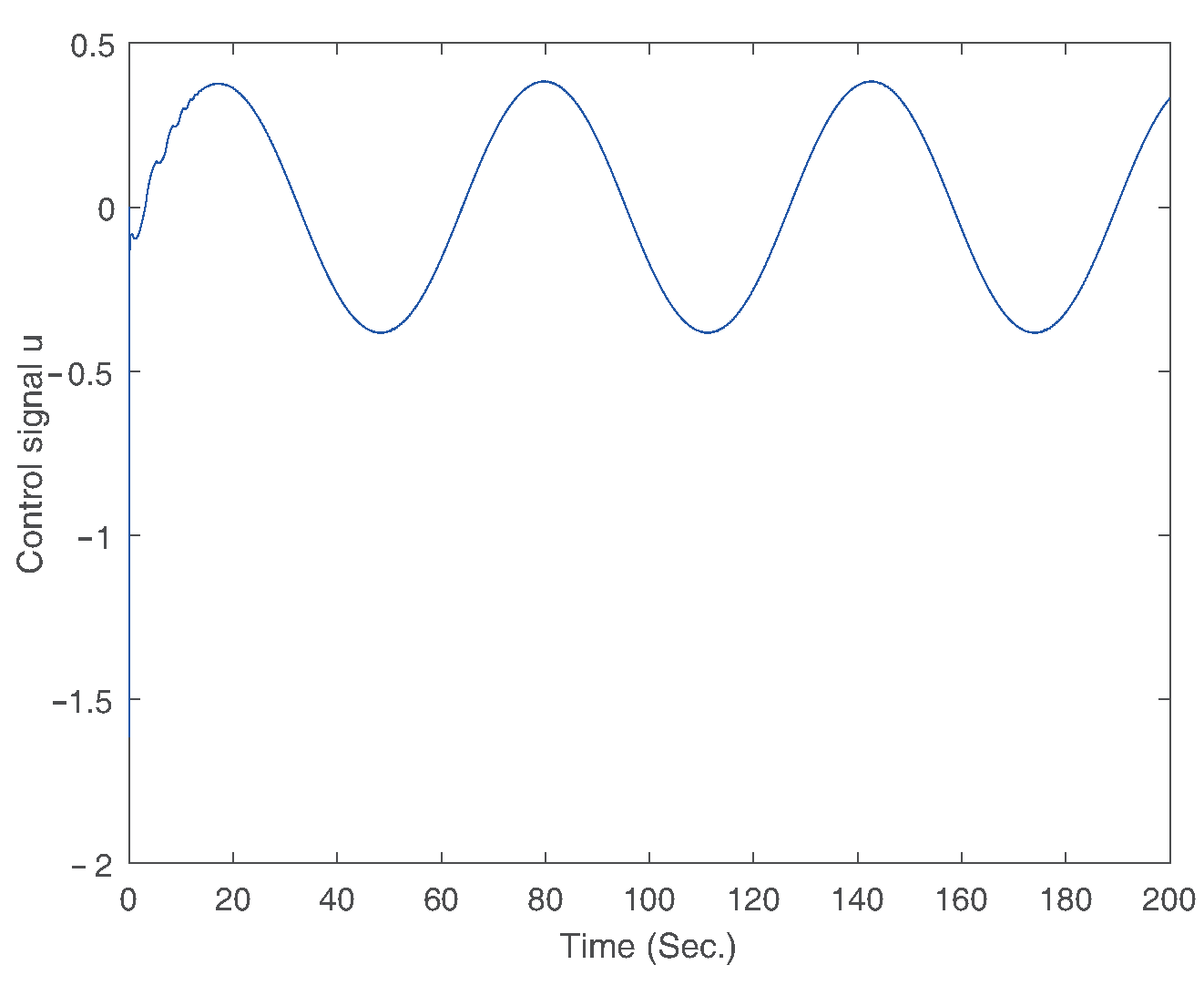
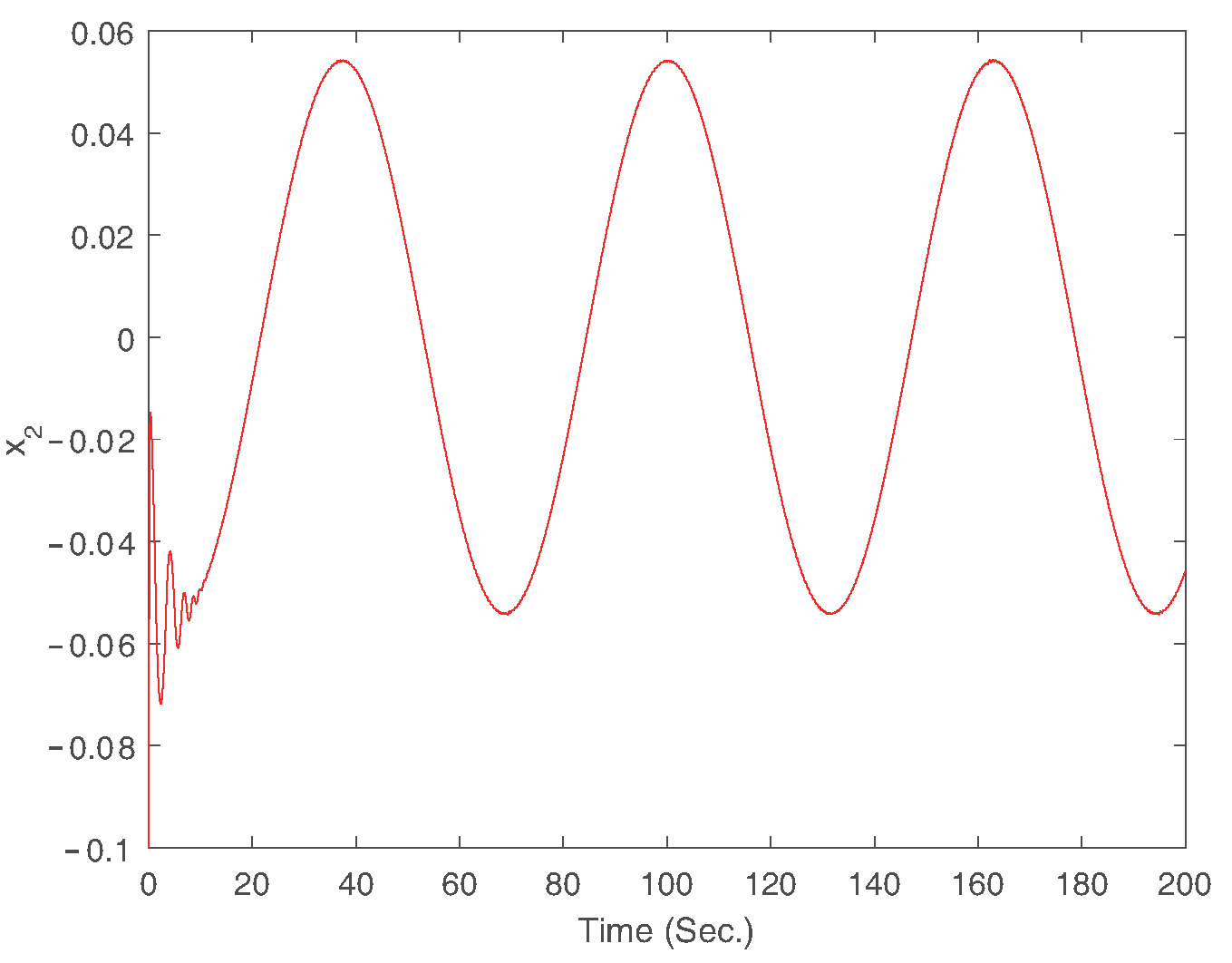
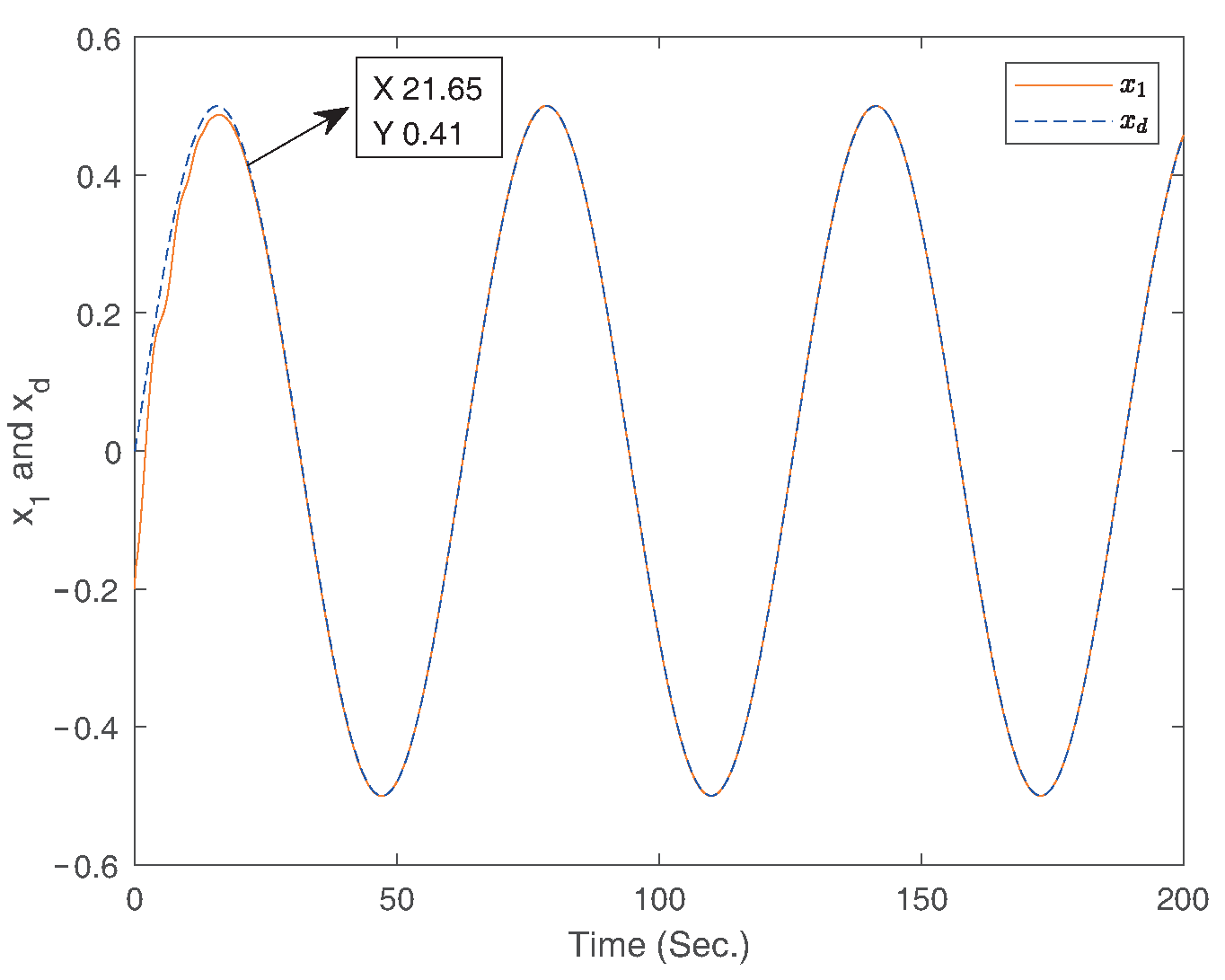
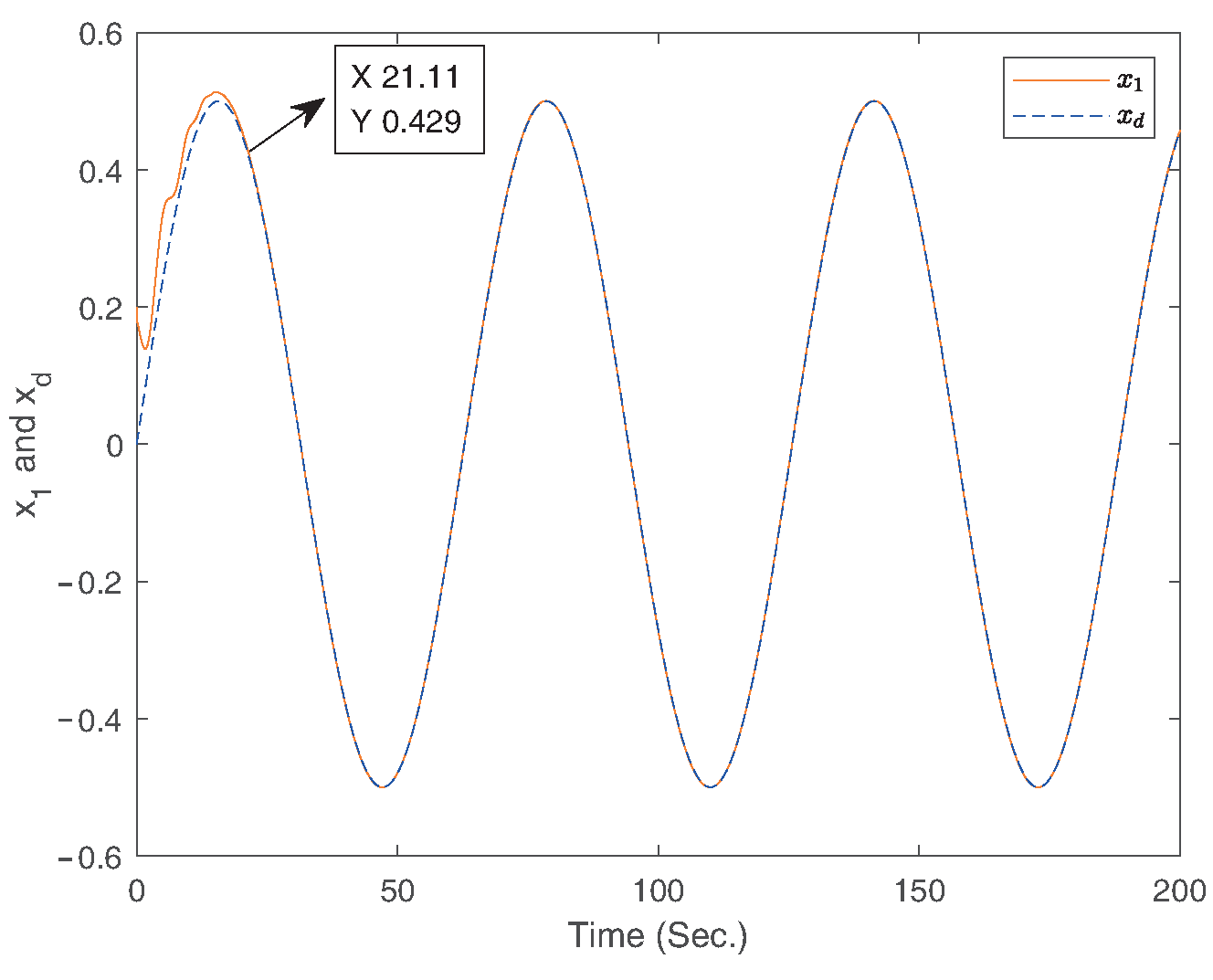
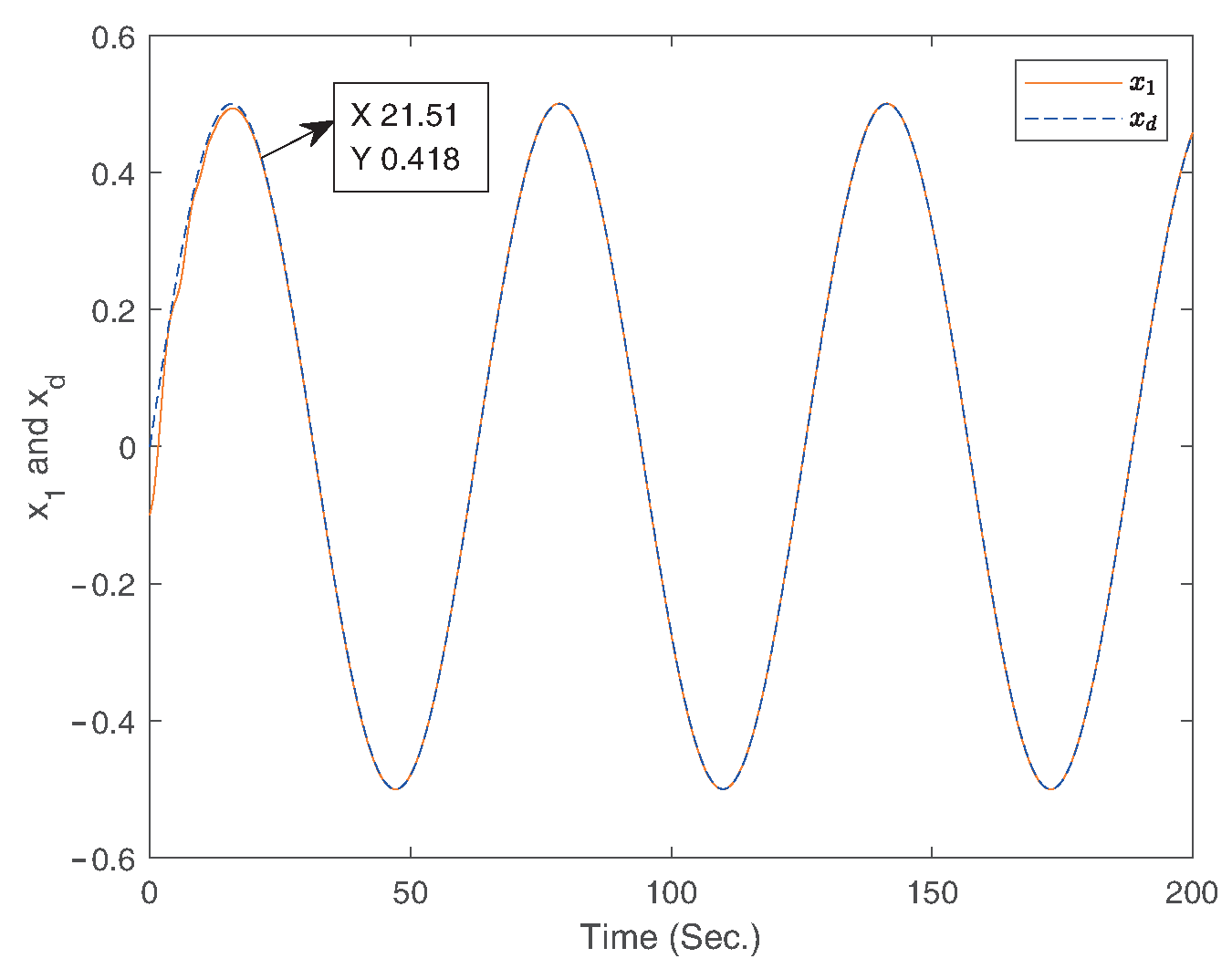
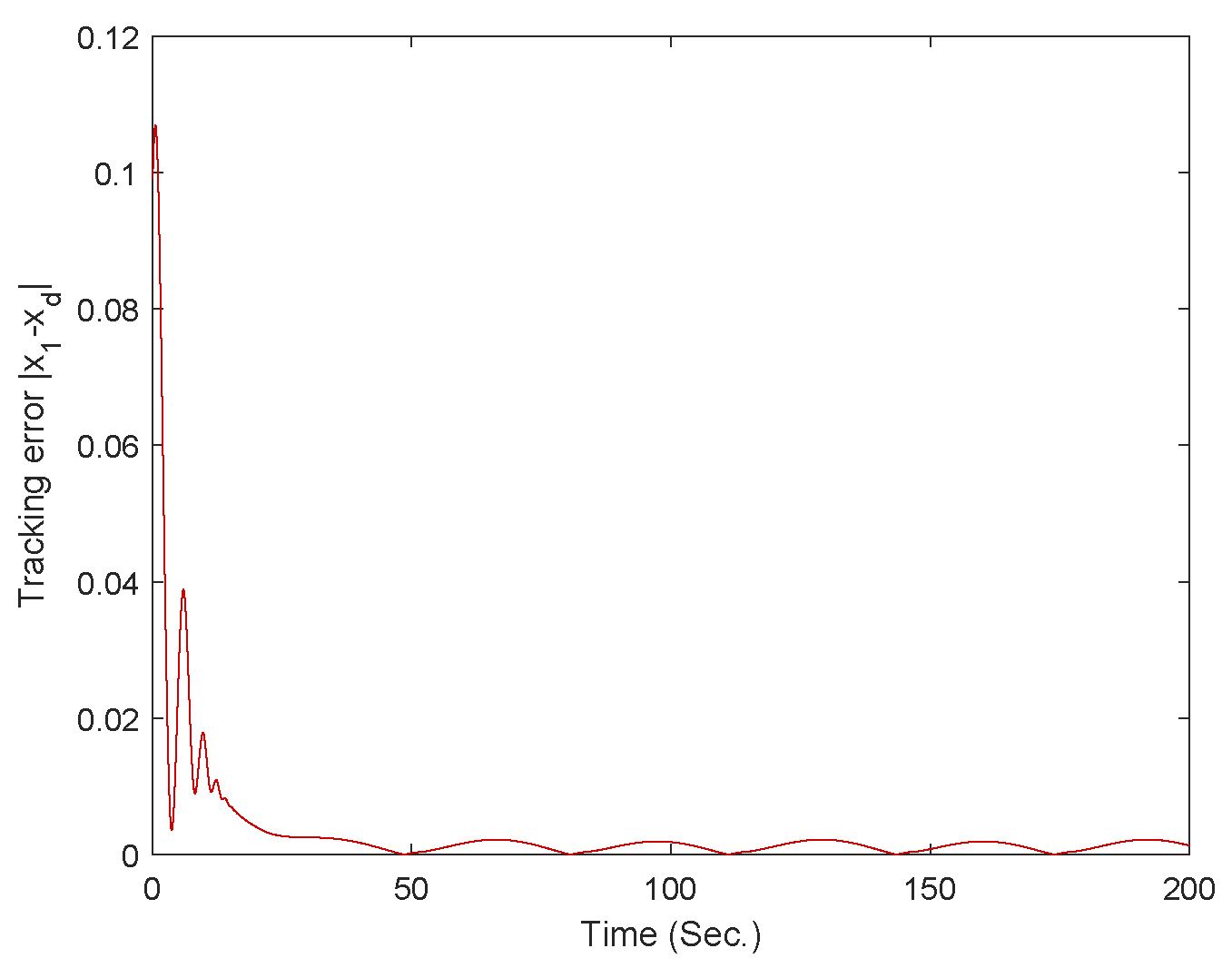
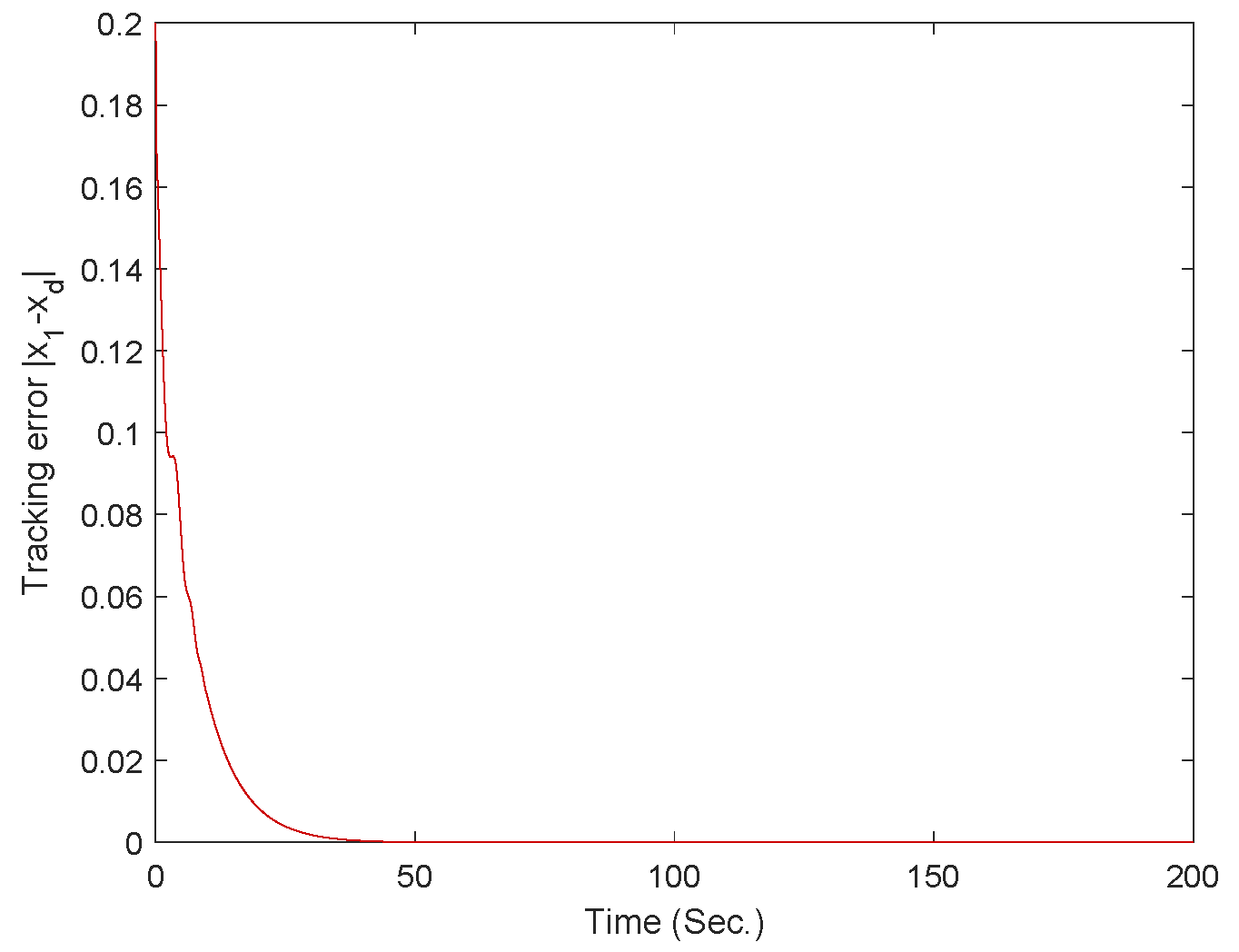
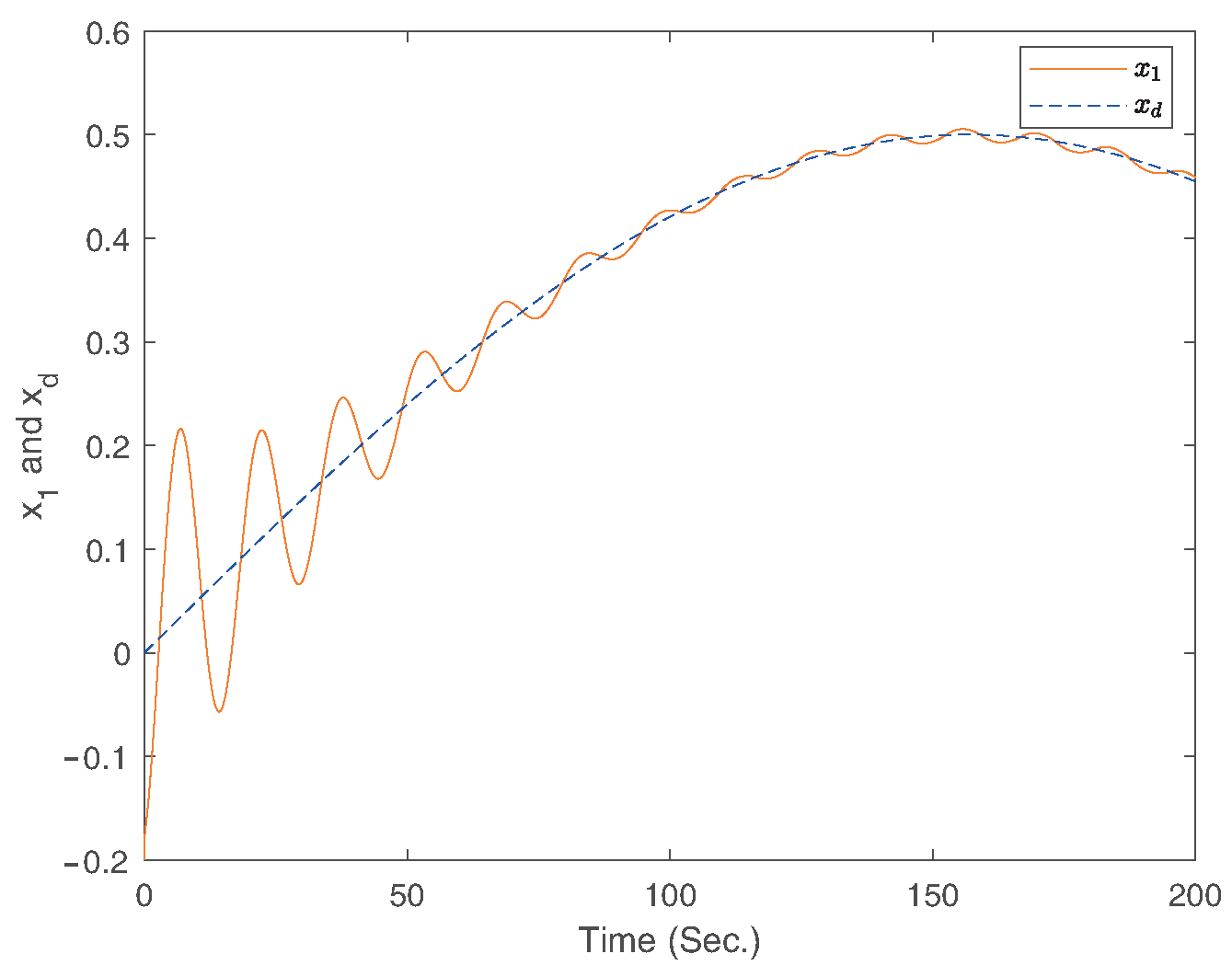
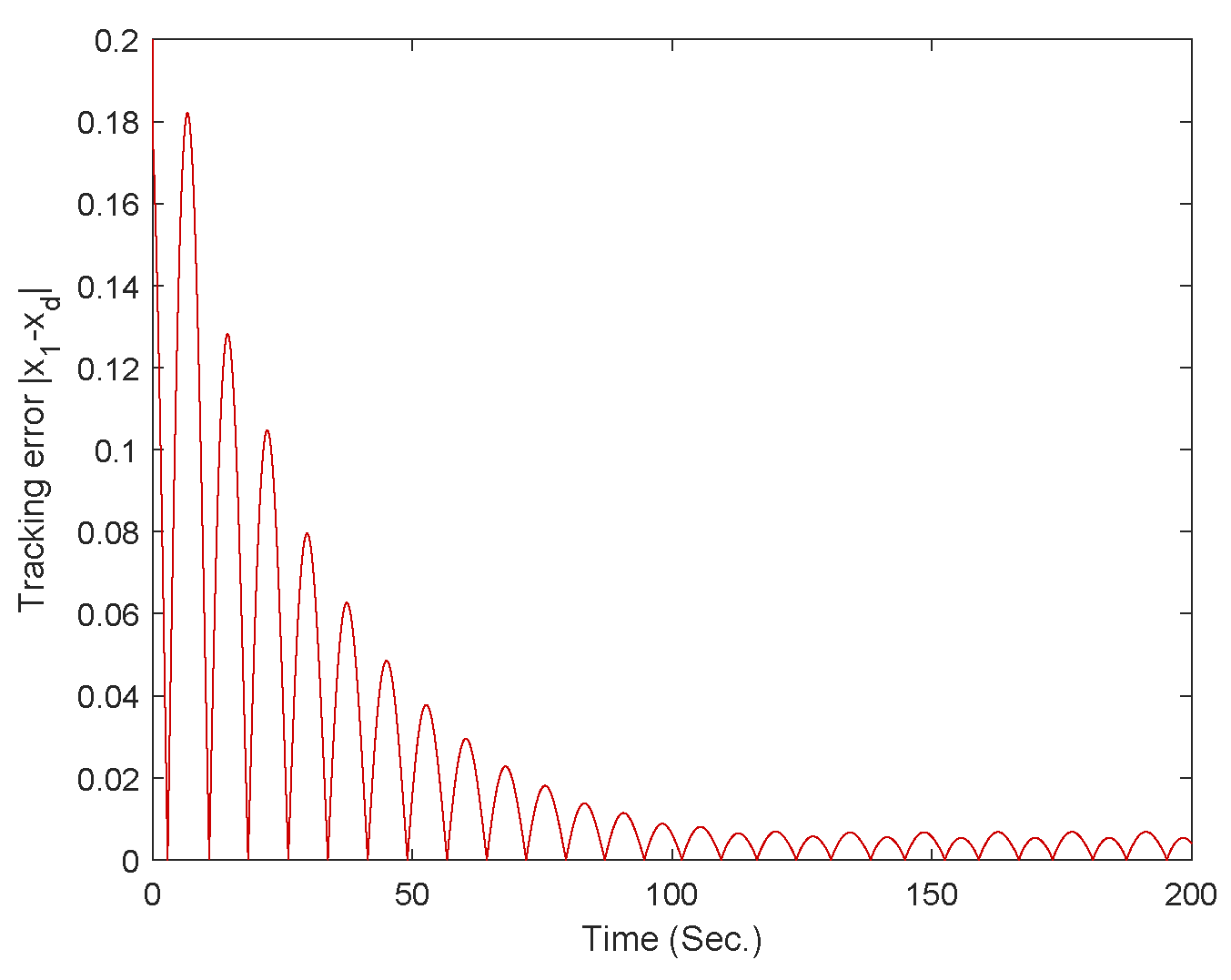
3. Simulation Example
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| The mismatched disturbances | |
| The unknown nonlinear functions | |
| ,, , , p | The parameters of the fixed-time command filter |
| The output of fixed-time command filter | |
| The desired tracking signal | |
| The tracking error | |
| The compensated tracking error signal | |
| The parameter of the disturbance observer | |
| , , , , , | The parameters of the controller |
| , | The parameters of adaptive updating laws |
| The error compensating signal | |
| The virtual controllers |
References
- Zhu, D.; He, Y.; Li, F. Trajectory tracking of delta parallel robot via adaptive backstepping fractional-order non-singular sliding mode control. Mathematics 2024, 12, 2236. [Google Scholar] [CrossRef]
- Chalishajar, D.; Kasinathan, D.; Kasinathan, R.; Kasinathan, R. T-controllability of higher-order fractional stochastic delay system via integral contractor. J. Control Decis. 2024, 1–24. [Google Scholar] [CrossRef]
- Chalishajar, D.; Kasinathan, D.; Kasinathan, R.; Kasinathan, R. Exponentialstability, T-controllability and optimal controllability of higher-order fractional neutral stochastic differential equation via integral contractor. Chaos Solitons Fractals 2024, 186, 115278. [Google Scholar] [CrossRef]
- Asadi, D.; Bagherzadeh, S.A. Nonlinear adaptive sliding mode tracking control of an airplane with wing damage. Proc. Inst. Mech. Eng. Part J. Aerosp. Eng. 2018, 232, 1405–1420. [Google Scholar] [CrossRef]
- Asadi, D.; Atkins, E.M. Multi-objective weight optimization for trajectory planning of an airplane with structural damage. J. Intell. Robot. Syst. 2018, 91, 667–690. [Google Scholar] [CrossRef]
- Ahmed, A.S.; L-Nahhas, M.A.A.; Omar, O.A.; Chalishajar, D.N.; Ahmed, H.M. Controllability of impulsive nonlinear ψ-Hilfer fractional integro-differential equations. Results Control. Optim. 2024, 16, 100566. [Google Scholar] [CrossRef]
- Xie, X.; Wei, C.; Gu, Z.; Shi, K. Relaxed resilient fuzzy stabilization of discrete-time Takagi-Sugeno systems via a higher order time-variant balanced matrix method. IEEE Trans. Fuzzy Syst. 2022, 30, 5044–5050. [Google Scholar] [CrossRef]
- Jin, X.; Lü, S.; Yu, J. Adaptive NN-based consensus for a class of nonlinear multiagent systems with actuator faults and faulty networks. IEEE Trans. Neural Netw. Learn. Syst. 2021, 33, 3474–3486. [Google Scholar] [CrossRef] [PubMed]
- Chalishajar, D.; Chalishajar, H. Trajectory controllability of second order nonlinear integro-differential system: An analytical and a numerical estimation. Differ. Equ. Dyn. Syst. 2015, 23, 467–481. [Google Scholar] [CrossRef]
- Bagherzadeh, S.A.; Asadi, D. Detection of the ice assertion on aircraft using empirical mode decomposition enhanced by multi-objective optimization. Mech. Syst. Signal Process. 2017, 18, 9–24. [Google Scholar] [CrossRef]
- Asadi, D.; Ahmadi, K. Nonlinear robust adaptive control of an airplane with structural damage. Proc. Inst. Mech. Eng. Part J. Aerosp. Eng. 2020, 234, 2076–2088. [Google Scholar] [CrossRef]
- Asadi, D.; Farsadi, A.T. Active flutter control of thin walled wing-engine system using piezoelectric actuators. Aerosp. Sci. Technol. 2020, 102, 105853. [Google Scholar] [CrossRef]
- Kang, S.; Liu, P.X.; Wang, H. Command filter-based adaptive fuzzy decentralized control for large-scale nonlinear systems. Nonlinear Dyn. 2021, 105, 3239–3253. [Google Scholar] [CrossRef]
- Zhu, Z.; Liang, H.; Liu, Y.; Xue, H. Command filtered event-triggered adaptive control for MIMO stochastic multiple time-delay systems. Int. J. Robust Nonlinear Control 2022, 32, 715–736. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, N.; Liu, Y.; Zhao, X. Adaptive fault-tolerant control for switched nonlinear systems based on command filter technique. Appl. Math. Comput. 2021, 392, 125725. [Google Scholar] [CrossRef]
- Yu, J.; Chen, B.; Yu, H.; Lin, C.; Zhao, L. Neural networks-based command filtering control of nonlinear systems with uncertain disturbance. Inf. Sci. 2018, 426, 50–60. [Google Scholar] [CrossRef]
- Liu, G.; Liang, H.; Pan, Y.; Ahn, C.K. Antagonistic interaction-based bipartite consensus control for heterogeneous networked systems. IEEE Trans. Syst. Man Cybern. Syst. 2022, 53, 71–81. [Google Scholar] [CrossRef]
- Xiao, Y.; de Ruiter, A.; Ye, D.; Sun, Z. Attitude coordination control for flexible spacecraft formation flying with guaranteed performance bounds. IEEE Trans. Aerosp. Electron. Syst. 2022, 59, 1534–1550. [Google Scholar]
- Yu, J.; Shi, P.; Zhao, L. Finite-time command filtered backstepping control for a class of nonlinear systems. Automatica 2018, 92, 173–180. [Google Scholar] [CrossRef]
- Capone, A.; Hirche, S. Backstepping for partially unknown nonlinear systems using Gaussian processes. IEEE Control Syst. Lett. 2019, 3, 416–421. [Google Scholar] [CrossRef]
- Fedele, G.; D’Alfonso, L. A kinematic model for swarm finite-time trajectory tracking. IEEE Trans. Cybern. 2018, 49, 3806–3815. [Google Scholar] [CrossRef] [PubMed]
- Wang, H.; Yu, W.; Ren, W.; Lu, J. Distributed adaptive finite-time consensus for second-order multiagent systems with mismatched disturbances under directed networks. IEEE Trans. Cybern. 2019, 51, 1347–1358. [Google Scholar] [CrossRef]
- Xiao, W.; Ren, H.; Zhou, Q.; Li, H.; Lu, R. Distributed finite-time containment control for nonlinear multiagent systems with mismatched disturbances. IEEE Trans. Cybern. 2022, 52, 6939–6948. [Google Scholar] [CrossRef]
- Wang, H.; Yu, W.; Ren, W.; Lu, J. Finite-time output consensus of higher-order multi-agent systems with mismatched disturbances and unknown state elements. IEEE Trans. Syst. Man Cybern. Syst. 2019, 66, 2571–2581. [Google Scholar]
- Wang, W.; Tong, S. Adaptive fuzzy containment control of nonlinear strict-feedback systems with full state constraints. IEEE Trans. Fuzzy Syst. 2019, 27, 2024–2038. [Google Scholar] [CrossRef]
- Shotorbani, A.M.; Mohammadi-Ivatloo, B.; Wang, L.; Marzband, M.; Sabahi, M. Application of finite-time control Lyapunov function in low-power PMSG wind energy conversion systems for sensorless MPPT. Int. Electr. Power Energy Syst. 2019, 106, 169–182. [Google Scholar] [CrossRef]
- Asadi, D. Actuator Fault detection, identification, and control of a multirotor air vehicle using residual generation and parameter estimation approaches. Int. J. Aeronaut. Space Sci. 2024, 25, 176–189. [Google Scholar] [CrossRef]
- Huo, X.; Karimi, H.R.; Zhao, X.; Wang, B.; Zong, G. Adaptive-critic design for decentralized event-triggered control of constrained nonlinear interconnected systems within an identifier-critic framework. IEEE Trans. Cybern. 2021, 52, 7478–7491. [Google Scholar] [CrossRef]
- Wang, N.; Gao, Y.; Yang, C.; Zhang, X. Reinforcement learning-based finite-time tracking control of an unknown unmanned surface vehicle with input constraints. Neurocomputing 2022, 484, 26–37. [Google Scholar] [CrossRef]
- Wang, L.; Liu, P.X.; Wang, H. Fast finite-time control for nonaffine stochastic nonlinear systems against multiple actuator constraints via output feedback. IEEE Trans. Cybern. 2022, 53, 3253–3262. [Google Scholar] [CrossRef] [PubMed]
- Sui, S.; Tong, S. Finite-time fuzzy adaptive ppc for nonstrict-feedback nonlinear mimo systems. IEEE Trans. Cybern. 2022, 53, 732–742. [Google Scholar] [CrossRef]
- Gao, M.; Ding, L.; Jin, X. Elm-based adaptive faster fixed-time control of robotic manipulator systems. IEEE Trans. Neural Netw. Learn. Syst. 2021, 34, 4646–4658. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, Z. Fuzzy adaptive practical fixed-time consensus for second-order nonlinear multiagent systems under actuator faults. IEEE Trans. Cybern. 2020, 51, 1150–1162. [Google Scholar] [CrossRef]
- Ren, P.; Wang, F.; Zhu, R. Adaptive fixed-time fuzzy control of uncertain nonlinear quantized systems. Int. J. Fuzzy Syst. 2021, 23, 794–803. [Google Scholar] [CrossRef]
- Qin, H.; Yang, H.; Sun, Y.; Zhang, Y. Adaptive interval type-2 fuzzy fixed-time control for underwater walking robot with error constraints and actuator faults using prescribed performance terminal sliding-mode surfaces. Int. J. Fuzzy Syst. 2021, 23, 1137–1149. [Google Scholar] [CrossRef]
- Arabi, E.; Yucelen, T.; Singler, J.R. Finite-time distributed control with time transformation. Int. J. Robust Nonlinear Control 2021, 31, 107–130. [Google Scholar] [CrossRef]
- Yu, J.; Shi, P.; Liu, J.; Lin, C. Neuroadaptive finite-time control for nonlinear MIMO systems with input constraint. IEEE Trans. Cybern. 2020, 52, 6676–6683. [Google Scholar] [CrossRef] [PubMed]
- Li, K.; Hua, C.; You, X.; Guan, X. Finite-time observer-based leader-following consensus for nonlinear multiagent systems with input delays. IEEE Trans. Cybern. 2020, 51, 5850–5858. [Google Scholar] [CrossRef] [PubMed]
- Zhao, L.; Yu, J.; Lin, C.; Yu, H. Distributed adaptive fixed-time consensus tracking for second-order multi-agent systems using modified terminal sliding mode. Appl. Math. Comput. 2017, 312, 23–35. [Google Scholar] [CrossRef]
- Han, H.; Liu, H.; Li, J.; Qiao, J. Cooperative fuzzy-neural control for wastewater treatment process. IEEE Trans. Ind. 2020, 17, 5971–5981. [Google Scholar] [CrossRef]
- Chen, M.; Wang, H.; Liu, X. Adaptive fuzzy practical fixed-time tracking control of nonlinear systems. IEEE Trans. Fuzzy Syst. 2019, 29, 664–673. [Google Scholar] [CrossRef]
- Sun, H.; Hou, L.; Zong, G.; Yu, X. Fixed-time attitude tracking control for spacecraft with input quantization. IEEE Trans. Aerosp. Electron. Syst. 2018, 55, 124–134. [Google Scholar] [CrossRef]
- Qian, C.; Lin, W. A continuous feedback approach to global strong stabilization of nonlinear systems. IEEE Trans. Autom. 2001, 46, 1061–1079. [Google Scholar] [CrossRef]
- Deng, C.; Yang, G. Distributed adaptive fuzzy control for nonlinear multiagent systems under directed graphs. IEEE Trans. Fuzzy Syst. 2017, 26, 1356–1366. [Google Scholar]
- Yu, W.; Ren, W.; Zheng, W.X.; Chen, G.; Lü, J. Distributed control gains design for consensus in multi-agent systems with second-order nonlinear dynamics. Automatica 2013, 49, 2107–2115. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, Y.; Yu, Y.; Sun, C. Fixed-time event-triggered consensus for nonlinear multiagent systems without continuous communications. IEEE Trans. Syst. Man Cybern. Syst. 2018, 49, 2221–2229. [Google Scholar] [CrossRef]
- Shi, X.; Zhou, Z.; Zhou, D.; Li, R.; Chen, X. Observer-based event-triggered fixed-time control for nonlinear system with full-state constraints and input saturation. Int. J. Control 2022, 95, 432–446. [Google Scholar] [CrossRef]
- Xu, H.; Yu, D.; Sui, S.; Zhao, Y.; Chen, C.P.; Wang, Z. Nonsingular practical fixed-time adaptive output feedback control of MIMO nonlinear systems. IEEE Trans. Neural Netw. Learn. Syst. 2022, 34, 7222–7234. [Google Scholar] [CrossRef]
- Liu, R.; Liu, M.; Shi, Y.; Qu, J. Adaptive fixed-time fuzzy control for uncertain nonlinear systems with asymmetric time-varying full-state constraints. Int. J. Fuzzy Syst. 2023, 25, 1597–1611. [Google Scholar] [CrossRef]









Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Z.; Zhang, J.; Xing, L.; Sun, L. Fixed-Time Command-Filtered Control for Nonlinear Systems with Mismatched Disturbances. Mathematics 2024, 12, 3816. https://doi.org/10.3390/math12233816
Wu Z, Zhang J, Xing L, Sun L. Fixed-Time Command-Filtered Control for Nonlinear Systems with Mismatched Disturbances. Mathematics. 2024; 12(23):3816. https://doi.org/10.3390/math12233816
Chicago/Turabian StyleWu, Zhiqiang, Jian Zhang, Lei Xing, and Liyang Sun. 2024. "Fixed-Time Command-Filtered Control for Nonlinear Systems with Mismatched Disturbances" Mathematics 12, no. 23: 3816. https://doi.org/10.3390/math12233816
APA StyleWu, Z., Zhang, J., Xing, L., & Sun, L. (2024). Fixed-Time Command-Filtered Control for Nonlinear Systems with Mismatched Disturbances. Mathematics, 12(23), 3816. https://doi.org/10.3390/math12233816




