Improved Quantization Method of Coupled Circuits in Charge Discrete Space
Abstract
1. Introduction
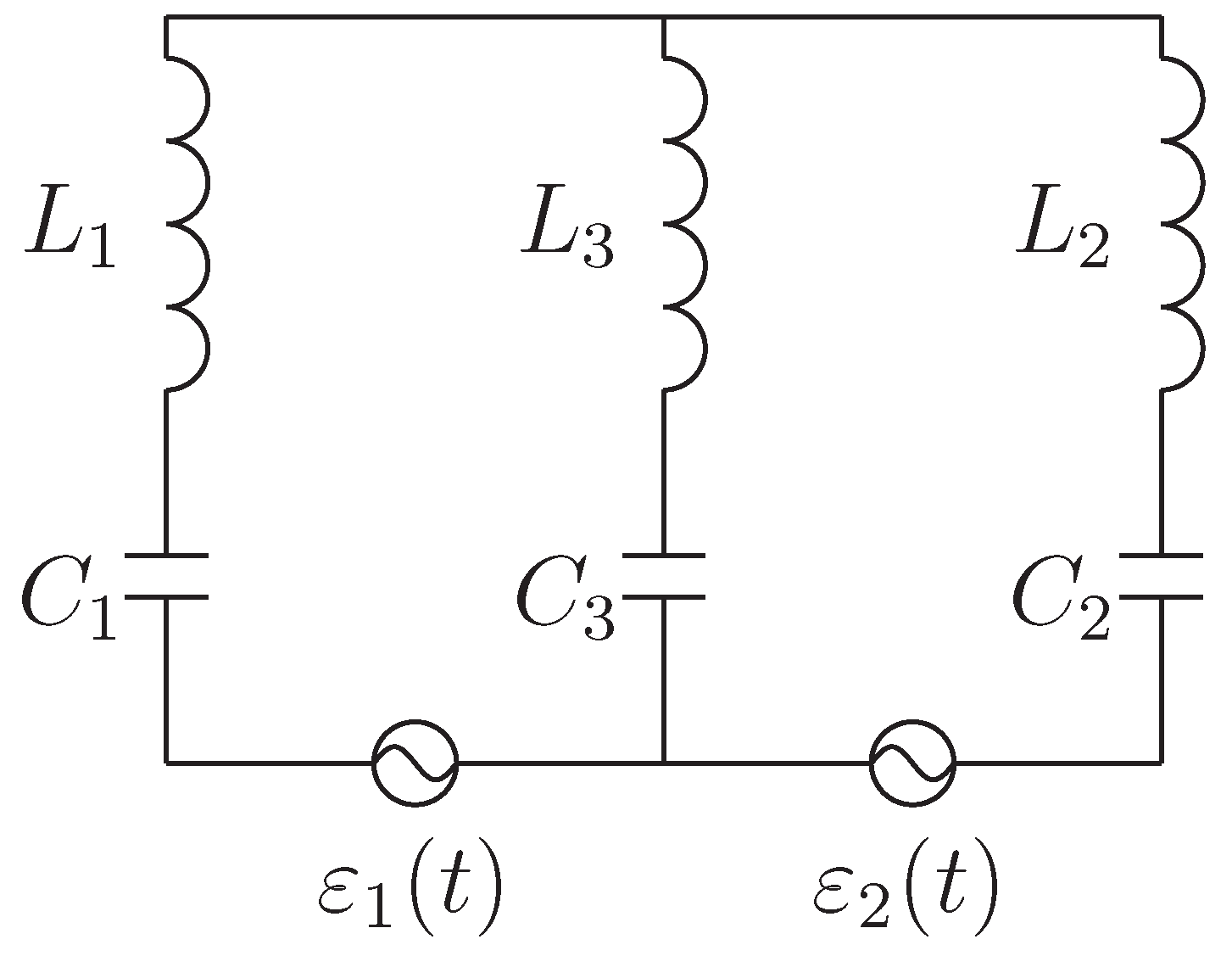
2. Schrödinger Equation of the System
3. Resolution of the Schrödinger Equation: Perturbation Method Suitable Case (PMSC)
4. Resolution of the Schrödinger Equation: Improved Perturbation Method Suitable Case (IPMSC)
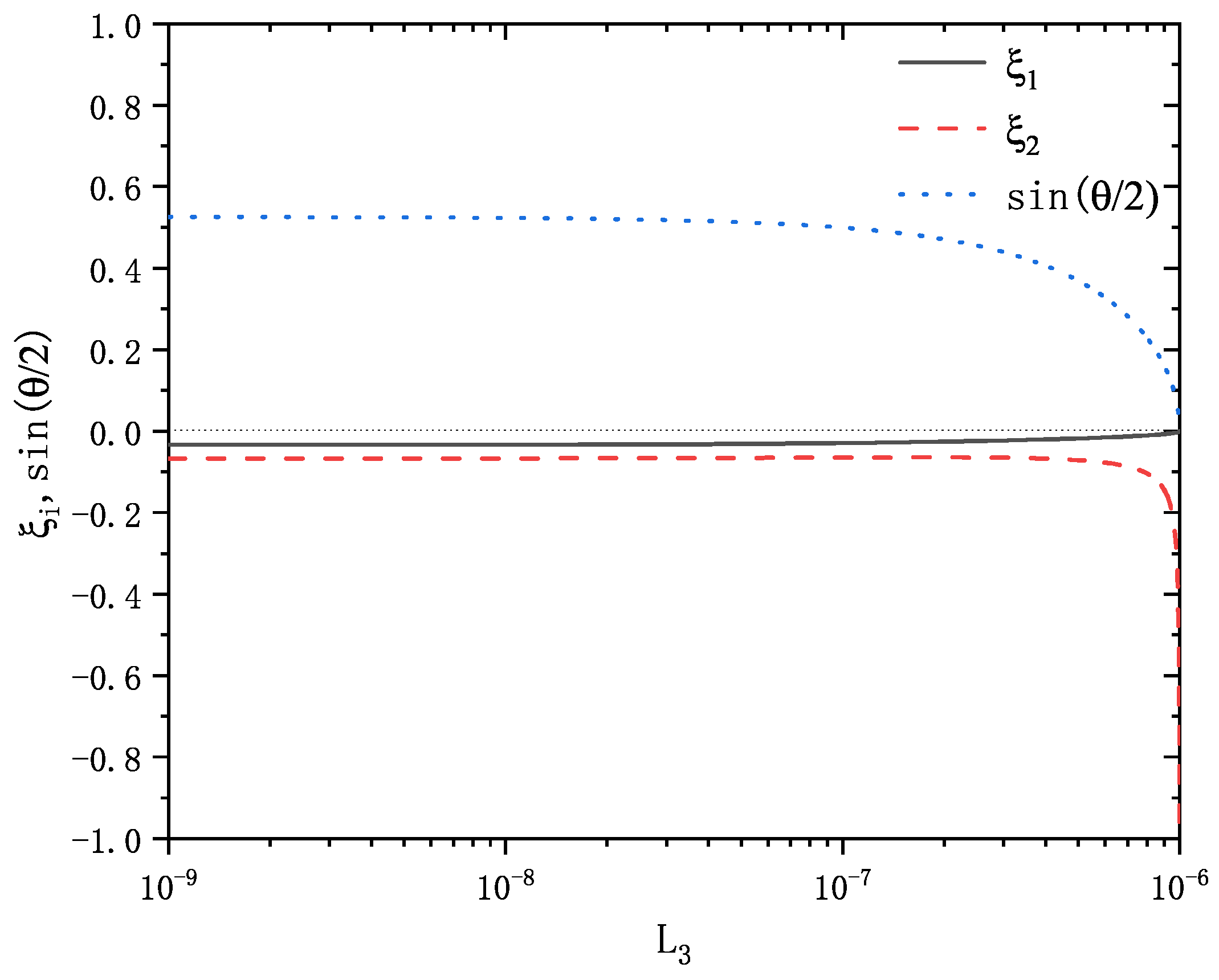
5. Discussion: Quantum Fluctuation in the System
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Decoupled Schrödinger Equations in the -Representation
References
- Buot, F.A. Mesoscopic physics and nanoelectronics: Nanoscience and nanotechnology. Phys. Rep. 1993, 234, 73–174. [Google Scholar] [CrossRef]
- Louisell, W.H. Quantum Statistical Properties of Radiation, 3rd ed.; John Wiley: New York, NY, USA, 1973; pp. 231–235. [Google Scholar]
- Zhang, Z.M.; He, L.S.; Zhou, S.K. A quantum theory of an RLC circuit with a source. Phys. Lett. A 1998, 244, 196–200. [Google Scholar] [CrossRef]
- Fan, H.Y.; Pan, X.Y. Quantization and squeezed state of two L-C Circuits with mutual-inductance. Chin. Phys. Lett. 1998, 15, 625–627. [Google Scholar] [CrossRef]
- Zhang, S.; Um, C.I.; Yeon, K.H. Zero-point energy in a mesoscopic nonlinear inductance-capacitance circuit. Chin. Phys. Lett. 2002, 19, 985–987. [Google Scholar]
- Yan, Z.Y.; Xu, S.L.; MA, J.Y. Path Integral Solutions of RLC Mesoscopic Circuit with Source. Mod. Phys. Lett. B 2012, 26, 1250058–1250065. [Google Scholar] [CrossRef]
- Fan, H.Y.; Liang, X.T. Quantum Fluctuation in Thermal Vacuum State for Mesoscopic LC Electric Circuit. Chin. Phys. Lett. 2000, 17, 174–176. [Google Scholar] [CrossRef]
- Pedrosa, I.A. Quantum description of a time-dependent mesoscopic RLC circuit. Phys. Scr. 2012, T151, 014042–014044. [Google Scholar] [CrossRef]
- Li, Y.Q.; Chen, B. Quantum theory for mesoscopic electric circuits. Phys. Rev. B 1996, 53, 4027–4032. [Google Scholar] [CrossRef]
- Flores, J.C. Mesoscopic circuits with charge discreteness: Quantum transmission lines. Phys. Rev. B 2001, 64, 235309–235313. [Google Scholar] [CrossRef]
- Wang, J.S.; Liu, T.K.; Zhan, M.S. Coulomb blockade and quantum fluctuations of mesoscopic inductance coupling circuit. Phys. Lett. A 2000, 276, 155–161. [Google Scholar] [CrossRef]
- Liu, J.X.; Yan, Z.Y. Quantum Effect in the Mesoscopic RLC circuits with a source. Commu. Theor. Phys. 2005, 44, 1091–1094. [Google Scholar] [CrossRef]
- Ji, Y.H.; Luo, H.M.; Guo, Q. The time evolution of charge and current in mesoscopic LC circuit with charge discreteness. Phys. Lett. A 2005, 349, 104–108. [Google Scholar] [CrossRef]
- Yan, Z.Y.; Zhang, X.H.; Ma, J.Y. Quantum Effect in a Diode Included Nonlinear Inductance-Capacitance Mesoscopic Circuit. Commu. Theor. Phys. 2009, 52, 534–538. [Google Scholar] [CrossRef]
- Liu, J.X.; Yan, Z.Y.; Song, Y.H. Quantum effects of mesoscopic inductance and capacity coupling circuits. Commu. Theor. Phys. 2006, 45, 1126–1130. [Google Scholar]
- Yan, Z.Y.; Zhang, X.H.; Han, Y.H. Quantum Effect in mesoscopic open electron resonator. Commu. Theor. Phys. 2008, 50, 521–524. [Google Scholar] [CrossRef]
- Wang, J.S.; Su, C.Y. Quantum Effect in a Diode Included Nonlinear Inductance-Capacitance Mesoscopic Circuit. Int. J. Theor. Phys. 2009, 37, 1213–1216. [Google Scholar] [CrossRef]
- Yurke, B.; Denker, J.S. Quantum network theory. Phys. Rev. A 1984, 29, 1419–1437. [Google Scholar] [CrossRef]
- Kockum, A.F.; Miranowicz, A.; De Liberato, S.; Savasta, S.; Nori, F. Ultrastrong coupling between light and matter. Nat. Rev. Phys. 2019, 1, 19–40. [Google Scholar] [CrossRef]
- Le Boité, A. Theoretical methods for ultrastrong light-matter interactions. Adv. Quantum Technol. 2020, 3, 1900140. [Google Scholar] [CrossRef]
- Blais, A.; Grimsmo, A.L.; Girvin, S.M.; Wallraff, A. Circuit quantum electrodynamics. Rev. Mod. Phys. 2021, 93, 025005-72. [Google Scholar] [CrossRef]
- Borletto, F.; Giacomelli, L.; Ciuti, C. Circuit QED theory of direct and dual Shapiro steps with finite-size transmission line resonators. arXiv 2024. [Google Scholar] [CrossRef]
- Wang, Z.X.; Guo, D.R. Introduction to Special Functions; Science Press: Bejing, China, 1965; pp. 601–644. [Google Scholar]
- Forati, E.; Langley, B.W.; Nersisyan, A. On the electromagnetic couplings in superconducting qubit circuits. arXiv 2024. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, J.-Y.; Zhao, W.; Wang, W.; Yan, Z.-Y. Improved Quantization Method of Coupled Circuits in Charge Discrete Space. Mathematics 2024, 12, 3753. https://doi.org/10.3390/math12233753
Ma J-Y, Zhao W, Wang W, Yan Z-Y. Improved Quantization Method of Coupled Circuits in Charge Discrete Space. Mathematics. 2024; 12(23):3753. https://doi.org/10.3390/math12233753
Chicago/Turabian StyleMa, Jin-Ying, Weiran Zhao, Weilin Wang, and Zhan-Yuan Yan. 2024. "Improved Quantization Method of Coupled Circuits in Charge Discrete Space" Mathematics 12, no. 23: 3753. https://doi.org/10.3390/math12233753
APA StyleMa, J.-Y., Zhao, W., Wang, W., & Yan, Z.-Y. (2024). Improved Quantization Method of Coupled Circuits in Charge Discrete Space. Mathematics, 12(23), 3753. https://doi.org/10.3390/math12233753




