New Coupled Optical Solitons to Birefringent Fibers for Complex Ginzburg–Landau Equations with Hamiltonian Perturbations and Kerr Law Nonlinearity
Abstract
1. Introduction
2. Governing Model
3. Mathematical Analysis
4. Method for Searching for Propagation of W-Shaped-like Solitons Combined with Other Families of Solitons
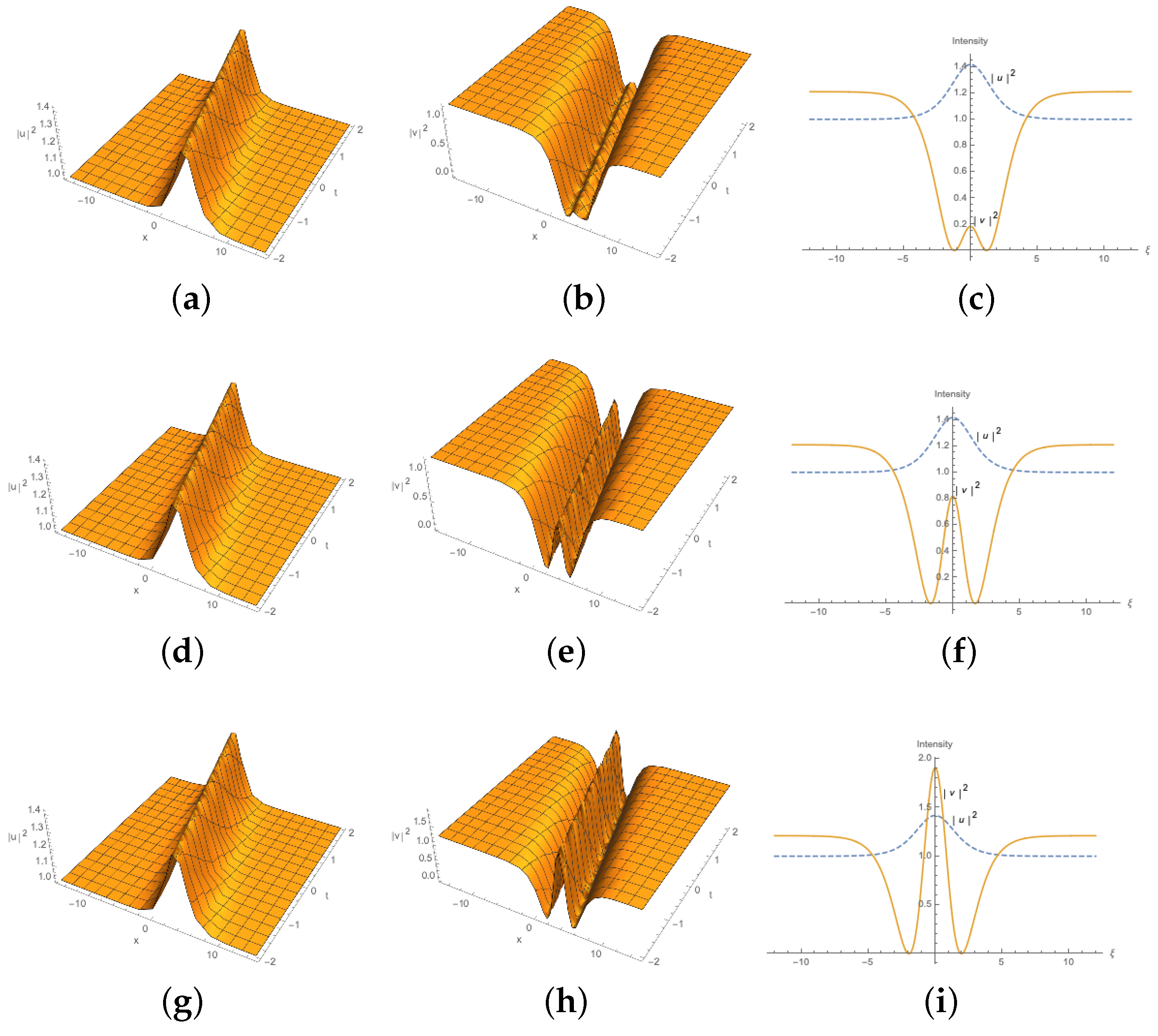
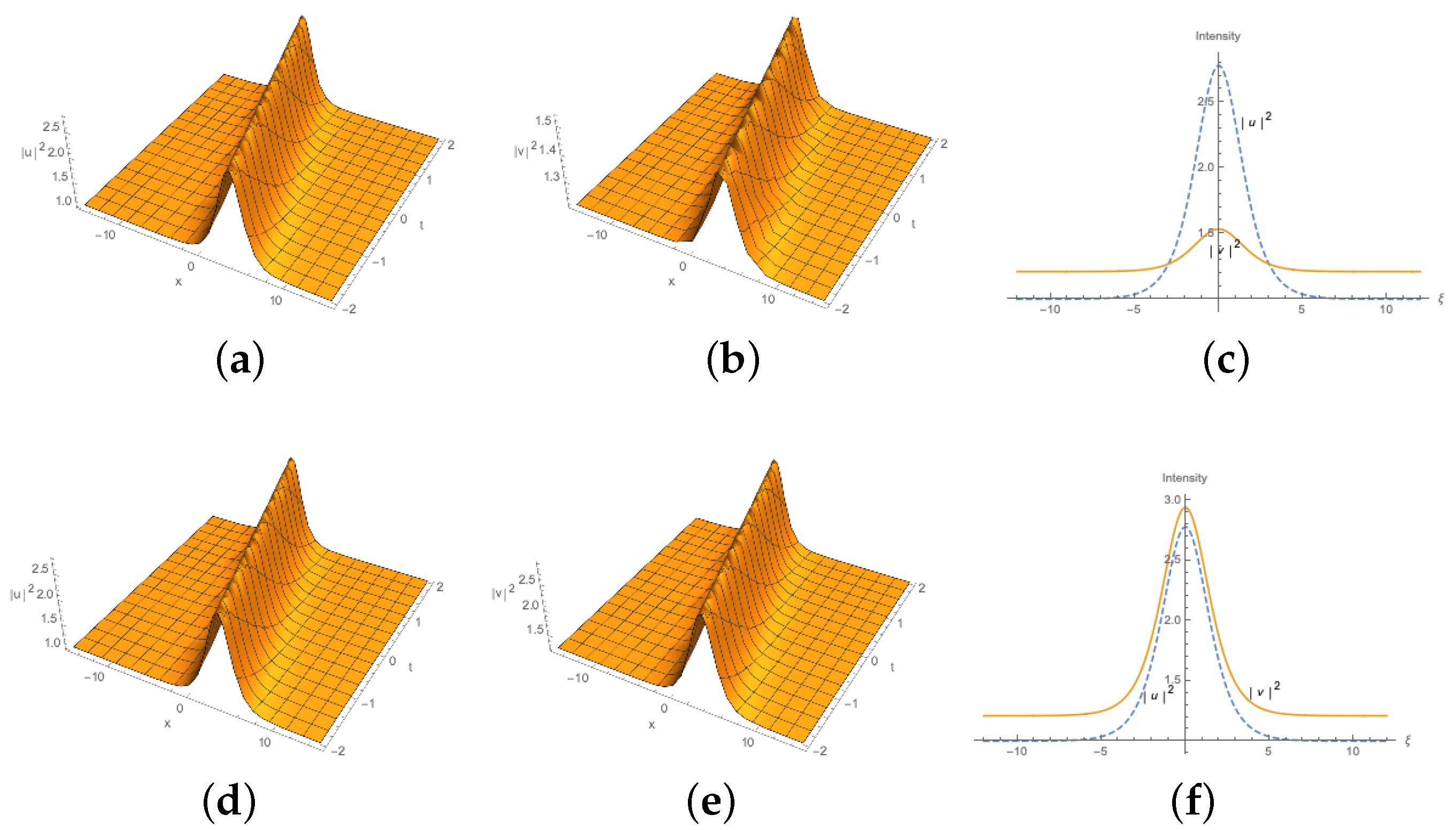
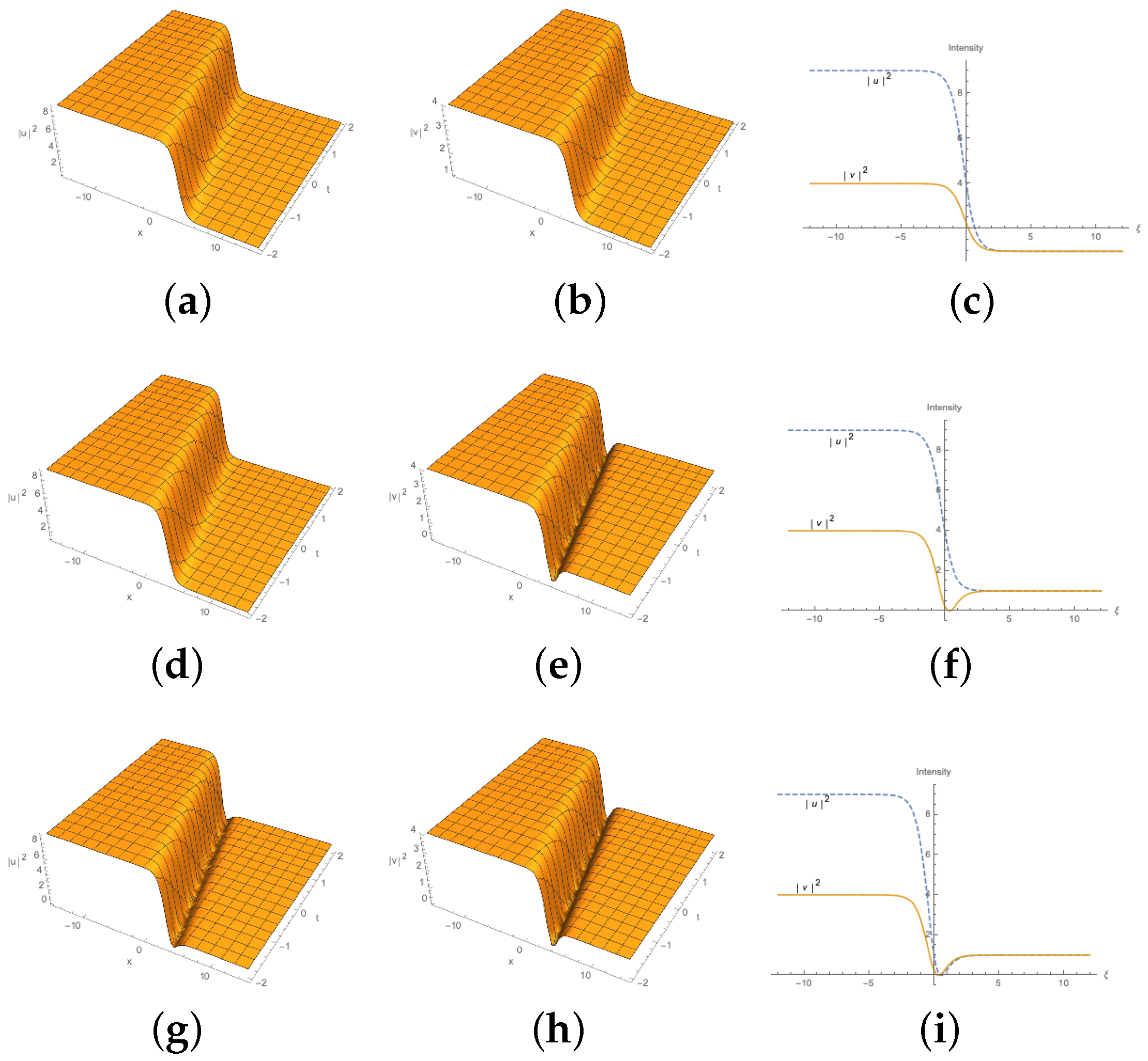
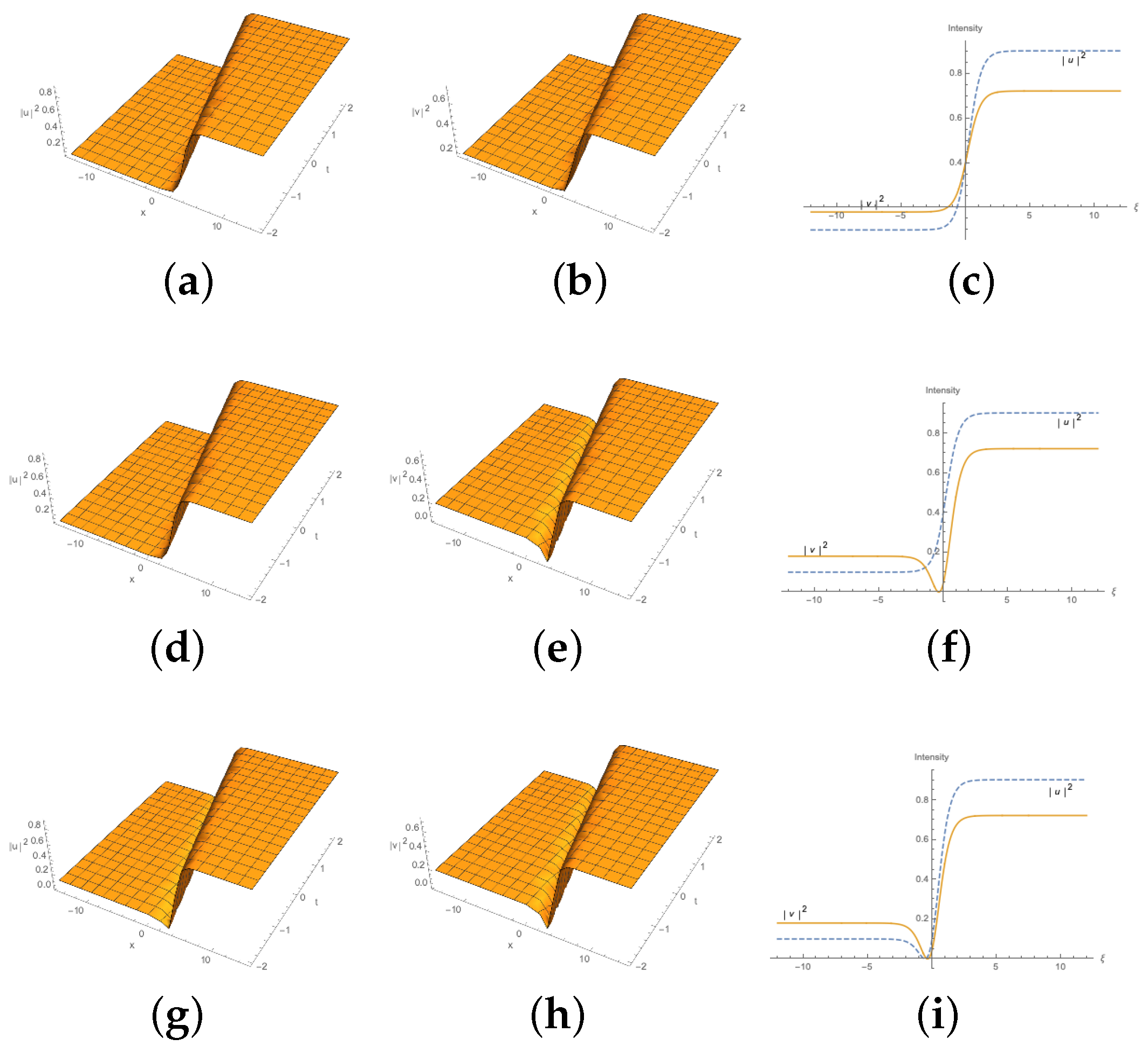
5. Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hietarinta, J.; Joshi, N.; Nijhoff, F.W. Discrete and integrability. In Cambridge Texts in Applied Mathematics; Cambridge University Press: Cambridge, UK, 2016; Volume 54. [Google Scholar]
- Hirota, R. Nonlinear partial difference equations. i. A difference analogue of the Korteweg-de Vries equation. J. Phys. Soc. Jpn. 1977, 43, 1424–1433. [Google Scholar] [CrossRef]
- Nijhoff, F.W.; Quispel, G.R.W.; Capel, H.W. Direct linearization of nonlinear difference-difference equations. Phys. Lett. A 1983, 97, 125–128. [Google Scholar] [CrossRef]
- Inc, M.; Rezazadeh, H.; Vahidi, J.; Eslami, M.; Akinlar, M.A.; Ali, M.N.; Chu, Y.M. New solitary wave solutions for the conformable Klein-Gordon equation with quantic nonlinearity. Aims Math. 2020, 5, 6972–6984. [Google Scholar] [CrossRef]
- Rezazadeh, H.; Younis, M.; Eslami, M.; Bilal, M.; Younas, U. New exact traveling wave solutions to the (2 + 1)-dimensional chiral nonlinear Schrödinger equation. Math. Model Nat. Phenom. 2021, 16, 38. [Google Scholar] [CrossRef]
- Ghanbari, B.; Günerhan, H.; Srivastava, H. An application of the Atangana-Baleanu fractional derivative in mathematical biology: A three-species predator–prey model. Chaos Solitons Fractals 2020, 138, 109910. [Google Scholar] [CrossRef]
- Ghanbari, B.; Inc, M. A new generalized exponential rational function method to find exact special solutions for the resonance nonlinear Schrödinger equation. Eur. Phys. J. Plus. 2018, 133, 142. [Google Scholar] [CrossRef]
- Zhao, T.H.; Wang, M.K.; Chu, Y.-M. Monotonicity and convexity involving general- ized elliptic integral of the first kind. Rev. Real Acad. Cienc. Exactas Físicas Nat. Ser. Matemáticas RACSAM 2021, 115, 46. [Google Scholar]
- Roshani, M.; Phan, G.; Faraj, R.H.; Phan, N.-H.; Roshani, G.H.; Nazemi, B.; Corniani, E.; Nazemi, E.; Hanus, R.; Zych, M.; et al. Proposing a gamma radiation based intelligent system for simultane- ous analyzing and detecting type and amount of petroleum by-products. Nucl. Eng. Technol. 2021, 53, 1277–1283. [Google Scholar] [CrossRef]
- Wang, B.H.; Lu, P.H.; Dai, C.Q.; Chen, Y.X. Vector optical soliton and periodic solutions of a coupled fractional nonlinear Schrödinger equation. Results Phys. 2020, 17, 103036. [Google Scholar] [CrossRef]
- Gardner, C.S.; Green, J.M.; Kruskal, M.D.; Miura, R.M. Method for solving the ko- rteweg-de vries equation. Phys. Rev. Lett. 1967, 19, 1095–1097. [Google Scholar] [CrossRef]
- Dodd, R.K.; Eilbeck, J.C.; Gibbon, J.D.; Morris, H.C. Solitons and Nonlinear Wave Equations; Academic Press: New York, NY, USA, 1982. [Google Scholar]
- Djoko, M.; Tabi, C.B.; Kofane, T.C. Effects of the septic nonlinearity and the initial value of the radius of orbital angular momentum beams on data transmission in optical fibers using the cubic-quintic-septic complex Ginzburg-Landau equation in presence of higher-order dispersions. Chaos Solitons Fractals 2021, 147, 110957. [Google Scholar] [CrossRef]
- Stegeman, G.I.; Segev, M. Optical spatial solitons and their interactions: Universality and diversity. Science 1999, 286, 1518–1523. [Google Scholar] [CrossRef]
- Desaix, M.; Helczynski, L.; Anderson, D.; Lisak, M. Propagation properties of chirped soliton pulses in optical nonlinear Kerr media. Phys. Rev. E 2002, 65, 056602. [Google Scholar] [CrossRef] [PubMed]
- Kruglov, V.I.; Peacock, A.C.; Harvey, J.D. Exact self-similar solutions of the general- ized nonlinear Schrödinger equation with distributed coefficients. Phys. Rev. Lett. 2003, 90, 113902. [Google Scholar] [CrossRef] [PubMed]
- Abbagari, S.; Houwe, A.; Doka, S.Y.; Inc, M.; Bouetou, T.B. Specific optical solitons solutions to the coupled Radhakrishnan–Kundu–Lakshmanan model and modulation instability gain spectra in birefringent fibers. Opt. Quantum Electron. 2022, 35, 1–25. [Google Scholar] [CrossRef]
- Porsezian, K.; Kalithasan, B. Cnoidal and solitary wave solutions of the coupled higher order nonlinear Schrödinger equation in nonlinear optics. Chaos Solitons Fractals 2007, 31, 188–196. [Google Scholar] [CrossRef]
- Kaminow, I. Polarization in optical fibers. IEEE J. Quantum Electron. 1981, 17, 15–22. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Alngar, M.E.M.; El-Horbaty, M.M.; Biswas, A.; Kara, A.H.; Ekici, M.; Asma, M.; Alzahran, A.K.; Belic, M.R. Solitons and conservation laws in magneto-optic waveguides having parabolic-nonlocal law of refractive index. Phys. Lett. A 2020, 384, 126814. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Alngar, M.E.M.; Shohib, R.M.A.; Biswas, A.; Yildirim, Y.; Dakova, A.; Alshomrani, A.S.; Alshehri, H.M.; Belic, M.R. Cubic-quartic solitons in couplers with optical metamaterials having polynomial law of nonlinearity. Optik 2021, 248, 168087. [Google Scholar] [CrossRef]
- Triki, H.; Zhou, Q.; Liu, W.; Biswas, A.; Moraru, L.; Yildirim, Y.; Alshehri, H.M.; Belic, M.R. Chirped optical soliton propagation in birefringent fibers modeled by coupled Fokas-Lenells system. Chaos Solitons Fractals 2022, 155, 111751. [Google Scholar] [CrossRef]
- Abbagari, S.; Saliou, Y.; Houwe, A.; Akinyemi, L.; Inc, M.; Bouetou, T.B. Modulated wave and modulation instability gain brought by the cross-phase modulation in birefringent fibers having anti-cubic nonlinearity. Optik 2022, 242, 128191. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Alngar, M.E.M.; Shohib, R.M.A.; Biswas, A.; Triki, H.; Yildirim, Y.; Alshomrani, A.S.; Alshehri, H.M. Cubic-quartic optical solitons in birefringent fibers with Sasa-Satsuma equation. Nucl. Eng. Technol. 2021, 53, 1277–1283. [Google Scholar] [CrossRef]
- Rehman, H.U.; Ullah, N.; Imran, N.M.A.; Akgul, A. Optical Solitons of Two Non-linear Models in Birefringent Fibres Using Extended Direct Algebraic Method. Int. J. Appl. Comput. Math. 2021, 7, 227. [Google Scholar] [CrossRef]
- Khalifa, A.S.; Badra, N.M.; Ahmed, H.M.; Rabie, W.B. Retrieval of optical solitons in fiber Bragg gratings for high-order coupled system with arbitrary refractive index. Optik 2023, 287, 171116. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Gepreel, K.A.; El-Horbaty, M. Highly dispersive optical solitons in fiber Bragg gratings with stochastic perturbed Fokas-Lenells model having spatio-temporal dispersion and multiplicative white noise. Optik 2023, 286, 170975. [Google Scholar] [CrossRef]
- Zayed, E.M.E.; Shohib, R.M.A.; Alngar, M.E.M. Dispersive optical solitons in birefringent fibers for (2+1)-dimensional NLSE with Kerr law nonlinearity and spatio-temporal dispersion having multiplicative white noise via Itô calculus. Optik 2022, 267, 69667. [Google Scholar] [CrossRef]
- Zhang, J. Optical solitons of Sasa-Satsuma equation in birefringent fibers. Optik 2022, 270, 170070. [Google Scholar] [CrossRef]
- Yomba E, Method of searching coupled optical solitons to magneto- optic waveguides having parabolic-nonlocal law of refractive index. Phys. Scr. 2024, 99, 045238. [CrossRef]
- Yomba, E. Method of searching for a W-shaped like soliton combined with other families of solitons in coupled equations: Application to magneto-optic waveguides with quadratic-cubic nonlinearity. Opt. Quant. Electron. 2024, 56, 752. [Google Scholar] [CrossRef]
- Samir, I.; Badra, N.; Ahmed, H.M.; Arnous, A.H. Solitons in birefringent fibers for CGL equation with Hamiltonian perturbations and Kerr law nonlinearity using modified extended direct algebraic method. Commun. Nonlinear Sci. Numer. Simulat. 2021, 102, 105945. [Google Scholar] [CrossRef]
- He, J.H.; Wu, X.H. Exp-function method for nonlinear wave equations. Chaos Solitons Fractals 2006, 30, 700–708. [Google Scholar] [CrossRef]
- Ma, W.X. Four-component combined integrable equations possessing bi-Hamiltonian formulations. Mod. Phys. Lett. B 2024, 38, 2450319. [Google Scholar] [CrossRef]
- Ma, W.X. A combined Liouville integrable hierarchy associated with a fourth-order matrix spectral problem. Commun. Theor. Phys. 2024, 76, 075001. [Google Scholar] [CrossRef]
- Benoudina, N.; Zhang, W.Y.; Zhang, Y.; Wazwaz, A.M. New study of (3 + 1)-dimensional nonlinear evolution equation with main part mKdV equation and novel solitary wave solutions. Int. J. Mod. Phys. B 2024, 38, 22. [Google Scholar] [CrossRef]
- Ma, W.X. Integrable Couplings and Two-Dimensional Unital Algebras. Axioms 2024, 13, 481. [Google Scholar] [CrossRef]
- Yomba, E. sn-cn, sn-dn, cn-dn Jacobi elliptic functions and solitons solutions in birefringent fibers for CGL equations with Hamiltonian perturbations and Kerr law nonlinearity. Optik 2022, 271, 170136. [Google Scholar] [CrossRef]
- Kruglov, V.I.; Harvey, J.D. Solitary waves in optical fibers governed by higher-order dispersion. Phys. Rev. A 2018, 98, 063811. [Google Scholar] [CrossRef]




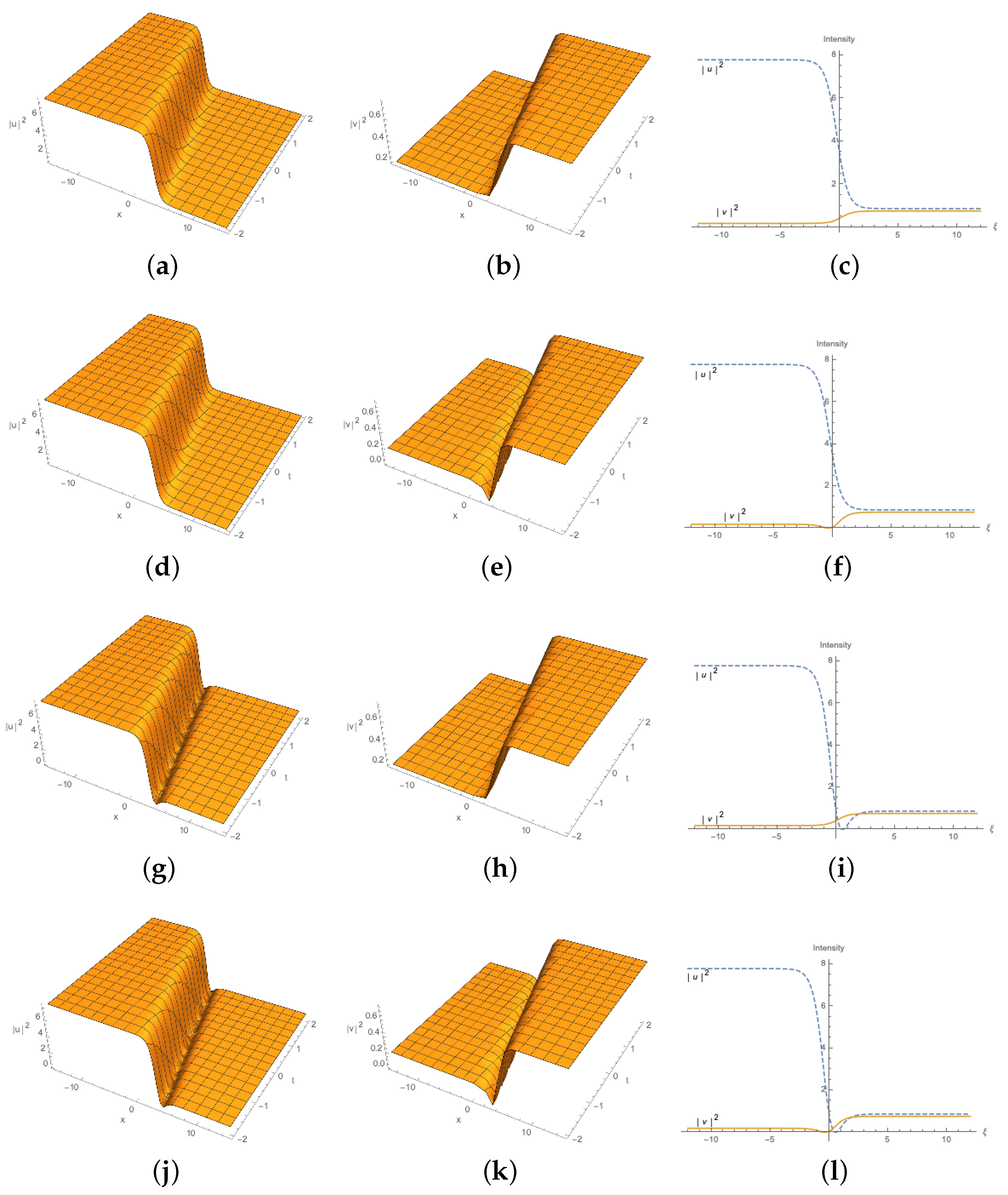
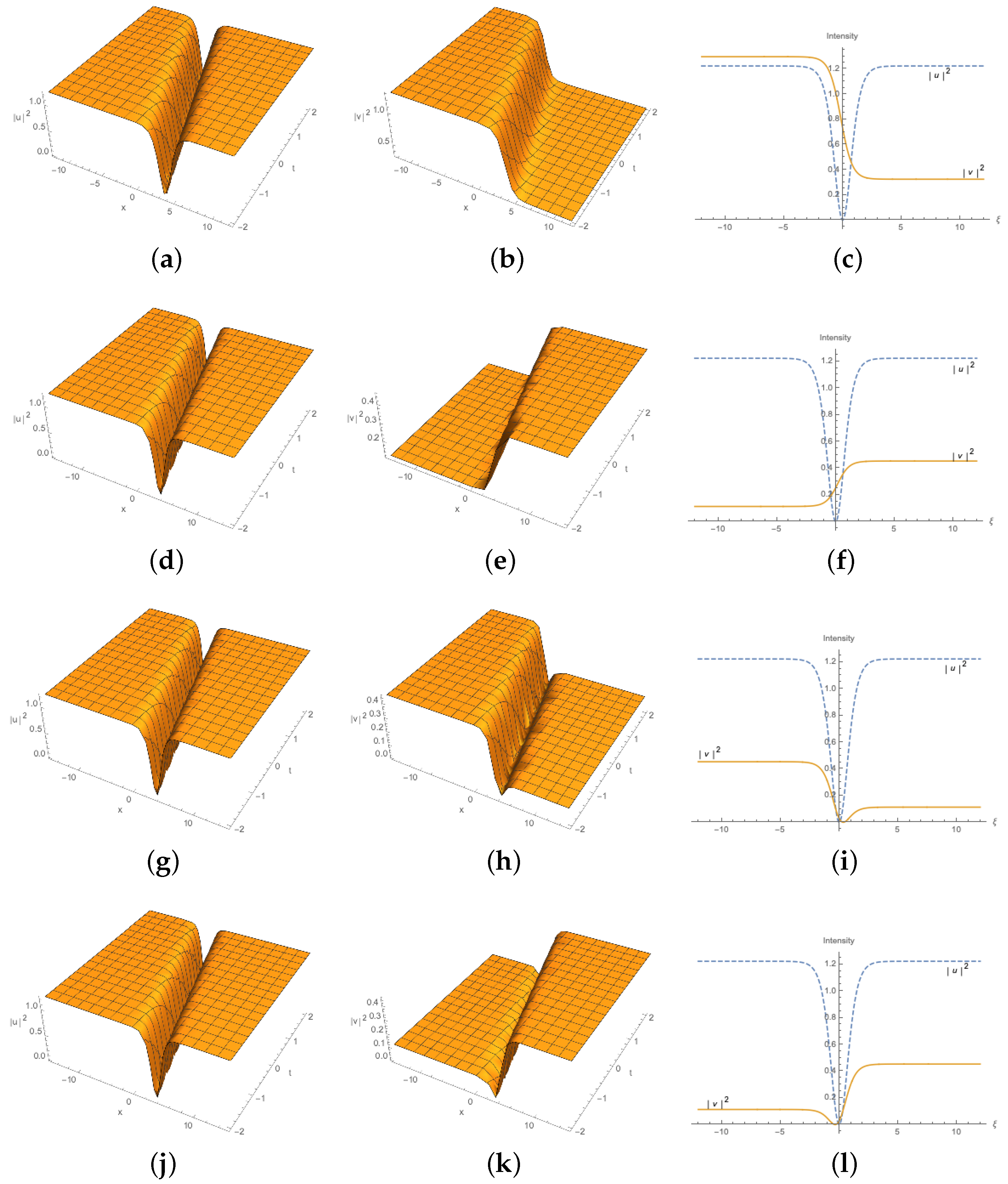
| Figure Number | Maximum Error | Figure Number | Maximum Error |
|---|---|---|---|
| Figure 1a–c | Figure 1d–f | ||
| Figure 1g–i | Figure 1j–l | ||
| Figure 1m–o | Figure 2a–c | ||
| Figure 2d–f | Figure 2g–i | ||
| Figure 2j–l | Figure 3a–c | ||
| Figure 3d–f | Figure 3g–i | ||
| Figure 4a–c | Figure 4d–f | ||
| Figure 5a–c | Figure 5d–f | ||
| Figure 6a–c | Figure 6d–f | ||
| Figure 7a–c | Figure 7d–f | ||
| Figure 7g–i | Figure 8a–c | ||
| Figure 8d–f | Figure 8g–i | ||
| Figure 9a–c | Figure 9d–f | ||
| Figure 9>g–i | Figure 9j–l | ||
| Figure 10a–c | Figure 10d–f | ||
| Figure 10g–i | Figure 10j–l |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yomba, E.; Ramchandra Nair, P. New Coupled Optical Solitons to Birefringent Fibers for Complex Ginzburg–Landau Equations with Hamiltonian Perturbations and Kerr Law Nonlinearity. Mathematics 2024, 12, 3073. https://doi.org/10.3390/math12193073
Yomba E, Ramchandra Nair P. New Coupled Optical Solitons to Birefringent Fibers for Complex Ginzburg–Landau Equations with Hamiltonian Perturbations and Kerr Law Nonlinearity. Mathematics. 2024; 12(19):3073. https://doi.org/10.3390/math12193073
Chicago/Turabian StyleYomba, Emmanuel, and Poonam Ramchandra Nair. 2024. "New Coupled Optical Solitons to Birefringent Fibers for Complex Ginzburg–Landau Equations with Hamiltonian Perturbations and Kerr Law Nonlinearity" Mathematics 12, no. 19: 3073. https://doi.org/10.3390/math12193073
APA StyleYomba, E., & Ramchandra Nair, P. (2024). New Coupled Optical Solitons to Birefringent Fibers for Complex Ginzburg–Landau Equations with Hamiltonian Perturbations and Kerr Law Nonlinearity. Mathematics, 12(19), 3073. https://doi.org/10.3390/math12193073







