Solution of Inverse Photoacoustic Problem for Semiconductors via Phase Neural Network

Abstract

The inverse photoacoustic problem is an ill-posed mathematical physics problem. There are many methods of solving the inverse photoacoustic problem, from parameter reduction to the development of complex regularization algorithms. The idea of this work is that semiconductor physical properties are determined from phase characteristic measurements because phase measurements are more sensitive than amplitude measurements. To solve the inverse photoacoustic problem, the thermoelastic properties and thickness of the sample are estimated using a neural network approach. The neural network was trained on a large database of photoacoustic phases calculated from a theoretical Si n-type model in the range of 20 Hz to 20 kHz, to which random Gaussian noise was applied. It is shown that in solving the inverse photoacoustic problem, high accuracy and precision can be achieved by applying phase measurement and neural network approaches. This study showed that a multi-parameter inverse problem can be solved using phase networks.

1. Introduction

Frequency photoacoustics (PA) is the first [1,2] and most widespread photothermal (PT) method [3,4,5,6,7] used to determine numerous physical properties of various materials, from metals [8,9,10] and semiconductors [11,12,13,14,15,16] through modern multifunctional and low-dimensional materials [17,18,19,20,21,22] and biological tissues [23,24,25,26,27,28,29] to complex nanoelectronic devices and sensors [10,12,30,31,32,33,34]. In this method, the sample is excited by a sinusoidally modulated light beam, and the amplitudes and phase shifts of the pressure fluctuations in the gaseous environment of the sample are recorded at each modulation frequency.

The size of the amplitude and the phase shift in semiconductors depend on the optical, thermal, elastic and electronic properties of the tested sample because these properties control the processes excited by the laser–material interaction and the heating of the sample (PT effect) produced by this interaction [35,36,37]. However, the influence of some properties is expressed at low excitation frequencies (thermal and optical) and others at high modulation frequencies (elastic and electronic) [35,36,37]. In addition, some properties, such as elasticity, have a greater effect on the phase in the lower frequency range than on the amplitude. In any case, the inverse PA problem, that is, determining the properties of the sample from the measured signals, represents a multi-parameter, nonlinear and ill-posed problem of mathematical physics, which is very complex to solve.

In the literature, one can find several approaches to solving this problem, but there is no unique algorithm for solving it [8,10,19,37]. Each of the approaches is adapted to the characteristics of measured PA amplitudes and/or phases for a given material and to the frequency range that can be achieved with a given experimental setup. Most often, the measured PA amplitude is used, and the inverse problem is reduced to a one-parameter one due to the availability of only low-frequency measured amplitudes [38,39,40,41,42], and only the thermal diffusivity of the sample is determined [1,2].

However, an approach has recently been developed in which neural networks (NNs) are used for multi-parameter solving of the inverse PA problem [8,10,35,37,43,44] or for the removal of influences of detector transfer characteristics [45]. NNs have proven to be a powerful tool in solving many multi-parameter problems [45,46,47]. It has been shown that this approach measures the thermoelastic properties of materials with great accuracy and precision if both amplitude and phase measurements are used simultaneously, and the accuracy remains very high even when only amplitude measurements are used [48]. Since the measured amplitudes of PA signals are in the range of several orders of magnitude, the sensitivity of amplitude measurements is low, and the data themselves must be scaled during network processing.

It is known that phase measurements are more sensitive, and the measured phases move in the range of 2π, so it can be expected that using only phase measurements could lead to more accurate values of the sample parameters, without additional scaling of the input data [49,50,51,52]. Indeed, it is true that both amplitude and phase are recorded by detecting the photoacoustic response. The shift of the photoacoustic signal is recorded as phase, which is more stable in the experimental measurement, which practically reduces the measurement uncertainty. In practical work, to determine the amplitude value, it is necessary to know the exact power absorbed in the sample [53]. Determining the power absorbed in the sample requires precise determination of the power of light falling on the surface of the sample, then precise determination of the reflection and absorption of the surface layer of the sample, which is a demanding process.

In this work, phase neural networks were developed to predict three properties of a semiconductor sample: thermal diffusivity, linear expansion coefficient and sample thickness. This procedure is part of the inverse solution of the photoacoustic problem, where the properties of matter are determined from the photoacoustic signal. Network training was performed on numerical photoacoustic signals obtained from a theoretical mathematical model in the frequency range 20 Hz to 20 kHz, which is given in Section 2. In addition to the presentation of the formation of the phase neural network, the Gaussian noise is added to the input data of the phase neural network in order to analyze the measurement errors that occur during a real experiment, as described in Section 3. It has been shown by the results that, in this way, the problems caused by overtraining the network on theoretical data can be overcome because the thermoelastic properties of semiconductors can be determined by phase networks with an expected error of less than 2%, as described in Section 4. A relevant discussion and conclusions are provided in Section 5.

2. Phase of Photoacoustic Response of Semiconductors—Direct PA Problem

The theoretical mathematical consideration is reflected in the consideration of the physical processes of the circular sample, a plate that is directly placed on the photoacoustic cell, in the configuration supported by the sample, when the sample is illuminated with modulated light of the form $I=I_0\mathrm{Re}\left(1+e^{i\mathsf{\omega }t}\right)$, where $I_0$ is the amplitude of the incident light, $\omega =2\pi f$ and $f$ is the modulation frequency. The result is absorption, photogenerated electron–hole pairs, $\delta n_p(x,f)$, as shown in Appendix A, and deexcitation relaxation processes that cause heating through the sample. These processes lead to a temperature distribution $T(x,f)$, as shown in Appendix B, that causes various effects explained by the composite piston theory [1,2,11,12,54]. These changes in the frequency domain are recorded as a photoacoustic response in the form of a pressure change in the photoacoustic cell $\delta p_{\mathrm{total}}\left(f\right)=A\left(f\right)e^{-i\phi \left(f\right)}$, where $A\left(f\right)$ is amplitude and $\phi \left(f\right)$ is the phase of the total photoacoustic signal. The total photoacoustic signal in the frequency domain 20 Hz to 20 kHz is considered.

During the interaction of laser radiation with a semiconductor, there is thermalization of the lattice (excitation of phonons) but also photogeneration of electron–hole pairs when the energy gap of the semiconductor is lower than the energy of the excitation photons [11,12,54]. Because of the electron–phonon interaction, the properties of the subsystem of quasi-free charge carriers, electrons and cavities change, but there is also a feedback effect of excited electrons on the phonon subsystem. This effect has been studied in the literature using the non-adiabatic small polaron model, and it has been shown that it can significantly affect the thermal properties of a sample exposed to excitation electromagnetic radiation [55,56,57,58,59]. However, due to the strong covalent bonds between the structural elements in silicon, the importance of this effect is very small, so it is neglected in the consideration of the photoacoustic effect in semiconductors.

In further consideration, the conduction of optically generated heat through a semiconductor sample was observed based on the classic Fourier theory of heat conduction, and the influence of photogenerated carriers was taken into account through additional heat sources proportional to the concentration of photogenerated excess charge [3,10]. Temperature variations on the surface of the sample, due to the small depth of penetration into the air that fills the PA cell, lead to expansion and contraction of a thin layer of gas and form a so-called thermal piston that causes pressure fluctuations in the cell. The detection of the thermal component piston model is directly due to the process of diffusion through the sample $\delta p_{\mathrm{TD}}\left(f\right)$ [1,2]. Temperature variations along the sample produce elastic waves within the sample, and these waves cause displacement of the sample surface that forms a mechanical piston in the PA cell. This is the cause of the thermoelastic component of the PA signal $\delta p_{\mathrm{T}\mathrm{E}}\left(f\right)$ [11,12]. And finally, the photogenerated carriers propagate through the sample, causing additional displacement of the sample surface, so that apart from heat sources, they affect the appearance of an additional plasma-elastic component $\delta p_{\mathrm{P}\mathrm{E}}\left(f\right)$ of the mechanical piston [12]:

$$\delta p_{\mathrm{TD}}(f)={\displaystyle \frac{\gamma p_0\sqrt{{\mu }_g}}{l_0{T}_0\sqrt{2}}}T(l)e^{i(\omega t-\pi /4)},$$

(1)

$$\delta p_{\mathrm{TE}}(f)={\alpha }_T{\displaystyle \frac{3\pi \gamma p_0{R}_s^4}{V_0{l}^3}}{\displaystyle \underset{-l/2}{\overset{l/2}{\int }}zT(x,f)dx},$$

(2)

$$\delta p_{\mathrm{PE}}(x,t)=d_n{\displaystyle \frac{p_0\gamma }{V_0}}{\displaystyle \frac{R_s^4}{l^3}}{M}_n=d_n{\displaystyle \frac{p_0\gamma }{V_0}}{\displaystyle \frac{R_s^4}{l^3}}{\displaystyle \underset{-l/2}{\overset{l/2}{\int }}x\delta n_p(x,f)dx},$$

(3)

where $p_0$, $T_0$ and $V_0$—pressure, temperature and volume of photoacoustic cell cavity; $l_0$ is depth of cell; $R_s$ and $l$—sample radius and thickness as tiles; ${\mu }_g$—thermal diffusion length; ${\alpha }_{\mathrm{T}}$—coefficient of linear expansion; $\gamma$—adiabatic ratio; $d_n$—coefficient of electronic deformation; $T(l)$—distribution of the temperature of the rear side of the sample—non-illuminated side; $T(x,f)$—distribution of temperature in the sample; $\delta n_p(x,f)$—distribution of excess carrier (holes) in the semiconductor.

Therefore, the total photoacoustic signal of a semiconductor is the sum of three components with different dominant effects:

$$\delta p_{\mathrm{total}}\left(f\right)=\delta p_{\mathrm{TD}}\left(f\right)+\delta p_{\mathrm{TE}}\left(f\right)+\delta p_{\mathrm{PE}}\left(f\right).$$

(4)

The development of a complex direct theoretical mathematical model that includes all dominant effects enables obtaining a functional multi-parameter dependence, i.e., determining the total photoacoustic signal as a functional dependence of various properties of matter, from optical, mechanical, thermal, elastic, electronic and all other properties that are related to them. The direct model set up in this way enables the inverse solution of the photoacoustic problem, that is, the determination of the properties of matter and the environment that are directly included in the consideration. The advantage of the photoacoustic method is that a signal that is multi-parameter dependent can be obtained with one measurement, and the disadvantage is that inverse solving is an ill-posed problem of mathematical physics, because it can have several equal solutions, only one of which can correspond to the physics of the problem.

Therefore, the nonlinear dependence of the total photoacoustic signal is shown, and we will mention some of those parameters, from 15 to 20 parameters, that are included in the mathematical theoretical model; the parameters of thermal diffusivity $D_{\mathrm{T}}$, linear expansion ${\alpha }_{\mathrm{T}}$, conductivity $k$, coefficient of electronic deformation $d_n$, carrier lifetime $\tau$, surface recombination rate $s_1$ and $s_2$, absorption $\beta$, gap energy ${\epsilon }_g$, photon energy $h\nu$ and adiabatic ratio $\gamma$, as well as many others can be analyzed and solved inversely. The advantage of artificial intelligence in inverse photoacoustic solving enables a different approach compared to numerical and analytical solutions, and properties can be determined very precisely and in real time, Equations (1)–(4), Appendix A and Appendix B.

The inverse solution of the photoacoustic signal of the semiconductor, n-type silicon, showed that the parameters can be very accurately determined from the neural network that was trained on the coupled characteristics of the amplitudes and phases of the photoacoustic signals, as shown in articles [43,44]. It has been shown that the amplitude-phase neural network is more dominant in prediction compared to the phase neural network and the amplitude neural network in determining the properties of thermal diffusion, linear expansion and thickness. In this article, by developing the methodology of adding random Gaussian noise, we showed that the formed neural network on the phases can be improved and show the degree of sensitivity.

3. Silicon n-Type Phase Neural Network

Machine learning, as a field of artificial intelligence, is a discipline that plays an important role in the development of learning mechanisms in solving practical problems. The basic requirement of this development of neural networks is usability and application potential. The method used in machine learning, which was developed based on the logic of PA, is suitable because it is based on physical considerations and logic that are precisely mathematically defined [1,2,3,10,12] and leads to the formation of unique conclusions. The direct photoacoustic problem is solved by developing a theoretical mathematical model, Equations (1)–(4), as a nonlinear problem in the multi-parameter space of the model parameters $D_{\mathrm{T}}$, ${\alpha }_{\mathrm{T}}$ and $l$, where the inverse solution using the standard procedure is possible with approximations that increase the error solutions.

By simulating a theoretical mathematical model for semiconductor samples of Equations (1)–(4), a base of 5491 lines was obtained, with variations in diffusivity and expansion around the values of pure silicon 9 × 10⁻⁵ m²s⁻¹ and 2.6 × 10⁻⁶ K ⁻¹ in the range of 10%, where we expect the characteristic properties of n-type silicon, and thickness in the range of 100 to 1000 microns. The values of other parameters of silicon n-type used in the numerical simulation are given in Appendix C. Training and testing of the phase neural network was conducted in this range of parameter change values, because testing of the recognition of photoacoustic signals of other materials would not give results on the quality of this phase neural network (PNN). For the I test, we separated every 50th line and formed a network on the rest of the base, Figure 1.

It has been shown that neural networks trained on amplitude-phase characteristics are very precise and that networks trained on the phases of the shade of the loci [43,44,60] lead us to a more detailed analysis. For comparison, a network with a simpler structure was chosen for the formation of a phase neural network, for several reasons. One is that the simple structure of neural networks, i.e., a single-layer neural network, makes it easy to connect data, apply and test, and requires less memory resources on simpler computers. The second is that multi-layer structures, in addition to the requirement for a larger memory space, require more time to connect the data, and the results show the fluctuation in the optimization of different hyperparameters, i.e., the loss of the quality of the trained network according to certain parameters and the gain in quality in the results. The PNN is trained on phase $\phi \left(f_j\right)$ simulations in the auditory frequency domain 20 Hz–20 kHz, with a vector determined by $j=1,\dots ,72$ values at the input $l=1$, which represents the number of neurons of the input layer, as seen in Figure 1. The hidden layer $l=2$ is one, where $i=50$, and the output layer is determined by three neurons $Q_k, k=1,2,3$ with corresponding sample parameters $D_{\mathrm{T}}$, ${\alpha }_{\mathrm{T}}$ and $l$.

The architecture of the PNN is shown in Figure 2. Phases φ(f_j) as input data are corrected with weights $w_{j,i}^1$ and bias ${\theta }_i^1$ is added to them:

$$m_i={\displaystyle \sum _{j=1}^{72}{w}_{j,i}^1\phi \left(f_j\right)+{\theta }_i^1, i=1,\dots ,50.}$$

The sigmoidal function $\sigma \left(z\right)={\left(1+e^{-z}\right)}^{-1}$ normalizes $m_i$ to the range [0, 1]. Thus, $Q_k$ is calculated as the normalized sum corrected $n_k, k=1,2,3$ by weights $w_{i,k}^2 , k=1,2,3$ to which bias ${\theta }_k^2$ is added:

$$Q_k=\sigma \left({\displaystyle \sum _{i=1}^{50}{w}_{i,k}^2\sigma \left({\displaystyle \sum _{j=1}^{72}{w}_{j,i}^1 \phi \left(f_j\right)+{\theta }_i^1}\right)+{\theta }_k^2}\right), k=1,2,3.$$

The value $Q_k, k=1,2,3$ thus obtained is different from that of, D_T, α_T and l learned by the Levenberg–Marquardt algorithm. The mean square error (mse) is calculated, which is reduced in reverse beck-propagation. The reduction of the mse during the epoch continues until the termination criteria determine the performance of the newly formed neural network. The performance of the network determines the reliability that the network can have during prediction.

In Figure 1, the phase base of photoacoustic signals is shown, which is used for PNN training. One of the possibilities that can happen during the training of the neural network based on the photoacoustic signals of the theoretical mathematical model is overfitting [61,62,63,64,65]. When NNs fit too well with data based on which they were trained, they show overtraining, which can be seen with high values of NN training performance; then, they show high precision in prediction on known signals, but large errors in prediction appear on unknown signals. For this reason, we trained on the same base with different noise % levels. PNNs of the same architecture and the same base of input signals, but with different degrees of added noise, were formed.

The change in training performance of a phase neural network, Figure 3, of the same architecture and with the same input and output base data can be seen by adding a different % noise at the input, which we chose to be a random Gaussian noise, of 1 to 5%. Adding a different % of noise level leads to the effect of increasing the robustness of the data, which can be the key to achieving such an improvement of the NN, since the NN better recognizes data of the phase photoacoustic signal on which the network has not been trained. This resulted in a network trained on the basis of phases, NN0, and networks trained on the same basis, NN1 to NN5, with 1 to 5% noise added.

Table 1. Performance and number of epochs of networks on signals with a certain % noise level.

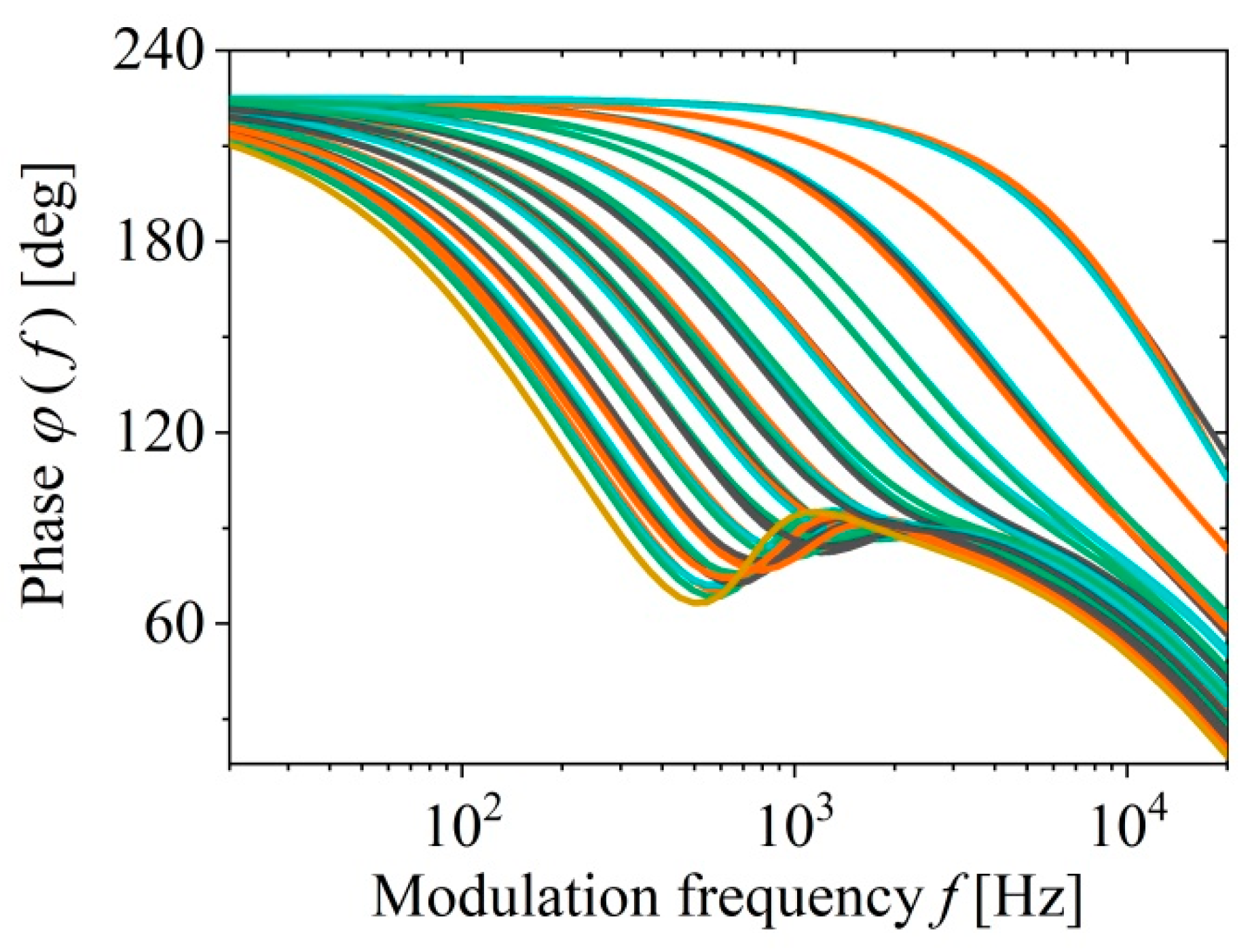
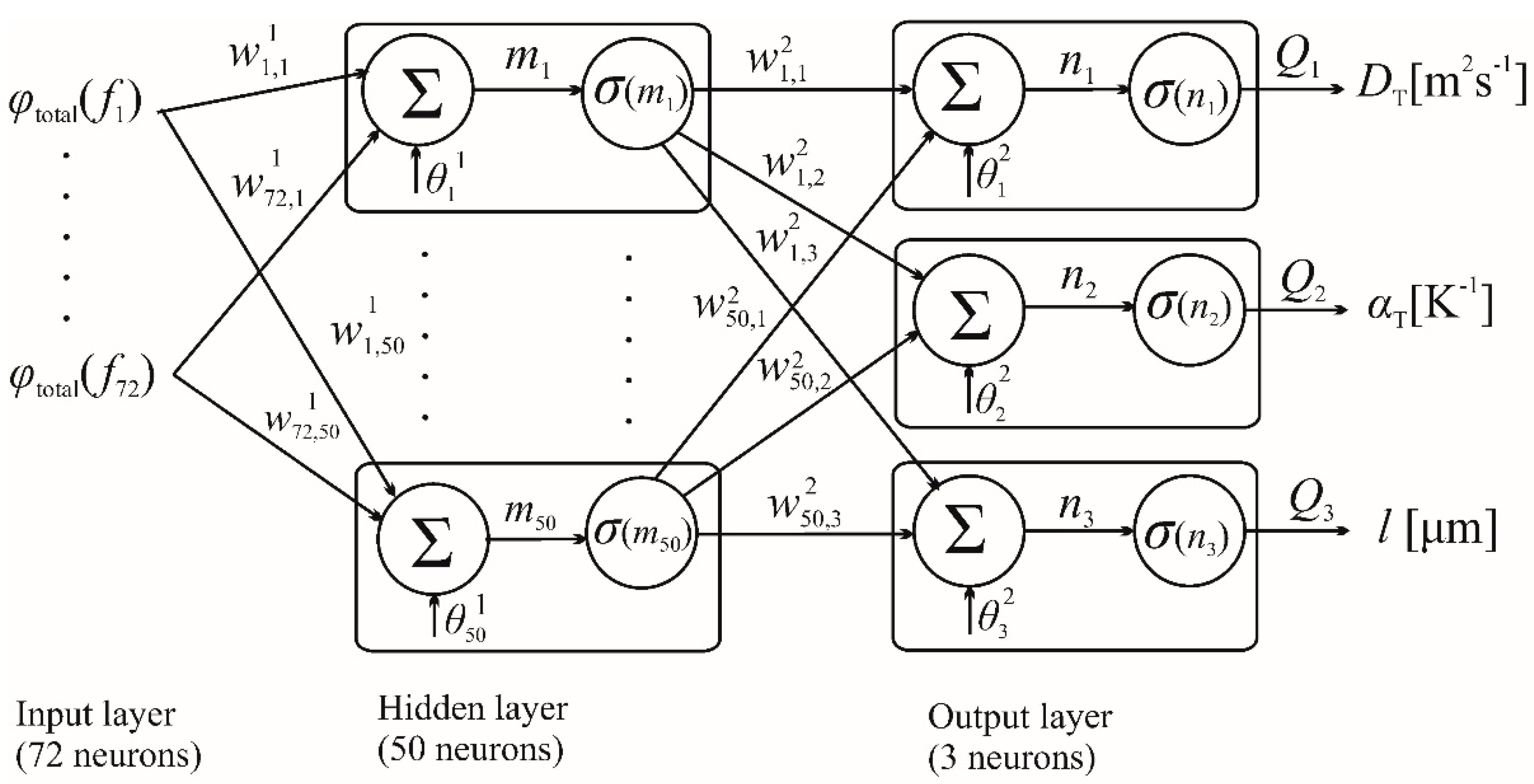
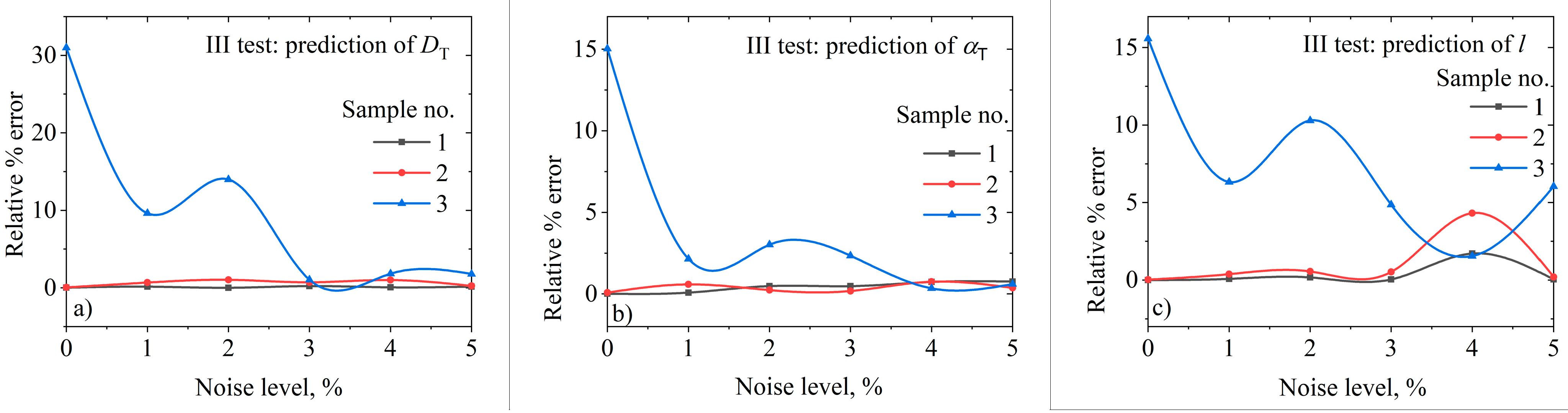
NN	Noise	Performance	Epoch
NN0	0	0.0000017246	1000
NN1	1%	0.015800	44
NN2	2%	0.037952	9
NN3	3%	0.052391	8
NN4	4%	0.072174	6
NN5	5%	0.070721	5

shows that if the level % of noise that is added to the input data of the base before training is increased, it leads to a decrease in the performance of neural networks NN1-NN5 compared to the original neural network NN0. On the other hand, the increase in the level % of the added noise led to a shortening of the training time, which reduced the number of epochs from 1000 (which was the termination criterion for NN training) to 5 epochs. With this, the total training time of NN was significantly shortened, and it depends on the configuration of the device. It seems that increasing the level % of added noise reduced the good characteristics of the obtained networks, Table 1, Figure 3, but the justification of the added noise was confirmed only on the predictions of the numerical phases of the experiment [43,44].

To determine which network is most reliable in predicting the data, we performed three tests: the I test on phase data of photoacoustic signals, on which the network was not trained, and were extracted from the database before training; the II test on numerically simulated data showing photoacoustic phases in the operating range of the phase neural network; the III test, which is a prediction on phases of numerical experiments of three samples, nos. 1, 2 and 3.

4. Inverse Solution of the Photoacoustic Problem

The accuracy of PNN operation with different % noise levels can be analyzed in three different tests, on bases formed in different ways. The base for the I test was formed by extracting the signal from the base obtained by the numerical simulation of the model in the range of parameter changes of 10% from the reference values of pure Si. Every 50th signal was extracted from the base, making a total of 110 signals. The basis for the II test was formed by numerical random selection of parameters and in the range of PNN operation. The basis for the III test is a numerical experiment for three signals of samples of the same composition of matter but of different thicknesses.

The I test on the phases of 110 photoacoustic signals shows that the network with the lowest noise level, NN0, has the lowest error and error of the maximum %, and the average % tends to increase in the prediction of diffusivity $D_{\mathrm{T}}$, expansion ${\alpha }_{\mathrm{T}}$ and thickness $l$ with an increase in the level % of added noise, Figure 4 and

Table 2. The I test: the % relative errors, max and averaged parameter prediction $D_{\mathrm{T}}$, ${\alpha }_{\mathrm{T}}$ and $l$ for PNN with specified % noise level.

I Test	Max % Error			Average % Error
	${\mathit{D}}_{\mathbf{T}}$	${\mathit{\alpha }}_{\mathbf{T}}$	$\mathit{l}$	${\mathit{D}}_{\mathbf{T}}$	${\mathit{\alpha }}_{\mathbf{T}}$	$\mathit{l}$
0	0.0273	0.0542	0.0488	0.0040	0.0137	0.0041
1%	4.9486	4.0797	2.6102	0.6748	0.7016	0.3984
2%	10.7968	7.8927	6.0712	2.0360	2.3332	1.0478
3%	11.8123	7.9326	5.3684	2.7558	2.8824	1.2435
4%	10.3026	7.8952	7.6432	3.0014	2.9103	2.5467
5%	10.8236	8.5714	7.6446	3.4590	3.1694	1.8964

.

The II test is a test on phases randomly selected from the range of the parameters. The network without added noise has the largest error on the phases of samples of small thicknesses. By adding noise (1%), thin sample errors are reduced to the optimal level for this test and then (>1%) tend to increase (Figure 5 and

Table 3. The II test: the % relative errors, max and averaged parameter prediction for phasic neural networks with specified % noise level.

II Test	Max % Error			Average % Error
	${\mathit{D}}_{\mathbf{T}}$	${\mathit{\alpha }}_{\mathbf{T}}$	$\mathit{l}$	${\mathit{D}}_{\mathbf{T}}$	${\mathit{\alpha }}_{\mathbf{T}}$	$\mathit{l}$
0	31.6433	15.3729	16.3180	2.3443	1.3165	1.1680
1%	6.4489	2.6458	5.2358	1.0022	0.7648	0.5999
2%	14.2476	4.7840	13.8016	1.7041	1.6064	1.2381
3%	6.7114	6.9429	5.4485	1.8062	2.3598	1.0484
4%	7.0108	7.8894	4.3250	2.2337	2.4526	2.0073
5%	8.3557	8.6263	6.7657	2.6509	2.6872	1.3180

).

Justification of adding noise in different % levels led to an increase in the robustness of the data (signal), i.e., it prevented overfitting during the training of the NN1–5 networks, which can be seen on the parameter predictions of the NN0–NN5 networks on the signal phases of the numerical experiments, sample nos. 1–3. The prediction values of the diffusion, linear expansion and thickness for the various parameters are given in the blue columns in

Table 4. The III test: prediction of three parameters by phase neural networks (with certain % noise level) and relative % prediction error of three samples.

PNN	Sample No. 1			Sample No. 2			Sample No. 3
Parameters	${\mathit{D}}_{\mathbf{T}}^{\mathbf{ANN}}$	${\mathit{\alpha }}_{\mathbf{T}}^{\mathbf{ANN}}$	${\mathit{l}}^{\mathbf{ANN}}$	${\mathit{D}}_{\mathbf{T}}^{\mathbf{ANN}}$	${\mathit{\alpha }}_{\mathbf{T}}^{\mathbf{ANN}}$	${\mathit{l}}^{\mathbf{ANN}}$	${\mathit{D}}_{\mathbf{T}}^{\mathbf{ANN}}$	${\mathit{\alpha }}_{\mathbf{T}}^{\mathbf{ANN}}$	${\mathit{l}}^{\mathbf{ANN}}$
Unit	10−5 m2s−1	10−6 K−1	102 μm	10−5 m2s−1	10−6 K−1	102 μm	10−5 m2s−1	10−6 K−1	102 μm
0%	9.0011	2.6003	8.2997	8.9958	2.6020	4.1689	11.7889	2.2092	1.4795
Rel % error	0.0127	0.0135	0.0030	0.0464	0.0785	0.0265	30.9883	15.0313	15.5833
1%	9.0131	2.5980	8.3060	9.0610	2.5849	4.1845	9.8674	2.5442	1.3610
Rel % error	0.1457	0.0777	0.0761	0.6780	0.5819	0.3777	9.6194	2.1460	6.3307
2%	9.0009	2.5875	8.2861	9.0930	2.6061	4.1931	10.2581	2.5214	1.4117
Rel % error	0.0099	0.4800	0.1666	1.0341	0.2357	0.5538	13.9786	3.0199	10.2928
3%RGN	9.0196	2.5876	8.3133	9.0642	2.6045	4.1918	9.0941	2.6609	1.3422
Rel % error	0.2183	0.4766	0.0394	0.7130	0.1749	0.5226	1.0450	2.3427	4.8579
4%	8.9949	2.58092	8.1578	8.9099	2.6194	3.9900	8.8366	2.6089	1.2998
Rel % error	0.0569	0.7338	1.7136	1.0010	0.7477	4.3163	1.8158	0.3425	1.5488
5%	9.0141	2.5802	8.3038	8.9779	2.5904	4.1616	8.8399	2.6157	1.2026
Rel % error	0.1566	0.7605	0.0460	0.2459	0.3692	0.2023	1.7782	0.6030	6.0439

, while the values of the relative % prediction errors are given in the white columns.

Table 4 establishes that the parameter prediction by the NN0 network has satisfactory precision (small relative % error) on sample nos. 1 and 2, while the lowest precision in the recognition of the parameter from the phase of sample no. 3 is from 15 to 31% relative error. Parameter predictions by networks NN1–5 are such that the precision in guessing parameters from sample phase nos. 1 and 2 is satisfactory (small variations compared to prediction by network NN0 which are <1%), while the precision of parameter prediction from sample phase no. 3 has a tendency to increase, which can be seen in Figure 6.

Figure 7, shows the results of relative % errors of predictions of three parameters (a) diffusion, (b) expansion and (c) thickness of three samples, no. 1 with the black line, no. 2 with the red line and no. 3 with the blue line, based on information in Table 4, from the phases of the photoacoustic signals. Actually at a higher % noise level, from 3 to 3.5%, the optimal NN can be determined, which simultaneously determines precisely in the prediction of all three parameters of diffusion, expansion and thickness, with a relative % error <2.5%.

The results of the inverse solution of the photoacoustic problem from the coupled amplitude-phase characteristics [31,32] give very precise parameter predictions (diffusivity, expansion and thickness) that are more precise than the predictions from the phase neural network NN0. One of the reasons for the lower precision of the phase neural network may be the reduction in the size of the input vector compared to the input vector of the amplitude-phase neural network. The phase neural network gives a satisfactory prediction for larger sample thicknesses, Figure 7 red and black line, but the low precision of a thinner sample, Figure 7 blue line, can be corrected by adding random Gaussian noise at a certain %. Thus, we have shown that thinner samples can be used to determine the noise that exists in the phase during measurement ~3.5%.

5. Discussion and Conclusions

One of the conclusions is that phase data of photoacoustic signals with very low or non-existent % noise can lead to the formation of an overfitting NN, that is, which, due to excessive adaptation to the phases of photoacoustic signals, has high precision on known data, I test, and low precision on unknown data, III test.

In fact, the procedure of adding different levels of % noise to the input data led to an increase in the robustness of the input data, photoacoustic signals, which leads to such an adjustment of weights and gradients during training that it does not lead to an increase in the performance of the formed networks. The formed NNs were trained in a shorter time, and they improved the prediction of data that have variation compared to basic database data. Somehow, by adding noise to the input data of the database, there was an easier prediction of the input data or a reduction in errors in the variation of the input data.

The application of this methodology of adding noise in the preparation of networks that are formed on the data of a mathematical–theoretical model leads to an easier application of NNs in predictions on real data, namely photoacoustic signals. The analysis determines a more general network or, rather, increases the range of added noise in which the data, photoacoustic signals, are recognized with better precision. The range of added noise in % is from 3 to 3.5 for the phase NN on photoacoustic signals. In this way, we have determined the range of % of noise in which the phase NN has better optimization compared to NN0.

The phase neural network without additional noise shows high precision in the prediction of numerical simulations and photoacoustic experimental characteristics of thicker samples. This confirmed that the theoretical mathematical photoacoustic model corresponds to the thickness range of n-type silicon samples, from ~250 microns to 1000 microns. For the photoacoustic characteristics of thinner samples, the phasic neural network prediction has (15–30)% parameter prediction errors. By adding random Gaussian noise of varying degrees, the prediction of the phase neural network on thicker samples remains accurate and relatively unchanged, and on thinner samples, the prediction error drops to an optimal <2.5% for networks with added (3–4)% noise. Finally, our results show that a neural network trained on a large data set of theoretically simulated n-type silicon photoacoustic phases with added Gaussian noise can be a powerful tool for simultaneous, reliable and precise determination of thermal diffusivity, thermal expansion and thickness of a semiconductor sample in real time.