Identifiability and Parameter Estimation of Within-Host Model of HIV with Immune Response
Abstract
1. Introduction
2. Within-Host Model of HIV
2.1. Equilibria and Stability
2.2. Stability Analysis of Infection-Free Equilibrium
2.3. Existence and Local Stability of Infection Equilibrium
3. Structural and Practical Identifiability Analysis and Parameter Estimation
3.1. Structural Identifiability
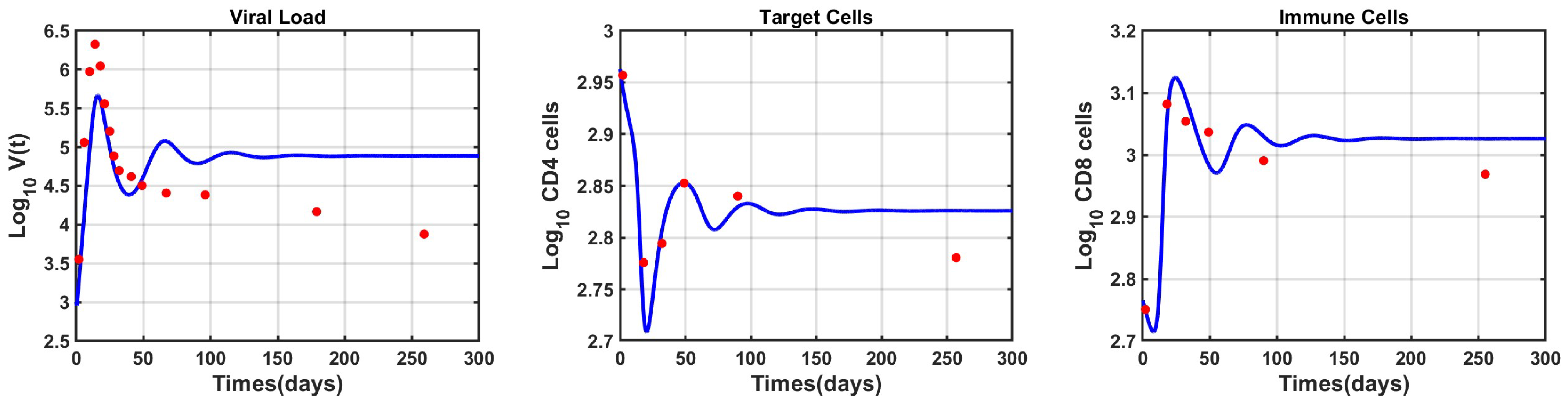
Parameter Estimation
3.2. Practical Identifiability
- We numerically solve the model using the true parameters and collect the output vector at the specific discrete experimental time points.
- We generate 1000 datasets from the statistical model (14) with a given measurement error. These datasets are generated from a normal distribution with the mean corresponding to the output vector obtained in step 1, represented as . The standard deviation is calculated as a of the mean using the formula . Figure 3 presents a series of 12 graphs illustrating the impact of varying measurement errors on the distribution of the generated datasets. Each graph corresponds the viral load, CD4 cell count, and CD8 cell count to a different value of .
- We approximate the parameter set by fitting the within-host model (1) to all simulated datasets.
- Next, we compute the average relative estimation error for each parameter in the model viawhere represents the kth element of p, denotes the kth parameter in the true parameter set , and indicates the kth parameter in the set .
- We repeat steps 1 to 5, gradually increasing the level of noise by considering values of , and .
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Joint United Nations Programme on HIV/AIDS (UNAIDS). Global HIV & AIDS Statistics—Fact Sheet. Available online: https://www.unaids.org/en/resources/fact-sheet (accessed on 20 August 2024).
- Nowak, M.A.; Bangham, C.R. Population Dynamics of Immune Responses to Persistent Viruses. Science 1996, 272, 74–79. [Google Scholar] [CrossRef] [PubMed]
- Perelson, A.S.; Kirschner, D.E.; De Boer, R. Dynamics of HIV Infection of CD4+ T Cells. Math. Biosci. 1993, 114, 81–125. [Google Scholar] [CrossRef] [PubMed]
- Perelson, A.S.; Neumann, A.U.; Markowitz, M.; Leonard, J.M.; Ho, D.D. HIV-1 Dynamics In Vivo: Virion Clearance Rate, Infected Cell Life-Span, and Viral Generation Time. Science 1996, 271, 1582–1586. [Google Scholar] [CrossRef] [PubMed]
- Perelson, A.S.; Nelson, P.W. Mathematical Analysis of HIV-1 Dynamics In Vivo. SIAM Rev. 1999, 41, 3–44. [Google Scholar] [CrossRef]
- Rong, L.; Gilchrist, M.A.; Feng, Z.; Perelson, A.S. Modeling Within-Host HIV-1 Dynamics and the Evolution of Drug Resistance: Trade-Offs Between Viral Enzyme Function and Drug Susceptibility. J. Theor. Biol. 2007, 247, 804–818. [Google Scholar] [CrossRef]
- Nowak, M.; May, R.M. Virus Dynamics: Mathematical Principles of Immunology and Virology; Oxford University Press: Oxford, UK, 2000. [Google Scholar]
- Conway, J.M.; Ribeiro, R.M. Modeling the Immune Response to HIV Infection. Curr. Opin. Syst. Biol. 2018, 12, 61–69. [Google Scholar] [CrossRef]
- D’Orso, I.; Forst, C.V. Mathematical Models of HIV-1 Dynamics, Transcription, and Latency. Viruses 2023, 15, 2119. [Google Scholar] [CrossRef]
- Eller, M.A.; Goonetilleke, N.; Tassaneetrithep, B.; Eller, L.A.; Costanzo, M.C.; Johnson, S.; Betts, M.R.; Krebs, S.J.; Slike, B.M.; Nitayaphan, S.; et al. Expansion of Inefficient HIV-Specific CD8 T Cells During Acute Infection. J. Virol. 2016, 90, 4005–4016. [Google Scholar] [CrossRef] [PubMed]
- Tuncer, N.; Gulbudak, H.; Cannataro, V.L.; Martcheva, M. Structural and Practical Identifiability Issues of Immuno-Epidemiological Vector-Host Models with Application to Rift Valley Fever. Bull. Math. Biol. 2016, 78, 1796–1827. [Google Scholar] [CrossRef]
- Tuncer, N.; Le, T.T. Structural and Practical Identifiability Analysis of Outbreak Models. Math. Biosci. 2018, 299, 1–18. [Google Scholar] [CrossRef]
- Tuncer, N.; Martcheva, M.; LaBarre, B.; Payoute, S. Structural and Practical Identifiability Analysis of Zika Epidemiological Models. Bull. Math. Biol. 2018, 80, 2209–2241. [Google Scholar] [CrossRef] [PubMed]
- Chowell, G.; Dahal, S.; Liyanage, Y.R.; Tariq, A.; Tuncer, N. Structural Identifiability Analysis of Epidemic Models Based on Differential Equations: A Tutorial-Based Primer. J. Math. Biol. 2023, 87, 79. [Google Scholar] [CrossRef] [PubMed]
- Miao, H.; Xia, X.; Perelson, A.S.; Wu, H. ON Identifiability of Nonlinear ODE Models and Applications in Viral Dynamics. SIAM Rev. Soc. Ind. Appl. Math. 2011, 53, 3–39. [Google Scholar] [CrossRef] [PubMed]
- Dankwa, E.A.; Brouwer, A.F.; Donnelly, C.A. Structural identifiability of compartmental models for infectious disease transmission is influenced by data type. Epidemics 2022, 41, 100643. [Google Scholar] [CrossRef]
- Renardy, M.; Kirschner, D.; Eisenberg, M. Structural Identifiability Analysis of Age-Structured PDE Epidemic Models. J. Math. Biol. 2022, 84, 9. [Google Scholar] [CrossRef]
- Massonis, G.; Banga, J.R.; Villaverde, A.F. Structural Identifiability and Observability of Compartmental Models of the COVID-19 Pandemic. Annu. Rev. Control 2021, 51, 441–459. [Google Scholar] [CrossRef]
- Gallo, L.; Frasca, M.; Latora, V.; Russo, G. Lack of practical identifiability may hamper reliable predictions in COVID-19 epidemic models. Sci. Adv. 2022, 8, eabg5234. [Google Scholar] [CrossRef]
- Bellu, G.; Saccomani, M.P.; Audoly, S.; D’Angiò, L. DAISY: A New Software Tool to Test Global Identifiability of Biological and Physiological Systems. Comput. Methods Programs Biomed. 2007, 88, 52–61. [Google Scholar] [CrossRef]
- Eisenberg, M.C.; Robertson, S.L.; Tien, J.H. Identifiability and estimation of multiple transmission pathways in Cholera and waterborne disease. J. Theor. Biol. 2013, 324, 84–102. [Google Scholar] [CrossRef]
- Ljung, L.; Glad, T. On Global Identifiability for Arbitrary Model Parametrizations. Automatica 1994, 30, 265–276. [Google Scholar] [CrossRef]
- Pohjanpalo, H. System Identifiability Based on the Power Series Expansion of the Solution. Math. Biosci. 1978, 41, 21–33. [Google Scholar] [CrossRef]
- Walter, E.; Pronzato, L.; Norton, J. Identification of Parametric Models from Experimental Data; Springer: Berlin/Heidelberg, Germany, 1997; Volume 1. [Google Scholar]
- Ciupe, S.M.; Tuncer, N. Identifiability of Parameters in Mathematical Models of SARS-CoV-2 Infections in Humans. Sci. Rep. 2022, 12, 14637. [Google Scholar] [CrossRef] [PubMed]
- Raue, A.; Kreutz, C.; Maiwald, T.; Bachmann, J.; Schilling, M.; Klingmüller, U.; Timmer, J. Structural and Practical Identifiability Analysis of Partially Observed Dynamical Models by Exploiting the Profile Likelihood. Bioinformatics 2009, 25, 1923–1929. [Google Scholar] [CrossRef] [PubMed]
- Banks, H.; Hu, S.; Thompson, W. Modeling and Inverse Problems in the Presence of Uncertainty; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Liyanage, Y.R.; Heitzman-Breen, N.; Tuncer, N.; Ciupe, S.M. Identifiability Investigation of Within-Host Models of Acute Virus Infection. bioRxiv 2024. [Google Scholar] [CrossRef]
- Wu, H.; Zhu, H.; Miao, H.; Perelson, A.S. Parameter Identifiability and Estimation of HIV/AIDS Dynamic Models. Bull. Math. Biol. 2008, 70, 785–799. [Google Scholar] [CrossRef]
- Raue, A.; Karlsson, J.; Saccomani, M.P.; Jirstrand, M.; Timmer, J. Comparison of Approaches for Parameter Identifiability Analysis of Biological Systems. Bioinformatics 2014, 30, 1440–1448. [Google Scholar] [CrossRef]
- Timsina, A.N.; Liyanage, Y.R.; Martcheva, M.; Tuncer, N. A Novel Within-Host Model of HIV and Nutrition. Math. Biosci. Eng. 2024, 21, 5577–5603. [Google Scholar] [CrossRef]
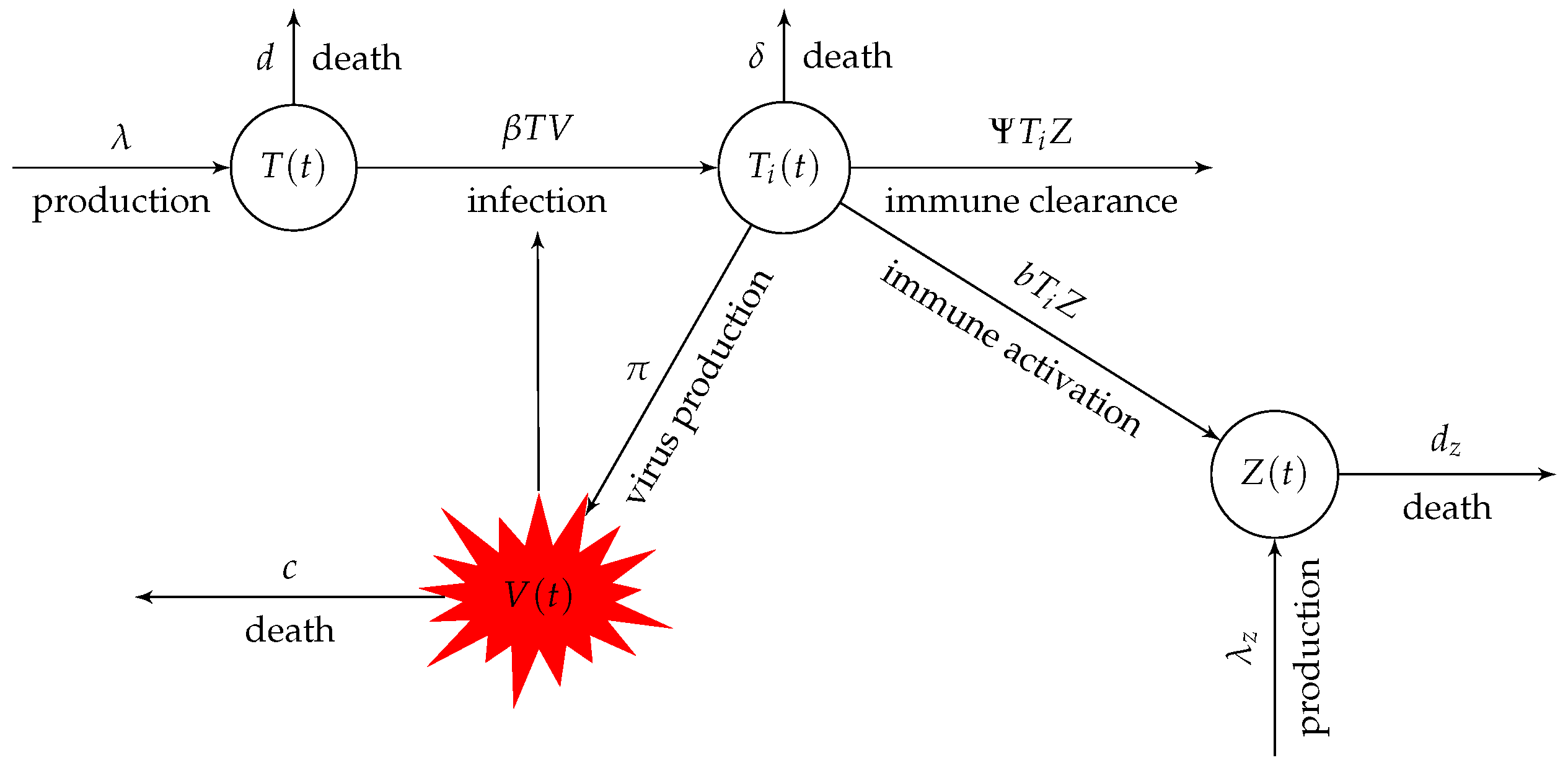

| Variables | Definition | Units |
|---|---|---|
| Number of target cells (CD4) at time t | ||
| Number of infected cells (CD8) at time t | ||
| Number of immune cells at time t | ||
| Number of viral particles at time t |
| Parameters | Definition | Unit |
|---|---|---|
| Production rate of target cells | ||
| Rate of infection of target cells by virus | ||
| d | Death rate of target cells | |
| Death rate of infected cells | ||
| Death rate of infected cells by immune cells | ||
| Production rate of immune cells | ||
| b | Antigen-driven proliferation rate of immune cell | |
| Death rate of immune cells | ||
| Virus production rate by infected cells | ||
| c | Death rate of the virus |
| Day 2 | Day 6 | Day 10 | Day 14 | Day 18 | Day 21 | Day 25 | Day 28 | |
| Viral load (RNA copies/mL) | ||||||||
| Day 32 | Day 41 | Day 49 | Day 67 | Day 96 | Day 179 | Day 259 | ||
| Viral load (RNA copies/mL) | ||||||||
| Day 2 | Day 18 | Day 32 | Day 49 | Day 90 | Day 257 | |||
| CD4 count (cells/) | ||||||||
| Day 2 | Day 18 | Day 32 | Day 49 | Day 90 | Day 255 | |||
| CD8 count (cells/) |
| Parameters | Parameter Space | Value |
|---|---|---|
| 96.7 | ||
| 2.3 × 10−7 | ||
| d | 0.13 | |
| 0.31 | ||
| 0.002 | ||
| 4.23 | ||
| b | 0.006 | |
| 0.027 | ||
| (0, 5 × 105) | 12,725.36 | |
| c | 0.68 |
| Parameter | d | b | c | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| ARE | ||||||||||
| ARE | ||||||||||
| ARE | 27 | 2 | 12 | |||||||
| ARE | 1618 | 1957 | 199 | 54 |
| Parameter | d | b | c | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| ARE | ||||||||||
| ARE | ||||||||||
| ARE | ||||||||||
| ARE |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liyanage, Y.R.; Mirsaleh Kohan, L.; Martcheva, M.; Tuncer, N. Identifiability and Parameter Estimation of Within-Host Model of HIV with Immune Response. Mathematics 2024, 12, 2837. https://doi.org/10.3390/math12182837
Liyanage YR, Mirsaleh Kohan L, Martcheva M, Tuncer N. Identifiability and Parameter Estimation of Within-Host Model of HIV with Immune Response. Mathematics. 2024; 12(18):2837. https://doi.org/10.3390/math12182837
Chicago/Turabian StyleLiyanage, Yuganthi R., Leila Mirsaleh Kohan, Maia Martcheva, and Necibe Tuncer. 2024. "Identifiability and Parameter Estimation of Within-Host Model of HIV with Immune Response" Mathematics 12, no. 18: 2837. https://doi.org/10.3390/math12182837
APA StyleLiyanage, Y. R., Mirsaleh Kohan, L., Martcheva, M., & Tuncer, N. (2024). Identifiability and Parameter Estimation of Within-Host Model of HIV with Immune Response. Mathematics, 12(18), 2837. https://doi.org/10.3390/math12182837






