On General Alternating Tornheim-Type Double Series

Abstract

In this paper, we express ${\sum }_{n,m\ge 1}{\displaystyle \frac{{\mathit{\epsilon }}_1^n{\mathit{\epsilon }}_2^m{M}_n^{\left(u\right)}{M}_m^{\left(v\right)}}{n^r{m}^s{(n+m)}^t}}$ as a linear combination of alternating multiple zeta values, where ${\mathit{\epsilon }}_i\in \{1,-1\}$ and $M_k^{\left(u\right)}\in \{H_k^{\left(u\right)},{\overline{H}}_k^{\left(u\right)}\}$, with $H_k^{\left(u\right)}$ and ${\overline{H}}_k^{\left(u\right)}$ being harmonic and alternating harmonic numbers, respectively. These sums include Subbarao and Sitaramachandrarao’s alternating analogues of Tornheim’s double series as a special case. Our method is based on employing two different techniques to evaluate the specific integral associated with a 3-poset Hasse diagram.

1. Introduction

Given $\mathit{\alpha }=({\mathit{\alpha }}_1,{\mathit{\alpha }}_2,\dots ,{\mathit{\alpha }}_r)$ as a sequence of positive integers and $\mathit{\epsilon }=({\mathit{\epsilon }}_1,{\mathit{\epsilon }}_2,\dots ,{\mathit{\epsilon }}_r)$ with ${\mathit{\epsilon }}_i\in \{1,-1\}$, and $({\mathit{\alpha }}_r,{\mathit{\epsilon }}_r)\ne (1,1)$, an alternating multiple zeta value (AMZV) $\zeta \left(\mathit{\alpha }\right)$ is defined as [1,2,3]

$$\zeta (\mathit{\alpha };\mathit{\epsilon })=\sum _{1\le k_1<k_2<\cdots <k_r}{\displaystyle \frac{{\mathit{\epsilon }}_1^{k_1}{\mathit{\epsilon }}_2^{k_2}\cdots {\mathit{\epsilon }}_r^{k_r}}{k_1^{{\mathit{\alpha }}_1}{k}_2^{{\mathit{\alpha }}_2}\cdots k_r^{{\mathit{\alpha }}_r}}}.$$

We usually put a bar on top of $k_j$ if ${\mathit{\epsilon }}_j=-1$. For example, $\zeta (\overline{2},3,\overline{4})=\zeta (2,3,4;-1,1,-1)$. The numbers ${\left|\mathit{\alpha }\right|}_r={\mathit{\alpha }}_1+{\mathit{\alpha }}_2+\cdots +{\mathit{\alpha }}_r$ and r are called the weight and depth of $\zeta (\mathit{\alpha };\mathit{\epsilon })$, respectively. In particular, if ${\mathit{\epsilon }}_i=1$ for all $1\le i\le r$, then

$$\zeta (\mathit{\alpha },\mathit{\epsilon })=\zeta \left(\mathit{\alpha }\right)=\sum _{1\le k_1<k_2<\cdots <k_r}{\displaystyle \frac{1}{k_1^{{\mathit{\alpha }}_1}{k}_2^{{\mathit{\alpha }}_2}\cdots k_r^{{\mathit{\alpha }}_r}}}$$

is the multiple zeta values [4,5]. We let ${\left\{a\right\}}^k$ denote k repetitions of a. For example, $\zeta ({\left\{2\right\}}^3,5)=\zeta (2,2,2,5)$ and $\zeta (2,{\left\{\overline{3}\right\}}^2)=\zeta (2,\overline{3},\overline{3})$.

The generalized harmonic numbers and the generalized alternating harmonic numbers are defined as

$$H_0^{\left(s\right)}=0={\overline{H}}_0^{\left(s\right)},H_n^{\left(s\right)}=\sum _{j=1}^n{\displaystyle \frac{1}{j^s}},\mathrm{and}{\overline{H}}_n^{\left(s\right)}=\sum _{j=1}^n{\displaystyle \frac{{(-1)}^{j-1}}{j^s}},$$

where s and n are positive integers. In particular, $H_n^{\left(1\right)}=H_n$ is the classical harmonic number, and ${\overline{H}}_n^{\left(1\right)}={\overline{H}}_n$ is the alternating harmonic number. The well-known formula for integers $q\ge 2$,

$$\sum _{n=1}^{\infty }{\displaystyle \frac{H_n}{n^q}}=\left(1+{\displaystyle \frac{q}{2}}\right)\zeta (q+1)-{\displaystyle \frac{1}{2}}\sum _{k=1}^{q-2}\zeta (k+1)\zeta (q-k)$$

was systematically developed by Nielsen [6]. This formula was originally discovered by Euler and later rediscovered by Ramanujan. Another famous formula

$$\sum _{n=1}^{\infty }{\displaystyle \frac{{\overline{H}}_n}{n^q}}=\zeta \left(q\right)log\left(2\right)-{\displaystyle \frac{q}{2}}\zeta (q+1)-\zeta \left(\overline{q+1}\right)+{\displaystyle \frac{1}{2}}\sum _{k=1}^q\zeta \left(\overline{k}\right)\zeta \left(\overline{q-k+1}\right).$$

was proved by Sitaramachandrarao [6,7]. Harmonic numbers are encountered in Feynman diagram calculations [8], appear in equilibrium analyses [9], and are also applied to the quicksort algorithm [10], among others. Moreover, series involving harmonic numbers have applications across various mathematical disciplines and related fields (see [11,12,13,14,15,16]).

For an r-tuple $\mathit{\alpha }=({\mathit{\alpha }}_1,{\mathit{\alpha }}_2,\dots ,{\mathit{\alpha }}_r)$ of positive integers, the Mordell–Tornheim multiple zeta values are defined as

$${\zeta }_{MT}({\mathit{\alpha }}_1,\dots ,{\mathit{\alpha }}_{r-1};{\mathit{\alpha }}_r)=\sum _{m_1,\dots ,m_r\ge 1}{\displaystyle \frac{1}{m_1^{{\mathit{\alpha }}_1}\cdots m_{r-1}^{{\mathit{\alpha }}_{r-1}}{(m_1+\cdots +m_{r-1})}^{{\mathit{\alpha }}_r}}}.$$

The examination of the special values of this function, specifically for $r=3$ at positive integer points, was first undertaken by Tornheim [17] and independently by Mordell [18], particularly in the case where ${\mathit{\alpha }}_1={\mathit{\alpha }}_2={\mathit{\alpha }}_3$. These values were later rediscovered by Witten [19] in his work on the volume formula for certain moduli spaces pertinent to theoretical physics. Therefore, we usually refer to this double series as a Tornheim–Witten double series, a Mordell–Tornheim double series, or simply a Tornheim double series.

Bradley and Zhou [20] demonstrated that this value can be expressed as a linear combination of multiple zeta values. Recently, the author of [21] gave an explicit formula for the Mordell–Tornheim multiple zeta values:

$$\begin{array}{c}{\displaystyle {\zeta }_{MT}(a_1+1,\dots ,a_n+1;s+1)}\hfill \\ =\sum _{{\displaystyle \genfrac{}{}{0pt}{}{d_1+\cdots +d_n=w}{d_i\ge 0}}}\zeta (a_1+1,\dots ,d_n+s+2)\sum _{\sigma \in S_n}{\sigma }_a\left\{\prod _{j=2}^n\left({\displaystyle \genfrac{}{}{0pt}{}{{\sum }_{k=j}^n{d}_k-{\sum }_{k=j+1}^n{a}_k}{a_j}}\right)\right\},\hfill \end{array}$$

(1)

where $w={\sum }_{j=1}^n{a}_j$, $S_n$ is the symmetric group of n objects, and ${\sigma }_a$ represents the permutations induced by $\sigma \in S_n$ on the nonnegative integer set $a_1,a_2,\dots ,a_n$.

Subbarao and Sitaramachandrarao [22] introduced the alternating analogues of Mordell–Tornheim series, which were defined as

$$R(p,q,r)=\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^n}{n^p{m}^q{(n+m)}^r}},\mathrm{and}T(p,q,r)=\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{n+m}}{n^p{m}^q{(n+m)}^r}}.$$

They posed the problem to evaluate $T(r,r,r)$ and $R(r,r,r)$ for any positive integer r. Tsumura [23,24] provided evaluation formulas for $T(r,r,r)$ and $R(r,r,r)$ for any positive odd integer r. He [25,26] also provided evaluation formulas for $T(r,s,t)$ and $R(r,s,t)$ for positive integers $r,s,t$ when $r+s+t$ is odd. Zhao [27] expressed them as a linear combination of alternating double zeta values.

Kuba [28] studied two general Tornheim series:

$$\sum _{n,m\ge 1}{\displaystyle \frac{H_n^{\left(a\right)}{H}_m^{\left(c\right)}}{n^b{m}^d{(n+m)}^s}}\mathrm{and}\sum _{n,m\ge 1}{\displaystyle \frac{H_{n+m}^{\left(a\right)}}{n^b{m}^d{(n+m)}^s}},$$

which are generalizations of Tornheim’s double series. Inspired by these insights, in this paper, we aim to study the following generalized form and express it as a linear combination of alternating multiple zeta values:

$$A:=\sum _{n,m\ge 1}{\displaystyle \frac{{\mathit{\epsilon }}_1^n{\mathit{\epsilon }}_2^m{M}_n^{\left(u\right)}{M}_m^{\left(v\right)}}{n^r{m}^s{(n+m)}^t}},$$

where ${\mathit{\epsilon }}_i\in \{1,-1\}$ and $M_k^{\left(u\right)}\in \{H_k^{\left(u\right)},{\overline{H}}_k^{\left(u\right)}\}$. This general form includes both R-series and T-series as concrete examples. For example, we obtain the formula

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{\overline{H}}_n{\overline{H}}_m}{nm(n+m)}}\hfill \\ =2\zeta (\overline{1},\overline{1},\overline{1},\overline{2})+4\zeta (1,\overline{1},1,\overline{2})+2\zeta (\overline{1},\overline{1},3)+4\zeta (1,\overline{1},\overline{3})+4\zeta (1,\overline{2},\overline{2})+2\zeta (2,\overline{1},\overline{2})\hfill \\ +4\zeta (1,4)+2\zeta (2,3),\hfill \end{array}$$

This paper is organized as follows. In Section 2, we introduce the algebraic structure for alternating multiple zeta values [29,30,31] and present a combinatorial generalization of the iterated integral associated with a 3-poset, represented by a Hasse diagram.

In Section 3, we use the 3-poset integrals to express these two alternating Mordell–Tornheim series as a linear combination of alternating double zeta values, which are the same as the expressions given by Zhao [27].

In Section 4, we decompose A-series as a linear combination of S-series (see Equation (15) for the exact definition), which are power-series expansions of some integrals associated with a 3-poset Hasse diagram. This leads to the calculation of 21 different types of S-series. We provide details of the evaluations of these S-series in Section 5 and Section 6.

In Section 7, we integrate the S-series expressions from the previous two sections with the results from Section 3, compiling all the A-series we plan to evaluate.

In the final section, we showcase the practical implications of our findings through examples like

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{H}_n^{\left(2\right)}{H}_m}{nm(n+m)}}\hfill \\ =3\zeta (1,1,2,\overline{2})+4\zeta (1,2,1,\overline{2})+3\zeta (2,1,1,\overline{2})+3\zeta (1,1,\overline{4})+6\zeta (1,3,\overline{2})+\zeta (3,1,\overline{2})\hfill \\ +4\zeta (1,2,\overline{3})+3\zeta (2,1,\overline{3})+3\zeta (2,2,\overline{2})+6\zeta (1,\overline{5})+3\zeta (2,\overline{4})+\zeta (3,\overline{3}).\hfill \end{array}$$

This paper introduces a method for evaluating a specific 3-poset integral in two different ways: one approach uses the shuffle relations and their associated Lyndon words, and the other uses the corresponding infinite-series expansions. Indeed, this method can be applied not only to double series but also extended to triple series or any finite number of infinite-series sums. An explanation is provided in the concluding remarks section.

2. Algebraic Settings and Integrals Associated with 3-Posets

Let $\mathbb{Q}⟨x,y,z⟩$ be the $\mathbb{Q}$-algebra of polynomials in three non-commutative variables, graded by degree, where x, y, and z are each assigned a degree of 1. The algebra $\mathbb{Q}⟨x,y,z⟩$ is identified with the graded $\mathbb{Q}$-vector space $\mathfrak{H}$, which is spanned by the monomials in the variables x, y, and z (see [29,30,31,32]).

Let ${\mathfrak{H}}^0$ be the subalgebra of $\mathfrak{H}$ generated by words not beginning with y and not ending with x. The words in ${\mathfrak{H}}^0$ are called “admissible words”.

In other words, the subalgebra ${\mathfrak{H}}^0$ is generated by admissible words. Let $Z:{\mathfrak{H}}^0\to \mathbb{R}$ be the $\mathbb{Q}$-linear map that assigns to each word $u_1{u}_2\cdots u_k$ in ${\mathfrak{H}}^0$, where $u_i\in \{x,y,z\}$, the multiple integral

$${\int }_{0<t_1<\cdots <t_k<1}{w}_{u_1}\left(t_1\right)w_{u_2}\left(t_2\right)\cdots w_{u_k}\left(t_k\right).$$

(2)

Here, $w_x\left(t\right)=dt/(1-t)$, $w_y\left(t\right)=dt/t$, and $w_z\left(t\right)=-dt/(1+t)$. As the word $u_1{u}_2\cdots u_k$ is in ${\mathfrak{H}}^0$, we always have $w_{u_1}\left(t\right)\ne dt/t$ and $w_{u_k}\left(t\right)\ne dt/(1-t)$, so the integral converges.

Let us define the bilinear product ⧢ (the shuffle product) on $\mathfrak{H}$ by the rules

$$1⧢w=w⧢1=w,$$

(3)

for any word w, and

$$w_1{x}_1⧢w_2{x}_2=(w_1⧢w_2{x}_2)x_1+(w_1{x}_1⧢w_2)x_2,$$

(4)

for any words $w_1$, $w_2$, any letters $x_i=x$, y, or z ($i=1,2$), and then extend the above rules to the whole algebra $\mathfrak{H}$ by linearity. It is known that each of the above products is commutative and associative [33,34]. We denote the algebras $({\mathfrak{H}}^0,+,⧢)$ by ${\mathfrak{H}}_{⧢}^0$. By the standard shuffle product identity of iterated integrals, the evaluation map Z is again an algebra homomorphism for the multiplication ⧢ (see [34]):

$$Z(w_1⧢w_2)=Z\left(w_1\right)Z\left(w_2\right).$$

(5)

We introduce a combinatorial generalization of the iterated integral, the integral associated with a 3-poset. We review the definitions and basic properties of 3-labeled posets (we call them 3-posets for short in this paper) and the associated integrals (see [35]).

Definition 1. 

A 3-poset is a pair  $(X,{\mu }_X)$, where  $X=(X,\le )$  is a finite partially ordered set (poset for short) and  ${\mu }_X$  is a map from X to  $\{0,1,-1\}$. We often omit  ${\mu }_X$  and simply say “a 3-poset X”. The  ${\mu }_X$  is called the label map of X.

A 3-poset $(X,{\mu }_X)$ is called admissible if ${\mu }_X\left(x\right)\ne 1$ for all maximal elements $x\in X$ and ${\mu }_X\left(x\right)\ne 0$ for all minimal elements $x\in X$.

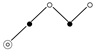A 3-poset X is depicted as a Hasse diagram in which an element x with ${\mu }_X\left(x\right)=0$, ${\mu }_X\left(x\right)=1$, ${\mu }_X\left(x\right)=-1$ is represented by ∘, •, ⊚, respectively. For example, the diagram represents the 3-poset $X=\{x_1,x_2,x_3,x_4,x_5\}$ with order $x_1<x_2<x_3>x_4<x_5$ and label $({\mu }_X\left(x_1\right),\dots ,{\mu }_X\left(x_5\right))=(-1,1,0,1,0)$. For convenience, we use 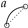to represent a circles arranged in a chain.

Definition 2. 

For an admissible 3-poset X, we define the associated integral

$$I\left(X\right)={\int }_{{\Delta }_X}\prod _{x\in X}{\omega }_{{\mu }_X\left(x\right)}\left(t_x\right),$$

(6)

where

$$\begin{array}{ccc}{\Delta }_X\hfill & =& \left\{{\left(t_x\right)}_x\in {[0,1]}^X|t_x<t_y\mathrm{if}x<y\right\}and\hfill \\ {\omega }_0\left(t\right)\hfill & =& {\displaystyle \frac{dt}{t}},{\omega }_1\left(t\right)={\displaystyle \frac{dt}{1-t}},w_{-1}\left(t\right)={\displaystyle \frac{-dt}{1+t}}.\hfill \end{array}$$

Note that the admissibility of a 3-poset corresponds to the convergence of the associated integral. We also recall an algebraic setup for 3-posets. Let $\mathfrak{P}$ be the $\mathbb{Q}$-algebra generated by the isomorphism classes of 3-posets, whose multiplication is given by the disjoint union of 3-posets. Then, the integral (6) defines a $\mathbb{Q}$-algebra homomorphism $I:{\mathfrak{P}}^0\to \mathbb{R}$ from the subalgebra ${\mathfrak{P}}^0$ of $\mathfrak{P}$ generated by the classes of admissible 3-posets. We refer to this type of integral as a 3-poset integral.

There is a $\mathbb{Q}$-linear map $W:\mathfrak{P}\to \mathfrak{H}$ that transforms a 3-poset into a finite sum of words in x, y, and z. This transformation is characterized by the following two conditions: the first condition states that for a totally ordered $X=x_1<x_2<\cdots <x_k$, $W\left(X\right)=z_{\mu \left(x_1\right)}{z}_{\mu \left(x_2\right)}\cdots z_{\mu \left(x_k\right)}$, and the second condition asserts that if a and b are non-comparable in X, then $W\left(X\right)$ can be expressed as $W\left(X_a^b\right)+W\left(X_b^a\right)$, where $X_a^b$ represents the 3-poset obtained from X by adjoining a new relation $a<b$. This W sends ${\mathfrak{P}}^0$ onto ${\mathfrak{H}}^0$ and satisfies

$$I=Z\circ W:{\mathfrak{P}}^0\to \mathbb{R}.$$

(7)

It is known that 2-posets are special cases of 3-posets (see [35,36]).

Let $w_1,w_2,w_3,w_4\in \{x,z\}$. We list some useful identities for the algebra $({\mathfrak{H}}^0,+,⧢)$: For any nonnegative integers $a,b,c$, and d, we have

$$\begin{array}{c}{\displaystyle w_1{y}^a⧢w_2{y}^b}\hfill \\ =\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{a+b_2}{a}}\right)w_2{y}^{b_1}{w}_1{y}^{a+b_2}+\sum _{a_1+a_2=a}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+b}{b}}\right)w_1{y}^{a_1}{w}_2{y}^{a_2+b},\hfill \end{array}$$

(8)

$$\begin{array}{c}{\displaystyle w_1{y}^a{w}_2{y}^b⧢w_3{y}^c}\hfill \\ =\sum _{c_1+c_2+c_3=c}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+c_3}{b}}\right)w_3{y}^{c_1}{w}_1{y}^{a+c_2}{w}_2{y}^{b+c_3}\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{c_1+c_2=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+c_2}{b}}\right)w_1{y}^{a_1}{w}_3{y}^{a_2+c_1}{w}_2{y}^{b+c_2}\hfill \\ +\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)w_1{y}^a{w}_2{y}^{b_1}{w}_3{y}^{b_2+c},\hfill \end{array}$$

(9)

$$\begin{array}{c}{\displaystyle w_1{y}^a{w}_2{y}^b⧢w_3{y}^c{w}_4{y}^d}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)w_3{y}^c{w}_4{y}^{d_1}{w}_1{y}^{a+d_2}{w}_2{y}^{b+d_3}\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)w_3{y}^{c_1}{w}_1{y}^{a_1+c_2}{w}_4{y}^{a_2+d_1}{w}_2{y}^{b+d_2}\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)w_3{y}^{c_1}{w}_1{y}^{a+c_2}{w}_2{y}^{b_1+c_3}{w}_4{y}^{b_2+d}\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)w_1{y}^{a_1}{w}_3{y}^{a_2+c}{w}_4{y}^{a_3+d_1}{w}_2{y}^{b+d_2}\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)w_1{y}^{a_1}{w}_3{y}^{a_2+c_1}{w}_2{y}^{b_1+c_2}{w}_4{y}^{b_2+d}\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)w_1{y}^a{w}_2{y}^{b_1}{w}_3{y}^{b_2+c}{w}_4{y}^{b_3+d}.\hfill \end{array}$$

(10)

Note that the variables in the summand are assumed to be nonnegative integers throughout this paper. For example, we use the notation ${\sum }_{a_1+a_2=a}$ to indicate ${\sum }_{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{a_i\ge 0}}}$.

In particular, when $w_1=w_2=w_3=w_4=x$ or z, i.e., $w_i$ are the same elements, the shuffle relations are simplified as follows [21,32]:

$$\begin{array}{ccc}x{y}^p\hfill & ⧢& x{y}^q=\sum _{d_1+d_2=p+q}\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{p}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{q}}\right)\right]x{y}^{d_1}x{y}^{d_2},\hfill \end{array}$$

(11)

$$\begin{array}{ccc}x{y}^{a_1}x{y}^{a_2}\hfill & ⧢& x{y}^b=\sum _{{\displaystyle \genfrac{}{}{0pt}{}{d_1+d_2+d_3}{=a_1+a_2+b}}}\left\{\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{b}}\right){\delta }_{d_1,a_1}+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{a_2}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a_1-d_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{a_2}}\right)\right\}x{y}^{d_1}x{y}^{d_2}x{y}^{d_3},\hfill \end{array}$$

(12)

$$\begin{array}{ccc}x{y}^{a_1}x{y}^{a_2}⧢x{y}^{b_1}x{y}^{b_2}\hfill & =& \sum _{{\displaystyle \genfrac{}{}{0pt}{}{d_1+d_2+d_3+d_4}{=a_1+a_2+b_1+b_2}}}\left\{\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{a_2}}\right){\delta }_{d_1,b_1}+\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{b_2}}\right){\delta }_{d_1,a_1}\right.\hfill \\ \hfill & \hfill & +\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{a_2+b_2-d_4}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{a_2}}\right)\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{b_1}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{b_1-d_1}}\right)\right]\hfill \\ \hfill & \hfill & \left.+\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{a_2+b_2-d_4}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{b_2}}\right)\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a_1}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a_1-d_1}}\right)\right]\right\}x{y}^{d_1}x{y}^{d_2}x{y}^{d_3}x{y}^{d_4}.\hfill \end{array}$$

(13)

3. The Formulas for Alternating Mordell–Tornheim Series

In this section, we utilize the 3-poset integrals to represent two alternating Mordell–Tornheim series, the R-series and T-series, as a linear combination of alternating double zeta values, consistent with the expressions provided by Zhao [27].

Proposition 1. 

Given three nonnegative integers a, b, and s, we have

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^n}{n^{a+1}{m}^{b+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{a+b_2}{a}}\right)\zeta (\overline{b_1+1},\overline{a+b_2+s+2})+\sum _{a_1+a_2=a}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+b}{b}}\right)\zeta (\overline{a_1+1},a_2+b+s+2).\hfill \end{array}$$

Proof. 

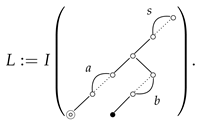Let us consider the following 3-poset integral: This integral L can be written as

$${\int }_{0<u_1<u_2<\cdots <u_{s+1}<1}F\left(u_1\right)G\left(u_1\right){\displaystyle \frac{d{u}_1}{u_1}}{\displaystyle \frac{d{u}_2}{u_2}}\cdots {\displaystyle \frac{d{u}_{s+1}}{u_{s+1}}},$$

where

$$\begin{array}{ccc}F\left(u_1\right)\hfill & =& {\int }_{0<t_1<\cdots <t_{a+1}<u_1}{\displaystyle \frac{-d{t}_1}{1+t_1}}{\displaystyle \frac{d{t}_2}{t_2}}\cdots {\displaystyle \frac{d{t}_{a+1}}{t_{a+1}}},\hfill \\ G\left(u_1\right)\hfill & =& {\int }_{0<w_1<\cdots <w_{b+1}<u_1}{\displaystyle \frac{d{w}_1}{1-w_1}}{\displaystyle \frac{d{w}_2}{w_2}}\cdots {\displaystyle \frac{d{w}_{b+1}}{w_{b+1}}}.\hfill \end{array}$$

Convert $F\left(u_1\right)$ and $G\left(u_1\right)$ into forms that represent infinite series:

$$F\left(u_1\right)=\sum _{n=1}^{\infty }{\displaystyle \frac{{(-1)}^n{u}_1^n}{n^{a+1}}},G\left(u_1\right)=\sum _{m=1}^{\infty }{\displaystyle \frac{u_1^m}{m^{b+1}}}.$$

Then, the 3-poset integral L can be rewritten as

$$\begin{array}{ccc}L\hfill & =& \sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^n}{n^{a+1}{m}^{b+1}}}{\int }_{0<u_1<\cdots <u_{s+1}<1}{u}_1^{n+m-1}d{u}_1{\displaystyle \frac{d{u}_2}{u_2}}\cdots {\displaystyle \frac{d{u}_{s+1}}{u_{s+1}}}\hfill \\ & =& \sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^n}{n^{a+1}{m}^{b+1}{(n+m)}^{s+1}}}.\hfill \end{array}$$

This is exactly the representation of $R(a+1,b+1,s+1)$. On the other hand, using Equation (7), where $I=Z\circ W:{\mathfrak{P}}^0\to \mathbb{R}$ and W sends ${\mathfrak{P}}^0$ onto ${\mathfrak{H}}^0$, we have

$$L=Z\left(\left(z{y}^{a}x{y}^b\right)y^{s+1}\right).$$

By applying Equation (8), we obtain

$$\left(z{y}^{a}x{y}^b\right)y^{s+1}=\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{a+b_2}{a}}\right)x{y}^{b_1}z{y}^{a+b_2}+\sum _{a_1+a_2=a}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+b}{b}}\right)z{y}^{a_1}x{y}^{a_2+b}.$$

By applying the Z map to the above shuffle relation, we have

$$L=\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{a+b_2}{a}}\right)\zeta (\overline{b_1+1},\overline{a+b_2+s+2})+\sum _{a_1+a_2=a}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+b}{b}}\right)\zeta (\overline{a_1+1},a_2+b+s+2).$$

Therefore, we conclude our result. □

Next, we apply similar methods to handle the T-series. Therefore, we explain only the important parts and appropriately reduce some of the detailed explanations.

Proposition 2. 

For any three nonnegative integers a, b, and s, we have

$$\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{n+m}}{n^{a+1}{m}^{b+1}{(n+m)}^{s+1}}}=\sum _{d_1+d_2=a+b}\zeta (d_1+1,\overline{d_2+s+2})\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{b}}\right)\right].$$

Proof. 

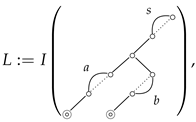Let us consider the following 3-poset integral: which has the following infinite-series expansion:

$$\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{n+m}}{n^{a+1}{m}^{b+1}{(n+m)}^{s+1}}}.$$

This is exactly the representation of $T(a+1,b+1;s+1)$. On the other hand, using the W map to transform this 3-poset diagram into ${\mathfrak{H}}^0$, we have

$$L=Z\left(\left(z{y}^{a}z{y}^b\right)y^{s+1}\right).$$

By applying Equation (11) and using the Z mapping, we obtain

$$L=\sum _{d_1+d_2=a+b}\zeta (d_1+1,\overline{d_2+s+2})\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{b}}\right)\right].$$

Combining the infinite-series expansion of L, we obtain the desired result. □

4. The Decomposition Relation of the $\mathbf{A}$-Series

Given $\mathit{\alpha }=(a_1,a_2,a_3,a_4;s)$ of positive integers and $\mathit{\epsilon }=({\mathit{\epsilon }}_1,{\mathit{\epsilon }}_2)$ with ${\mathit{\epsilon }}_i\in \{1,-1\}$, we define

$$A(\mathit{\alpha };\mathit{\epsilon })=\sum _{n,m\ge 1}{\displaystyle \frac{{\mathit{\epsilon }}_1^n{\mathit{\epsilon }}_2^m{M}_n^{\left(a_1\right)}{M}_m^{\left(a_3\right)}}{n^{a_2}{m}^{a_4}{(n+m)}^s}},$$

(14)

where $M_k^{\left(u\right)}\in \{H_k^{\left(u\right)},{\overline{H}}_k^{\left(u\right)}\}$. We simplify the notations according to the following rules: when ${\mathit{\epsilon }}_i=-1$, we place a bar on top of $a_{2i}$, denoted as ${\overline{a}}_{2i}$; if $M_n^{\left(a_i\right)}={\overline{H}}_n^{\left(a_i\right)}$, we place a bar on top of $a_i$, denoted as ${\overline{a}}_i$. For example,

$$A(\overline{a},b,c,\overline{d};s)=\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^m{\overline{H}}_n^{\left(a\right)}{H}_m^{\left(c\right)}}{n^b{m}^d{(n+m)}^s}}\mathrm{and}A(a,\overline{b},\overline{c},\overline{d};s)=\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{m+n}{H}_n^{\left(a\right)}{\overline{H}}_m^{\left(c\right)}}{n^b{m}^d{(n+m)}^s}}.$$

In order to compute the $A(\mathit{\alpha };\mathit{\epsilon })$ series, we transform these series into a linear combination of the $S(\mathit{\alpha };\mathit{\epsilon })$ series defined below.

We define

$$S(\mathit{\alpha };\mathit{\epsilon })=\sum _{n,m\ge 1}{\displaystyle \frac{{\mathit{\epsilon }}_1^n{\mathit{\epsilon }}_2^m{M}_{n-1}^{\left(a_1\right)}{M}_{m-1}^{\left(a_3\right)}}{n^{a_2}{m}^{a_4}{(n+m)}^s}},$$

(15)

where $M_k^{\left(u\right)}\in \{1,H_k^{\left(u\right)},{\overline{H}}_k^{\left(u\right)}\}$. An additional condition for simplifying the notation is that if $M_n^{\left(a_i\right)}=1$, we set $a_i$ to 0. For example,

$$S(0,\overline{b},c,\overline{d};s)=\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{m+n}{H}_{m-1}^{\left(c\right)}}{n^b{m}^d{(n+m)}^s}}.$$

It is evident that both the A-series and S-series exhibit symmetric properties:

$$A(a,b,c,d;s)=A(c,d,a,b;s),\mathrm{and}S(a,b,c,d;s)=S(c,d,a,b;s).$$

Since $H_n^{\left(a\right)}=H_{n-1}^{\left(a\right)}+{\displaystyle \frac{1}{n^a}}$ and ${\overline{H}}_n^{\left(a\right)}={\overline{H}}_{n-1}^{\left(a\right)}+{\displaystyle \frac{{(-1)}^{n-1}}{n^a}}$, we have

$$\begin{array}{c}{\displaystyle A(a+1,\overline{b+1},\overline{c+1},d+1;s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^n{H}_n^{(a+1)}{\overline{H}}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^n{H}_{n-1}^{(a+1)}{\overline{H}}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}+\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{n+m-1}{H}_{n-1}^{(a+1)}}{n^{b+1}{m}^{c+d+2}{(n+m)}^{s+1}}}\hfill \\ +\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^n{\overline{H}}_{m-1}^{(c+1)}}{n^{a+b+2}{m}^{d+1}{(n+m)}^{s+1}}}+\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{n+m-1}}{n^{a+b+2}{m}^{c+d+2}{(n+m)}^{s+1}}}\hfill \\ =S(a+1,\overline{b+1},\overline{c+1},d+1;s+1)-S(a+1,\overline{b+1},0,\overline{c+d+2};s+1)\hfill \\ +S(0,\overline{a+b+2},\overline{c+1},d+1;s+1)-S(0,\overline{a+b+2},0,\overline{c+d+2};s+1).\hfill \end{array}$$

Using the same technique, we can transform all the evaluations of $A(\mathit{\alpha };\mathit{\epsilon })$ into the evaluations of $S(\mathit{\alpha };\mathit{\epsilon })$. We list them as follows:

$$\begin{array}{c}{\displaystyle A(a+1,b+1,c+1,d+1;s+1)}\hfill \\ =S(a+1,b+1,c+1,d+1;s+1)+S(a+1,b+1,0,c+d+2;s+1)\hfill \\ +S(0,a+b+2,c+1,d+1;s+1)+S(0,a+b+2,0,c+d+2;s+1),\hfill \end{array}$$

(16)

$$\begin{array}{c}{\displaystyle A(a+1,b+1,c+1,\overline{d+1};s+1)=A(c+1,\overline{d+1},a+1,b+1;s+1)}\hfill \\ =S(a+1,b+1,c+1,\overline{d+1};s+1)+S(a+1,b+1,0,\overline{c+d+2};s+1)\hfill \\ +S(0,a+b+2,c+1,\overline{d+1};s+1)+S(0,a+b+2,0,\overline{c+d+2};s+1),\hfill \end{array}$$

(17)

$$\begin{array}{c}{\displaystyle A(a+1,b+1,\overline{c+1},d+1;s+1)=A(\overline{c+1},d+1,a+1,b+1;s+1)}\hfill \\ =S(a+1,b+1,\overline{c+1},d+1;s+1)-S(a+1,b+1,0,\overline{c+d+2};s+1)\hfill \\ +S(0,a+b+2,\overline{c+1},d+1;s+1)-S(0,a+b+2,0,\overline{c+d+2};s+1),\hfill \end{array}$$

(18)

$$\begin{array}{c}{\displaystyle A(a+1,b+1,\overline{c+1},\overline{d+1};s+1)=A(\overline{c+1},\overline{d+1},a+1,b+1;s+1)}\hfill \\ =S(a+1,b+1,\overline{c+1},\overline{d+1};s+1)+S(0,a+b+2,\overline{c+1},\overline{d+1};s+1)\hfill \\ -S(a+1,b+1,0,c+d+2;s+1)-S(0,a+b+2,0,c+d+2;s+1),\hfill \end{array}$$

(19)

$$\begin{array}{c}{\displaystyle A(a+1,\overline{b+1},c+1,\overline{d+1};s+1)}\hfill \\ =S(a+1,\overline{b+1},c+1,\overline{d+1};s+1)+S(a+1,\overline{b+1},0,\overline{c+d+2};s+1)\hfill \\ +S(0,\overline{a+b+2},c+1,\overline{d+1};s+1)+S(0,\overline{a+b+2},0,\overline{c+d+2};s+1),\hfill \end{array}$$

(20)

$$\begin{array}{c}{\displaystyle A(a+1,\overline{b+1},\overline{c+1},d+1;s+1)=A(\overline{c+1},d+1,a+1,\overline{b+1};s+1)}\hfill \\ =S(a+1,\overline{b+1},\overline{c+1},d+1;s+1)-S(a+1,\overline{b+1},0,\overline{c+d+2};s+1)\hfill \\ +S(0,\overline{a+b+2},\overline{c+1},d+1;s+1)-S(0,\overline{a+b+2},0,\overline{c+d+2};s+1),\hfill \end{array}$$

(21)

$$\begin{array}{c}{\displaystyle A(a+1,\overline{b+1},\overline{c+1},\overline{d+1};s+1)=A(\overline{c+1},\overline{d+1},a+1,\overline{b+1};s+1)}\hfill \\ =S(a+1,\overline{b+1},\overline{c+1},\overline{d+1};s+1)+S(0,\overline{a+b+2},\overline{c+1},\overline{d+1};s+1)\hfill \\ -S(a+1,\overline{b+1},0,c+d+2;s+1)-S(0,\overline{a+b+2},0,c+d+2;s+1),\hfill \end{array}$$

(22)

$$\begin{array}{c}{\displaystyle A(\overline{a+1},b+1,\overline{c+1},d+1;s+1)}\hfill \\ =S(\overline{a+1},b+1,\overline{c+1},d+1;s+1)-S(\overline{a+1},b+1,0,\overline{c+d+2};s+1)\hfill \\ -S(0,\overline{a+b+2},\overline{c+1},d+1;s+1)+S(0,\overline{a+b+2},0,\overline{c+d+2};s+1),\hfill \end{array}$$

(23)

$$\begin{array}{c}{\displaystyle A(\overline{a+1},b+1,\overline{c+1},\overline{d+1};s+1)=A(\overline{c+1},\overline{d+1},\overline{a+1},b+1;s+1)}\hfill \\ =S(\overline{a+1},b+1,\overline{c+1},\overline{d+1};s+1)-S(0,\overline{a+b+2},\overline{c+1},\overline{d+1};s+1)\hfill \\ -S(\overline{a+1},b+1,0,c+d+2;s+1)+S(0,\overline{a+b+2},0,c+d+2;s+1),\hfill \end{array}$$

(24)

$$\begin{array}{c}{\displaystyle A(\overline{a+1},\overline{b+1},\overline{c+1},\overline{d+1};s+1)}\hfill \\ =S(\overline{a+1},\overline{b+1},\overline{c+1},\overline{d+1};s+1)-S(\overline{a+1},\overline{b+1},0,c+d+2;s+1)\hfill \\ -S(0,a+b+2,\overline{c+1},\overline{d+1};s+1)+S(0,a+b+2,0,c+d+2;s+1).\hfill \end{array}$$

(25)

By organizing the above equations and considering their symmetry, we only need to explore the following 21 types of $S(\mathit{\alpha },\mathit{\epsilon })$ series to fully represent all possible $A(\mathit{\alpha },\mathit{\epsilon })$ series:

$$\begin{array}{cc}S(a+1,b+1,c+1,d+1;s+1),& S(a+1,b+1,c+1,\overline{d+1};s+1),\hfill \\ S(a+1,b+1,\overline{c+1},d+1;s+1),& S(a+1,b+1,\overline{c+1},\overline{d+1};s+1),\hfill \\ S(a+1,\overline{b+1},c+1,\overline{d+1};s+1),& S(a+1,\overline{b+1},\overline{c+1},d+1;s+1),\hfill \\ S(a+1,\overline{b+1},\overline{c+1},\overline{d+1};s+1),& S(\overline{a+1},b+1,\overline{c+1},d+1;s+1),\hfill \\ S(\overline{a+1},b+1,\overline{c+1},\overline{d+1};s+1),& S(\overline{a+1},\overline{b+1},\overline{c+1},\overline{d+1};s+1),\hfill \\ S(a+1,b+1,0,d+1;s+1),& S(a+1,b+1,0,\overline{d+1};s+1),\hfill \\ S(a+1,\overline{b+1},0,d+1;s+1),& S(a+1,\overline{b+1},0,\overline{d+1};s+1),\hfill \\ S(\overline{a+1},b+1,0,d+1;s+1),& S(\overline{a+1},b+1,0,\overline{d+1};s+1),\hfill \\ S(\overline{a+1},\overline{b+1},0,d+1;s+1),& S(\overline{a+1},\overline{b+1},0,\overline{d+1};s+1),\hfill \end{array}$$

and the following three forms:

$$S(0,b+1,0,d+1;s+1),S(0,\overline{b+1},0,d+1;s+1),S(0,\overline{b+1},0,\overline{d+1};s+1).$$

The first ten S-series are discussed in the next section, and the subsequent eight S-series are studied in Section 6. For the last three S-series, it should be noted that

$$S(0,b+1,0,d+1;s+1)=\sum _{n,m\ge 1}{\displaystyle \frac{1}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}.$$

This series is ${\zeta }_{MT}(b+1,d+1;s+1)$. We apply Equation (1) and obtain (see Equation (11) in [21])

$${\zeta }_{MT}(b+1,d+1;s+1)=\sum _{d_1+d_2=b+d}\zeta (d_1+1,d_2+s+2)\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{b}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{d}}\right)\right].$$

(26)

Also,

$$S(0,\overline{b+1},0,d+1;s+1)=\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^n}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}$$

which corresponds to $R(b+1,d+1,s+1)$. Its expression is determined by Proposition 1. Similarly, the series

$$S(0,\overline{b+1},0,\overline{d+1};s+1)=\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{n+m}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}$$

is determined by Proposition 2.

5. The First Ten $\mathbf{S}$-Series

First, we calculate the first ten types of the S-series. We demonstrate our method using $S(\overline{a+1},\overline{b+1},\overline{c+1},\overline{d+1};s+1)$ as an example. The basic calculation principles for the remaining nine types follow a similar approach to the one demonstrated.

Theorem 1. 

Given five nonnegative integers $a,b,c,d$, and s, we have

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{\overline{H}}_{n-1}^{(a+1)}{\overline{H}}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}=S(\overline{a+1},\overline{b+1},\overline{c+1},\overline{d+1};s+1)}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},\overline{d_1+1},\overline{a+d_2+1},\overline{b+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (c_1+1,\overline{a_1+c_2+1},a_2+d_1+1,\overline{b+d_2+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (c_1+1,\overline{a+c_2+1},b_1+c_3+1,\overline{b_2+d+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+c+1},a_3+d_1+1,\overline{b+d_2+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a_1+1,\overline{a_2+c_1+1},b_1+c_2+1,\overline{b_2+d+s+2})\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (\overline{a+1},\overline{b_1+1},\overline{b_2+c+1},\overline{b_3+d+s+2}).\hfill \end{array}$$

Proof. 

Let

$$\begin{array}{ccc}B\left(u_1\right)\hfill & =& {\int }_{0<s_1<s_2<\cdots <s_{b+1}<u_1}F\left(s_1\right){\displaystyle \frac{-d{s}_1}{1+s_1}}{\displaystyle \frac{d{s}_2}{s_2}}\cdots {\displaystyle \frac{d{s}_{b+1}}{s_{b+1}}},\hfill \\ D\left(u_1\right)\hfill & =& {\int }_{0<w_1<w_2<\cdots <w_{d+1}<u_1}G\left(w_1\right){\displaystyle \frac{-d{w}_1}{1+w_1}}{\displaystyle \frac{d{w}_2}{w_2}}\cdots {\displaystyle \frac{d{w}_{d+1}}{w_{d+1}}},\hfill \end{array}$$

where

$$\begin{array}{ccc}F\left(s_1\right)\hfill & =& {\int }_{0<t_1<t_2<\cdots <t_{a+1}<s_1}{\displaystyle \frac{d{t}_1}{1-t_1}}{\displaystyle \frac{d{t}_2}{t_2}}\cdots {\displaystyle \frac{d{t}_{a+1}}{t_{a+1}}},\hfill \\ G\left(w_1\right)\hfill & =& {\int }_{0<u_1<u_2<\cdots <u_{c+1}<w_1}{\displaystyle \frac{d{u}_1}{1-u_1}}{\displaystyle \frac{d{u}_2}{u_2}}\cdots {\displaystyle \frac{d{u}_{c+1}}{u_{c+1}}}.\hfill \end{array}$$

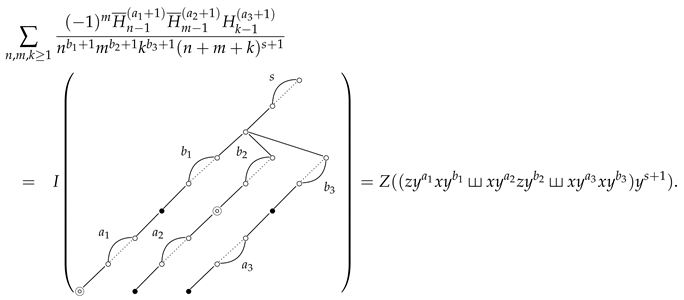
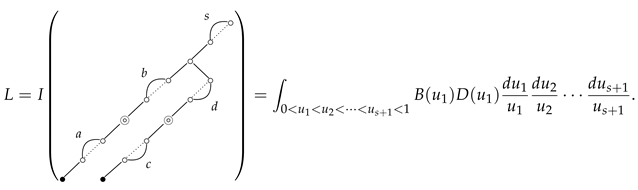Then, we can express the following 3-poset integral as an iterated integral: Transform $F\left(s_1\right)$ and $G\left(w_1\right)$ into representations as infinite series:

$$F\left(s_1\right)=\sum _{k=1}^{\infty }{\displaystyle \frac{s_1^k}{k^{a+1}}},G\left(w_1\right)=\sum _{k=1}^{\infty }{\displaystyle \frac{w_1^k}{k^{c+1}}}.$$

Substituting this form of $F\left(s_1\right)$ into $B\left(u_1\right)$, we have

$$\begin{array}{ccc}B\left(u_1\right)\hfill & =& \sum _{k=1}^{\infty }{\displaystyle \frac{(-1)}{k^{a+1}}}{\int }_{0<s_1<s_2<\cdots <s_{b+1}<u_1}{\displaystyle \frac{s_1^k}{1+s_1}}d{s}_1{\displaystyle \frac{d{s}_2}{s_2}}\cdots {\displaystyle \frac{d{s}_{b+1}}{s_{b+1}}}\hfill \\ & =& \sum _{1\le k<n}{\displaystyle \frac{{(-1)}^{n+k}}{k^{a+1}{n}^{b+1}}}{u}_1^n=\sum _{n=1}^{\infty }{\displaystyle \frac{{(-1)}^{n+1}{\overline{H}}_{n-1}^{(a+1)}}{n^{b+1}}}{u}_1^n.\hfill \end{array}$$

Similarly, we rewrite $D\left(u_1\right)$ as

$$D\left(u_1\right)=\sum _{m=1}^{\infty }{\displaystyle \frac{{(-1)}^{m+1}{\overline{H}}_{m-1}^{(c+1)}}{m^{d+1}}}{u}_1^m.$$

The 3-poset integral L becomes

$$\begin{array}{ccc}L\hfill & =& \sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{n+m}{\overline{H}}_{n-1}^{(a+1)}{\overline{H}}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}}}{\int }_{0<u_1<u_2<\cdots <u_{s+1}<1}{u}_1^{n+m-1}d{u}_1{\displaystyle \frac{d{u}_2}{u_2}}\cdots {\displaystyle \frac{d{u}_{s+1}}{u_{s+1}}}\hfill \\ & =& \sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{n+m}{\overline{H}}_{n-1}^{(a+1)}{\overline{H}}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}=S(\overline{a+1},\overline{b+1},\overline{c+1},\overline{d+1};s+1).\hfill \end{array}$$

Applying Equation (7), where $I=Z\circ W:{\mathfrak{P}}^0\to \mathbb{R}$ and W maps ${\mathfrak{P}}^0$ onto ${\mathfrak{H}}^0$, we have

$$L=Z\left(\left(x{y}^{a}z{y}^{b}x{y}^{c}z{y}^d\right)y^{s+1}\right).$$

By Equation (10), we obtain

$$\begin{array}{c}{\displaystyle (x{y}^{a}z{y}^b⧢x{y}^{c}z{y}^d)y^{s+1}}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)x{y}^{c}z{y}^{d_1}x{y}^{a+d_2}z{y}^{b+d_3+s+1}\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)x{y}^{c_1}x{y}^{a_1+c_2}z{y}^{a_2+d_1}z{y}^{b+d_2+s+1}\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)x{y}^{c_1}x{y}^{a+c_2}z{y}^{b_1+c_3}z{y}^{b_2+d+s+1}\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)x{y}^{a_1}x{y}^{a_2+c}z{y}^{a_3+d_1}z{y}^{b+d_2+s+1}\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)x{y}^{a_1}x{y}^{a_2+c_1}z{y}^{b_1+c_2}z{y}^{b_2+d+s+1}\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)x{y}^{a}z{y}^{b_1}x{y}^{b_2+c}z{y}^{b_3+d+s+1}.\hfill \end{array}$$

Using the Z map to transform the above shuffle relation, we have

$$\begin{array}{c}{\displaystyle L=Z\left((x{y}^{a}z{y}^b⧢x{y}^{c}z{y}^d)y^{s+1}\right)}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},\overline{d_1+1},\overline{a+d_2+1},\overline{b+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (c_1+1,\overline{a_1+c_2+1},a_2+d_1+1,\overline{b+d_2+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (c_1+1,\overline{a+c_2+1},b_1+c_3+1,\overline{b_2+d+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+c+1},a_3+d_1+1,\overline{b+d_2+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a_1+1,\overline{a_2+c_1+1},b_1+c_2+1,\overline{b_2+d+s+2})\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (\overline{a+1},\overline{b_1+1},\overline{b_2+c+1},\overline{b_3+d+s+2}).\hfill \end{array}$$

This is the representation of $S(\overline{a+1},\overline{b+1},\overline{c+1},\overline{d+1};s+1)$. □

For the remaining nine types, we omit the derivation process due to the similarity in methods and only provide the results. Two of these types use a 3-poset Hasse diagram that is actually a 2-poset Hasse diagram. Thus, we can use Equation (13) to simplify their expressions. We discuss these two types at the end of this section.

$$\begin{array}{c}{\displaystyle S(a+1,b+1,c+1,\overline{d+1};s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^m{H}_{n-1}^{(a+1)}{H}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (c+1,\overline{d_1+1},a+d_2+1,b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{c_1+1},\overline{a_1+c_2+1},\overline{a_2+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{c_1+1},a+c_2+1,\overline{b_1+c_3+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},a_2+c+1,\overline{a_3+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a_1+1},\overline{a_2+c_1+1},\overline{b_1+c_2+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,\overline{b_1+1},b_2+c+1,\overline{b_3+d+s+2}),\hfill \end{array}$$

(27)

$$\begin{array}{c}{\displaystyle S(a+1,b+1,\overline{c+1},d+1;s+1)=\sum _{n,m\ge 1}\frac{H_{n-1}^{(a+1)}{\overline{H}}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =-\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},d_1+1,a+d_2+1,b+d_3+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{c_1+1},a_1+c_2+1,a_2+d_1+1,b+d_2+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{c_1+1},a+c_2+1,b_1+c_3+1,b_2+d+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+c+1},a_3+d_1+1,b+d_2+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a_1+1},\overline{a_2+c_1+1},b_1+c_2+1,b_2+d+s+2)\hfill \\ -\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,\overline{b_1+1},\overline{b_2+c+1},b_3+d+s+2),\hfill \end{array}$$

(28)

$$\begin{array}{c}{\displaystyle S(a+1,b+1,\overline{c+1},\overline{d+1};s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^m{H}_{n-1}^{(a+1)}{\overline{H}}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =-\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},\overline{d_1+1},a+d_2+1,b+d_3+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (c_1+1,\overline{a_1+c_2+1},\overline{a_2+d_1+1},b+d_2+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (c_1+1,a+c_2+1,\overline{b_1+c_3+1},\overline{b_2+d+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+c+1},\overline{a_3+d_1+1},b+d_2+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a_1+1,a_2+c_1+1,\overline{b_1+c_2+1},\overline{b_2+d+s+2})\hfill \\ -\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,b_1+1,\overline{b_2+c+1},\overline{b_3+d+s+2}),\hfill \end{array}$$

(29)

$$\begin{array}{c}{\displaystyle S(a+1,\overline{b+1},\overline{c+1},d+1;s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^n{H}_{n-1}^{(a+1)}{\overline{H}}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =-\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},\overline{d_1+1},a+d_2+1,\overline{b+d_3+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (c_1+1,\overline{a_1+c_2+1},\overline{a_2+d_1+1},\overline{b+d_2+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (c_1+1,a+c_2+1,\overline{b_1+c_3+1},b_2+d+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+c+1},\overline{a_3+d_1+1},\overline{b+d_2+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a_1+1,a_2+c_1+1,\overline{b_1+c_2+1},b_2+d+s+2)\hfill \\ -\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,b_1+1,\overline{b_2+c+1},b_3+d+s+2),\hfill \end{array}$$

(30)

$$\begin{array}{c}{\displaystyle S(a+1,\overline{b+1},\overline{c+1},\overline{d+1};s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{H}_{n-1}^{(a+1)}{\overline{H}}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =-\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},d_1+1,a+d_2+1,\overline{b+d_3+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{c_1+1},a_1+c_2+1,a_2+d_1+1,\overline{b+d_2+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{c_1+1},a+c_2+1,b_1+c_3+1,\overline{b_2+d+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+c+1},a_3+d_1+1,\overline{b+d_2+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a_1+1},\overline{a_2+c_1+1},b_1+c_2+1,\overline{b_2+d+s+2})\hfill \\ -\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,\overline{b_1+1},\overline{b_2+c+1},\overline{b_3+d+s+2}),\hfill \end{array}$$

(31)

$$\begin{array}{c}{\displaystyle S(\overline{a+1},b+1,\overline{c+1},d+1;s+1)=\sum _{n,m\ge 1}\frac{{\overline{H}}_{n-1}^{(a+1)}{\overline{H}}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},\overline{d_1+1},\overline{a+d_2+1},b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (c_1+1,\overline{a_1+c_2+1},a_2+d_1+1,b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (c_1+1,\overline{a+c_2+1},b_1+c_3+1,b_2+d+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+c+1},a_3+d_1+1,b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a_1+1,\overline{a_2+c_1+1},b_1+c_2+1,b_2+d+s+2)\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (\overline{a+1},\overline{b_1+1},\overline{b_2+c+1},b_3+d+s+2),\hfill \end{array}$$

(32)

$$\begin{array}{c}{\displaystyle S(\overline{a+1},b+1,\overline{c+1},\overline{d+1};s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^m{\overline{H}}_{n-1}^{(a+1)}{\overline{H}}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},d_1+1,\overline{a+d_2+1},b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{c_1+1},a_1+c_2+1,\overline{a_2+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{c_1+1},\overline{a+c_2+1},\overline{b_1+c_3+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+c+1},\overline{a_3+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a_1+1},a_2+c_1+1,\overline{b_1+c_2+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (\overline{a+1},b_1+1,\overline{b_2+c+1},\overline{b_3+d+s+2}).\hfill \end{array}$$

(33)

The remaining two S-series are and Using Equation (13) rather than Equation (10) simplifies their expressions.

$$\begin{array}{c}{\displaystyle S(a+1,b+1,c+1,d+1;s+1)=\sum _{n,m\ge 1}\frac{H_{n-1}^{(a+1)}{H}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{{\displaystyle \genfrac{}{}{0pt}{}{d_1+d_2+d_3+d_4}{=a+b+c+d}}}\left\{\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{b}}\right){\delta }_{d_1,c}+\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{d}}\right){\delta }_{d_1,a}\right.\hfill \\ +\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{b+d-d_4}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{b}}\right)\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{c}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{c-d_1}}\right)\right]\hfill \\ \left.+\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{b+d-d_4}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{d}}\right)\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a-d_1}}\right)\right]\right\}\zeta (d_1+1,d_2+1,d_3+1,d_4+s+2).\hfill \end{array}$$

(34)

$$\begin{array}{c}{\displaystyle S(a+1,\overline{b+1},c+1,\overline{d+1};s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{H}_{n-1}^{(a+1)}{H}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{{\displaystyle \genfrac{}{}{0pt}{}{d_1+d_2+d_3+d_4}{=a+b+c+d}}}\left\{\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{b}}\right){\delta }_{d_1,c}+\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{d}}\right){\delta }_{d_1,a}\right.\hfill \\ +\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{b+d-d_4}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{b}}\right)\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{c}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{c-d_1}}\right)\right]\hfill \\ \left.+\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{b+d-d_4}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{d}}\right)\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a-d_1}}\right)\right]\right\}\zeta (d_1+1,d_2+1,d_3+1,\overline{d_4+s+2}).\hfill \end{array}$$

(35)

In fact, Equation (34) has been proved in Theorem 5 in [21].

6. The Following Eight $\mathbf{S}$-Series

To illustrate the derivation process for the last eight types of the S-series, we start with $S(a+1,\overline{b+1},0,d+1;s+1)$. The basic steps for deriving the remaining seven types are similar.

Theorem 2. 

Given four nonnegative integers $a,b,d$, and s, we have

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^n{H}_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}=\sum _{b_1+b_2=b}\left(\genfrac{}{}{0pt}{}{b_2+d}{b_2}\right)\zeta (a+1,\overline{b_1+1},b_2+d+s+2)}\hfill \\ +\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{d_1+1},a+d_2+1,\overline{b+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+d_1+1},\overline{b+d_2+s+2}).\hfill \end{array}$$

Proof. 

Let

$$F\left(s_1\right)={\int }_{0<t_1<t_2<\cdots <t_{a+1}<s_1}{\displaystyle \frac{-d{t}_1}{1+t_1}}{\displaystyle \frac{d{t}_2}{t_2}}\cdots {\displaystyle \frac{d{t}_a}{t_a}},$$

and

$$B\left(u_1\right)={\int }_{0<s_1<s_2<\cdots <s_{b+1}<u_1}F\left(s_1\right){\displaystyle \frac{-d{s}_1}{1+s_1}}{\displaystyle \frac{d{s}_2}{s_2}}\cdots {\displaystyle \frac{d{s}_{b+1}}{s_{b+1}}}.$$

We have where

$$D\left(u_1\right)={\int }_{0<w_1<w_2<\cdots <w_{d+1}<u_1}{\displaystyle \frac{d{w}_1}{1-w_1}}{\displaystyle \frac{d{w}_2}{w_2}}\cdots {\displaystyle \frac{d{w}_{d+1}}{w_{d+1}}}.$$

Transform $F\left(s_1\right)$ and $D\left(u_1\right)$ into representations as infinite series:

$$F\left(s_1\right)=\sum _{k=1}^{\infty }{\displaystyle \frac{{(-1)}^k}{k^{a+1}}}{s}_1^k,D\left(u_1\right)=\sum _{m=1}^{\infty }{\displaystyle \frac{u_1^m}{m^{d+1}}}.$$

Substituting this form of $F\left(s_1\right)$ into $B\left(u_1\right)$, we have

$$\begin{array}{ccc}B\left(u_1\right)\hfill & =& \sum _{k=1}^{\infty }{\displaystyle \frac{{(-1)}^{k+1}}{k^{a+1}}}{\int }_{0<s_1<s_2<\cdots <s_{b+1}<u_1}{\displaystyle \frac{s_1^k}{1+s_1}}d{s}_1{\displaystyle \frac{d{s}_2}{s_2}}\cdots {\displaystyle \frac{d{s}_{b+1}}{s_{b+1}}}\hfill \\ & =\hfill & \sum _{1\le k<n}{\displaystyle \frac{{(-1)}^n}{k^{a+1}{n}^{b+1}}}{u}_1^n=\sum _{n=1}^{\infty }{\displaystyle \frac{{(-1)}^n{H}_{n-1}^{(a+1)}}{n^{b+1}}}{u}_1^n.\hfill \end{array}$$

The 3-poset integral L becomes

$$\begin{array}{ccc}L\hfill & =& \sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^n{H}_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}}}{\int }_{0<u_1<u_2<\cdots <u_{s+1}<1}{u}_1^{n+m-1}d{u}_1{\displaystyle \frac{d{u}_2}{u_2}}\cdots {\displaystyle \frac{d{u}_{s+1}}{u_{s+1}}}\hfill \\ & =\hfill & \sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^n{H}_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}=S(a+1,\overline{b+1},0,d+1;s+1).\hfill \end{array}$$

Applying Equation (7), where $I=Z\circ W:{\mathfrak{P}}^0\to \mathbb{R}$ and W maps ${\mathfrak{P}}^0$ onto ${\mathfrak{H}}^0$, we have

$$L=Z\left((z{y}^{a}z{y}^b⧢x{y}^d)y^{s+1}\right).$$

By Equation (9), we obtain

$$\begin{array}{ccc}(z{y}^{a}z{y}^b⧢x{y}^d)y^{s+1}\hfill & =& \sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)x{y}^{d_1}z{y}^{a+d_2}z{y}^{b+d_3+s+1}\hfill \\ \hfill & \hfill & +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)z{y}^{a_1}x{y}^{a_2+d_1}z{y}^{b+d_2+s+1}\hfill \\ \hfill & \hfill & +\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)z{y}^{a}z{y}^{b_1}x{y}^{b_2+d+s+1}.\hfill \end{array}$$

Using the Z map to transform the above shuffle relation, we have

$$\begin{array}{c}{\displaystyle L=Z\left((z{y}^{a}z{y}^b⧢x{y}^d)y^{s+1}\right)=\sum _{n,m\ge 1}\frac{{(-1)}^n{H}_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{d_1+1},a+d_2+1,\overline{b+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+d_1+1},\overline{b+d_2+s+2})\hfill \\ +\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a+1,\overline{b_1+1},b_2+d+s+2).\hfill \end{array}$$

This is the representation of $S(a+1,\overline{b+1},0,d+1;s+1)$. □

We use a similar method to express the remaining seven types of $S(\mathit{\alpha },\mathit{\epsilon })$ as a linear combination of alternating multiple zeta values:

$$\begin{array}{c}{\displaystyle S(a+1,b+1,0,d+1;s+1)}\hfill \\ =\sum _{n,m\ge 1}{\displaystyle \frac{H_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}=\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a+1,b_1+1,b_2+d+s+2)\hfill \\ +\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (d_1+1,a+d_2+1,b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,a_2+d_1+1,b+d_2+s+2),\hfill \end{array}$$

(36)

$$\begin{array}{c}{\displaystyle S(a+1,b+1,0,\overline{d+1};s+1)}\hfill \\ =\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^m{H}_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}=\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a+1,\overline{b_1+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{d_1+1},a+d_2+1,b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+d_1+1},b+d_2+s+2),\hfill \end{array}$$

(37)

$$\begin{array}{c}{\displaystyle S(a+1,\overline{b+1},0,\overline{d+1};s+1)}\hfill \\ =\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{n+m}{H}_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}=\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a+1,b_1+1,\overline{b_2+d+s+2})\hfill \\ +\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (d_1+1,a+d_2+1,\overline{b+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,a_2+d_1+1,\overline{b+d_2+s+2}),\hfill \end{array}$$

(38)

$$\begin{array}{c}{\displaystyle S(\overline{a+1},b+1,0,d+1;s+1)}\hfill \\ =\sum _{n,m\ge 1}{\displaystyle \frac{{\overline{H}}_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}=-\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a+1},b_1+1,b_2+d+s+2)\hfill \\ -\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{d_1+1},\overline{a+d_2+1},b+d_3+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},a_2+d_1+1,b+d_2+s+2),\hfill \end{array}$$

(39)

$$\begin{array}{c}{\displaystyle S(\overline{a+1},b+1,0,\overline{d+1};s+1)}\hfill \\ =\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^m{\overline{H}}_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}=-\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a+1},\overline{b_1+1},\overline{b_2+d+s+2})\hfill \\ -\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (d_1+1,\overline{a+d_2+1},b+d_3+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+d_1+1},b+d_2+s+2),\hfill \end{array}$$

(40)

$$\begin{array}{c}{\displaystyle S(\overline{a+1},\overline{b+1},0,d+1;s+1)}\hfill \\ =\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^n{\overline{H}}_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}=-\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a+1},\overline{b_1+1},b_2+d+s+2)\hfill \\ -\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (d_1+1,\overline{a+d_2+1},\overline{b+d_3+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+d_1+1},\overline{b+d_2+s+2}),\hfill \end{array}$$

(41)

$$\begin{array}{c}{\displaystyle S(\overline{a+1},\overline{b+1},0,\overline{d+1};s+1)}\hfill \\ =\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^{n+m}{\overline{H}}_{n-1}^{(a+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}=-\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a+1},b_1+1,\overline{b_2+d+s+2})\hfill \\ -\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{d_1+1},\overline{a+d_2+1},\overline{b+d_3+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},a_2+d_1+1,\overline{b+d_2+s+2}).\hfill \end{array}$$

(42)

In fact, we can apply Equation (12) to Equations (36) and (38), but the resulting expressions will not be significantly simplified. Therefore, we leave this to interested readers (see Theorem 6 in [21]).

7. The Expressions of $\mathbf{A}$-Series

By combining the results from the previous two sections and using Equations (16) through (25), we can list the expressions for all the A-series. For example, consider

$$\sum _{n,m\ge 1}{\displaystyle \frac{{(-1)}^m{H}_n^{(a+1)}{H}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}=A(a+1,b+1,c+1,\overline{d+1};s+1).$$

Applying Equation (18), we find that

$$\begin{array}{c}{\displaystyle A(a+1,b+1,c+1,\overline{d+1};s+1)}\hfill \\ =S(a+1,b+1,c+1,\overline{d+1};s+1)+S(a+1,b+1,0,\overline{c+d+2};s+1)\hfill \\ +S(0,a+b+2,c+1,\overline{d+1};s+1)+S(0,a+b+2,0,\overline{c+d+2};s+1).\hfill \end{array}$$

There are four S-series in the expression. For the first S-series, $S(a+1,b+1,c+1,\overline{d+1};s+1)$, we use Equation (27) and obtain

$$\begin{array}{c}{\displaystyle S(a+1,b+1,c+1,\overline{d+1};s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^m{H}_{n-1}^{(a+1)}{H}_{m-1}^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (c+1,\overline{d_1+1},a+d_2+1,b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{c_1+1},\overline{a_1+c_2+1},\overline{a_2+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{c_1+1},a+c_2+1,\overline{b_1+c_3+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},a_2+c+1,\overline{a_3+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a_1+1},\overline{a_2+c_1+1},\overline{b_1+c_2+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,\overline{b_1+1},b_2+c+1,\overline{b_3+d+s+2}).\hfill \end{array}$$

To address the second S-series, $S(a+1,b+1,0,\overline{c+d+2};s+1)$, Equation (37) is employed. By substituting d with $c+d+1$ in Equation (37) while keeping the other parameters unchanged, the expression for the second S-series can be derived as follows:

$$\begin{array}{c}{\displaystyle S(a+1,b+1,0,\overline{c+d+2};s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^m{H}_{n-1}^{(a+1)}}{n^{b+1}{m}^{c+d+2}{(n+m)}^{s+1}}}\hfill \\ =\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c+d+1}{b_2}}\right)\zeta (a+1,\overline{b_1+1},\overline{b_2+c+d+s+3})\hfill \\ +\sum _{d_1+d_2+d_3=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{d_1+1},a+d_2+1,b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=c+d+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+d_1+1},b+d_2+s+2).\hfill \end{array}$$

We apply Theorem 2 to the third S-series, $S(0,a+b+2,c+1,\overline{d+1};s+1)$. The S-series mentioned in Theorem 2 is written as $S(a+1,\overline{b+1},0,d+1;s+1)$. Due to symmetry, this S-series can also be written as $S(0,d+1,a+1,\overline{b+1};s+1)$. To find the expression for the S-series we need, we can replace the parameters in Theorem 2: change a to c, b to d, and d to $a+b+1$. This results in the required S-series $S(0,a+b+2,c+1,\overline{d+1};s+1)$ being expressed as follows:

$$\begin{array}{c}{\displaystyle S(0,a+b+2,c+1,\overline{d+1};s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^m{H}_m^{(c+1)}}{n^{a+b+2}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{b_1+b_2=d}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+a+b+1}{b_2}}\right)\zeta (c+1,\overline{b_1+1},b_2+a+b+s+3)\hfill \\ +\sum _{d_1+d_2+d_3=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d_2}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_3}{d}}\right)\zeta (\overline{d_1+1},c+d_2+1,\overline{d+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=c}{d_1+d_2=a+b+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_2}{d}}\right)\zeta (\overline{a_1+1},\overline{a_2+d_1+1},\overline{d+d_2+s+2}).\hfill \end{array}$$

Now, w apply the results from Proposition 1 to the fourth S-series. The S-series in Proposition 1 is $S(0,\overline{a+1},0,b+1;s+1)$. Utilizing symmetry, this S-series can be represented as $S(0,b+1,0,\overline{a+1};s+1)$. Therefore, by replacing parameter a with $c+d+1$ and parameter b with $a+b+1$ in Proposition 1, we obtain the desired $S(0,a+b+2,0,\overline{c+d+2};s+1)$, which is expressed as follows:

$$\begin{array}{c}{\displaystyle S(0,a+b+2,p,\overline{c+d+2};s+1)=\sum _{n,m\ge 1}\frac{{(-1)}^m}{n^{a+b+2}{m}^{c+d+2}{(n+m)}^{s+1}}}\hfill \\ =\sum _{b_1+b_2=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d+b_2+1}{b_2}}\right)\zeta (\overline{b_1+1},\overline{c+d+b_2+s+3})\hfill \\ +\sum _{a_1+a_2=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+a+b+1}{a_2}}\right)\zeta (\overline{a_1+1},a_2+a+b+s+3).\hfill \end{array}$$

By combining the expressions of the four S-series mentioned above, we arrive at the final representation of $A(a+1,b+1,c+1,\overline{d+1};s+1)$.

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^m{H}_n^{(a+1)}{H}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (c+1,\overline{d_1+1},a+d_2+1,b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{c_1+1},\overline{a_1+c_2+1},\overline{a_2+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{c_1+1},a+c_2+1,\overline{b_1+c_3+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},a_2+c+1,\overline{a_3+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a_1+1},\overline{a_2+c_1+1},\overline{b_1+c_2+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,\overline{b_1+1},b_2+c+1,\overline{b_3+d+s+2})\hfill \\ +\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c+d+1}{b_2}}\right)\zeta (a+1,\overline{b_1+1},\overline{b_2+c+d+s+3})\hfill \\ +\sum _{d_1+d_2+d_3=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{d_1+1},a+d_2+1,b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=c+d+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{b_1+b_2=d}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+a+b+1}{b_2}}\right)\zeta (c+1,\overline{b_1+1},b_2+a+b+s+3)\hfill \\ +\sum _{d_1+d_2+d_3=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d_2}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_3}{d}}\right)\zeta (\overline{d_1+1},c+d_2+1,\overline{d+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=c}{d_1+d_2=a+b+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_2}{d}}\right)\zeta (\overline{a_1+1},\overline{a_2+d_1+1},\overline{d+d_2+s+2})\hfill \\ +\sum _{b_1+b_2=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d+b_2+1}{b_2}}\right)\zeta (\overline{b_1+1},\overline{c+d+b_2+s+3})\hfill \\ +\sum _{a_1+a_2=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+a+b+1}{a_2}}\right)\zeta (\overline{a_1+1},a_2+a+b+s+3),\hfill \end{array}$$

(43)

Building upon the demonstrated example, we proceed to transform the required A-series into four S-series using Equations (16)–(25). Leveraging the representation outcomes from the preceding two sections and Propositions 1 and 2, with suitable substitutions for the respective parameters, we derive a representation that converts the four desired S-series into a linear combination of alternating multiple zeta values. Since the methods are similar, we no longer demonstrate each step individually. Below, we list the expressions for all A-series.

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{H_n^{(a+1)}{\overline{H}}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =-\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},d_1+1,a+d_2+1,b+d_3+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{c_1+1},a_1+c_2+1,a_2+d_1+1,b+d_2+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{c_1+1},a+c_2+1,b_1+c_3+1,b_2+d+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+c+1},a_3+d_1+1,b+d_2+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a_1+1},\overline{a_2+c_1+1},b_1+c_2+1,b_2+d+s+2)\hfill \\ -\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,\overline{b_1+1},\overline{b_2+c+1},b_3+d+s+2)\hfill \\ -\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c+d+1}{b_2}}\right)\zeta (a+1,\overline{b_1+1},\overline{b_2+c+d+s+3})\hfill \\ -\sum _{d_1+d_2+d_3=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{d_1+1},a+d_2+1,b+d_3+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=c+d+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+d_1+1},b+d_2+s+2)\hfill \\ -\sum _{b_1+b_2=d}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+a+b+1}{b_2}}\right)\zeta (\overline{c+1},b_1+1,b_2+a+b+s+3)\hfill \\ -\sum _{d_1+d_2+d_3=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d_2}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_3}{d}}\right)\zeta (\overline{d_1+1},\overline{c+d_2+1},d+d_3+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=c}{d_1+d_2=a+b+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_2}{d}}\right)\zeta (\overline{a_1+1},a_2+d_1+1,d+d_2+s+2)\hfill \\ -\sum _{b_1+b_2=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d+1+b_2}{b_2}}\right)\zeta (\overline{b_1+1},\overline{c+d+b_2+s+3})\hfill \\ -\sum _{a_1+a_2=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+a+b+1}{a_2}}\right)\zeta (\overline{a_1+1},a_2+a+b+s+3),\hfill \end{array}$$

(44)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^m{H}_n^{(a+1)}{\overline{H}}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =-\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},\overline{d_1+1},a+d_2+1,b+d_3+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (c_1+1,\overline{a_1+c_2+1},\overline{a_2+d_1+1},b+d_2+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (c_1+1,a+c_2+1,\overline{b_1+c_3+1},\overline{b_2+d+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+c+1},\overline{a_3+d_1+1},b+d_2+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a_1+1,a_2+c_1+1,\overline{b_1+c_2+1},\overline{b_2+d+s+2})\hfill \\ -\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,b_1+1,\overline{b_2+c+1},\overline{b_3+d+s+2})\hfill \\ -\sum _{b_1+b_2=d}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+a+b+1}{b_2}}\right)\zeta (\overline{c+1},\overline{b_1+1},b_2+a+b+s+3)\hfill \\ -\sum _{d_1+d_2+d_3=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d_2}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_3}{d}}\right)\zeta (d_1+1,\overline{c+d_2+1},\overline{d+d_3+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=c}{d_1+d_2=a+b+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_2}{d}}\right)\zeta (a_1+1,\overline{a_2+d_1+1},\overline{d+d_2+s+2})\hfill \\ -\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c+d+1}{b_2}}\right)\zeta (a+1,b_1+1,b_2+c+d+s+3)\hfill \\ -\sum _{d_1+d_2+d_3=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (d_1+1,a+d_2+1,b+d_3+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=c+d+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,a_2+d_1+1,b+d_2+s+2)\hfill \\ -\sum _{d_1+d_2=a+b+c+d+2}\zeta (d_1+1,d_2+s+2)\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a+b+1}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{c+d+1}}\right)\right],\hfill \end{array}$$

(45)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^n{H}_n^{(a+1)}{\overline{H}}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =-\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},\overline{d_1+1},a+d_2+1,\overline{b+d_3+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (c_1+1,\overline{a_1+c_2+1},\overline{a_2+d_1+1},\overline{b+d_2+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (c_1+1,a+c_2+1,\overline{b_1+c_3+1},b_2+d+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+c+1},\overline{a_3+d_1+1},\overline{b+d_2+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a_1+1,a_2+c_1+1,\overline{b_1+c_2+1},b_2+d+s+2)\hfill \\ -\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,b_1+1,\overline{b_2+c+1},b_3+d+s+2)\hfill \\ -\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c+d+1}{b_2}}\right)\zeta (a+1,b_1+1,\overline{b_2+c+d+s+3})\hfill \\ -\sum _{d_1+d_2+d_3=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (d_1+1,a+d_2+1,\overline{b+d_3+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=c+d+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,a_2+d_1+1,\overline{b+d_2+s+2})\hfill \\ -\sum _{b_1+b_2=d}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+a+b+1}{b_2}}\right)\zeta (\overline{c+1},\overline{b_1+1},\overline{b_2+a+b+s+3})\hfill \\ -\sum _{d_1+d_2+d_3=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d_2}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_3}{d}}\right)\zeta (d_1+1,\overline{c+d_2+1},d+d_3+s+2)\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=c}{d_1+d_2=a+b+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_2}{d}}\right)\zeta (a_1+1,\overline{a_2+d_1+1},d+d_2+s+2)\hfill \\ -\sum _{d_1+d_2=a+b+c+d+2}\zeta (d_1+1,\overline{d_2+s+2})\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a+b+1}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{c+d+1}}\right)\right],\hfill \end{array}$$

(46)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{H}_n^{(a+1)}{\overline{H}}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =-\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},d_1+1,a+d_2+1,\overline{b+d_3+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{c_1+1},a_1+c_2+1,a_2+d_1+1,\overline{b+d_2+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{c_1+1},a+c_2+1,b_1+c_3+1,\overline{b_2+d+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+c+1},a_3+d_1+1,\overline{b+d_2+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a_1+1},\overline{a_2+c_1+1},b_1+c_2+1,\overline{b_2+d+s+2})\hfill \\ -\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (a+1,\overline{b_1+1},\overline{b_2+c+1},\overline{b_3+d+s+2})\hfill \\ -\sum _{b_1+b_2=d}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+a+b+1}{b_2}}\right)\zeta (\overline{c+1},b_1+1,\overline{b_2+a+b+s+3})\hfill \\ -\sum _{d_1+d_2+d_3=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d_2}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_3}{d}}\right)\zeta (\overline{d_1+1},\overline{c+d_2+1},\overline{d+d_3+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=c}{d_1+d_2=a+b+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_2}{d}}\right)\zeta (\overline{a_1+1},a_2+d_1+1,\overline{d+d_2+s+2})\hfill \\ -\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c+d+1}{b_2}}\right)\zeta (a+1,\overline{b_1+1},b_2+c+d+s+3)\hfill \\ -\sum _{d_1+d_2+d_3=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{d_1+1},a+d_2+1,\overline{b+d_3+s+2})\hfill \\ -\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=c+d+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+d_1+1},\overline{b+d_2+s+2})\hfill \\ -\sum _{b_1+b_2=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+b+1+b_2}{b_2}}\right)\zeta (\overline{b_1+1},\overline{a+b+b_2+s+3})\hfill \\ -\sum _{a_1+a_2=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c+d+1}{a_2}}\right)\zeta (\overline{a_1+1},a_2+c+d+s+3),\hfill \end{array}$$

(47)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{\overline{H}}_n^{(a+1)}{\overline{H}}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},\overline{d_1+1},\overline{a+d_2+1},b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (c_1+1,\overline{a_1+c_2+1},a_2+d_1+1,b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (c_1+1,\overline{a+c_2+1},b_1+c_3+1,b_2+d+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+c+1},a_3+d_1+1,b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a_1+1,\overline{a_2+c_1+1},b_1+c_2+1,b_2+d+s+2)\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (\overline{a+1},\overline{b_1+1},\overline{b_2+c+1},b_3+d+s+2)\hfill \\ +\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c+d+1}{b_2}}\right)\zeta (\overline{a+1},\overline{b_1+1},\overline{b_2+c+d+s+3})\hfill \\ +\sum _{d_1+d_2+d_3=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (d_1+1,\overline{a+d_2+1},b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=c+d+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{b_1+b_2=d}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+a+b+1}{b_2}}\right)\zeta (\overline{c+1},\overline{b_1+1},\overline{b_2+a+b+s+3})\hfill \\ +\sum _{d_1+d_2+d_3=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d_2}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_3}{d}}\right)\zeta (d_1+1,\overline{c+d_2+1},d+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=c}{d_1+d_2=a+b+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_2}{d}}\right)\zeta (a_1+1,\overline{a_2+d_1+1},d+d_2+s+2)\hfill \\ +\sum _{d_1+d_2=a+b+c+d+2}\zeta (d_1+1,\overline{d_2+s+2})\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a+b+1}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{c+d+1}}\right)\right],\hfill \end{array}$$

(48)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^m{\overline{H}}_n^{(a+1)}{\overline{H}}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},d_1+1,\overline{a+d_2+1},b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{c_1+1},a_1+c_2+1,\overline{a_2+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{c_1+1},\overline{a+c_2+1},\overline{b_1+c_3+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},\overline{a_2+c+1},\overline{a_3+d_1+1},b+d_2+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (\overline{a_1+1},a_2+c_1+1,\overline{b_1+c_2+1},\overline{b_2+d+s+2})\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (\overline{a+1},b_1+1,\overline{b_2+c+1},\overline{b_3+d+s+2})\hfill \\ +\sum _{b_1+b_2=d}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+a+b+1}{b_2}}\right)\zeta (\overline{c+1},b_1+1,\overline{b_2+a+b+s+3})\hfill \\ +\sum _{d_1+d_2+d_3=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d_2}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_3}{d}}\right)\zeta (\overline{d_1+1},\overline{c+d_2+1},\overline{d+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=c}{d_1+d_2=a+b+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_2}{d}}\right)\zeta (\overline{a_1+1},a_2+d_1+1,\overline{d+d_2+s+2})\hfill \\ +\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c+d+1}{b_2}}\right)\zeta (\overline{a+1},b_1+1,b_2+c+d+s+3)\hfill \\ +\sum _{d_1+d_2+d_3=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{d_1+1},\overline{a+d_2+1},b+d_3+s+2)\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=c+d+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (\overline{a_1+1},a_2+d_1+1,b+d_2+s+2)\hfill \\ +\sum _{b_1+b_2=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+b+1+b_2}{b_2}}\right)\zeta (\overline{b_1+1},\overline{a+b+b_2+s+3})\hfill \\ +\sum _{a_1+a_2=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c+d+1}{a_2}}\right)\zeta (\overline{a_1+1},a_2+c+d+s+3),\hfill \end{array}$$

(49)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{\overline{H}}_n^{(a+1)}{\overline{H}}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ =\sum _{d_1+d_2+d_3=d}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (\overline{c+1},\overline{d_1+1},\overline{a+d_2+1},\overline{b+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{c_1+c_2=c}{d_1+d_2=d}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_1+c_2}{a_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (c_1+1,\overline{a_1+c_2+1},a_2+d_1+1,\overline{b+d_2+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2+c_3=c}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a+c_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_3}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (c_1+1,\overline{a+c_2+1},b_1+c_3+1,\overline{b_2+d+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2+a_3=a}{d_1+d_2=d}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{a_3+d_1}{a_3}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+c+1},a_3+d_1+1,\overline{b+d_2+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{\genfrac{}{}{0pt}{}{b_1+b_2=b}{c_1+c_2=c}}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+c_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_1+c_2}{b_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+d}{b_2}}\right)\zeta (a_1+1,\overline{a_2+c_1+1},b_1+c_2+1,\overline{b_2+d+s+2})\hfill \\ +\sum _{b_1+b_2+b_3=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c}{b_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b_3+d}{b_3}}\right)\zeta (\overline{a+1},\overline{b_1+1},\overline{b_2+c+1},\overline{b_3+d+s+2})\hfill \\ +\sum _{b_1+b_2=b}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+c+d+1}{b_2}}\right)\zeta (\overline{a+1},\overline{b_1+1},b_2+c+d+s+3)\hfill \\ +\sum _{d_1+d_2+d_3=c+d+1}\left({\displaystyle \genfrac{}{}{0pt}{}{a+d_2}{a}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_3}{b}}\right)\zeta (d_1+1,\overline{a+d_2+1},\overline{b+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=a}{d_1+d_2=c+d+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{b+d_2}{b}}\right)\zeta (a_1+1,\overline{a_2+d_1+1},\overline{b+d_2+s+2})\hfill \\ +\sum _{b_1+b_2=d}\left({\displaystyle \genfrac{}{}{0pt}{}{b_2+a+b+1}{b_2}}\right)\zeta (\overline{c+1},\overline{b_1+1},b_2+a+b+s+3)\hfill \\ +\sum _{d_1+d_2+d_3=a+b+1}\left({\displaystyle \genfrac{}{}{0pt}{}{c+d_2}{c}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_3}{d}}\right)\zeta (d_1+1,\overline{c+d_2+1},\overline{d+d_3+s+2})\hfill \\ +\sum _{{\displaystyle \genfrac{}{}{0pt}{}{a_1+a_2=c}{d_1+d_2=a+b+1}}}\left({\displaystyle \genfrac{}{}{0pt}{}{a_2+d_1}{a_2}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d+d_2}{d}}\right)\zeta (a_1+1,\overline{a_2+d_1+1},\overline{d+d_2+s+2})\hfill \\ +\sum _{d_1+d_2=a+b+c+d+2}\zeta (d_1+1,d_2+s+2)\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a+b+1}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{c+d+1}}\right)\right].\hfill \end{array}$$

(50)

Because $A(a+1,b+1,c+1,d+1;s+1)$ and $A(a+1,\overline{b+1},c+1,\overline{d+1};s+1)$ rely on 2-poset Hasse diagrams, their expressions are relatively simple. The expression for $A(a+1,b+1,c+1,d+1;s+1)$ is available in Equation (26) in [21]. Consequently, we only provide the expression for $A(a+1,\overline{b+1},c+1,\overline{d+1};s+1)$ here.

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{H}_n^{(a+1)}{H}_m^{(c+1)}}{n^{b+1}{m}^{d+1}{(n+m)}^{s+1}}}\hfill \\ {\displaystyle =\sum _{\genfrac{}{}{0pt}{}{d_1+d_2+d_3+d_4}{=a+b+c+d}}\left\{\left(\genfrac{}{}{0pt}{}{d_3}{a}\right)\left(\genfrac{}{}{0pt}{}{d_4}{b}\right){\delta }_{d_1,c}+\left(\genfrac{}{}{0pt}{}{d_3}{c}\right)\left(\genfrac{}{}{0pt}{}{d_4}{d}\right){\delta }_{d_1,a}+\left(\genfrac{}{}{0pt}{}{d_3}{b+d-d_4}\right)\left(\genfrac{}{}{0pt}{}{d_4}{b}\right)\left[\left(\genfrac{}{}{0pt}{}{d_2}{c}\right)+\left(\genfrac{}{}{0pt}{}{d_2}{c-d_1}\right)\right]\right.}\hfill \\ \left.+\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{b+d-d_4}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_4}{d}}\right)\left[\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a-d_1}}\right)\right]\right\}\zeta (d_1+1,d_2+1,d_3+1,\overline{d_4+s+2})\hfill \\ {\displaystyle +\sum _{\genfrac{}{}{0pt}{}{d_1+d_2+d_3}{=a+b+c+d+1}}\left\{\left(\genfrac{}{}{0pt}{}{d_3}{a+b+1}\right){\delta }_{d_1,c}+\left(\genfrac{}{}{0pt}{}{d_3}{c+d+1}\right){\delta }_{d_1,a}+\left(\genfrac{}{}{0pt}{}{d_2}{a}\right)\left(\genfrac{}{}{0pt}{}{d_3}{b}\right)+\left(\genfrac{}{}{0pt}{}{d_2}{c}\right)\left(\genfrac{}{}{0pt}{}{d_3}{d}\right)\right.}\hfill \\ \left.+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{a-d_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{b}}\right)+\left({\displaystyle \genfrac{}{}{0pt}{}{d_2}{c-d_1}}\right)\left({\displaystyle \genfrac{}{}{0pt}{}{d_3}{d}}\right)\right\}\zeta (d_1+1,d_2+1,\overline{d_3+s+2})\hfill \\ {\displaystyle +\sum _{d_1+d_2=a+b+c+d+2}\left[\left(\genfrac{}{}{0pt}{}{d_2}{a+b+1}\right)+\left(\genfrac{}{}{0pt}{}{d_2}{c+d+1}\right)\right]\zeta (d_1+1,\overline{d_2+s+2}).}\hfill \end{array}$$

(51)

8. Examples and Concluding Remarks

Here, we substitute $a=b=c=d=s=0$ into the formulas for the A-series from the previous section and list the results for reference. We have numerically verified these equations using Mathematica 13.

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^m{H}_n{H}_m}{nm(n+m)}}\hfill \\ =\zeta (1,\overline{1},1,2)+\zeta (\overline{1},\overline{1},\overline{1},2)+\zeta (\overline{1},1,\overline{1},\overline{2})+\zeta (\overline{1},1,\overline{1},2)+\zeta (\overline{1},\overline{1},\overline{1},\overline{2})+\zeta (1,\overline{1},1,\overline{2})\hfill \\ +\zeta (1,\overline{1},\overline{3})+\zeta (\overline{1},1,3)+\zeta (\overline{1},2,2)+\zeta (\overline{2},1,2)+\zeta (\overline{1},\overline{1},3)+\zeta (\overline{1},\overline{2},2)\hfill \\ +\zeta (1,\overline{1},3)+\zeta (\overline{1},1,\overline{3})+\zeta (\overline{1},2,\overline{2})+\zeta (\overline{2},1,\overline{2})+\zeta (\overline{1},\overline{1},\overline{3})+\zeta (\overline{1},\overline{2},\overline{2})\hfill \\ +2\zeta (\overline{1},\overline{4})+\zeta (\overline{2},\overline{3})+2\zeta (\overline{1},4)+\zeta (\overline{2},3),\hfill \end{array}$$

(52)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{H_n{\overline{H}}_m}{nm(n+m)}}\hfill \\ =-\left(3\zeta (\overline{1},1,1,2)+2\zeta (\overline{1},\overline{1},1,2)+\zeta (1,\overline{1},\overline{1},2)+\zeta (\overline{2},1,2)+\zeta (\overline{2},\overline{1},2)\right.\hfill \\ +\zeta (1,\overline{1},\overline{3})+3\zeta (\overline{1},1,3)+2\zeta (\overline{1},\overline{1},3)+2\zeta (\overline{1},2,2)+2\zeta (\overline{1},\overline{2},2)\hfill \\ \left.+2\zeta (\overline{1},\overline{4})+\zeta (\overline{2},\overline{3})+2\zeta (\overline{1},4)+\zeta (\overline{2},3)\right),\hfill \end{array}$$

(53)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^m{H}_n{\overline{H}}_m}{nm(n+m)}}\hfill \\ =-\left(\zeta (\overline{1},\overline{1},1,2)+2\zeta (1,\overline{1},\overline{1},2)+3\zeta (1,1,\overline{1},\overline{2})+\zeta (2,\overline{1},\overline{2})+\zeta (2,1,2)\right.\hfill \\ +\zeta (\overline{1},\overline{1},3)+2\zeta (1,\overline{1},\overline{3})+3\zeta (1,1,3)+2\zeta (1,\overline{2},\overline{2})+2\zeta (1,2,2)\hfill \\ \left.+4\zeta (1,4)+2\zeta (2,3)\right),\hfill \end{array}$$

(54)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{H}_n{H}_m}{nm(n+m)}}\hfill \\ =6\zeta (1,1,1,\overline{2})+6\zeta (1,1,\overline{3})+4\zeta (1,2,\overline{2})+2\zeta (2,1,\overline{2})+4\zeta (1,\overline{4})+2\zeta (2,\overline{3}),\hfill \end{array}$$

(55)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^n{H}_n{\overline{H}}_m}{nm(n+m)}}\hfill \\ =-\left(\zeta (\overline{1},\overline{1},1,\overline{2})+2\zeta (1,\overline{1},\overline{1},\overline{2})+3\zeta (1,1,\overline{1},2)+\zeta (2,\overline{1},2)+\zeta (2,1,\overline{2})\right.\hfill \\ +3\zeta (1,1,\overline{3})+2\zeta (1,\overline{1},3)+\zeta (\overline{1},\overline{1},\overline{3})+2\zeta (1,2,\overline{2})+2\zeta (1,\overline{2},2)\hfill \\ \left.+4\zeta (1,\overline{4})+2\zeta (2,\overline{3})\right),\hfill \end{array}$$

(56)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{H}_n{\overline{H}}_m}{nm(n+m)}}\hfill \\ =-\left(3\zeta (\overline{1},1,1,\overline{2})+2\zeta (\overline{1},\overline{1},1,\overline{2})+\zeta (1,\overline{1},\overline{1},\overline{2})+\zeta (\overline{2},\overline{1},\overline{2})+\zeta (\overline{2},1,\overline{2})\right.\hfill \\ +3\zeta (\overline{1},1,\overline{3})+2\zeta (\overline{1},\overline{1},\overline{3})+\zeta (1,\overline{1},3)+2\zeta (\overline{1},\overline{2},\overline{2})+2\zeta (\overline{1},2,\overline{2})\hfill \\ \left.+2\zeta (\overline{1},\overline{4})+\zeta (\overline{2},\overline{3})+2\zeta (\overline{1},4)+\zeta (\overline{2},3)\right),\hfill \end{array}$$

(57)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{\overline{H}}_n{\overline{H}}_m}{nm(n+m)}}\hfill \\ =2\zeta (\overline{1},\overline{1},\overline{1},2)+4\zeta (1,\overline{1},1,2)+2\zeta (\overline{1},\overline{1},\overline{3})+4\zeta (1,\overline{1},3)+4\zeta (1,\overline{2},2)+2\zeta (2,\overline{1},2)\hfill \\ +4\zeta (1,\overline{4})+2\zeta (2,\overline{3}),\hfill \end{array}$$

(58)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^m{\overline{H}}_n{\overline{H}}_m}{nm(n+m)}}\hfill \\ =2\zeta (\overline{1},1,\overline{1},2)+\zeta (\overline{1},\overline{1},\overline{1},2)+\zeta (\overline{1},\overline{1},\overline{1},\overline{2})+2\zeta (\overline{1},1,\overline{1},\overline{2})+\zeta (\overline{2},\overline{1},\overline{2})+\zeta (\overline{2},\overline{1},2)\hfill \\ +2\zeta (\overline{1},1,\overline{3})+\zeta (\overline{1},\overline{1},3)+\zeta (\overline{1},\overline{1},\overline{3})+2\zeta (\overline{1},1,3)+\zeta (\overline{1},\overline{2},\overline{2})+\zeta (\overline{1},2,\overline{2})+\zeta (\overline{1},\overline{2},2)\hfill \\ +\zeta (\overline{1},2,2)+2\zeta (\overline{1},\overline{4})+\zeta (\overline{2},\overline{3})+2\zeta (\overline{1},4)+\zeta (\overline{2},3),\hfill \end{array}$$

(59)

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{\overline{H}}_n{\overline{H}}_m}{nm(n+m)}}\hfill \\ =2\zeta (\overline{1},\overline{1},\overline{1},\overline{2})+4\zeta (1,\overline{1},1,\overline{2})+2\zeta (\overline{1},\overline{1},3)+4\zeta (1,\overline{1},\overline{3})+4\zeta (1,\overline{2},\overline{2})+2\zeta (2,\overline{1},\overline{2})\hfill \\ +4\zeta (1,4)+2\zeta (2,3).\hfill \end{array}$$

(60)

Since the number of corresponding terms for multiple zeta values increases significantly when the parameters are greater than zero, we provide only one additional example here.

$$\begin{array}{c}{\displaystyle \sum _{n,m\ge 1}\frac{{(-1)}^{n+m}{H}_n^{\left(2\right)}{H}_m}{nm(n+m)}}\hfill \\ =3\zeta (1,1,2,\overline{2})+4\zeta (1,2,1,\overline{2})+3\zeta (2,1,1,\overline{2})+3\zeta (1,1,\overline{4})+6\zeta (1,3,\overline{2})+\zeta (3,1,\overline{2})\hfill \\ +4\zeta (1,2,\overline{3})+3\zeta (2,1,\overline{3})+3\zeta (2,2,\overline{2})+6\zeta (1,\overline{5})+3\zeta (2,\overline{4})+\zeta (3,\overline{3}).\hfill \end{array}$$

(61)

In this paper, we present a method by evaluating a particular 3-poset integral using two distinct techniques: one employs the shuffle relations with their corresponding Lyndon words, and the other utilizes the corresponding infinite-series expansions. For example,

When we express the shuffle relation of the above equation as a sum of Lyndon words ([29,32]) and then convert it to alternating multiple zeta values using the Z function, the relationship becomes complex. For example, the above shuffle equation $z{y}^{a_1}x{y}^{b_1}⧢x{y}^{a_2}z{y}^{b_2}⧢x{y}^{a_3}x{y}^{b_3}$ can be expressed as a sum of 15 different Lyndon words. The coefficient of each Lyndon word is made up of a sum of finite products of binomial coefficients. However, once the shuffle relations are determined, the expressions of alternating multiple zeta values can be easily obtained.

Using our method, it becomes straightforward to derive the desired representation of the infinite-series sum as a linear combination of alternating multiple zeta values.