A New State Assessment Method for Hydrodynamic Journal Bearings under Different Assembly Characteristics
Abstract
1. Introduction
2. Fluid–Solid Coupling Analysis of the HJB
2.1. Analysis of Oil Film Lubricating Properties
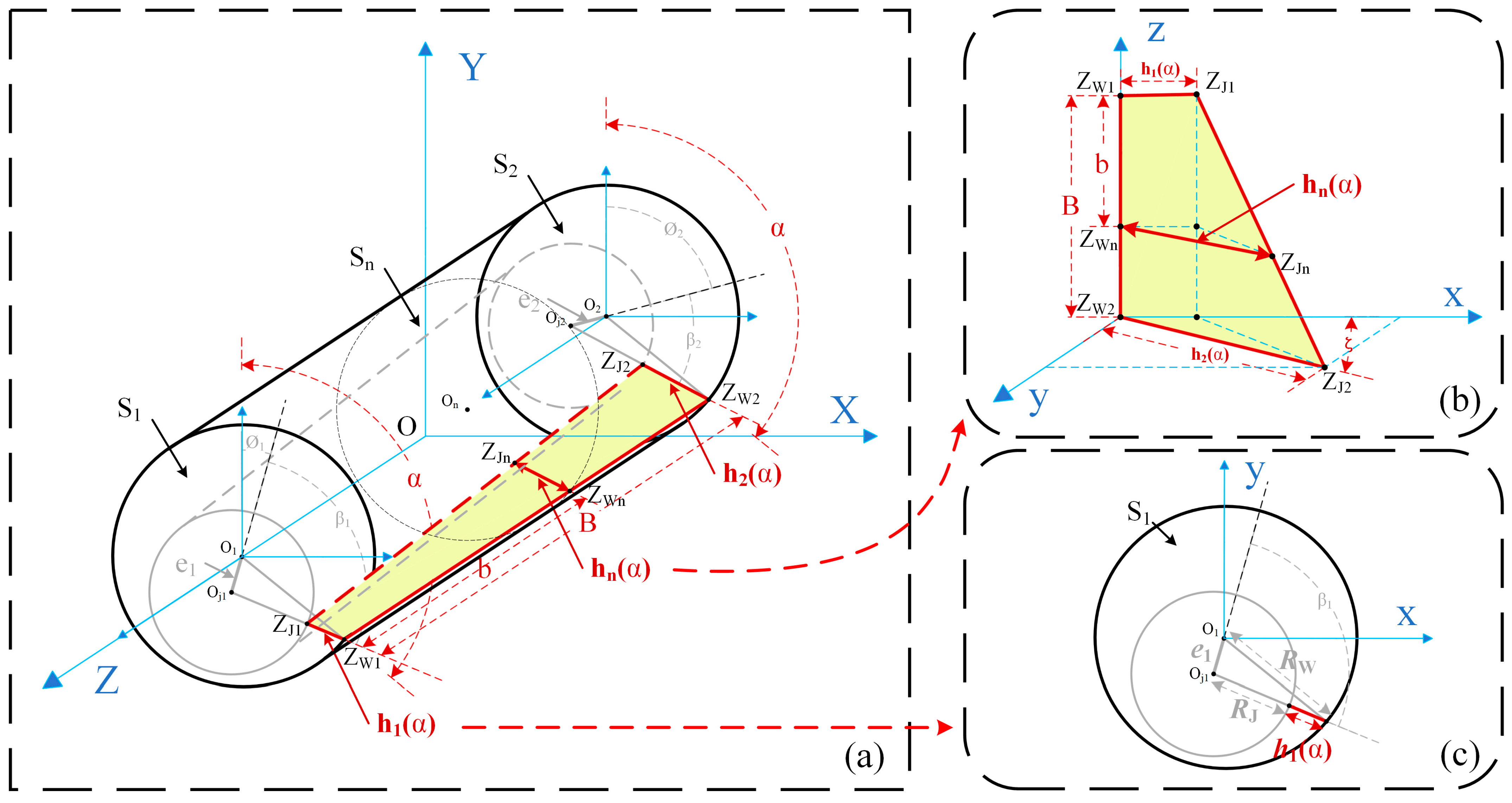
2.1.1. Solution of Oil Film Thickness Distribution
2.1.2. Reynolds Equations and Assumptions
- (1)
- For the purpose of simplifying the calculation of shear stress, the lubricating oil under consideration is assumed to behave as a Newtonian fluid. According to Newton’s law of viscosity, shear stress within a fluid is directly proportional to the velocity gradient perpendicular to the direction of flow.
- (2)
- Due to the extremely small thickness of the oil film, the effect of fluid flow in the direction perpendicular to the film thickness is disregarded.
- (3)
- To streamline the continuity equation, the fluid is assumed to be incompressible, meaning that changes in volume due to pressure variations are considered negligible compared to those resulting from velocity gradients.
- (4)
- To simplify the modeling of fluid flow behavior, the analysis excludes considerations of fluid particle rotation or deformation, focusing solely on the translational characteristics of fluid particles.
- (5)
- To simplify the velocity field near the solid boundary, a no-slip condition is assumed between the fluid and solid surfaces, meaning the velocities of both are equal at the boundary.
- (6)
- To simplify the calculation of pressure distribution within the oil film, the effect of pressure variation in the direction perpendicular to the oil film thickness is neglected.
- (7)
- To simplify the momentum balance equation, the influences of inertial forces and gravitational effects on the oil film particles are neglected.
2.1.3. Solution of Oil Film Pressure Distribution
2.2. Analysis of Elastic Deformation
3. Definition of Assembly Characteristics
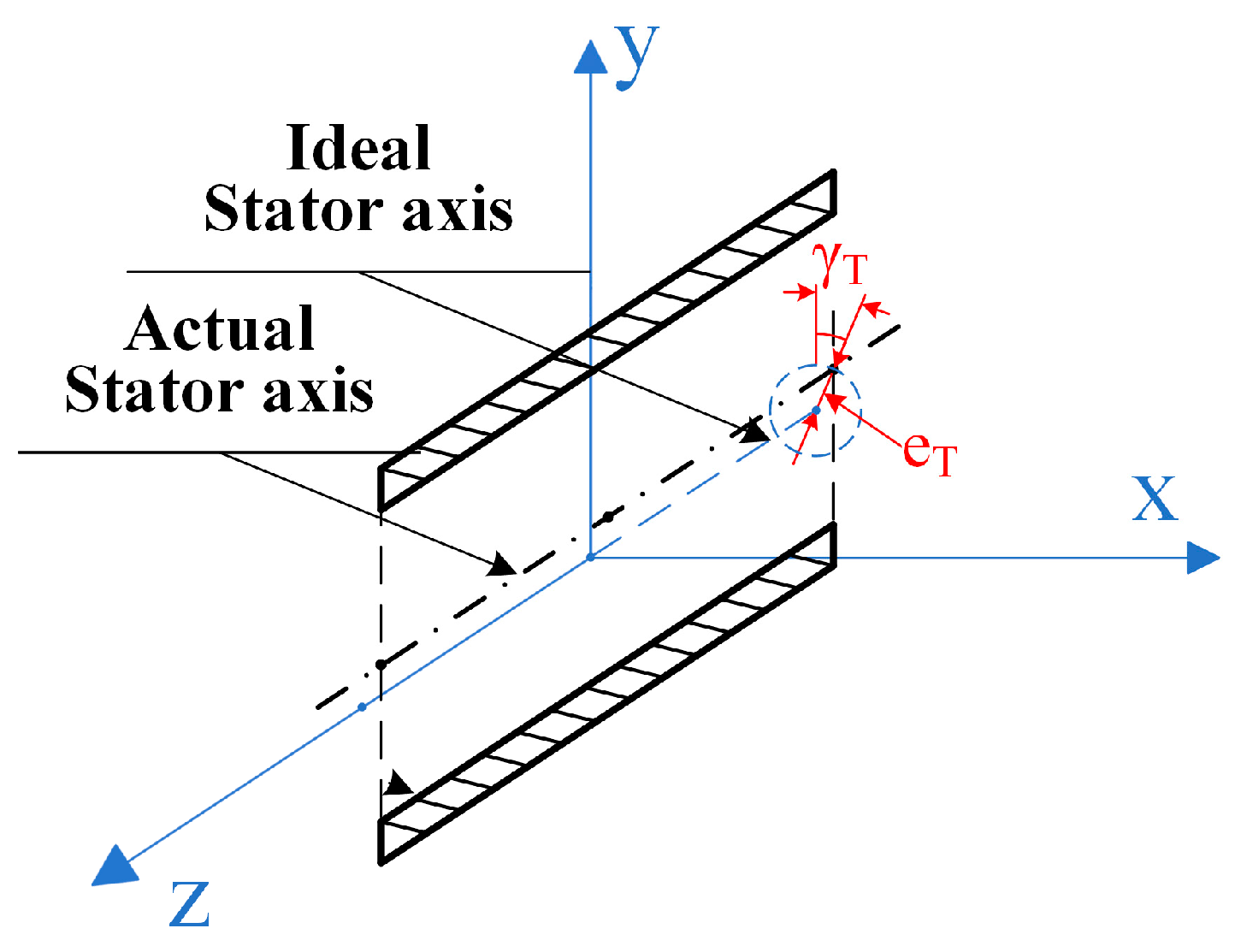
3.1. Stator Axis Translation
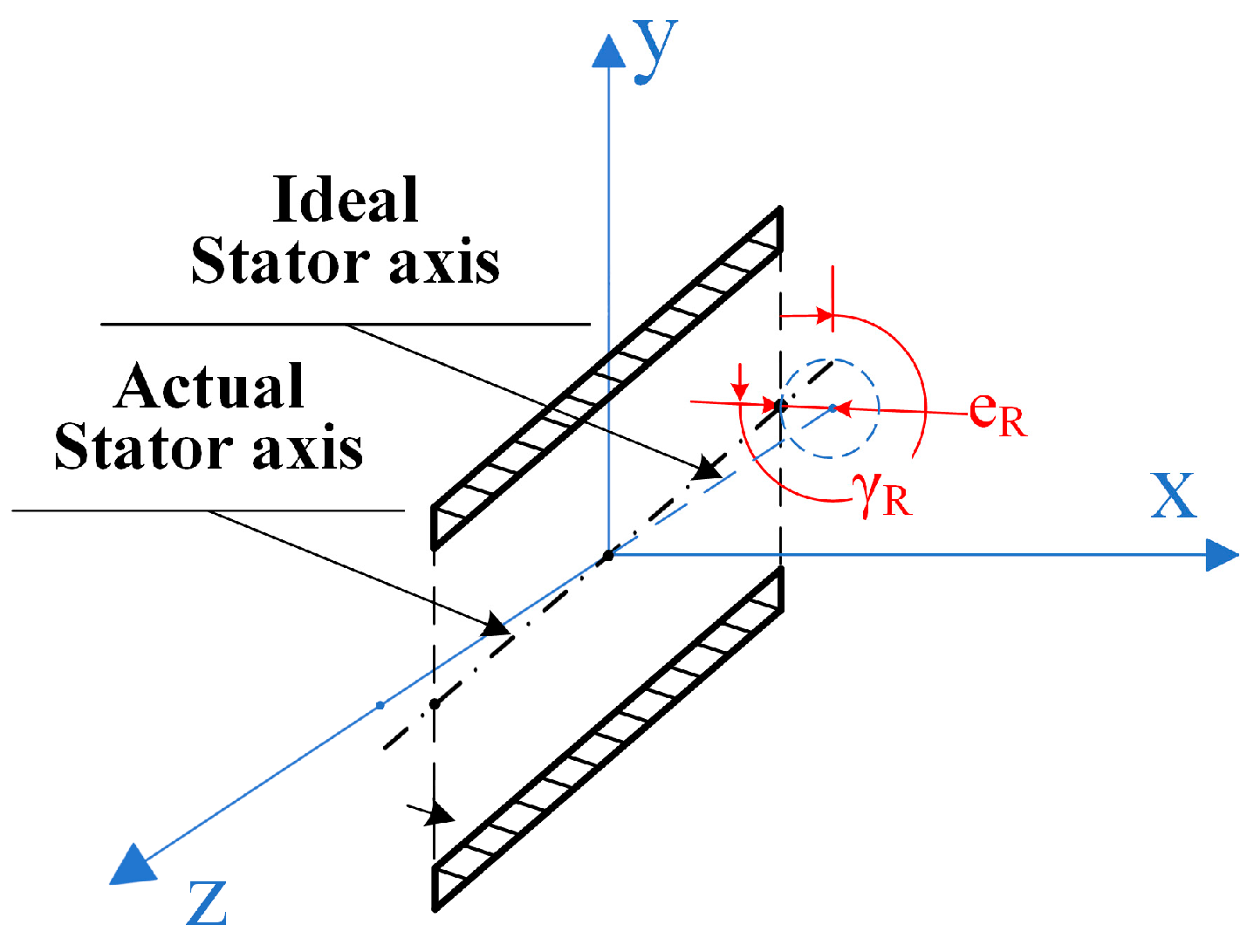
3.2. Stator Axis Rotation
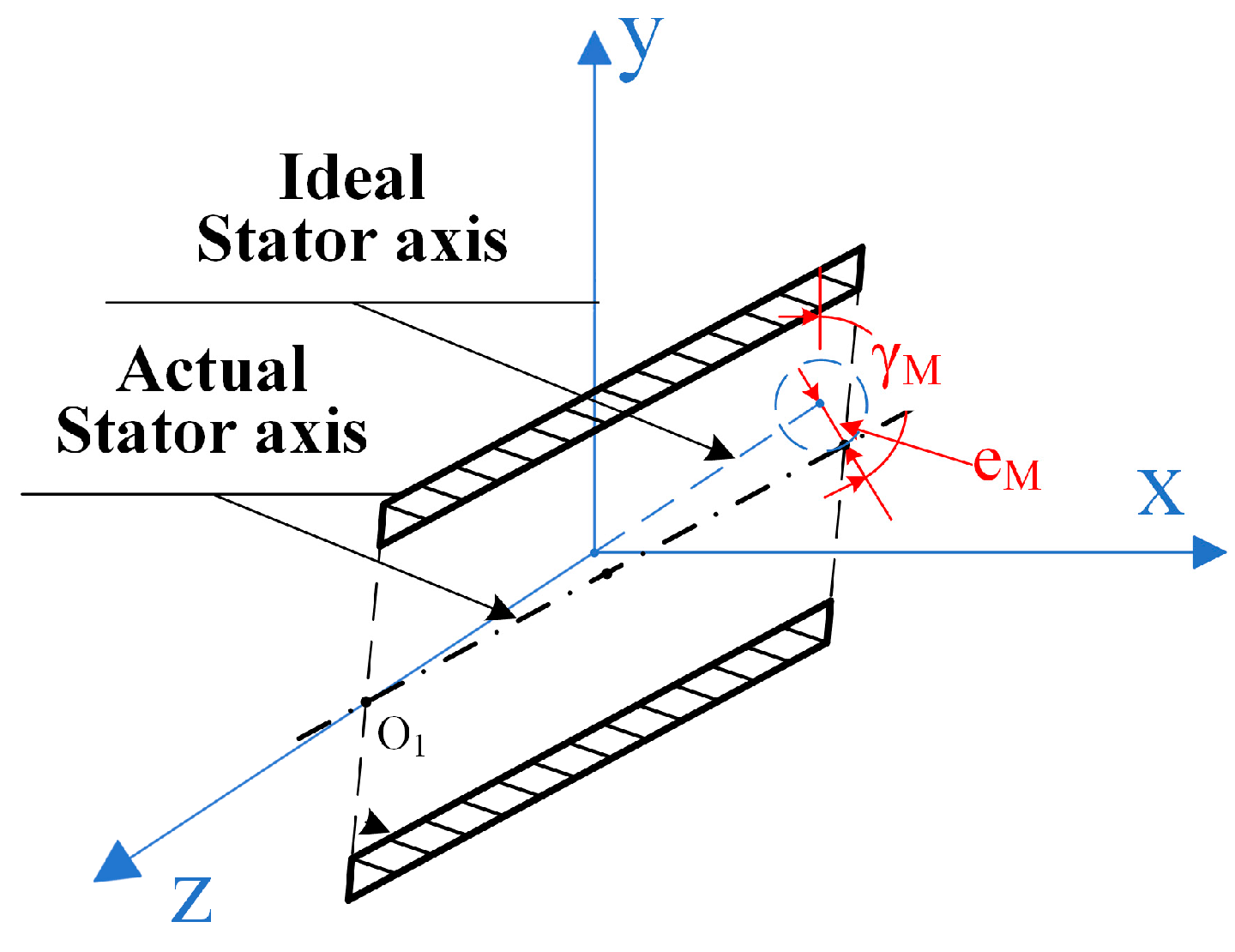
3.3. Stator Axis Misalignment
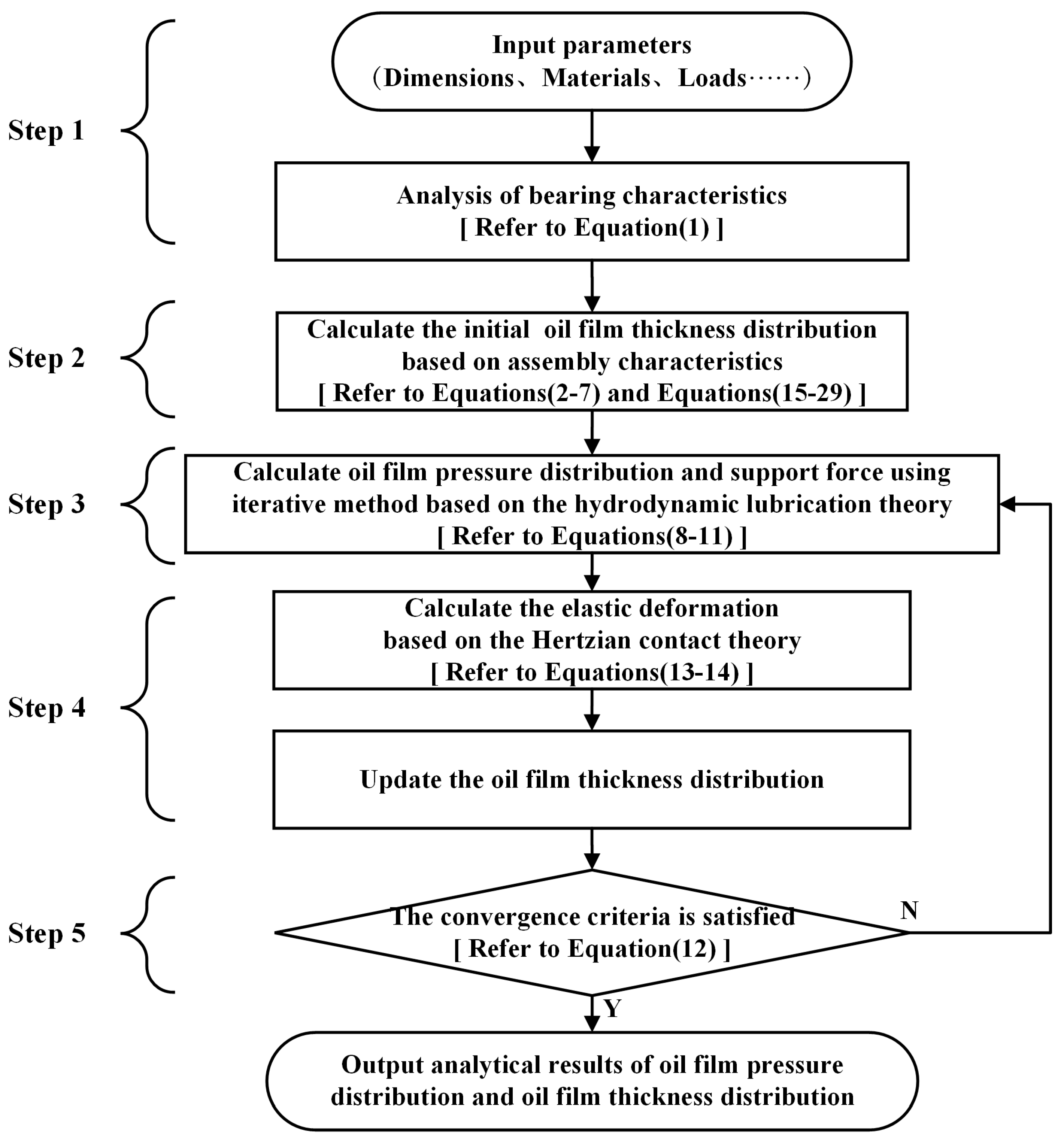
4. Solving Procedure
5. Results and Discussion
5.1. Simulation
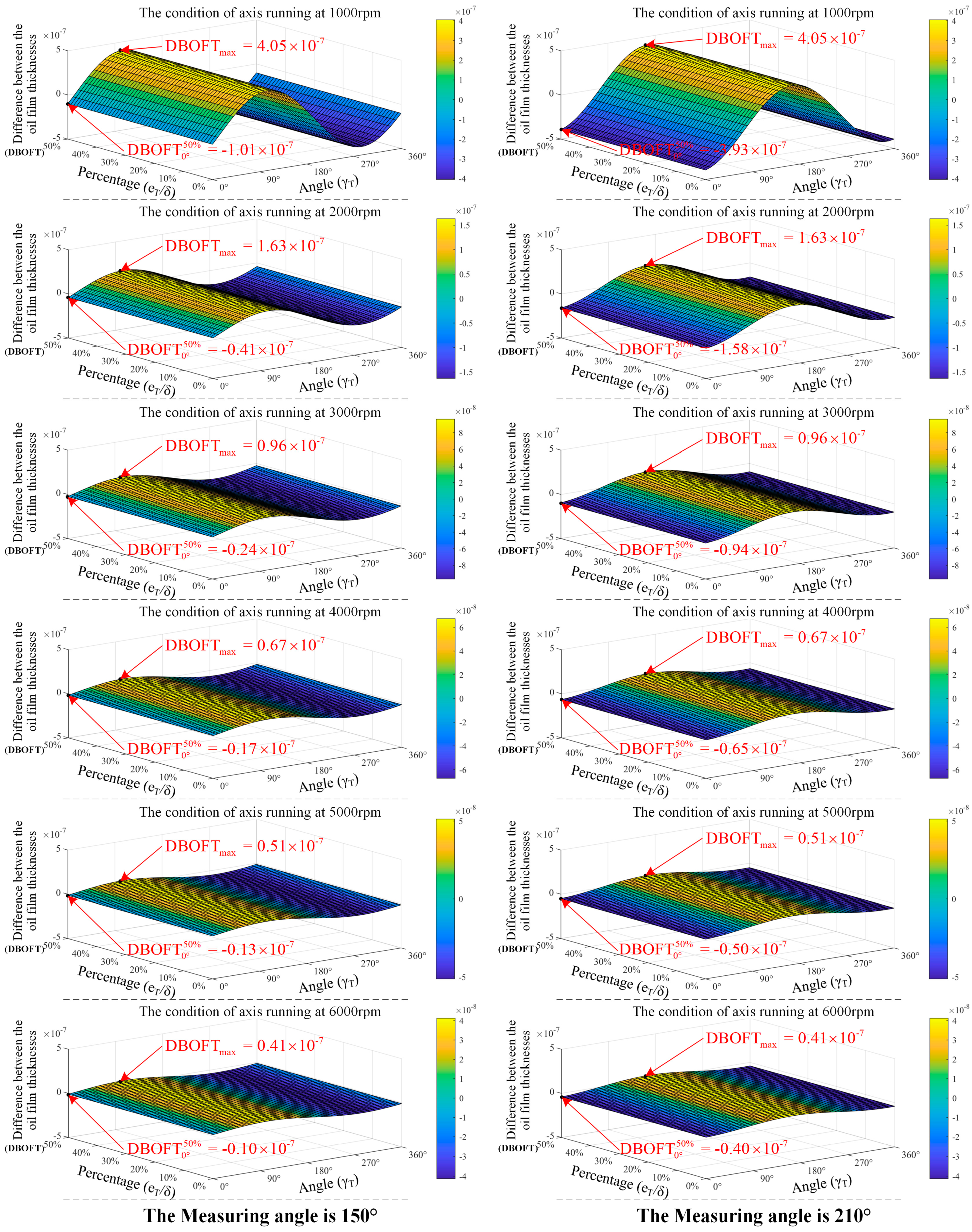
- (1)
- The DBOFT is expressed in nanometer scales.
- (2)
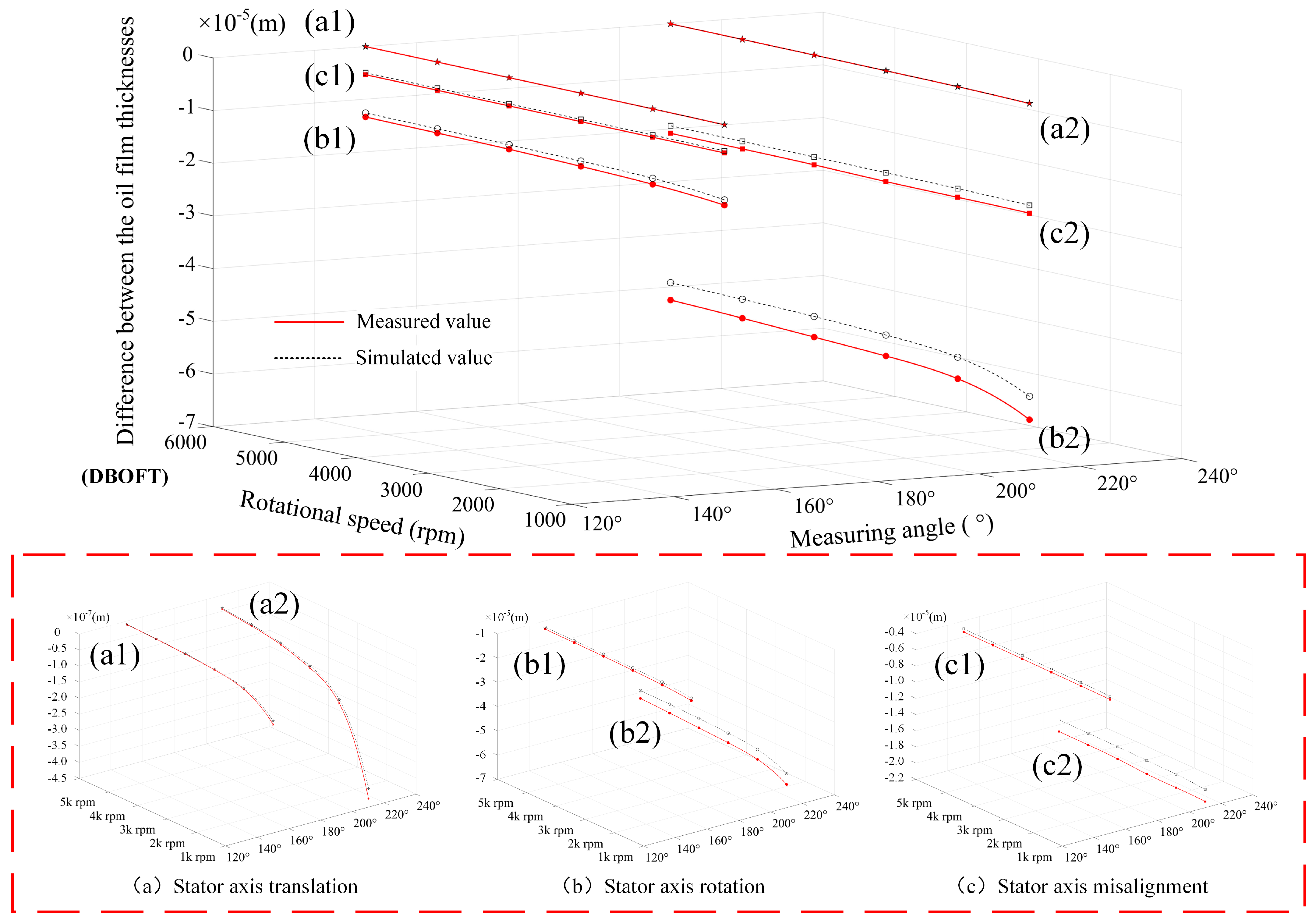
- Through the horizontal comparison of Figure 7, the maximum DBOFT remains unaffected by different measuring angles.
- (3)
- Through the longitudinal comparison of Figure 7, the absolute value of the DBOFT decreases as the rotational speed increases gradually, indicating a tendency towards stable operation of the Rotor-Bearing system.
- (4)
- According to the subgraphs in Figure 7, the DBOFT remains unaffected by varying linear distance () when the angular displacement () is constant.
- (5)
- The variation range of the DBOFT is significantly influenced by the rotational speed when the linear distance () and the angular displacement () are constant. There is a specific scenario where and . When the range of rotational speed is , the variation range of the DBOFT is at a measuring angle of 150 degrees and at a measuring angle of 210 degrees, with the DBOFT decreasing by 90.1 percent and 89.8 percent, respectively.
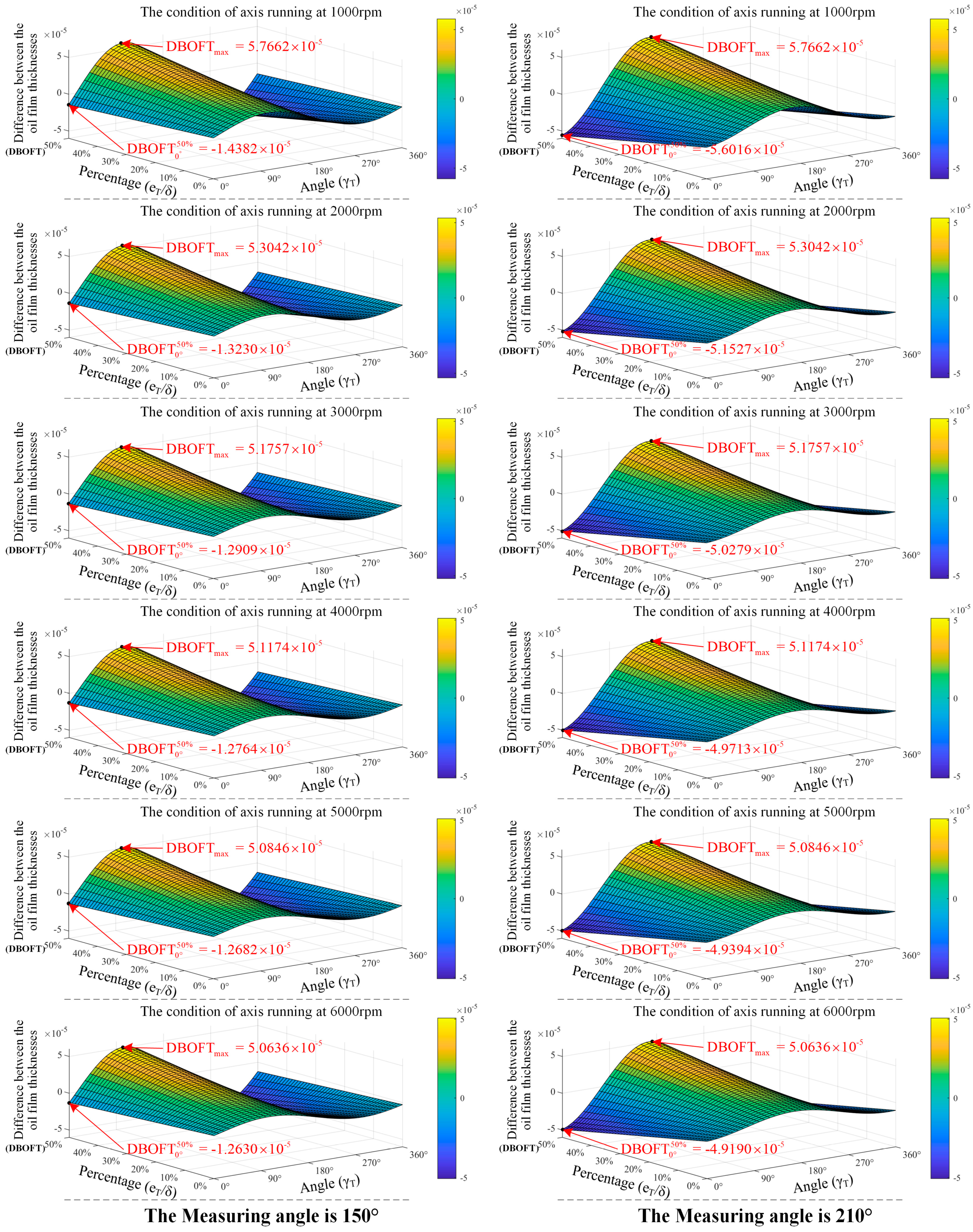
- (1)
- Similar to the radial clearance, the magnitude order of the DBOFT is micron. Therefore, the rotation scenario can be effectively distinguished from the translation scenario.
- (2)
- Through the horizontal comparison of Figure 8, the maximum DBOFT remains unaffected by different measuring angles, which is analogous to the translation scenario.
- (3)
- Through the longitudinal comparison of Figure 8, the absolute value of the DBOFT decreases as the rotational speed increases gradually, which is analogous to the translation scenario, indicating a tendency toward stable operation of the Rotor-Bearing system.
- (4)
- The variation range of the DBOFT is less influenced by the rotational speed when the linear distance () and the angular displacement () are constant. There is a specific scenario where and . When the range of rotational speed is , the variation range of the DBOFT is at a measuring angle of 150 degrees and at a measuring angle of 210 degrees, both of which exhibit a decrease of 12.2 percent.
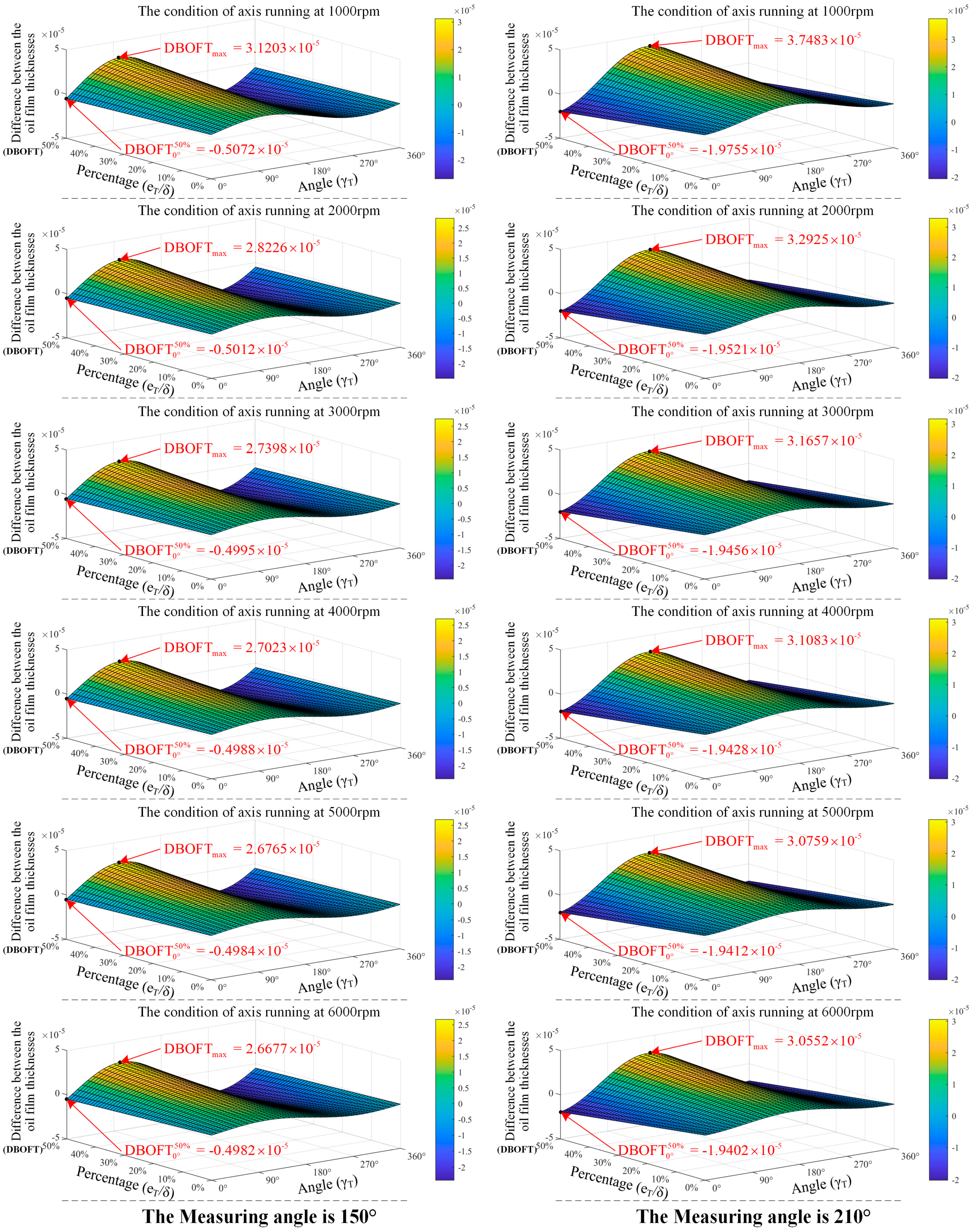
- (1)
- Similar to the radial clearance, the magnitude order of the DBOFT is micron. Therefore, the misalignment scenario can be effectively distinguished from the translation scenario.
- (2)
- Through the horizontal comparison of Figure 9, the maximum DBOFT varies with different measuring angles. Therefore, the misalignment scenario can be effectively distinguished from the translation scenario and the rotation scenario.
- (3)
- Through the longitudinal comparison of Figure 9, the absolute value of the DBOFT decreases as the rotational speed increases gradually, which is analogous to the translation scenario and the rotation scenario, indicating a tendency toward stable operation of the Rotor-Bearing system.
- (4)
- The variation range of the DBOFT is subtly influenced by the rotational speed when the linear distance () and the angular displacement () are constant. There is a specific scenario where and . When the range of rotational speed is , the variation range of the DBOFT is at a measuring angle of 150 degrees and at a measuring angle of 210 degrees, both of which exhibit a decrease of 1.8 percent.
5.2. Experiments
5.3. Discussion
6. Conclusions and Future Work
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclatures
| The bearing capacity coefficient | |
| The external load | |
| The clearance ratio | |
| The dynamic viscosity of the lubricating oil | |
| The angular velocity of the shaft journal | |
| The width of the bearing shell | |
| The diameter of the bearing shell | |
| The diameter of the shaft journal | |
| The given angle | |
| The attitude angle of the shaft journal | |
| The difference between any given angle and the attitude angle | |
| The radial clearance | |
| , | The two opposing faces of the bearing shell |
| , | The centers of and |
| , | The centers of the shaft journal within , |
| , | The attitude angles of the shaft journal within , |
| , | The eccentric distances of shaft journal within , |
| , | Points located on circles of the bearing shell at within , |
| , | Points located on circles of the shaft journal at within , |
| , | Oil film thicknesses at the two points (, ) |
| The angle between and | |
| , | The radius of the bearing shell and the shaft journal |
| b | The distance between the two cross-sections (, ) |
| The point located on the circle of the bearing shell at within | |
| The oil film thickness at the point () | |
| , | Parameters used in calculating |
| , , | Parameters used in calculating |
| H | The oil film thickness |
| P | The oil film pressure |
| The linear velocity of the shaft journal | |
| x, y, z | Cartesian axes |
| The dimensionless oil film thickness | |
| The dimensionless oil film pressure | |
| , | Dimensionless Cartesian axes |
| , , , , , | Coefficient matrixes |
| The relative error value | |
| The aggregate of radial deformations occurring on the two cylinders | |
| , , | Parameters used in calculating |
| , | Poisson’s ratio of the bearing shell and the shaft journal |
| , | Elastic Modulus of the bearing shell and the shaft journal |
| , | Angular displacement and linear distance within the cross-section () in the stator axis translation scenario |
| , | The eccentric distances of the shaft journal within the two designated cross-sections (, ) in the stator axis translation scenario |
| , | The attitude angles of the shaft journal within the two designated cross-sections (, ) in the stator axis translation scenario |
| , | Variations in attitude angles under the condition of stator axis translation |
| , | Angular displacement and linear distance within the cross-section () in the stator axis rotation scenario |
| , | The eccentric distances of the shaft journal within the two designated cross-sections (, ) in the stator axis rotation scenario |
| , | The attitude angles of the shaft journal within the two designated cross-sections (, ) in the stator axis rotation scenario |
| , | Variations in attitude angles under the condition of stator axis rotation |
| , | Angular displacement and linear distance within the cross-section () in the stator axis misalignment scenario |
| , | The eccentric distances of the shaft journal within the two designated cross-sections (, ) in the stator axis misalignment scenario |
| , | The attitude angle of the shaft journal within the two designated cross-sections (, ) in the stator axis misalignment scenario |
| Variations in attitude angle under the condition of stator axis misalignment |
References
- Gui, W.; Chen, X.; Zhou, H.; Yang, S.; Lu, G. Transient running stability assessment of industrial rotating machinery based on bag of correlated vibration feature representation. Meas. Sci. Technol. 2023, 34, 015110. [Google Scholar] [CrossRef]
- Zheng, H.; Qiao, X. Reliability Analysis Method of Rotating Machinery Based on Conditional Random Field. Comput. Intell. Neurosci. 2022, 2022, 7326730. [Google Scholar] [CrossRef] [PubMed]
- Ribeiro, E.A.; Alves, D.S.; Cavalca, K.L.; Bavastri, C.A. Stability analysis and optimization of a hybrid rotating machinery support combining journal bearings with viscoelastic supports. Mech. Mach. Theory 2021, 156, 104166. [Google Scholar] [CrossRef]
- da Silva, D.A.; Daniel, G.B.; Cavalca, K.L. Development of a Robust Test Rig for Tilting Pad Journal Bearing Validation. Machines 2022, 10, 189. [Google Scholar] [CrossRef]
- Yan, K.; Huang, D. Investigation on static and dynamic characteristics of high-speed and heavy-load tilting pad journal bearings. Ind. Lubr. Tribol. 2022, 74, 1063–1073. [Google Scholar] [CrossRef]
- Visnadi, L.B.; de Castro, H.F. Influence of bearing clearance and oil temperature uncertainties on the stability threshold of cylindrical journal bearings. Mech. Mach. Theory 2019, 134, 57–73. [Google Scholar] [CrossRef]
- Pérez, N.; Rodríguez, C. Vertical rotor model with hydrodynamic journal bearings. Eng. Fail. Anal. 2021, 119, 104964. [Google Scholar] [CrossRef]
- Sadiq, M.; Ghopa, W.; Nuawi, M.; Rasani, M.; Khamis, N.; Mansor, M. Investigation of stiffness and damping coefficients in fluid film bearing with bio-oils and mineral-based oil. Energy Rep. 2022, 8, 419–429. [Google Scholar] [CrossRef]
- Sadiq, M.I.; Ghopa, W.A.W.; Nuawi, M.Z.; Rasani, M.R.; Sabri, M.A.M. Experimental and Numerical Investigation of Static and Dynamic Characteristics of Bio-Oils and SAE40 in Fluid Film Journal Bearing. Materials 2022, 15, 3595. [Google Scholar] [CrossRef]
- Tofighi-Niaki, E.; Safizadeh, M.S. Dynamic of a Flexible Rotor-Bearing System Supported by Worn Tilting Journal Bearings Experiencing Rub-Impact. Lubricants 2023, 11, 212. [Google Scholar] [CrossRef]
- Erkaya, S.; Doğan, S.; Ulus, Ş. Effects of joint clearance on the dynamics of a partly compliant mechanism: Numerical and experimental studies. Mech. Mach. Theory 2015, 88, 125–140. [Google Scholar] [CrossRef]
- Xu, F.; Ding, N.; Li, N.; Liu, L.; Hou, N.; Xu, N.; Guo, W.; Tian, L.; Xu, H.; Lawrence, W.C.; et al. A review of bearing failure Modes, mechanisms and causes. Eng. Fail. Anal. 2023, 152, 107518. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, C.; Bao, M.; Gu, L.; Wang, L.; Wei, X.; Li, W. Scratch damage on guiding surfaces of high-speed bearings under oil cut-off condition. Eng. Fail. Anal. 2022, 140, 106624. [Google Scholar] [CrossRef]
- Xiong, G.; Mao, Z.; Zhang, J.; Wang, Z.; Wang, H.; Jiang, Z. Coupled effects of misalignment and viscoelastic deformation on dynamically loaded journal bearings. Int. J. Mech. Sci. 2023, 251, 108347. [Google Scholar] [CrossRef]
- Zeng, W.; Lu, W.; Xu, F. Model-based low-speed rotation error prediction for the rigid shaft-bearing system considering the assembly deviation. Mech. Mach. Theory 2023, 184, 105294. [Google Scholar] [CrossRef]
- Zhou, H.; Huang, X.; Wen, G.; Lei, Z.; Dong, S.; Zhang, P.; Chen, X. Construction of health indicators for condition monitoring of rotating machinery: A review of the research. Expert Syst. Appl. 2022, 203, 117297. [Google Scholar] [CrossRef]
- Ma, J.; Fu, C.; Zhang, H.; Chu, F.; Shi, Z.; Gu, F.; Ball, A.D. Modelling non-Gaussian surfaces and misalignment for condition monitoring of journal bearings. Measurement 2021, 174, 108983. [Google Scholar] [CrossRef]
- Wei, S.; Wang, J.; Cui, J.; Song, S.; Li, H.; Fu, J. Online monitoring of oil film thickness of journal bearing in aviation fuel gear pump. Measurement 2022, 204, 112050. [Google Scholar] [CrossRef]
- Ding, N.; Li, H.; Yin, Z.; Jiang, F. A novel method for journal bearing degradation evaluation and remaining useful life prediction under different working conditions. Measurement 2021, 177, 109273. [Google Scholar] [CrossRef]
- Reynolds, O. On the Theory of Lubrication and Its Application to Mr. Beauchamp Tower’s Experiments, including an experimental determination of the viscosity of olive oil. Proc. R. Soc. Lond. 1886, 177, 157–234. [Google Scholar]
- Lin, L.; He, M.; Ma, W.; Wang, Q.; Zhai, H.; Deng, C. Dynamic Characteristic Analysis of the Multi-Stage Centrifugal Pump Rotor System with Uncertain Sliding Bearing Structural Parameters. Machines 2022, 10, 473. [Google Scholar] [CrossRef]
- Wei, B.; Jiao, Y.; Wu, X. Numerical Calculation of Fluid Film Force on Journal Bearings Based on a Biconjugate Gradient-Stabilized Algorithm. J. Tribol. 2022, 144, 114502. [Google Scholar] [CrossRef]
- Çam, M.Y.; Giacopini, M.; Dini, D.; Biancofiore, L. A numerical algorithm to model wall slip and cavitation in two-dimensional hydrodynamically lubricated contacts. Tribol. Int. 2023, 184, 108444. [Google Scholar] [CrossRef]
- Lv, F.; Zhang, X.; Ji, C.; Rao, Z. Theoretical and experimental investigation on local turbulence effect on mixed-lubrication journal bearing during speeding up. Phys. Fluids 2022, 34, 113104. [Google Scholar] [CrossRef]
- Mao, Y.; Li, L.; Li, D.; Zheng, J. Analysis of the Turbulent Lubrication of a Textured Hydrodynamic Journal Bearing. Lubricants 2023, 11, 362. [Google Scholar] [CrossRef]
- Du, F.; Li, D.; Sa, X.; Li, C.; Yu, Y.; Li, C.; Wang, J.; Wang, W. Overview of Friction and Wear Performance of Sliding Bearings. Coatings 2022, 12, 1303. [Google Scholar] [CrossRef]
- Zhang, X.; Yin, Z.; Dong, Q. An experimental study of axial misalignment effect on seizure load of journal bearings. Tribol. Int. 2019, 131, 476–487. [Google Scholar] [CrossRef]
- Abdou, K.M.; Saber, E. Effect of rotor misalignment on stability of journal bearings with finite width. Alex. Eng. J. 2020, 59, 3407–3417. [Google Scholar] [CrossRef]
- Jeon, W.J.; Hong, S.H. A New Type of Misaligned Journal Bearing with Flexible Structure. Lubricants 2023, 11, 256. [Google Scholar] [CrossRef]
- Sysaykeo, D.; Linares, J.M.; Mermoz, E. Wear Behavior of a Bio-inspired Bearing for off-center Loads. J. Bionic Eng. 2020, 17, 1251–1262. [Google Scholar] [CrossRef]
- Jang, J.Y.; Khonsari, M.M. On the characteristics of misaligned journal bearings. Lubricants 2015, 3, 27–53. [Google Scholar] [CrossRef]
- Guoan, Y. Practical Techniques for Diagnosing Sliding Bearing Failures, 1st ed.; China Petrochemical Press: Beijing, China, 2012; pp. 14–23. [Google Scholar]
- Zhang, J.; Dong, Q. Lubrication performance analysis of crankshaft bush in compressor. Eng. Fail. Anal. 2018, 90, 277–289. [Google Scholar] [CrossRef]
- Du, F.; Chen, C.; Zhang, K. Fluid Characteristics Analysis of the Lubricating Oil Film and the Wear Experiment Investigation of the Sliding Bearing. Coatings 2022, 12, 67. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, M.; Qin, D.; Yan, Z. Performance of high-speed hydrodynamic sliding bearings with lubricating oils combining laminar and turbulent flows. Adv. Mech. Eng. 2020, 12, 1–13. [Google Scholar] [CrossRef]
- Reis, V.L.; Daniel, G.B.; Cavalca, K.L. Dynamic analysis of a lubricated planar slider-crank mechanism considering friction and Hertz contact effects. Mech. Mach. Theory 2014, 74, 257–273. [Google Scholar] [CrossRef]
- Goldsmith, W.; Frasier, J.T. Impact: The Theory and Physical Behavior of Colliding Solids. J. Appl. Mech. 1961, 28, 639. [Google Scholar] [CrossRef]
- Dubowsky, S.; Freudenstein, F. Dynamic analysis of mechanical systems with clearances—Part 1: Formation of dynamic response. J. Manuf. Sci. Eng. Trans. ASME 1971, 93, 305–309. [Google Scholar] [CrossRef]
- Wang, S.C.; Xie, B.R.; Huang, S.M. Design and Analysis of Small Size Eddy Current Displacement Sensor. Sensors 2022, 22, 7444. [Google Scholar] [CrossRef] [PubMed]
- Tiainen, T.; Viitala, R.; Holopainen, T.P.; Hemming, B. Analysis of total rotor runout components with multi-probe roundness measurement method. Measurement 2021, 179, 109422. [Google Scholar] [CrossRef]
- Li, W.; Hu, J.; Su, Z.; Wang, D. Analysis and design of axial inductive displacement sensor. Measurement 2022, 187, 110159. [Google Scholar] [CrossRef]
- Talantsev, A.; Paz, E.; Böhnert, T.; Araújo, A.; Ferreira, R. Magnetic tunnel junction platforms for linear positioning and nanoscale displacement sensing. Measurement 2023, 223, 113663. [Google Scholar] [CrossRef]

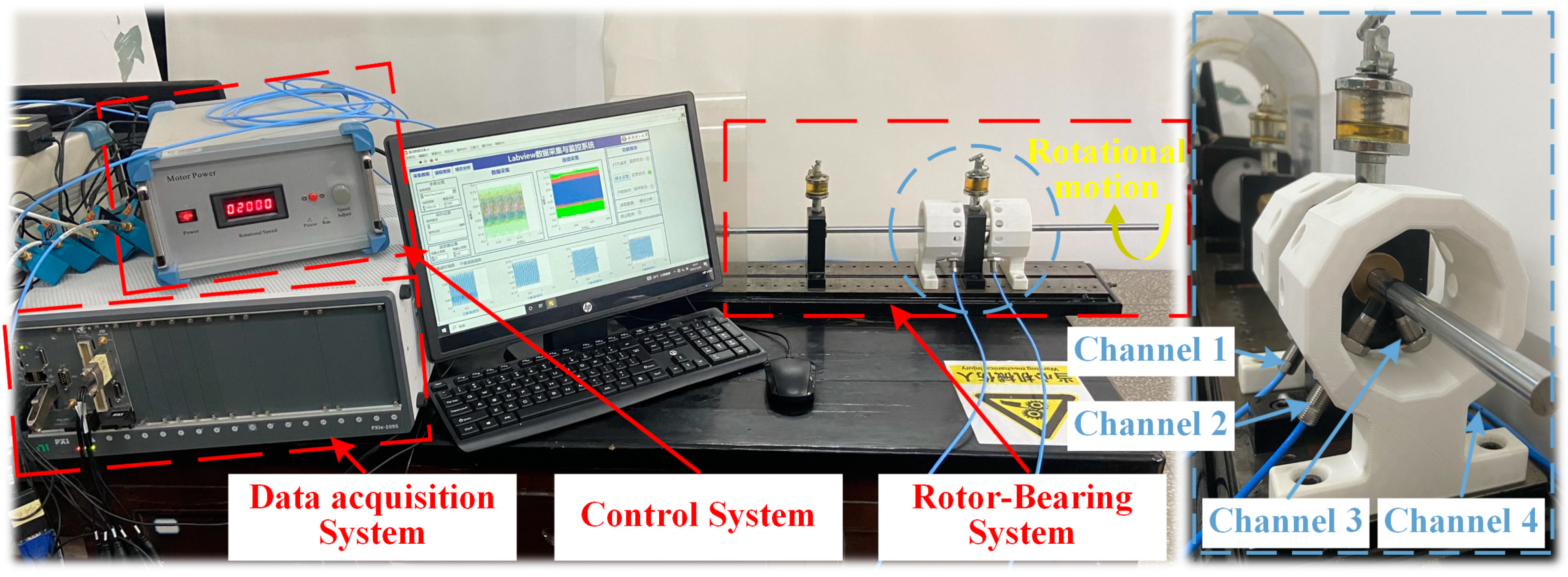
| cc | Value/Units | Parameter | Value/Units |
|---|---|---|---|
| Diameter of bearing shell, | m | Diameter of shaft journal, | m |
| Poisson’s ratio of bearing shell, | 0.33 | Poisson’s ratio of shaft journal, | 0.29 |
| Elastic modulus of bearing shell, | Elastic modulus of shaft journal, | ||
| Width of bearing shell, | m | Dynamic viscosity of lubricating oil, |
| Measuring Angles/° | Rotational Speeds/ | Simulated Values of the DBOF/ | Measured Values of the DBOFT/ | Acc |
|---|---|---|---|---|
| 150 | 90.18% | |||
| 91.11% | ||||
| 92.31% | ||||
| 89.47% | ||||
| 92.86% | ||||
| 90.91% | ||||
| 210 | 92.47% | |||
| 93.49% | ||||
| 93.07% | ||||
| 92.86% | ||||
| 92.59% | ||||
| 93.02% |
| Measuring Angles/° | Rotational Speeds/ | Simulated Values of the DBOF/ | Measured Values of the DBOFT/ | Acc |
|---|---|---|---|---|
| 150 | 93.49% | |||
| 92.10% | ||||
| 92.77% | ||||
| 93.76% | ||||
| 94.03% | ||||
| 94.38% | ||||
| 210 | 92.66% | |||
| 92.64% | ||||
| 92.67% | ||||
| 92.72% | ||||
| 93.22% | ||||
| 93.71% |
| Measuring Angles/° | Rotational Speeds/ | Simulated Values of the DBOF/ | Measured Values of the DBOFT/ | Acc |
|---|---|---|---|---|
| 150 | 92.84% | |||
| 92.34% | ||||
| 92.31% | ||||
| 92.63% | ||||
| 92.78% | ||||
| 92.97% | ||||
| 210 | 92.97% | |||
| 92.19% | ||||
| 92.02% | ||||
| 92.88% | ||||
| 93.14% | ||||
| 93.14% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, H.; Dai, J.; Liu, Q.; Mei, J.; He, J. A New State Assessment Method for Hydrodynamic Journal Bearings under Different Assembly Characteristics. Mathematics 2024, 12, 2400. https://doi.org/10.3390/math12152400
Lu H, Dai J, Liu Q, Mei J, He J. A New State Assessment Method for Hydrodynamic Journal Bearings under Different Assembly Characteristics. Mathematics. 2024; 12(15):2400. https://doi.org/10.3390/math12152400
Chicago/Turabian StyleLu, Hong, Jiashun Dai, Qi Liu, Jiangnuo Mei, and Jiji He. 2024. "A New State Assessment Method for Hydrodynamic Journal Bearings under Different Assembly Characteristics" Mathematics 12, no. 15: 2400. https://doi.org/10.3390/math12152400
APA StyleLu, H., Dai, J., Liu, Q., Mei, J., & He, J. (2024). A New State Assessment Method for Hydrodynamic Journal Bearings under Different Assembly Characteristics. Mathematics, 12(15), 2400. https://doi.org/10.3390/math12152400





