An Improved Method for Calculating Wave Velocity Fields in Fractured Rock Based on Wave Propagation Probability
Abstract
1. Introduction
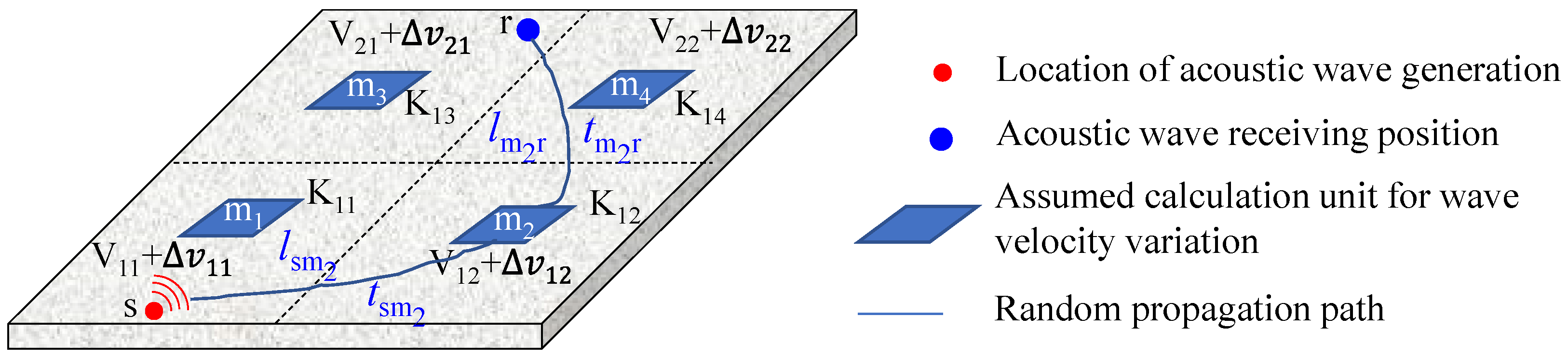
2. Method
2.1. Probability Calculation of Acoustic Wave Path Propagation Based on Diffusion Theory
2.2. Time Shift Solution
2.3. Wave Velocity Field Calculation
3. Numerical and Experimental Verification
3.1. Numerical Verification
3.1.1. Numerical Model
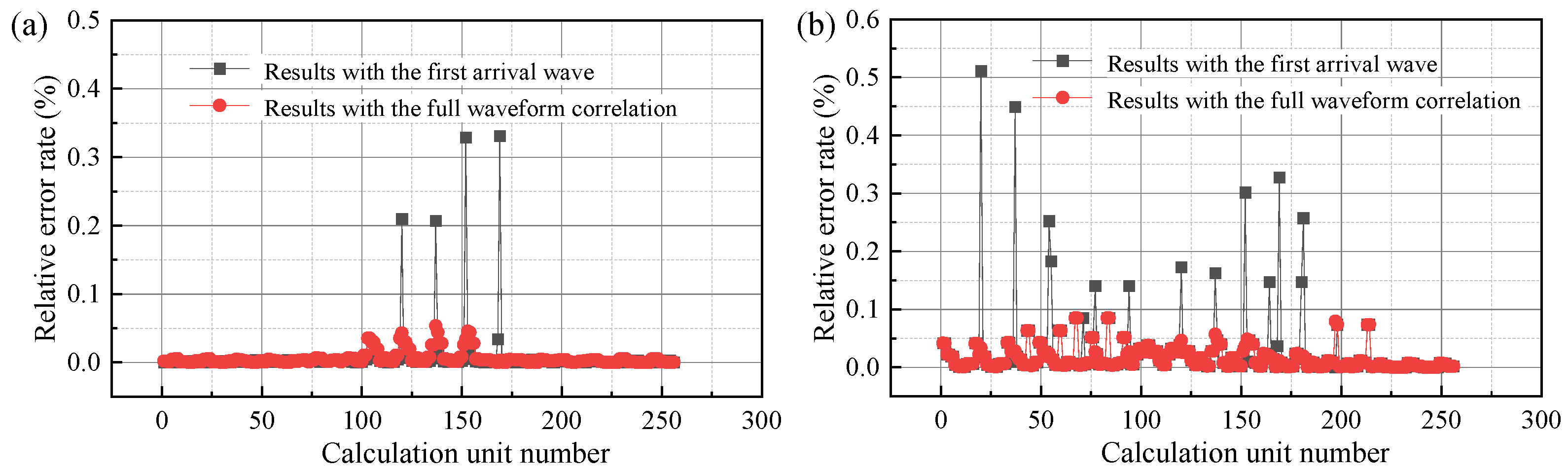
3.1.2. Results
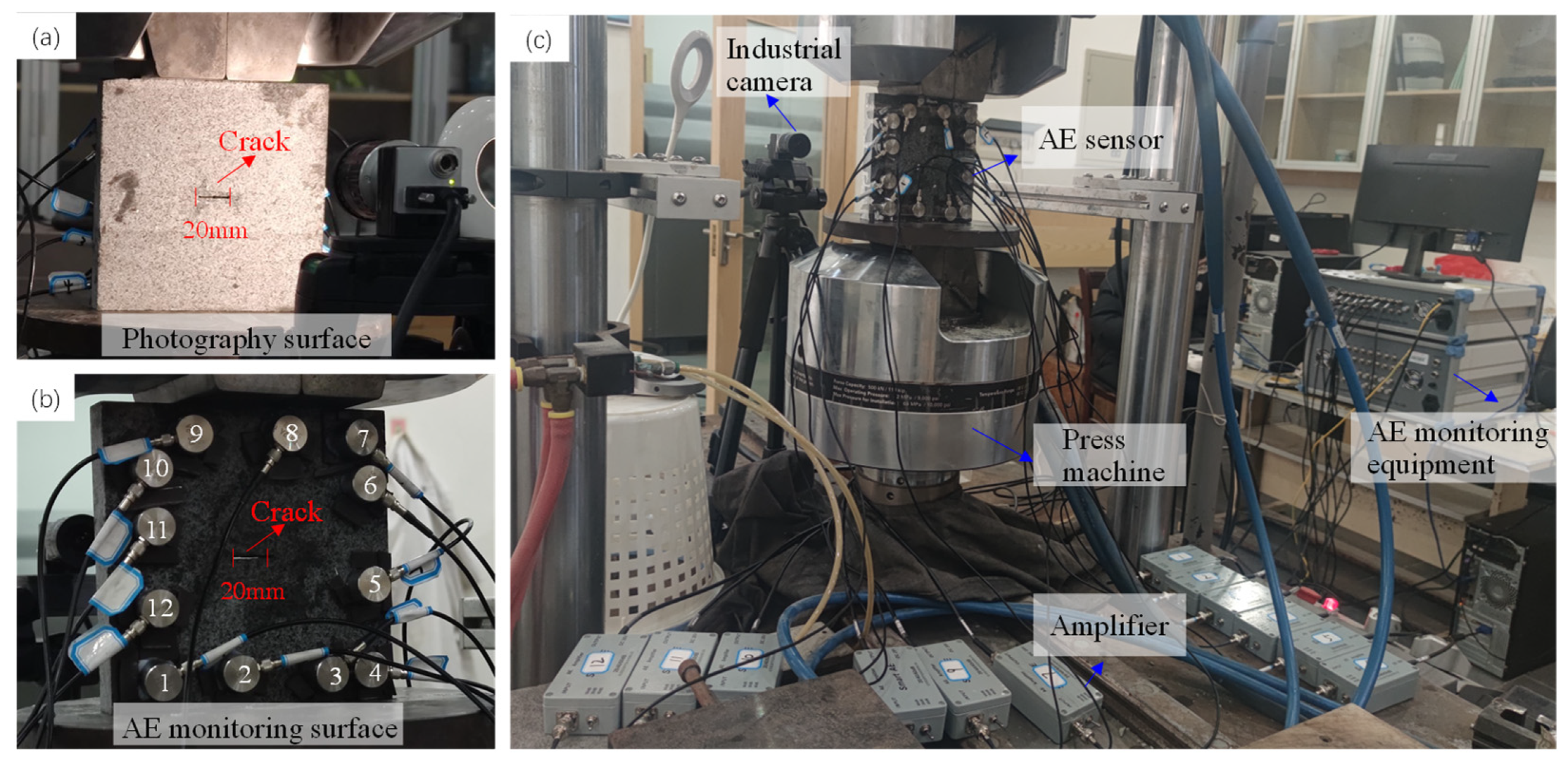
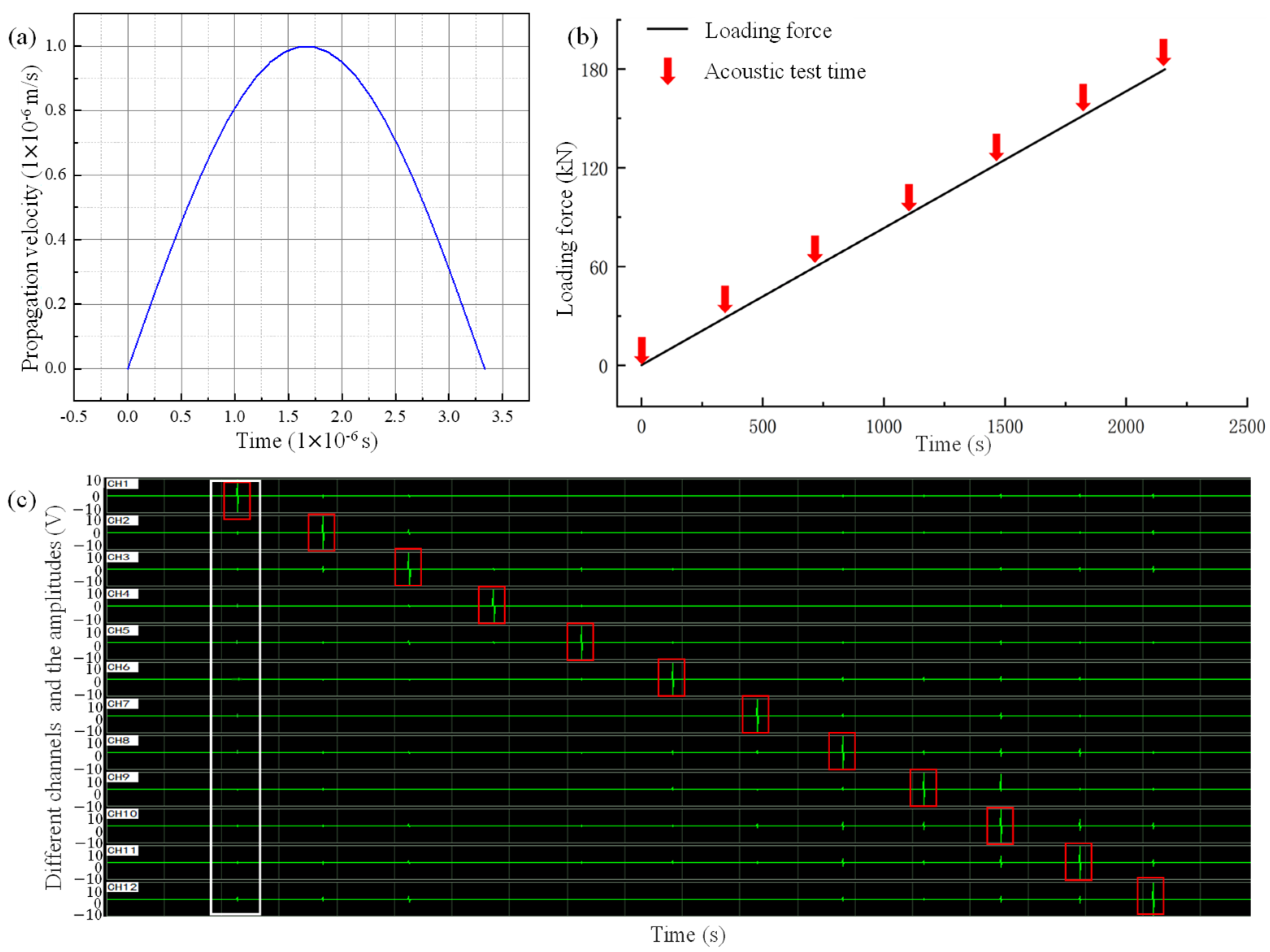
3.2. Experimental Verification
3.2.1. Experiment Process
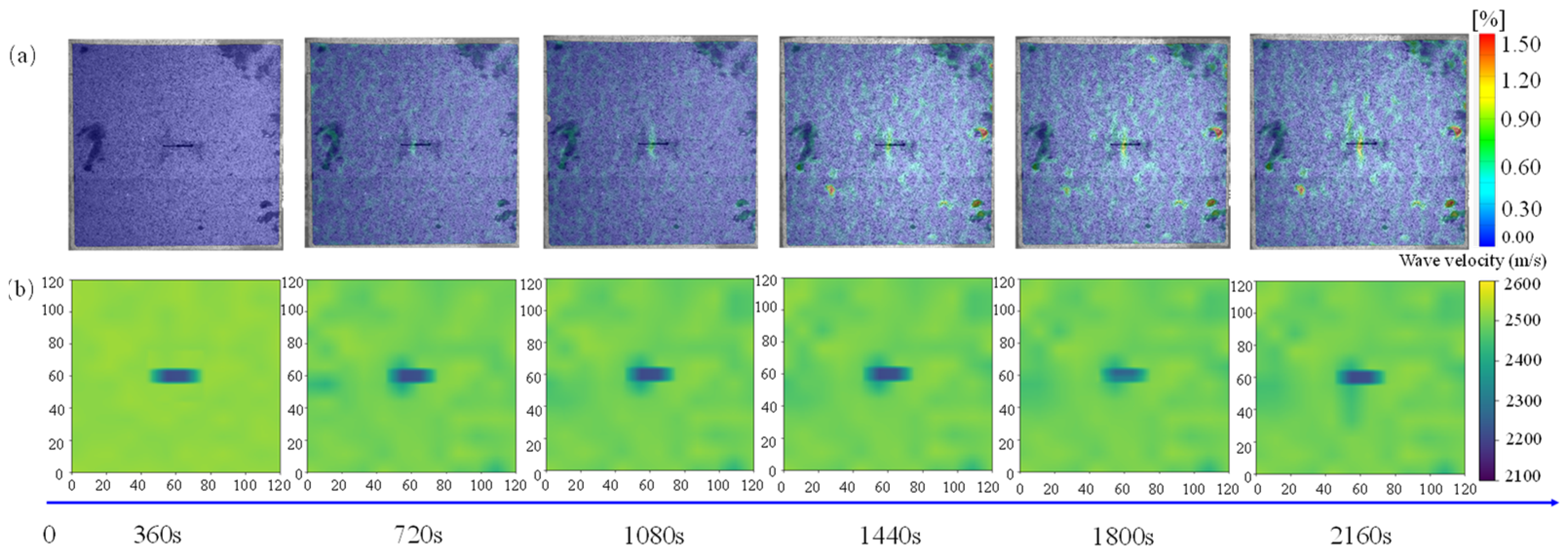
3.2.2. Results
4. Discussion
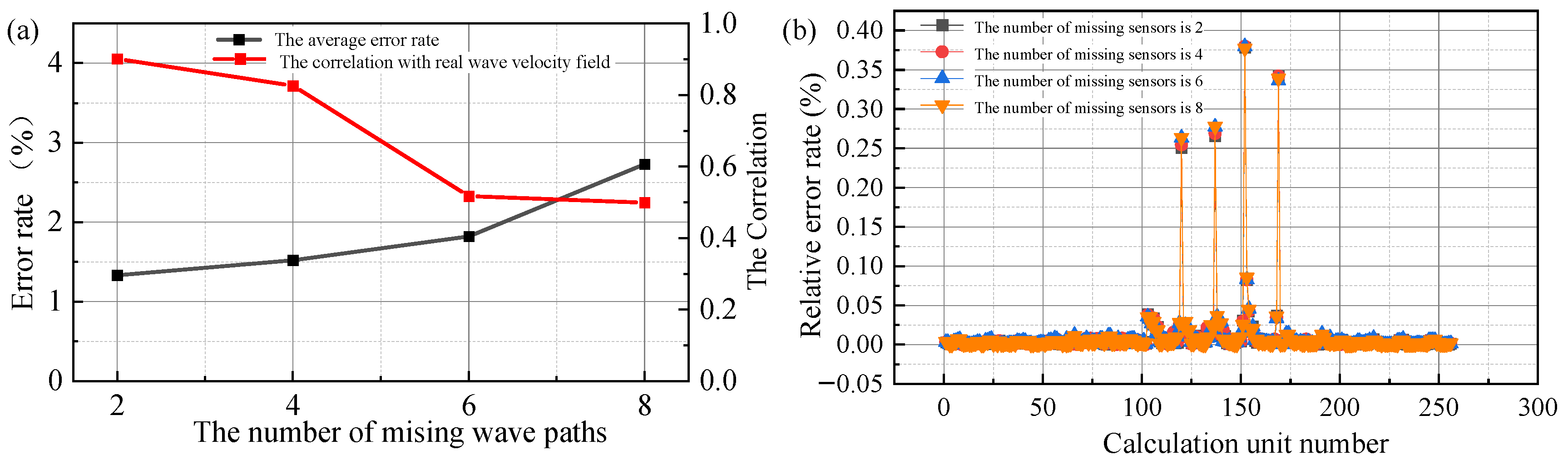
4.1. The Influence of the AE Sensor Missing on the Calculation Results
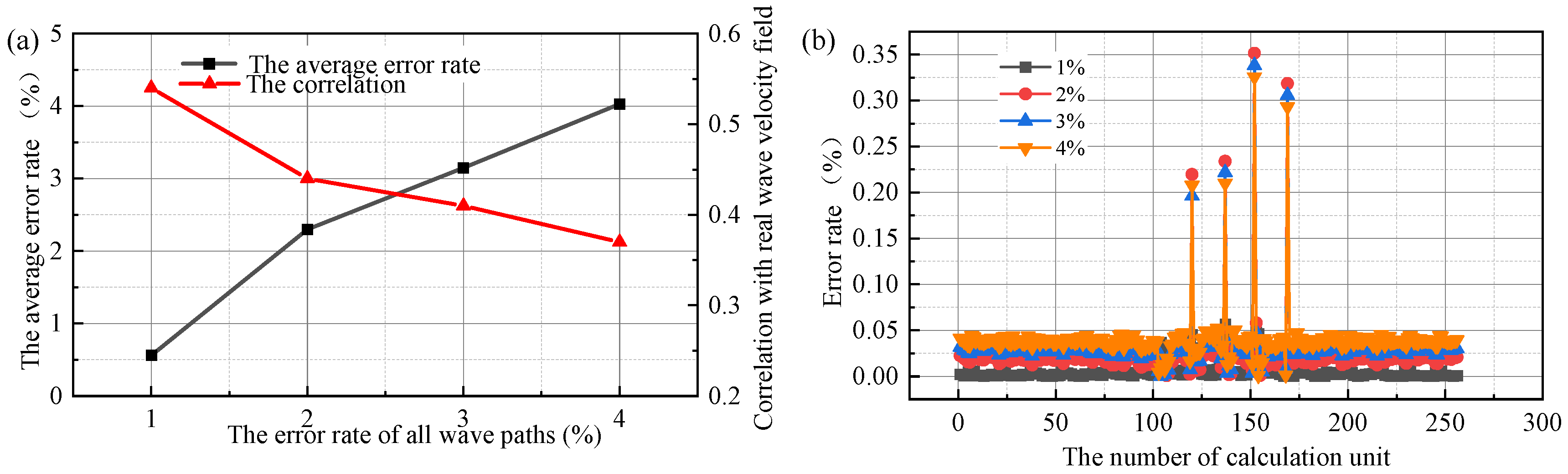
4.2. The Influence of the Wave Path Measurement Error on Calculation Results
5. Conclusions
- (1)
- Based on the stochastic theory of wave propagation in a multi-scattering medium, an imaging method for the wave velocity field based on the probability distribution of wave propagation is proposed. This method combines the advantages of the time shift of the first arrival wave and the coda wave, which not only allows for the determination of the wave velocity field distribution in the medium with existing damage but also enhances the sensitivity of wave velocity field evolution perception in the medium.
- (2)
- Numerical models of fractures with different characteristics were established, and the wave velocity field of models with different fractures was inverted using the proposed method. The relative error rate between the computational units solved by the proposed method and the first arrival wave was analyzed, and the results showed that the computational accuracy of the proposed method has been relatively improved.
- (3)
- An experiment with a rock slab containing fractures was conducted, and the evolution of the wave velocity field during the loading process was calculated using the proposed method. The results were compared and analyzed with digital speckle results, indicating that the proposed method can accurately obtain the distribution of the wave velocity field during the damage evolution process of rocks.
- (4)
- Considering the loss of sensors and the error of measured time shift data caused by medium deformation damage, the influence of reducing the number of sensors by two, four, six, and eight and the error level of propagation path measured time shift by 1%, 2%, 3% and 4% on the calculation results are analyzed respectively. The results show that these two situations will affect the calculation results, and the influence of reducing the number of sensors is smaller than that of measuring time shifts with error.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lu, J.; Zhou, Z.; Chen, X.; Wang, P.; Rui, Y.; Cai, X. Experimental investigation on mode I fracture characteristics of rock-concrete interface at different ages. Constr. Build. Mater. 2022, 349, 128735. [Google Scholar] [CrossRef]
- Tan, L.; Zhou, Z.; Cai, X.; Rui, Y. Analysis of mechanical behaviour and fracture interaction of multi-hole rock mass with DIC measurement. Measurement 2022, 191, 110794. [Google Scholar] [CrossRef]
- Sun, X.; Zhang, M.; Gao, W.; Guo, C.; Kong, Q. A novel method for steel bar all-stage pitting corrosion monitoring using the feature-level fusion of ultrasonic direct waves and coda waves. Struct. Health Monit. 2023, 22, 714–729. [Google Scholar] [CrossRef]
- Zhou, J.; Liu, L.; Zhao, Y.; Zhu, M.; Wang, R.; Zhuang, D. An Improved Rock Damage Characterization Method Based on the Shortest Travel Time Optimization with Active Acoustic Testing. Mathematics 2024, 12, 161. [Google Scholar] [CrossRef]
- Shokouhi, P.; Rivière, J.; Lake, C.R.; Le Bas, P.-Y.; Ulrich, T.J. Dynamic acousto-elastic testing of concrete with a coda-wave probe: Comparison with standard linear and nonlinear ultrasonic techniques. Ultrasonics 2017, 81, 59–65. [Google Scholar] [CrossRef] [PubMed]
- Babaei, M.; Kiarasi, F.; Marashi, S.M.H.; Ebadati, M.; Masoumi, F.; Asemi, K. Stress wave propagation and natural frequency analysis of functionally graded graphene platelet-reinforced porous joined conical–cylindrical–conical shell. Waves Random Complex Media 2021, 1–33. [Google Scholar] [CrossRef]
- Dong, L.; Cao, H.; Hu, Q.; Zhang, S.; Zhang, X. Error distribution and influencing factors of acoustic emission source location for sensor rectangular network. Measurement 2024, 225, 113983. [Google Scholar] [CrossRef]
- Dong, L.; Chen, Y.; Sun, D.; Zhang, Y.; Deng, S. Implications for identification of principal stress directions from acoustic emission characteristics of granite under biaxial compression experiments. J. Rock Mech. Geotech. Eng. 2023, 15, 852–863. [Google Scholar] [CrossRef]
- Zhang, G.; Wu, S.; Zhang, S.; Guo, P. P-wave velocity tomography and acoustic emission characteristics of sandstone under uniaxial compression. Rock Soil. Mech. 2023, 44, 9. [Google Scholar] [CrossRef]
- Lan, M.; Tang, Y.; Ma, J.; Liu, Z. Cooperative acoustic emission locating with velocity tomography in true triaxial experiment. Eng. Fract. Mech. 2023, 292, 109633. [Google Scholar] [CrossRef]
- Liu, D.; Cao, K.; Tang, Y.; Zhong, A.; Jian, Y.; Gong, C.; Meng, X. Ultrasonic and X-CT measurement methods for concrete deterioration of segmental lining under wetting-drying cycles and sulfate attack. Measurement 2022, 204, 111983. [Google Scholar] [CrossRef]
- Wojtczak, E.; Rucka, M.; Skarżyński, Ł. Monitoring the fracture process of concrete during splitting using integrated ultrasonic coda wave interferometry, digital image correlation and X-ray micro-computed tomography. NDT E Int. 2022, 126, 102591. [Google Scholar] [CrossRef]
- Zhang, Y.; Yao, X.; Liang, P.; Wang, K.; Sun, L.; Tian, B.; Liu, X.; Wang, S. Fracture evolution and localization effect of damage in rock based on wave velocity imaging technology. J. Cent. South Univ. 2021, 28, 2752–2769. [Google Scholar] [CrossRef]
- Zhang, W.; Sun, Q.; Zhang, Y.; Xue, L.; Kong, F. Porosity and wave velocity evolution of granite after high-temperature treatment: A review. Environ. Earth Sci. 2018, 77, 350. [Google Scholar] [CrossRef]
- Varamashvili, N.D.; Asanidze, B.Z.; Jakhutashvili, M.N. Ultrasonic Tomography and Pulse Velocity for Nondestructive Testing of Concrete Structures. J. Georgian Geophys. Soc. 2020, 23, 14–20. [Google Scholar] [CrossRef]
- Krauß, M.; Hariri, K. Determination of initial degree of hydration for improvement of early-age properties of concrete using ultrasonic wave propagation. Cem. Concr. Compos. 2006, 28, 299–306. [Google Scholar] [CrossRef]
- Farin, M.; Moulin, E.; Chehami, L.; Benmeddour, F.; Nicard, C.; Campistron, P.; Bréhault, O.; Dupont, L. Monitoring saltwater corrosion of steel using ultrasonic coda wave interferometry with temperature control. Ultrasonics 2022, 124, 106753. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Ding, W.; Dong, P.; Okude, N.; Asaue, H.; Shiotani, T. Identification of hydration stages: An innovative study of ultrasonic coda waves using integrated sensing element (ISE). Constr. Build. Mater. 2023, 401, 132764. [Google Scholar] [CrossRef]
- Zheng, Q.; Qian, J.; Zhang, H.; Chen, Y.; Zhang, S. Velocity tomography of cross-sectional damage evolution along rock longitudinal direction under uniaxial loading. Tunn. Undergr. Space Technol. 2024, 143, 105503. [Google Scholar] [CrossRef]
- Liu, B.; Wang, J.; Yang, S.; Xu, X.; Ren, Y. Forward prediction for tunnel geology and classification of surrounding rock based on seismic wave velocity layered tomography. J. Rock Mech. Geotech. Eng. 2023, 15, 179–190. [Google Scholar] [CrossRef]
- Yoon, J.; Kim, H.; Shin, S.W.; Sim, S.-H. Rheology-based determination of injectable grout fluidity for preplaced aggregate concrete using ultrasonic tomography. Constr. Build. Mater. 2020, 260, 120447. [Google Scholar] [CrossRef]
- Shan, S.; Liu, Z.; Cheng, L.; Pan, Y. Metamaterial-enhanced coda wave interferometry with customized artificial frequency-space boundaries for the detection of weak structural damage. Mech. Syst. Signal Process. 2022, 174, 109131. [Google Scholar] [CrossRef]
- Cheng, W.; Fan, Z.; Tan, K.H. Characterisation of corrosion-induced crack in concrete using ultrasonic diffuse coda wave. Ultrasonics 2023, 128, 106883. [Google Scholar] [CrossRef] [PubMed]
- Zotz-Wilson, R.; Douma, L.A.N.R.; Sarout, J.; Dautriat, J.; Dewhurst, D.; Barnhoorn, A. Ultrasonic Imaging of the Onset and Growth of Fractures Within Partially Saturated Whitby Mudstone Using Coda Wave Decorrelation Inversion. J. Geophys. Res. Solid Earth 2020, 125, e2020JB020042. [Google Scholar] [CrossRef]
- Zhang, C.; Yan, Q.; Zhang, Y.; Liao, X.; Zhong, H. Intelligent monitoring of concrete-rock interface debonding via ultrasonic measurement integrated with convolutional neural network. Constr. Build. Mater. 2023, 400, 131865. [Google Scholar] [CrossRef]
- Qu, S.; Hilloulin, B.; Saliba, J.; Sbartaï, M.; Abraham, O.; Tournat, V. Imaging concrete cracks using Nonlinear Coda Wave Interferometry (INCWI). Constr. Build. Mater. 2023, 391, 131772. [Google Scholar] [CrossRef]
- Xu, X.; Ran, B.; Jiang, N.; Xu, L.; Huan, P.; Zhang, X.; Li, Z. A systematic review of ultrasonic techniques for defects detection in construction and building materials. Measurement 2024, 226, 114181. [Google Scholar] [CrossRef]
- Pandey, K.; Taira, T.; Dresen, G.; Goebel, T.H. Inferring damage state and evolution with increasing stress using direct and coda wave velocity measurements in faulted and intact granite samples. Geophys. J. Int. 2023, 235, 2846–2861. [Google Scholar] [CrossRef]
- Zhong, B.; Zhu, J. Applications of Stretching Technique and Time Window Effects on Ultrasonic Velocity Monitoring in Concrete. Appl. Sci. 2022, 12, 7130. [Google Scholar] [CrossRef]
- Planès, T.; Larose, E. A review of ultrasonic Coda Wave Interferometry in concrete. Cem. Concr. Res. 2013, 53, 248–255. [Google Scholar] [CrossRef]
- Hilloulin, B.; Zhang, Y.; Abraham, O.; Loukili, A.; Grondin, F.; Durand, O.; Tournat, V. Small crack detection in cementitious materials using nonlinear coda wave modulation. NDT E Int. 2014, 68, 98–104. [Google Scholar] [CrossRef]
- Marcantonio, V.; Monarca, D.; Colantoni, A.; Cecchini, M. Ultrasonic waves for materials evaluation in fatigue, thermal and corrosion damage: A review. Mech. Syst. Signal Process. 2019, 120, 32–42. [Google Scholar] [CrossRef]
- Liu, Z.; Yao, Q.; Kong, B.; Yin, J. Macro-micro mechanical properties of building sandstone under different thermal damage conditions and thermal stability evaluation using acoustic emission technology. Constr. Build. Mater. 2020, 246, 118485. [Google Scholar] [CrossRef]
- Tourin, A.; Derode, A.; Peyre, A.; Fink, M. Transport parameters for an ultrasonic pulsed wave propagating in a multiple scattering medium. J. Acoust. Soc. Am. 2000, 108, 503–512. [Google Scholar] [CrossRef] [PubMed]
- Biwa, S.; Yamamoto, S.; Kobayashi, F.; Ohno, N. Computational multiple scattering analysis for shear wave propagation in unidirectional composites. Int. J. Solids Struct. 2004, 41, 435–457. [Google Scholar] [CrossRef]
- Margerin, L.; Planès, T.; Mayor, J.; Calvet, M. Sensitivity kernels for coda-wave interferometry and scattering tomography: Theory and numerical evaluation in two-dimensional anisotropically scattering media. Geophys. J. Int. 2016, 204, 650–666. [Google Scholar] [CrossRef]
- Xie, F.; Ren, Y.; Zhou, Y.; Larose, E.; Baillet, L. Monitoring Local Changes in Granite Rock Under Biaxial Test: A Spatiotemporal Imaging Application With Diffuse Waves. J. Geophys. Res. Solid Earth 2018, 123, 2214–2227. [Google Scholar] [CrossRef]
- Pacheco, C.; Snieder, R. Time-lapse travel time change of multiply scattered acoustic waves. J. Acoust. Soc. Am. 2005, 118, 1300–1310. [Google Scholar] [CrossRef]
- Snieder, R. Coda Wave Interferometry for Estimating Nonlinear Behavior in Seismic Velocity. Science 2002, 295, 2253–2255. [Google Scholar] [CrossRef]
- Zhou, J.; Zhou, Z.; Zhao, Y.; Cai, X. Global Wave Velocity Change Measurement of Rock Material by Full-Waveform Correlation. Sensors 2021, 21, 7429. [Google Scholar] [CrossRef]



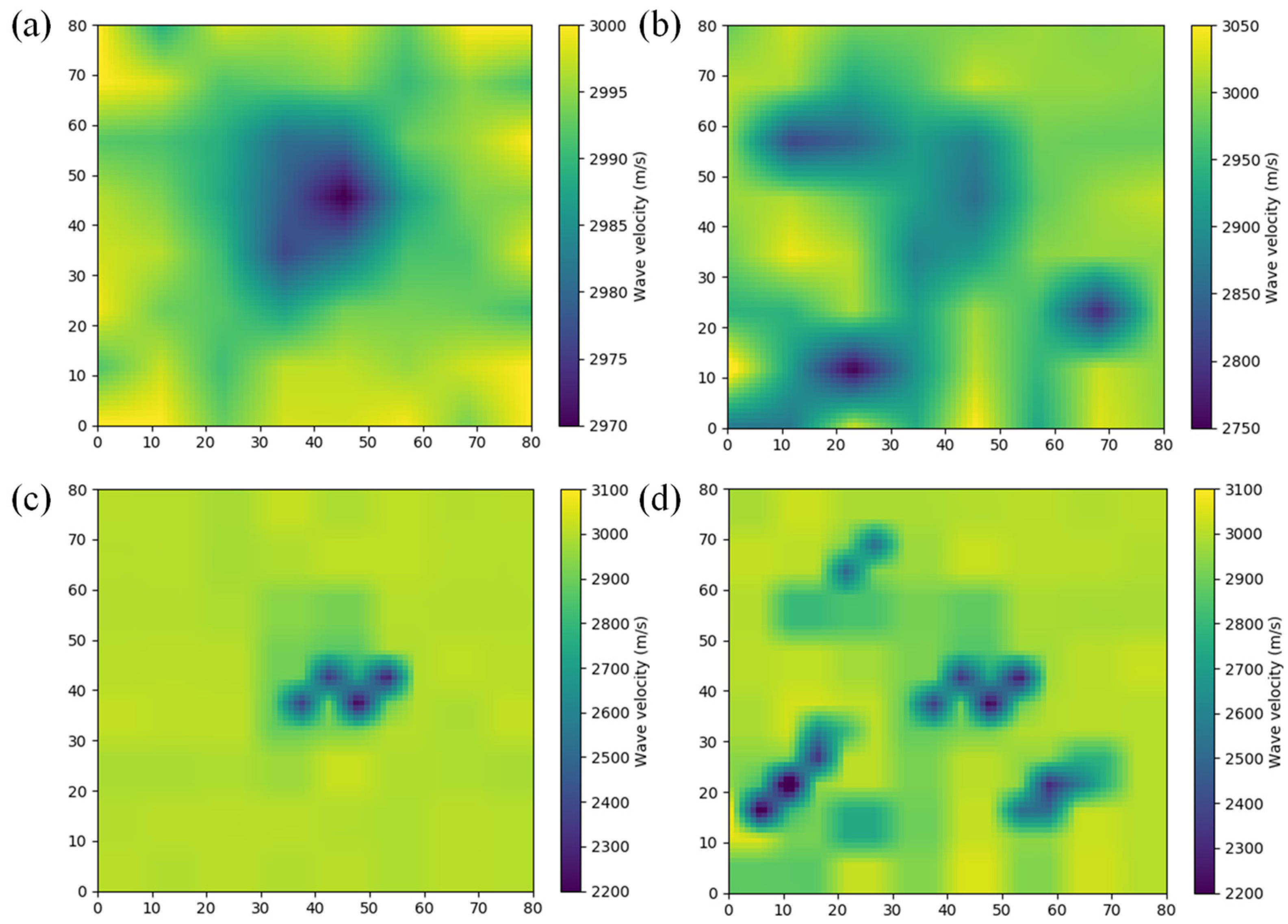

| Crack’s Number | Coordinate of the Crack Center Point | Crack Length (m) | Crack Inclination Angle (°) | |
|---|---|---|---|---|
| X | Y | |||
| 1 | 40 | 40 | 10 | 30 |
| 2 | 50 | 40 | 11 | 53 |
| 3 | 10 | 20 | 13 | 60 |
| 4 | 25 | 65 | 8 | 34 |
| 5 | 18 | 30 | 7 | 63 |
| 6 | 57 | 20 | 8 | 78 |
| Sensors’ Number | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
| X | 10 | 40 | 60 | 80 | 80 | 80 | 70 | 40 | 20 | 0 | 0 | 0 |
| Y | 0 | 0 | 0 | 10 | 40 | 60 | 80 | 80 | 80 | 70 | 40 | 20 |
| Sensor number | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
| Effective ray number | 9 | 9 | 9 | 6 | 6 | 6 | 3 | 3 | 3 | 0 | 0 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, J.; Liu, L.; Zhao, Y.; Zhuang, D.; Liu, Z.; Qin, X. An Improved Method for Calculating Wave Velocity Fields in Fractured Rock Based on Wave Propagation Probability. Mathematics 2024, 12, 2177. https://doi.org/10.3390/math12142177
Zhou J, Liu L, Zhao Y, Zhuang D, Liu Z, Qin X. An Improved Method for Calculating Wave Velocity Fields in Fractured Rock Based on Wave Propagation Probability. Mathematics. 2024; 12(14):2177. https://doi.org/10.3390/math12142177
Chicago/Turabian StyleZhou, Jing, Lang Liu, Yuan Zhao, Dengdeng Zhuang, Zhizhen Liu, and Xuebin Qin. 2024. "An Improved Method for Calculating Wave Velocity Fields in Fractured Rock Based on Wave Propagation Probability" Mathematics 12, no. 14: 2177. https://doi.org/10.3390/math12142177
APA StyleZhou, J., Liu, L., Zhao, Y., Zhuang, D., Liu, Z., & Qin, X. (2024). An Improved Method for Calculating Wave Velocity Fields in Fractured Rock Based on Wave Propagation Probability. Mathematics, 12(14), 2177. https://doi.org/10.3390/math12142177







