Analyzing Convergence in Sequences of Uncountable Iterated Function Systems—Fractals and Associated Fractal Measures †
Abstract
1. Introduction
2. Foundational Concepts
- (i)
- δ is a metric on ;
- (ii)
- is a compact metric space;
- (iii)
- The function is a contraction on .
- (b)
- is compact (hence, complete).
- (b)
- M is a contraction on ;
- (c)
- such that ( is called the Hutchinson measure or the fractal measure associated to the U.I.F.S. and to the function p).
- (d)
- .
3. Integration of Vector Functions with Lebesgue Measure and Markov-Type Operator: Introducing the Fractal Vector Measure
- (1)
- ;
- (2)
- ;
- (i)
- such that ;
- (ii)
- can be chosen such that (where for a vector measure ).
- (ii)
- Let be such that (as before) and be the fractal measure associated to the U.I.F.S. and to the function p. We know that (the attractor associated to the U.I.F.S.). For , fixed, we define . We will prove that and . Let be a partition of T with Borel sets. We have the following:We deduce that ; hence, .We define (I, the identity operator). Then, from , we have (or ) which means the following: . But, . So, has the continuous inverse . Thus, we obtain the following: and .
4. Analyzing Convergence Properties of Sequences of Uncountable Iterated Function Systems (U.I.F.S.)
- , for sufficiently large n (using Lemma 2);
- (using Lemma 1).
5. The Case of Weak Convergence
6. Examples
6.1. Continuity of Measures under Uncountable Iterated Function Systems
- (i)
- ;
- (ii)
- . If we consider a Borel set, we have . Let us consider (arbitrarily, fixed) and a sequence such that . We will denote and . We fix (arbitrarily). Let . We denote if n is sufficiently large. We deduce that ; hence, . Similarly, ; consequently, . If is a normalized measure, we will have the following: . Hence, the application is continuous.
6.2. Illustration of the Invariant Set Condition for Theorem 7
6.3. Example of Computing the Monge–Kantorovich Norm for a Dirac Measure in a Hilbert Space
6.4. Example of Norm Computation for Linear Operators in
6.5. Example of Variational Norm Computation for a Measure in
6.6. Analysis of Operators in Spaces
- (1°)
- We consider the case and we will prove that there exists such that . Indeed, if , we have
- (2°)
- Let us now consider .
- (i)
- We prove that for any :With the space being dense in , we will find a sequence such that and then a subsequence and a Borel set with the following properties:
- (a)
- ;
- (b)
- .
Taking (arbitrarily, fixed), we will find such that . Let us denote . We have. Thus, we have ; hence, . - (ii)
- Let us again consider .Then, and . Consequently, .
- (iii)
- We prove that is the topology of weak convergence of the operators. Let . We havesimilar to point (i), we find a sequence and a Borel set such that and . Let (arbitrarily, fixed). We will find such that . We haveHence, for and for any , , that is, in the topology of the weak convergence of the operators.
- (3°)
- If , in the same way as in one can prove that in the topology of the weak convergence of the operators.
7. Conclusions
- (a)
- Unlike the traditional approach, which employs a finite set of contractions, we have explored the construction of iterated function systems using an uncountable set of contractions, broadening the scope of applicability.
- (b)
- Our investigation introduces a novel perspective on fractal measures by considering them as vector measures rather than the classical positive normalized measures. This expanded understanding opens up new avenues for analysis and application.
- (c)
- Furthermore, we have delved into the study of sequences of (uncountable) iterated function systems, examining various convergence properties. This adds depth to our understanding of the behavior and limitations of such systems.
- (1°)
- We will delve into the problem of convergence concerning the sequences of attractors and fractal vector measures within a broader framework. This inquiry aims to provide insights into the stability and long-term behavior of these systems under diverse conditions.
- (2°)
- Additionally, we will explore the application of our findings to the theory of differential equations. By bridging the gap between fractal geometry and differential equations, we aim to uncover new perspectives and potential applications in areas such as dynamical systems and mathematical modeling.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
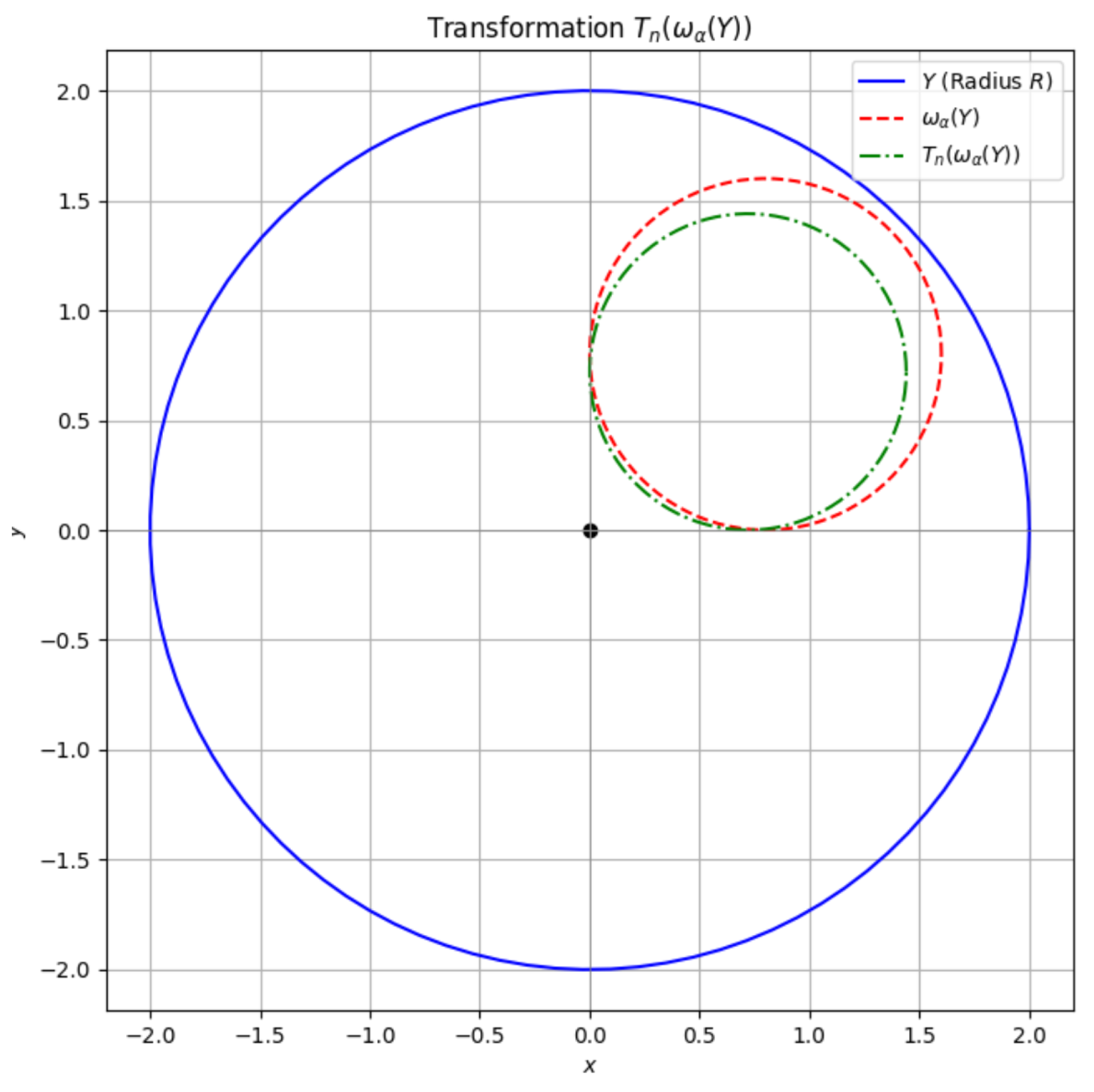
- Plot 1: The Ball Y and Mapping [language=Python]
- Plot 2: Applying to [language=Python]
References
- Mierlus-Mazilu, I.; Nită, L. Sequences of Uncountable Iterated Function Systems: The Convergence of the Sequences of Fractals and Fractal Measures Associated. In Mathematical Methods for Engineering Applications (Springer Proceedings in Mathematics & Statistics); Springer: Cham, Switzerland, 2024; Volume 439, pp. 43–52. [Google Scholar]
- Chițescu, I. Spații de Funcții; Ed. Științifică și Enciclopedică: Bucharest, Romania, 1983. [Google Scholar]
- Chițescu, I.; Ioana, L.; Miculescu, R.; Niță, L. Sesquilinear uniform vector integral. Proc. Indian Acad. Sci. (Math. Sci.) 2015, 125, 229–236. [Google Scholar] [CrossRef]
- Chițescu, I.; Ioana, L.; Miculescu, R.; Niță, L. Monge–Kantorovich norms on spaces of vector measures. Results Math. 2016, 70, 349–371. [Google Scholar] [CrossRef]
- Cobzas, S.; Miculescu, R.; Nicolae, A. Lipschitz Functions; Lecture Notes in Mathematics; Springer International Publishing: New York, NY, USA, 2019; pp. 365–556. [Google Scholar]
- D’Aniello, E.; Steele, T.H. Attractors for classes of iterated function systems. Eur. J. Math. 2019, 5, 116–137. [Google Scholar] [CrossRef]
- Mendivil, F.; Vrscay, E.R. Self-affine vector measures and Vector Calculus on Fractals. Fractals Multimed. 2002, 132, 137–155. [Google Scholar]
- Mierluș-Mazilu, I.; Niță, L. About Some Monge–Kantovorich Type Norm and Their Applications to the Theory of Fractals. Mathematics 2022, 10, 4825. [Google Scholar] [CrossRef]
- Mihail, A. Recurrent iterated function systems. Rev. Roumaine Math. Pures Appl. 2008, 53, 43–53. [Google Scholar]
- Sirețchi, G. Spatii Concrete in Analiza Funcționala; Centrul de Multiplicare al Universitatii Bucuresti: Bucharest, Romania, 1982. (In Romanian) [Google Scholar]
- Igbida, N.; Mazón, J.M.; Rossi, J.D.; Toledo, J. A Monge–Kantorovich mass transport problem for a discrete distance. J. Funct. Anal. 2011, 260, 494–3534. [Google Scholar] [CrossRef]
- La Torre, D.; Maki, E.; Mendivil, F.; Vrscay, E.R. Iterated function systems with place-dependent probabilities and the inverse problem of measure approximation using moments. Fractals 2018, 26, 1850076. [Google Scholar] [CrossRef]
- Mendivil, F. Computing the Monge–Kantorovich distance. Comput. Appl. Math. 2017, 36, 1389–1402. [Google Scholar] [CrossRef]
- Rachev, S.T.; Klebanov, L.B.; Stoyanov, S.V.; Fabozzi, F.J. Monge–Kantorovich Mass Transference Problem, Minimal Distances and Minimal Norms. In The Methods of Distances in the Theory of Probability and Statistics; Springer: New York, NY, USA, 2013. [Google Scholar]
- Strobin, F. On the existence of the Hutchinson measure for generalized iterated function systems. Qual. Theory Dyn. Syst. 2020, 19, 1–21. [Google Scholar] [CrossRef]
- Secelean, N.A. Măsură și Fractali; Ed. Universității Lucian Blaga: Sibiu, Romania, 2002. [Google Scholar]
- Niță, L. Fractal Vector Measures in the case of an Uncountable Iterated Function System. RJM-CS 2015, 5, 151–163. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mierluș–Mazilu, I.; Niță, L. Analyzing Convergence in Sequences of Uncountable Iterated Function Systems—Fractals and Associated Fractal Measures. Mathematics 2024, 12, 2106. https://doi.org/10.3390/math12132106
Mierluș–Mazilu I, Niță L. Analyzing Convergence in Sequences of Uncountable Iterated Function Systems—Fractals and Associated Fractal Measures. Mathematics. 2024; 12(13):2106. https://doi.org/10.3390/math12132106
Chicago/Turabian StyleMierluș–Mazilu, Ion, and Lucian Niță. 2024. "Analyzing Convergence in Sequences of Uncountable Iterated Function Systems—Fractals and Associated Fractal Measures" Mathematics 12, no. 13: 2106. https://doi.org/10.3390/math12132106
APA StyleMierluș–Mazilu, I., & Niță, L. (2024). Analyzing Convergence in Sequences of Uncountable Iterated Function Systems—Fractals and Associated Fractal Measures. Mathematics, 12(13), 2106. https://doi.org/10.3390/math12132106






