Quantitative Assessment of Bed-Separation Dynamic Development Caused by Inclined Coal Seam Longwall Mining
Abstract
1. Introduction
2. Materials and Methods
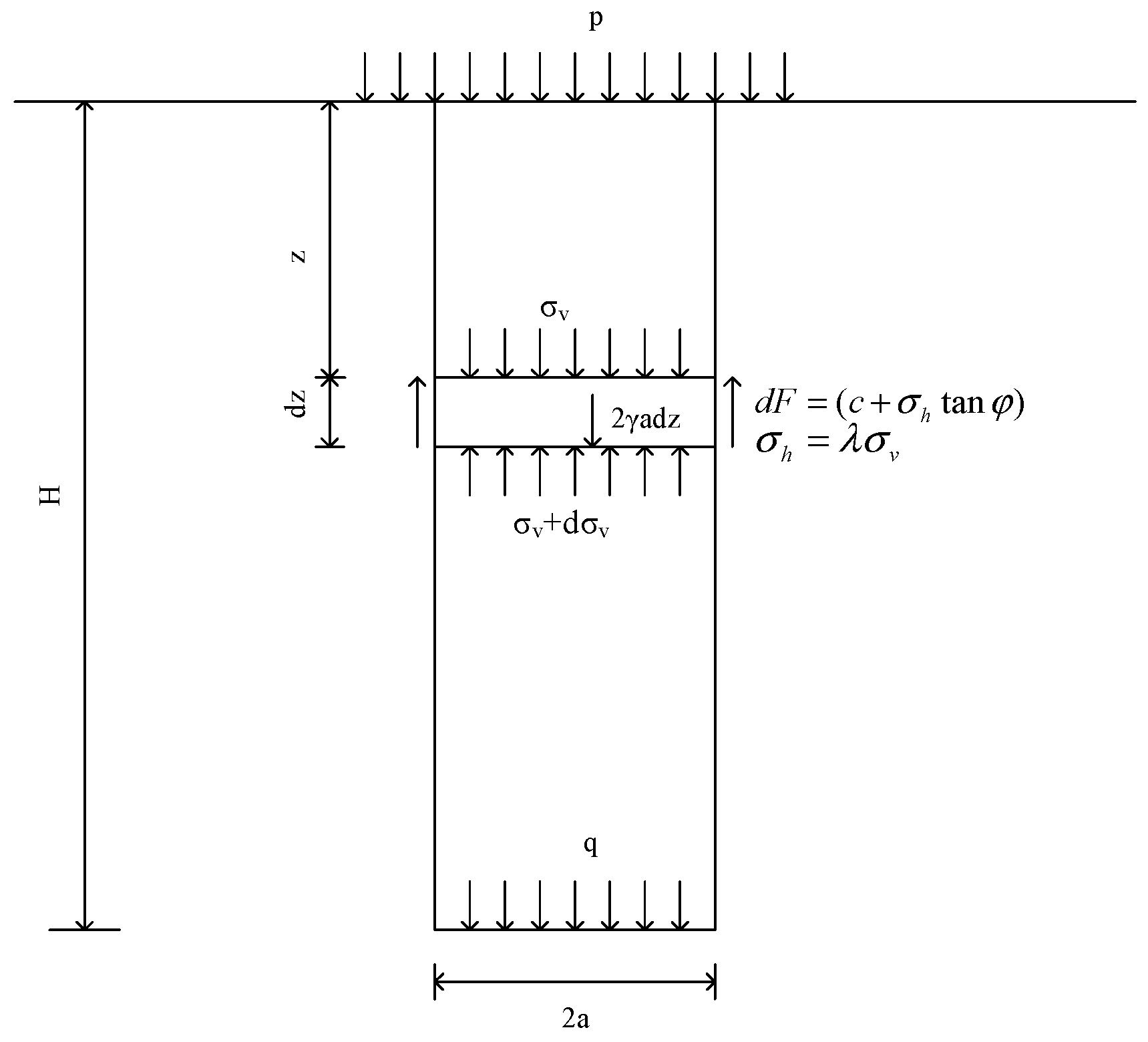
2.1. Calculation of Overlying Load Based on Stress Transfer
2.2. Calculation of Overlying Load Based on Stress Transfer
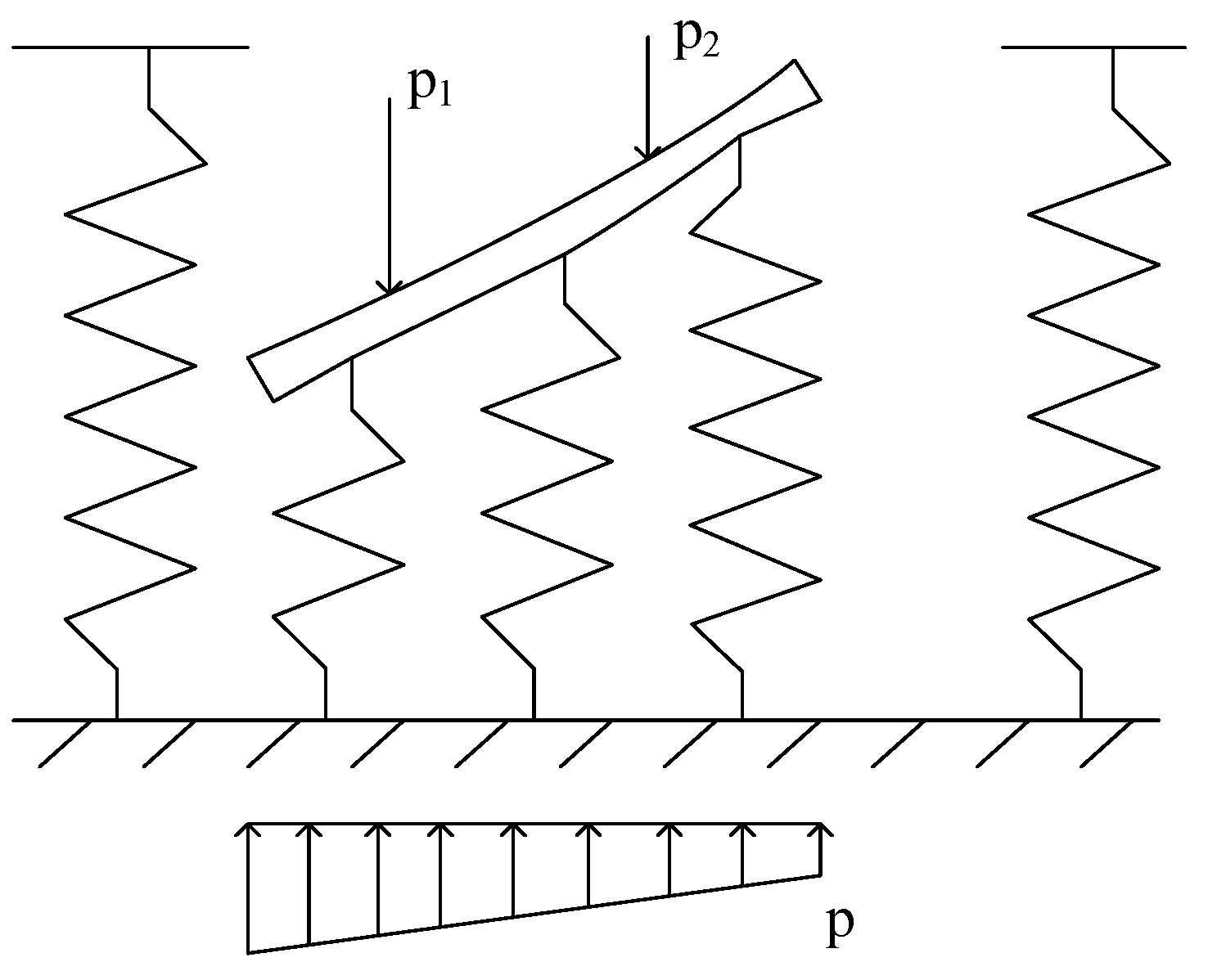
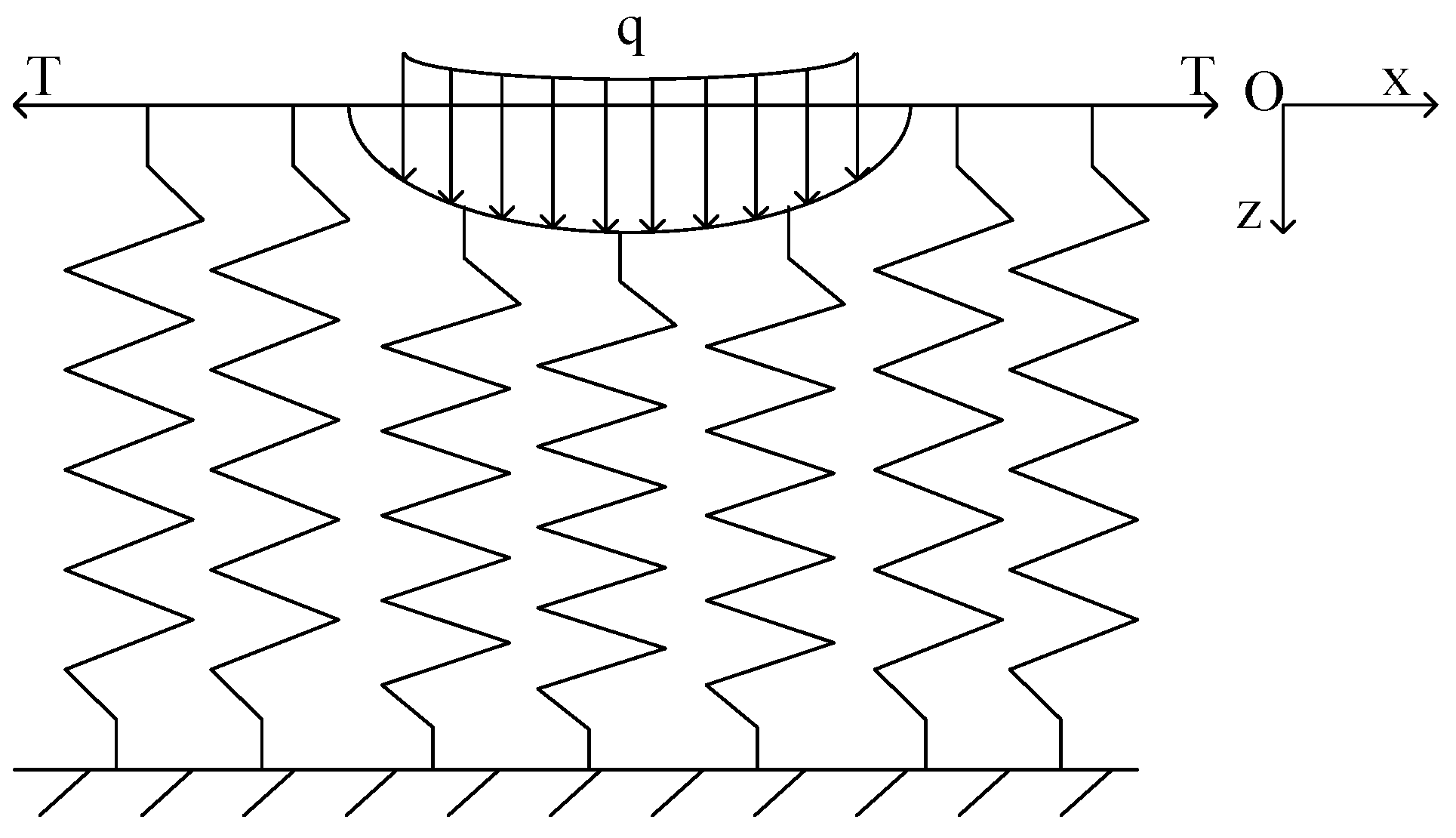
2.3. Energy Method for Solving Additional Lateral Loads and Mid-Plane Stresses for Thin Plate Deflection Values
2.3.1. Establishment of Thin Plate Model
2.3.2. Establishment of Thin Plate Model
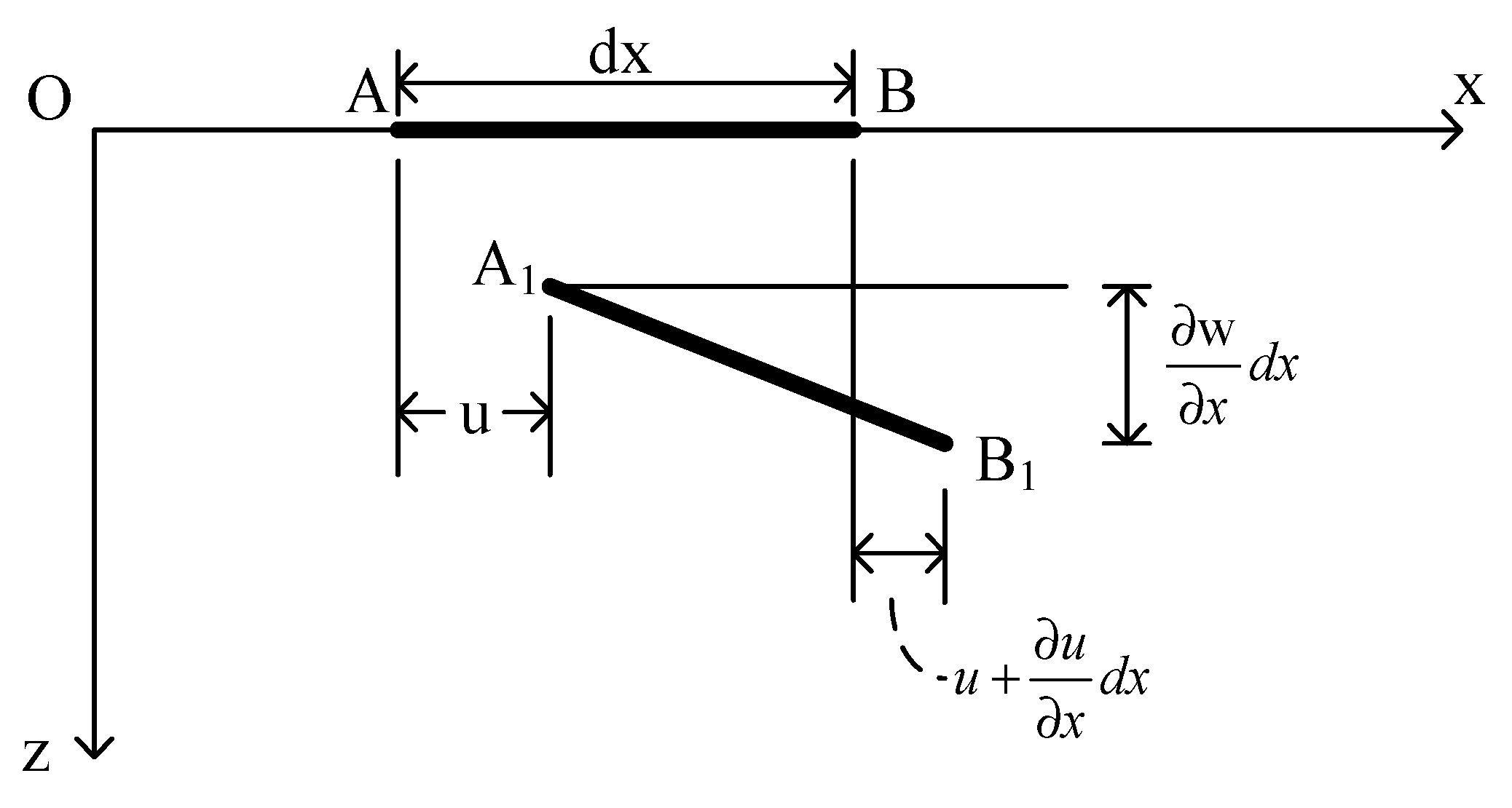
2.3.3. Strain Energy Generated by the Bending of the Plate by the Action of the Mid-Plane Stresses
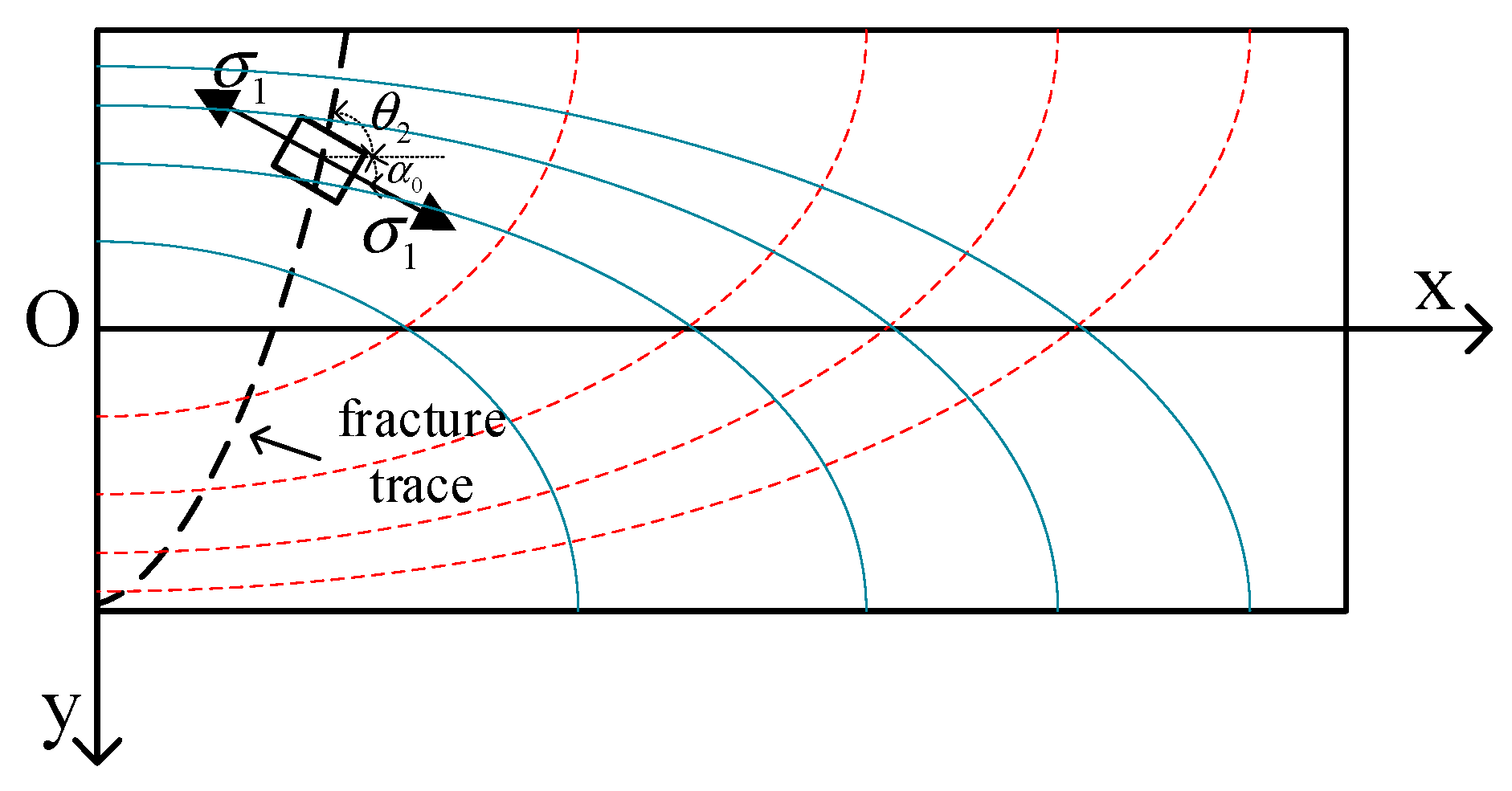
2.4. Solving the Key Strata Breakage Distance and Angle Based on the First Strength Theory
3. Dynamic Development of Key Strata Deflection
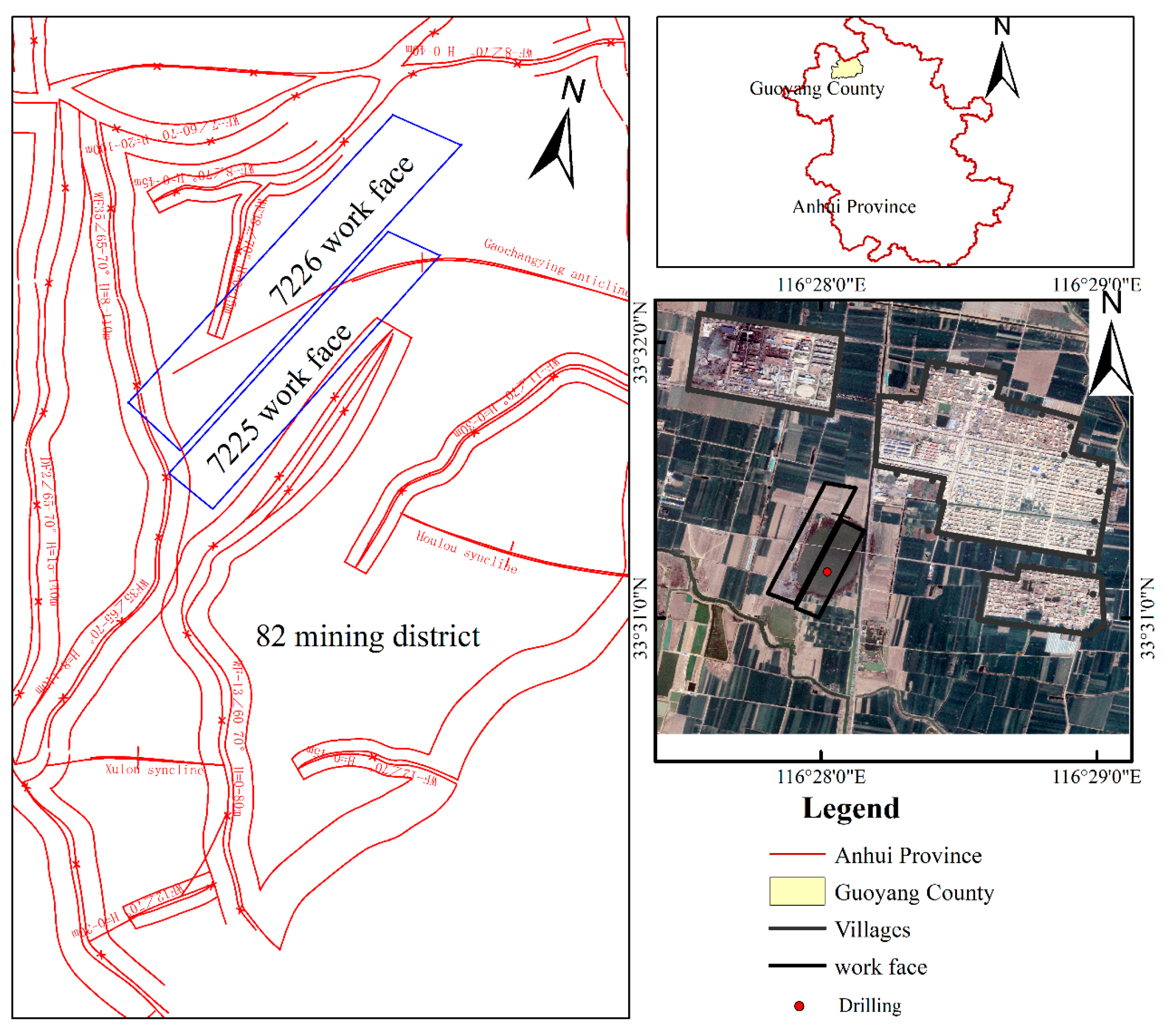
3.1. Engineering Geology Background
3.2. Deflection Function Based on Minimum Potential Energy Inclined Key Strata Thin Plate
3.2.1. Four-Sided Solid-Supported Inclined Key Strata Plate Deflection before Initial Fracture
3.2.2. Three-Side Solid-Supported and One-Side Simple-Supported Inclined Key Strata Plate Deflection before Periodic Fracture
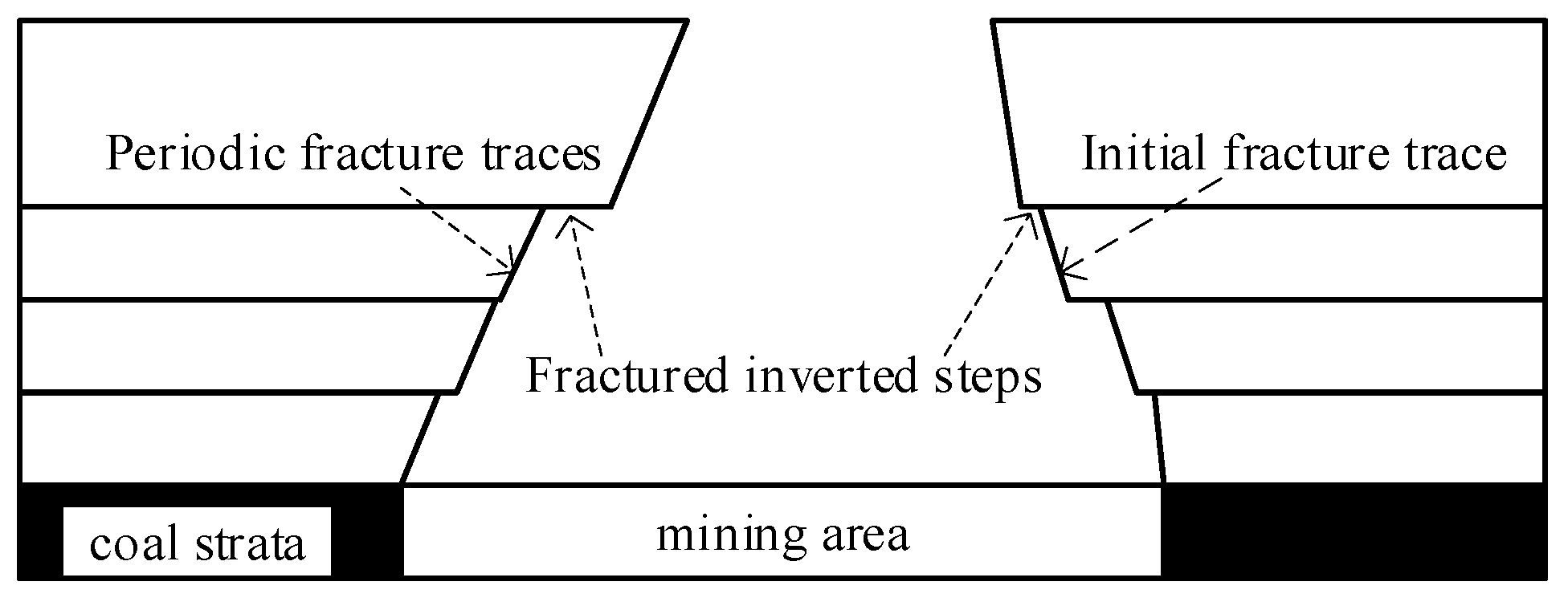
3.3. Calculation of Inclined Key Strata Breaking Distance and Fracture Trace Angle
4. Large-Deflection Inclined Thin-Plate Model to Calculate the Dynamic Development of Key Strata Deflections and Bed-Separation
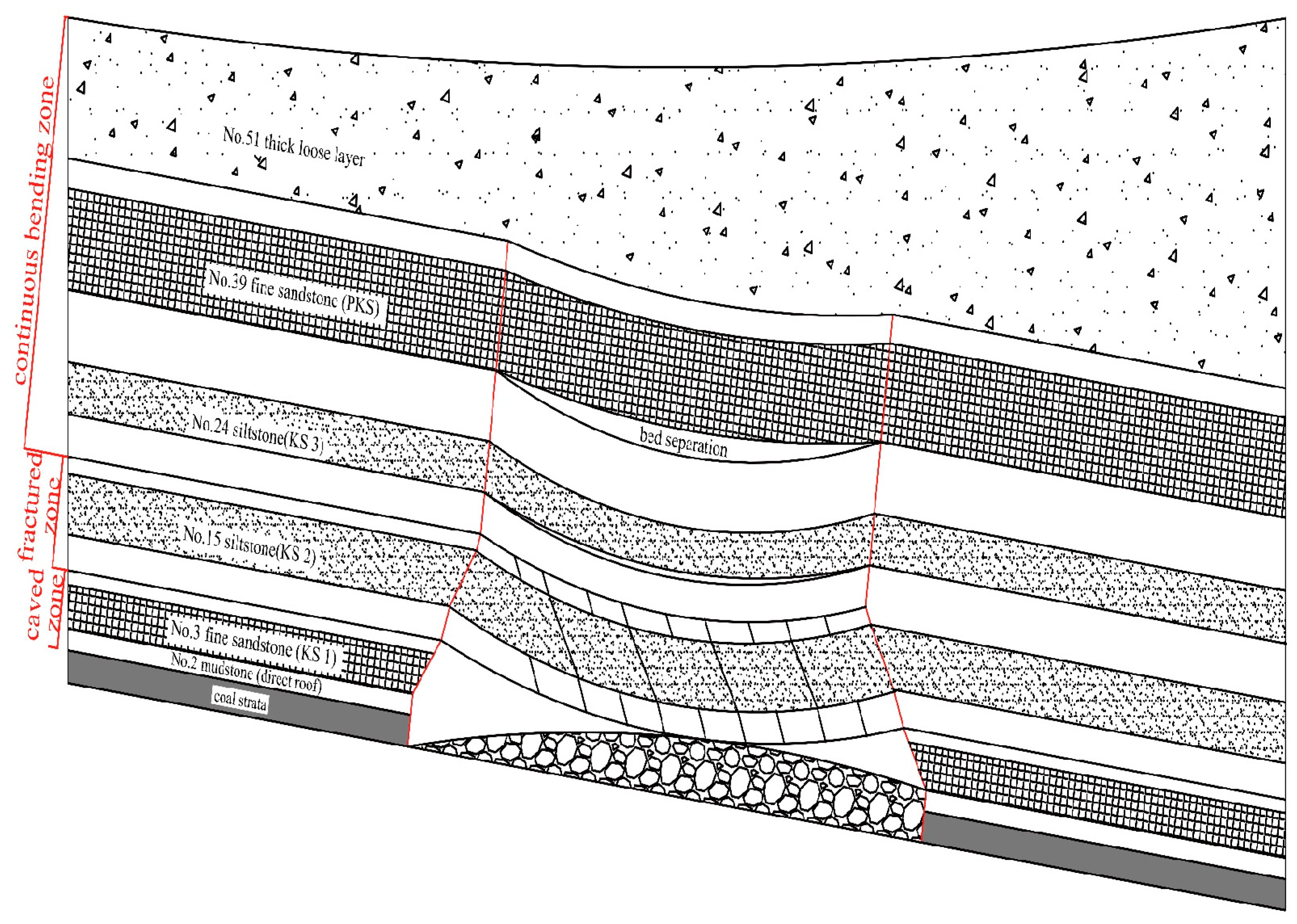
4.1. Breakage of the Main Roof and the “Vertical Three Zones” Height Development
4.2. Deflection of the Principal Key Strata and the Dynamics of the Height and Volume of Bed-Separation Development
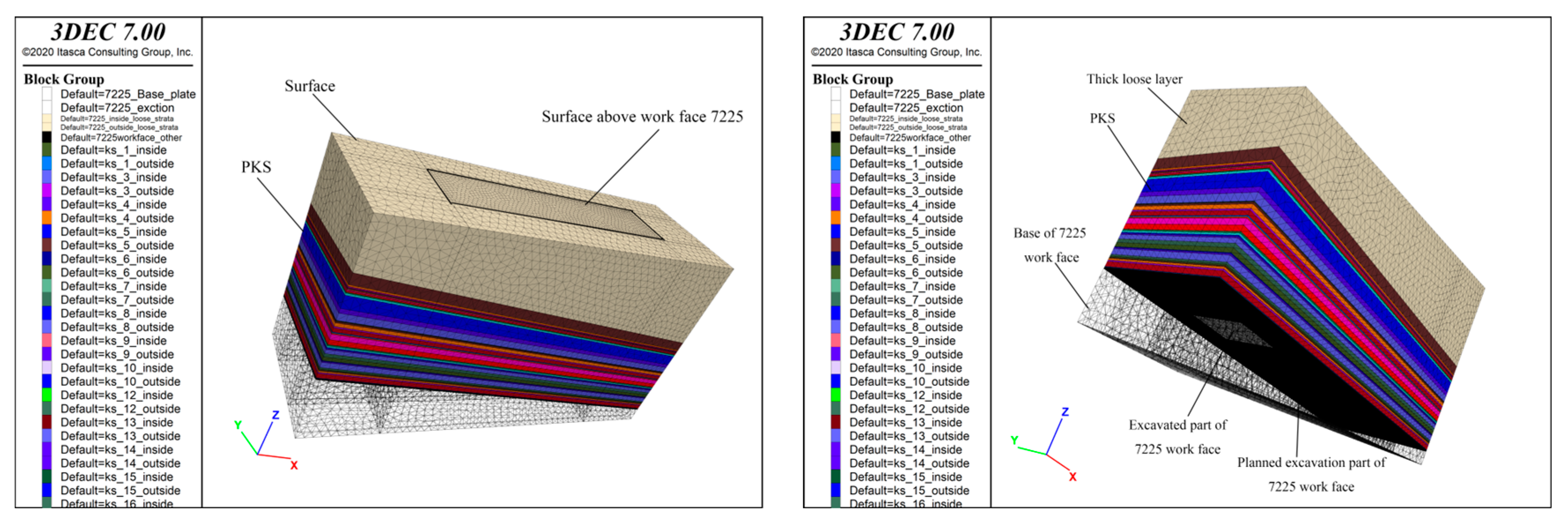
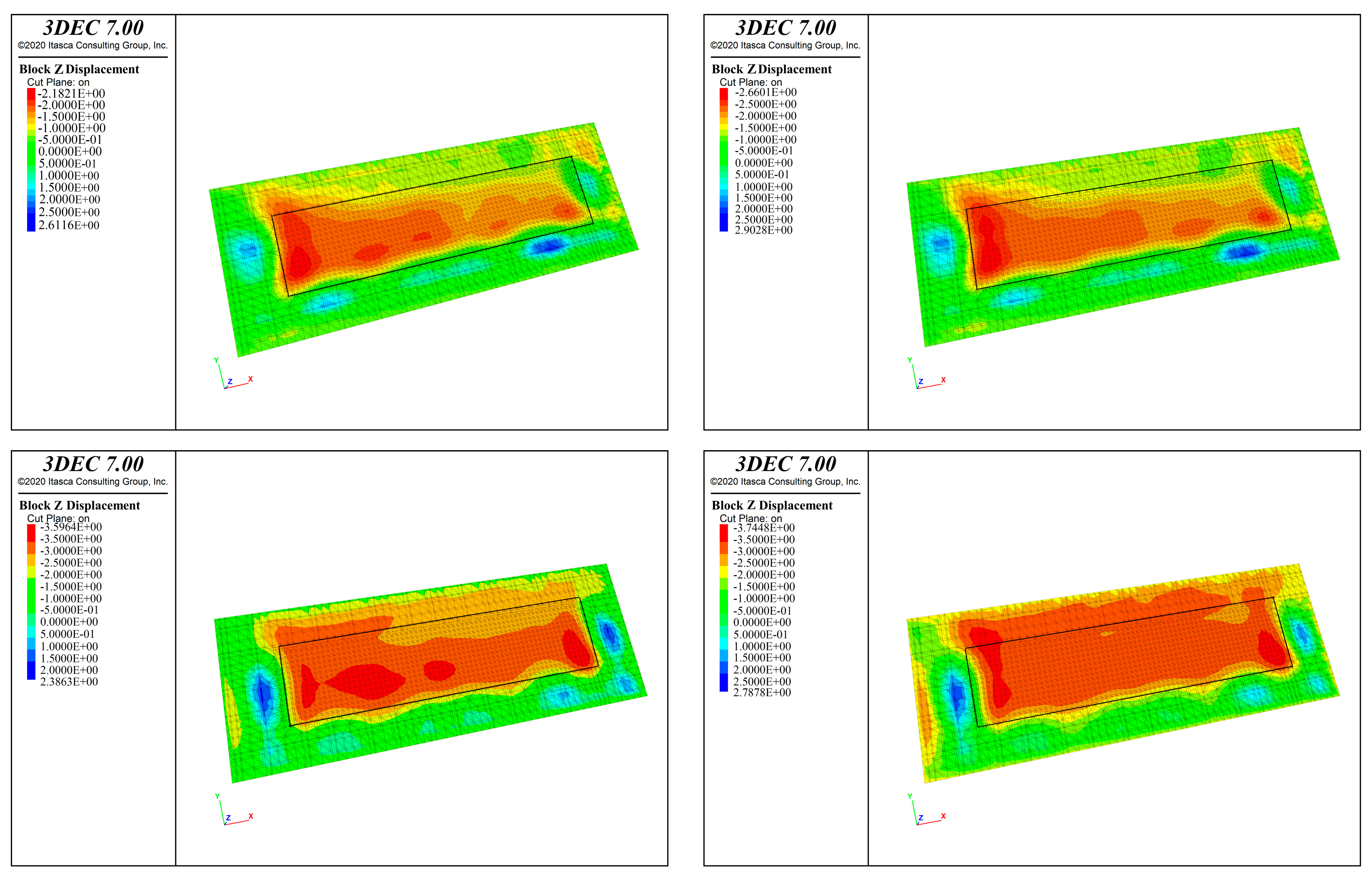
4.3. Verification of the Principal Key Strata Deflection and Lower Delamination Accuracy Based on 3DEC
5. Impact of Bed-Separation under the Principal Key Strata
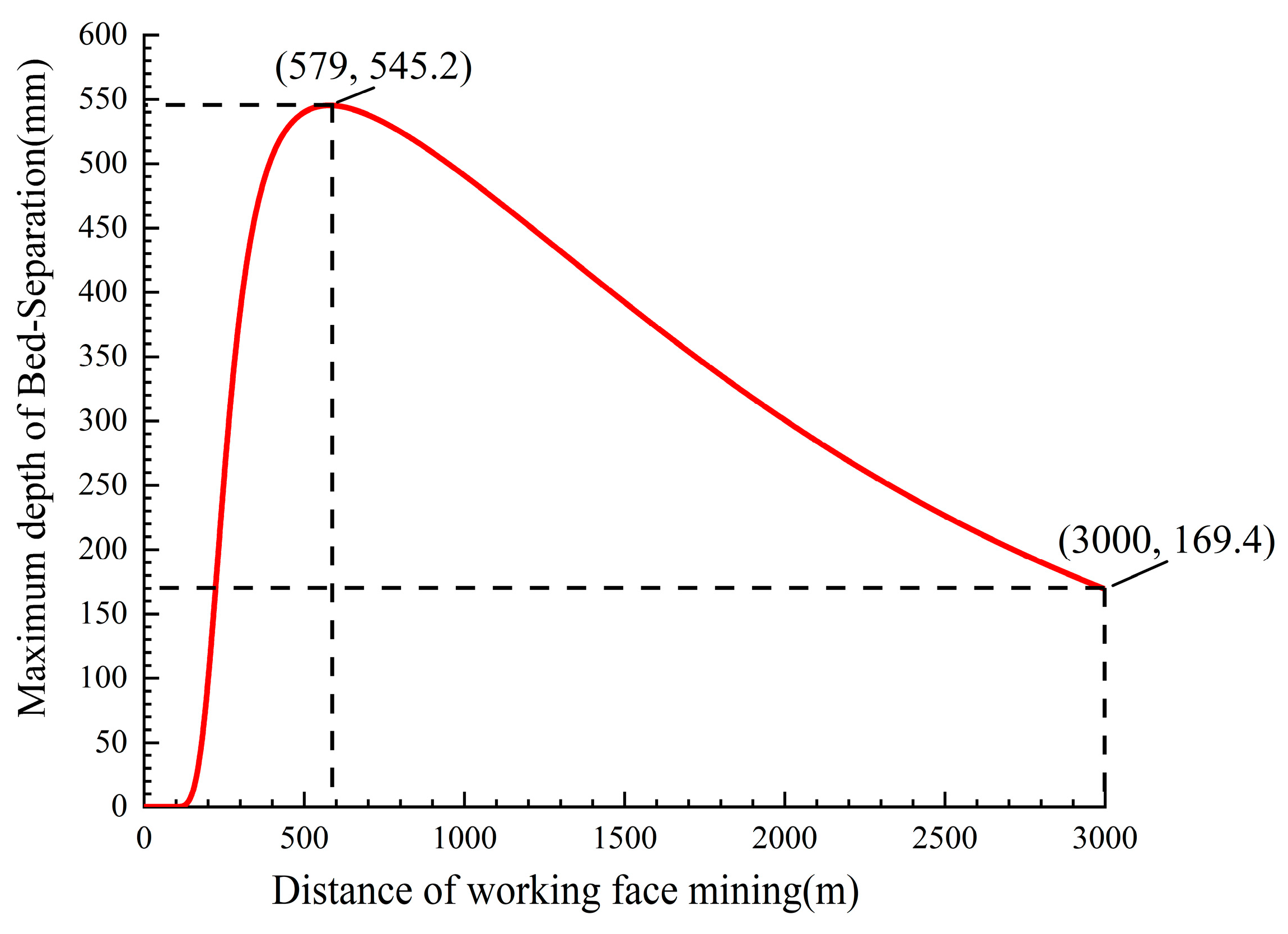
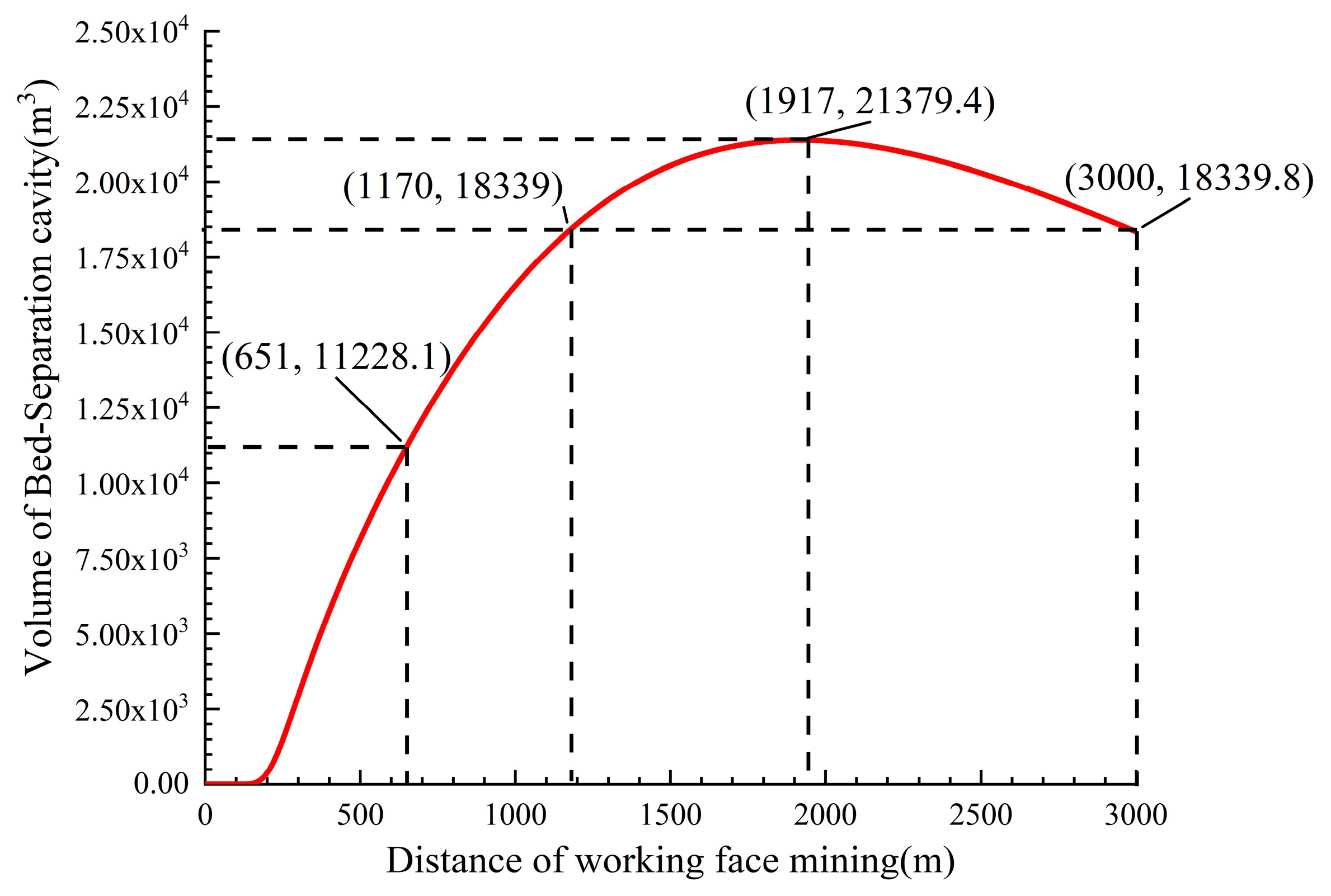
5.1. Influence of Work Face Mining Length on the Development Height and Cavity Volume of the Bed-Separation
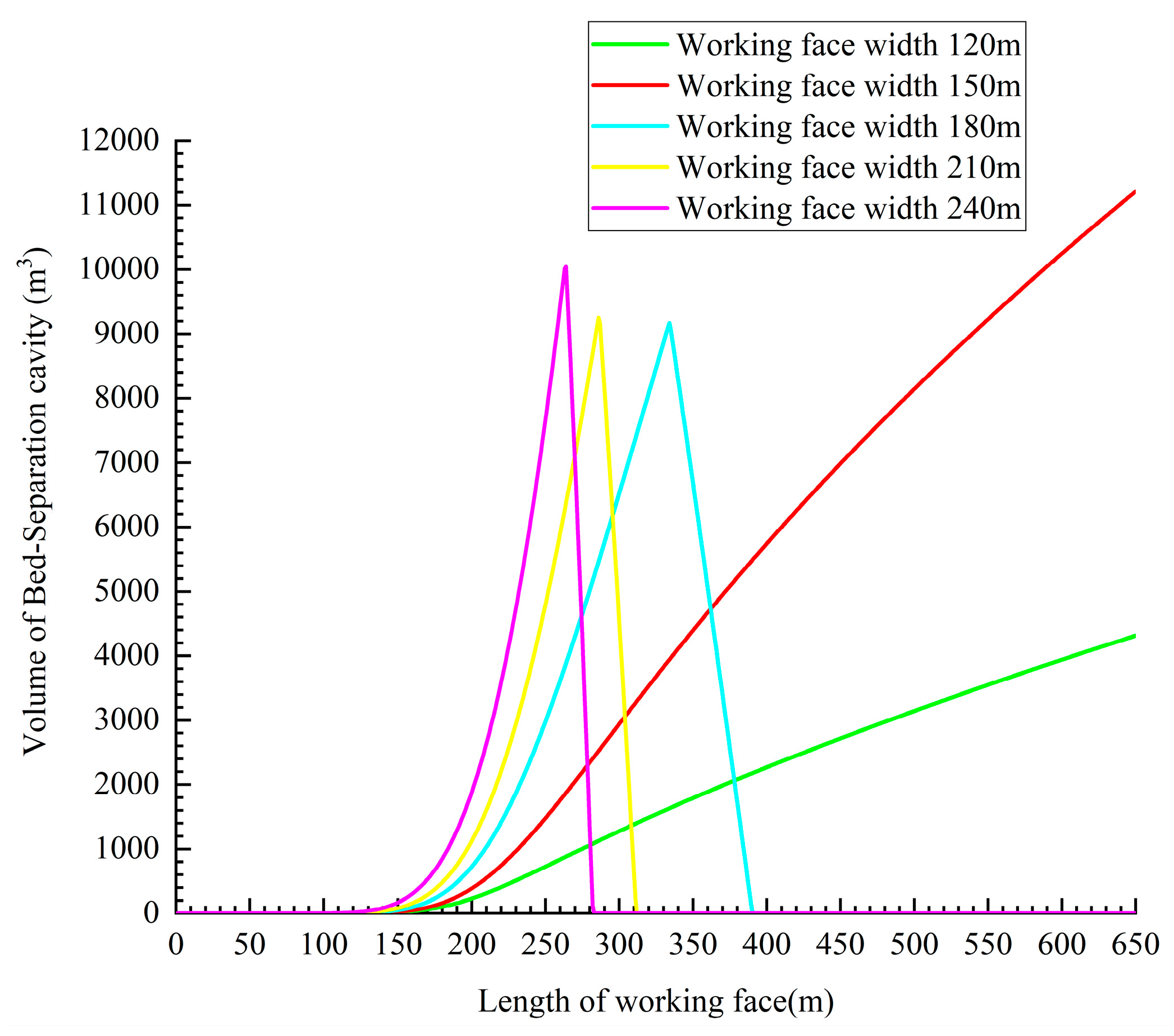
5.2. Influence of Working Face Width on the Development Height and Cavity Volume of the Bed-Separation
6. Conclusions
- Based on the drilling data obtained above the 7225 working face, each rock stratum’s physical and mechanical properties from the coal seam to the ground were determined. The thick, loose layer overlying the 7225 working face was determined to contain four key strata, numbered 3, 15, 24, and 39. It was also determined that the main roof plate was No.3, 1.47 m above the 7225 work face, and the PKS was No.39, 171.99 m above the 7225 work face;
- A model of a large-deflection inclined thin plate with one unsupported side and three supported sides was created by mining the 7225 inclined face. Then, the initial breakage distance of the main roof plate was 33 m, and the initial breakage angle was 61.2°; the periodic breakage distance was 21 m, and the periodic breakage angle was 55.4°. The distribution range of the “Vertical Three Zones” from the 7225 face to the ground surface included the height of the caved zone of 38.07 m, the height of the fissured zone of 41.13 m, the height of the bending zone of 187.56 m excluding the thick loose layer. It also analyses the relationship between the spatial location of each key strata’s starting and ending deformation points within the “Vertical Three Zones” and the mining activities’ distance;
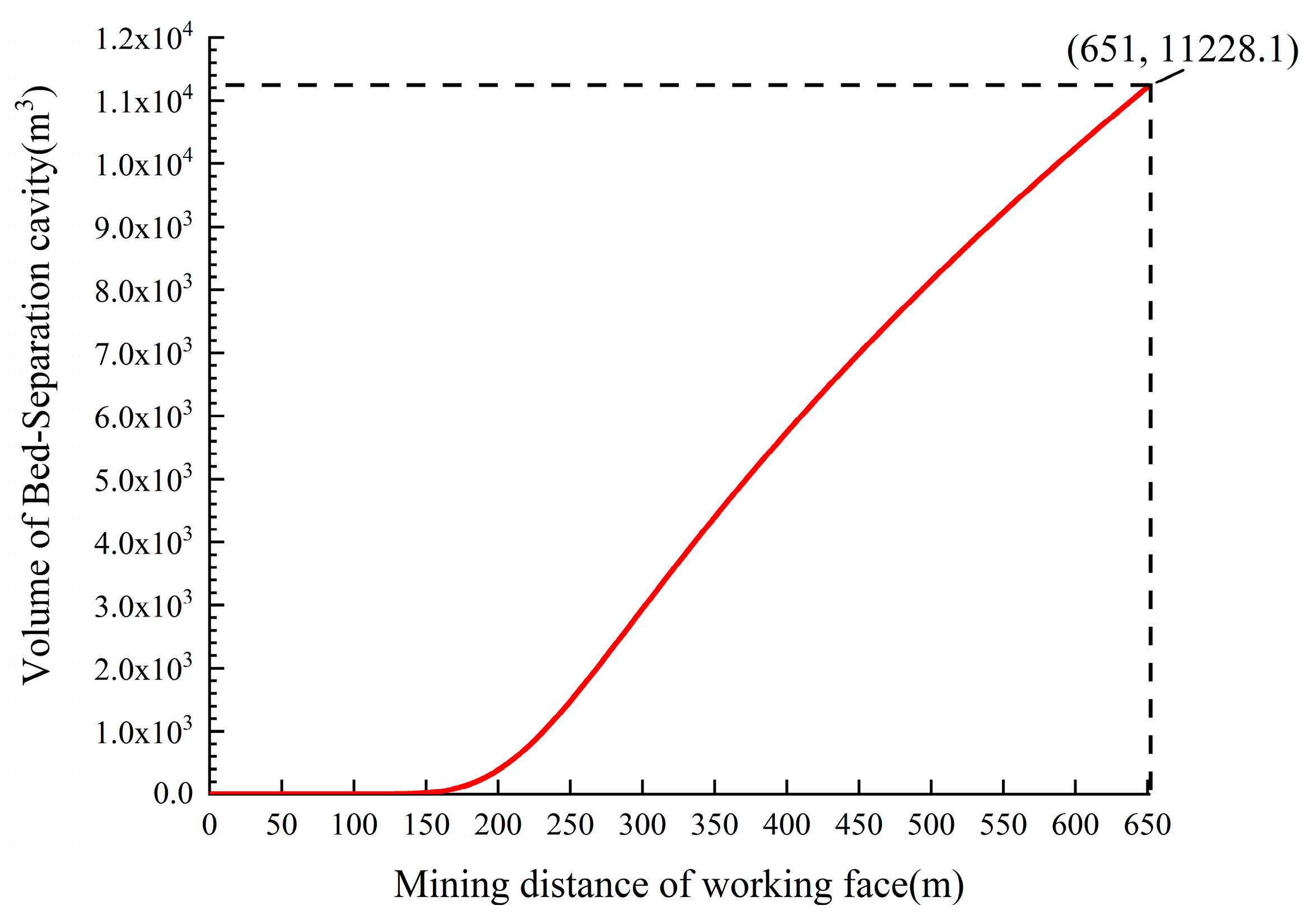
- The large-deflection inclined thin plate model was applied to the 7225 inclined working face mining process. The PKS deflection value develops from 0 mm to 2714 mm with the mining of the working face, and the maximum value of the space volume is 56,485 m3, verified by 3DEC numerical simulation. The dynamic development of bed-separation below the PKS within the overburden strata, the maximum development height is 545.2 mm, and the maximum value of Bed-separation cavity volume is 11,228.1 m3. Finally, the dynamic development of bed-separation height and cavity under the working face’s different mining length and width conditions is discussed. As the length of the working face increases, the Bed-separation height increases and then decreases, and the maximum value of the development height is 545.2 mm, corresponding to the mining distance of 579 m. The volume of the Bed-separation cavity increases until it starts to decrease after 1917 m. The Bed-separation height and cavity volume show an increasing trend when the working face width increases within 150 m. After the working face width exceeds 180 m, the Bed-separation cavity develops and closes rapidly;
- The large-deflection inclined thin plate model proposed in this paper can effectively explore the dynamic development of PKS deflection and Bed-separation induced by Longwall mining inclined workings overlying thick loose layers. Of course, the large-deflection inclined thin plate model has limitations in its application to the analysis of overlying stratigraphic deflection caused by open-pit mining and partially sharp inclined working face mining. In the future, the model will be applied more to the dynamic assessment of bed-separation development caused by horizontal or inclined coal seam mining, and it will provide a theoretical basis for the rational use of Bed-separation cavities, such as grouting backfill.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| List of Symbols | |||
| U | The strain energy of thin plate bending | Shear force on the differential unit | |
| U1 | The strain energy caused by the Elongation of the mid-plane | The load on the differential unit | |
| U2 | The additional strain energy caused by the mid-plane bending strain | k | the foundation reaction coefficient |
| Key strata principal tensile stress | N | A vector sum of the gravity along the inclined plate | |
| Key strata principal compressive stresses | MX | Thin plate bending moment in the x-axis | |
| The angle between the principal tensile stress and the horizontal plane | MY | Thin plate bending moment in the y-axis | |
| The angle between the fracture trace and the horizontal plane | MXY | Thin plate torque | |
| W | The work by the external force on the thin plate | QX | Lateral vertical shear in the x-axis |
| The work by the lateral load and the elastic foundation combined force | QY | Lateral vertical shear of the y-axis | |
| The work by the mid-plane force along the mid-plane | w | Thin plate deflection | |
| The minimum potential energy | Lateral load at the junction point between the uphill direction and the cut line | ||
| Key strata deflection coefficient | Lateral load at the junction point between the downhill direction and the finishing line | ||
| Unit length mid-plane tension in the x-axis | Key strata ultimate tensile stress | ||
| Unit length mid-plane tension in the y-axis | The angle between the key strata initial fracture trace and the horizontal plane | ||
| Unit length mid-plane shear | The angle between the key strata periodic fracture trace and the horizontal plane | ||
| ux | Small displacements of mid-plane in the x-axis | The average angle of the initial fracture trace between the two adjacent key strata | |
| uy | Small displacements of mid-plane in the y-axis | The average angle of periodic fracture trace between the two adjacent key strata | |
| Total unit elongation in the x-axis | The height of the caved zone | ||
| Total unit elongation in the y-axis | m | Coal seam mining height | |
| Total unit shear strains | k′ | Rock expansion coefficient | |
| Key strata tensile stress in the x-axis | The height of the fractured zone | ||
| Key strata tensile stress in the y-axis | The mining distance of the work face | ||
| Key strata shear stress | Continuous bending zone key strata starting deflection position | ||
| D | Bending stiffness of the thin plate | Continuous bending zone key strata ending deflection position | |
| v | Poisson’s ratio of thin plate | The deflection curve of the Bed-separation | |
| a | Length of plate | The volume of the Bed-separation cavity | |
| b | Width of plate | ||
References
- Hou, D.F.; Li, D.H.; Xu, G.S.; Zhang, Y.B. Superposition model for analyzing the dynamic ground subsidence in mining area of thick loose layer. Int. J. Min. Sci. Technol. 2018, 28, 663–668. [Google Scholar] [CrossRef]
- Gao, X.C.; Wang, L.Q.; Wang, Q.; Hu, X.Y.; Wang, Y.C.; Zhang, Y.F. Stability Analysis and the Random Response of Anti-Sliding Pile for Erdaogou Landslide Considering Spatial Variability. Mathematics 2023, 11, 16. [Google Scholar] [CrossRef]
- Wang, F.; Xu, J.L.; Xie, J.L. Effects of arch structure in unconsolidated layers on fracture and failure of overlying strata. Int. J. Rock Mech. Min. Sci. 2019, 114, 141–152. [Google Scholar] [CrossRef]
- Li, Z.; Xu, J.L.; Ju, J.F.; Zhu, W.B.; Xu, J.M. The effects of the rotational speed of voussoir beam structures formed by key strata on the ground pressure of stopes. Int. J. Rock Mech. Min. Sci. 2018, 108, 67–79. [Google Scholar] [CrossRef]
- Zhu, W.B.; Xu, J.M.; Xu, J.L.; Chen, D.Y.; Shi, J.X. Pier-column backfill mining technology for controlling surface subsidence. Int. J. Rock Mech. Min. Sci. 2017, 96, 58–65. [Google Scholar] [CrossRef]
- Qu, Q.D.; Xu, J.L.; Wu, R.L.; Qin, W.; Hu, G.Z. Three-zone characterisation of coupled strata and gas behaviour in multi-seam mining. Int. J. Rock Mech. Min. Sci. 2015, 78, 91–98. [Google Scholar] [CrossRef]
- Ma, H.W.; Sui, W.H.; Ni, J.M. Environmentally sustainable mining: A case study on surface subsidence control of grouting into overburden. Environ. Earth Sci. 2019, 78, 15. [Google Scholar] [CrossRef]
- Xu, J.M.; Juan, P.; Zhu, W.B. Movement Laws of the Overlying Strata at the Working Face Ends and Their Effects on the Surface Deformation. Minerals 2022, 12, 19. [Google Scholar] [CrossRef]
- Jin, L.X.; Liu, P.T.; Yao, W.B.; Wei, J.J. A Comprehensive Evaluation of Resilience in Abandoned Open-Pit Mine Slopes Based on a Two-Dimensional Cloud Model with Combination Weighting. Mathematics 2024, 12, 26. [Google Scholar] [CrossRef]
- Wu, Q.L.; Wu, Q.S.; Xue, Y.C.; Kong, P.; Gong, B. Analysis of Overlying Strata Movement and Disaster-Causing Effects of Coal Mining Face under the Action of Hard Thick Magmatic Rock. Processes 2018, 6, 18. [Google Scholar] [CrossRef]
- Park, T.; Lee, S.Y.; Seo, J.W.; Voyladjis, G.Z. Structural dynamic behavior of skew sandwich plates with laminated composite faces. Compos. Pt. B-Eng. 2008, 39, 316–326. [Google Scholar] [CrossRef]
- Boulanger, R.W.; Khosravi, M.; Khosravi, A.; Wilson, D.W. Remediation of liquefaction effects for an embankment using soil-cement walls: Centrifuge and numerical modeling. Soil Dyn. Earthq. Eng. 2018, 114, 38–50. [Google Scholar] [CrossRef]
- Park, T.; Lee, S.Y.; Voyiadjis, G.Z. Finite element vibration analysis of composite skew laminates containing delaminations around quadrilateral cutouts. Compos. Pt. B-Eng. 2009, 40, 225–236. [Google Scholar] [CrossRef]
- Amornfa, K.; Quang, H.; Tuan, T.V.V. Effect of groundwater level change on piled raft foundation in Ho Chi Minh City, Viet Nam using 3D-FEM. Geomech. Eng. 2023, 32, 387–396. [Google Scholar] [CrossRef]
- Avcar, M.; Hadji, L.; Akan, R. The influence of Winkler-Pasternak elastic foundations on the natural frequencies of imperfect functionally graded sandwich beams. Geomech. Eng. 2022, 31, 99–112. [Google Scholar] [CrossRef]
- Bashiri, A.H.; Akbas, S.D.; Abdelrahman, A.A.; Assie, A.; Eltaher, M.A.; Mohamed, E.F. Vibration of multilayered functionally graded deep beams under thermal load. Geomech. Eng. 2021, 24, 545–557. [Google Scholar] [CrossRef]
- Yu, M.L.; Zuo, J.P.; Sun, Y.J.; Mi, C.N.; Li, Z.D. Investigation on fracture models and ground pressure distribution of thick hard rock strata including weak interlayer. Int. J. Min. Sci. Technol. 2022, 32, 137–153. [Google Scholar] [CrossRef]
- Lee, S.Y.; Wooh, S.C.; Yhim, S.S. Dynamic behavior of folded composite plates analyzed by the third order plate theory. Int. J. Solids Struct. 2004, 41, 1879–1892. [Google Scholar] [CrossRef]
- Gravanis, E.; Sarris, E. A working model for estimating CO2-induced uplift of cap rocks under different flow regimes in CO2 sequestration. Geomech. Energy Environ. 2023, 33, 15. [Google Scholar] [CrossRef]
- Mi, C.N.; Zuo, J.P.; Sun, Y.J.; Zhao, S.K. Investigation on Rockburst Mechanism Due to Inclined Coal Seam Combined Mining and its Control by Reducing Stress Concentration. Nat. Resour. Res. 2022, 31, 3341–3364. [Google Scholar] [CrossRef]
- Sun, Y.J.; Zuo, J.P.; Karakus, M.; Liu, L.; Zhou, H.W.; Yu, M.L. A New Theoretical Method to Predict Strata Movement and Surface Subsidence due to Inclined Coal Seam Mining. Rock Mech. Rock Eng. 2021, 54, 2723–2740. [Google Scholar] [CrossRef]
- Zhang, J.F. Analytical Solution and Engineering Application of the Inclined Rectangle Plate under Longitudinal and Lateral Load. In Proceedings of the International Conference on Structures and Building Materials, Guangzhou, China, 7–9 January 2011; pp. 696–700. [Google Scholar]
- Imrak, E.; Fetvaci, C. The Deflection Solution of a Clamped Rectangular Thin Plate Carrying Uniformly Load. Mech. Based Des. Struct. Mech. 2009, 37, 462–474. [Google Scholar] [CrossRef]
- Bakker, M.C.M.; Rosmanit, M.; Hofmeyer, H. Approximate large-deflection analysis of simply supported rectangular plates under transverse loading using plate post-buckling solutions. Thin-Walled Struct. 2008, 46, 1224–1235. [Google Scholar] [CrossRef]
- Li, X.L.; Liu, C.W.; Liu, Y.; Xie, H. The Breaking Span of Thick and Hard Roof Based on the Thick Plate Theory and Strain Energy Distribution Characteristics of Coal Seam and Its Application. Math. Probl. Eng. 2017, 2017, 14. [Google Scholar] [CrossRef]
- Liao, F.; Meng, D.Y.; Yang, Z.Y.; Wang, H.; Wu, R.J. Research on the Calculation Method of Internal Force of Combined Energy Dissipation Shed-tunnel Roof. In Proceedings of the 5th Annual International Conference on Material Science and Engineering (ICMSE), Xiamen, China, 20–22 October 2017. [Google Scholar]
- Wu, L.Y.; Bai, H.B.; Ma, D. Prediction and Prevention of Water Inrush Hazards from Bed Separation Space. Mine Water Environ. 2021, 40, 657–670. [Google Scholar] [CrossRef]
- Li, Y.X.; Ma, J.; Yang, K.M.; Jiang, K.G.; Gu, X.R.; Peng, L.S.; Chen, X.Y. Isolated overburden grout injection technology mining and grouting parameters discussion and optimization. Environ. Earth Sci. 2024, 83, 30. [Google Scholar] [CrossRef]
- Wang, W.R.; Lu, Y.; Zhao, D.Z.; Zhang, J.M.; Bai, X.S. Research on large deflection deformation reconstruction of elastic thin plate based on strain monitoring. Measurement 2020, 149, 11. [Google Scholar] [CrossRef]
- Liu, G.J.; Mu, Z.L.; Chen, J.J.; Yang, J.; Cao, J.L. Rock burst risk in an island longwall coal face by stress field. Geosci. J. 2018, 22, 609–622. [Google Scholar] [CrossRef]
- Sun, Y.J.; Zuo, J.P.; Karakus, M.; Wang, J.T. Investigation of movement and damage of integral overburden during shallow coal seam mining. Int. J. Rock Mech. Min. Sci. 2019, 117, 63–75. [Google Scholar] [CrossRef]
- Veiskarami, M.; Kumar, J.; Valikhah, F. Effect of the Flow Rule on the Bearing Capacity of Strip Foundations on Sand by the Upper-Bound Limit Analysis and Slip Lines. Int. J. Geomech. 2014, 14, 11. [Google Scholar] [CrossRef]
- Gesualdo, A.; Minutolo, V.; Nunziante, L. Local collapse in soft rock bank cavities. J. Geotech. Geoenviron. Eng. 2001, 127, 1037–1042. [Google Scholar] [CrossRef]
- Karamanski, T.; Kazakov, K. Modified model of a plate based on the Winkler foundation. In Proceedings of the 12th International Conference on Computational Methods and Experimental Measurements, Malta, 20–22 June 2005; pp. 417–423. [Google Scholar]
- Mofid, M.; Noroozi, M. A Plate on Winkler Foundation with Variable Coefficient. Sci. Iran. Trans. A-Civ. Eng 2009, 16, 249–255. [Google Scholar]
- Domagalski, L.; Jedrysiak, J. On the elastostatics of thin periodic plates with large deflections. Meccanica 2012, 47, 1659–1671. [Google Scholar] [CrossRef]
- Xu, W.; Zhao, S.J.; Zhang, W.Z.; Zhao, X.B. Numerical Simulation of Crack Propagation and Branching Behaviors in Heterogeneous Rock-like Materials. Buildings 2024, 14, 17. [Google Scholar] [CrossRef]
- Zhang, K.Y.; Liu, F.; Xia, K.W.; Xu, Y.; Dong, P.; Yu, C.Y. On the calibration and verification of Voronoi-based discontinuous deformation analysis for modeling rock fracture. J. Rock Mech. Geotech. Eng. 2023, 15, 2025–2038. [Google Scholar] [CrossRef]
- Liu, T. Mining rock impact and control engineering and its application. J. China Coal Socirty 1995, 20, 1–5. [Google Scholar]
- McNally, G.H.; Willey, P.L.; Creech, M. Geological Factorrs Influencing Longwall-iduced Subsidence. In Proceedings of the Symposiun on Geology in Longwall Mining, Sydney, Australia, 12–13 November 1996; pp. 257–267. [Google Scholar]
- Holla, L.; Barclay, E. Mine Subsidence in the Southern Coalfield, NSW, Australia; NSW Department of Mineral Resources: Maitland, NSW, Australia, 2000.




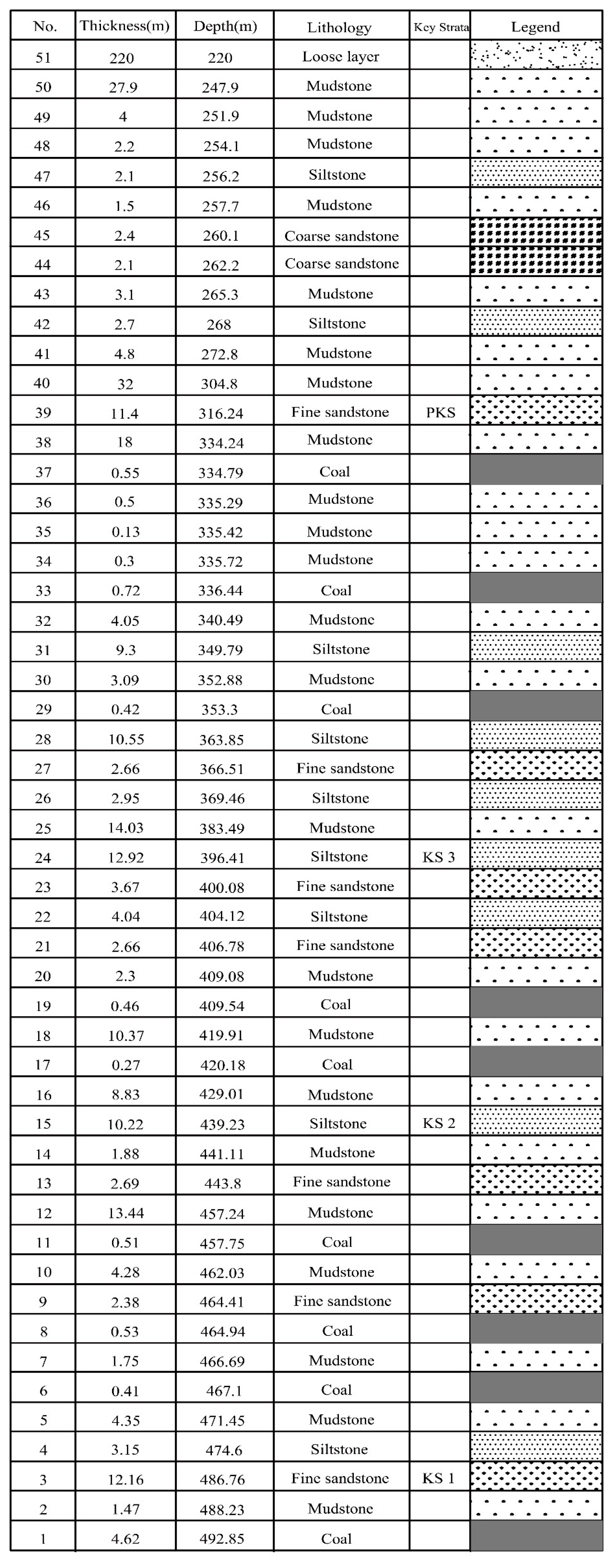





| Lithology | Density (kg/m3) | Tensile Strength (MPa) | Elastic Modulus (GPa) | Shear Modulus (GPa) | Cohesion (MPa) | Angle of Internal Friction (°) | Poisson’s Ratio |
|---|---|---|---|---|---|---|---|
| Loose layer | 2200 | 0.1 | 0.3 | 0.115 | 1.5 | 20 | 0.3 |
| Mudstone | 2600 | 1.2 | 13 | 5.28 | 1.73 | 31.3 | 0.23 |
| Siltstone | 2700 | 4.41 | 22.9 | 9.7 | 4.74 | 31.4 | 0.18 |
| Coarse sandstone | 2700 | 4.02 | 34.2 | 14.37 | 5.17 | 37.5 | 0.19 |
| Fine sandstone | 2700 | 4.34 | 34.1 | 14.96 | 3.15 | 35.9 | 0.14 |
| Coal | 1300 | 0.3 | 5.6 | 2.15 | 0.5 | 25 | 0.3 |
| Rock Strata Number | Initial Breakage Distance (m) | Initial Fracture Trace Angle (°) | Periodic Breakage Distance (m) | Periodic Fracture Trace Angle (°) |
|---|---|---|---|---|
| No. 3 fine sandstone (KS 1) | 33 | 61.2 | 21 | 55.4 |
| No. 4 to No. 14 soft rocks | 78.2 | 62.3 | ||
| No.15 siltstone (KS 2) | 38 | 64.4 | 21 | 56.2 |
| No. 16 to No. 23 soft rocks | 76.2 | 60.4 |
| Selection Models | Caved Zone Height (m) | Fractured Zone Height (m) | Continuous Bending Zone Height (m) |
|---|---|---|---|
| Empirical formulas | 31.07~46.61 | 33.27~44.47 | 175.68~202.42 |
| Model calculations | 38.07 | 41.13 | 187.56 |
| Distance of Working Face Mining (m) | PKS Deflection Start Position (m) | PKS Deflection End Position (m) | PKS Maximum Deflection Value (mm) | PKS Deflection Space Volume (m3) | Corresponding Date of Mining |
|---|---|---|---|---|---|
| 135 | 32 | 71 | 9.6 | 13.5 | 15 September 2015 |
| 300 | 32 | 236 | 1407.6 | 10768 | 27 October 2015 |
| 414 | 32 | 350 | 2090.8 | 24,933 | 2 December 2015 |
| 535 | 32 | 471 | 2683.2 | 44,172 | 26 December 2015 |
| 651 | 32 | 586 | 2714 | 56,485 | 15 April 2016 |
| Width of Working Face (m) | Initial Breakage Distance (m) | Periodic Breakage Distance (m) | PKS Deflection Start Position (m) | PKS Deflection End Position (m) | Bed-Separation Maximum Deflection Value (mm) | Bed-Separation Deflection Space Volume (m3) |
|---|---|---|---|---|---|---|
| 120 | 32 | 16 | 36 | 585 | 259 | 4312 |
| 150 | 33 | 21 | 32 | 587 | 545 | 11,228 |
| 180 | 34 | 22 | 29 | 329 | 825 | 9169 |
| 210 | 35 | 24 | 26 | 251 | 866 | 9251 |
| 240 | 35 | 27 | 24 | 223 | 884 | 10,043 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Yang, K.; Wei, X.; Tang, W.; Jiang, K. Quantitative Assessment of Bed-Separation Dynamic Development Caused by Inclined Coal Seam Longwall Mining. Mathematics 2024, 12, 1960. https://doi.org/10.3390/math12131960
Li Y, Yang K, Wei X, Tang W, Jiang K. Quantitative Assessment of Bed-Separation Dynamic Development Caused by Inclined Coal Seam Longwall Mining. Mathematics. 2024; 12(13):1960. https://doi.org/10.3390/math12131960
Chicago/Turabian StyleLi, Yaxing, Keming Yang, Xiangping Wei, Wei Tang, and Kegui Jiang. 2024. "Quantitative Assessment of Bed-Separation Dynamic Development Caused by Inclined Coal Seam Longwall Mining" Mathematics 12, no. 13: 1960. https://doi.org/10.3390/math12131960
APA StyleLi, Y., Yang, K., Wei, X., Tang, W., & Jiang, K. (2024). Quantitative Assessment of Bed-Separation Dynamic Development Caused by Inclined Coal Seam Longwall Mining. Mathematics, 12(13), 1960. https://doi.org/10.3390/math12131960








