Adaptive Iterative Learning Constrained Control for Linear Motor-Driven Gantry Stage with Fault-Tolerant Non-Repetitive Trajectory Tracking
Abstract
1. Introduction
- The designed controller achieves the desired tracking precision without requiring precise modeling or precise a priori knowledge of the system;
- By formulating a Barrier Composite Energy Function (BCEF) and integrating it into the controller design, the tracking control challenge associated with a LMDGS subject to state variables constraints is properly addressed;
- By incorporating the contributions of the controller’s output fault term, the designed control scheme can effectively mitigate to a significant extent the adverse effects of actuator failures, ensuring system’s convergence.
- By introducing in the design the desired trajectory correction function, non-repetitive desired trajectories can be effectively tracked while ensuring convergence of the system’s tracking error.
2. Problem Statement and Preliminaries
3. Modification of Reference Trajectories
- (1)
- are 2nd order differentiable;
- (2)
- are uniformly bounded over ;
- (3)
- whereas , where representes the ith order time derivative;
- (4)
- , over , where .
4. Controller Design and BCEF
5. Convergence Analysis
5.1. Finiteness of
5.2. Convergence of State Tracking Errors
5.2.1. Fictitious State Tracking Errors
5.2.2. State Tracking Errors
5.3. Boundedness of System States
6. Experimental Results and Discussion
6.1. Experimental Platform
6.2. Validation Experiments
6.2.1. TMILC Performance Experiment
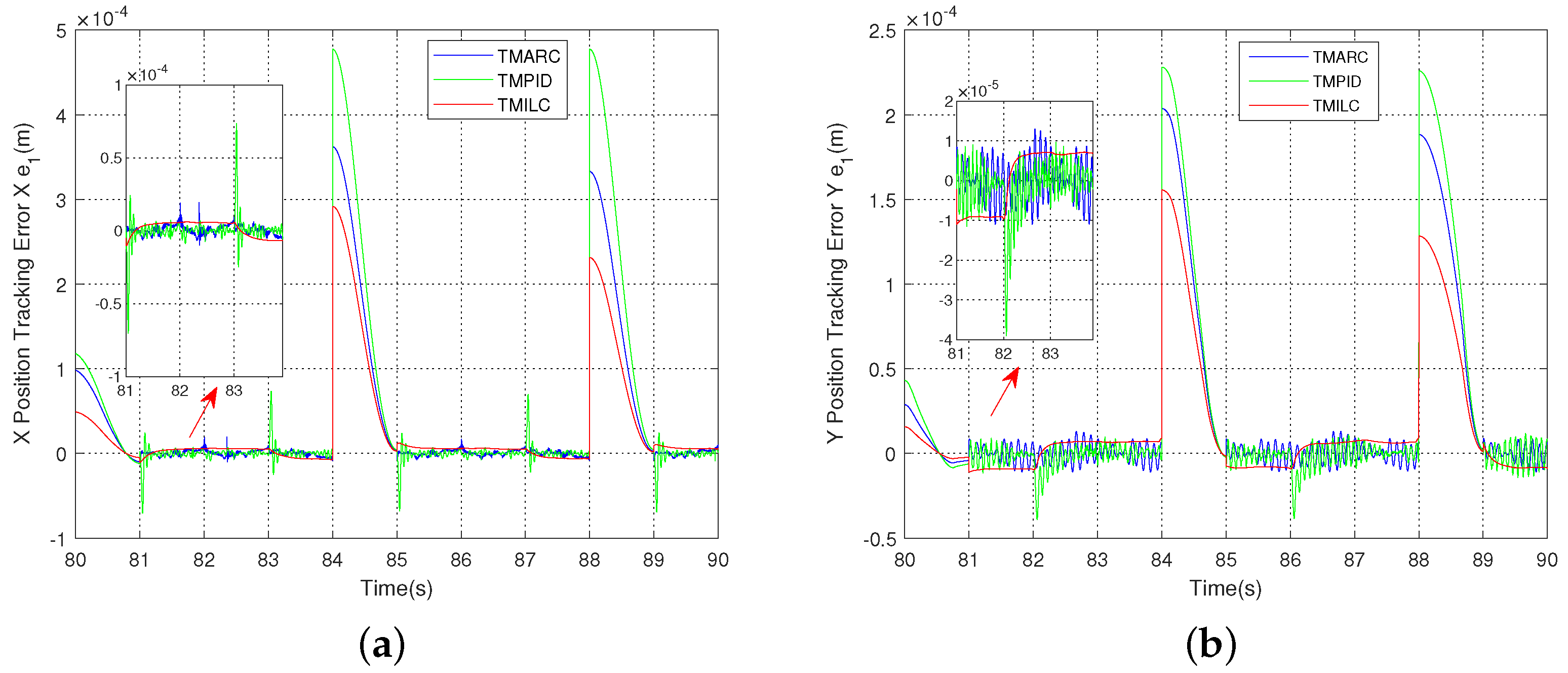
6.2.2. Comparison of TMARC, TMPID, and TMILC
7. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| n | Number of iterations |
| j | Order number, . |
| System states at n-th iteration. | |
| Expected trajectory at n-th iteration. | |
| The revised expected trajectory at n-th iteration. | |
| trajectory modifier functions. | |
| System state tracking errors at n-th iteration. | |
| System fictitious state tracking errors at n-th iteration. | |
| The parametric uncertainty at n-th iteration. | |
| External disturbances and unknown system dynamics at n-th iteration. | |
| The unknown control input gain function. | |
| Control input at n-th iteration. | |
| the control input subject to actuator faults at n-th iteration. | |
| System output, . | |
| barrier composite energy function at n-th iteration. | |
| First derivative of • | |
| Estimated value of • | |
| Error between and •, i.e., | |
| The constraint function of system position state tracking error at the n-th iteration |
References
- Zhou, S.; Shi, Y.; Wang, D.; Xu, X.; Xu, M.; Deng, Y. Election Optimizer Algorithm: A New Meta-Heuristic Optimization Algorithm for Solving Industrial Engineering Design Problems. Mathematics 2024, 12, 1513. [Google Scholar] [CrossRef]
- Pan, H.; Chang, X.; Sun, W. Multitask Knowledge Distillation Guides End-to-End Lane Detection. IEEE Trans. Ind. Inform. 2023, 19, 9703–9712. [Google Scholar] [CrossRef]
- Pan, H.; Hong, Y.; Sun, W.; Jia, Y. Deep Dual-Resolution Networks for Real-Time and Accurate Semantic Segmentation of Traffic Scenes. IEEE Trans. Intell. Transp. Syst. 2023, 24, 3448–3460. [Google Scholar] [CrossRef]
- Kang, Z.; Lin, W.; Liu, Z.; Xu, R. Accurate Contour Error Estimation-based robust contour control for dual-linear-motor-driven gantry stages. Mechatronics 2024, 100, 103174. [Google Scholar] [CrossRef]
- Shi, P.; Yu, X.; Yang, X.; Rodríguez-Andina, J.J.; Sun, W.; Gao, H. Composite Adaptive Synchronous Control of Dual-Drive Gantry Stage with Load Movement. IEEE Open J. Ind. Electron. Soc. 2023, 4, 63–74. [Google Scholar] [CrossRef]
- Li, T.; Tao, L.; Xu, B. Linear Parameter Varying Observer-Based Adaptive Dynamic Surface Sliding Mode Control for PMSM. Mathematics 2024, 12, 1219. [Google Scholar] [CrossRef]
- Gao, H.; Liu, Y.; Sun, W.; Yu, X. Adaptive Wavelet Tracking Control of Dual-Linear-Motor-Driven Gantry Stage with Suppression of Crossbeam Rotation. IEEE/ASME Trans. Mechatron. 2023, 29, 97–105. [Google Scholar] [CrossRef]
- Sun, W.; Liu, J.; Gao, H. A Composite High-Speed and High-Precision Positioning Approach for Dual-Drive Gantry Stage. IEEE Trans. Autom. Sci. Eng. 2024, 1–12. [Google Scholar] [CrossRef]
- Liu, Y.; Sun, W. High-Performance Position Control for Repetitive Tasks of Motor-Driven Servo Systems Based on Periodic Disturbance Observer. IEEE/ASME Trans. Mechatron. 2023, 28, 2461–2470. [Google Scholar] [CrossRef]
- Yuan, Y.; Sun, W. An Integrated Kinematic Calibration and Dynamic Identification Method with Only Static Measurements for Serial Robot. IEEE/ASME Trans. Mechatron. 2023, 28, 2762–2773. [Google Scholar] [CrossRef]
- Hou, S.; Qiu, Z.; Chu, Y.; Gao, J.; Fei, J. Emotional Intelligent Finite-Time Tracking Control for a Class of Nonlinear Systems. IEEE Internet Things J. 2024, 11, 20421–20432. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, J.; Gomes, L.; Sun, W. Adaptive Robust Control for Networked Strict-Feedback Nonlinear Systems with State and Input Quantization. Electronics 2021, 10, 2783. [Google Scholar] [CrossRef]
- Pan, H.; Luo, M.; Wang, J.; Huang, T.; Sun, W. A Safe Motion Planning and Reliable Control Framework for Autonomous Vehicles. IEEE Trans. Intell. Veh. 2024, 1–14. [Google Scholar] [CrossRef]
- Guo, Z.; Zhen, S.; Liu, X.; Zhong, H.; Yin, J.; Chen, Y.H. Design and application of a novel approximate constraint tracking robust control for permanent magnet synchronous motor. Comput. Chem. Eng. 2023, 173, 108206. [Google Scholar] [CrossRef]
- Xu, Q.Y.; He, W.Y.; Zheng, C.T.; Xu, P.; Wei, Y.S.; Wan, K. Adaptive Fuzzy Iterative Learning Control for Systems with Saturated Inputs and Unknown Control Directions. Mathematics 2022, 10, 3462. [Google Scholar] [CrossRef]
- Li, C.; Yao, B.; Wang, Q. Modeling and Synchronization Control of a Dual Drive Industrial Gantry Stage. IEEE/ASME Trans. Mechatron. 2018, 23, 2940–2951. [Google Scholar] [CrossRef]
- Zhou, L.; Trumper, D.L. Magnetically Levitated Linear Stage with Linear Bearingless Slice Hysteresis Motors. IEEE/ASME Trans. Mechatron. 2021, 26, 1084–1094. [Google Scholar] [CrossRef]
- Li, C.; Sun, Y.; Pu, S. Accurate physical modeling and synchronization control of dual-linear-motor-driven gantry with dynamic load. AIP Adv. 2021, 11, 025133. [Google Scholar] [CrossRef]
- Yu, C.; Ma, J.; Pan, H.; Basin, M.V. Adaptive Iterative Learning Constrained Control for Linear-Motor-Driven Gantry Stage. IEEE/ASME Trans. Mechatron. 2023, 1–12. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Z. Distributed model free adaptive fault-tolerant consensus tracking control for multiagent systems with actuator faults. Inf. Sci. 2024, 664, 120313. [Google Scholar] [CrossRef]
- Pan, H.; Zhang, C.; Sun, W. Fault-Tolerant Multiplayer Tracking Control for Autonomous Vehicle via Model-Free Adaptive Dynamic Programming. IEEE Trans. Reliab. 2023, 72, 1395–1406. [Google Scholar] [CrossRef]
- Wang, C.; Zhou, Z.; Wang, J. Distributed Fault Diagnosis via Iterative Learning for Partial Differential Multi-Agent Systems with Actuators. Mathematics 2024, 12, 955. [Google Scholar] [CrossRef]
- Zhu, Z.; Zhao, H.; Sun, H. Stackelberg-Theoretic Optimal Robust Control for Constrained Permanent Magnet Linear Motor with Inequality Constraints. IEEE/ASME Trans. Mechatron. 2022, 27, 5439–5450. [Google Scholar] [CrossRef]
- Gong, J.; Yu, X.; Pan, H.; Rodríguez-Andina, J.J. Adaptive Fault Tolerant Control of Linear Motors under Sensor and Actuator Faults. IEEE Trans. Transp. Electrif. 2024, 1. [Google Scholar] [CrossRef]
- Pan, H.; Zhang, D.; Sun, W.; Yu, X. Event-Triggered Adaptive Asymptotic Tracking Control of Uncertain MIMO Nonlinear Systems with Actuator Faults. IEEE Trans. Cybern. 2022, 52, 8655–8667. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Pan, H.; Zhang, D. Event-Triggered Adaptive Finite-Time Control for MIMO Nonlinear Systems with Actuator Faults. IEEE Trans. Ind. Electron. 2023, 70, 7343–7352. [Google Scholar] [CrossRef]
- Chen, Y.; Jiang, W.; Charalambous, T. Machine learning based iterative learning control for non-repetitive time-varying systems. Int. J. Robust Nonlinear Control 2023, 33, 4098–4116. [Google Scholar] [CrossRef]
- Meng, D.; Zhang, J. Robust Tracking of Nonrepetitive Learning Control Systems with Iteration-Dependent References. IEEE Trans. Syst. Man Cybern. Syst. 2021, 51, 842–852. [Google Scholar] [CrossRef]
- Wang, L.; Dong, L.; Yang, R.; Chen, Y. Dynamic ILC for Linear Repetitive Processes Based on Different Relative Degrees. Mathematics 2022, 10, 4824. [Google Scholar] [CrossRef]
- Pan, H.; Chang, X.; Zhang, D. Event-Triggered Adaptive Control for Uncertain Constrained Nonlinear Systems with Its Application. IEEE Trans. Ind. Inform. 2020, 16, 3818–3827. [Google Scholar] [CrossRef]
- Li, J.; Li, J. Adaptive iterative learning control for coordination of second-order multi-agent systems. Int. J. Robust Nonlinear Control 2014, 24, 3282–3299. [Google Scholar] [CrossRef]
- Gao, H.; Li, Z.; Yu, X.; Qiu, J. Hierarchical Multiobjective Heuristic for PCB Assembly Optimization in a Beam-Head Surface Mounter. IEEE Trans. Cybern. 2022, 52, 6911–6924. [Google Scholar] [CrossRef] [PubMed]
- Jin, X. Fault tolerant finite-time leader–follower formation control for autonomous surface vessels with LOS range and angle constraints. Automatica 2016, 68, 228–236. [Google Scholar] [CrossRef]
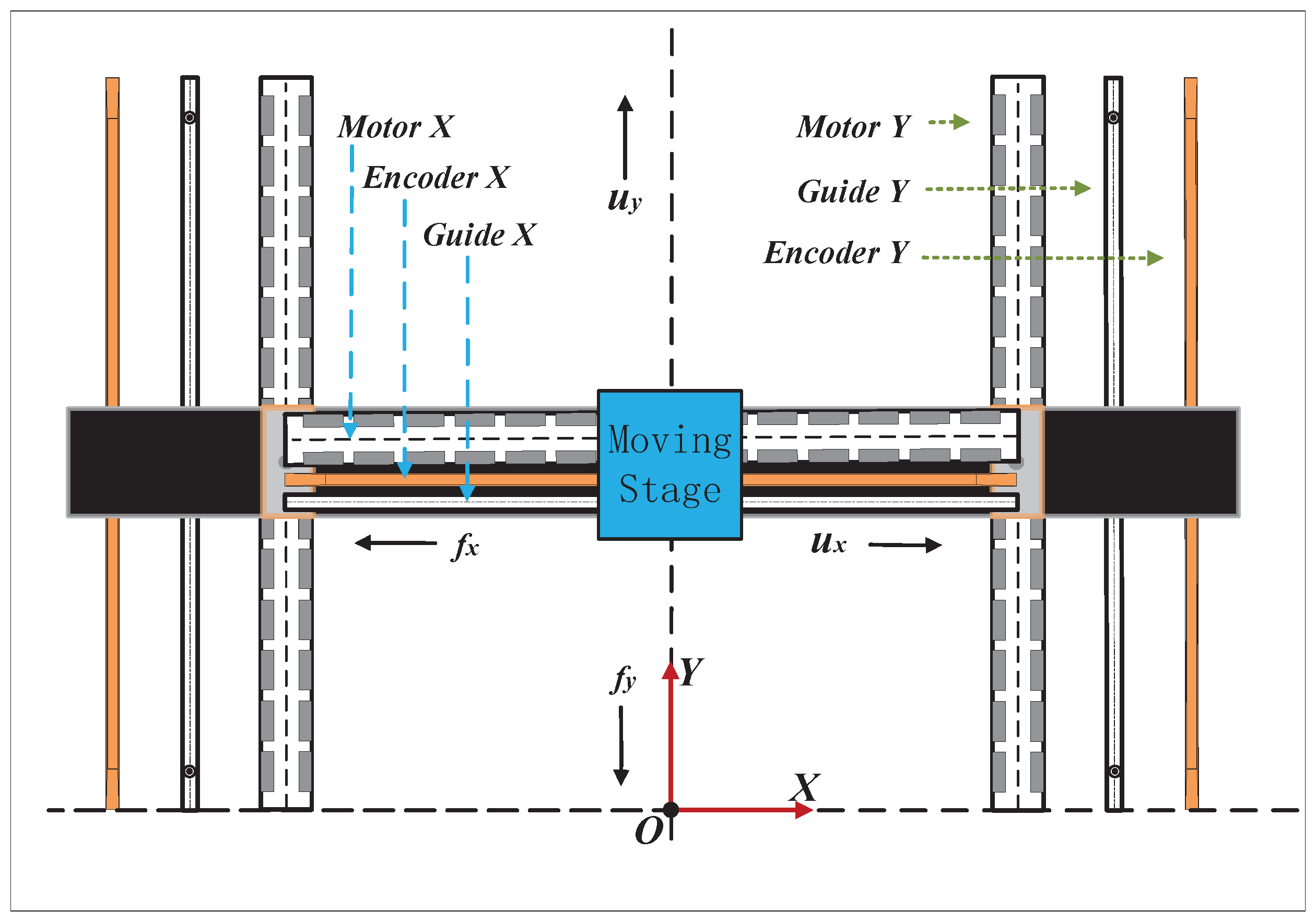

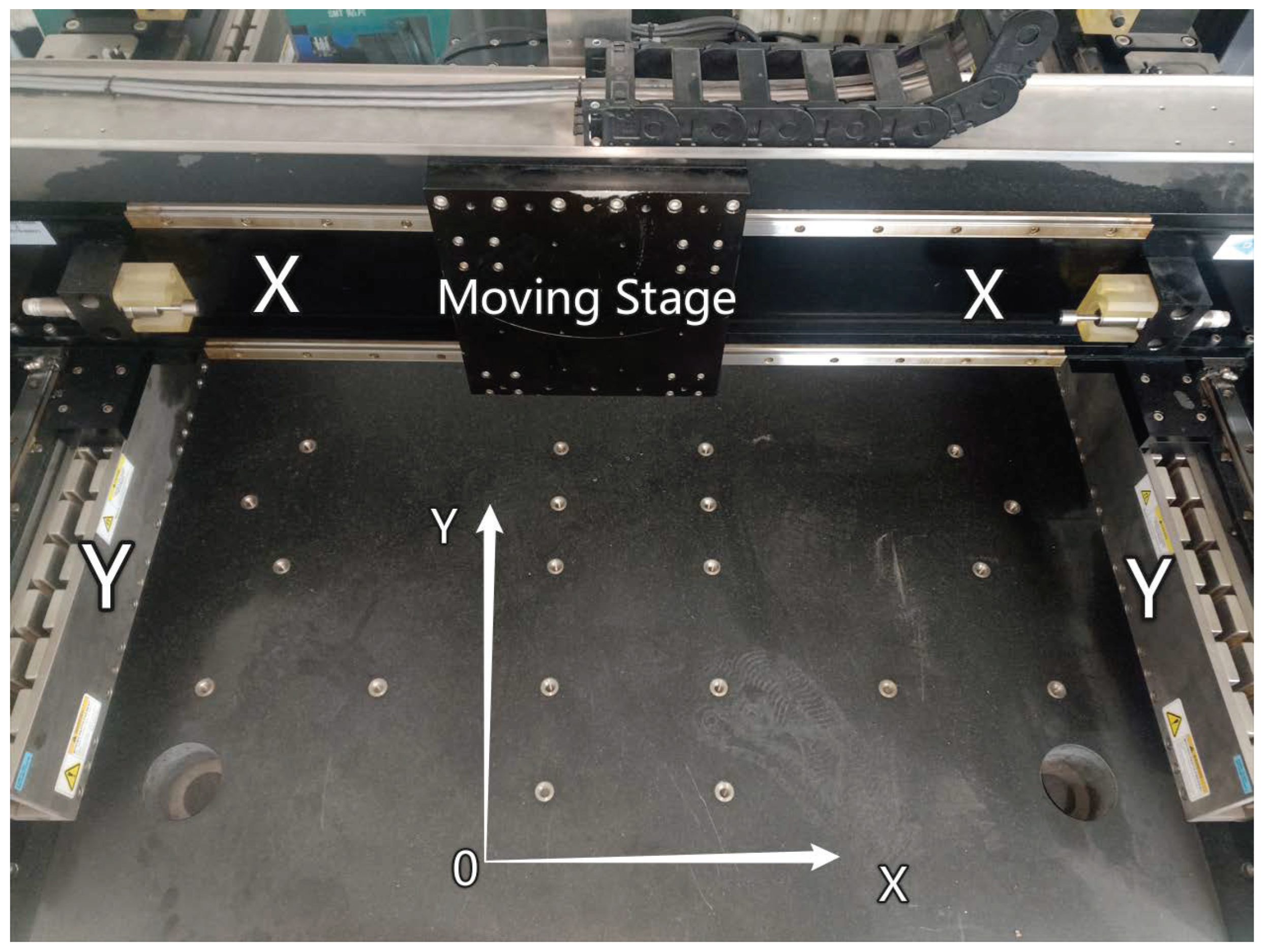
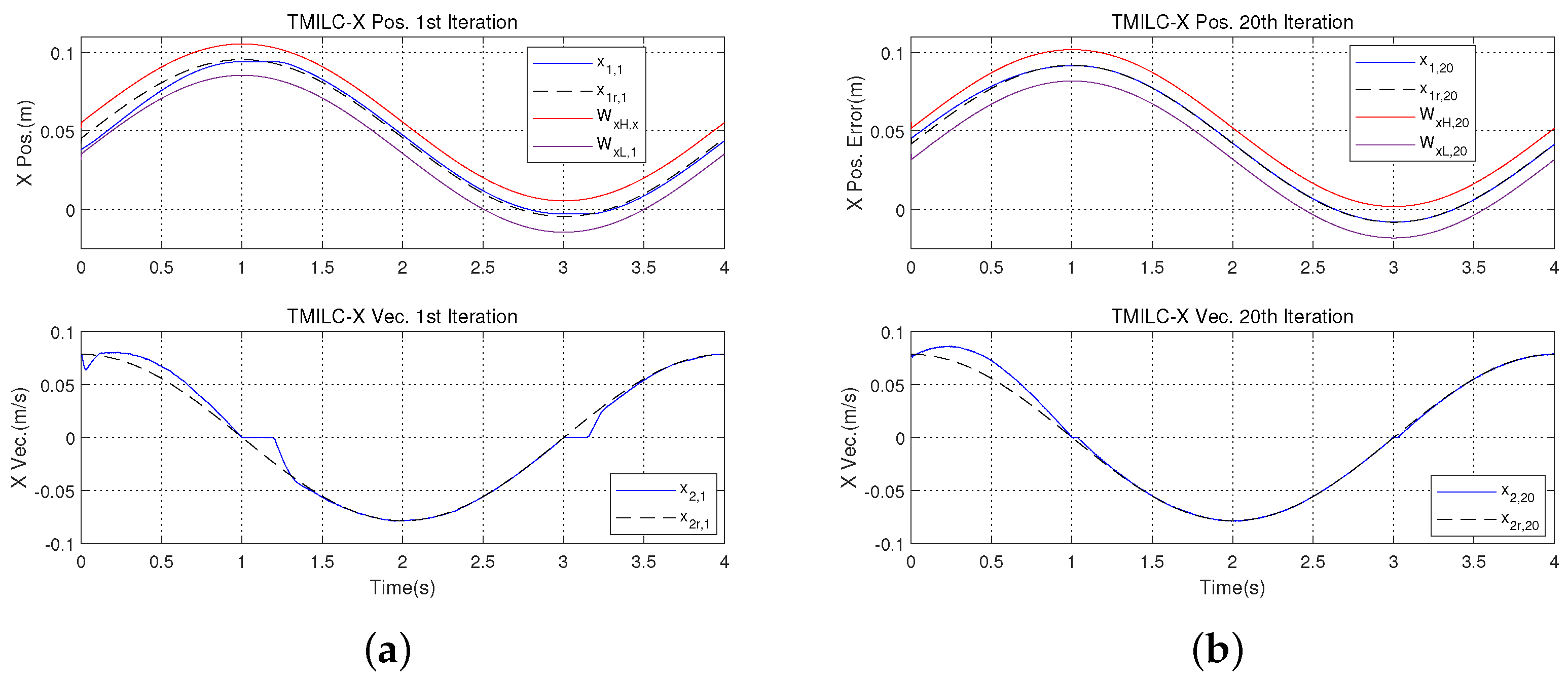
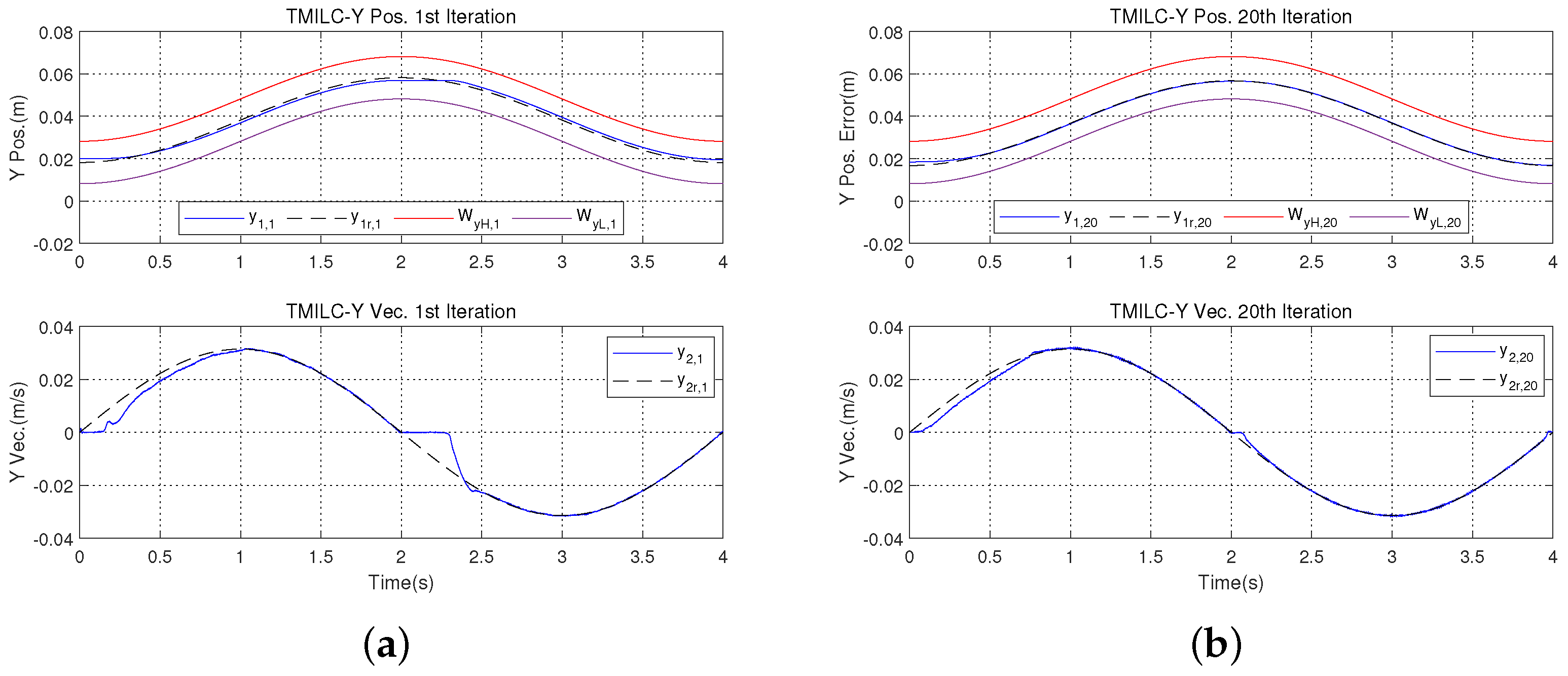


| Index | m | f | ||||
|---|---|---|---|---|---|---|
| Unit | kg | N·s/mm | N | 1 | N/V | 1 |
| X | 15 | 0.1 | 3 | 0.03 | 10 | 9.738 |
| Y | 25 | 0.1 | 3 | 0.03 | 80 | 30.531 |
| Index | TMILC | TMARC | TMPID |
|---|---|---|---|
| Max | 1.0315 | 1.9304 | 7.3413 |
| Max | 1.1047 | 1.2986 | 3.9029 |
| RMS | 3.3453 | 5.3372 | 9.7100 |
| RMS | 5.3772 | 7.6146 | 6.6105 |
| Index | TMILC | TMARC | TMPID |
|---|---|---|---|
| Max | 6.9881 | 2.6380 | 4.3013 |
| Max | 7.2846 | 1.7175 | 1.4549 |
| RMS | 1.4865 | 4.0457 | 6.8210 |
| RMS | 1.1971 | 7.3033 | 4.3452 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, C. Adaptive Iterative Learning Constrained Control for Linear Motor-Driven Gantry Stage with Fault-Tolerant Non-Repetitive Trajectory Tracking. Mathematics 2024, 12, 1673. https://doi.org/10.3390/math12111673
Yu C. Adaptive Iterative Learning Constrained Control for Linear Motor-Driven Gantry Stage with Fault-Tolerant Non-Repetitive Trajectory Tracking. Mathematics. 2024; 12(11):1673. https://doi.org/10.3390/math12111673
Chicago/Turabian StyleYu, Chaohai. 2024. "Adaptive Iterative Learning Constrained Control for Linear Motor-Driven Gantry Stage with Fault-Tolerant Non-Repetitive Trajectory Tracking" Mathematics 12, no. 11: 1673. https://doi.org/10.3390/math12111673
APA StyleYu, C. (2024). Adaptive Iterative Learning Constrained Control for Linear Motor-Driven Gantry Stage with Fault-Tolerant Non-Repetitive Trajectory Tracking. Mathematics, 12(11), 1673. https://doi.org/10.3390/math12111673





