Ulam Stability for Boundary Value Problems of Differential Equations—Main Misunderstandings and How to Avoid Them
Abstract
1. Introduction
- -
- In Section 2, we provide a brief overview of the concepts of Ulam type stability and its applications to various problems such as:
- *
- Initial value problems to any type of differential equation with various types of derivatives, such as an ordinary derivative or a partial derivative of integer order, any type of fractional derivatives—we briefly make a review and give an algorithm for the application of Ulam type stability;
- *
- Boundary value problems to any type of differential equation with various types of derivatives—we emphasize the basic misunderstandings of the application of Ulam type stability;
- *
- A new methodology—we give one of the possible ways to avoid the mentioned misunderstandings by introducing a parameter in the boundary condition;
- *
- All of the above theoretical explanations are illustrated with appropriate examples.
- -
- In Section 3, we apply the proposed new methodology to study Ulam type stability for a linear boundary value problem for a differential equation with instantaneous impulses and the Caputo fractional derivative with respect to another function.
- -
- To summarize and to emphasize some possible applications of the suggested approach, we finish the paper with a conclusion.
2. Brief Overview of the Concepts of Ulam Type Stability
- 1.
- Statement of the problem: Define the main problem of study which consists of two parts:
- -
- The differential equation where is the unknown function, and is an applied derivative. Note , , the applied derivative could be an ordinary derivative or a fractional derivative, and the function F could have more than two arguments (it could contain any type of integral or derivative);
- -
- The initial condition (IVP), the boundary condition (BVP), or both IVP and BVP.
- 2.
- Integral presentation: Obtain the equivalent integral presentation (integral equation) of the solution of the problem defined in Step 1.
- 3.
- Existence of solution: Define an operator based on the integral equation obtained in Step 2, and prove the existence (and uniqueness) of a fixed point (or points), i.e., prove the existence of a solution of the problem defined in Step 1.
- 4.
- Definition of Ulam type stability: Define in an appropriate way the differential inequality deeply connected with the problem given in Step 1. Based on this inequality, define the Ulam type stability (US) of the problem given in Step 1.
- 5.
- Proof of US: Based on Step 2, Step 3, and Step 4, prove the Ulam type stability of the solutions of the studied problem.
2.1. Initial Value Problems
2.2. Boundary Value Problems
2.2.1. Some Misunderstandings in Study Ulam Stability for Boundary Value Problems
- P1.
- P2.
2.2.2. How to Avoid the Misunderstandings
3. Ulam Stability of BVP for Impulsive -Caputo Fractional Differential Equation
3.1. Preliminary Results
3.1.1. Some Results from Fractional Calculus
3.1.2. Preliminary Results for Scalar Linear Impulsive -Caputo Fractional Differential Equations
3.2. Application of the Algorithm for Ulam Type Stability to BVPs
- Step 1. Statement of the problem
- (A1).
- The function is smooth and increasing with almost everywhere in .
- (A2).
- The sequence
- (A3).
- The sequence
- (A4).
- The constants , are such that .
- (A5).
- The function .
- (A6).
- There exists a constant such that for and .
- Step 2. Integral presentation.
- Step 3. Existence of a solution.
- Step 4. Definition of Ulam type stability
- Step 5. Proof of US.
3.3. Partial Cases
3.3.1. Boundary Value Problem for a Fractional Differential Equation without Any Impulses
3.3.2. Boundary Value Problem for an Impulsive Caputo Fractional Differential Equation
3.3.3. Initial Value Problem for an Impulsive Fractional Differential Equation
3.3.4. Initial Value Problem for a Fractional Differential Equation without Any Impulses
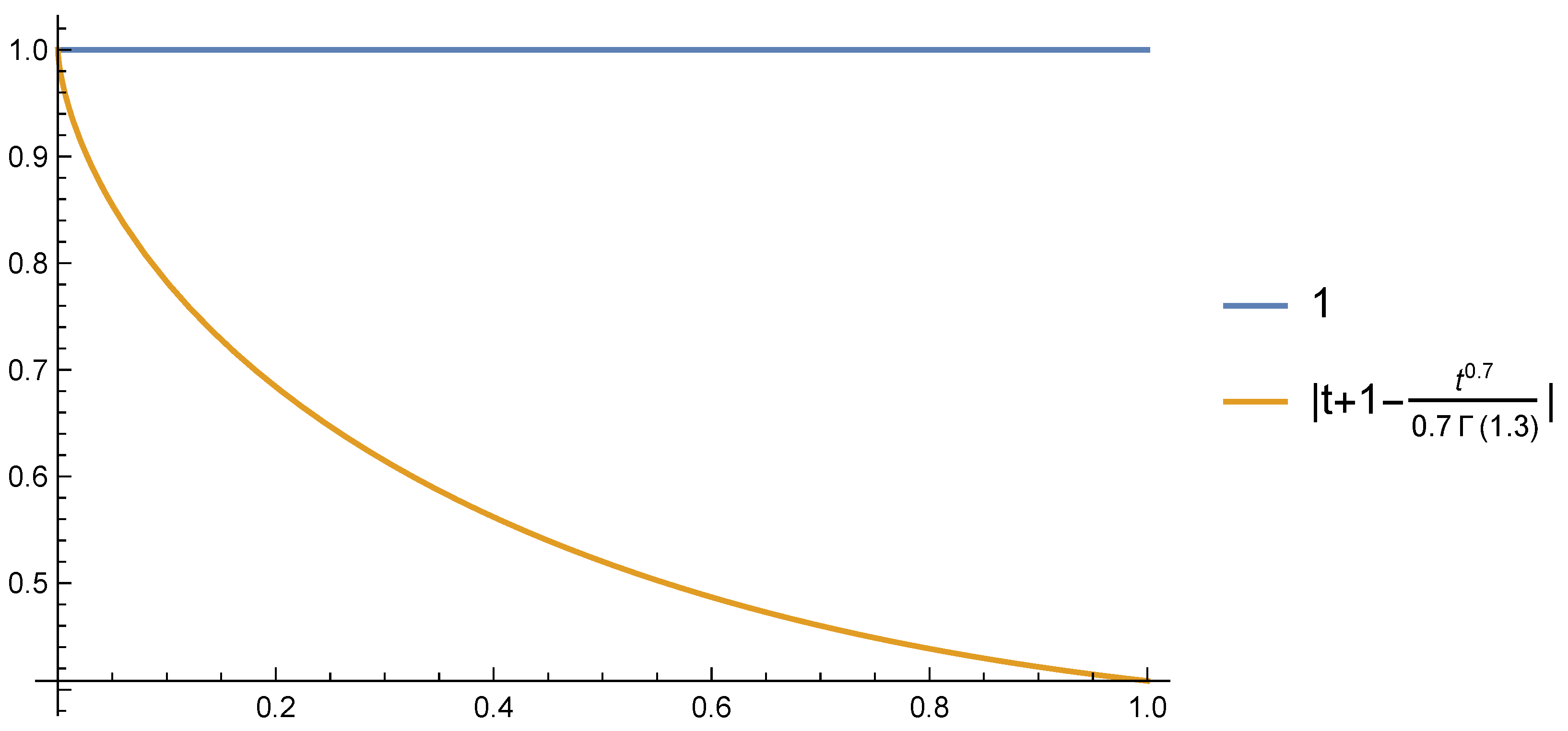
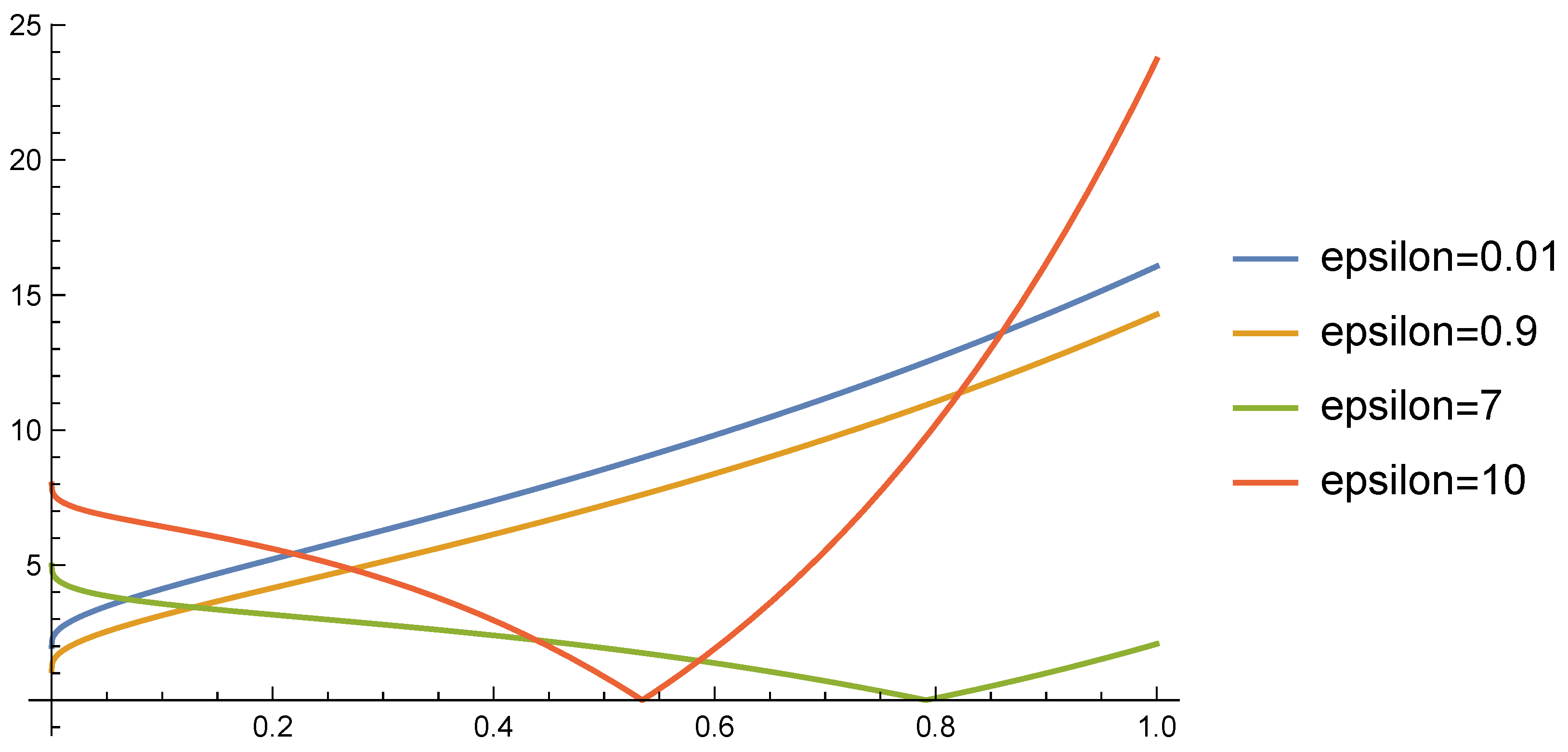
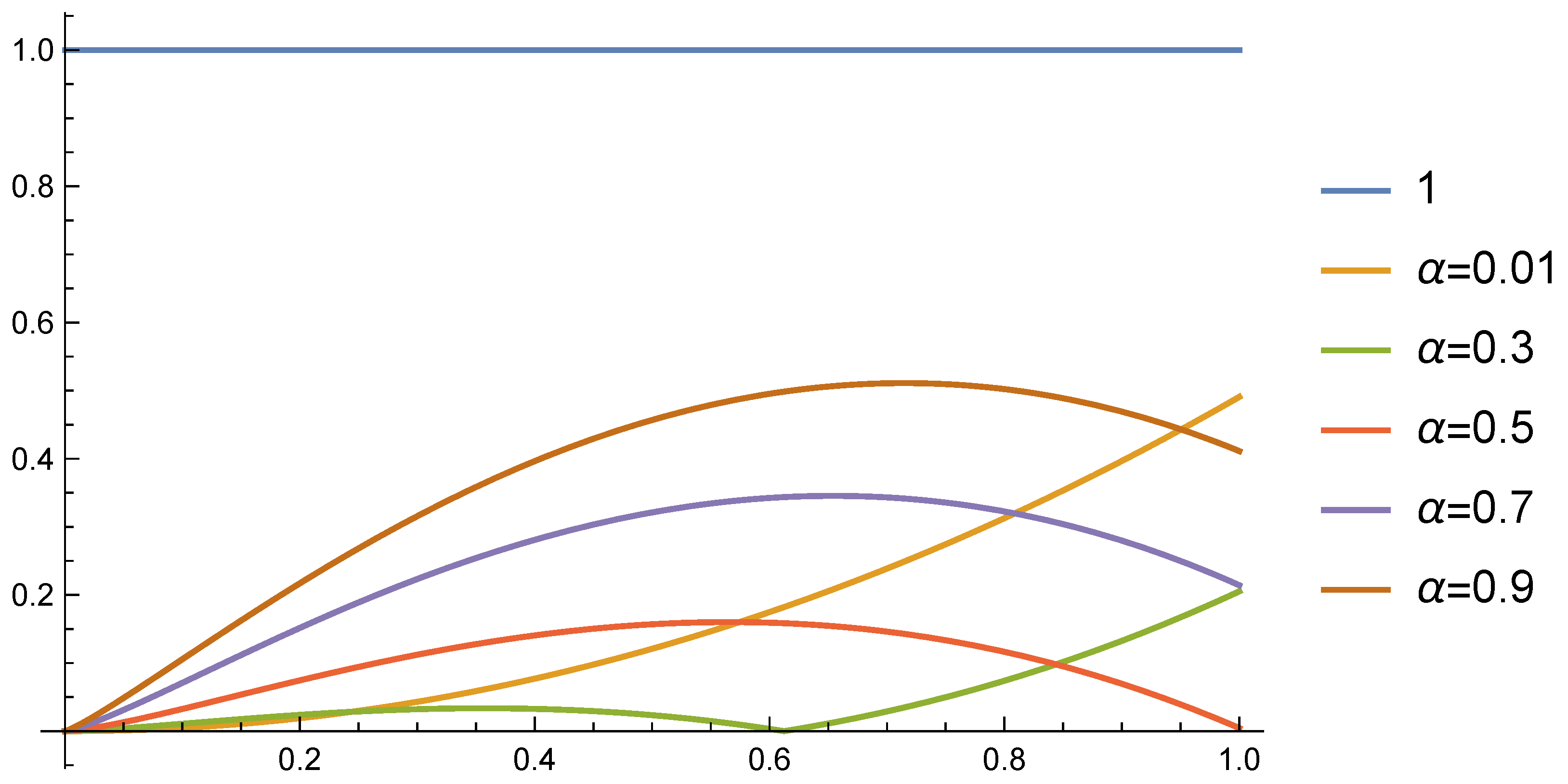
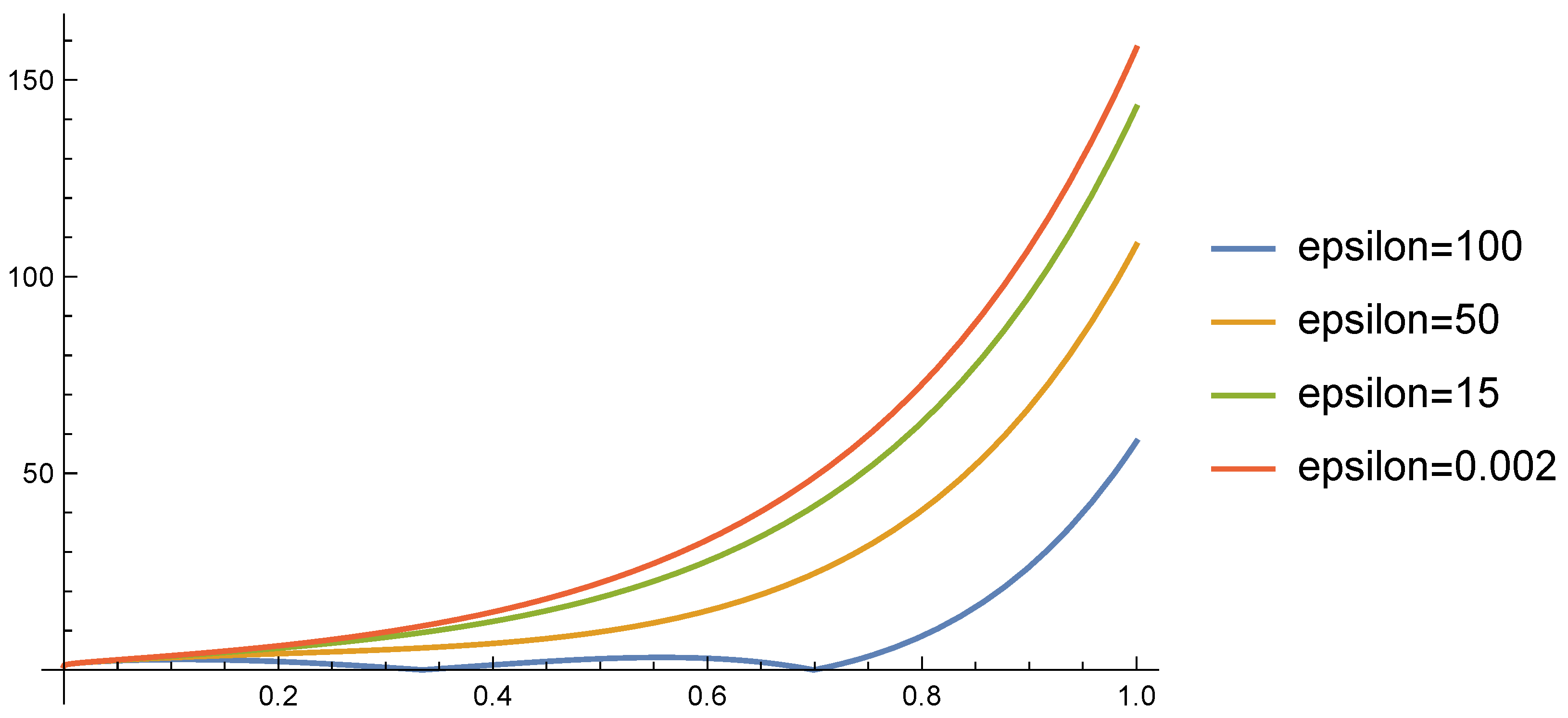
3.4. Application
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ulam, S.M. A Collection of Mathematical Problems; Interscience Tracts in Pure and Applied Mathematics, no. 8; Interscience Publishers: New York, NY, USA, 1960. [Google Scholar]
- Hyers, D.H. On the stability of the linear functional equation. Proc. Nat. Acad. Sci. USA 1941, 27, 222–224. [Google Scholar] [CrossRef]
- Tamilvanan, K.; Alkhaldi, A.H.; Jakhar, J.; Chugh, R.; Jakhar, J.; Rassias, J.M. Ulam Stability Results of Functional Equations in Modular Spaces and 2-Banach Spaces. Mathematics 2023, 11, 371. [Google Scholar] [CrossRef]
- Ciepliński, K. On Ulam Stability of a Functional Equation. Results Math. 2020, 75, 151. [Google Scholar] [CrossRef]
- Jung, S.-M. Hyers–Ulam–Rassias Stability of Functional Equations in Nonlinear Analysis; Springer: New York, NY, USA, 2011. [Google Scholar]
- Cadariu, L. Stabilitatea Ulam–Hyers–Bourgin Pentru Ecuatii Functionale; Universitatea de Vest din Timisoara: Timisoara, Romania, 2007. [Google Scholar]
- Hyers, D.H.; Isac, G.; Rassias, T. Stability of Functional Equations in Several Variables; Birkhauser: Basel, Switzerland, 1998. [Google Scholar]
- Agarwal, R.; Hristova, S.; O’Regan, D. Generalized Proportional Caputo Fractional Differential Equations with Noninstantaneous Impulses: Concepts, Integral Representations, and Ulam Type-Stability. Mathematics 2022, 10, 2315. [Google Scholar] [CrossRef]
- Batista, M. On stability of non-inflectional elastica. Comptes Rendus. Mec. 2020, 348, 137–148. [Google Scholar] [CrossRef]
- Joshi, H.; Jha, B.K. 2D memory-based mathematical analysis for the combined impact of calcium influx and efflux on nerve cells. Comput. Math. Appl. 2023, 134, 33–44. [Google Scholar] [CrossRef]
- Rus, I.A. Ulam stability of ordinary differential equations. Stud. Univ.-Babes-Bolyai Math. 2009, LIV, 125–133. [Google Scholar]
- Rus, I.A. Ulam stabilities of ordinary differential equations in a Banach space. Carpathian J. Math. 2010, 26, 103–107. [Google Scholar]
- Tripathy, A.K. Hyers-Ulam Stability of Ordinary Differential Equations; Chapman and Hall/CRC: New York, NY, USA, 2021. [Google Scholar]
- Liao, Y.; Wang, J. A note on stability of impulsive differential equations. Bound Value Probl. 2014, 2014, 67. [Google Scholar] [CrossRef]
- Zada, A.; Faisal, S.; Li, Y. On the Hyers-Ulam stability of first-order impulsive delay differential equations. J. Funct. Spaces 2006, 2006, 8164978. [Google Scholar] [CrossRef]
- Hristova, R.A.S.; O’Regan, D. Ulam type stability results for non-instantaneous impulsive differential equations with finite state dependent delay. Dyn. Syst. Appl. 2019, 28, 47–61. [Google Scholar]
- Wang, J.R.; Feckan, M.; Zhou, Y. Ulam’s type stability of impulsive ordinary differential equations. J. Math. Anal. Appl. 2012, 395, 258–264. [Google Scholar] [CrossRef]
- Wang, J.; Lv, L.; Zhou, Y. Ulam stability and data dependence for fractional differential equations with Caputo derivative. Electron. J. Qual. Theory Differ. Equ. 2011, 63, 1–10. [Google Scholar] [CrossRef]
- Limpanukorn, N.; Ngiamsunthorn, P.S. Existence and Ulam stability of solution to fractional order hybrid differential equations of variable order. Thai J. Math. 2020, 18, 453–463. [Google Scholar]
- Wang, X.; Luo, D.; Zhu, Q. Ulam-Hyers stability of Caputo type fuzzy fractional differential equations with time-delays. Chaos Solitons Fractals 2022, 156, 111822. [Google Scholar] [CrossRef]
- Chen, C.; Bohner, M.; Jia, B. Ulam-Hyers stability of Caputo fractional difference equations. Math. Meth. Appl. Sci. 2019, 42, 7461–7470. [Google Scholar] [CrossRef]
- Liu, K.; Feckan, M.; O’Regan, D.; Wang, J.R. Hyers–Ulam Stability and Existence of Solutions for Differential Equations with Caputo–Fabrizio Fractional Derivative. Mathematics 2019, 7, 333. [Google Scholar] [CrossRef]
- Liu, H.; Li, Y. Hyers–Ulam stability of linear fractional differential equations with variable coefficients. Adv. Differ. Equ. 2020, 2020, 404. [Google Scholar] [CrossRef]
- Wang, J.; Zhou, Y.; Feckan, M. Nonlinear impulsive problems for fractional differential equations and Ulam stability. Comput. Math. Appl. 2012, 64, 3389–3405. [Google Scholar] [CrossRef]
- Harikrishnan, S.; Ibrahim, R.W.; Kanagarajan, K. Fractional Ulam-stability of fractional impulsive differential equation involving Hilfer-Katugampola fractional differential operator. Univers. J. Math. Appl. 2018, 1, 106–112. [Google Scholar] [CrossRef]
- Agarwal, R.; Hristova, S.; O’Regan, D. Existence and Ulam type stability for nonlinear Riemann–-Liouville fractional differential equations with constant delay. Electron. J. Qual. Theory Differ. Equ. 2020, 67, 1–18. [Google Scholar] [CrossRef]
- Vanterler da, C.; Sousa, J.; Capelas de Oliveira, E.; Rodrigues, F.G. Ulam-Hyers stabilities of fractional functional differential equations. AIMS Math. 2020, 5, 1346–1358. [Google Scholar] [CrossRef]
- O’Regan, D.; Hristova, S.; Agarwal, R.P. Ulam-Type Stability Results for Variable Order Ψ-Tempered Caputo Fractional Differential Equations. Fractal Fract. 2024, 8, 11. [Google Scholar] [CrossRef]
- El-Sayed, A.M.A.; Al Issa, S.M.; Elmiari, M. Ulam-type Stability for a Boundary Value Problem of Implicit Fractional-orders Differential Equation. Adv. Dynam. Syst. Appl. (ADSA) 2021, 16, 75–89. [Google Scholar] [CrossRef]
- Rahman, G.; Agarwal, R.P.; Ahmad, D. Existence and stability analysis of n-th order multi term fractional delay differential equation. Chaos Solitons Fractals 2022, 155, 111709. [Google Scholar] [CrossRef]
- Ahmad, D.; Agarwal, R.P.; ur Rahman, G. Formulation, solution’s existence, and stability analysis for multi-term system of fractional-order differential equations. Symmetry 2022, 14, 1342. [Google Scholar] [CrossRef]
- Shah, K.; Tunc, S. Existence theory and stability analysis to a system of boundary value problem. J. Taibah Uni. Sci. 2017, 11, 1330–1342. [Google Scholar] [CrossRef]
- Zada, A.; Ali, S.; Li, Y. Ulam-type stability for a class of implicit fractional differential equations with non-instantaneous integral impulses and boundary condition. Adv. Differ. Equ. 2017, 2017, 317. [Google Scholar] [CrossRef]
- Salim, A.; Benchohra, M.; Lazreg, J.E.; N’Guerekata, G. Boundary Value Problem for Nonlinear Implicit Generalized Hilfer-Type Fractional Differential Equations with Impulses. Abstr. Appl. Anal. 2021, 2021, 5592010. [Google Scholar] [CrossRef]
- Benkerrouche, A.; Souid, M.S.; Stamov, G.; Stamova, I. Multiterm Impulsive Caputo–Hadamard Type Differential Equations of Fractional Variable Order. Axioms 2022, 11, 634. [Google Scholar] [CrossRef]
- Zamir, M.; Shah, K.; Chohan, M.I. Stability theory and existence of solution to a multi-point boundary value problem of fractional diferential equations. Math. Sci. 2019, 13, 53–60. [Google Scholar] [CrossRef]
- Treanbucha, C.; Sudsutad, W. Stability analysis of boundary value problems for Caputo proportional fractional derivative of a function with respect to another function via impulsive Langevin equation. AIMS Math. 2021, 6, 6647–6686. [Google Scholar] [CrossRef]
- Agarwal, R.; Hristova, S.; O’Regan, D. Boundary Value Problems for Fractional Differential Equations of Caputo Type and Ulam Type Stability: Basic Concepts and Study. Axioms 2023, 12, 226. [Google Scholar] [CrossRef]
- Agarwal, R.P.; Hristova, S. Ulam-Type Stability for a Boundary-Value Problem for Multi-Term Delay Fractional Differential Equations of Caputo Type. Axioms 2022, 11, 742. [Google Scholar] [CrossRef]
- Wahash, H.A.; Panchal, S.K.; Abdo, M.S. Existence and stability of a nonlinear fractional differential equation involving a ψ-Caputo operator. Adv. Theory Nonlinear Anal. Its Appl. 2020, 4, 266–278. [Google Scholar] [CrossRef]
- Almeida, R. A Caputo fractional derivative of a function with respect to another function. Commun. Nonlinear Sci. Numer. Simul. 2017, 44, 460–481. [Google Scholar] [CrossRef]
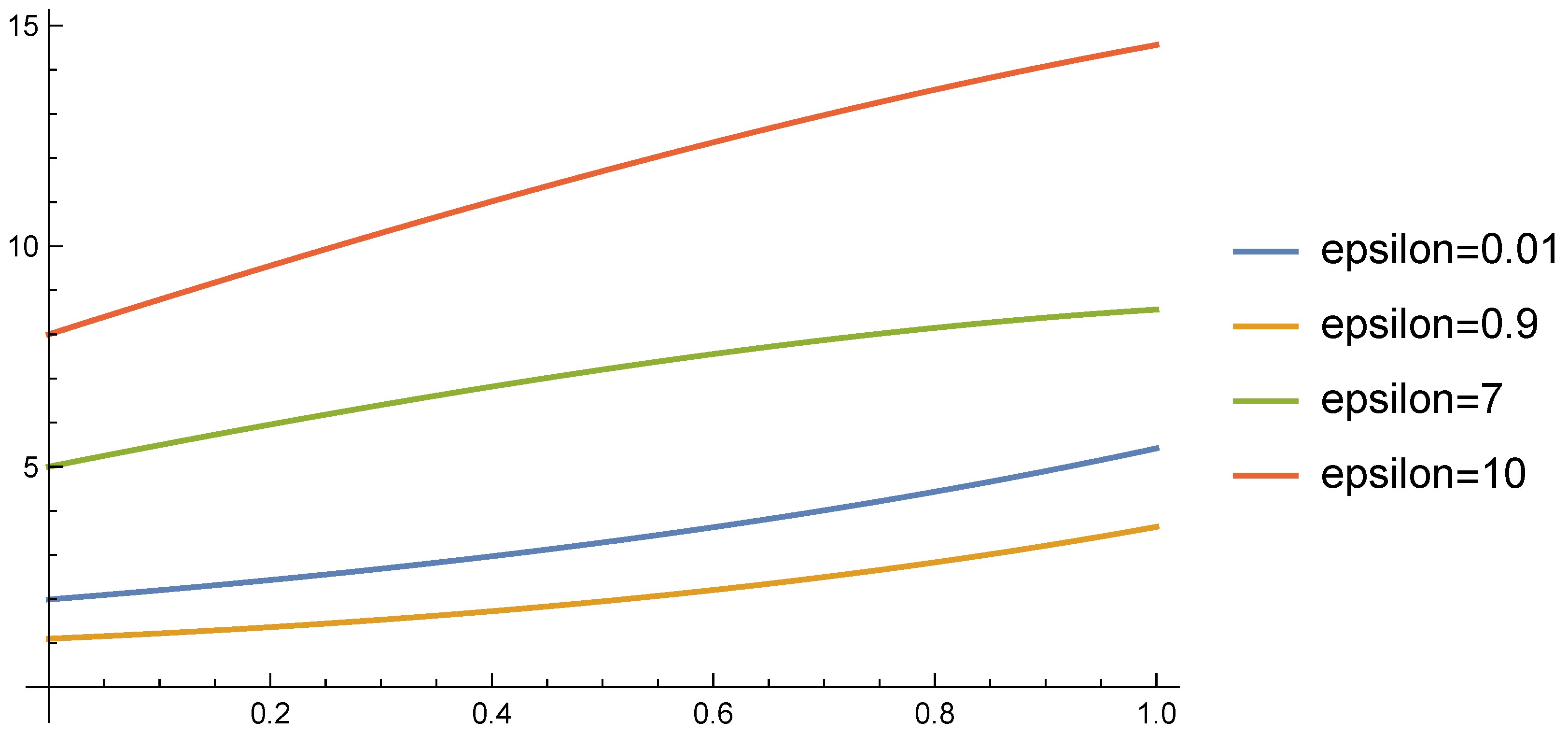
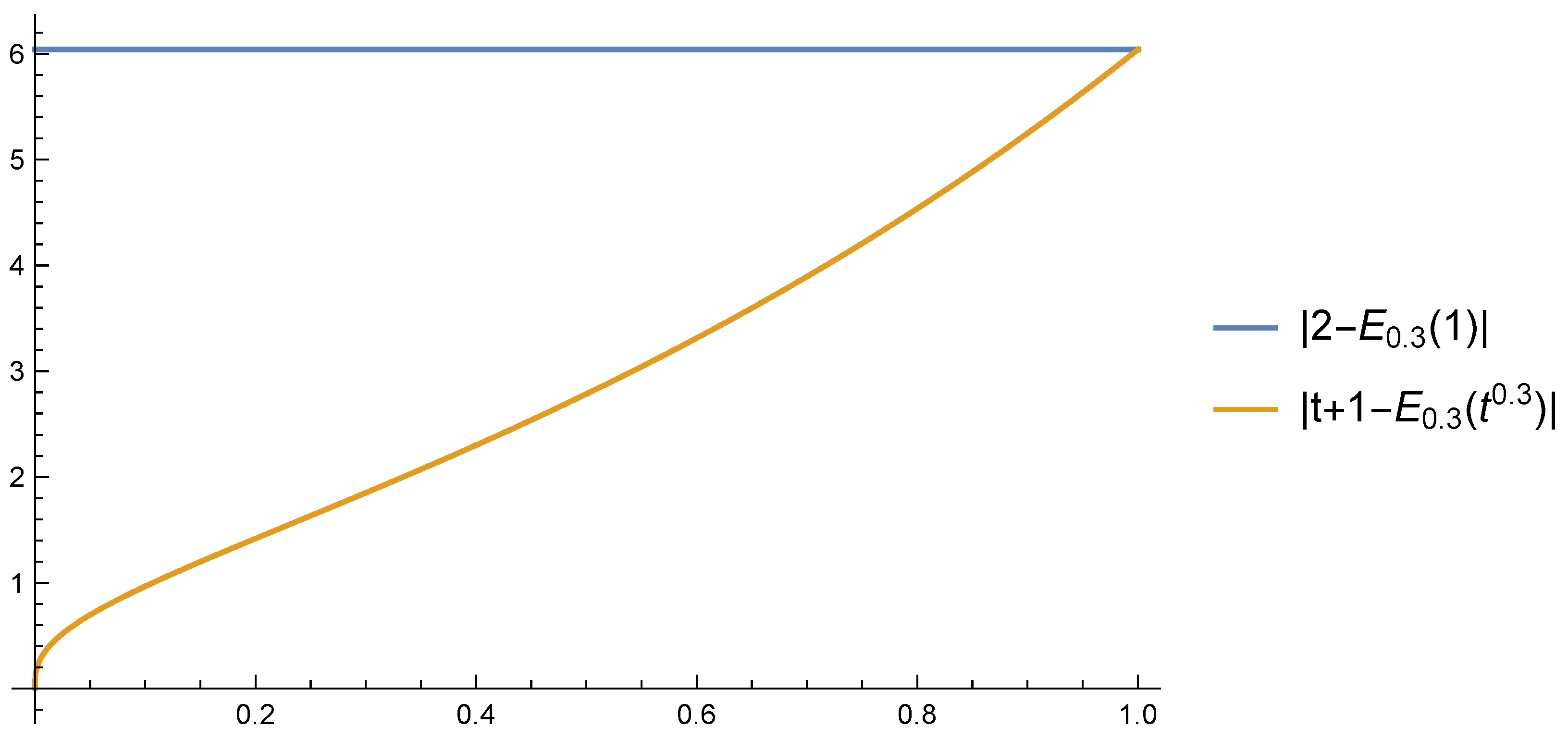
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Agarwal, R.P.; Hristova, S.; O’Regan, D. Ulam Stability for Boundary Value Problems of Differential Equations—Main Misunderstandings and How to Avoid Them. Mathematics 2024, 12, 1626. https://doi.org/10.3390/math12111626
Agarwal RP, Hristova S, O’Regan D. Ulam Stability for Boundary Value Problems of Differential Equations—Main Misunderstandings and How to Avoid Them. Mathematics. 2024; 12(11):1626. https://doi.org/10.3390/math12111626
Chicago/Turabian StyleAgarwal, Ravi P., Snezhana Hristova, and Donal O’Regan. 2024. "Ulam Stability for Boundary Value Problems of Differential Equations—Main Misunderstandings and How to Avoid Them" Mathematics 12, no. 11: 1626. https://doi.org/10.3390/math12111626
APA StyleAgarwal, R. P., Hristova, S., & O’Regan, D. (2024). Ulam Stability for Boundary Value Problems of Differential Equations—Main Misunderstandings and How to Avoid Them. Mathematics, 12(11), 1626. https://doi.org/10.3390/math12111626







