Equilibrium Figures for a Rotating Compressible Capillary Two-Layer Liquid
Abstract
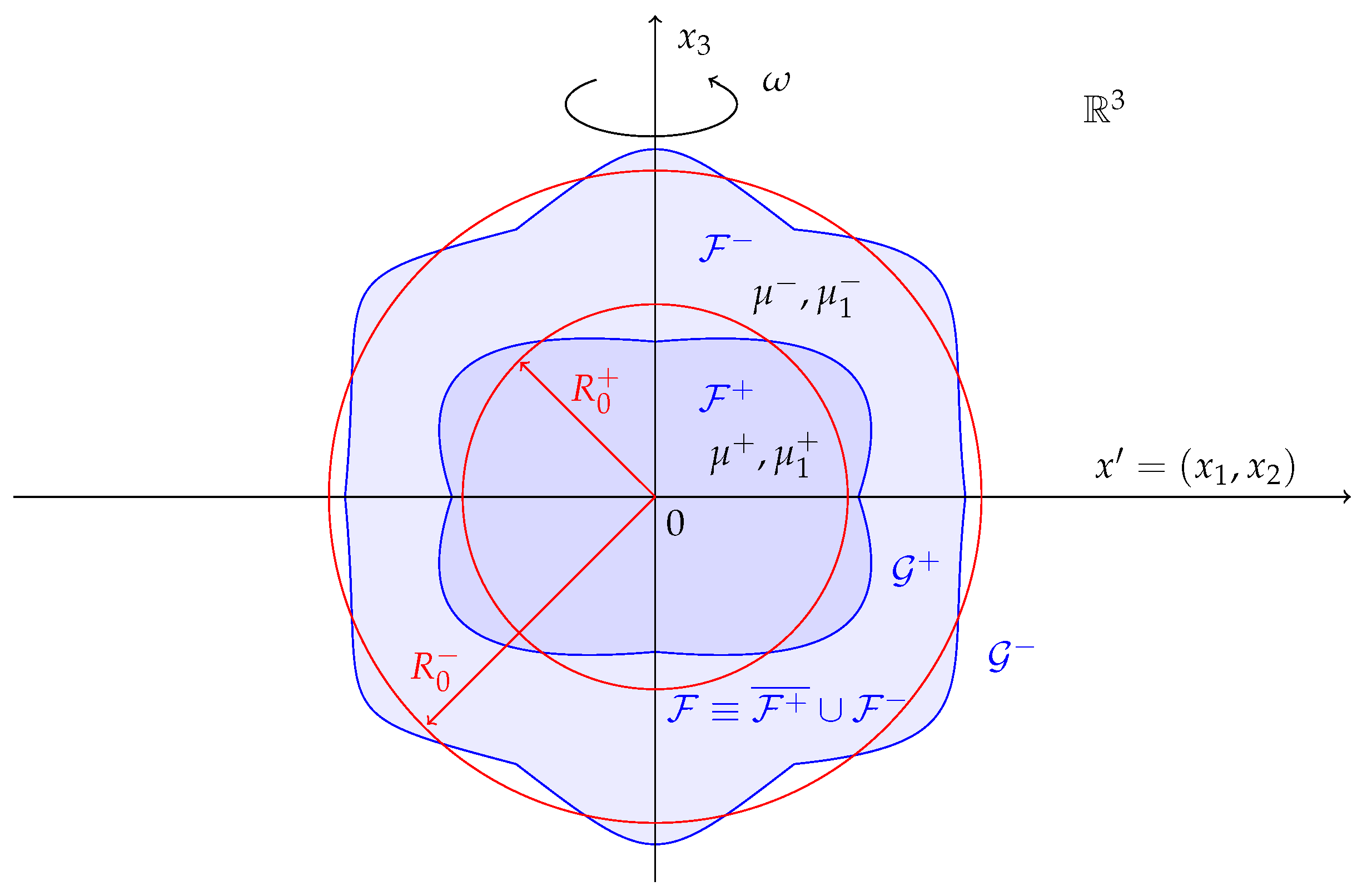
1. Introduction
2. Proof of Theorem 1
3. General Case
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Solonnikov, V.A.; Tani, A. Equilibrium figures of slowly rotating viscous compressible barotropic capillary liquid. Adv. Math. Sci. Appl. 1993, 2, 139–145. [Google Scholar]
- Lyapunov, A.M. On Stability of Ellipsoidal Shapes of Equilibrium of Revolving Liquid; Editiion of the Academy of Science: St. Petersburg, Russia, 1884. (In Russian) [Google Scholar]
- Lyapunov, A.M. Sur les Questions qui Appartiennent aux Surfaces des Figures D’equilibre Dérivées des Ellopsoïdes; News of the Academy of Sciences: St. Petersburg, Russia, 1916; p. 139. [Google Scholar]
- Poincaré, H. Figures D’équilibre D’une Mass Fluide; Gautier-Villars: Paris, France, 1902. [Google Scholar]
- Globa-Mikhailenko, B. Figures ellipsoïdales d’équilibre d’une masse fluide en rotation quand on tient compte de la pression capillaire. C. R. 1915, 160, 233. [Google Scholar]
- Charrueau, A. Ètude d’une masse liquide de révolution homogène, sans pesanteur et à tension superficielle, animée d’une rotation uniforme. Ann. Ècole Norm. Supérieure 1926, 43, 129–176. [Google Scholar] [CrossRef][Green Version]
- Charrueau, A. Sur les figures d’équilibre relatif d’une masse liquide en rotation à tension superficielle. C. R. 1927, 184, 1418. [Google Scholar][Green Version]
- Appell, P. Figures d’Équilibre d’une Mass Liquide Homogène en Rotation—Traité de Mécanique Rationnelle, v. IV, Fasc. I, 2nd ed.; Gautier-Villars: Paris, France, 1932. [Google Scholar][Green Version]
- Chandrasekhar, S. Ellipsoidal Figures of Equilibrium; Yale University Press: New Haven, CT, USA; London, UK, 1969. [Google Scholar][Green Version]
- Solonnikov, V.A. On the problem of non-stationary motion of two viscous incompressible liquids. Probl. Mat. Analiz. 2006, 34, 103–121, English translation in J. Math. Sci. 2007, 142, 1844–1866. [Google Scholar] [CrossRef]
- Pukhnachov, V.V. Motion of a Viscous Fluid with Free Boundaries; Textbook; Novosibirsk State University: Novosibirsk, Russia, 1989; 96p. (In Russian) [Google Scholar]
- Pukhnachov, V.V. Thermocapillary convection under low gravity. Fluid Dyn. Trans. 1989, 14, 145–204. [Google Scholar]
- Denisova, I.V. Problem on the motion of two compressible fluids separated by a closed free interface. Zap. Nauch. Semin. POMI 1997, 243, 61–86, English translation in J. Math. Sci. 2000, 99, 837–853. [Google Scholar] [CrossRef]
- Solonnikov, V.A.; Tani, A. Free boundary problem for a viscous compressible flow with surface tension. In Constantin Carathéodory: An International Tribute; World Scientific: Singapore, 1991; pp. 1270–1303. [Google Scholar]
- Kubo, T.; Shibata, Y.; Soga, K. On some two-phase problem for compressible and compressible viscous fluid flow separated by sharp interface. Discret. Contin. Dyn. Syst. Ser. S 2016, 36, 3741–3774. [Google Scholar] [CrossRef]
- Piasecki, T.; Zatorska, E. Maximal Regularity for Compressible Two-Fluid System. J. Math. Fluid Mech. 2022, 24, 39. [Google Scholar] [CrossRef]
- Denisova, I.V.; Solonnikov, V.A. Hölder Space Theory for the Rotation Problem of a Two-Phase Drop. Mathematics 2022, 10, 4799. [Google Scholar] [CrossRef]
- Blaschke, W. Vorlesungen über Differentialgeometrie und Geometrische Grundlagen von Einsteins Relativitätstheorie. I; Springer: Berlin/Heidelberg, Germany, 1924. [Google Scholar]
- Solonnikov, V.A. Unsteady motions of a finite isolated mass of a self-gravitating fluid. Algebra i Analiz 1989, 1, 207–249, English translation in Leningr. Math. J. 1990, 1, 227–276. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Denisova, I.V.; Solonnikov, V.A. Equilibrium Figures for a Rotating Compressible Capillary Two-Layer Liquid. Mathematics 2024, 12, 94. https://doi.org/10.3390/math12010094
Denisova IV, Solonnikov VA. Equilibrium Figures for a Rotating Compressible Capillary Two-Layer Liquid. Mathematics. 2024; 12(1):94. https://doi.org/10.3390/math12010094
Chicago/Turabian StyleDenisova, Irina Vladimirovna, and Vsevolod Alexeevich Solonnikov. 2024. "Equilibrium Figures for a Rotating Compressible Capillary Two-Layer Liquid" Mathematics 12, no. 1: 94. https://doi.org/10.3390/math12010094
APA StyleDenisova, I. V., & Solonnikov, V. A. (2024). Equilibrium Figures for a Rotating Compressible Capillary Two-Layer Liquid. Mathematics, 12(1), 94. https://doi.org/10.3390/math12010094





