Exact Solutions of the Bloch Equations to the Asymmetric Hyperbolic Cosine Pulse with Chirped Frequency
Abstract
1. Introduction
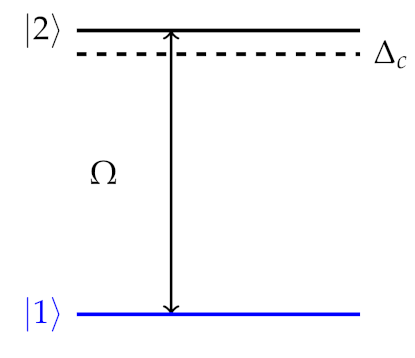
2. Model
3. The Exact Solutions: Method
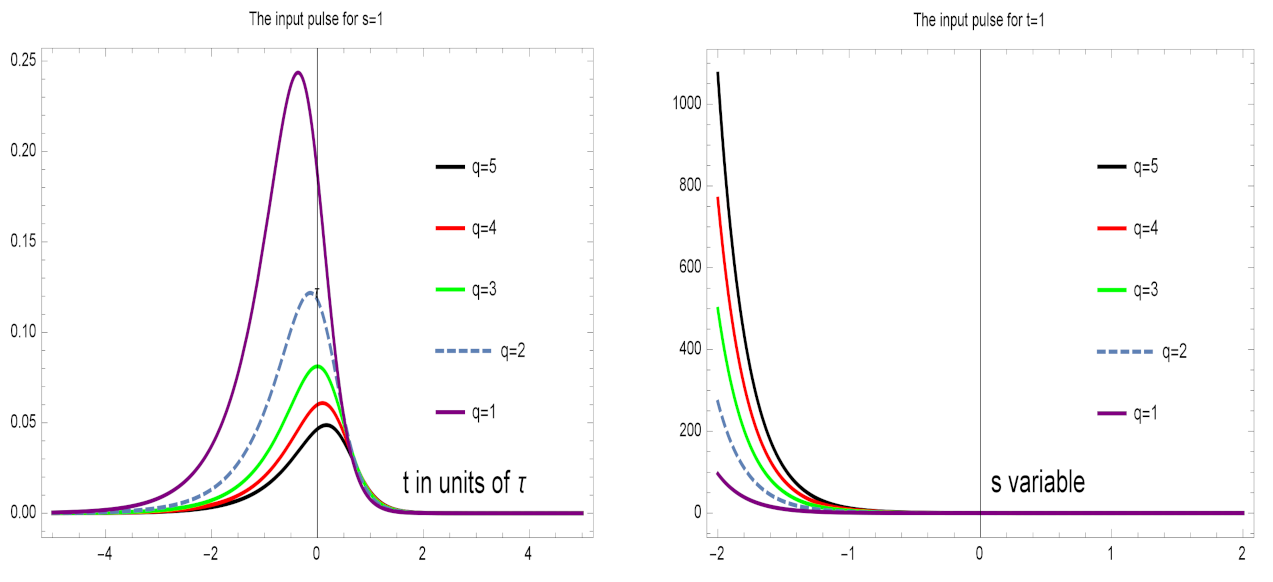
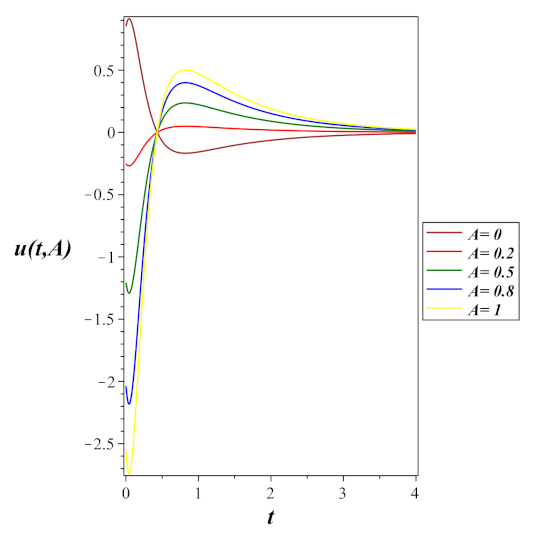
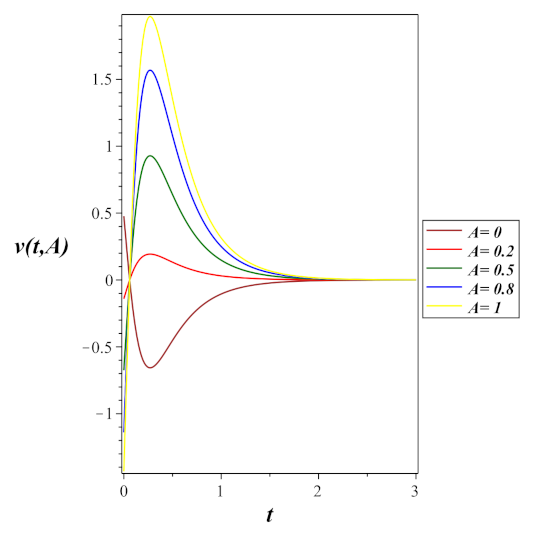
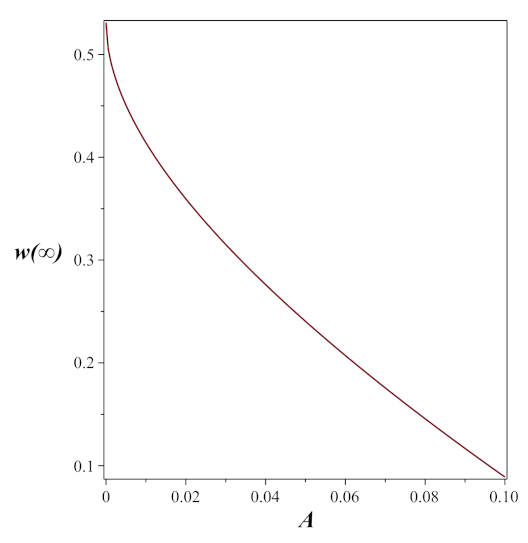
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bloch, F. Nuclear induction. Phys. Rev. 1946, 70, 460. [Google Scholar] [CrossRef]
- Slobodeniuk, A.; Koutenskỳ, P.; Bartoš, M.; Trojánek, F.; Malỳ, P.; Novotnỳ, T.; Kozák, M. Semiconductor Bloch equation analysis of optical Stark and Bloch-Siegert shifts in monolayer WSe 2 and MoS 2. Phys. Rev. B 2022, 106, 235304. [Google Scholar] [CrossRef]
- Boutabba, N. Kerr-effect analysis in a three-level negative index material under magneto cross-coupling. J. Opt. 2018, 20, 025102. [Google Scholar] [CrossRef]
- Wendler, F.; Knorr, A.; Malic, E. Carrier multiplication in graphene under Landau quantization. Nat. Commun. 2014, 5, 3703. [Google Scholar] [CrossRef] [PubMed]
- Liang, D.; Zhu, Y.; Li, H. Collective Resonance of D States in Rubidium Atoms Probed by Optical Two-Dimensional Coherent Spectroscopy. Phys. Rev. Lett. 2022, 128, 103601. [Google Scholar] [CrossRef] [PubMed]
- Zlatanov, K.N.; Vitanov, N.V. Adiabatic generation of arbitrary coherent superpositions of two quantum states: Exact and approximate solutions. Phys. Rev. A 2017, 96, 013415. [Google Scholar] [CrossRef]
- Schreiber, M.A.; Popp, J.; Seitner, L.; Haider, M.; Jirauschek, C. Implementation of Partially Reflecting Boundary Conditions in the Generalized Maxwell-Bloch Equations. In Proceedings of the 2022 International Conference on Numerical Simulation of Optoelectronic Devices (NUSOD), Turin, Italy, 12–16 September 2022; IEEE: Piscataway, NJ, USA, 2022; pp. 99–100. [Google Scholar]
- Ossiander, M.; Golyari, K.; Scharl, K.; Lehnert, L.; Siegrist, F.; Bürger, J.; Zimin, D.; Gessner, J.; Weidman, M.; Floss, I.; et al. The speed limit of optoelectronics. Nat. Commun. 2022, 13, 1620. [Google Scholar] [CrossRef]
- Allen, L.; Eberly, J.H. Optical Resonance and Two-Level Atoms; Courier Corporation: Chelmsford, MA, USA, 1975. [Google Scholar]
- Letokhov, V.S. Nonlinear Selective Photoprocesses in Atoms and Molecules; Moscow Izdatel Nauka: Moscow, Russia, 1983. [Google Scholar]
- Andreev, A.V.; Emel’yanov, V.I.; Il’inski, Y.A. Cooperative Phenomena in Optics. Science 1988. Available online: https://catalog.loc.gov/vwebv/search?searchCode=LCCN&searchArg=88162206&searchType=1&permalink=y (accessed on 23 February 2023).
- Singh, H.; Srivastava, H. Numerical simulation for fractional-order Bloch equation arising in nuclear magnetic resonance by using the Jacobi polynomials. Appl. Sci. 2020, 10, 2850. [Google Scholar] [CrossRef]
- Reeves, L.; Shaw, K. Nuclear magnetic resonance studies of multi-site chemical exchange. I. Matrix formulation of the Bloch equations. Can. J. Chem. 1970, 48, 3641–3653. [Google Scholar] [CrossRef]
- Xu, Z.; Zhou, Y.; Peng, X.; Li, L.; Qiu, X.; Zhou, M.; Xu, X. Measuring the enhancement factor of the hyperpolarized Xe in nuclear magnetic resonance gyroscopes. Phys. Rev. A 2021, 103, 023114. [Google Scholar] [CrossRef]
- Xiao, W.; Wu, T.; Peng, X.; Guo, H. Atomic spin-exchange collisions in magnetic fields. Phys. Rev. A 2021, 103, 043116. [Google Scholar] [CrossRef]
- Kocharovskaya, O.; Zhu, S.Y.; Scully, M.O.; Mandel, P.; Radeonychev, Y. Generalization of the Maxwell-Bloch equations to the case of strong atom-field coupling. Phys. Rev. A 1994, 49, 4928. [Google Scholar] [CrossRef] [PubMed]
- Wein, S.C.; Loredo, J.C.; Maffei, M.; Hilaire, P.; Harouri, A.; Somaschi, N.; Lemaître, A.; Sagnes, I.; Lanco, L.; Krebs, O.; et al. Photon-number entanglement generated by sequential excitation of a two-level atom. Nat. Photonics 2022, 16, 374–379. [Google Scholar] [CrossRef]
- Grira, S.; Boutabba, N.; Eleuch, H. Atomic population inversion in a two-level atom for shaped and chirped laser pulses: Exact solutions of Bloch equations with dephasing. Results Phys. 2021, 26, 104419. [Google Scholar] [CrossRef]
- Devi, A.; Gunapala, S.D.; Premaratne, M. Coherent and incoherent laser pump on a five-level atom in a strongly coupled cavity-QED system. Phys. Rev. A 2022, 105, 013701. [Google Scholar] [CrossRef]
- Boutabba, N.; Grira, S.; Eleuch, H. Atomic population inversion and absorption dispersion-spectra driven by modified double-exponential quotient pulses in a three-level atom. Results Phys. 2021, 24, 104108. [Google Scholar] [CrossRef]
- Zlatanov, K.N.; Vasilev, G.S.; Ivanov, P.A.; Vitanov, N.V. Exact solution of the Bloch equations for the nonresonant exponential model in the presence of dephasing. Phys. Rev. A 2015, 92, 043404. [Google Scholar] [CrossRef]
- Grira, S.; Boutabba, N.; Eleuch, H. Exact solutions of the Bloch equations of a two-level atom driven by the generalized double exponential quotient pulses with dephasing. Mathematics 2022, 10, 2105. [Google Scholar] [CrossRef]
- Boutabba, N.; Grira, S.; Eleuch, H. Analysis of a q-deformed hyperbolic short laser pulse in a multi-level atomic system. Sci. Rep. 2022, 12, 9308. [Google Scholar] [CrossRef]
- Zhang, J.; Garwood, M.; Park, J.Y. Full analytical solution of the bloch equation when using a hyperbolic-secant driving function. Magn. Reson. Med. 2017, 77, 1630–1638. [Google Scholar] [CrossRef] [PubMed]
- Silver, M.; Joseph, R.; Hoult, D. Selective spin inversion in nuclear magnetic resonance and coherent optics through an exact solution of the Bloch-Riccati equation. Phys. Rev. A 1985, 31, 2753. [Google Scholar] [CrossRef] [PubMed]
- Vasilev, G.; Ivanov, P.; Vitanov, N. Exact solution of the optical Bloch equation for the Demkov model. arXiv 2014, arXiv:1402.5648. [Google Scholar]
- Longmire, C.L.; Hamilton, R.M.; Hahn, J.M. A Nominal Set of High-Altitude EMP Environments; Technical Report; Mission Research Corp.: Santa Barbara, CA, USA; Oak Ridge National Lab.: Oak Ridge, TN, USA, 1987. [Google Scholar]
- Pretzler, G.; Kasper, A.; Witte, K. Angular chirp and tilted light pulses in CPA lasers. Appl. Phys. B 2000, 70, 1–9. [Google Scholar] [CrossRef]
- Wang, M.; Li, P.; Li, S.; Xu, Y.; Yao, C. Hundred-watt level average power CPA system with all-fiberized laser amplifiers based on CFBG stretcher and CVBG compressor. Optik 2022, 253, 168597. [Google Scholar] [CrossRef]
- Roeder, S.; Zobus, Y.; Brabetz, C.; Bagnoud, V. How the laser beam size conditions the temporal contrast in pulse stretchers of chirped-pulse amplification lasers. High Power Laser Sci. Eng. 2022, 10, e34. [Google Scholar] [CrossRef]
- Li, H.; MacArthur, J.; Littleton, S.; Dunne, M.; Huang, Z.; Zhu, D. Femtosecond-Terawatt Hard X-Ray Pulse Generation with Chirped Pulse Amplification on a Free Electron Laser. Phys. Rev. Lett. 2022, 129, 213901. [Google Scholar] [CrossRef]
- Mao, D.; He, Z.; Zhang, Y.; Du, Y.; Zeng, C.; Yun, L.; Luo, Z.; Li, T.; Sun, Z.; Zhao, J. Phase-matching-induced near-chirp-free solitons in normal-dispersion fiber lasers. Light Sci. Appl. 2022, 11, 25. [Google Scholar] [CrossRef]
- Ghotra, H.S. Laser wakefield and direct laser acceleration of electron by chirped laser pulses. Optik 2022, 260, 169080. [Google Scholar] [CrossRef]
- Meystre, P.; Scully, M.O. Quantum Optics; Springer: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Rogovin, D.; Scully, M.O. Does the “two-level atom” picture of a josephson junction have a theoretical foundation in BCS? Ann. Phys. 1974, 88, 371–396. [Google Scholar] [CrossRef]
- Jabri, H.; Eleuch, H. Enhanced unconventional photon-blockade effect in one-and two-qubit cavities interacting with nonclassical light. Phys. Rev. A 2022, 106, 023704. [Google Scholar] [CrossRef]
- Zhao, C.; Peng, R.; Yang, Z.; Chao, S.; Li, C.; Zhou, L. Atom-Mediated Phonon Blockade and Controlled-Z Gate in Superconducting Circuit System. Ann. Phys. 2021, 533, 2100039. [Google Scholar] [CrossRef]
- Glushkov, A.V. Relativistic Quantum Theory. Quantum Mechanics of Atomic Systems; Astroprint: Odessa, Ukraine, 2008. [Google Scholar]
- Shore, B.W. The Theory of Coherent Atomic Excitation. In two volumes. Vol. 1, Simple Atoms and Fields. Vol. 2, Multilevel Atoms and Incoherence. Science 1990, 250, 1735. [Google Scholar]
- Chollangi, A.; Ravi; Krishnan, N.; Bhowmick, K. An Investigation of Transmission Properties of Double-Exponential Pulses in Core-Clad Optical Fibers for Communication Application. In Lecture Notes of the Institute for Computer Sciences, Social Informatics and Telecommunications Engineering; Springer International Publishing: Cham, Switzerland, 2019; Volume 276. [Google Scholar]
- Chollangi, A.; Thakur, A.K.; Rakesh, R.; Alharbi, S.; Bhowmick, K. Investigation of transmission properties of a practical double exponential pulse for communication and sensing application. Optik 2022, 255, 168735. [Google Scholar] [CrossRef]
- Arkhipov, M.V.; Arkhipov, R.M.; Pakhomov, A.V.; Babushkin, I.V.; Demircan, A.; Morgner, U.; Rosanov, N.N. Generation of unipolar half-cycle pulses via unusual reflection of a single-cycle pulse from an optically thin metallic or dielectric layer. Opt. Lett. 2017, 42, 2189–2192. [Google Scholar] [CrossRef] [PubMed]
- Arkhipov, R.; Arkhipov, M.; Pakhomov, A.; Babushkin, I.; Rosanov, N. Half-cycle and unipolar pulses (Topical Review). Laser Phys. Lett. 2022, 19, 043001. [Google Scholar] [CrossRef]
- Morrow, D.J.; Ma, X. Rapid and facile reconstruction of time-resolved fluorescence data with exponentially modified Gaussians. Opt. Open, 2022; preprint. [Google Scholar]
- Abdalla, M.S.; Eleuch, H. Exact analytic solutions of the Schrödinger equations for some modified q-deformed potentials. J. Appl. Phys. 2014, 115, 234906. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grira, S.; Boutabba, N.; Eleuch, H. Exact Solutions of the Bloch Equations to the Asymmetric Hyperbolic Cosine Pulse with Chirped Frequency. Mathematics 2023, 11, 2159. https://doi.org/10.3390/math11092159
Grira S, Boutabba N, Eleuch H. Exact Solutions of the Bloch Equations to the Asymmetric Hyperbolic Cosine Pulse with Chirped Frequency. Mathematics. 2023; 11(9):2159. https://doi.org/10.3390/math11092159
Chicago/Turabian StyleGrira, Sofiane, Nadia Boutabba, and Hichem Eleuch. 2023. "Exact Solutions of the Bloch Equations to the Asymmetric Hyperbolic Cosine Pulse with Chirped Frequency" Mathematics 11, no. 9: 2159. https://doi.org/10.3390/math11092159
APA StyleGrira, S., Boutabba, N., & Eleuch, H. (2023). Exact Solutions of the Bloch Equations to the Asymmetric Hyperbolic Cosine Pulse with Chirped Frequency. Mathematics, 11(9), 2159. https://doi.org/10.3390/math11092159





