A Planar-3D Mathematical Model for Studying the Effect of Heterogeneity of Rock Fracture Toughness on Hydraulic Fracture Propagation: Early-Time Solution including the Stage before Propagation
Abstract
1. Introduction
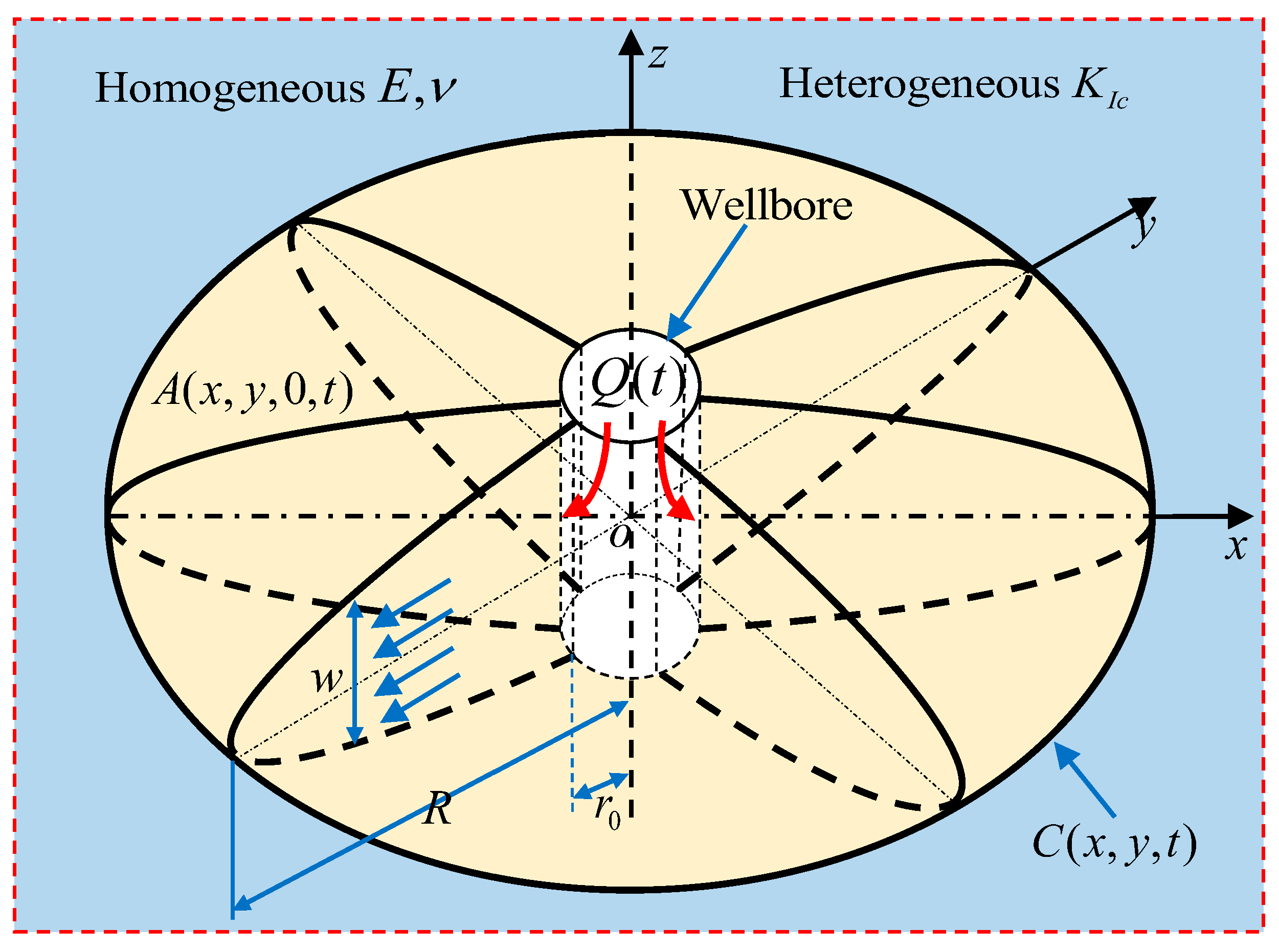
2. Mathematical Model
2.1. Assumptions
- (1)
- In the solid medium (rocks), there is only one planar fracture, and it propagates only on this plane and will not be out of this plane.
- (2)
- The solid medium is a linear elastic material with homogeneous Young’s modulus , Poisson’s ratio , and the heterogeneous fracture toughness .
- (3)
- The fluid flow is governed by lubrication theory, and the hydraulic fluid is incompressible and Newtonian, with a viscosity .
- (4)
- The fracture propagation is controlled by the rock fracture toughness according to the linear elastic fracture mechanics.
- (5)
- The in situ stress perpendicular to the fracture plane is homogeneous, and the fluid leak-off and the effect of gravity are neglected.
- (6)
- The fluid front coincides with the fracture front because of the negligible lag between the two fronts [39].
- (7)
- The initial fracture has a penny-shaped footprint with a radius , the formation process of which is not considered in this model.
2.2. Governing Equations
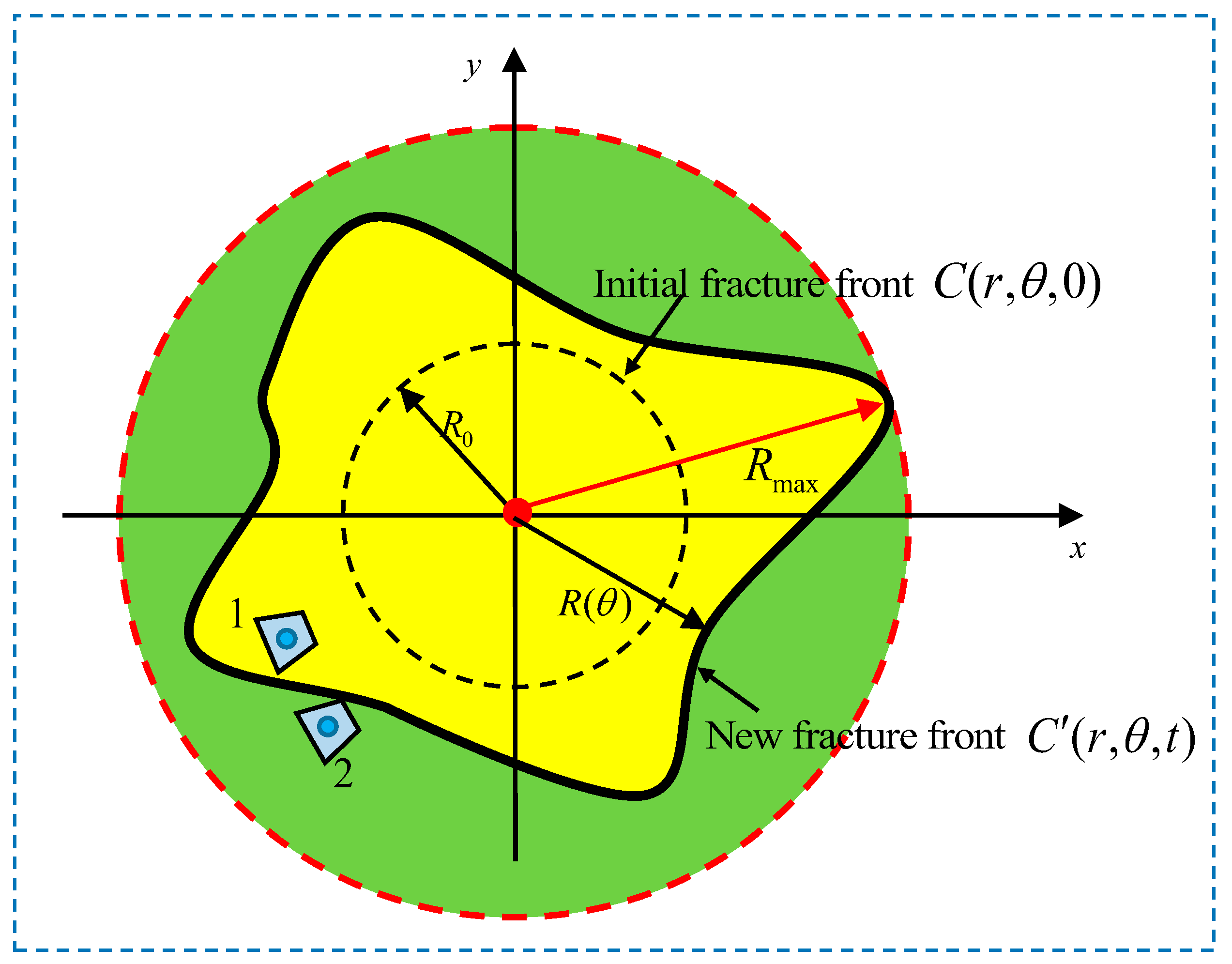
2.3. Boundary and Initial Conditions
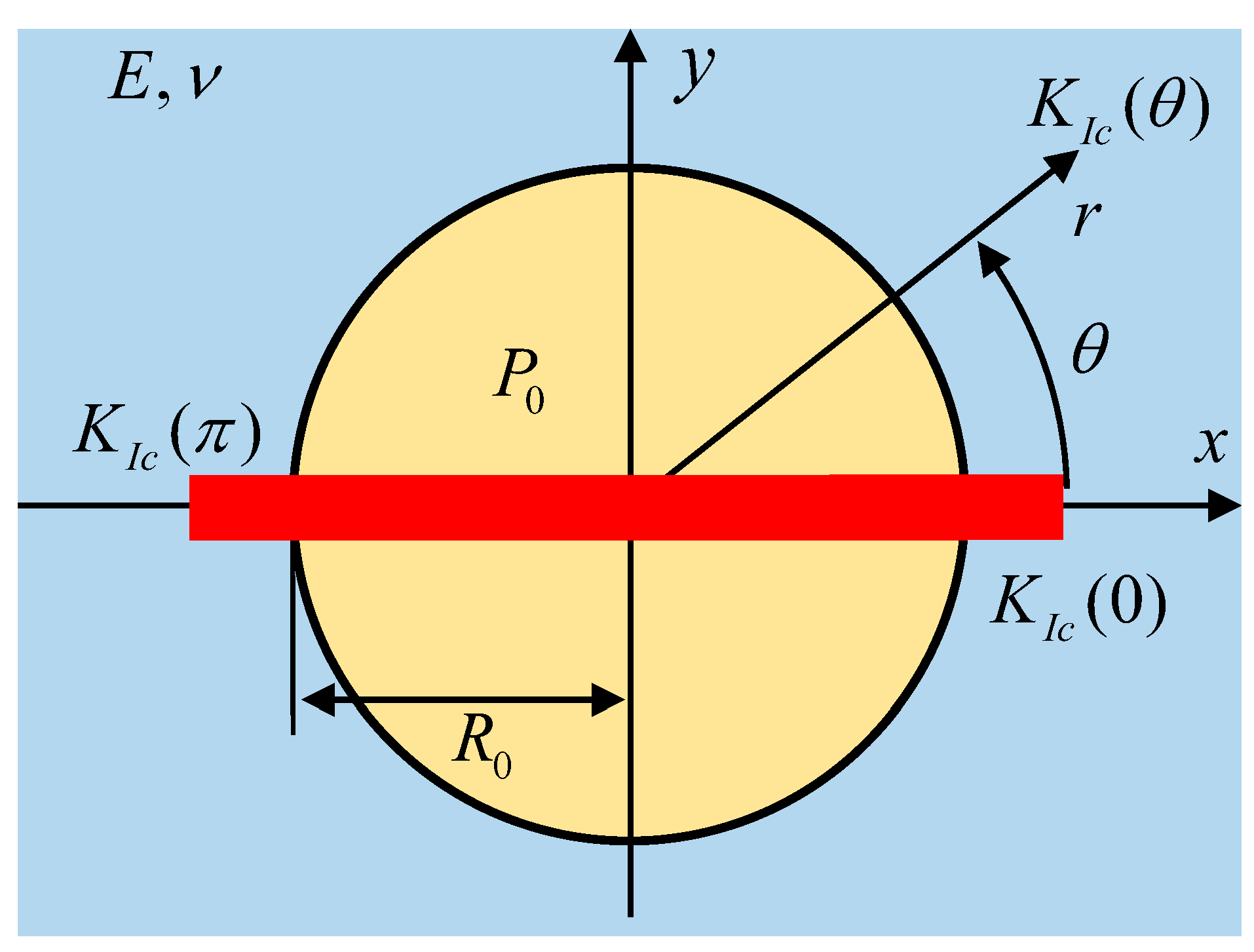
2.4. Distribution of Rock Toughness
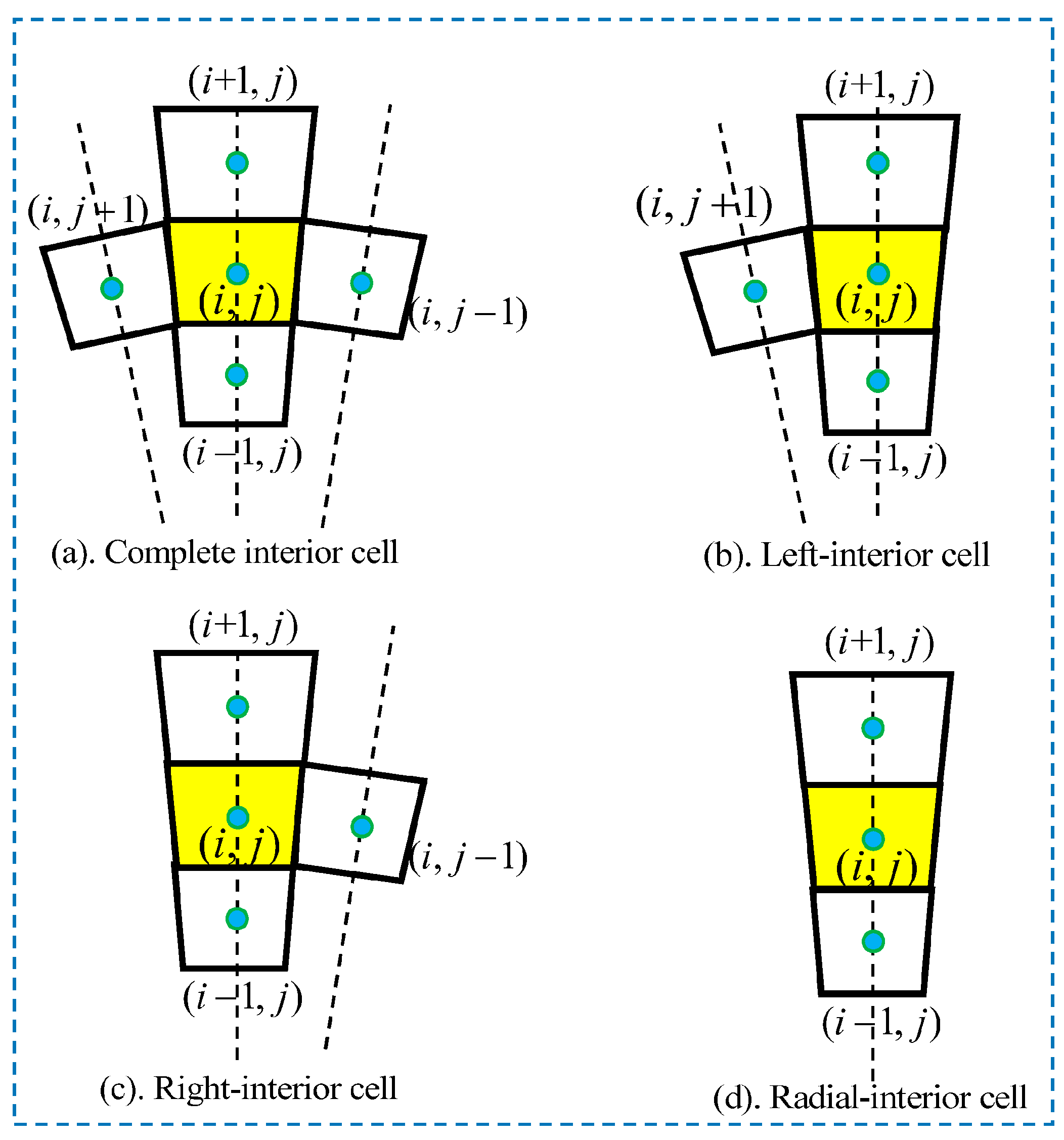
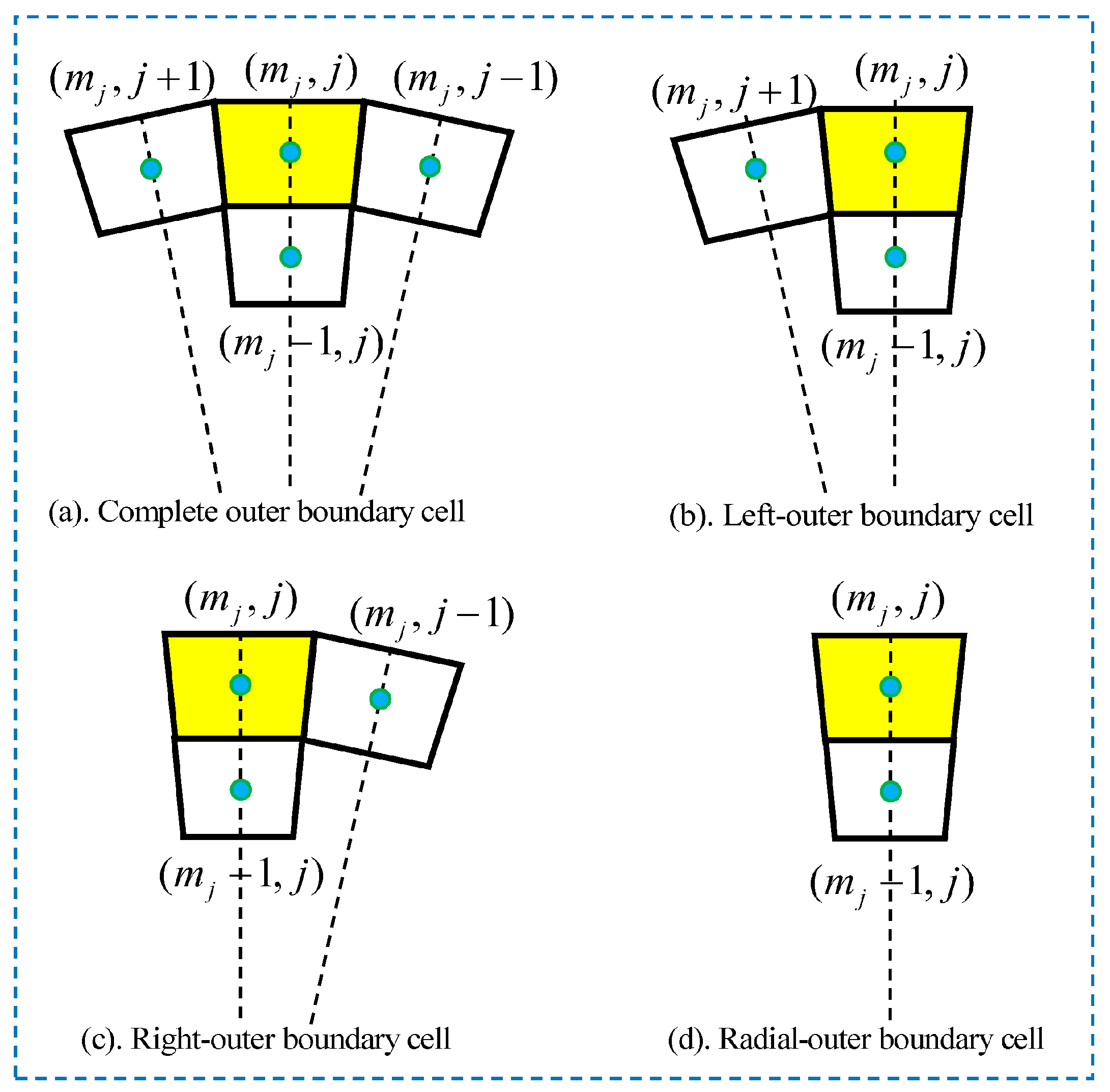
3. Model Discretization
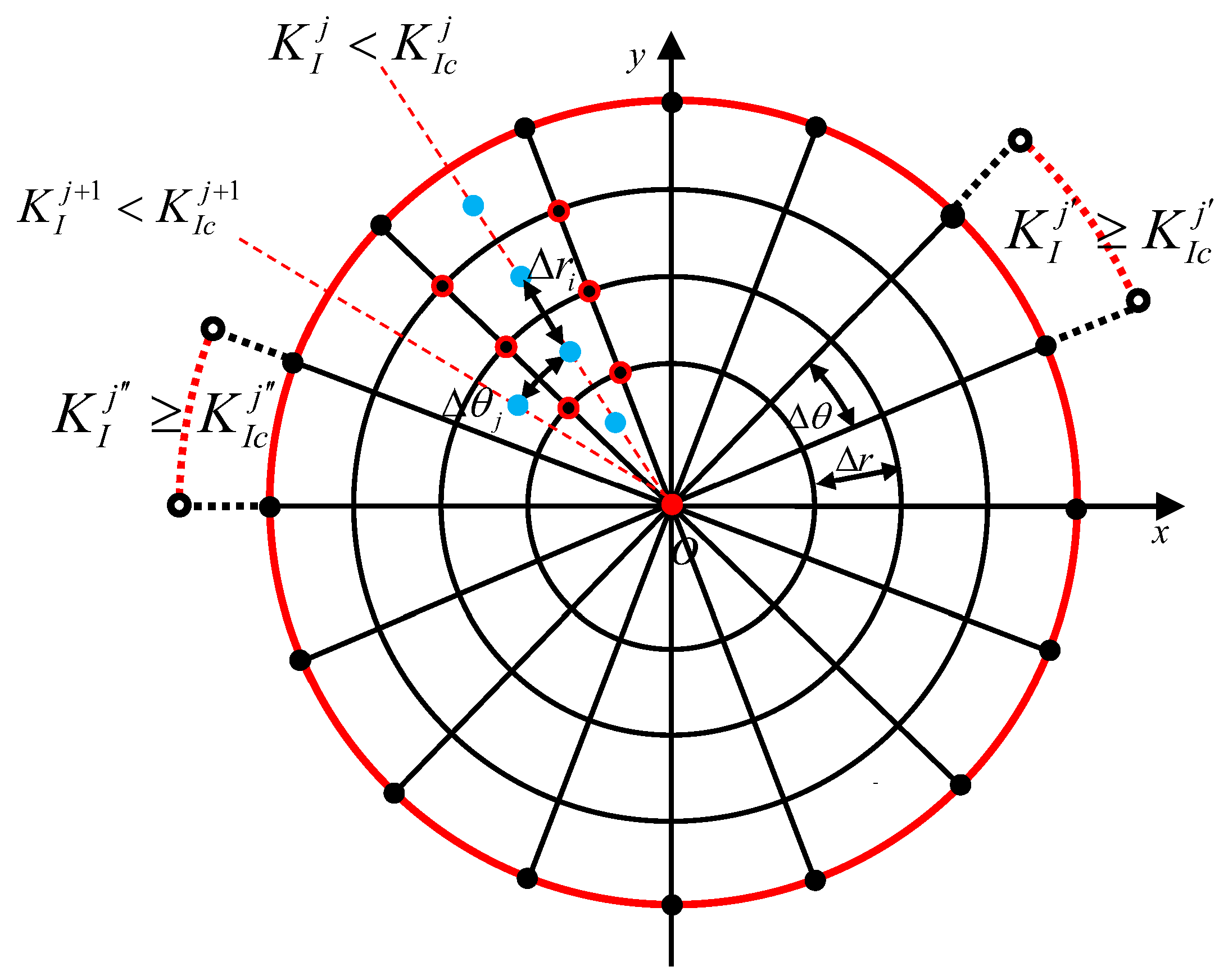
3.1. Fracture Discretization and Propagation
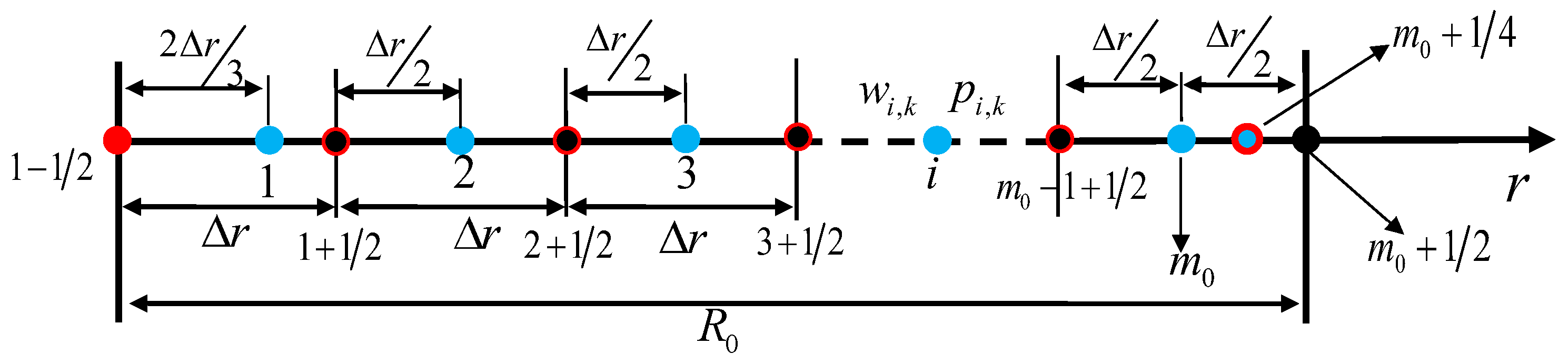
3.2. Discretization of Governing Equations
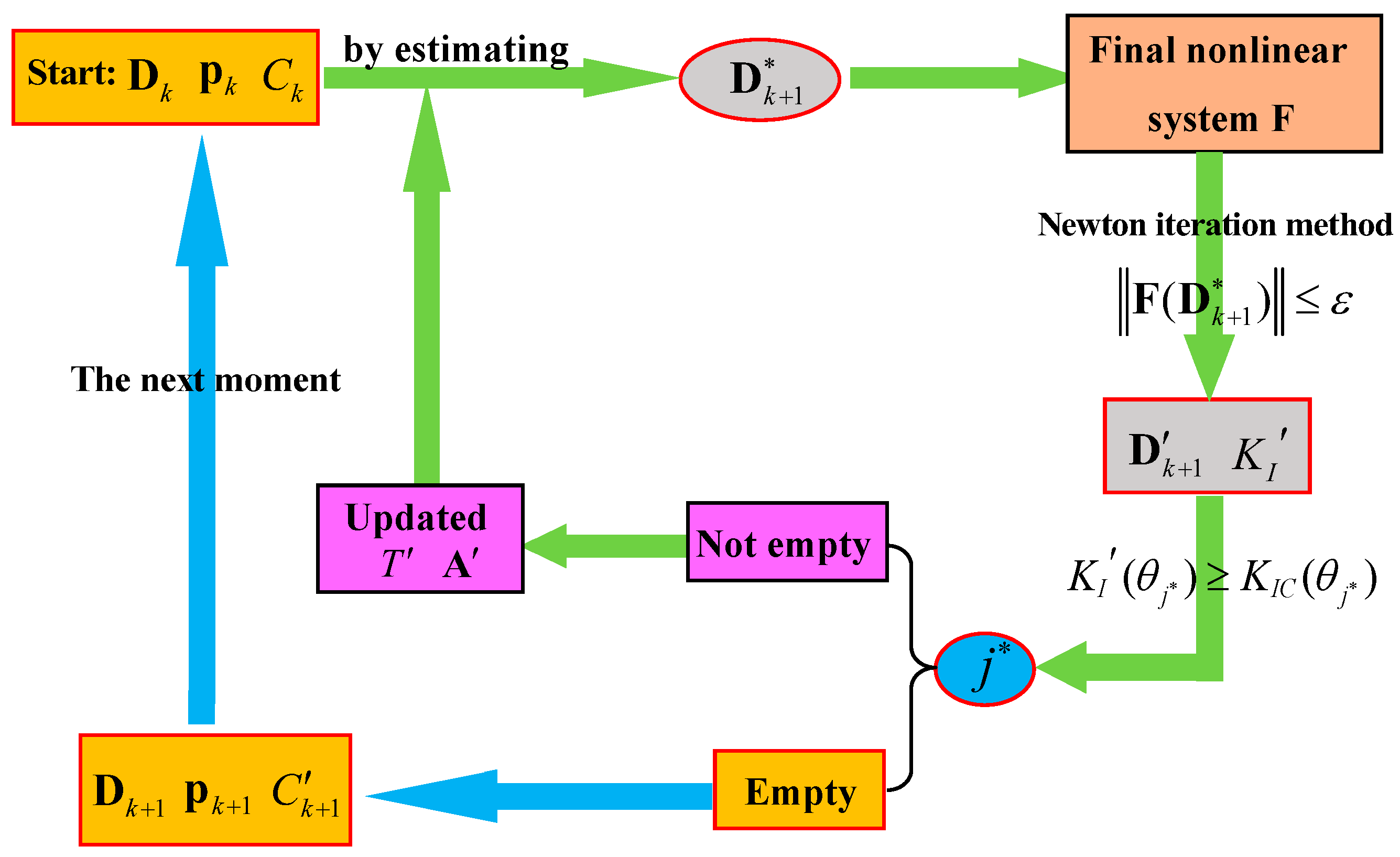
4. Numerical Method for Model Solutions
4.1. The Stage before Propagation
4.2. The Stage of Propagation
5. The Implicit Algorithm and Verification
5.1. Algorithm
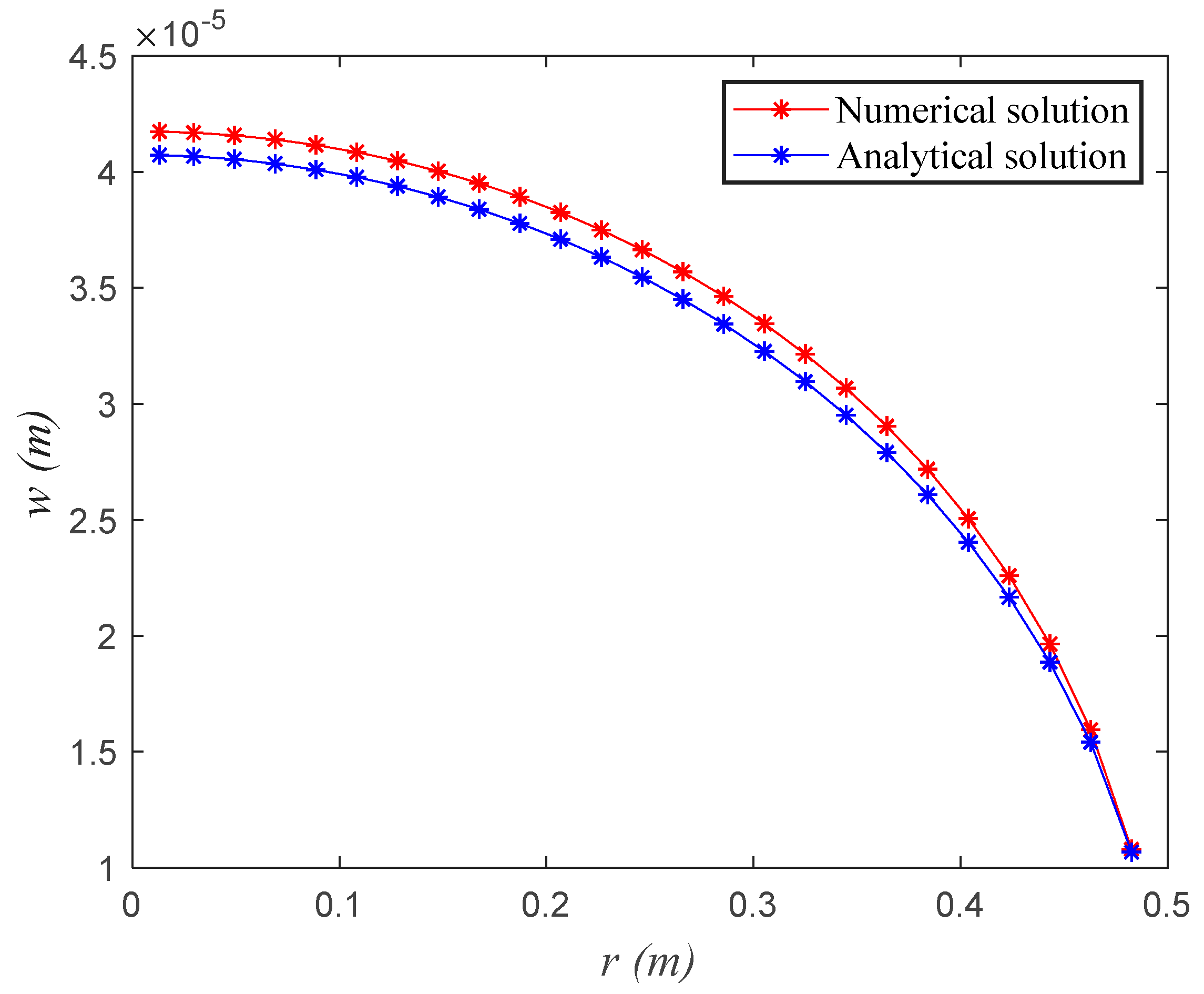
5.2. Verification of the Algorithm
6. Numerical Results and Analysis
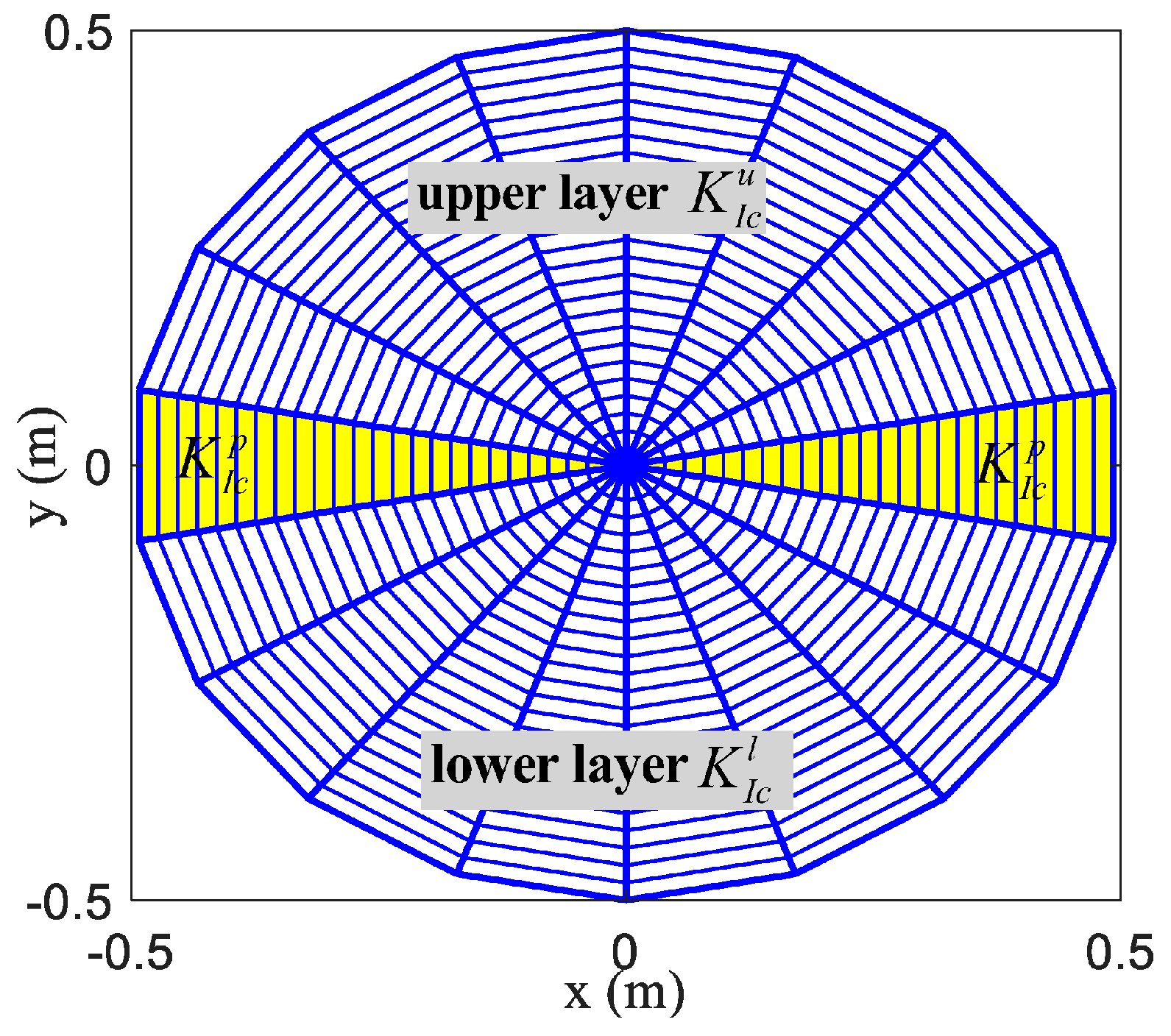
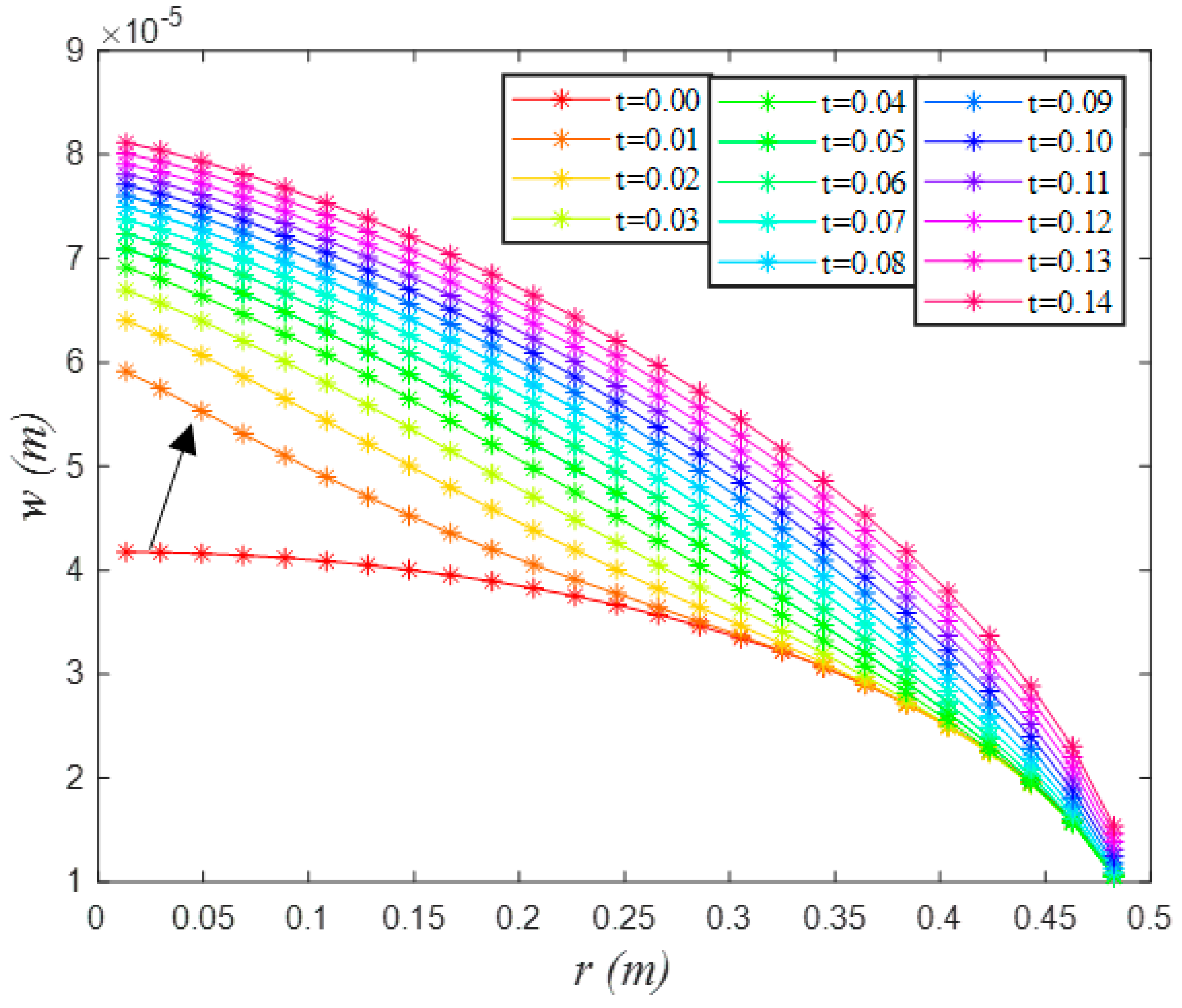
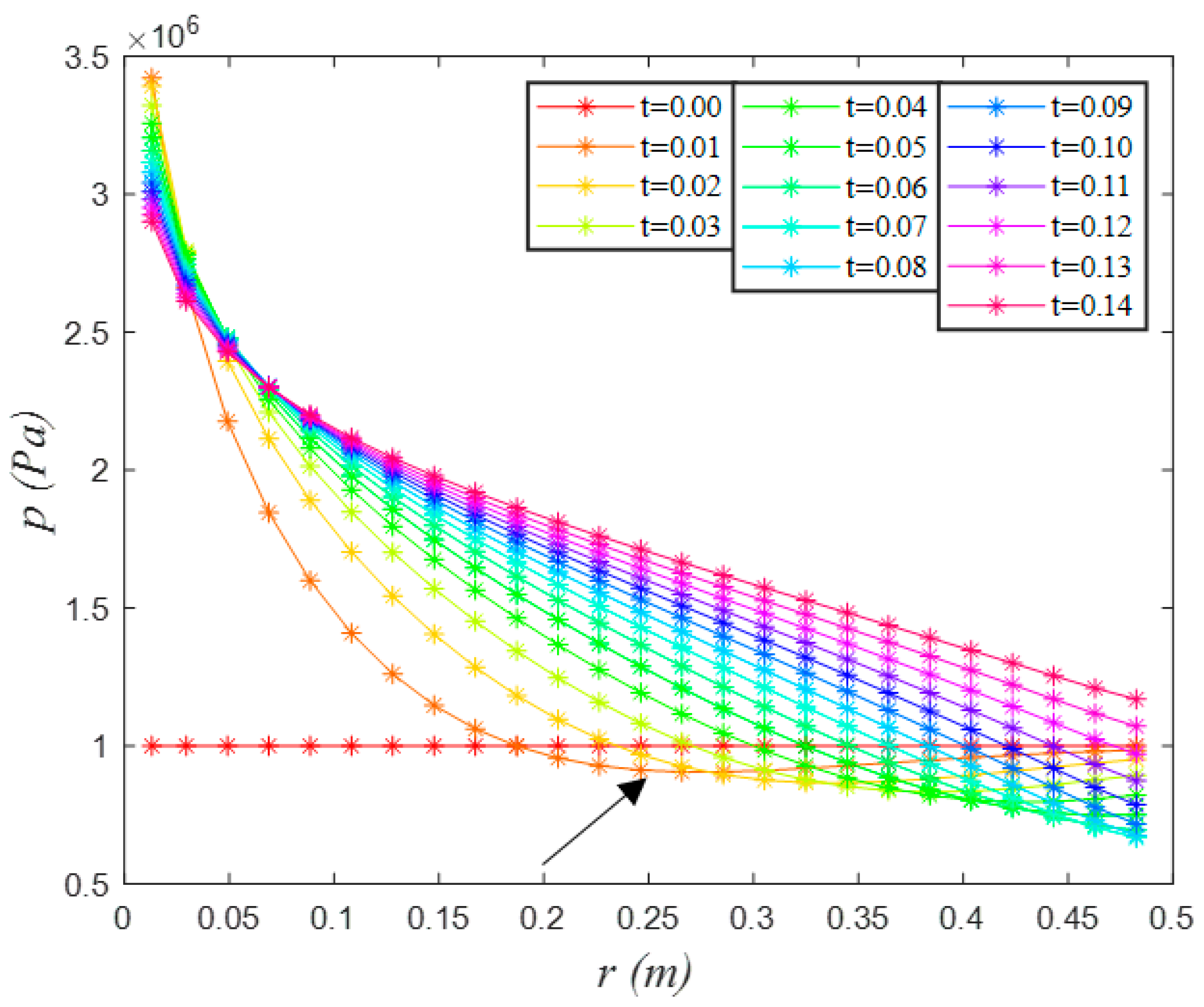
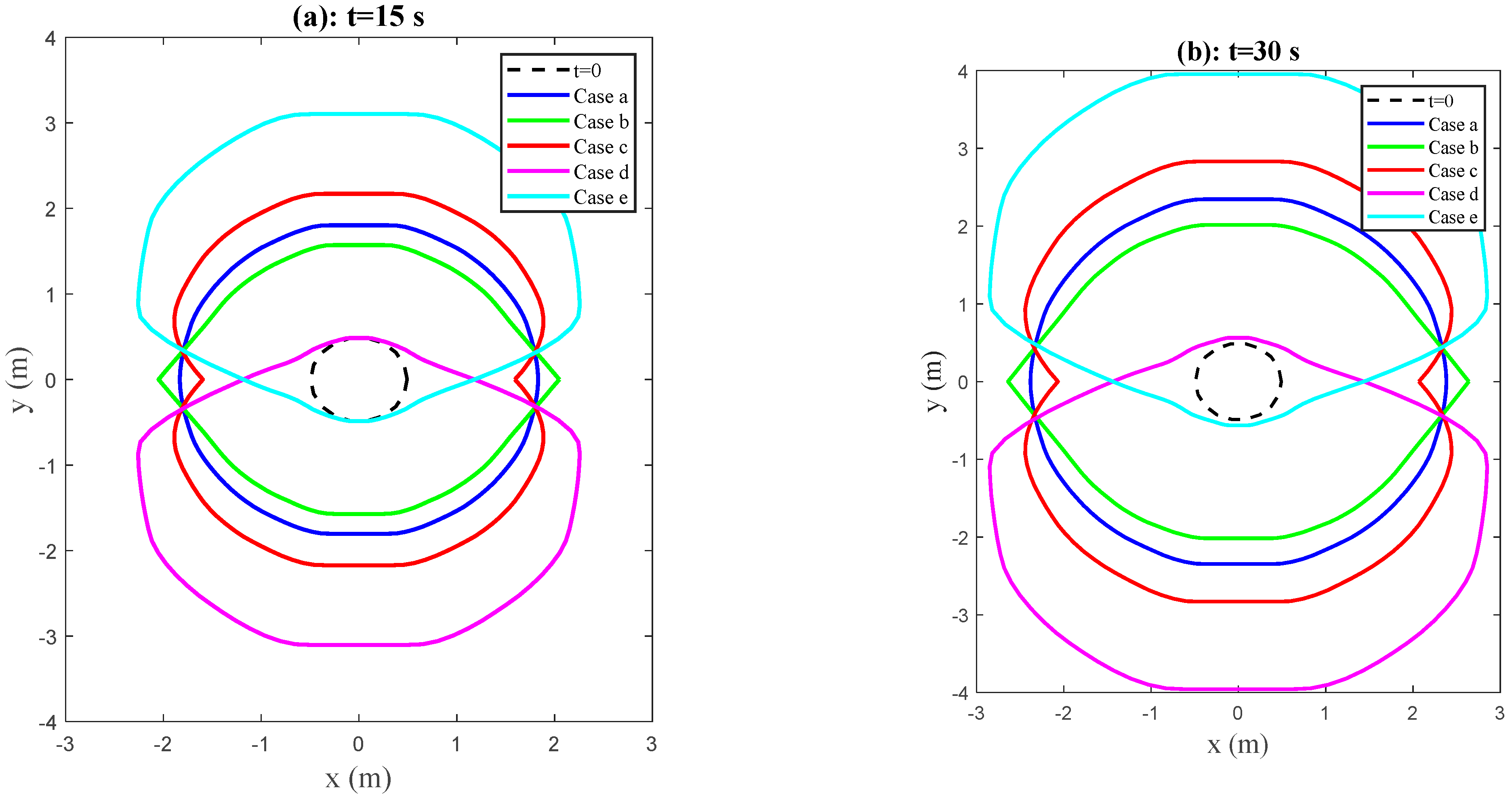
6.1. Numerical Results under the Weak Pay Zone and Symmetric Adjacent Layers
6.2. Results of Numerical Comparison Experiments
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Influence Function of 3D-DDM
Appendix B. Specific Discrete Forms of Governing Equations in the Stage before Fracture Propagation
Appendix C. Specific Discrete Forms of Governing Equations in the Stage of Fracture Propagation
References
- Klee, G.; Rummel, F.; Williams, A. Hydraulic fracturing stress measurements in Hong Kong. Int. J. Rock Mech. Min. Sci. 1999, 36, 731–741. [Google Scholar] [CrossRef]
- Serdyukov, S.V.; Kurlenya, M.V.; Patutin, A.V. Hydraulic fracturing for in situ stress measurement. J. Min. Sci. 2016, 52, 1031–1038. [Google Scholar] [CrossRef]
- Cheng, Y.; Zhang, Y. Hydraulic fracturing experiment investigation for the application of geothermal energy extraction. ACS Omega 2020, 5, 8667–8686. [Google Scholar] [CrossRef]
- Ren, X.; Zhou, L.; Zhou, J.; Lu, Z.; Su, X. Numerical analysis of heat extraction efficiency in a multilateral-well enhanced geothermal system considering hydraulic fracture propagation and configuration. Geothermics 2020, 87, 101834. [Google Scholar] [CrossRef]
- He, Q.; Suorineni, F.T.; Oh, J. Strategies for creating prescribed hydraulic fractures in cave mining. Rock Mech. Rock Eng. 2017, 50, 967–993. [Google Scholar] [CrossRef]
- Birch, D.J.; Meyer, S.G. Seismic Response to hydraulic fracturing in caving mines. In Proceedings of the Ninth International Conference on Deep and High Stress Mining, Johannesburg, South Africa, 24–25 June 2019; pp. 445–460. [Google Scholar]
- He, Q.; Suorineni, F.T.; Ma, T.; Oh, J. Parametric study and dimensional analysis on prescribed hydraulic fractures in cave mining. Tunn. Undergr. Space Technol. 2018, 78, 47–63. [Google Scholar] [CrossRef]
- Economides, M.J.; Nolte, K.G. Reservoir Stimulation, 3rd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2000. [Google Scholar]
- Batchelor, G.K. An Introduction to Fluid Dynamics; Cambridge University Press: Cambridge, UK, 1967. [Google Scholar]
- Sneddon, N.I. The distribution of stress in the neighbourhood of a crack in an elastic solid. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1946, 187, 229–260. [Google Scholar]
- Sneddon, N.I.; Lowengrub, M. Crack Problems in the Classical Theory of Elasticity; John Wiley & Sons: Hoboken, NJ, USA, 1969. [Google Scholar]
- Zeng, Q.; Yao, J.; Shao, J. Numerical study of hydraulic fracture propagation accounting for rock anisotropy. J. Pet. Sci. Eng. 2018, 160, 422–432. [Google Scholar] [CrossRef]
- Wang, X.; Shi, F.; Liu, H.; Wu, H. Numerical simulation of hydraulic fracturing in orthotropic formation based on the extended finite element method. J. Nat. Gas Sci. Eng. 2016, 33, 56–69. [Google Scholar] [CrossRef]
- Chen, B.; Cen, S.; Barron, A.R.; Owen, D.R.J.; Li, C. Numerical investigation of the fluid lag during hydraulic fracturing. Eng. Comput. 2018, 35, 2050–2077. [Google Scholar] [CrossRef]
- Baykin, A.N.; Golovin, S.V. Modelling of hydraulic fracture propagation in inhomogeneous poroelastic medium. J. Phys. Conf. Ser. 2016, 722, 012003. [Google Scholar] [CrossRef]
- Peirce, A.; Detournay, E. An implicit level set method for modelling hydraulically driven fractures. Comput. Methods Appl. Mech. Eng. 2008, 197, 2858–2885. [Google Scholar] [CrossRef]
- Dontsov, E.V.; Peirce, A.P. A multiscale implicit level set algorithm (ILSA) to model hydraulic fracture propagation incorporating combined viscous, toughness, and leak-off asymptotics. Comput. Methods Appl. Mech. Eng. 2017, 313, 53–84. [Google Scholar] [CrossRef]
- Li, K.; Jiang, X.; Ding, H.; Hu, X. Three-dimensional propagation simulation and parameter analysis of rock joint with displacement discontinuity method. Math. Probl. Eng. 2019, 2019, 3164817. [Google Scholar] [CrossRef]
- Yamamoto, K.; Shimamoto, T.; Sukemura, S. Multiple fracture propagation model for a three-dimensional hydraulic fracturing simulator. Int. J. Geomech. 2004, 4, 46–57. [Google Scholar] [CrossRef]
- Salimzadeh, S.; Usui, T.; Paluszny, A.; Zimmerman, R.W. Finite element simulations of interactions between multiple hydraulic fractures in a poroelastic rock. Int. J. Rock Mech. Min. Sci. 2017, 99, 9–20. [Google Scholar] [CrossRef]
- Li, Q.; Xing, H.; Liu, J.; Liu, X. A review on hydraulic fracturing of unconventional reservoir. Petroleum 2015, 1, 8–15. [Google Scholar] [CrossRef]
- Slatt, R.M. Important geological properties of unconventional resource shales. Cent. Eur. J. Geosci. 2011, 3, 435–448. [Google Scholar] [CrossRef]
- Warpinski, N.R.; Clark, J.A.; Schmidt, R.A.; Huddle, C.W. Laboratory investigation on the effect on in-situ stresses on hydraulic fracture containment. Soc. Pet. Eng. J. 1982, 22, 333–340. [Google Scholar] [CrossRef]
- Fung, R.L.; Vijayakumar, S.; Cormack, D.E. Calculation of vertical fracture containment in layered formations. SPE Form. Eval. 1987, 2, 518–522. [Google Scholar] [CrossRef]
- Smith, M.B.; Bale, A.B.; Britt, L.K.; Klein, H.H.; Siebrits, E.; Dang, X. Layered modulus effects on fracture propagation, proppant placement, and fracture modelling. In Proceedings of the SPE Annual Technical Conference and Exihibition, New Orleans, LA, USA, 30 September–3 October 2001; Society of Petroleum Engineers: Dallas, TX, USA, 2001. SPE 71654. [Google Scholar]
- Van Eekelen, H.A.M. Hydraulic fracture geometry: Fracture containment in layered formations. Soc. Pet. Eng. J. 1982, 22, 341–349. [Google Scholar] [CrossRef]
- Golovin, S.V.; Baykin, A.N. Influence of pore pressure on the development of a hydraulic fracture in poroelastic medium. Int. J. Rock Mech. Min. Sci. 2018, 108, 198–208. [Google Scholar] [CrossRef]
- Gao, Q.; Han, S.; Cheng, Y.; Yan, C.; Sun, Y.; Han, Z. Effects of non-uniform pore pressure field on hydraulic fracture propagation behaviors. Eng. Fract. Mech. 2019, 221, 106682. [Google Scholar] [CrossRef]
- Thiercelin, M.; Jeffrey, R.G.; Naceur, K.B. Influence of fracture toughness on the geometry of hydraulic fractures. SPE Prod. Eng. 1989, 4, 435–442. [Google Scholar] [CrossRef]
- Suo, Y.; Chen, Z.; Rahman, S.S.; Song, H. Experimental and numerical investigation of the effect of bedding layer orientation on fracture toughness of shale rocks. Rock Mech. Rock Eng. 2020, 53, 3625–3635. [Google Scholar] [CrossRef]
- Chandler, M.R.; Meredith, P.G.; Brantut, N.; Crawford, B.R. Fracture toughness anisotropy in shale. J. Geophys. Res. Solid Earth 2016, 121, 1706–1729. [Google Scholar] [CrossRef]
- Trimonova, M.A.; Zenchenko, E.V.; Turuntaev, S.B.; Golovin, Y.I.; Samodurov, A.A.; Tyurin, A.I.; Dubinya, N.V. Rock toughness importance for hydraulic fracture modelling. AIP Conf. Proc. 2018, 2050, 020308. [Google Scholar]
- Siebrits, E.; Peirce, A.P. An efficient multi-layer planar 3D fracture growth algorithm using a fixed mesh approach. Int. J. Numer. Methods Eng. 2002, 53, 691–717. [Google Scholar] [CrossRef]
- Budennyy, S. An enhanced pseudo-3D model of hydraulic fracture in multi-layered formation. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 9–11 October 2017. [Google Scholar]
- Zhang, X.; Wu, B.; Jeffrey, R.G.; Connel, L.D.; Zhang, G. A pseudo-3D model for hydraulic fracture growth in a layered rock. Int. J. Solids Struct. 2017, 115–116, 208–223. [Google Scholar] [CrossRef]
- Zhang, X.; Wu, B.; Connel, L.D.; Han, Y.; Jeffrey, R.G. A model for hydraulic fracture growth across multiple elastic layers. J. Pet. Sci. Eng. 2018, 167, 918–928. [Google Scholar] [CrossRef]
- Zia, H.; Lecampion, B.; Zhang, W. Impact of the anisotropy of fracture toughness on the propagation of planar 3D hydraulic fracture. Int. J. Fract. 2018, 211, 103–123. [Google Scholar] [CrossRef]
- Moukhtari, F.E.; Lecampion, B.; Zia, H. Propagation of a planar hydraulic fracture perpendicular to the isotropy plane of a transversely isotropic material. In Proceedings of the 53rd U.S. Rock Mechanics/Geomechanics Symposium, New York, NY, USA, 23–26 June 2019. [Google Scholar]
- Detournay, E.; Peirce, A. On the moving boundary conditions for a hydraulic fracture. Int. J. Eng. Sci. 2014, 84, 147–155. [Google Scholar] [CrossRef]
- Hills, D.A.; Kelly, P.A.; Dai, D.N.; Korsunsky, A.M. Solution of crack problems. In The Distributed Dislocation Technique, Solid Mechanics and Its Application; Kluwer Academic Publisher: Dordrecht, The Netherlands, 1996; Volume 44. [Google Scholar]
- Crouch, S.L.; Starfield, A.M. Boundary Element Method in Solid Mechanics; George Allen and Unwin: London, UK, 1983. [Google Scholar]
- Xiao, H.T.; Yue, Z.Q. A three-dimensional displacement discontinuity method for crack problems in layered rocks. Int. J. Rock Mech. Min. Sci. 2011, 48, 412–420. [Google Scholar] [CrossRef]
- Sesetty, V.; Ghassemi, A. A numerical study of sequential and simultaneous hydraulic fracturing in single and multi-lateral horizontal wells. J. Pet. Sci. Eng. 2015, 132, 65–76. [Google Scholar] [CrossRef]
- Zia, H.; Lecampion, B. PyFrac: A planar 3D hydraulic fracture simulator. Comput. Phys. Commun. 2020, 255, 107368. [Google Scholar] [CrossRef]
- Savitski, A.A.; Detournay, E. Propagation of a penny-shaped fluid-driven fracture in an impermeable rock: Asymptotic solutions. Int. J. Solid Struct. 2002, 39, 6311–6337. [Google Scholar] [CrossRef]
- Dontsov, E.V. An approximate solution for a penny-shaped hydraulic fracture that accounts for fracture toughness, fluid viscosity and leak-off. R. Soc. Open Sci. 2016, 3, 160737. [Google Scholar] [CrossRef]
- Kuriyama, K.; Mizuta, Y. Three-dimensional elastic analysis by the displacement discontinuity method with boundary division into triangular. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 111–123. [Google Scholar] [CrossRef]
- Tada, H.; Paris, P.C.; Irwin, G.R. The Stress Analysis of Cracks Handbook, 3rd ed.; ASME Press: New York, NY, USA, 2000. [Google Scholar]
- Zvyagin, A.V.; Luzhin, A.A.; Smirnov, N.N.; Shamina, A.A.; Shamin, A.Y. Stress intensity factors for branching cracks in space structures. Acta Astronaut. 2021, 180, 66–72. [Google Scholar] [CrossRef]
- Shamina, A.A.; Smirnov, N.N.; Zvyagin, A.V.; Luzhin, A.A.; Panfilov, D.I.; Udalov, A.S. Computational modeling of cracks different forms in three-dimensional space. Acta Astronaut. 2021, 186, 289–302. [Google Scholar] [CrossRef]
- Shamina, A.A.; Akulich, A.V.; Tyurenkova, V.V.; Smirnov, N.N.; Zvyaguin, A.V. The study of the strength of structures weakened by a system of cracks. Acta Astronaut. 2020, 168, 620–627. [Google Scholar] [CrossRef]
- Smirnov, N.N.; Li, K.; Skryleva, E.; Pestov, D.; Shamina, A.A.; Qi, C.; Kiselev, A.B. Mathematical modeling of hydraulic fracture formation and cleaning processes. Energies 2022, 15, 1967. [Google Scholar] [CrossRef]
- Kiselev, A.B.; Kairui Li Smirnov, N.N.; Pestov, D.A. Simulation of fluid flow thorough a hydraulic fracture of heterogeneous fracture-tough reservoir in the planar 3D formulation. In Fluid Dynamics; Maik Nauka/Interperiodica Publishing (Russian Federation): Moscow, Russia, 2021; Volume 56, pp. 164–177. [Google Scholar]
- Li, K.; Smirnov, N.N.; Kiselev, A.B.; Pestov, D.A.; Qi, C. The numerical asymptotic solution to initial condition problem of preexisting plane-strain hydraulic fracture with fluid lag. Eng. Fract. Mech. 2020, 239, 107296. [Google Scholar] [CrossRef]
- Li, K.; Smirnov, N.N.; Pestov, D.A.; Qi, C.; Kiselev, A.B. An approximate analytical solution for hydraulic fracture opening under non-uniform internal pressure. Mater. Phys. Mech. 2020, 44, 288–305. [Google Scholar]
- Li, K.; Smirnov, N.N.; Kiselev, A.B.; Wang, M.; Qi, C. An implicit algorithm with fast convergence for evolution of a preexisting-closed plane-strain hydraulic fracture. WSEAS Trans. Math. 2019, 18, 359–372. [Google Scholar]

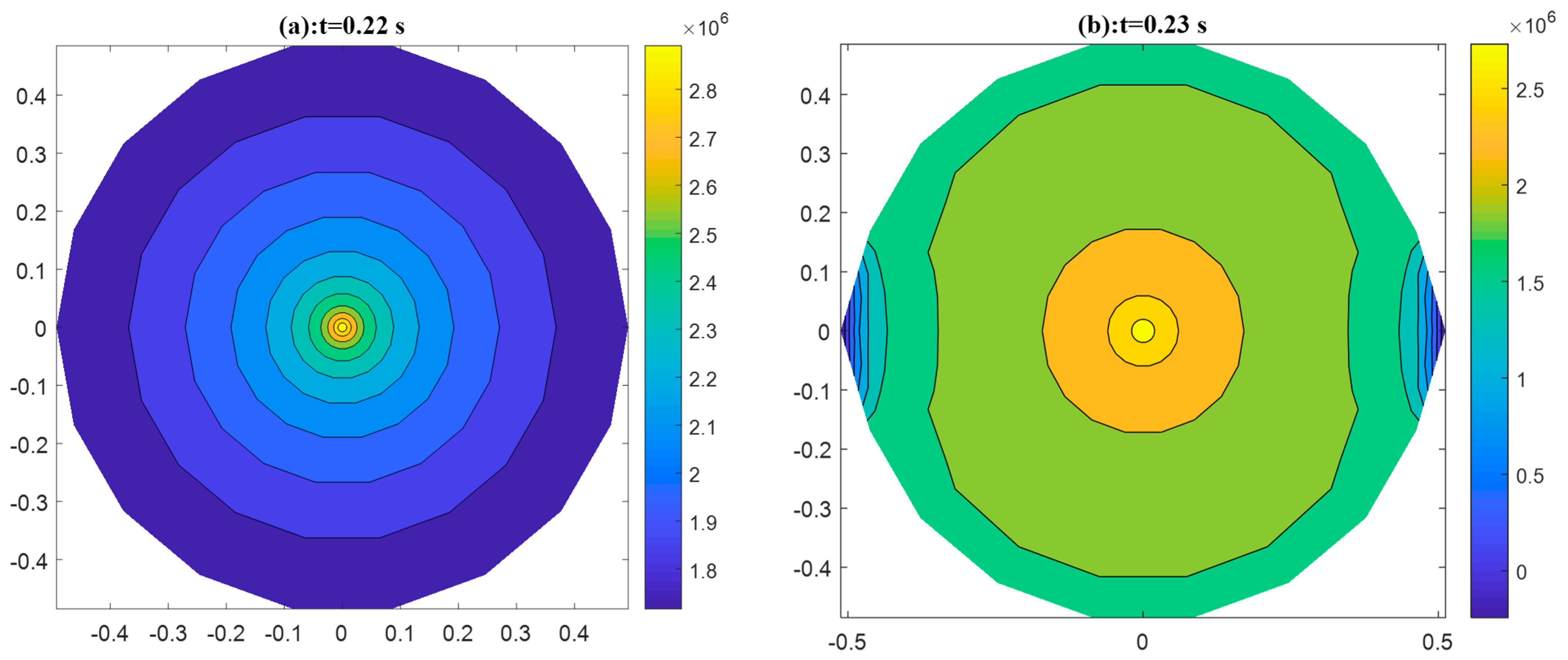
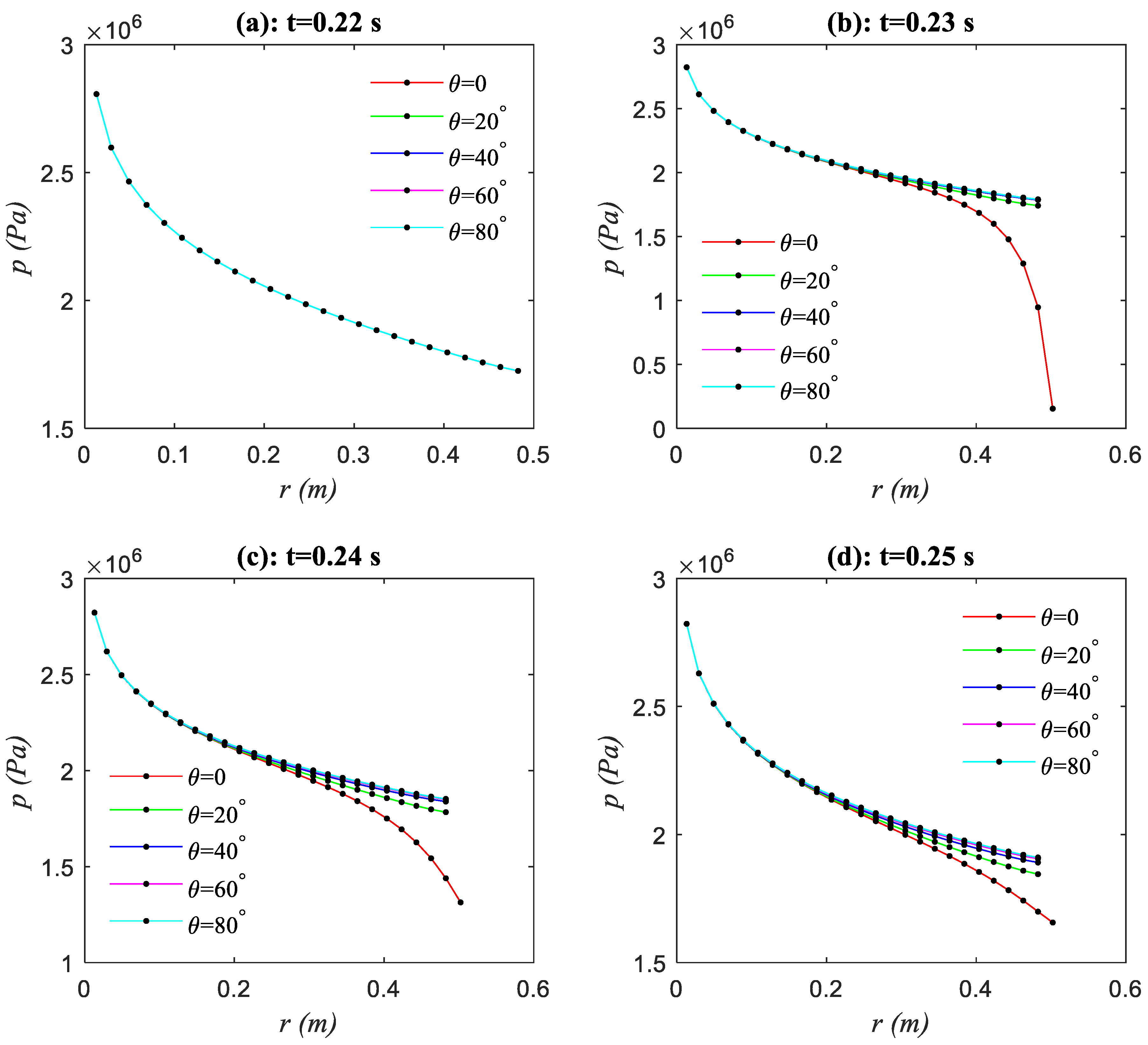
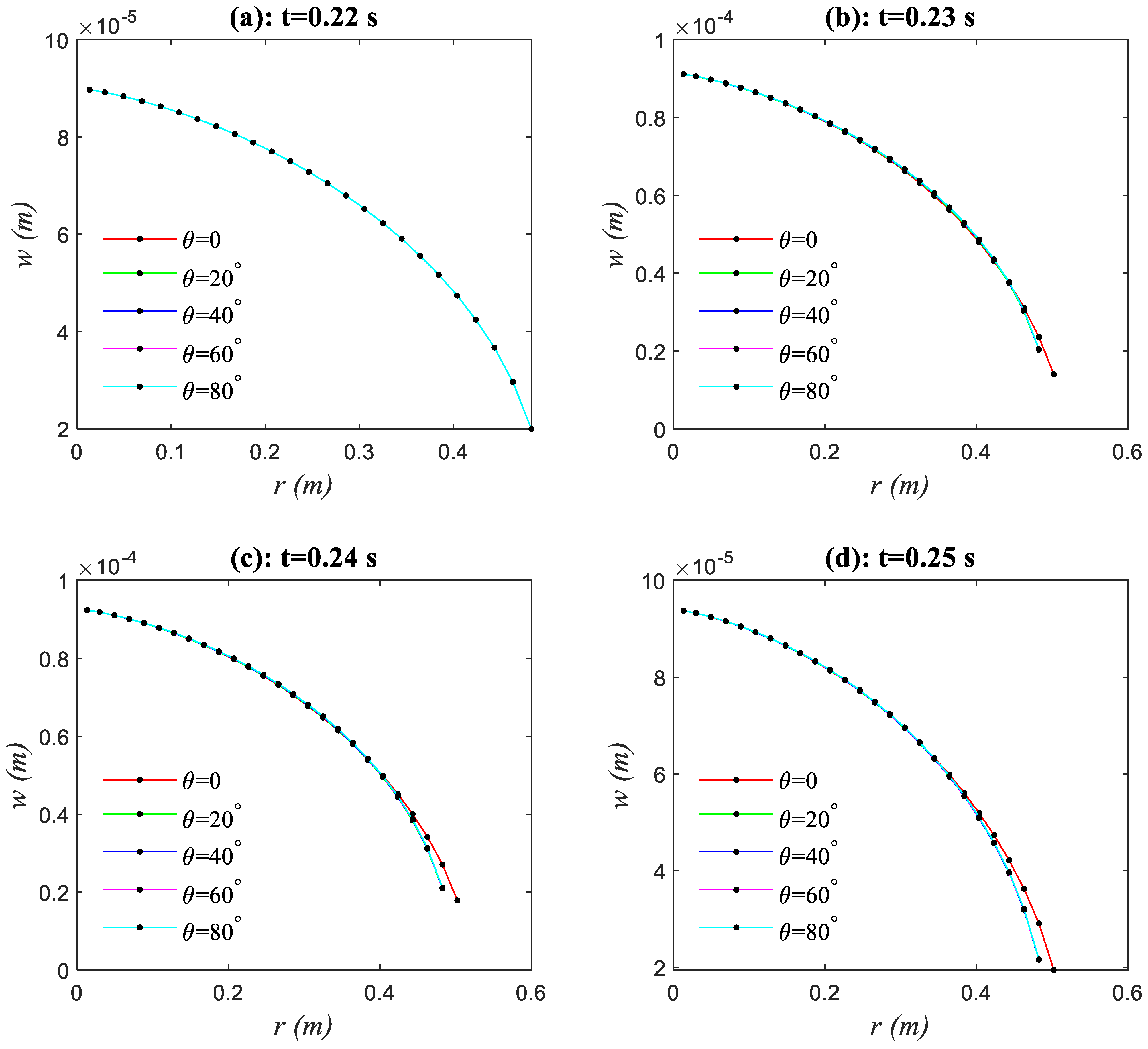
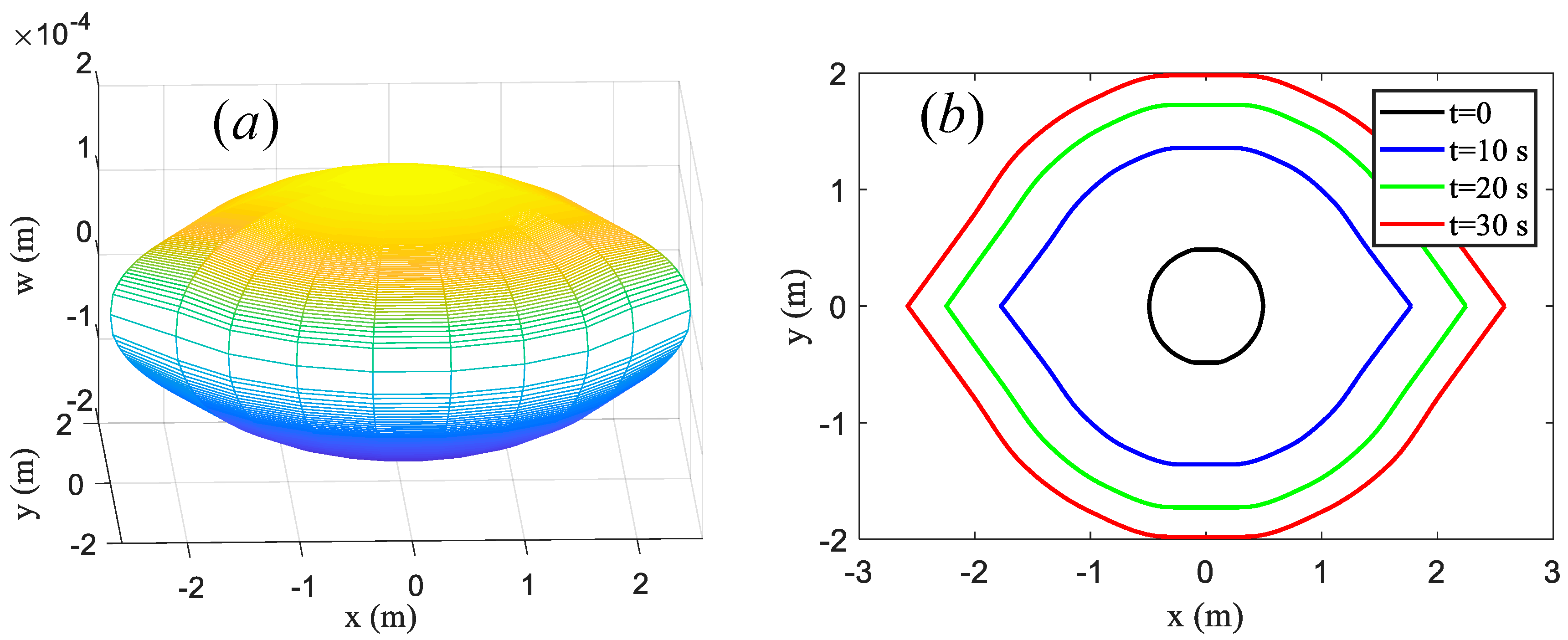



| (m) | 0.5 | ) | 1 |
| (GPa) | 30 | (m3/s) | |
| 0.2 | ) |
| Experiments | |||
|---|---|---|---|
| Case a | 1.5 | 1.5 | 1.5 |
| Case b | 1.5 | 2.0 | 2.0 |
| Case c | 1.5 | 1.0 | 1.0 |
| Case d | 1.5 | 2.0 | 1.0 |
| Case e | 1.5 | 1.0 | 2.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, K.; Smirnov, N.N.; Qi, C.; Wang, M.; Pestov, D.A.; Shamina, A.A. A Planar-3D Mathematical Model for Studying the Effect of Heterogeneity of Rock Fracture Toughness on Hydraulic Fracture Propagation: Early-Time Solution including the Stage before Propagation. Mathematics 2023, 11, 2083. https://doi.org/10.3390/math11092083
Li K, Smirnov NN, Qi C, Wang M, Pestov DA, Shamina AA. A Planar-3D Mathematical Model for Studying the Effect of Heterogeneity of Rock Fracture Toughness on Hydraulic Fracture Propagation: Early-Time Solution including the Stage before Propagation. Mathematics. 2023; 11(9):2083. https://doi.org/10.3390/math11092083
Chicago/Turabian StyleLi, Kairui, Nickolay N. Smirnov, Chengzhi Qi, Mingyang Wang, Dmitry A. Pestov, and Anastasia A. Shamina. 2023. "A Planar-3D Mathematical Model for Studying the Effect of Heterogeneity of Rock Fracture Toughness on Hydraulic Fracture Propagation: Early-Time Solution including the Stage before Propagation" Mathematics 11, no. 9: 2083. https://doi.org/10.3390/math11092083
APA StyleLi, K., Smirnov, N. N., Qi, C., Wang, M., Pestov, D. A., & Shamina, A. A. (2023). A Planar-3D Mathematical Model for Studying the Effect of Heterogeneity of Rock Fracture Toughness on Hydraulic Fracture Propagation: Early-Time Solution including the Stage before Propagation. Mathematics, 11(9), 2083. https://doi.org/10.3390/math11092083







