D-Optimal Designs for Binary and Weighted Linear Regression Models: One Design Variable
Abstract
1. Introduction
2. Models
Two-Parameter Models
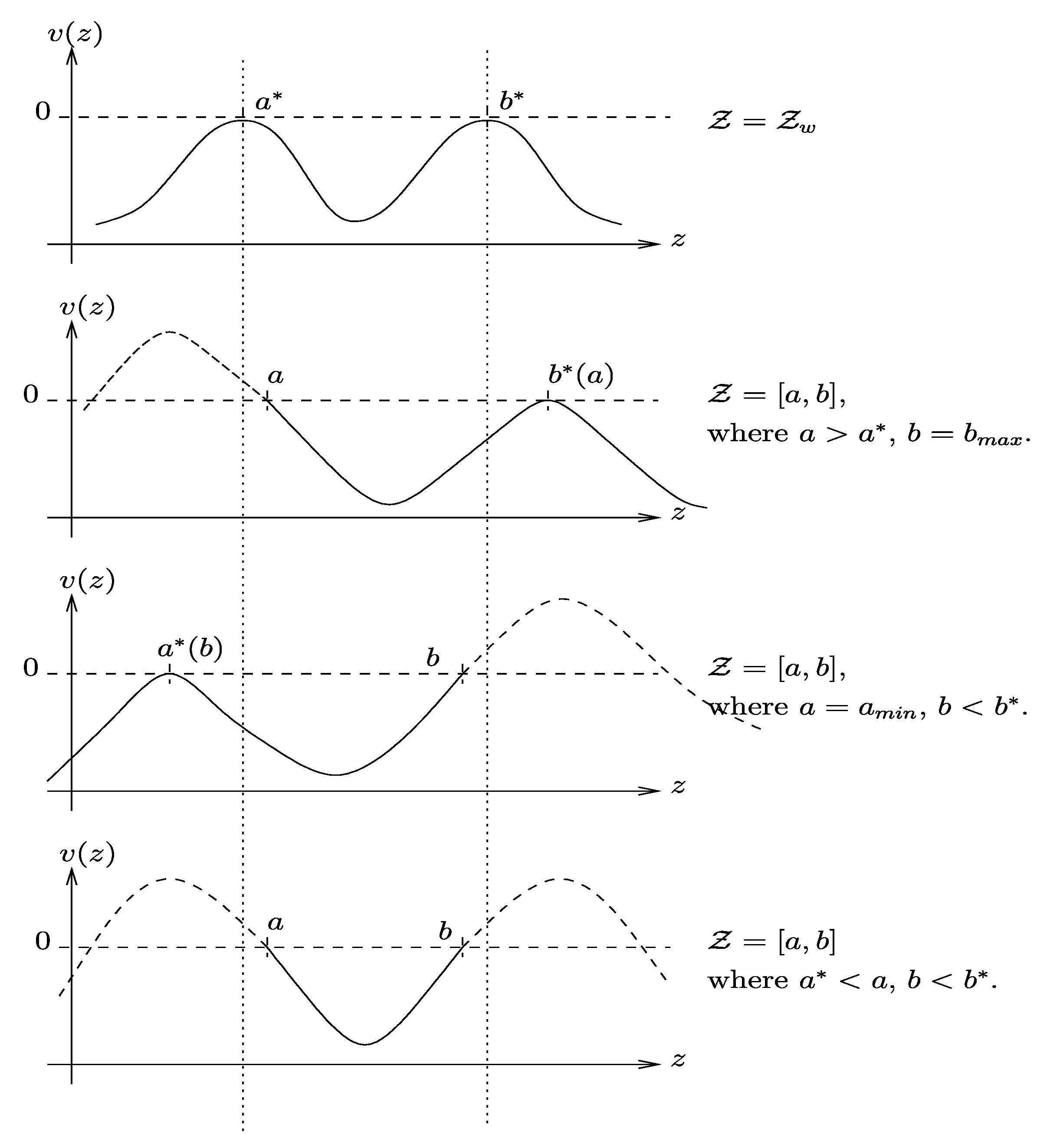
3. On the Number of Support Points
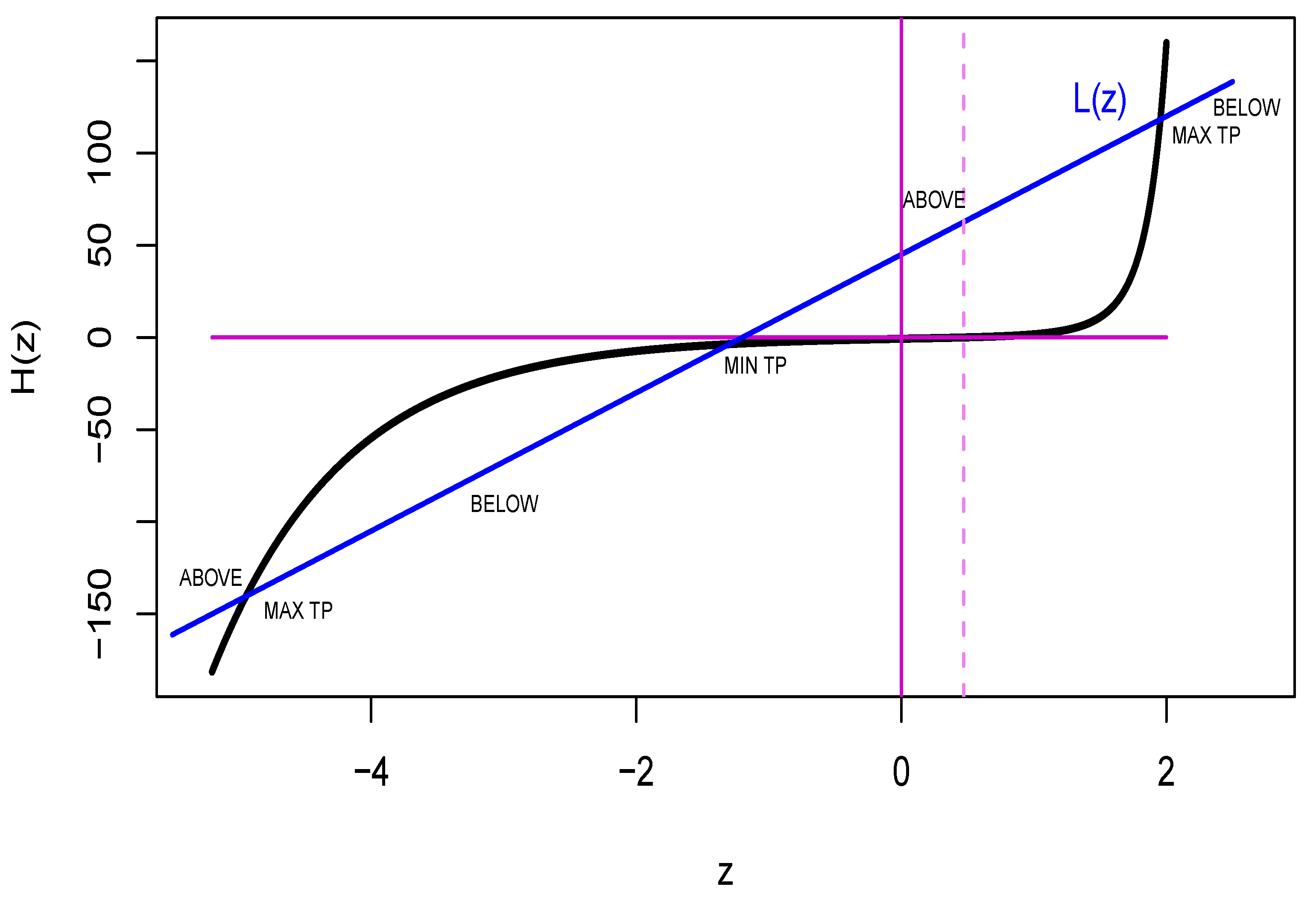
4. Determination of Support Points
4.1. A Conjecture Proved under a Sufficient Condition
- Case 1: , ;
- Case 2: , , .
- Case 3: , , , , ;
- Case 4: , , , , ;
- Case 5: , , , .
- , . We show that .Since , . Moreover,The right-hand side of the equation is always positive, because and . Therefore, .
- , . We show .Since , . MoreoverThis equation is always positive on the right-hand side because and . Therefore, .
- ,Now, , is the only possible solution to Equations (10) and (11). Thus, . Thus, , identify 2 max TP’s of . Moreover, they are TP’s at which has a common value of zero since . From the property of , the only possibility is that they are the values of z say , ) at which the two maximal TP’s of , i.e., , . Hence, the Equivalence Theorem is satisfied on and on any subset which contains , . Hence, the two-point designis D-optimal for all where and .
- Because , and , and .
- Since is increasing over , and since , then . Therefore, .
- Since is increasing over , and then . Therefore, .Hence, the two-point designis D-optimal for all where .
- ,Clearly, . We want negative. First, since . Secondwhere is the inverse function of for and for . Consider and recall that . We know from Theorem (1) that is the only solution where . Thus, the functions and cross only once at . Furthermore, since , while , then at . Hence, this inequality is true for . Therefore, . Thus, the two-point designis D-optimal for all , where and . Since for , this design is also D-optimal for .
- First, . Secondly, by above. Thus, . Consider now . Because , . We assumed . If is an increasing function, . Hence, . Thus, and the two-point designis D-optimal for all where and .
- ,This is the complementary to case 4 with andThus, the two point designis D-optimal for all where and . Since for . This is also D-optimal for .
- This is the complementary of case 6 withBecause and because of andSince andThus, the two-point designis D-optimal for all where and .
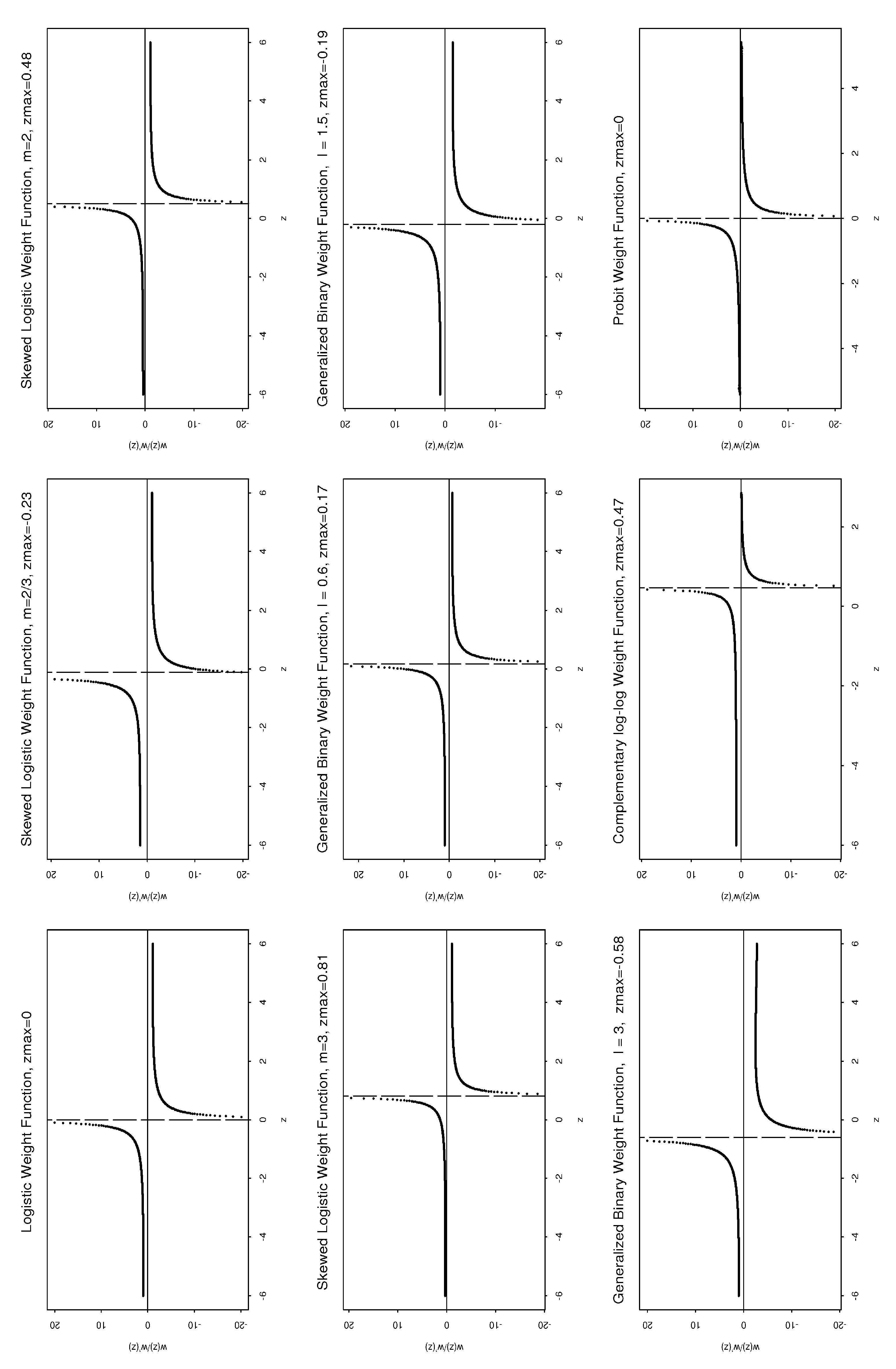
4.2. Examples of
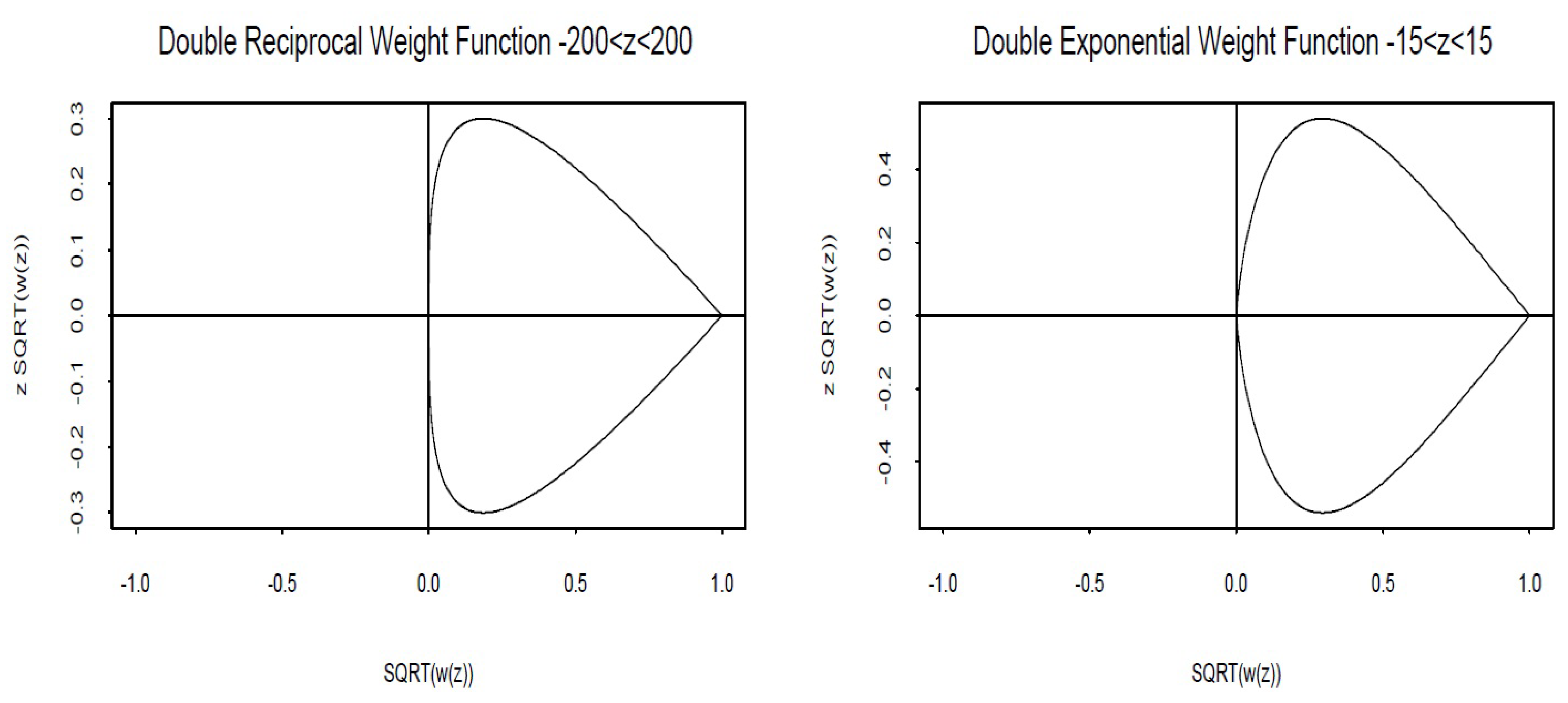
5. Group III and Group IV Weight Functions Results
5.1. Group III Weight Functions
5.2. Group IV Other Weight Functions
- Implications for support points of the D-optimal design on are
6. Results
- The function is first concave increasing then convex increasing;
- The function is increasing (this also guarantees that is closed convex). In some cases, the ratio is also increasing. (Note: shows that induced design space and the widest possible design space.)
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kiefer, J. Optimum experimental designs. J. R. Stat. Soc. Ser. B 1959, 21, 272–304. [Google Scholar] [CrossRef]
- Fedorov, V.V. Theory of Optimal Experiments; Academic Press: New York, NY, USA, 1972. [Google Scholar]
- Silvey, S. Optimal Design. An Introduction to the Theory for Parameter Estimation; Chapman and Hall: London, UK; New York, NY, USA, 1980. [Google Scholar]
- Pázman, A. Foundations of Optimum Experimental Design; Springer: Dordrecht, The Netherlands, 1986. [Google Scholar]
- Farrell, R.H.; Kiefer, J.; Walbran, A. Optimum Multivariate Designs. In Proceedings of the Fifth Berkeley Symposium on Mathematical Statistics and Probability, Held at the Statistical Laboratory, University of California, 21 June–18 July 1965 and 27 December 1965–7 January 1966; University of California Press: Berkeley, CA, USA, 1967; Volume 1, pp. 113–138. [Google Scholar]
- Kiefer, J.; Wolfowitz, J. The equivalence of two extremum problems. Can. J. Math. 1960, 12, 363–366. [Google Scholar] [CrossRef]
- Kiefer, J. Optimum Designs in Regression Problems, II. Ann. Math. Stat. 1961, 32, 298–325. [Google Scholar] [CrossRef]
- Ford, I.; Torsney, B.; Wu, C. The use of a canonical form in the construction of locally optimal designs for non-linear problems. J. R. Stat. Soc. Ser. B 1992, 54, 569–583. [Google Scholar] [CrossRef]
- Sitter, R.; Torsney, B. D-optimal designs for generalized linear models. MODA4-Advances in Model-Oriented Data Analysis. In Proceedings of the 4th International Workshop, Spetses, Greece, 5–9 June 1995; pp. 87–102. [Google Scholar]
- Sitter, R.R.; Torsney, B. Optimal Designs for Binary Response Experiments with Two Design Variable. Stat. Sin. 1995, 5, 405–419. [Google Scholar]
- Sebastiani, P.; Settimi, R. A note on D-optimal designs for logistic regression model. J. Stat. Plan. Inference 1997, 59, 359–368. [Google Scholar] [CrossRef]
- Gunduz, N. D-Optimal Designs for Weighted Linear Regression and Binary Regression Models. Ph.D. Thesis, University of Glasgow, Glasgow, UK, 1999. [Google Scholar]
- Torsney, B.; Gunduz, N. On Optimal Designs for High Dimensional Binary Regression Models. In Optimum Design 2000. Nonconvex Optimization and Its Applications; Atkinson, A., Bogacka, B., Zhigljavsky, A., Eds.; Springer: Boston, MA, USA, 2001; Volume 51. [Google Scholar]
- Gunduz, N.; Torsney, B. Some advances in optimal designs in contingent valuation studies. J. Stat. Plan. Inference 2006, 136, 1153–1165. [Google Scholar] [CrossRef]
- Haines, L.H.; Kabera, G.M.; Ndlovu, P.; O’Brien, T.E. D-optimal Designs for Logistic Regression in Two Variables. Moda Adv. Model Oriented Des. Anal. 2007, 193, 136–150. [Google Scholar]
- Idais, O. On local optimality of vertex type designs in generalized linear models. Stat Pap. 2021, 62, 1871–1898. [Google Scholar] [CrossRef]
- Jafari, H.; Khazai, S.; Khaki, Y.; Jafari, T. D-Optimal Design for Logistic Regression Model with Three Independent Variables. J. Asian Sci. Res. 2014, 4, 120–124. [Google Scholar]
- Kabera, G.; Haines, L.; Ndlovu, P. The analytic construction of D-optimal designs for the two-variable binary logistic regression model without interaction. Statistics 2015, 49, 1169–1186. [Google Scholar] [CrossRef]
- Haines, L.H.; Kabera, G.M.; Ndlovu, P. D-optimal designs for the two-variable binary logistic regression model with interaction. J. Stat. Plan. Inference 2018, 193, 136–150. [Google Scholar] [CrossRef]
- Gaffke, N.; Idais, O.; Schwabe, R. Locally optimal designs for gamma models. J. Stat. Plan. Inference 2019, 203, 199–214. [Google Scholar] [CrossRef]
- Zhai, Y.; Wang, C.; Lin, H.; Fang, Z. D-optimal designs for two-variable logistic regression model with restricted design space. Commun. Stat. Theory Methods 2023, 1–18. [Google Scholar] [CrossRef]
- Box, G.E.P.; Lucas, H.L. Design of experiments in nonlinear situations. Biometrika 1959, 46, 77–90. [Google Scholar] [CrossRef]
- Silvey, S. Discussion on “Results in the theory and Construction of D-optimal experimental designs”. J. R. Stat. Soc. Ser. B 1972, 34, 174–175. [Google Scholar]
- Sibson, R. Discussion on “Results in the theory and Construction of D-optimal experimental designs”. J. R. Stat. Soc. Ser. B 1972, 34, 181–183. [Google Scholar]
- Wynn, H.P. Results in the theory and construction of D-optimal experimemtal designs. J. R. Stat. Soc. Ser. B 1972, 34, 133–147. [Google Scholar]
- Wu, C. Optimal design for percentile estimation of a quantal response curve. In Optimal Design and Analysis of Experiments; Dodge, Y., Fedorov, V.H., Eds.; Elsevier (North Holland Publishing Co.): Amsterdam, The Netherlands, 1988; pp. 213–222. [Google Scholar]
- Musrati, A.K. Locally Optimal Designs for Binary and Weighted Regression Models. Master’s Thesis, University of Glasgow, Glasgow, UK, 1992. [Google Scholar]
- Torsney, B.; Musrati, A.K. On the Construction of Optimal Designs with Applications to Binary Response and to weighted Regression Models. In Model-Oriented Data Analysis, Proceedings of the 3rd International Workshop, Petrodvorets, Russia, 25–30 May 1992 (Contributions to Statistics); Muller, W.O., Wynn, H.P., Zhigliausky, A.A., Eds.; Physica-Verlag: Heidelberg, Germany, 1993; pp. 329–341. [Google Scholar]


| 1- Binary Weight Functions: Group I , | ||||
| Name | ||||
| (widest possible design space) | ||||
| Logistic | ||||
| Skewed Logistic | ||||
| Generalised | ||||
| Complementary log-log | ||||
| Probit | ||||
| 2-Binary Weight Functions: Group II , | ||||
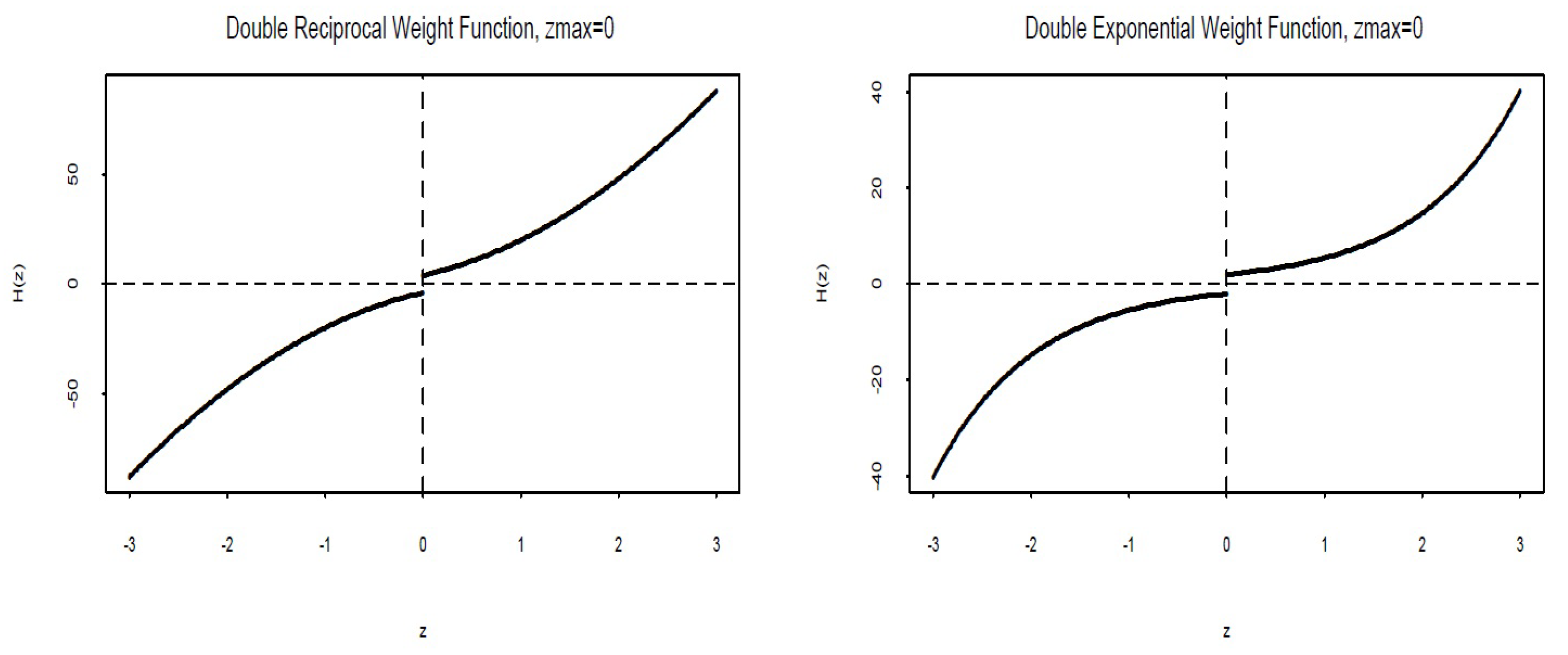
| Double reciprocal | ||||
| Double exponential | ||||
| 3-Density Weight functions: Group III | ||||
| Beta | ||||
| Gamma | ||||
| Normal | ||||
| 4-Other Weight functions: Group IV | ||||
| Name | ||
|---|---|---|
| Logistic | ||
| Skewed logistic | ||
| Generalised | ||
| Complementary log-log | ||
| Probit |
| Name | ||||
| Beta | ||||
| Beta | ||||
| Gamma | ||||
| Normal |
| Name | ||||
| Logistic | ||||
| Skewed logistic | ||||
| Generalised | ||||
| Complementary l. | ||||
| Probit |
| Name | |||||
| Beta | z | ||||
| Beta | |||||
| Gamma | |||||
| Normal |
| , | ↓ | ↓ | ||||
| , | ↑ | ↑ | ||||
| , | ↑ | ↑ | ||||
| ↑ | ↑ | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gündüz, N.; Torsney, B. D-Optimal Designs for Binary and Weighted Linear Regression Models: One Design Variable. Mathematics 2023, 11, 2075. https://doi.org/10.3390/math11092075
Gündüz N, Torsney B. D-Optimal Designs for Binary and Weighted Linear Regression Models: One Design Variable. Mathematics. 2023; 11(9):2075. https://doi.org/10.3390/math11092075
Chicago/Turabian StyleGündüz, Necla, and Bernard Torsney. 2023. "D-Optimal Designs for Binary and Weighted Linear Regression Models: One Design Variable" Mathematics 11, no. 9: 2075. https://doi.org/10.3390/math11092075
APA StyleGündüz, N., & Torsney, B. (2023). D-Optimal Designs for Binary and Weighted Linear Regression Models: One Design Variable. Mathematics, 11(9), 2075. https://doi.org/10.3390/math11092075






