Vegetation Patterns in the Hyperbolic Klausmeier Model with Secondary Seed Dispersal
Abstract
1. Introduction
2. Materials and Methods
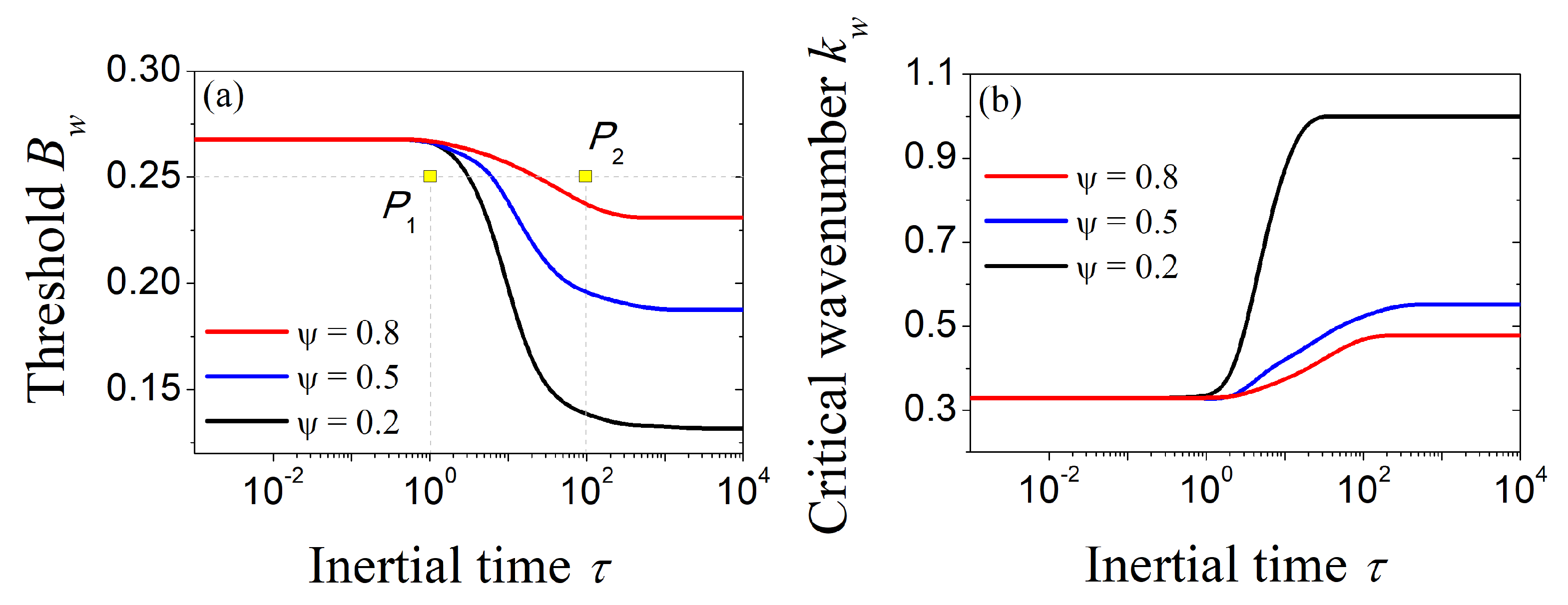
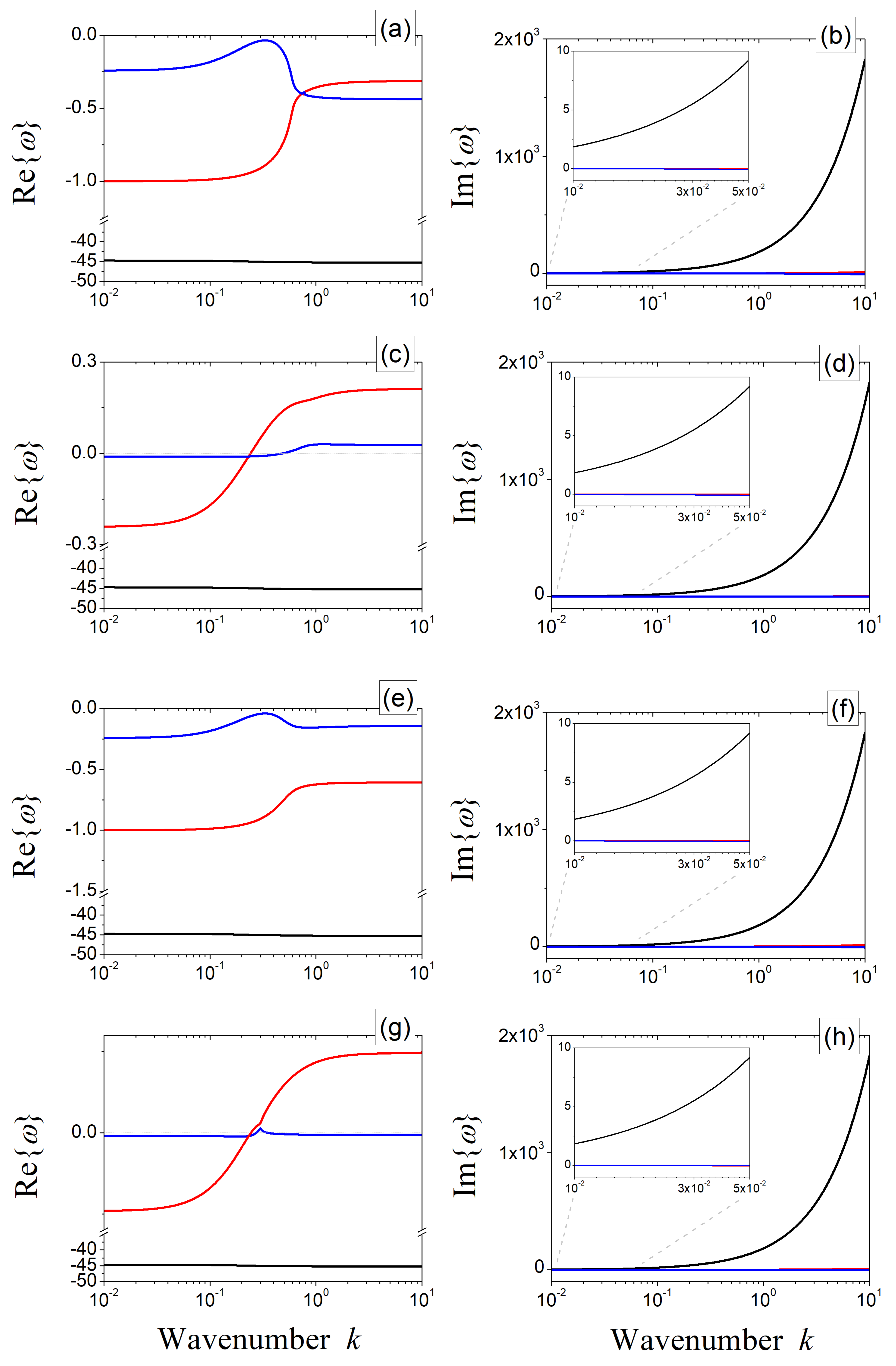
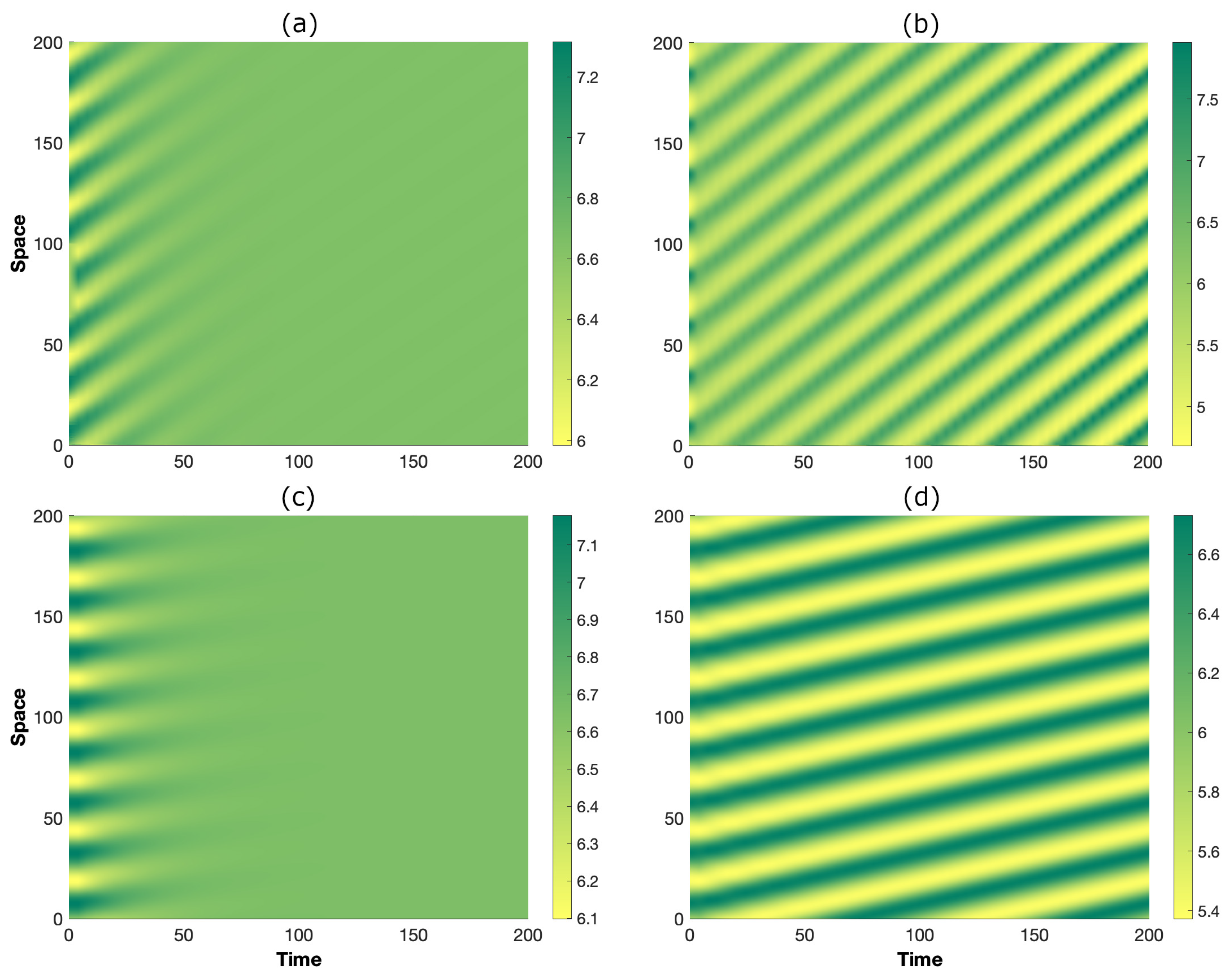
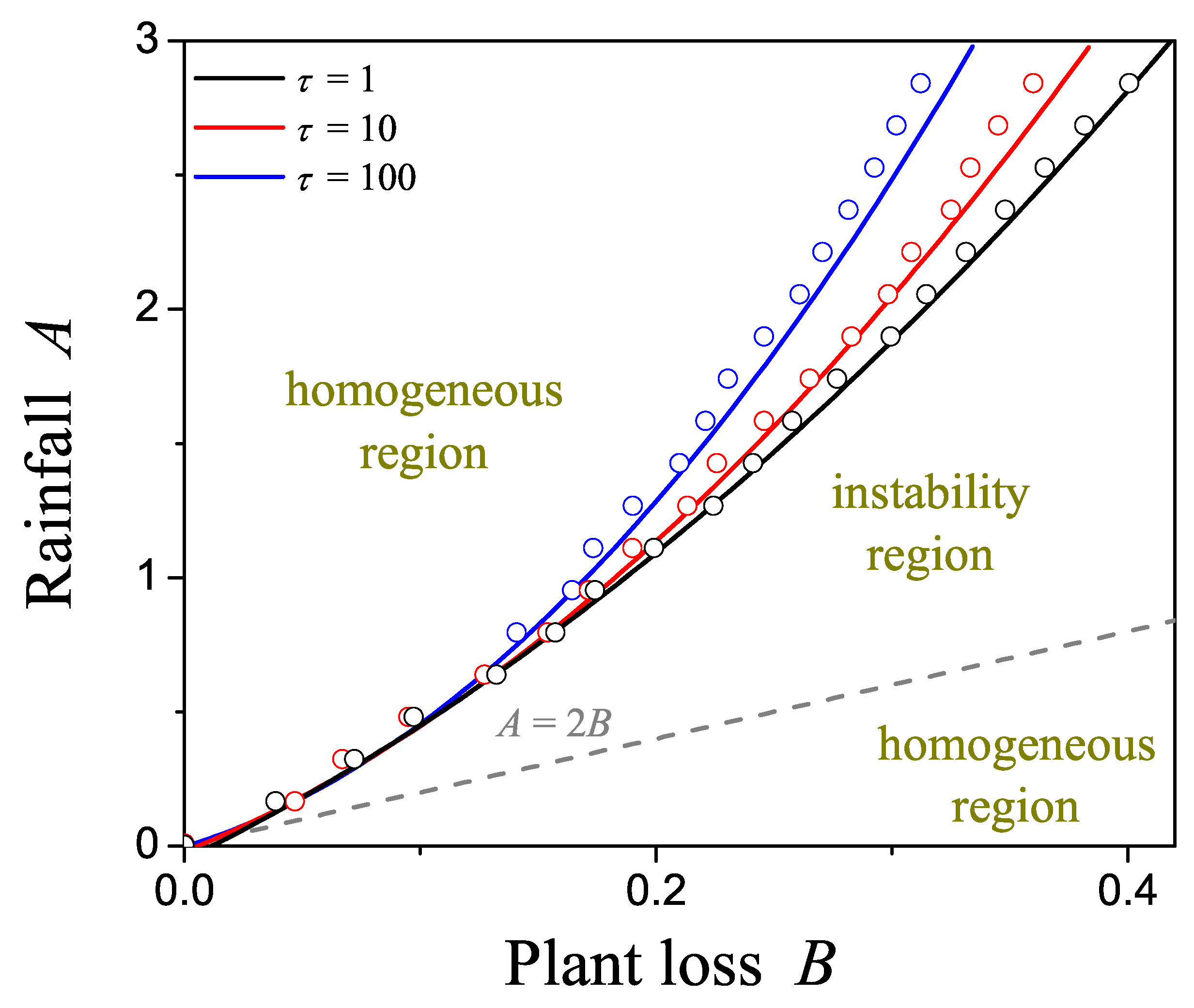
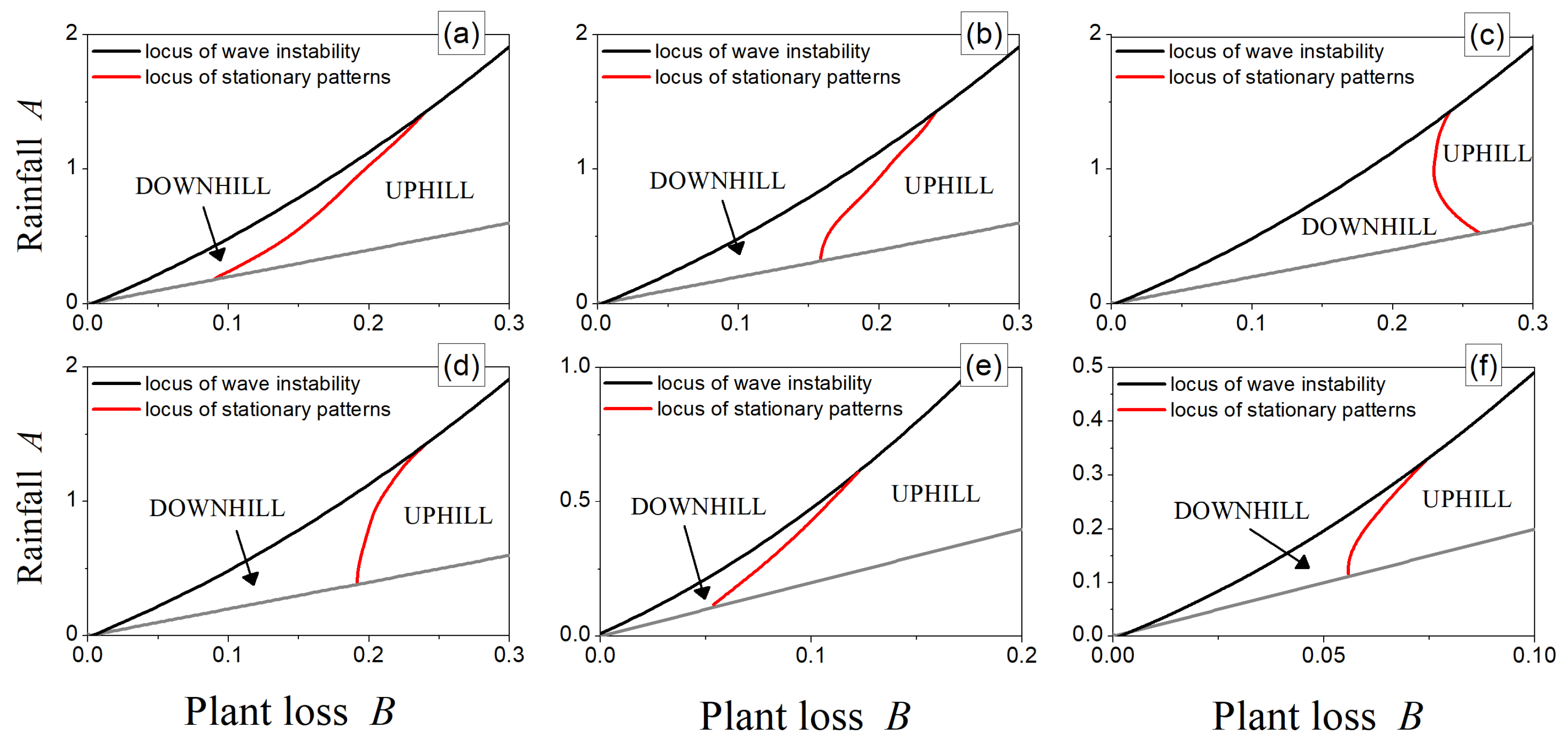
3. Results
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Murray, J.D. Mathematical Biology: I. An Introduction; Springer: New York, NY, USA, 2002. [Google Scholar]
- Murray, J.D. Mathematical Biology II: Spatial Models and Biomedical Applications; Springer: Berlin, Germany, 2003. [Google Scholar]
- Cross, M.; Greenside, H. Pattern Formation and Dynamics in Nonequilibrium Systems; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- Meron, E. Nonlinear Physics of Ecosystems; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Turing, A.M. The chemical basis of morphogenesis. Philos. Trans. R. Soc. Lond. 1952, 237, 37. [Google Scholar]
- Klausmeier, C.A. Regular and Irregular Patterns in Semiarid Vegetation. Science 1999, 284, 1826–1828. [Google Scholar] [CrossRef] [PubMed]
- HilleRisLambers, R.; Rietkerk, M.; van den Bosch, F.; Prins, H.H.; de Kroon, H. Vegetation pattern formation in semi-arid grazing systems. Ecology 2001, 82, 50–61. [Google Scholar] [CrossRef]
- Sherratt, J.A. An analysis of vegetation stripe formation in semi-arid landscapes. J. Math. Biol. 2005, 51, 183–197. [Google Scholar] [CrossRef] [PubMed]
- Borgogno, F.; D’Odorico, P.; Laio, F.; Ridolfi, L. Mathematical models of vegetation pattern formation in ecohydrology. Rev. Geophys. 2009, 47, RG1005. [Google Scholar] [CrossRef]
- van der Stelt, S.; Doelman, A.; Hek, G.; Rademacher, J.D. Rise and Fall of Periodic Patterns for a Generalized Klausmeier-Gray-Scott Model. J. Nonlinear Sci. 2013, 23, 39–95. [Google Scholar] [CrossRef]
- Siteur, K. Beyond Turing: The response of patterned ecosystems to environmental change. Ecol. Compl. 2014, 20, 81–96. [Google Scholar] [CrossRef]
- Marasco, A.; Iuorio, A.; Cartení, F.; Bonanomi, G.; Tartakovsky, D.M.; Mazzoleni, S.; Giannino, F. Vegetation pattern formation due to interactions between water availability and toxicity in plant-soil feedback. Bull. Math. Biol. 2014, 76, 2866–2883. [Google Scholar] [CrossRef]
- Consolo, G.; Valenti, G. Secondary seed dispersal in the Klausmeier model of vegetation for sloped semi-arid environments. Ecol. Model. 2019, 402, 66–75. [Google Scholar] [CrossRef]
- Eigentler, L.; Sherratt, J.A. An integrodifference model for vegetation patterns in semi-arid environments with seasonality. J. Math. Biol. 2020, 81, 875–904. [Google Scholar] [CrossRef]
- Rietkerk, M.; Ketner, P.; Burger, J.; Hoorens, B.; Olff, H. Multiscale soil and vegetation patchiness along a gradient of herbivore impact in a semi-arid grazing system in West Africa. Plant Ecol. 2000, 148, 207–224. [Google Scholar] [CrossRef]
- Von Hardenberg, J.; Meron, E.; Shachak, M.; Zarmi, Y. Diversity of vegetation patterns and desertification. Phys. Rev. Lett. 2001, 87, 198101. [Google Scholar] [CrossRef]
- Rietkerk, M. Self-organisation of vegetation in arid ecosystems. Am. Nat. 2002, 160, 524. [Google Scholar] [CrossRef]
- Gilad, E.; von Hardenberg, J.; Provenzale, A.; Shachak, M.; Meron, E. Ecosystem Engineers: From Pattern Formation to Habitat Creation. Phys. Rev. Lett. 2004, 93, 098105. [Google Scholar] [CrossRef]
- Thompson, S.; Katul, G.; McMahon, S.M. Role of biomass spread in vegetation pattern formation within arid ecosystems. Water Resour. Res. 2008, 44, W10421. [Google Scholar] [CrossRef]
- Thompson, S.; Katul, G. Secondary seed dispersal and its role in landscape organization. Geophys. Res. Lett. 2009, 36, L02402. [Google Scholar] [CrossRef]
- Deblauwe, V.; Couteron, P.; Bogaert, J.; Barbier, N. Determinants and dynamics of banded vegetation pattern migration in arid climates. Ecol. Monograph 2012, 82, 3–21. [Google Scholar] [CrossRef]
- Severino, G.; Giannino, F.; Cartení, F.; Mazzoleni, S.; Tartakovsky, D.M. Effects of Hydraulic Soil Properties on Vegetation Pattern Formation in Sloping Landscapes. Bull. Math. Biol. 2017, 79, 2773–2784. [Google Scholar] [CrossRef]
- Gandhi, P.; Werner, L.; Iams, S.; Gowda, K.; Silber, M. A topographic mechanism for arcing of dryland vegetation bands. J. R. Soc. Interface 2018, 15, 20180508. [Google Scholar] [CrossRef]
- Meron, E. From Patterns to Function in Living Systems: Dryland Ecosystems as a Case Study. Ann. Rev. Condens. Matt. Phys. 2018, 9, 79–103. [Google Scholar] [CrossRef]
- Gowda, K.; Iams, S.; Silber, M. Signatures of human impact on self-organized vegetation in the Horn of Africa. Sci. Rep. 2018, 8, 3622. [Google Scholar] [CrossRef] [PubMed]
- Marasco, A.; Giannino, F.; Iuorio, A. Modelling competitive interactions and plant-soil feedback in vegetation dynamics. Ric. Mat. 2020, 69, 553–577. [Google Scholar] [CrossRef]
- Saco, P.M.; Willgoose, G.R.; Hancock, G.R. Eco-geomorphology of banded vegetation patterns in arid and semi-arid regions. Hydrol. Earth Syst. Sci. 2007, 11, 1717–1730. [Google Scholar] [CrossRef]
- Ursino, N.; Rulli, M.C. Combined effect of fire and water scarcity on vegetation patterns in arid lands. Ecol. Model. 2010, 221, 2353–2362. [Google Scholar] [CrossRef]
- Sherratt, J.A.; Synodinos, A.D. Vegetation patterns and desertification waves in semi-arid environments: Mathematical models based on local facilitation in plants. Discrete Cont. Dyn. Syst. Ser. B 2012, 17, 2815–2827. [Google Scholar] [CrossRef]
- Sherratt, J.A. Pattern Solutions of the Klausmeier Model for Banded Vegetation in Semiarid Environments V: The Transition from Patterns to Desert. SIAM J. Appl. Math. 2013, 73, 1347–1367. [Google Scholar] [CrossRef]
- Thompson, S.E.; Assouline, S.; Chen, L.; Trahktenbrot, A.; Svoray, T.; Katul, G.G. Secondary dispersal driven by overland flow in drylands: Review and mechanistic model development. Mov. Ecol. 2014, 2, 4. [Google Scholar] [CrossRef]
- Zelnik, Y.R.; Uecker, H.; Feudel, U.; Meron, E. Desertification by front propagation? J. Theor. Biol. 2017, 418, 27–35. [Google Scholar] [CrossRef]
- MacFadyen, W. Vegetation patterns in the semi-desert plains of British Somaliland. Geograph. J. 1950, 115, 199–211. [Google Scholar] [CrossRef]
- Hemming, C.F. Vegetation arcs in Somaliland. J. Ecol. 1965, 53, 57–67. [Google Scholar] [CrossRef]
- Tongway, D.J. Banded Vegetation Patterning in Arid and Semiarid Environments; Springer: New York, NY, USA, 2001. [Google Scholar]
- Dunkerley, D.L.; Brown, K.J. Oblique vegetation banding in the Australian arid zone: Implications for theories of pattern evolution and maintenance. J. Arid Environ. 2002, 52, 163–181. [Google Scholar] [CrossRef]
- Dunkerley, D. Banded vegetation in some Australian semi-arid landscapes: 20 years of field observations to support the development and evaluation of numerical models of vegetation pattern evolution. Desert 2018, 23, 165–187. [Google Scholar]
- Montana, C.; Lopez-Portillo, J.; Mauchamp, A. The response of two woody species to the conditions created by a shifting ecotone in an arid ecosystem. J. Ecol. 1990, 78, 789–798. [Google Scholar] [CrossRef]
- Montaña, C. The colonisation of bare areas two-phase mosaics of an arid ecosystem. J. Ecol. 1992, 80, 315–327. [Google Scholar] [CrossRef]
- Worral, G.A. The Butanna grass pattern. J. Soil Sci. 1959, 10, 34–53. [Google Scholar] [CrossRef]
- Boaler, S.B.M.; Hodge, C.A.H. Observations on vegetation arcs in the northern region, Somali Republic. J. Ecol. 1964, 52, 511–544. [Google Scholar] [CrossRef]
- Valentin, C.; d’Herbés, J.M. Niger tiger bush as a natural water harvesting system. Catena 1999, 37, 231–256. [Google Scholar] [CrossRef]
- Kealy, B.J.; Wollkind, D.J. A nonlinear stability analysis of vegetative Turing pattern formation for an interaction–diffusion plant-surface water model system in an arid flat environment. Bull. Math. Biol. 2012, 74, 803–833. [Google Scholar] [CrossRef]
- Zelnik, Y.R.; Kinast, S.; Yizhaq, H.; Bel, G.; Meron, E. Regime shifts in models of dryland vegetation. Phil. Trans. R. Soc. A 2013, 321, 20120358. [Google Scholar] [CrossRef]
- Pueyo, M.; Mateu, J.; Rigol, A.; Vidal, M.; López-Sánchez, J.F.; Rauret, G. Use of the modified BCR three-step sequential extraction procedure for the study of trace element dynamics in contaminated soils. Environ. Poll. 2008, 152, 330–341. [Google Scholar] [CrossRef]
- Milchunas, D.G.; Lauenroth, W.K. Inertia in plant community structure: State changes after cessation of nutrient-enrichment stress. Ecol. Appl. 1995, 5, 452–458. [Google Scholar] [CrossRef]
- Garcia-Fayos, P.; Gasque, M. Consequences of a severe drought on spatial patterns of woody plants in a two-phase mosaic steppe of Stipa tenacissima. J. Arid Environ. 2002, 52, 199–208. [Google Scholar] [CrossRef]
- Deblauwe, V.; Couteron, P.; Lejeune, O.; Bogaert, J.; Barbier, N. Environmental modulation of self-organized periodic vegetation patterns in Sudan. Ecography 2011, 34, 990–1001. [Google Scholar] [CrossRef]
- Von Holle, B.; Delcourt, H.R.; Simberloff, D. The importance of biological inertia in plant community resistance to invasion. J. Veg. Sci. 2003, 14, 425–432. [Google Scholar] [CrossRef]
- Brown, J.H.; Whitham, T.G.; Morgan Ernest, S.K.; Gehring, C.A. Complex species interactions and the dynamics of ecological systems: Long-term experiments. Science 2001, 293, 643–650. [Google Scholar] [CrossRef]
- Consolo, G.; Grifó, G.; Valenti, G. Dryland vegetation pattern dynamics driven by inertial effects and secondary seed dispersal. Ecol. Model. 2022, 474, 110171. [Google Scholar] [CrossRef]
- AI-Ghoul, M.; Eu, B.C. Hyperbolic reaction-diffusion equations and irreversible thermodynamics: Cubic reversible reaction model. Phys. D 1996, 90, 119–153. [Google Scholar] [CrossRef]
- Hillen, T. Hyperbolic models for chemosensitive movement. Math. Models Methods Appl. Sci. 2002, 12, 1–28. [Google Scholar] [CrossRef]
- Mendez, V.; Fedotov, S.; Horsthemke, W. Reaction-Transport Systems; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Straughan, B. Heat Waves; Applied Mathematical Sciences; Springer: New York, NY, USA, 2011. [Google Scholar]
- Zemskov, E.P.; Horsthemke, W. Diffusive instabilities in hyperbolic reaction-diffusion equations. Phys. Rev. E 2016, 93, 032211. [Google Scholar] [CrossRef]
- Mvogo, A.; Macías-Díaz, J.E.; Kofané, T.C. Diffusive instabilities in a hyperbolic activator-inhibitor system with superdiffusion. Phys. Rev. E 2018, 97, 032129. [Google Scholar] [CrossRef]
- Curró, C.; Valenti, G. Pattern formation in hyperbolic models with cross-diffusion: Theory and applications. Phys. D 2021, 418, 132846. [Google Scholar] [CrossRef]
- Consolo, G.; Currò, C.; Valenti, G. Pattern formation and modulation in a hyperbolic vegetation model for semiarid environments. Appl. Math. Model. 2017, 43, 372–392. [Google Scholar] [CrossRef]
- Consolo, G.; Currò, C.; Valenti, G. Supercritical and subcritical Turing pattern formation in a hyperbolic vegetation model for flat arid environments. Phys. D 2019, 398, 141–163. [Google Scholar] [CrossRef]
- Consolo, G.; Currò, C.; Valenti, G. Turing vegetation patterns in a generalized hyperbolic Klausmeier model. Math. Methods Appl. Sci. 2020, 43, 10474–10489. [Google Scholar] [CrossRef]
- Consolo, G.; Curró, C.; Grifó, G.; Valenti, G. Oscillatory periodic pattern dynamics in hyperbolic reaction-advection-diffusion models. Phys. Rev. E 2022, 105, 034206. [Google Scholar] [CrossRef]
- Consolo, G.; Grifó, G. Eckhaus instability of stationary patterns in hyperbolic reaction-diffusion models on large finite domains. Part. Diff. Eq. Appl. 2022, 3, 57. [Google Scholar] [CrossRef]
- Ruggeri, T.; Sugiyama, M. Classical and Relativistic Rational Extended Thermodynamics of Gases; Springer: Cham, Switzerland, 2021. [Google Scholar]
- Barbera, E.; Curro, C.; Valenti, G. On discontinuous travelling wave solutions for a class of hyperbolic reaction-diffusion models. Phys. D 2015, 308, 116–126. [Google Scholar] [CrossRef]
- Sherratt, J.A. Pattern solutions of the Klausmeier Model for banded vegetation in semi-arid environments I. Nonlinearity 2010, 23, 2657–2675. [Google Scholar] [CrossRef]
- MATLAB® v 9.13.0; The MathWorks Inc.: Natick, MA, USA, 2022.

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grifò, G. Vegetation Patterns in the Hyperbolic Klausmeier Model with Secondary Seed Dispersal. Mathematics 2023, 11, 1084. https://doi.org/10.3390/math11051084
Grifò G. Vegetation Patterns in the Hyperbolic Klausmeier Model with Secondary Seed Dispersal. Mathematics. 2023; 11(5):1084. https://doi.org/10.3390/math11051084
Chicago/Turabian StyleGrifò, Gabriele. 2023. "Vegetation Patterns in the Hyperbolic Klausmeier Model with Secondary Seed Dispersal" Mathematics 11, no. 5: 1084. https://doi.org/10.3390/math11051084
APA StyleGrifò, G. (2023). Vegetation Patterns in the Hyperbolic Klausmeier Model with Secondary Seed Dispersal. Mathematics, 11(5), 1084. https://doi.org/10.3390/math11051084






