Cost-Effectiveness Mathematical Model to Evaluate the Impact of Improved Cardiac Ablation Strategies for Atrial Fibrillation Treatment
Abstract
1. Introduction
2. Materials and Methods
2.1. Description of the Model
2.2. Transformation of Time Horizon of Probability
2.3. Input Data
2.4. Scenarios
2.5. Probabilistic Sensitivity Analysis
3. Results
3.1. Validation of the Model
3.2. Predictions of the Model: Evaluation of Potential Scenarios
3.3. Evaluation of Potential Scenarios with a More Effective Ablation Strategy
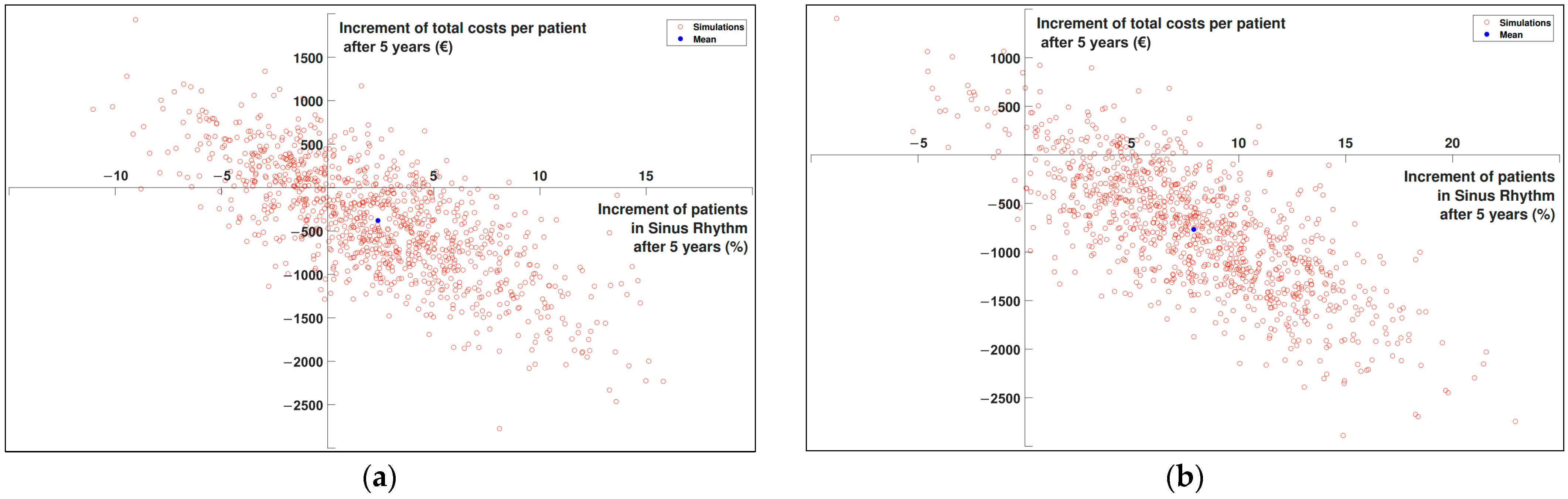
3.4. Probabilistic Sensitivity Analysis
3.5. Impact on Costs in Europe
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ball, J.; Carrington, M.J.; McMurray, J.J.V.; Stewart, S. Atrial Fibrillation: Profile and Burden of an Evolving Epidemic in the 21st Century. Int. J. Cardiol. 2013, 167, 1807–1824. [Google Scholar] [CrossRef]
- Chugh, S.S.; Havmoeller, R.; Narayanan, K.; Singh, D.; Rienstra, M.; Benjamin, E.J.; Gillum, R.F.; Kim, Y.H.; McAnulty, J.H.; Zheng, Z.J.; et al. Worldwide Epidemiology of Atrial Fibrillation: A Global Burden of Disease 2010 Study. Circulation 2014, 129, 837–847. [Google Scholar] [CrossRef] [PubMed]
- Hindricks, G.; Potpara, T.; Dagres, N.; Arbelo, E.; Bax, J.J.; Blomström-Lundqvist, C.; Boriani, G.; Castella, M.; Dan, G.A.; Dilaveris, P.E.; et al. 2020 ESC Guidelines for the Diagnosis and Management of Atrial Fibrillation Developed in Collaboration with the European Association for Cardio-Thoracic Surgery (EACTS). Eur. Heart J. 2021, 42, 373–498. [Google Scholar] [CrossRef]
- Fibrilación Auricular. Síntomas y Causas. Mayo Clinic. Available online: https://www.mayoclinic.org/es-es/diseases-conditions/atrial-fibrillation/symptoms-causes/syc-20350624 (accessed on 18 April 2021).
- Mark, D.B.; Anstrom, K.J.; Sheng, S.; Piccini, J.P.; Baloch, K.N.; Monahan, K.H.; Daniels, M.R.; Bahnson, T.D.; Poole, J.E.; Rosenberg, Y.; et al. Effect of Catheter Ablation vs Medical Therapy on Quality of Life among Patients with Atrial Fibrillation: The CABANA Randomized Clinical Trial. JAMA 2019, 321, 1275–1285. [Google Scholar] [CrossRef] [PubMed]
- Willems, S.; Verma, A.; Betts, T.R.; Murray, S.; Neuzil, P.; Ince, H.; Steven, D.; Sultan, A.; Heck, P.M.; Hall, M.C.; et al. Targeting Nonpulmonary Vein Sources in Persistent Atrial Fibrillation Identified by Noncontact Charge Density Mapping: UNCOVER AF Trial. Circ. Arrhythm. Electrophysiol. 2019, 12, e007233. [Google Scholar] [CrossRef]
- Anderson, L.H.; Black, E.J.; Civello, K.C.; Martinson, M.S.; Kress, D.C. Cost-Effectiveness of the Convergent Procedure and Catheter Ablation for Non-Paroxysmal Atrial Fibrillation. J. Med. Econ. 2014, 17, 481–491. [Google Scholar] [CrossRef] [PubMed]
- Velleca, M.; Costa, G.; Goldstein, L.; Bishara, M.; Ming Boo, L. A Review of the Burden of Atrial Fibrillation: Understanding the Impact of the New Millennium Epidemic across Europe. EMJ Cardiol. 2019, 7, 110–118. [Google Scholar] [CrossRef]
- Ringborg, A.; Nieuwlaat, R.; Lindgren, P.; Jönsson, B.; Fidan, D.; Maggioni, A.P.; Lopez-Sendon, J.; Stepinska, J.; Cokkinos, D.V.; Crijns, H.J.G.M. Costs of Atrial Fibrillation in Five European Countries: Results from the Euro Heart Survey on Atrial Fibrillation. EP Eur. 2008, 10, 403–411. [Google Scholar] [CrossRef] [PubMed]
- Arbelo, E.; Brugada, J.; Hindricks, G.; Maggioni, A.P.; Tavazzi, L.; Vardas, P.; Laroche, C.; Anselme, F.; Inama, G.; Jais, P.; et al. The Atrial Fibrillation Ablation Pilot Study: An European Survey on Methodology and Results of Catheter Ablation for Atrial Fibrillation Conducted by the European Heart Rhythm Association. Eur. Heart J. 2014, 35, 1466–1478. [Google Scholar] [CrossRef]
- Wolowacz, S.E.; Samuel, M.; Brennan, V.K.; Jasso-Mosqueda, J.G.; Van Gelder, I.C. The Cost of Illness of Atrial Fibrillation: A Systematic Review of the Recent Literature. Europace 2011, 13, 1375–1385. [Google Scholar] [CrossRef]
- Guo, J.; Nayak, H.M.; Besser, S.A.; Beaser, A.; Aziz, Z.; Broman, M.; Ozcan, C.; Tung, R.; Upadhyay, G.A. Impact of Atrial Fibrillation Ablation on Recurrent Hospitalization: A Nationwide Cohort Study. JACC Clin. Electrophysiol. 2019, 5, 330–339. [Google Scholar] [CrossRef] [PubMed]
- Lip, G.Y.H. The ABC Pathway: An Integrated Approach to Improve AF Management. Nat. Rev. Cardiol. 2017, 14, 627–628. [Google Scholar] [CrossRef] [PubMed]
- Mairesse, G.H.; Moran, P.; Van Gelder, I.C.; Elsner, C.; Rosenqvist, M.; Mant, J.; Banerjee, A.; Gorenek, B.; Brachmann, J.; Varma, N.; et al. Screening for Atrial Fibrillation: A European Heart Rhythm Association (EHRA) Consensus Document Endorsed by the Heart Rhythm Society (HRS), Asia Pacific Heart Rhythm Society (APHRS), and Sociedad Latinoamericana de Estimulación Cardíaca y Electrofisiolog. Europace 2017, 19, 1589–1623. [Google Scholar] [CrossRef]
- A Comparison of Rate Control and Rhythm Control in Patients with Atrial Fibrillation. N. Engl. J. Med. 2002, 347, 1825–1833. [CrossRef] [PubMed]
- Jacobs, M.S.; Kaasenbrood, F.; Postma, M.J.; Van Hulst, M.; Tieleman, R.G. Cost-Effectiveness of Screening for Atrial Fibrillation in Primary Care with a Handheld, Single-Lead Electrocardiogram Device in the Netherlands. Europace 2018, 20, 12–18. [Google Scholar] [CrossRef]
- Quesada, A.; Cózar, R.; Anguera, I. Registro Español de Ablación Con Catéter: XIX Informe Oficial de La Asociación Del Ritmo Cardiaco de La Sociedad Española de Cardiología (2019). Rev. Española Cardiol. 2020, 73, 1049–1060. [Google Scholar] [CrossRef]
- Sociedad Española de Salud Pública y Administración Sanitaria; Rodríguez Bezos, D.; Rodríguez Barrios, J.M. Gaceta Sanitaria; Sociedad Española de Salud Pública y Administración Sanitaria: Barcelona, Spain, 2010; Volume 24. [Google Scholar]
- Bowrin, K.; Briere, J.-B.; Levy, P.; Millier, A.; Tardu, J.; Toumi, M. Real-World Cost-Effectiveness of Rivaroxaban and Apixaban vs VKA in Stroke Prevention in Non-Valvular Atrial Fibrillation in the UK. J. Mark. Access Health Policy 2020, 8, 1782164. [Google Scholar] [CrossRef]
- Wodchis, W.P.; Bhatia, R.S.; Leblanc, K.; Meshkat, N.; Morra, D. A Review of the Cost of Atrial Fibrillation. Value Health 2012, 15, 240–248. [Google Scholar] [CrossRef]
- Ley de Tasas 20/2017, de 28 de Diciembre de La Generalitat Valenciana [2017/12159] [DOGV núm. 8202 de 30.12.2017]. Regional ACT. 2017, p. 258. Available online: https://dogv.gva.es/es/eli/es-vc/l/2017/12/28/20/ (accessed on 1 January 2023).
- Ministerio de Sanidad, Consumo y Bienestar Social. Portal Estadístico Del SNS. Estadisticas y Estudios. Informes y Recopilaciones. Available online: https://www.mscbs.gob.es/estadEstudios/estadisticas/inforRecopilaciones/anaDesarrolloGDR.htm (accessed on 8 July 2021).
- Calkins, H.; Reynolds, M.R.; Spector, P.; Sondhi, M.; Xu, Y.; Martin, A.; Williams, C.J.; Sledge, I. Treatment of Atrial Fibrillation With Antiarrhythmic Drugs or Radiofrequency Ablation. Circ. Arrhythmia Electrophysiol. 2009, 2, 349–361. [Google Scholar] [CrossRef]
- Meiltz, A.; Zimmermann, M.; Urban, P.; Bloch, A.; on behalf of the Association of Cardiologists of the Canton of Geneva. Atrial Fibrillation Management by Practice Cardiologists: A Prospective Survey on the Adherence to Guidelines in the Real World. EP Eur. 2008, 10, 674–680. [Google Scholar] [CrossRef]
- Kim, D.; Yang, P.-S.; Sung, J.-H.; Jang, E.; Yu, H.T.; Kim, T.-H.; Uhm, J.-S.; Kim, J.-Y.; Pak, H.-N.; Lee, M.-H.; et al. Less Dementia after Catheter Ablation for Atrial Fibrillation: A Nationwide Cohort Study. Eur. Heart J. 2020, 41, 4483–4493. [Google Scholar] [CrossRef] [PubMed]
- Biffi, M.; Boriani, G.; Bartolotti, M.; Reggiani, L.B.; Zannoli, R.; Branzi, A. Atrial Fibrillation Recurrence after Internal Cardioversion: Prognostic Importance of Electrophysiological Parameters. Heart 2002, 87, 443–448. [Google Scholar] [CrossRef] [PubMed]
- Nieuwlaat, R.; Prins, M.H.; Le Heuzey, J.-Y.; Vardas, P.E.; Aliot, E.; Santini, M.; Cobbe, S.M.; Widdershoven, J.W.M.G.; Baur, L.H.; Lévy, S.; et al. Prognosis, Disease Progression, and Treatment of Atrial Fibrillation Patients during 1 Year: Follow-up of the Euro Heart Survey on Atrial Fibrillation. Eur. Heart J. 2008, 29, 1181–1189. [Google Scholar] [CrossRef]
- Gage, B.F.; Yan, Y.; Milligan, P.E.; Waterman, A.D.; Culverhouse, R.; Rich, M.W.; Radford, M.J. Clinical Classification Schemes for Predicting Hemorrhage: Results from the National Registry of Atrial Fibrillation (NRAF). Am. Heart J. 2006, 151, 713–719. [Google Scholar] [CrossRef] [PubMed]
- Instituto Nacional de Estadística (INE)/Lista Completa de Operaciones. Available online: https://www.ine.es/dyngs/INEbase/listaoperaciones.htm (accessed on 28 January 2021).
- Levy, D.; Kenchaiah, S.; Larson, M.G.; Benjamin, E.J.; Kupka, M.J.; Ho, K.K.L.; Murabito, J.M.; Vasan, R.S. Long-Term Trends in the Incidence of and Survival with Heart Failure. N. Engl. J. Med. 2002, 347, 1397–1402. [Google Scholar] [CrossRef] [PubMed]
- Lakshminarayan, K.; Schissel, C.; Anderson, D.C.; Vazquez, G.; Jacobs, D.R.; Ezzeddine, M.; Luepker, R.V.; Virnig, B.A. Five-Year Rehospitalization Outcomes in a Cohort of Medicare Linkage Study. Stroke 2011, 42, 1556–1562. [Google Scholar] [CrossRef] [PubMed]
- Amlani, S.; Nadarajah, T.; Afzal, R.; Pal-Sayal, R.; Eikelboom, J.W.; Natarajan, M.K. Mortality and Morbidity Following a Major Bleed in a Registry Population with Acute ST Elevation Myocardial Infarction. J. Thromb. Thrombolysis 2010, 30, 434–440. [Google Scholar] [CrossRef]
- Vademecum. Es-Su Fuente de Conocimiento Farmacológico. Available online: https://www.vademecum.es/ (accessed on 26 April 2021).
- Ministerio de Sanidad, Consumo y Bienestar Social. Portal Estadístico Del SNS. Pesos de Los GRDs Del Sistema Nacional de Salud. Años Anteriores. Available online: https://www.mscbs.gob.es/estadEstudios/estadisticas/inforRecopilaciones/anaDesarrolloGDRanteriores.htm (accessed on 9 July 2021).
- Packer, D.L.; Mark, D.B.; Robb, R.A.; Monahan, K.H.; Bahnson, T.D.; Poole, J.E.; Noseworthy, P.A.; Rosenberg, Y.D.; Jeffries, N.; Mitchell, L.B.; et al. Effect of Catheter Ablation vs Antiarrhythmic Drug Therapy on Mortality, Stroke, Bleeding, and Cardiac Arrest among Patients with Atrial Fibrillation: The CABANA Randomized Clinical Trial. JAMA 2019, 321, 1261–1274. [Google Scholar] [CrossRef]
- Rodrigo, M.; Climent, A.M.; Hernández-Romero, I.; Liberos, A.; Baykaner, T.; Rogers, A.J.; Alhusseini, M.; Wang, P.J.; Fernández-Avilés, F.; Guillem, M.S.; et al. Noninvasive Assessment of Complexity of Atrial Fibrillation: Correlation with Contact Mapping and Impact of Ablation. Circ. Arrhythmia Electrophysiol. 2020, 13, e007700. [Google Scholar] [CrossRef]
- Qin, M.; Jiang, W.F.; Wu, S.H.; Xu, K.; Liu, X. Electrogram Dispersion-Guided Driver Ablation Adjunctive to High-Quality Pulmonary Vein Isolation in Atrial Fibrillation of Varying Durations. J. Cardiovasc. Electrophysiol. 2020, 31, 48–60. [Google Scholar] [CrossRef]
- Narayan, S.M.; Baykaner, T.; Krummen, D.; Shivkumar, K.; Clopton, P.; Miller, J. Firm Ablation at Rotors and Focal Sources Has Similarly High Efficacy for Paroxysmal and Persistent Atrial Fibrillation. J. Am. Coll. Cardiol. 2013, 61, E328. [Google Scholar] [CrossRef]
- Molero, R.; Soler Torro, J.M.; Martínez Alzamora, N.; Climent, M.A.; Guillem, M.S. Higher Reproducibility of Phase Derived Metrics from Electrocardiographic Imaging during Atrial Fibrillation in Patients Remaining in Sinus Rhythm after Pulmonary Vein Isolation. Comput. Biol. Med. 2021, 139, 104934. [Google Scholar] [CrossRef]
- Humphries, K.H.; Kerr, C.R.; Steinbuch, M.; Dorian, P. Limitations to Antiarrhythmic Drug Use in Patients with Atrial Fibrillation. CMAJ 2004, 171, 741–745. [Google Scholar] [CrossRef] [PubMed]
- Mansour, M.; Karst, E.; Heist, E.K.; Dalal, N.; Wasfy, J.H.; Packer, D.L.; Calkins, H.; Ruskin, J.N.; Mahapatra, S. The Impact of First Procedure Success Rate on the Economics of Atrial Fibrillation Ablation. JACC Clin. Electrophysiol. 2017, 3, 129–138. [Google Scholar] [CrossRef] [PubMed]
- Luik, A.; Radzewitz, A.; Kieser, M.; Walter, M.; Bramlage, P.; Hörmann, P.; Schmidt, K.; Horn, N.; Brinkmeier-Theofanopoulou, M.; Kunzmann, K.; et al. Cryoballoon versus Open Irrigated Radiofrequency Ablation in Patients with Paroxysmal Atrial Fibrillation. Circulation 2015, 132, 1311–1319. [Google Scholar] [CrossRef] [PubMed]
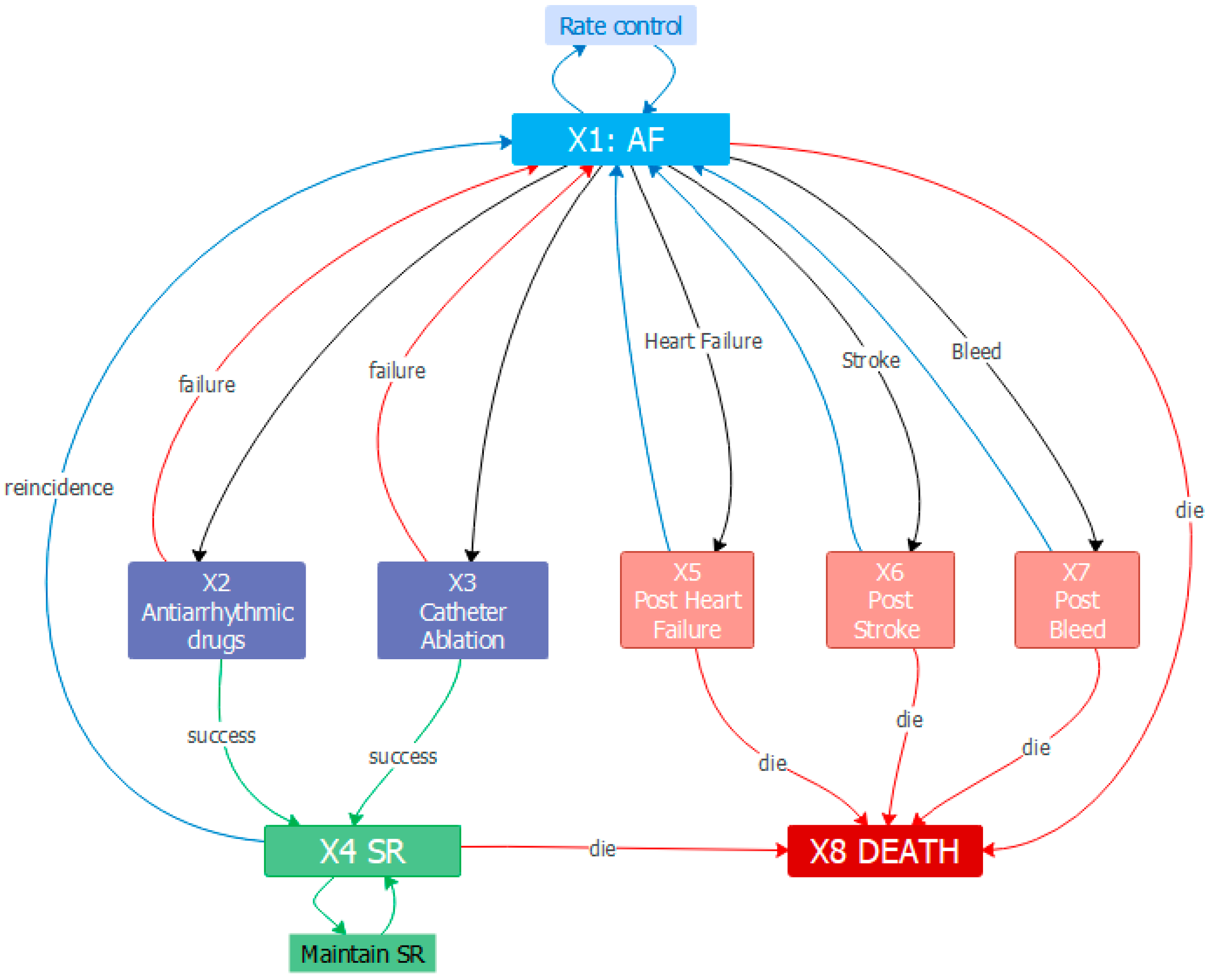



| Transition | Annual Probability (%) | References | Transition Probability |
|---|---|---|---|
| Effectiveness of CA | 57.00 | [10,23] | P34 |
| Effectiveness of AAD | 30.40 | [10] | P24 |
| Distribution for CA Distribution for AAD Distribution for RC Recurrence of AF after SR Occurrence of Heart Failure Occurrence of Stroke Occurrence of Major Bleed Mortality of SR Mortality of AF Mortality of Heart Failure Mortality of Stroke Mortality of Major Bleed | 4.80 34.60 44.00 29.00 5.00 5.00 4.20 0.87 2.40 22.00 23.70 19.70 | [24] [25] [10] [26] [27] [28] [24] [29] [3,18] [30] [31] [32] | P13 P12 P11 P41 P15 P16 P17 P48 P18 P58 P68 P78 |
| Costs of the Model (EUR 2020) | 3 Months | 12 Months | References |
|---|---|---|---|
| Cardiac Ablation, CA (X1) | 7888.01 | 10,247.92 | [21] |
| Antiarrhythmic drugs, AAD (X2) | 851.36 | 3405.45 | [33] |
| Atrial Fibrillation, AF (X3) | 802.82 | 3211.27 | [21,27,33] |
| Sinus Rhythm, SR (X4) | 14.83 | 59.32 | [9,21,27] |
| Stroke, ST (X5) | 5043.67 | 7452.12 | [34] |
| Heart Failure, HF (X6) | 4872.51 | 7280.97 | [34] |
| Major Bleed, MB (X7) | 3416.81 | 5825.26 | [18] |
| Death (X8) | 0.00 | 0.00 | - |
| Scenario | Total Cost per Patient | SR Patients | Mortality |
|---|---|---|---|
| Base case (A) | 10,343 EUR | 45.06% | 12.16% |
| 100% of patients under RC treatment (B) | 15,465 EUR | 0.0% | 21.73% |
| 100% of patients under AAD (C) | 8264 EUR | 56.43% | 9.08% |
| 100% of patients CA (D) | 10,696 EUR | 69.55% | 7.62% |
| Optimal scenario (E) | 8890 EUR | 60.58% | 8.64% |
| Scenario | Total Cost per Patient | SR Patients | Mortality |
|---|---|---|---|
| Base case (A) | 10,343 EUR | 45.06% | 12.16% |
| Base case with 82 % effectiveness of CA (A’) | 9872 EUR | 49.17% | 11.74% |
| 0 % of patients under treatment (B’) | 15,465 EUR | 0.0% | 21.73% |
| 100% of patients under AAD (C’) | 8264 EUR | 56.43% | 9.08% |
| 100% of patients CA (D’) | 8146 EUR | 75.77% | 6.87% |
| Suboptimal scenario (E’) | 8152 EUR | 62.50% | 8.43% |
| Situation under Assessment | Number of Ablations per Year | Annual Savings in Europe | Increase of Patients in SR after 5 Years |
|---|---|---|---|
| Base case | 283,500 | - | - |
| No increase of CA | 283,500 | 250 mills EUR | 155,000 |
| Doubled CA | 590,000 | 348 mills EUR | 573,500 |
| Quadrupled CA | 1,118,000 | 600 mills EUR | 1,000,000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sánchez-Périz, I.; Barrachina-Martínez, I.; Díaz-Carnicero, J.; Climent, A.M.; Vivas-Consuelo, D. Cost-Effectiveness Mathematical Model to Evaluate the Impact of Improved Cardiac Ablation Strategies for Atrial Fibrillation Treatment. Mathematics 2023, 11, 915. https://doi.org/10.3390/math11040915
Sánchez-Périz I, Barrachina-Martínez I, Díaz-Carnicero J, Climent AM, Vivas-Consuelo D. Cost-Effectiveness Mathematical Model to Evaluate the Impact of Improved Cardiac Ablation Strategies for Atrial Fibrillation Treatment. Mathematics. 2023; 11(4):915. https://doi.org/10.3390/math11040915
Chicago/Turabian StyleSánchez-Périz, Ixeia, Isabel Barrachina-Martínez, Javier Díaz-Carnicero, Andreu M. Climent, and David Vivas-Consuelo. 2023. "Cost-Effectiveness Mathematical Model to Evaluate the Impact of Improved Cardiac Ablation Strategies for Atrial Fibrillation Treatment" Mathematics 11, no. 4: 915. https://doi.org/10.3390/math11040915
APA StyleSánchez-Périz, I., Barrachina-Martínez, I., Díaz-Carnicero, J., Climent, A. M., & Vivas-Consuelo, D. (2023). Cost-Effectiveness Mathematical Model to Evaluate the Impact of Improved Cardiac Ablation Strategies for Atrial Fibrillation Treatment. Mathematics, 11(4), 915. https://doi.org/10.3390/math11040915







