Generalized Quasi Trees with Respect to Degree Based Topological Indices and Their Applications to COVID-19 Drugs

Abstract

The l-generalized quasi tree is a graph G for which we can find $W\subset V\left(G\right)$ with $\left|W\right|=l$ such that $G-W$ is a tree but for an arbitrary $Y\subset V\left(G\right)$ with $\left|Y\right|<l$, $G-Y$ is not a tree. In this paper, inequalities with respect to zeroth-order Randić and hyper-Zagreb indices are studied in the class of l-generalized quasi trees. The corresponding extremal graphs corresponding to these indices in the class of l-generalized quasi trees are also obtained. In addition, we carry QSPR analysis of COVID-19 drugs with zeroth-order Randić and hyper-Zagreb indices (energy).

1. Introduction

Let G denote a finite simple and connected graph of order n. Let $V\left(G\right)$ and $E\left(G\right)$ denote the set of vertices and edges of G, respectively. The degree $d_G\left(v\right)$ of a vertex $v\in V\left(G\right)$ is the number of edges incident on it. For simplicity, we write $d\left(v\right)$ instead of $d_G\left(v\right)$ when there is no ambiguity of notations. We denote path, cycle, star and compete graphs on n vertices by $P_n$, $C_n$, $S_n$ and $K_n$, respectively. An isolated vertex is represented by $K_1$. A tree with an edge $uv$ such that there are $a-1$ pendent vertices adjacent to u and $b-1$ pendant vertices adjacent to v, is called bistar (double star tree) of order and it is denoted by $S_{a,b}$. The minimum and maximum vertex degrees in a graph are respectively denoted by $\delta$ and $\Delta$. A vertex deleted subgraph $G-v$ of a graph G is obtained by deleting the vertex $v\in V\left(G\right)$ from G along with the edges incident on it. Similarly, for an edge $xy\in E\left(G\right),$ the graph $G-xy$ is the graph obtained by deleting the edge $xy$ from G. Let $X\subseteq V\left(G\right).$ Then the graph $G-X$ is obtained by deleting all vertices of X from G together with their incident edges. A tree is a connected graph that does not contain any cycles. Xu et al. [1] defined the notion of a quasi tree to be a graph G such that $G-\omega$ is a tree for some $\omega \in V\left(G\right)$. They also generalized this notion for any positive integer l and called it l-generalized quasi tree which is defined as a graph G for which there exists $W\subset V\left(G\right)$, with $\left|W\right|=l$ such that $G-W$ is a tree, while for any $Y\subset V\left(G\right)$ with $\left|Y\right|<l$, $G-Y$ is other than a tree. Here, the vertex $\omega \in V\left(G\right)$ and all $y\in W$ are the vertices deletion of whom from G result in a tree, thus theses vertices are called quasi vertices of G. We denote the set of l-quasi generalized trees with n vertices by $Q{T}_l^n$ and the set of all l quasi vertices by $V_l$. The notion of an apex tree and l-apex tree respectively refers to a quasi tree graph and l-generalized quasi tree. 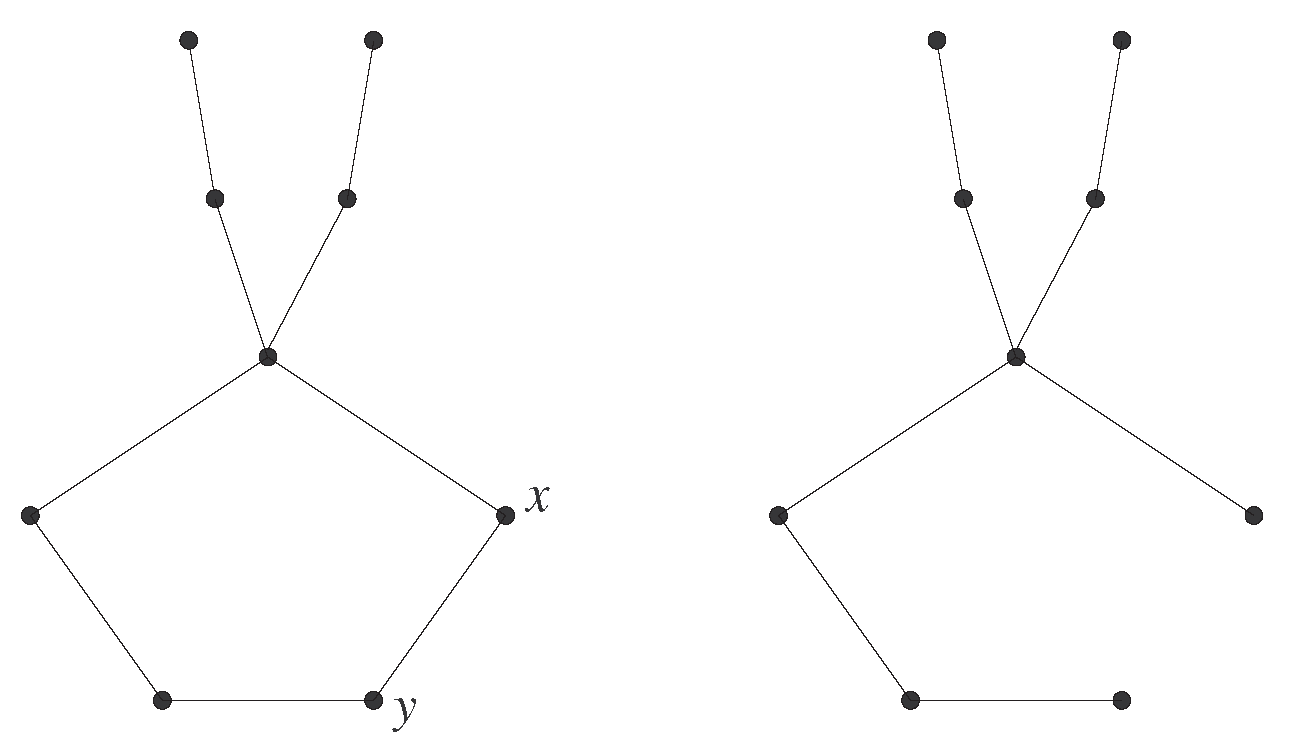Figure 1 represents a quasi tree graph or specifically a l-generalized quasi tree graph.

Figure 1. A l-Generalized Quasi Tree or a l-Apex Tree.

Now, we give a brief introduction to some important topological indices of our interest. Randić [2] defined the Randić index in 1975 as follows.

$$R\left(G\right)=\sum _{uv\in E}\frac{1}{\sqrt{d\left(u\right)d\left(v\right)}}.$$

Kier and Hall [3] defined the zeroth order Randić index as follows.

$${}^{0}R\left(G\right)=\sum _{v\in V}d{\left(v\right)}^{-1/2}.$$

(1)

In [4], Pavlović found a graph with maximal value of ${}^{0}R$. The hyper-Zagreb index [5] of a graph G is defined as

$$HM\left(G\right)=\sum _{uv\in E\left(G\right)}{\left[d_G\left(u\right)+d_G\left(v\right)\right]}^2.$$

The hyper-Zagreb matrix of G of order $n\times n$ is defined by

$$A_{HM}\left(G\right)=\left\{\begin{array}{cc}{\left[d_G\left(u\right)+d_G\left(v\right)\right]}^2\hfill & \mathrm{if} uv\in E\left(G\right)\hfill \\ 0\hfill & \mathrm{otherwise}.\hfill \end{array}\right.$$

Let ${\tau }_1\ge {\tau }_2\ge \cdots \ge {\tau }_n$ be the eigenvalues of $A_{HM}\left(G\right)$. Then, the hyper-Zagreb energy is defined as ${\mathcal{E}}_{HM}\left(G\right)={\sum }_{i=1}^n\left|{\tau }_i\right|$. The basic results, comparing the hyper-Zagreb index of a graph with its older congeners were presented by Gutman [6]. The hyper-Zagreb indices of graph operations such as join, corona, Cartesian product and more were studied in [5,7]. Liu and Tang [8] determined sharp upper bounds of cacti and determined the corresponding extremal graphs. In [9], Wang et al. studied upper and lower bounds of the hyper-Zagreb index and provided relation between Zagreb and hyper-Zagreb indices. Elumalai et al. [10] investigated some upper bounds on hyper-Zagreb index in terms of order, size, maximum degree, Zagreb indices and harmonic index. They also established some identities between the hyper-Zagreb index and its coindices. Nezhad and Azari [11] also studied some bounds on hyper-Zagreb index in terms of several graph parameters.

Akhter et al. [12] investigated the class of l-apex trees and determined the bounds on Zagreb index of the first kind and an upper bound on the Zagreb index of the second kind. Moreover, they characterized the corresponding graphs in this family. Recently, Wang et al. [13] obtained bounds for the class of apex trees and l-apex trees with respect to weighted Harary indices. Javaid et al. [14] studied bounds of Zagreb indices for the class of k-generalized quasi unicyclic graphs and obtained extremal graphs corresponding to the extremal values. In this paper, we study the extremal properties of the zeroth-order Randić index and the hyper-Zagreb index in the class of l-generalized quasi trees or the class of l-apex trees in short.

In Section 2, we discuss the main results related to zeroth-order Randić and hyper-Zagreb indices. In Section 3, we carry the QSPR analysis of COVID-19 drugs with zeroth-order Randić and hyper-Zagreb indices (energy) and end up the article with the conclusion.

2. Discussion and Main Results

We start this section with some auxiliary lemmas [3].

Lemma 1.

Let $x,y\in V\left(G\right)$ and $xy\notin E\left(G\right)$. Then

(i) 

${}^{0}R(G+xy)<{}^{0}R\left(G\right).$

(ii) 

$HM(G+xy)>HM\left(G\right).$

Lemma 2.

Let $G\in Q{T}_l^n$. The the following statements are satisfied.

(i) 

If G has minimum zeroth-order Randić index and w is a quasi vertex of $G,$ then w has maximum degree, i.e., d(w) = n − 1.

(ii) 

If G has maximum hyper-Zagreb index and w is a quasi vertex of G, then w has maximum degree, i.e., $d\left(w\right)=n-1.$

(iii) 

If G has minimum hyper-Zagreb index and w is a quasi vertex of G, then d(w) = 2.

Proof. 

Let $G\in Q{T}_l^n$ with minimum zeroth-order Randić index and let w be its quasi vertex. Assume a contrary that $d\left(w\right)<n-1$. Then, there is at least one vertex u such that $wu\notin E\left(G\right)$. Clearly, $G+wu\in Q{T}_l^n$ and ${}^{0}R(G+wu)<{}^{0}R\left(G\right)$ which is a contradiction against the minimality of ${}^{0}R\left(G\right)$. Hence, we have $d\left(w\right)=n-1$.

On similar lines we can obtain the result for the hyper-Zagreb index.

Now, for (iii), consider $G\in Q{T}_l^n$ with minimum value of the hyper-Zagreb index. Since, w is a quasi vertex, $d\left(w\right)\ne 1$. Suppose that $d\left(w\right)>2$. Then, for any edge $uw\in E\left(G\right)$, we have $HM(G-uw)<HM\left(G\right)$ and $G-uw\in Q{T}_l^n$, which is a contradiction. Thus, $d\left(w\right)=2$. Moreover, w is adjacent to two vertices of $V-V_l$. □

Lemma 3.

Let n, $y_i(1\le i\le n)$, a, $m>1$ be integers with $y_1+y_2+\cdots +y_n=a$. Then, the function $\varphi (y_1,y_2,\cdots ,y_n;a)={\sum }_{i=1}^n\frac{1}{\sqrt{y_i}}$ gives the minimum value if and only if $|y_i-y_j|\le 1$ for every $1\le i,j\le n$. For $y_1\ge y_2\ge m$, $\varphi (y_1,y_2,\cdots ,y_n;a)$ gives maximum only for $y_1=a-m-n+2$, $y_2=m$, $y_j=1$, where $3\le j\le n$ and the second maximum is obtained only for $y_1=a-m-n+1$, $y_2=m+1$, $y_j=1$, where $3\le j\le n$.

Proof. 

Since ${\varphi }^{\prime }\left(y\right)=-\frac{1}{2y^{3/2}}+\frac{1}{2{(y+1)}^{3/2}}<0$, for $y>0$, the function $\varphi \left(y\right)=\frac{1}{\sqrt{y}}-\frac{1}{\sqrt{y+1}}$ is strictly decreasing for $x>0$. If $y\ge x+2>0$, then $y-1>x$ and $\varphi (y-1)<\varphi \left(x\right)$. In other words $\frac{1}{\sqrt{y}}+\frac{1}{\sqrt{x}}>\frac{1}{\sqrt{y-1}}+\frac{1}{\sqrt{x+1}}$. This shows that the function $\varphi (y_1,y_2,\cdots ,y_n;a)$ is minimum if and only if $|y_i-y_j|\le 1$ for every $1\le i,j\le n$.

Now for the maximum value, if $y\ge x\ge 2,$ then $y>x-1$ and $\varphi \left(y\right)<\varphi (x-1).$ In other words $\frac{1}{\sqrt{y}}+\frac{1}{\sqrt{x}}<\frac{1}{\sqrt{y+1}}+\frac{1}{\sqrt{x-1}}$. This shows that the maximum is obtained only for $y_1=a-m-n+2$, $y_2=m$, $y_j=1$ where $3\le j\le n$, and the second maximum is obtained only for $y_1=a-m-n+1$, $y_2=m+1$, $y_j=1$ where $3\le j\le n$. □

Lemma 4.

Let n, $y_i(1\le i\le n),a\ge 1$ be integers with $y_1+y_2+\cdots +y_n=a$. For $y_1\ge y_2\ge 1$, the function $\varphi (y_1,y_2,\cdots ,y_n;a)={\sum }_{i=1}^n{y}_i^2$ gives the maximum value only for $y_1=a-n-1$, $y_j=1$ where $2\le j\le n$.

Proof. 

The function $\varphi \left(y\right)=y^2-{(y+1)}^2$ is strictly decreasing for $y>0$. If $y\ge x\ge 2,$ then $y>x-1$ and $\varphi \left(y\right)<\varphi (x-1).$ In other words $y^2+x^2<{\left(y+1\right)}^2+{\left(x-1\right)}^2$. This shows that the maximum is obtained only for $y_1=a-n-1$, $y_j=1$ where $2\le j\le n$. □

Lemma 5.

Let G with $x,y,z\in V\left(G\right)$ satisfying that $d\left(x\right)\ge d\left(y\right)$, $xz\notin E\left(G\right)$ and $yz\in E\left(G\right)$. Then, for a new graph H such that $H=G+xz-yz$, we have

$${}^{0}R\left(G\right)<{}^{0}R\left(H\right).$$

Proof. 

Let $d\left(x\right)=i$ and $d\left(y\right)=j$. Then, for the graphs G and H, we have, ${}^{0}R\left(G\right)-{}^{0}R\left(H\right)=\frac{1}{\sqrt{i}}+\frac{1}{\sqrt{j}}-\frac{1}{\sqrt{i+1}}-\frac{1}{\sqrt{j-1}}=\varphi \left(i\right)-\varphi (j-1)<0$, since the function $\varphi \left(y\right)=\frac{1}{\sqrt{y}}-\frac{1}{\sqrt{y+1}}$ is strictly decreasing function for $y>0$. □

Lemma 6.

Let $G\in Q{T}_l^n$. If ${}^{0}R\left(G\right)$ is maximum, then there is a spanning subgraph H of G such that ${}^{0}R\left(G\right)\le {}^{0}R\left(H\right)$. Further, any quasi vertex w is adjacent to two vertices in H which are not quasi vertices, and $d_G\left(w\right)\ge d_H\left(w\right)=2$.

Proof. 

Since G is an l-generalized quasi graph, there exists a subset $A\subset V\left(G\right)$ such that $G-A$ is tree and A is a minimal set with this property. It is clear that $d\left(w\right)\ge 2$ for any $w\in A$. If $\left|E\right(G\left)\right|=m$, then $m\ge 2l+n-l-1=n+l-1,$ and equality holds iff $d\left(w\right)=2$ for every $w\in A$ and no two vertices in A are adjacent. From Lemma 1, deleting some edges it implies that the existence of H, which is may not in $Q{T}_l^n$. □

Let G be a graph such that each vertex of G has a fixed weight $c\ge 1$. Then

$$H{M}_c\left(G\right)=\sum _{uv\in E\left(G\right)}{((d\left(u\right)+c)+(d\left(v\right)+c))}^2=\sum _{xy\in E\left(G\right)}{(d\left(u\right)+d\left(v\right)+2c)}^2$$

Lemma 7.

Let T be a tree of order n such that every vertex of T has a constant weight $c\ge 1$. Then

$$H{M}_c\left(T\right)\le H{M}_c\left(S_n\right),$$

where $S_n$ is the unique tree with the maximum value of $H{M}_c\left(T\right)$.

Proof. 

Let T be a tree such that $T\ncong S_n$, $T\ncong S_{a,b}$ and $H{M}_c\left(T\right)$ is maximum. We have $diam\left(T\right)\ge 4$. It follows that there exist three vertices $x,y,z\in V\left(T\right)$ such that $xy,yz\in E\left(T\right)$ with $d\left(x\right)=r,d\left(y\right)=s,d\left(z\right)=t$, where $r,s,t\ge 1$. Without loss of generality, suppose that $r\ge t$. Let $N\left(x\right)-y=\{x_1,x_2,\cdots ,x_{r-1}\}$ and $N\left(z\right)-y=\{z_1,z_2,\cdots ,z_{t-1}\}$. Now, we construct a new tree $T^{\ast }$ such that $T^{\ast }=T-\{z{z}_1,z{z}_2,\cdots ,z{z}_{t-1}\}+\{x{z}_1,x{z}_2,\cdots ,x{z}_{t-1}\}$. By comparing the values of $H{M}_c$ for T and $T^{\ast }$, we have

$$\begin{array}{cc}\hfill H{M}_c\left(T^{\ast }\right)-H{M}_c\left(T\right)& =\sum _{i=1}^{r-1}{(r+t+d\left(x_i\right)+2c-1)}^2+\sum _{i=1}^{t-1}{(r+t+d\left(y_i\right)+2c-1)}^2\hfill \\ & +{(r+s+t+2c-1)}^2+(s+2c+1)-\sum _{i=1}^{r-1}{(r+d\left(x_i\right)+2c)}^2-\hfill \\ & \sum _{i=1}^{t-1}{(t+d\left(y_i\right)+2c)}^2-{(r+s+2c)}^2-{(t+s+2c)}^2\hfill \\ \hfill & >{(r+s+t+2c-1)}^2+{(s+2c+1)}^2\hfill \\ \hfill & -(r+s+2c)-{(t+s+2c)}^2>0\hfill \end{array}$$

which contradicts the maximality of $H{M}_c\left(T\right)$. To obtain the required result, we show that for $r,t\ge 2$ and $r+t=n$, we have $H{M}_c\left(S_{r,t}\right)<H{M}_c\left(S_n\right)$. For this, we have

$$\begin{array}{cc}\hfill H{M}_c\left(S_n\right)-H{M}_c\left(S_{r,t}\right)& =(n-2){(n+2c)}^2-(r-1){(r+1+2c)}^2\hfill \\ & -(t-1){(t+1+2c)}^2>0\hfill \end{array}$$

since $r<n-1$, which implies that $r+1+2c<n+2c$. Similarly $t+1+2c<n+2c$. □

In the following theorem, we characterized the minimal graph with the minimum zeroth-order Randić index.

Theorem 1.

Let $G\in Q{T}_l^n$, where $n\ge 3$ and $l\ge 1$. Then, we have

$${}^{0}R\left(G\right)\ge \frac{l}{\sqrt{n-l}}+\frac{2}{\sqrt{l+2}}+\frac{n-l-2}{\sqrt{l+2}}.$$

The equality holds if and only if $G\cong K_l+P_{n-l}$.

Proof. 

Let $G\in Q{T}_l^n$ such that G has minimum ${}^{0}R\left(G\right)$. As $G\in Q{T}_l^n$, there exists an l-quasi vertices subset $V_l\subset V\left(G\right)$. Lemma 1 implies that $V_l$ forms a complete subgraph in $G.$ Then, by Lemma 2, we have $G\cong K_l+T_{n-l}$. Now, we have the following:

$$\begin{array}{cc}\hfill {}^{0}R\left(G\right)& ={}^{0}R(K_l+T_{n-l})\hfill \\ \hfill & =\sum _{v\in V\left(K_l\right)}\frac{1}{\sqrt{d\left(v\right)+n-l}}+\sum _{v\in V\left(K_l\right)}\frac{1}{\sqrt{d\left(v\right)+l}}\hfill \\ \hfill & =\frac{l}{\sqrt{n-l}}+\sum _{v\in V\left(K_l\right)}\frac{1}{\sqrt{d\left(v\right)+l}}.\hfill \end{array}$$

By applying Lemma 3 with the fact that every tree has at least two vertices of degree one, the term ${\sum }_{v\in V\left(K_l\right)}\frac{1}{\sqrt{d\left(v\right)+l}}$ reached its minimum value when $T_{n-l}\cong P_{n-l}$. Hence, the identity (1) becomes

$${}^{0}R\left(G\right)\ge \frac{l}{\sqrt{n-l}}+\frac{2}{\sqrt{l+1}}+\frac{n-l-2}{\sqrt{l+2}}$$

and the equality holds if and only if $G\cong K_l+P_{n-l}$. □

The following result gives a maximal value of the zeroth-order Randić index and the corresponding maximal graph in the class of l-generalized quasi trees.

Theorem 2.

Let $G\in Q{T}_l^n$ with $n\ge 3$ and $l\ge 1$.

(i) 

If $n\ge 3$ and l = 1, then ${}^{0}R\left(G\right)\le \frac{1}{\sqrt{n-2}}+n+\sqrt{2}-3$. The equality holds if and only if $G\cong K_1{+}_{a,b}{S}_{n-1}$, where a is the center and b is a pendant vertex of $S_{n-1}$.

(ii) 

If $n\ge 4$ and $l\ge 2$, then ${}^{0}R\left(G\right)\le \frac{1}{\sqrt{n-2}}+\frac{1}{\sqrt{l+2}}+n+l(\frac{1}{\sqrt{2}}-1)-2.$ The equality holds if and only if $G\cong \overline{K_l}{+}_{a,b}{S}_{n-l-2,2}(a,b)$, where a and b are vertices with degrees $n-l-2$ and 2, respectively.

Proof. 

Let $G\in Q{T}_l^n$ such that G has maximum zeroth-order Randić index. As G is an l-generalized quasi tree, there exists an l-quasi vertices subset, say, $V_l$. Lemmas 1, 5 and 6, we deduced the presence of a graph K with vertex set $V\left(K\right)=V\left(G\right)$, ${}^{0}R\left(G\right)\le {}^{0}R\left(K\right)$ and in K, we have: $V_l$ forms an empty graph, i.e., $\overline{K_l}$, $d\left(w\right)=2$ whenever w is a quasi vertex and quasi vertices have common neighbors $w_1,w_2$ in G. So, the graph K can be expressed as $K\cong \overline{K_l}{+}_{w_1,w_2}{T}_{n-l}$ and we have

$$\begin{array}{cc}\hfill {}^{0}R\left(K\right)& ={}^{0}R\left(\overline{K_l}{+}_{w_1,w_2}{T}_{n-l}\right)=\sum _{v\in V\left(\overline{K_l}{+}_{w_1,w_2}{T}_{n-l}\right)}\frac{1}{\sqrt{d\left(v\right)}}\hfill \\ \hfill & =\sum _{v\in V\left(\overline{K_l}\right)}\frac{1}{\sqrt{d\left(v\right)}}+\sum _{v\in V(T_{n-l},v\ne w_1,v\ne w_2)}\frac{1}{\sqrt{d\left(v\right)}}+\frac{1}{\sqrt{d\left(w_1\right)+l}}+\frac{1}{\sqrt{d\left(w_2\right)+l}}\hfill \end{array}$$

From Lemma 3, we found that the term ${\sum }_{v\in V(T_{n-l},v\ne w_1,v\ne w_2)}\frac{1}{\sqrt{d\left(v\right)}}$ is maximum only if $T_{n-l}\cong S_{n-l}$, $w_1$ is the center and $w_2$ is a pendant vertices of $S_{n-l}$, respectively. For $l=1$, we obtain an l-generalized quasi-tree and for $l\ge 2$ this property is no longer valid. We must take the second maximum of the term ${\sum }_{v\in V(T_{n-l},v\ne w_1,v\ne w_2)}\frac{1}{\sqrt{d\left(v\right)}}$. Now, $K\in Q{T}_l^n$, $G\cong K$ and $T_{n-l}\cong S_{n-l-2,2}(w_1,w_2)$. Hence, the result follows. □

The following two results are on the minimality and the maximality of the hyper-Zagreb index for the class of l-generalized quasi trees.

Theorem 3.

Let $G\in Q{T}_l^n$, where $n\ge 5$ and $l\ge 1$. Then, for the hyper-Zagreb index, we have

$$HM\left(G\right)\le 2l(l+1){(n-1)}^2+(l+1)(n-l-1){(n+l)}^2.$$

The equality holds if and only if $G\cong K_l+S_{n-l}$.

Proof. 

Suppose $G\in Q{T}_l^n$ with maximum hyper-Zagreb index. From Lemmas 1 and 2, we deduced that, the set of quasi vertices $V_l$, form a complete subgraph and $G\cong K_l+T_{n-l}$. Now, we need to prove that $T_{n-l}\cong S_{n-l}$. Here, we have

$$\begin{array}{cc}\hfill HM\left(G\right)& =HM(K_l+T_{n-l})\hfill \\ \hfill & =\sum _{xy\in E\left(K_l\right)}{(d_G\left(x\right)+d_G\left(v\right))}^2+\sum _{x\in V\left(K_l\right),y\in V\left(T_{n-l}\right)}{(d_G\left(x\right)+d_G\left(y\right))}^2\hfill \\ & +\sum _{xy\in E\left(T_{n-l}\right)}{(d_G\left(x\right)+d_G\left(v\right))}^2\hfill \\ \hfill & =2l(l-1){(n-1)}^2+l\sum _{x\in V\left(T_{n-l}\right)}{(d_{T_{n-l}}\left(v\right)+n+l-1)}^2\hfill \\ & +\sum _{xy\in E\left(T_{n-l}\right)}{(d_{T_{n-l}}\left(x\right)+d_{T_{n-l}}\left(y\right)+2l)}^2\hfill \end{array}$$

From Lemma 4, the maximum value of the function ${\sum }_{x\in V\left(T_{n-l}\right)}{(d_{T_{n-l}}\left(v\right)+n+l-1)}^2$ is obtained if and only if $T_{n-l}$ has a vertex of degree $n-l-1$, i.e., $T_{n-l}\cong S_{n-l}$. Moreover, by Lemma 7, we find that ${\sum }_{xy\in E\left(T_{n-l}\right)}{(d_{T_{n-l}}\left(x\right)+d_{T_{n-l}}\left(y\right)+2l)}^2$ is maximum only for $T_{n-l}\cong S_{n-l}$. Hence, $G\cong K_l+S_{n-l}$. □

Theorem 4.

Let $G\in Q{T}_l^n$. Then

(i) 

For $n\ge 3$ and $l=1$, we have

$$HM\left(G\right)\ge 16n$$

and the equality is achieved if and only if $G\cong C_n$.

(ii) 

For $n\ge 4$ and $l\ge 2$, we have

$$HM\left(G\right)\ge \left\{\begin{array}{c}136,n=4\hfill \\ 16n+70,n\ge 5\hfill \end{array}\right.$$

and the equality is achieved if and only if $G\cong A,$ where

(i) 

A is a graph of 2 cycles of length 3 with a common edge for $n=4$, or

(ii) 

A is a graph of 2 cycles having a common path of length at least two for $n\ge 5$, or

(iii) 

A is a graph of 2 cycles joined by a path of length at least two for $n\ge 7$.

Proof. 

Let $G\in Q{T}_l^n$ be such that G has the smallest value of hyper-Zagreb index. Let $V_l$ be a subset of quasi vertices of $V\left(G\right)$. Then, by Lemma 2 (iii), $d\left(w\right)=2$ for every $w\in V_l$. Moreover, w is adjacent to two vertices of $V-V_l$. This implies that G is connected graph with $n+l-1$ edges, and thus G has l cycles.

First we show that G has no pendant vertices. On contrary suppose that there is a pendant vertex, say, v. Then, there exist a path $v,x,\cdots ,u$, which joins the vertex v to the vertex u which belongs to a cycle denoted by C in G. Suppose $N\left(x\right)=\{x_1,x_2,\cdots ,x_a\}$ and $d\left(x_i\right)=a_i$ for $1\le i\le a$. We have $d\left(x\right)=a+1$. It follows that $t\ge 1$ and $a_i\ge 2$ for at least one i. Now, we construct a new l-cyclic graph $G^{\ast }$, with n vertices such that $G^{\ast }=G-vx+v{v}_1+v{v}_2$, where $v_1$ and $v_2$ are two consecutive vertices of C. Let $d_G\left(v_1\right)=r$ and $d_G\left(v_2\right)=s$, where $r,s\ge 2$. We obtain $d_{G^{\ast }}\left(x\right)=a,d_{G^{\ast }}\left(v_1\right)=r,d_{G^{\ast }}\left(v_2\right)=s,d_{G^{\ast }}\left(v\right)=2$ and $d_{G^{\ast }}\left(x_i\right)=a_i$ for every $1\le i\le a$. In addition, we have the following

$$\begin{array}{cc}\hfill HM\left(G\right)-HM\left(G^{\ast }\right)& =\sum _{i=1}^a{(a+1+a_i)}^2+{(a+2)}^2+{(r+s)}^2\hfill \\ & -\sum _{i=1}^a{(a+a_i)}^2-{(r+2)}^2-{(s+2)}^2>0.\hfill \end{array}$$

for $a\ge 2,$ and the equality holds only for $r=2$ or $s=2$. This is a contradiction against the minimality of $HM\left(G\right)$. Hence, $a=1$ and x is a pendant vertex of $G^{\ast }$. Now, we will repeat the same procedure on the pendant vertex x. In this way, we will find an l-cyclic graph of order n with a pendant vertex adjacent to a vertex u on C. For $d\left(u\right)\ge 3$, we have $a\ge 2$ and $HM\left(G\right)-HM\left(G^{\ast }\right)>0$, a contradiction. Assume that all vertices of G have degree greater than or equal to two.

(i) If $l=1$, then G is a connected unicyclic graph with no pendant vertices, hence $G\cong C_n$.

(ii) If $l=2$, then G is a connected bicyclic graph with no pendant vertices. From handshaking theorem, we have ${\sum }_{v\in V\left(G\right)}=2(n+1)$. This implies that the degree sequence of G is $D_1=[3^2,2^{n-2}]$ or $D_2=[4^1,2^{n-1}]$.

If the degree sequence is $D_1,$ then

(a) G consists of two cycles with a common path of length greater than or equal to one $(p\ge 1)$, or

(b) G consists of two cycles joined by a path of length greater than or equal to one, $(p\ge 1)$.

If the degree sequence is $D_2$, then

(c) G consists of two cycles with a common vertex.

In cases of (a) and (b), if $p=1$, then $HM\left(G\right)=16n+72=A_1$. If $p\ge 2$, then $HM\left(G\right)=16n+70=A_2$. For case (c), we have $HM\left(G\right)=16n+96=A_3$. Clearly, we have $A_3>A_1>A_2$. Hence, the minimum of $HM\left(G\right)$ can be achieved only for the graphs lie in (b), but for $n=4,5$, we have unique graphs which lie in (a). For $n=6$, we have two extremal graphs: a graph with $C_5$ and $C_4$ with a common path $P_3,$ and a graph with $C_5$ and $C_5$ with a common path $P_4$. □

3. An Application of Zeroth-Order Randić and Hyper-Zagreb Indices for the COVID-19 Drugs

COVID-19 is a pandemic which is caused by severe acute respiratory syndrome coronavirus 2 (SARS-CoV-2), a new coronavirus. SARS-CoV-2 is a positive single-stranded RNA virus containing proteins. There are several drugs which have been used as the treatment of this pandemic, such as chloroquine, hydroxychloroquine, azithromycin, remdesivir, lopinavir, ritonavir, arbidol, favipiravir, theaflavin, thalidomide, and ribavirin (see [15]). Various topological indices were calculated to be used in QSPR and QSAR models of drugs used for the treatment of COVID-19 [16,17,18,19,20,21,22]. Some of these drugs showed good effects, while others have no effect for this disease. In the present study, we consider lopinavir, favipiravir, and ritonavir, along with their analogs. CID10009410, CID44271905, CID3010243, and CID271958 structures which are structural analogs of lopinavir, CID89869520 structure which is favipiravir analog, and lopinavir-d8 (CID71749833) which is ritonavir analog are considered. The values of enthalpy of vaporization (E), flash point (FP), molar refractivity (MR), polarizability (P), and molar volume (MV) of these potential drugs against COVID-19 are taken from ChemSpider [23]. The structures of the drugs used in the regression analysis are show in Figure 2 and Figure 3. Table 1 shows the physicochemical properties of potential drugs that can be used as treatment of COVID-19 and the values of the zeroth-order Randić index, the hyper-Zagreb index and the hyper-Zagreb energy.

Figure 2. Chemical structures of lopinavir and its analogs.

Figure 3. Chemical structures of favipiravir, ritonavir, and their analogs.

Table 1. The physicochemical properties of potential drugs which can be a treatment of COVID-19 and their corresponding topological indices.

PubChem ID	E	FP	MR	P	MV	${}^0\mathit{R}$	HM	${\mathcal{E}}_{\mathit{HM}}$
CID10009410	141.1	513.7	179.2	71	544.7	33.9237	1138	1299.38
CID44271905	140.8	512.7	179.2	71	540.5	33.0535	1100	1263.26
CID44271958	138.9	505.1	169.5	67.2	507.9	31.313	1032	1199.54
CID3010243	140	509.5	174.6	69.2	522.7	32.3464	1088	1242.76
CID89869520	63.2	185.5	41.3	16.4	110	9.30096	288	323.739
CID71749833	140.8	512.7	179.2	71	540.5	39.8988	1454	1595.41

From Table 2, we see that the zeroth-order Randić index is better correlated with the physicochemical properties of COVID-19 drugs as compared to the hyper-Zagreb index and hyper-Zagreb energy. The hyper-Zagreb index is the least related among these three graph invariants.

Table 2. Correlation of ${}^{0}R$, HM and ${\mathcal{E}}_{HM}$ with the physicochemical properties of potential drugs which can be used as treatment of COVID-19.

	E	FP	MR	P	MV
${}^{0}R$	0.961998682	0.961835272	0.968022428	0.967940266	0.96851878
HM	0.926811693	0.926613386	0.934964522	0.934848043	0.935564875
${\mathcal{E}}_{HM}$	0.948446222	0.948281522	0.955063957	0.954972667	0.955515338

From Table 3, the signifiant observation is that $R^2$ is high (average $0.93$) for the zeroth-order Randić index and the physicochemical properties of COVID-19 drugs. The second highest is for the hyper-Zagreb energy (average $0.91$) and the least is for the hyper-Zagreb index.

Table 3. Coefficient of determination $R^2$ with the physicochemical properties of potential drugs to be used which can be used as treatment of COVID-19 along with ${}^{0}R$, HM and ${\mathcal{E}}_{HM}$.

	E	FP	MR	P	MV
${}^{0}R$	0.9254	0.9251	0.9371	0.9369	0.938
HM	0.859	0.8586	0.8742	0.8739	0.8753
${\mathcal{E}}_{HM}$	0.8996	0.8992	0.9121	0.912	0.913

The following linear regression models give the best estimate for the physicochemical properties of potential drugs which can be used as treatment of COVID-19 along with the zeroth order Randić index, the hyper-Zagreb index and the hyper-Zagreb energy.

$$\begin{array}{cc}\hfill {}^{0}R& =0.322E-11.173,{}^{0}R=0.0765FP-4.9656,{}^{0}R=0.1851MR+1.4973,\hfill \\ \hfill {}^{0}R& =0.4674P+1.4777,{}^{0}R=0.0593MV+2.6237,HM=11.391E-435.35,\hfill \\ \hfill HM& =2.7006FP-216.26,HM=6.5488MR+9.2379,HM=16.535P+8.5837,\hfill \\ \hfill HM& =2.0989MV+48.963,{\mathcal{E}}_{HM}=0.322E-11.173,HM=0.322FP-11.173,\hfill \\ \hfill {\mathcal{E}}_{HM}& =0.322MR-11.173,HM=0.322P-11.173,HM=0.322MV-11.173.\hfill \end{array}$$

4. Conclusions

An l-generalized quasi tree of order n is defined as a graph G for which there exists a subset $W\subset V\left(G\right)$ with $\left|W\right|=l$ such that $G-W$ is a tree but for any $Y\subset V\left(G\right)$ and $\left|Y\right|<l$, $G-Y$ is not a tree. In this paper, we investigated the bounds on the zeroth-order Randić and the hyper-Zagreb indices. For the zeroth-order Randić index, $K_l+P_{n-l}$ gives the minimum value while $S_{n-1}$ and $S_{n-l-2,2}(a,b)$ gives the maximum value for $l=1$ and $l\ge 2$, respectively. $K_l+S_{n-l}$ gives the maximum value of the hyper-Zagreb index. The unique graph $C_n$ gives the minimum value for the hyper-Zagerb index for $l=1$, while for $l=2$, the graphs are not unique. Finding the minimum value graph with respect to the hyper-Zagreb index for $l\ge 3$ will be an interesting problem to investigate. Besides the regression analysis of physicochemical properties of COVID-19 drugs and the topological indices (${}^{0}R,HM,{\mathcal{E}}_{HM}$), we see that they give better correlation. Based on this observation, these drugs proved that they are very effective for the treatment of this pandemic.