The Evolutionary Dynamics of a Sex-Structured Population with Non-Overlapping Generations
Abstract
1. Introduction
2. Model Assumptions
3. Model Description
4. Fixed Points and Their Stability
- Monomorphic fixed point with respect to allele a has the following coordinates:where .In this case, females and males in the population are represented only by individuals with the aa genotype, while the AA and Aa genotypes are absent.
- Monomorphic fixed point with respect to allele A has the coordinateswhere . The fixed point corresponds to the situation when the population consists of individuals from both sex groups with only the AA genotype.
- Polymorphic fixed point has the coordinateswhere and , ,which has its meaning if the following conditions hold: , , and . Therefore, equilibrium exists when , or , and . In the case of polymorphism, females and males in the population are represented by three genotypes: AA, Aa, and aa.
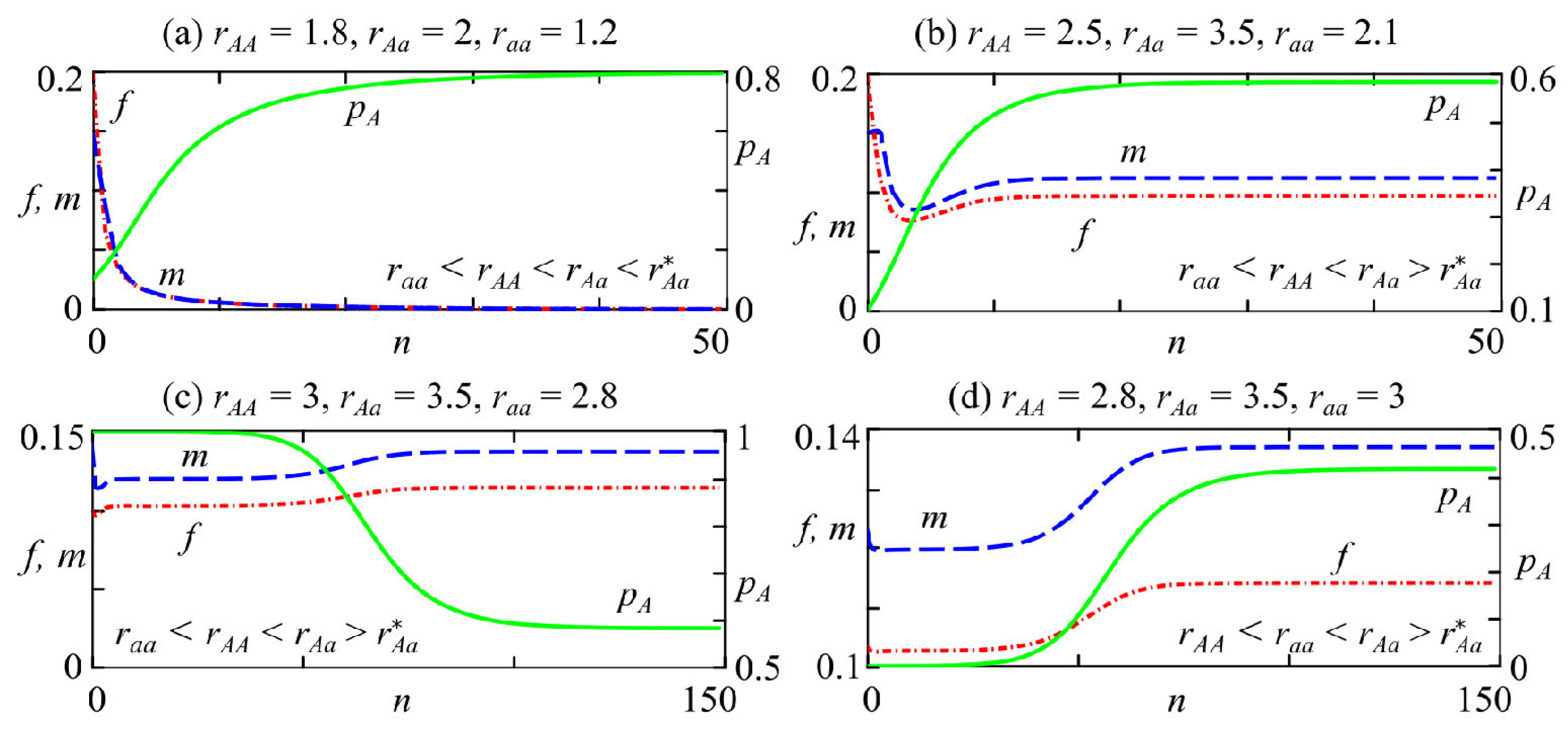
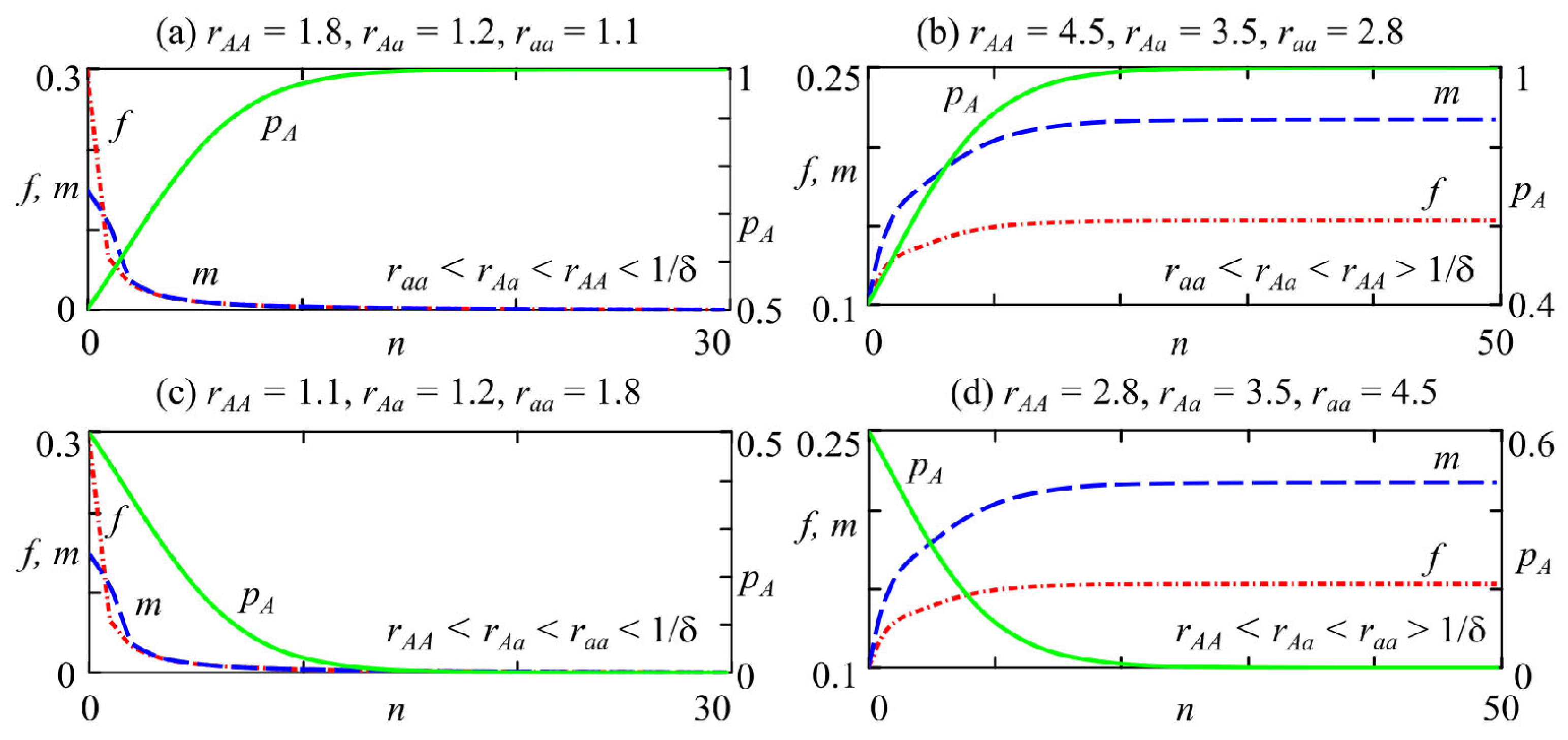
5. Type of Selection and Dynamics of System (2)
5.1. Increased Reproductive Potential of Heterozygotes
5.2. Intermediate Values of the Reproductive Potential of Heterozygotes
5.3. Reduced Reproductive Potential of Heterozygotes
6. Dynamics of the Genetic Composition of the Population with Changes in the Reproductive Potential of Genotypes
7. Discussion
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix A.1. Dynamic Equations of Allele Frequencies in a Population with a Sex Structure and Non-Overlapping Generations under Natural Selection
- For deriving such a model, let us consider a breeding scheme with the following assumptions [35]: The trait is located on an autosome, which means that both parents determine the genotype of the offspring.
- The production of gametes of all genotypes is assumed to be equal in the male (gfij = g2) and female (gmij = g1) part of the population, i.e., males and females may have different production gametes, but within one sex, all genotypes have the same one.
- Gene frequencies in the male and female parts of the population may differ.
| The n-th Generation | ||||||
| Females | Males | |||||
| Genotypes | AA | Aa | aa | AA | Aa | aa |
| Genotype frequencies | pAA | pAa | paa | qAA | qAa | qaa |
| The (n + 1)th Generation | ||||||
| Gametes | A | a | A | a | ||
| Gamete frequencies | pA~ pAA+ pAa/2 | pa~ paa+ pAa/2 | qA~ qAA+ qAa/2 | qa~ qaa+ qAa/2 | ||
| Zygotes | AA | Aa | aa | |||
| Fitnesses | wAA | wAa | waa | vAA | vAa | vaa |
| Zygote frequencies | pAA, qAA ~ pAqA | pAa, qAa ~ pAqa + paqA | paa, qaa ~ paqa | |||
Appendix A.2. Population Dynamics in a Case When the Birth Rate of a Population Is Determined Genetically: A Trait Is Not Sex-Limited
References
- Darwin, C. On the Origin of Species; John Murray: London, UK, 1859. [Google Scholar]
- Fisher, R.A. The Genetical Theory of Natural Selection; Clarendon Press: Oxford, UK, 1930. [Google Scholar]
- Wright, S. The genetical theory of natural selection: A review. J. Hered. 1930, 21, 340–356. [Google Scholar] [CrossRef]
- Haldane, J.B.S. The Causes of Evolution; Longman Green: London, UK, 1932. [Google Scholar]
- Chetverikov, S.S. About some moments of evolutionary process from the point of view of contemporary genetics. Zhurnal Eksp. Biol. 1926, 2, 3–54. (In Russian) [Google Scholar]
- Verhulst, P.F. Deuxième Mémoire sur la loi d’Accroissement de la Population; Mémoires de l’Académie Royale des Sciences, des Lettres et des Beaux-Arts de Belgique; Hayez: Bruxelles, Belgium, 1847; Volume 20, pp. 1–32. [Google Scholar]
- Volterra, V. Variations and fluctuations of the number of individuals in animal species living together. ICES J. Mar. Sci. 1928, 3, 3–51. [Google Scholar] [CrossRef]
- Gauze, G.F. Analysis of struggle for survival in mixed populations. Zool. Zhurnal 1935, 14, 243–270. [Google Scholar]
- Thompson, J.N. Rapid evolution as an ecological process. Trends Ecol. Evol. 1998, 13, 329–332. [Google Scholar] [CrossRef] [PubMed]
- Duffy, M.A.; Sivars-Becker, L. Rapid evolution and ecological host-parasite dynamics. Ecol. Lett. 2007, 10, 44–53. [Google Scholar] [CrossRef] [PubMed]
- Ellner, S.P.; Geber, M.A.; Hairston, N.G. Does rapid evolution matter? Measuring the rate of contemporary evolution and its impacts on ecological dynamics. Ecol. Lett. 2011, 14, 603–614. [Google Scholar] [CrossRef] [PubMed]
- Greenman, J.V.; Benton, T.G.; Boots, M.; White, A.R. The evolution of oscillatory behavior in age-structured species. Am. Nat. 2005, 166, 68–78. [Google Scholar] [CrossRef][Green Version]
- Kausrud, K.L.; Mysterud, A.; Steen, H.; Vik, J.O.; Østbye, E.; Cazelles, B.; Framstad, E.; Eikeset, A.M.; Mysterud, I.; Solhøy, T.; et al. Linking climate change to lemming cycles. Nature 2008, 456, 93–97. [Google Scholar] [CrossRef]
- Barraquand, F.; Louca, S.; Abbott, K.C.; Cobbold, C.A.; Cordoleani, F.; DeAngelis, D.L.; Elderd, B.D.; Fox, J.W.; Greenwood, P.; Hilker, F.M.; et al. Moving forward in circles: Challenges and opportunities in modelling population cycles. Ecol. Lett. 2017, 20, 1074–1092. [Google Scholar] [CrossRef]
- Pimentel, D. Population regulation and genetic feedback. Science 1968, 159, 1432–1437. [Google Scholar] [CrossRef]
- Roughgarden, J. Density-dependent natural selection. Ecology 1971, 52, 453–468. [Google Scholar] [CrossRef]
- Charlesworth, B. Selection in density-regulated populations. Ecology 1971, 52, 469–474. [Google Scholar] [CrossRef]
- Frisman, E.; Shapiro, A. Fluctuations in the Size of Isolated Single-Species Populations and Natural Selection. Biom. J. 1982, 24, 531–542. [Google Scholar] [CrossRef]
- Frisman, E.Y.; Zhdanova, O.L. Evolutionary transition to complex population dynamic patterns in a two-age population. Russ. J. Genet. 2009, 45, 1124–1133. [Google Scholar] [CrossRef]
- Tyutyunov, Y.; Zhadanovskaya, E.; Bourguet, D.; Arditi, R. Landscape refuges delay resistance of the European corn borer to Bt-maize: A demo-genetic dynamic model. Theor. Popul. Biol. 2008, 74, 138–146. [Google Scholar] [CrossRef]
- Tyutyunov, Y.V.; Kovalev, O.V.; Titova, L.I. Spatial demogenetic model for studying phenomena observed upon introduction of the ragweed leaf beetle in the South of Russia. Math. Model. Nat. Phenom. 2013, 8, 80–95. [Google Scholar] [CrossRef][Green Version]
- De Vries, C.; Caswell, H. Selection in two-sex stage-structured populations: Genetics, demography, and polymorphism. Theor. Popul. Biol. 2019, 130, 160–169. [Google Scholar] [CrossRef]
- De Vries, C.; Caswell, H. Stage-structured evolutionary demography: Linking life histories, population genetics, and ecological dynamics. Am. Nat. 2019, 193, 545–559. [Google Scholar] [CrossRef]
- De Vries, C.; Desharnais, R.A.; Caswell, H. A matrix model for density-dependent selection in stage-classified populations, with application to pesticide resistance in Tribolium. Ecol. Model. 2020, 416, 108875. [Google Scholar] [CrossRef]
- Neverova, G.P.; Zhdanova, O.L.; Frisman, E.Y. Effects of natural selection by fertility on the evolution of the dynamic modes of population number: Bistability and multistability. Nonlinear Dyn. 2020, 101, 687–709. [Google Scholar] [CrossRef]
- Neverova, G.P.; Frisman, E.Y. Dynamic modes of population size and its genetic structure for species with nonoverlapping generations and stage development. Commun. Nonlinear Sci. Numer. Simul. 2021, 94, 105554. [Google Scholar] [CrossRef]
- Tyutyunov, Y.V. Spatial Demo-Genetic Predator–Prey Model for Studying Natural Selection of Traits Enhancing Consumer Motility. Mathematics 2023, 11, 3378. [Google Scholar] [CrossRef]
- Wright, S. Evolution and the Genetics of Populations. Vol. 2: The Theory of Gene Frequencies; University of Chicago Press: Chicago, IL, USA, 1969; 512p. [Google Scholar]
- Barton, N.; Briggs, D.; Eisen, J.; Goldstein, D.; Patel, N. Evolution; Cold Spring Harbor Laboratory Press: New York, NY, USA, 2007. [Google Scholar]
- Yamamichi, M.; Hoso, M. Roles of maternal effects in maintaining genetic variation: Maternal storage effect. Evolution 2017, 71, 449–457. [Google Scholar] [CrossRef] [PubMed]
- De Aquino Soares, A.; Wardil, L.; Klaczko, L.B.; Dickman, R. Hidden role of mutations in the evolutionary process. Phys. Rev. E 2021, 104, 044413. [Google Scholar] [CrossRef] [PubMed]
- Charlesworth, B. Selection in populations with overlapping generations. I. The use of Malthusian parameters in population genetics. Theor. Popul. Biol. 1970, 1, 352–370. [Google Scholar] [CrossRef] [PubMed]
- Charlesworth, B. Selection in populations with overlapping generations. III. Conditions for genetic equilibrium. Theor. Popul. Biol. 1972, 3, 377–395. [Google Scholar] [CrossRef] [PubMed]
- Charlesworth, B.; Giesel, J.T. Selection in populations with overlapping generations. II. Relations between gene frequency and demographic variables. Am. Nat. 1972, 106, 388–401. [Google Scholar] [CrossRef]
- Ratner, V.A. A mathematical theory of evolution of Mendelian populations. In Problems of Evolution; Nauka Pub: Novosibirsk, Russia, 1973; Volume 3, pp. 151–213. [Google Scholar]
- Gottlieb, L.D. Genetic stability in a peripheral isolate of Stephanomeria exigua ssp. coronaria that fluctuates in population size. Genetics 1974, 76, 551–556. [Google Scholar] [CrossRef]
- Gaines, M.S.; Leroy, R.; McClenaghan, L.R., Jr.; Rose, R.K. Temporal patterns of allozymic variation in fluctuating populations of Microtus ochrogaster. Evolution 1978, 32, 723–739. [Google Scholar] [CrossRef] [PubMed]
- Frisman, E.Y. Primary Genetic Divergence (Theoretical Analysis and Modeling); Dal’nevost. Nauch. Tsentr Akad. Nauk SSSR: Vladivostok, Russia, 1986. (In Russian) [Google Scholar]
- Frisman, E.; Kulakov, M. Transition from bi- to quadro-stability in models of population dynamics and evolution. Mathematics 2023, 11, 4134. [Google Scholar] [CrossRef]
- Ginzburg, E.K. Description of the Inheritance of Quantitative Traits; Nauka: Novosibirsk, Russia, 1984. (In Russian) [Google Scholar]
- Abrams, P.A.; Matsuda, H. Prey adaptation as a cause of predator–prey cycles. Evolution 1997, 51, 1742–1750. [Google Scholar] [CrossRef] [PubMed]
- Morozov, A.Y.; Pasternak, A.F.; Arashkevich, E.G. Revisiting the role of individual variability in population persistence and stability. PLoS ONE 2013, 8, e70576. [Google Scholar] [CrossRef] [PubMed]
- Farkas, J.Z.; Morozov, A.Y. Modelling effects of rapid evolution on persistence and stability in structured predator-prey systems. Mathem. Model. Nat. Phenom. 2014, 9, 26–46. [Google Scholar] [CrossRef]
- Cortez, M.H.; Weitz, J.S. Coevolution can reverse predator–prey cycles. Proc. Natl. Acad. Sci. USA 2014, 111, 7486–7491. [Google Scholar] [CrossRef] [PubMed]
- Bukin, Y.S. Coevolution in a predator–prey system: An ecogenetic model. Russ. J. Genet. Appl. Res. 2014, 4, 543–548. [Google Scholar] [CrossRef]
- Yamamichi, M. Effects of rapid evolution on population cycles and extinction in predator–prey systems. In Diversity of Functional Traits and Interactions; Mougi, A., Ed.; Springer: Singapore, 2020; pp. 19–49. [Google Scholar] [CrossRef]
- Mougi, A. Rapid evolution of prey maintains predator diversity. PLoS ONE 2019, 14, e0227111. [Google Scholar] [CrossRef]
- Baker, R.; Pleimling, M. The effect of habitats and fitness on species coexistence in systems with cyclic dominance. J. Theor. Biol. 2020, 486, 110084. [Google Scholar] [CrossRef]
- Zhdanova, O.; Frisman, E. Alternative attractors in an ecological-genetic model of populations with non-overlapping generations. Ecol. Complex. 2017, 31, 135–143. [Google Scholar] [CrossRef]
- Bertram, J.; Masel, J. Density-dependent selection and the limits of relative fitness. Theor. Popul. Biol. 2019, 129, 81–92. [Google Scholar] [CrossRef]
- Frisman, E.; Zhdanova, O.; Neverova, G.P. Ecological and genetic models in population biophysics. Biophysics 2020, 65, 810–825. [Google Scholar] [CrossRef]
- Yamamichi, M. How does genetic architecture affect eco-evolutionary dynamics? A theoretical perspective. Philos. Trans. R. Soc. B 2022, 377, 20200504. [Google Scholar] [CrossRef] [PubMed]
- Nei, M.; Maruyama, T.; Chakraborty, R. The bottleneck effect and genetic variability in populations. Evolution 1975, 29, 1–10. [Google Scholar] [CrossRef]
- Frankham, R.; Lees, K.; Montgomery, M.E.; England, P.R.; Lowe, E.H.; Briscoe, D.A. Do population size bottlenecks reduce evolutionary potential? Anim. Conserv. 1999, 2, 255–260. [Google Scholar] [CrossRef]
- Ploshnitsa, A.I.; Goltsman, M.E.; Happ, G.; Macdonald, M.D.W.; Kennedy, L.J. Historical and modern neutral genetic variability in Mednyi Arctic foxes passed through a severe bottleneck. J. Zool. 2013, 289, 68–76. [Google Scholar] [CrossRef]
- Olazcuaga, L.; Lincke, B.; DeLacey, S.; Durkee, L.F.; Melbourne, B.A.; Hufbauer, R.A. Population demographic history and evolutionary rescue: Influence of a bottleneck event. Evol. Appl. 2023, 16, 1483–1495. [Google Scholar] [CrossRef] [PubMed]
- Gomulkiewicz, R.; Holt, R.D. When does evolution by natural selection prevent extinction? Evolution 1995, 49, 201–207. [Google Scholar] [CrossRef]
- Orr, H.A.; Unckless, R.L. The Population Genetics of Evolutionary Rescue. PLoS Genet. 2014, 10, e1004551. [Google Scholar] [CrossRef]
- Hedrick, P.W.; Fredrickson, R.J. Blackwell Publishing Ltd Captive breeding and the reintroduction of Mexican and red wolves. Mol. Ecol. 2008, 17, 344–350. [Google Scholar] [CrossRef]
- Aguilar, A.; Roemer, G.; Debenham, S.; Binns, M.; Garcelon, D.; Wayne, R.K. High MHC diversity maintained by balancing selection in an otherwise genetically monomorphic mammal. Proc. Natl. Acad. Sci. USA 2004, 101, 3490–3494. [Google Scholar] [CrossRef]
- Weber, D.S.; Stewart, B.S.; Schienman, J.; Lehman, N. Major histocompatibility complex variation at three class II loci in the northern elephant seal. Mol. Ecol. 2004, 13, 711–718. [Google Scholar] [CrossRef]
- Luenser, K.; Fickel, J.; Lehnen, A.; Speck, S.; Ludwig, A. Low level of genetic variability in European bisons (Bison bonasus) from the Bialowieza National Park in Poland. Eur. J. Wildl. Res. 2005, 51, 84–87. [Google Scholar] [CrossRef]
- O’Brien, S.J.; Wildt, D.E.; Bush, M.; Caro, T.M.; FitzGibbon, C.; Aggundey, I.; Leakey, R.E. East African cheetahs: Evidence for two population bottlenecks? Proc. Natl. Acad. Sci. USA 1987, 84, 508–511. [Google Scholar] [CrossRef] [PubMed]
- Dempster, E.R. Maintenance of genetic heterogeneity. In Cold Spring Harbor Symposia on Quantitative Biology; Cold Spring Harbor Laboratory Press: New York, NY, USA, 1955; Volume 20, pp. 25–32. [Google Scholar]
- Haldane, J.B.S.; Jayakar, S.D. Polymorphism due to selection of varying direction. J. Genet. 1963, 58, 237–242. [Google Scholar] [CrossRef]
- Svirezhev, Y.M.; Pasekov, V.P. Fundamentals of Mathematical Genetics; Nauka: Moscow, Russia, 1982; 512p. [Google Scholar]
- Hedrick, P.W. Genetic polymorphism in a temporally varying environment: Effects of delayed germination or diapause. Heredity 1995, 75, 164–170. [Google Scholar] [CrossRef]
- Zhdanova, O.L.; Frisman, E.Y. Genetic polymorphism under cyclical selection in long-lived species: The complex effect of age structure and maternal selection. J. Theor. Biol. 2021, 512, 110564. [Google Scholar] [CrossRef] [PubMed]
- Zhdanova, O.L.; Frisman, E.Y. Mathematical modeling of selection by sex-limited trait: To the question of maintenance of litter size polymorphism in natural populations of arctic foxes. Rus. J. Genet. 2021, 57, 227–237. [Google Scholar] [CrossRef]
- Turelli, M.; Schemske, D.W.; Bierzychudek, P. Stable two-allele polymorphisms maintained by fluctuating fitnesses and seed banks: Protecting the blues in Linanthus parryae. Evolution 2001, 55, 1283–1298. [Google Scholar] [PubMed]
- Dobrynin, P.; Liu, S.; Tamazian, G.; Xiong, Z.; Yurchenko, A.A.; Krasheninnikova, K.; O’Brien, S.J. Genomic legacy of the African cheetah, Acinonyx jubatus. Genome Biol. 2015, 16, 277. [Google Scholar] [CrossRef]
- Axenovich, T.I.; Zorkoltseva, I.V.; Akberdin, I.R.; Beketov, S.V.; Kashtanov, S.N.; Zakharov, I.A.; Borodin, P.M. Inheritance of litter size at birth in farmed arctic foxes (Alopex lagopus, Canidae, Carnivora). Heredity 2007, 98, 99–105. [Google Scholar] [CrossRef]
- Lack, D. The Natural Regulation of Animal Numbers; Oxford University Press: New York, NY, USA, 1954. [Google Scholar]
- Ricker, W.E. Stock and recruitment. J. Fish. Res. Board Can. 1954, 5, 559–623. [Google Scholar] [CrossRef]
- Dazho, R. Fundamentals of Ecology; Progress: Moscow, Russia, 1975; 416p. [Google Scholar]
- Odum, Y. Fundamentals of Ecology; Mir: Moscow, Russia, 1975. [Google Scholar]
- Baltensweiler, W.; Rubli, D. Dispersal: An important driving force of the cyclic population dynamics of the larch bud moth. For. Snow Landsc. Res. 1999, 74, 3–153. [Google Scholar]
- Esper, J.; Büntgen, U.; Frank, D.C.; Nievergelt, D.; Liebhold, A. 1200 years of regular outbreaks in alpine insects. Proc. R. Soc. B Biol. Sci. 2007, 274, 671–679. [Google Scholar] [CrossRef] [PubMed]
- Baltensweiler, W. The relevance of changes in the composition of larch bud moth populations for the dynamics of its numbers. In Dynamics of Populations, Proceedings of the Advanced Study Institute on ‘Dynamics of Numbers in Populations’, Oosterbeek, The Netherlands, 7–18 September 1970; den Boer, P.J., Gradwell, G.R., Eds.; Centre for Agricultural Publishing and Documentation: Wageningen, The Netherlands, 1971; pp. 208–219. Available online: https://edepot.wur.nl/469421#page=206 (accessed on 20 October 2023).
- Myers, J.H.; Cory, J.S. Population cycles in forest Lepidoptera revisited. Ann. Rev. Ecol. Evol. Syst. 2013, 44, 565–592. [Google Scholar] [CrossRef]
- Frisman, E.Y.; Revutskaya, O.L.; Neverova, G.P. Complex dynamic modes of a population with age and sex structures. Dokl. Biol. Sci. 2010, 431, 152–156. [Google Scholar] [CrossRef] [PubMed]
- Revutskaya, O.; Neverova, G.; Frisman, E. Complex dynamic modes in a two-sex age-structured population model. In Models of the Ecological Hierarchy: From Molecules to the Ecosphere; Jordán, F., Jørgensen, S.E., Eds.; Elsevier: Amsterdam, The Netherlands, 2012; pp. 149–162. [Google Scholar]
- Revutskaya, O.L.; Kulakov, M.P.; Neverova, G.P.; Frisman, E.Y. Changing of the dynamics modes in populations with age and sex structure. Dokl. Biol. Sci. 2017, 477, 239–243. [Google Scholar] [CrossRef]
- Revutskaya, O.L.; Frisman, E.Y. Harvesting impact on population dynamics with age and sex structure: Optimal harvesting and the hydra effect. Comput. Res. Model. 2022, 14, 1107–1130. [Google Scholar] [CrossRef]
- Severtsov, A.S. Basics of the Theory of Evolution; MSU: Moscow, Russia, 1987; 320p. [Google Scholar]
- Kuznetsov, A.P.; Sedova, J.V. Bifurcations of three- and four-dimensional maps: Universal properties. Izvestiya VUZ Appl. Nonlinear Dyn. 2012, 20, 26–43. (In Russian) [Google Scholar] [CrossRef]
- Grant, V. The Evolutionary Process: A Critical Review of Evolutionary Theory; Columbia University Press: New York, NY, USA, 1985; 499p. [Google Scholar]
- Bouzat, J.L. Conservation genetics of population bottlenecks: The role of chance, selection, and history. Conserv. Genet. 2010, 11, 463–478. [Google Scholar] [CrossRef]
- Frisman, E.Y.; Neverova, G.P.; Revutskaya, O.L. Complex dynamics of the population with a simple age structure. Ecol. Model. 2011, 222, 1943–1950. [Google Scholar] [CrossRef]
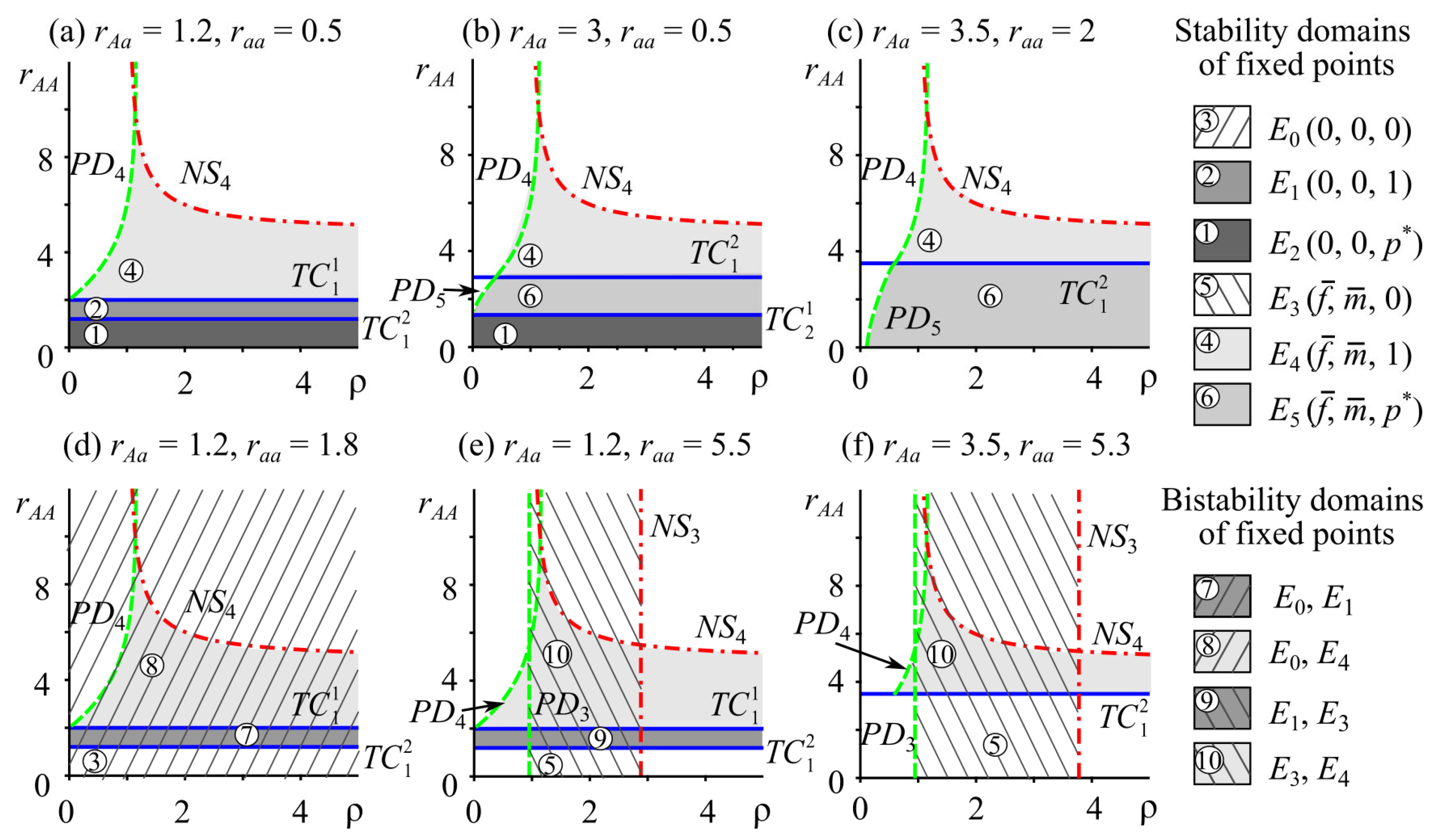
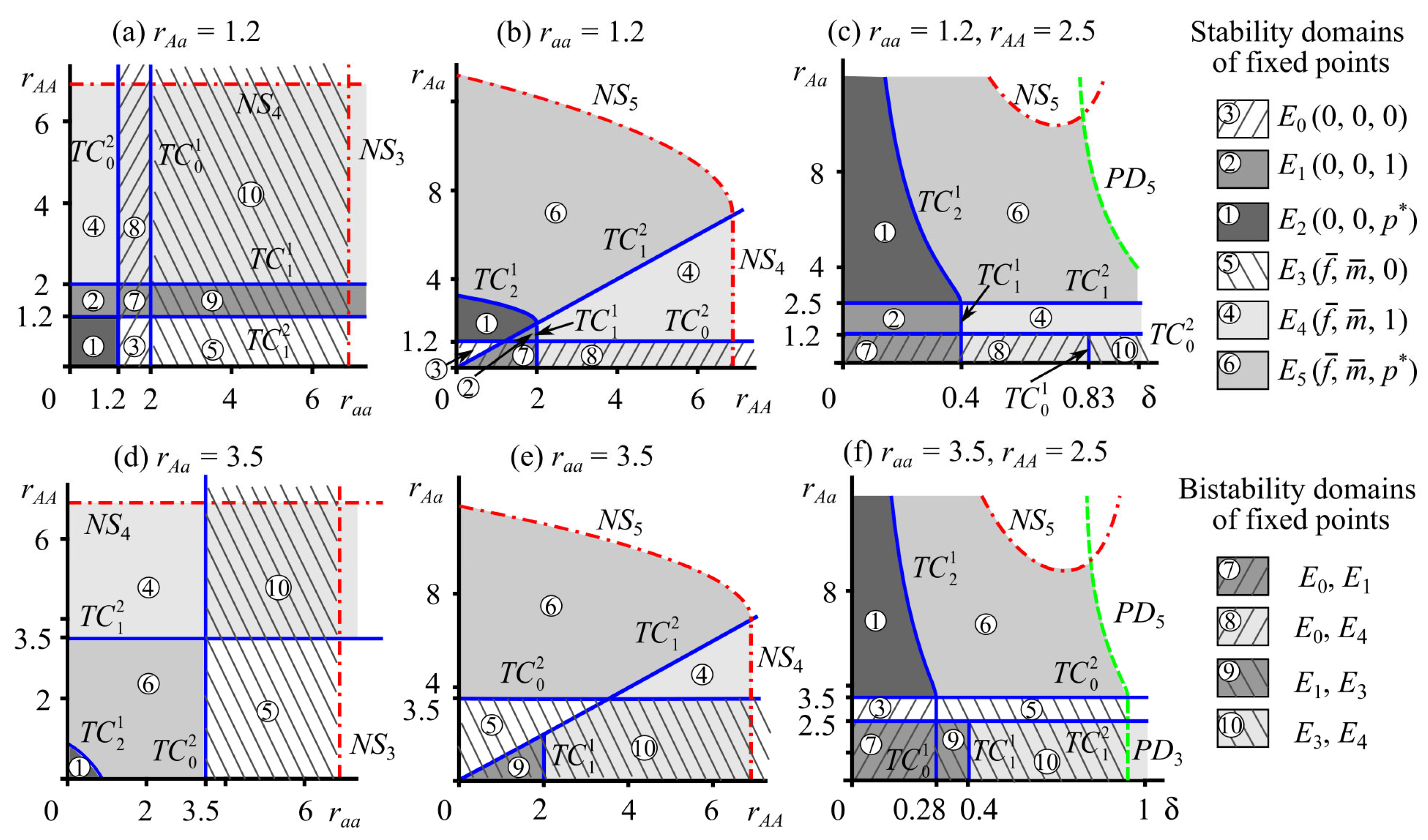
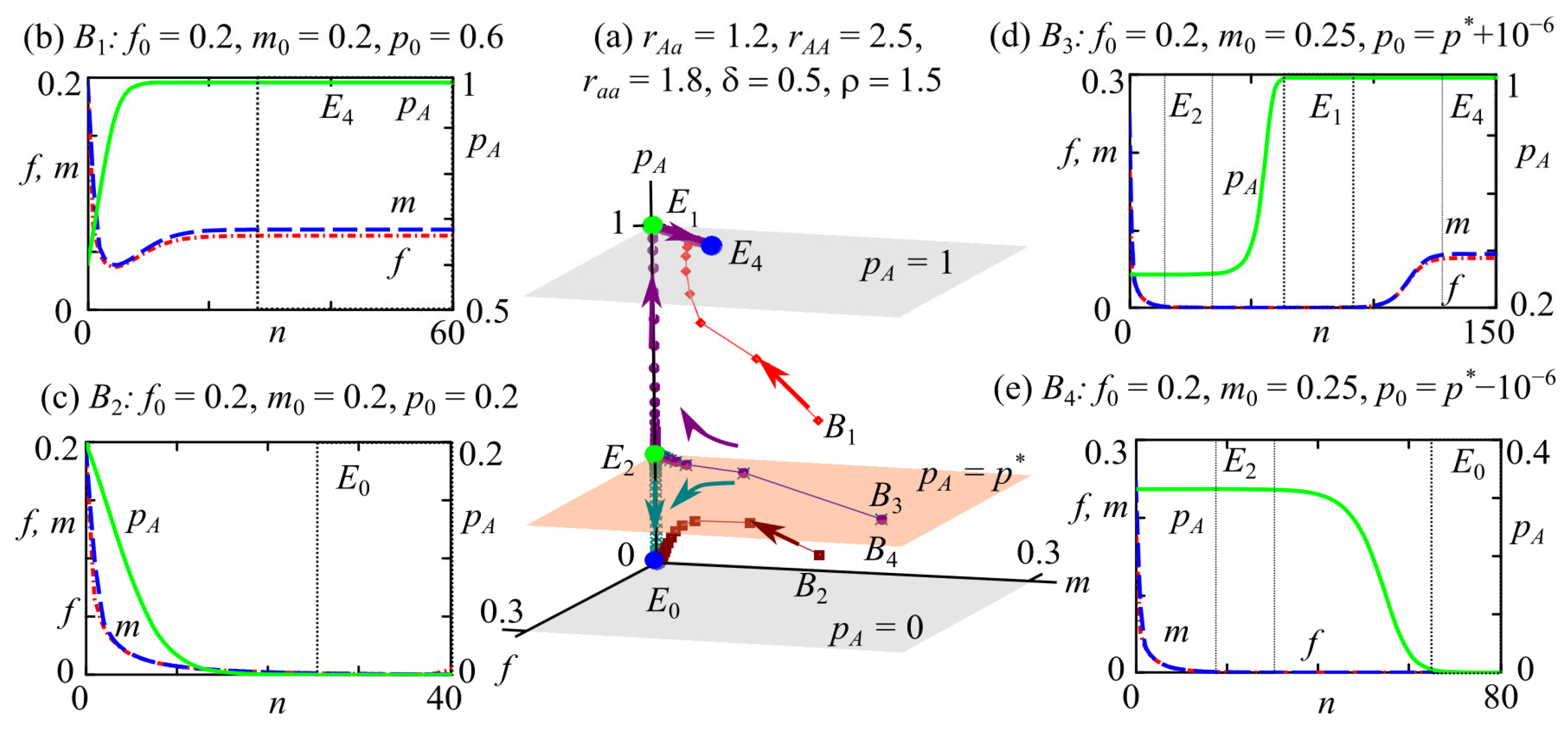
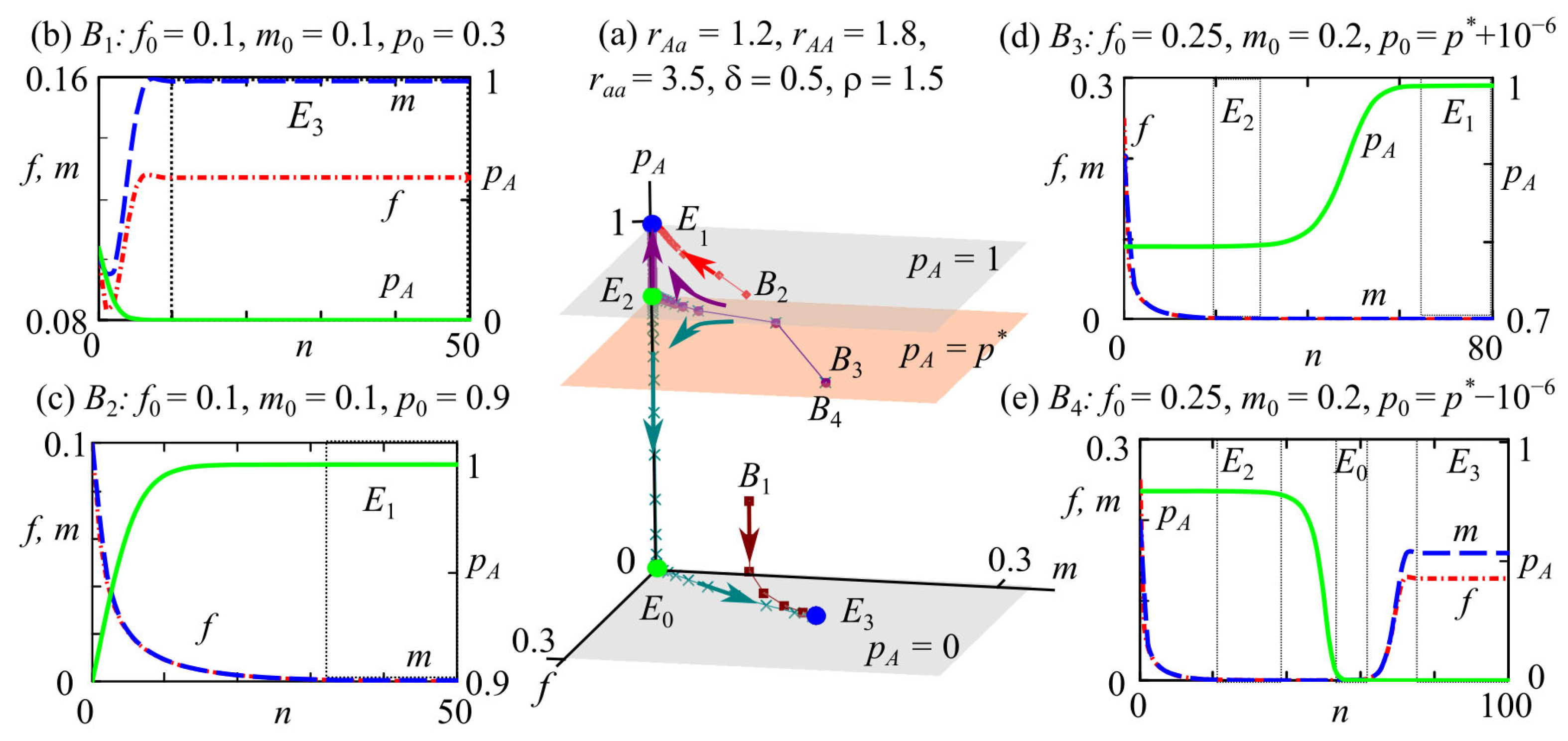
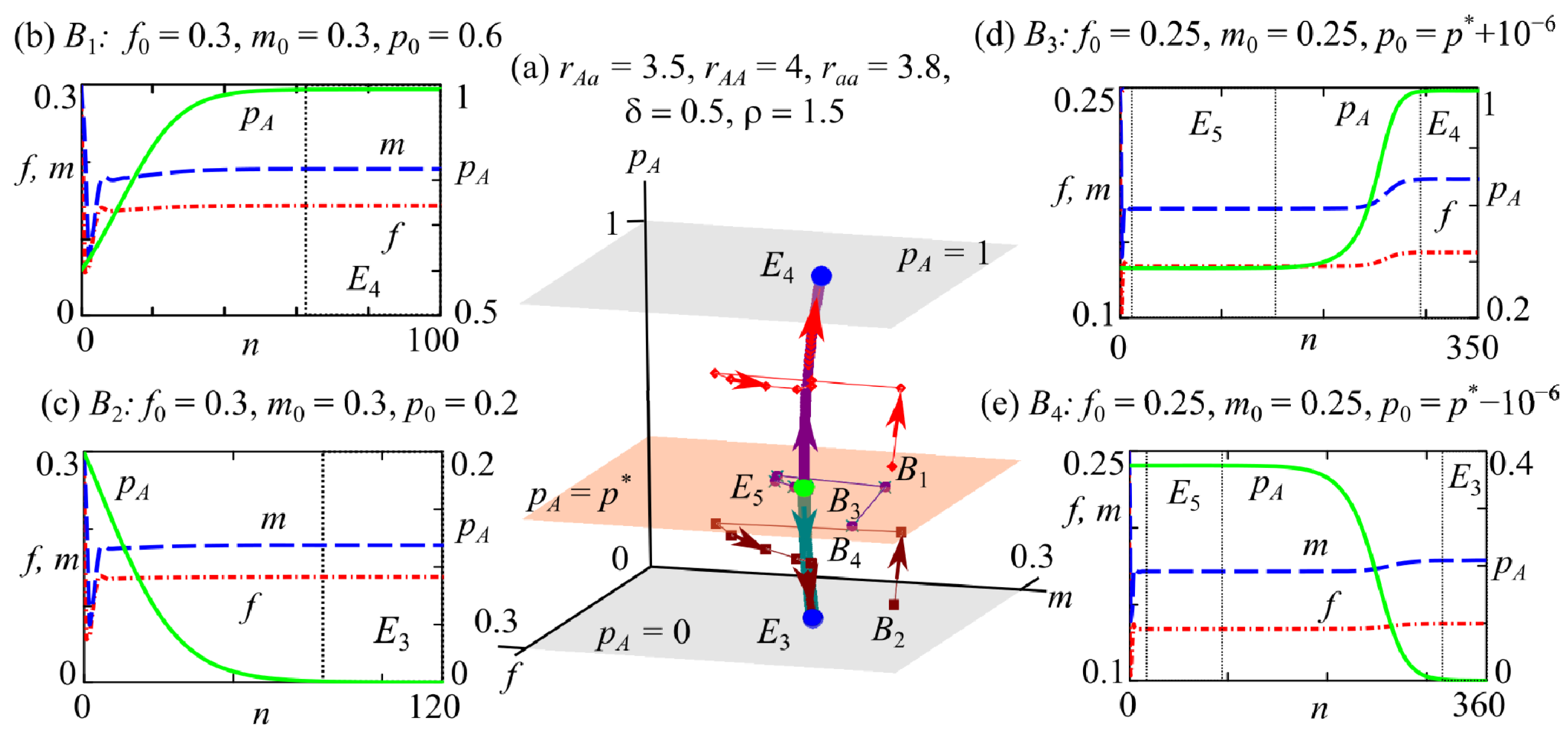

| Fixed Points | The Boundaries of Stability Domains | |
|---|---|---|
| Transcritical bifurcation line (TC) | ||
| E0, E3 | : ; : | |
| E1, E4 | : ; : | |
| E2, E5 | : ; : ; : | |
| Period doubling bifurcation line (PD) | Neimark–Sacker bifurcation line (NS) | |
| E3 | : | : |
| E4 | : | : |
| E5 | : , , | : , , |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Revutskaya, O.; Neverova, G.; Zhdanova, O.; Frisman, E. The Evolutionary Dynamics of a Sex-Structured Population with Non-Overlapping Generations. Mathematics 2023, 11, 4971. https://doi.org/10.3390/math11244971
Revutskaya O, Neverova G, Zhdanova O, Frisman E. The Evolutionary Dynamics of a Sex-Structured Population with Non-Overlapping Generations. Mathematics. 2023; 11(24):4971. https://doi.org/10.3390/math11244971
Chicago/Turabian StyleRevutskaya, Oksana, Galina Neverova, Oksana Zhdanova, and Efim Frisman. 2023. "The Evolutionary Dynamics of a Sex-Structured Population with Non-Overlapping Generations" Mathematics 11, no. 24: 4971. https://doi.org/10.3390/math11244971
APA StyleRevutskaya, O., Neverova, G., Zhdanova, O., & Frisman, E. (2023). The Evolutionary Dynamics of a Sex-Structured Population with Non-Overlapping Generations. Mathematics, 11(24), 4971. https://doi.org/10.3390/math11244971







