Equilibrium Strategy of Production and Order in a Two-Echelon Supply Chain with Demand Information Updates and Capacity Restriction
Abstract
:1. Introduction
- (1)
- What is the optimal production-and-order strategy in a two-echelon supply chain with demand information updates and capacity restrictions?
- (2)
- How are the decisions and performance of the supply chain affected by the variable wholesale prices and the quality of demand information updates?
- (3)
- Is there an equilibrium strategy of production and order among the supply chain members?
2. Literature Review
2.1. Production and Ordering Strategy with Demand Information Updates
2.2. Production Mode Selection in Inventory Models
2.3. Research Gap and Contribution
3. Model Description
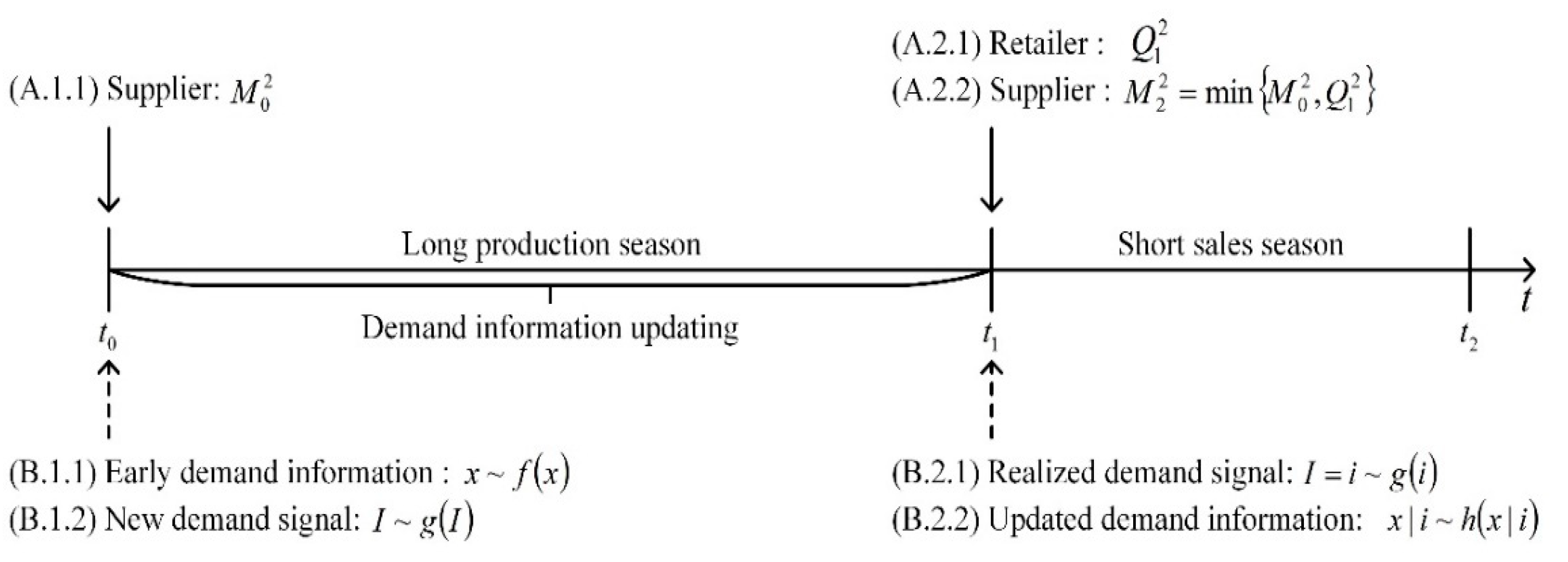
3.1. The Progress of Demand Information Updates
3.2. Costs
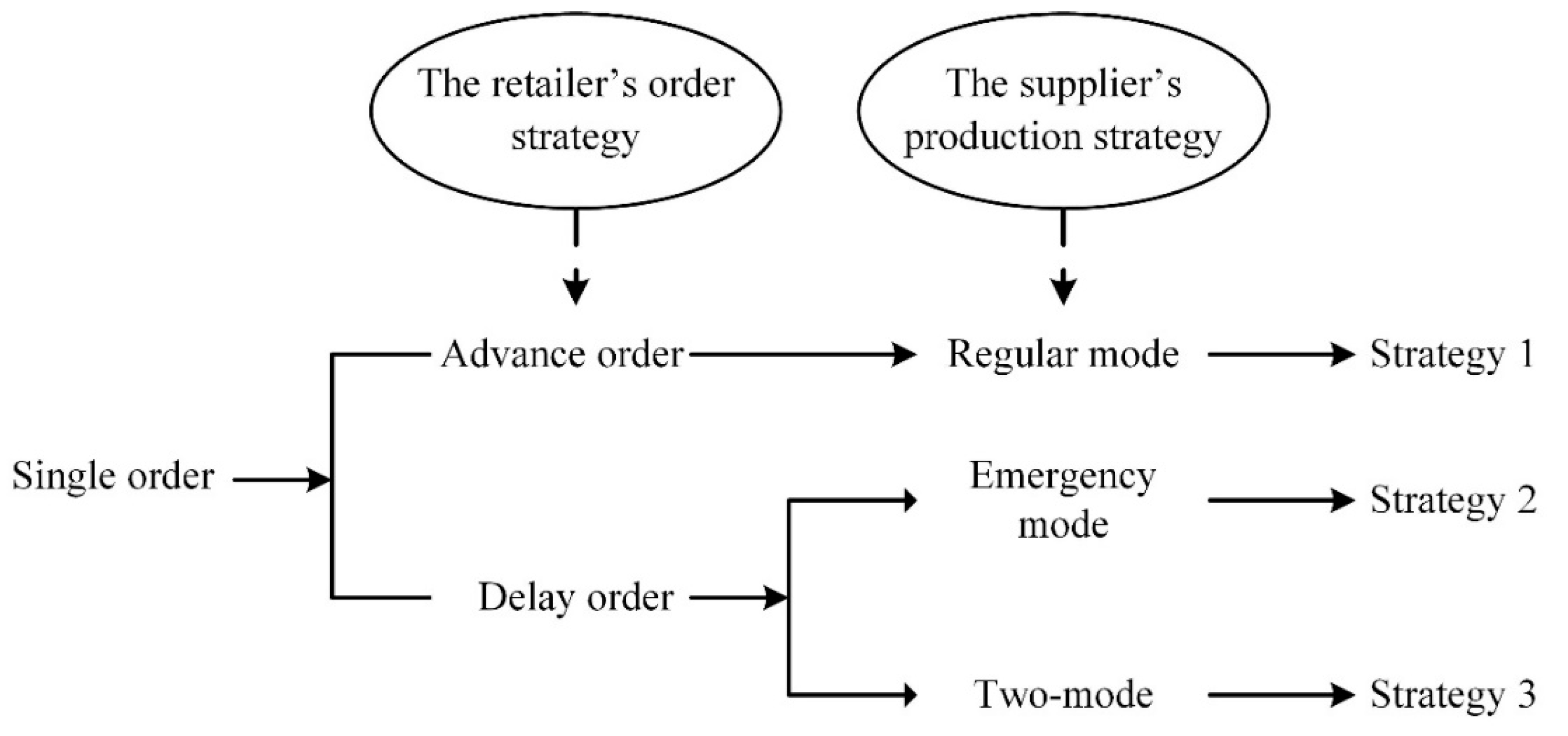
3.3. The Decision Progress in Three Strategies
3.4. Notation and Assumption
4. The Decision Analysis
4.1. Strategy 1: Advance Order with Regular Mode
4.2. Strategy 2: Delay Order with Emergency Mode
4.3. Strategy 3: Delay Order with Two-Mode Production
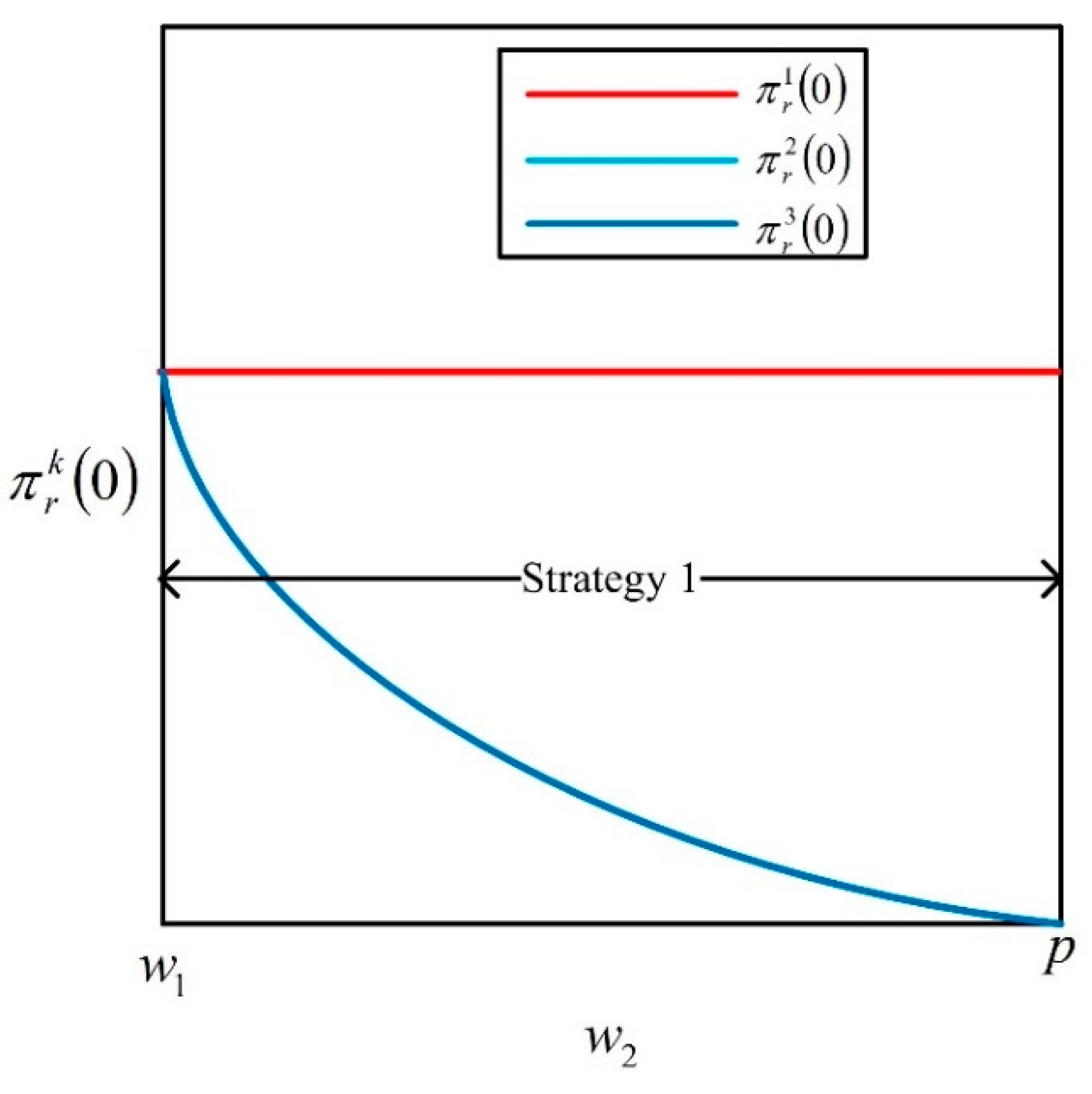
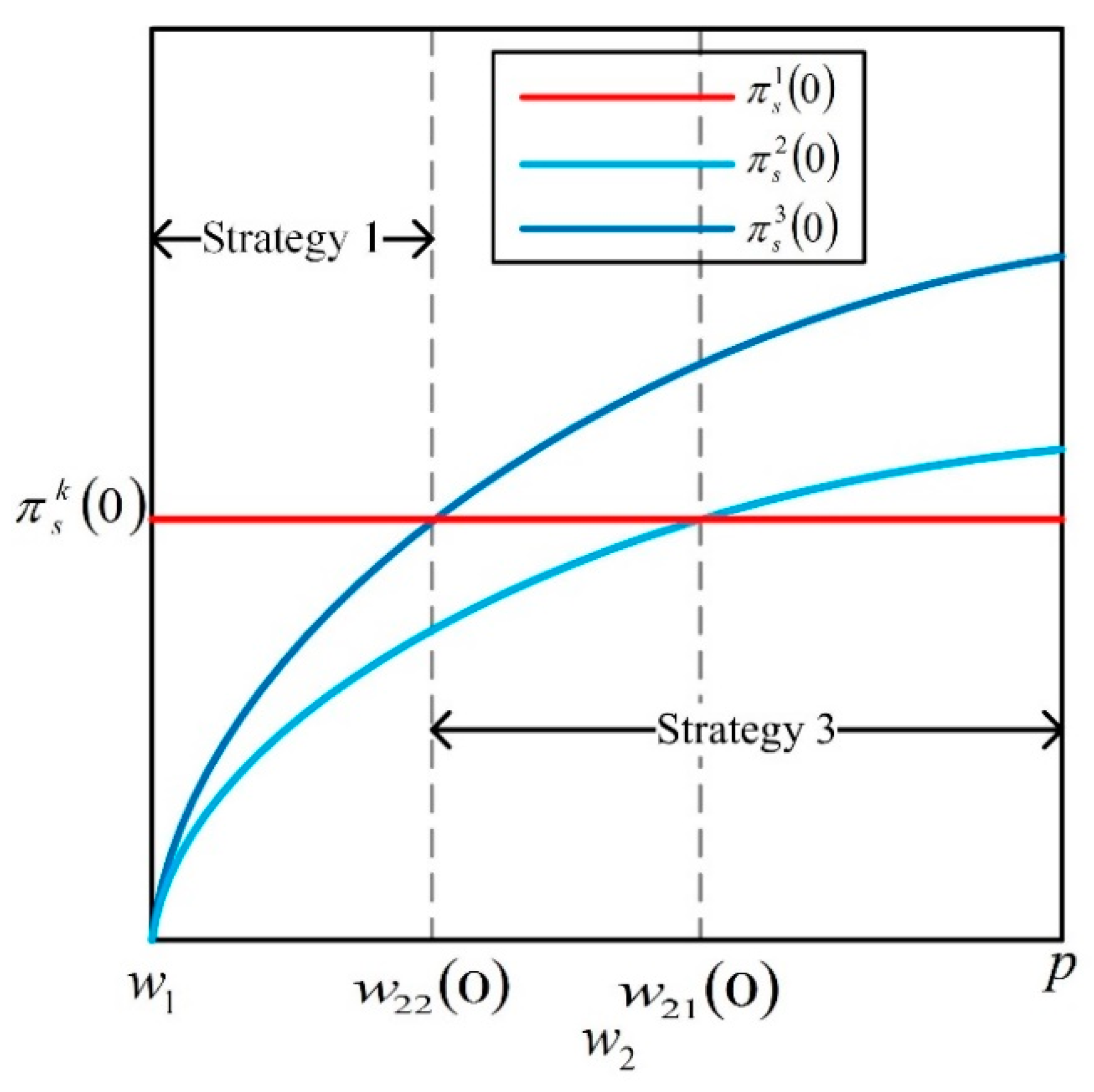
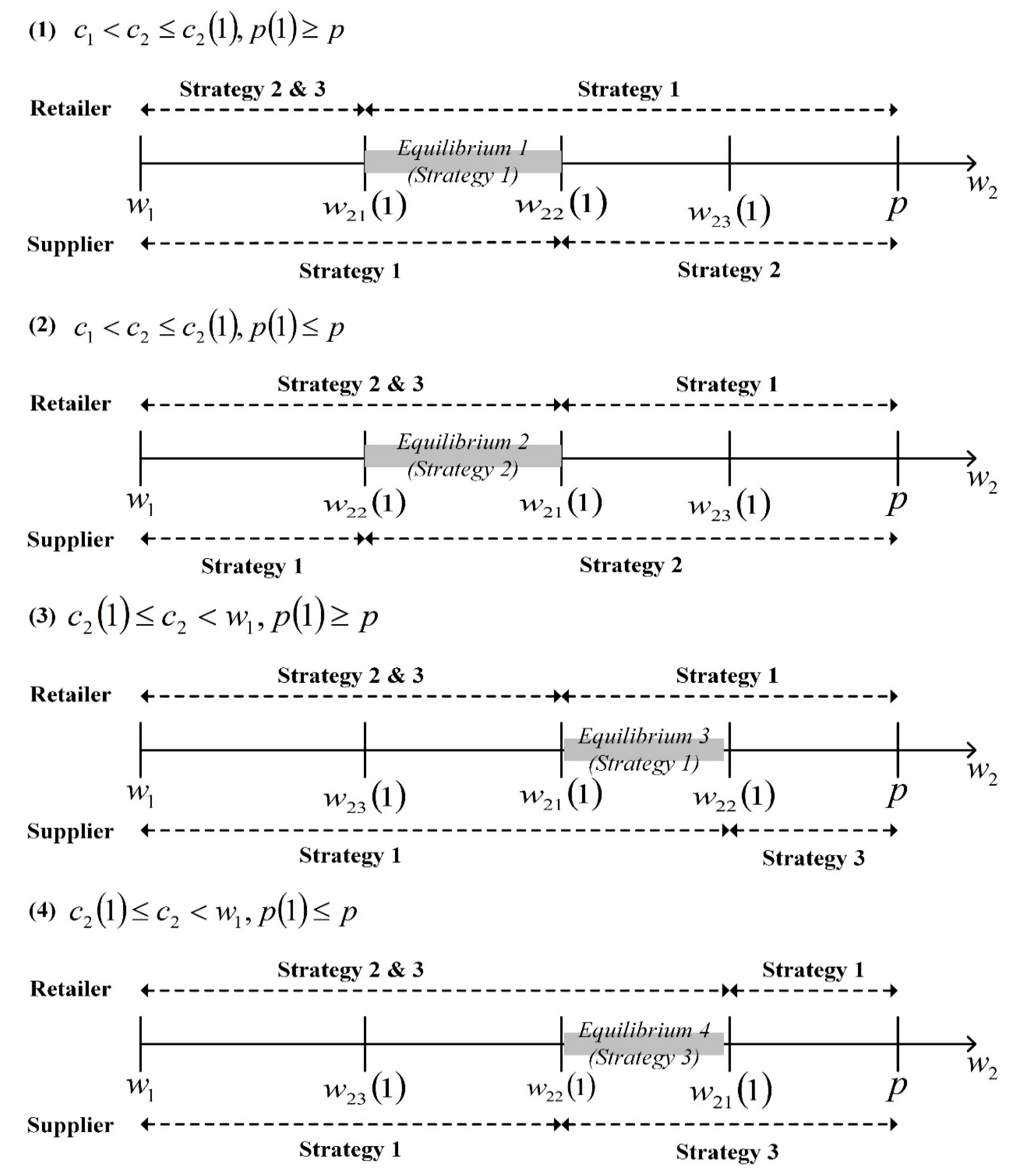
5. Strategy Selection
5.1. Imperfect Demand Signal with
5.2. Perfect Demand Signal with
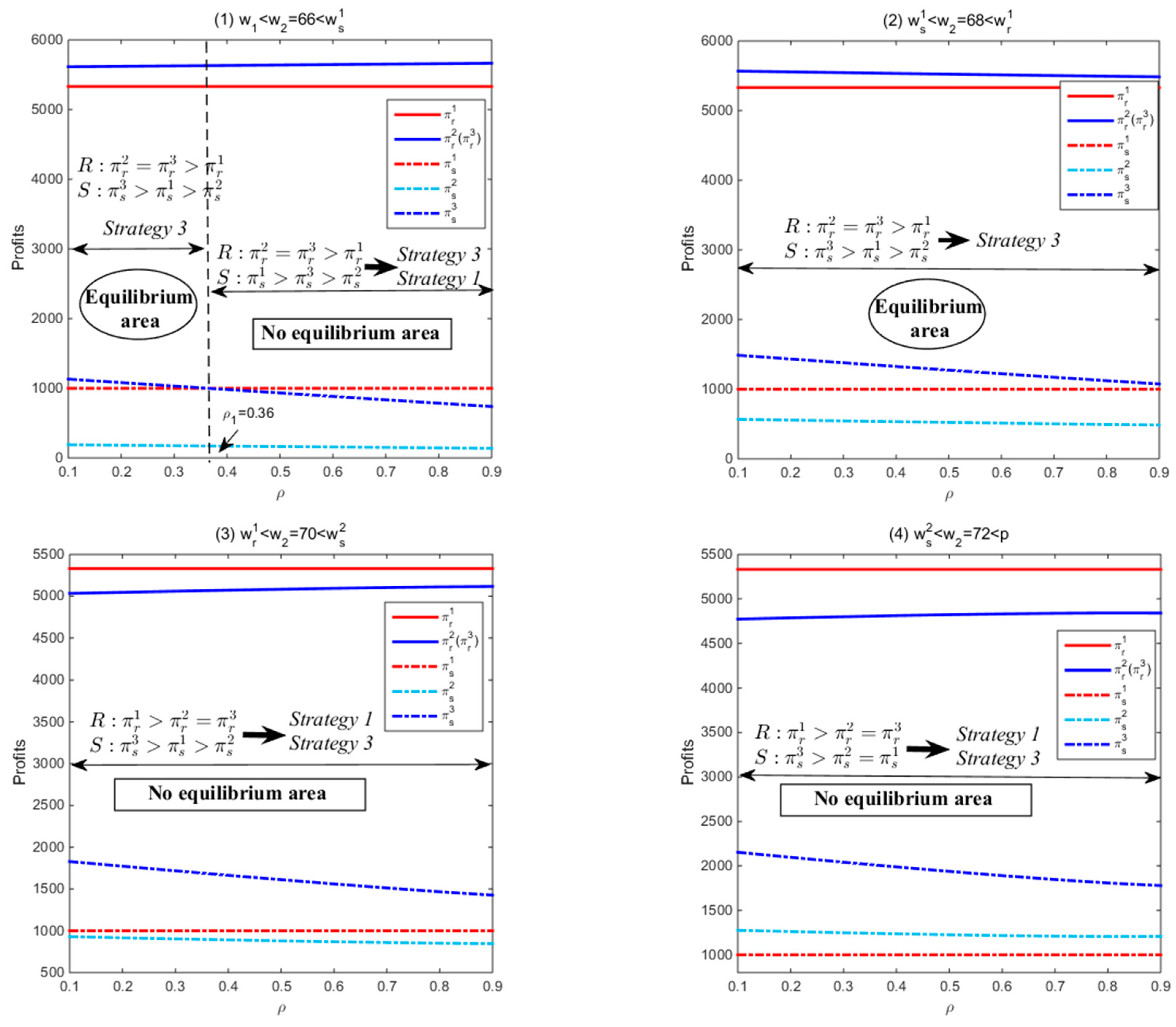
6. Numerical Simulation
7. Conclusions and Management Insights
7.1. Conclusions and Theoretical Contribution
7.2. Management Insights and Practical Application
7.3. Future Research
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Chew, E.P.; Lee, L.H.; Sim, C.K. The impact of supply chain visibility when lead time is random. OR Spectr. 2013, 35, 163–190. [Google Scholar] [CrossRef]
- Voigt, G.; Inderfurth, K. Supply chain coordination and setup cost reduction in case of asymmetric information. OR Spectr. 2011, 33, 99–122. [Google Scholar] [CrossRef]
- Zhai, Y.Z.; Xue, W.L.; Ma, L.J. Commitment decisions with demand information updates and a capital-constrained supplier. Int. Trans. Oper. Res. 2020, 27, 2294–2316. [Google Scholar] [CrossRef]
- Jin, M.; Wu, S.D. Capacity reservation contracts for high-tech industry. Eur. J. Oper. Res. 2007, 176, 1659–1677. [Google Scholar] [CrossRef]
- Shen, B.; Choi, T.M.; Minner, S. A review on supply chain contracting with information considerations: Information updating and information asymmetry. Int. J. Prod. Res. 2019, 57, 4898–4936. [Google Scholar] [CrossRef]
- Guo, L. The benefits of downstream information acquisition. Mark. Sci. 2009, 28, 457–471. [Google Scholar] [CrossRef]
- Zhao, Y.X.; Wang, S.Y.; Cheng, T.C.E.; Yang, X.Q.; Huang, Z.M. Coordination of supply chains by option contracts: A cooperative game theory approach. Eur. J. Oper. Res. 2010, 207, 668–675. [Google Scholar] [CrossRef]
- Yang, D.Q.; Xiao, T.J.; Choi, T.M.; Cheng, T.C.E. Optimal reservation pricing strategy for a fashion supply chain with forecast update and asymmetric cost information. Int. J. Prod. Res. 2018, 56, 1960–1981. [Google Scholar] [CrossRef]
- Zheng, M.M.; Shunko, M.; Gavirneni, N.; Shu, Y.; Wu, K. Reactive production with capacity restriction in supply chains with forecast updates. IISE Trans. 2019, 51, 142–1436. [Google Scholar] [CrossRef]
- Barnes-Schuster, D.; Bassok, Y.; Anupindi, R. Coordination and flexibility in supply contracts with options. Manuf. Serv. Oper. Manag. 2002, 4, 171–207. [Google Scholar] [CrossRef]
- Li, J.C.; Zhou, Y.W.; Huang, W.Y. Production and procurement strategies for seasonal product supply chain under yield uncertainty with commitment-option contracts. Int. J. Prod. Econ. 2017, 183, 208–222. [Google Scholar] [CrossRef]
- Xu, H. Managing production and procurement through option contracts in supply chains with random yield. Int. J. Prod. Econ. 2010, 126, 306–313. [Google Scholar] [CrossRef]
- Wu, M.C.; Ma, L.J.; Xue, W.L. Order timing for manufacturers with spot purchasing price uncertainty and demand information updates. J. Syst. Sci. Syst. Eng. 2020, 29, 631–654. [Google Scholar] [CrossRef]
- Zhou, Y.W.; Wang, S.D. Supply chain coordination for newsvendor-type products with two ordering opportunities and demand information update. J. Oper. Res. Soc. 2012, 63, 1655–1678. [Google Scholar] [CrossRef]
- Donohue, K.L. Efficient supply contracts for fashion goods with forecast updating and two production modes. Manag. Sci. 2000, 46, 1397–1411. [Google Scholar] [CrossRef]
- Zhou, Y.W.; Wang, S.D. Manufacturer-buyer coordination for newsvendor-type-products with two ordering opportunities and partial backorders. Eur. J. Oper. Res. 2009, 198, 958–974. [Google Scholar] [CrossRef]
- Choi, T.M.; Li, D.; Yan, H.M. Quick response policy with Bayesian information updates. Eur. J. Oper. Res. 2006, 170, 788–808. [Google Scholar] [CrossRef]
- Cachon, G.P.; Swinney, R. Purchasing, pricing and quick response in the presence of strategic consumers. Manag. Sci. 2009, 55, 497–511. [Google Scholar] [CrossRef]
- Cachon, G.P.; Swinney, R. The value of fast fashion: Quick response, enhanced design, and strategic consumer behavior. Manag. Sci. 2011, 57, 778–795. [Google Scholar] [CrossRef]
- Li, T.Y.; Fang, W.G.; Baykal-Gursoy, M. Two-stage inventory management with financing under demand updates. Int. J. Prod. Econ. 2021, 232, 107915. [Google Scholar] [CrossRef]
- Shi, Y.; Guo, X.L.; Yu, Y.G. Dynamic warehouse size, planning with demand forecast and contract flexibility. Int. J. Prod. Res. 2018, 56, 1313–1325. [Google Scholar] [CrossRef]
- Wu, J.H. Quantity flexibility contracts under Bayesian updating. Comput. Oper. Res. 2005, 32, 1267–1288. [Google Scholar] [CrossRef]
- Wang, Q.Z.; Tsao, D.B. Supply contract with bidirectional options: The buyer’s perspective. Int. J. Prod. Econ. 2006, 101, 30–52. [Google Scholar] [CrossRef]
- Zheng, M.M.; Wu, K.; Shu, Y. Newsvendor problems with demand forecast updating and supply constraints. Comput. Oper. Res. 2016, 67, 193–206. [Google Scholar] [CrossRef]
- Choi, T.M.; Li, D.; Yan, H. Optimal two-stage ordering policy with Bayesian information updating. J. Oper. Res. Soc. 2003, 54, 846–859. [Google Scholar] [CrossRef]
- Choi, T.M. Incorporating social media observations and bounded CrossMark rationality into fashion quick response supply chains in the big data era. Transp. Res. Part E-Logist. Transp. Rev. 2018, 114, 386–397. [Google Scholar] [CrossRef]
- Choi, T.M. Internet based elastic logistics platforms for fashion quick response systems in the digital era. Transp. Res. Part E-Logist. Transp. Rev. 2020, 143, 102096. [Google Scholar] [CrossRef]
- Chen, H.Y.; Chen, J.; Chen, Y.H. A coordination mechanism for a supply chain with demand information updates. Int. J. Prod. Econ. 2006, 103, 347–361. [Google Scholar] [CrossRef]
- Chen, H.; Chen, Y.F.; Chiu, C.H.; Choi, T.M.; Sethi, S. Coordination mechanism for the supply chain with lead-time consideration and price-dependent demand. Eur. J. Oper. Res. 2010, 203, 70–80. [Google Scholar] [CrossRef]
- Cheaitou, A.; Cheaytou, R. A two-stage capacity reservation supply contract with risky supplier and forecast updating. Int. J. Prod. Econ. 2019, 209, 42–60. [Google Scholar] [CrossRef]
- Gurnani, H.; Tang, C.S. Note: Optimal ordering decisions with uncertain cost and demand forecast updating. Manag. Sci. 1999, 45, 1456–1462. [Google Scholar] [CrossRef]
- Gao, M.Y.; Xia, L.X.; Xiao, Q.Z.; Goh, M. Incentive strategies for low-carbon supply chains with information updating of customer preferences. J. Clean. Prod. 2023, 410, 137612. [Google Scholar] [CrossRef]
- Peng, J.W.; Zhuo, W.Y.; Wang, J.R. Production and procurement plans via option contract with random yield and demand information updating. Comput. Ind. Eng. 2022, 170, 108338. [Google Scholar] [CrossRef]
- Wang, X.T.; Ma, P.; Zhang, Y.L. Pricing and inventory strategies under quick response with strategic and myopic customer. Int. Trans. Oper. Res. 2020, 27, 1729–1750. [Google Scholar] [CrossRef]
- Xu, J.; Duan, Y.R. Pricing, ordering, and quick response for online sellers in the presence of consumer disappointment aversion. Transp. Res. Part E-Logist. Transp. Rev. 2020, 137, 101925. [Google Scholar] [CrossRef]
- Yan, X.M.; Wang, Y. A newsvendor model with capital constraint and demand forecast updating. Int. J. Prod. Res. 2014, 52, 5012–5040. [Google Scholar] [CrossRef]
- Yang, H.L.; Peng, J.W. Coordination a supply chain with demand information updating. J. Ind. Manag. Optim. 2022, 18, 843–872. [Google Scholar] [CrossRef]
- Zhou, J.H.; Luo, Y. Bayes information updating and multiperiod supply chain screening. Int. J. Prod. Econ. 2023, 256, 108750. [Google Scholar] [CrossRef]
- Anand, K.S.; Girotra, K. The strategic perils of delayed differentiation. Manag. Sci. 2007, 53, 697–712. [Google Scholar] [CrossRef]
- Ahmadini, A.A.H.; Modibbo, U.M.; Shaikh, A.A.; Ali, I. Multi-objective optimization modelling of sustainable green supply chain in inventory and production management. Alex. Eng. J. 2021, 60, 5129–5146. [Google Scholar] [CrossRef]
- Chen, H.W.; Gupta, D.; Gurnani, H. Fast-ship commitment contracts in retail supply chains. IIE Trans. 2013, 45, 811–825. [Google Scholar] [CrossRef]
- Cui, Y.; Hu, D.B.; Chen, X.H.; Xu, X.H.; Xu, Z.H. Capital equilibrium strategy for uncertain multi-model systems. Inf. Sci. 2024, 653, 119607. [Google Scholar] [CrossRef]
- He, Y.J.; Zhang, J. Random yield risk sharing in a two-level supply chain. Int. J. Prod. Econ. 2008, 112, 769–781. [Google Scholar] [CrossRef]
- Karakatsoulis, G.; Skouri, K.; Lagodimos, A.G. EOQ with supply disruptions under different advance information regimes. Appl. Math. Model. 2024, 125, 772–788. [Google Scholar] [CrossRef]
- Luo, J.R.; Chen, X. Risk hedging via option contracts in a random yield supply chain. Ann. Oper. Res. 2017, 257, 697–719. [Google Scholar] [CrossRef]
- Li, Y.M.; Shan, Y.F.; Ling, S. Research on option pricing and coordination mechanism of festival food supply chain. Socio-Econ. Plan. Sci. 2022, 81, 101199. [Google Scholar] [CrossRef]
- Ma, S.; Zhang, L.L.; Cai, X.T. Optimizing joint technology selection, production planning and pricing decisions under emission tax: A Stackelberg game model and nested genetic algorithm. Expert Syst. Appl. 2024, 238, 122085. [Google Scholar] [CrossRef]
- Perera, S.C.; Sethi, S.P. A survey of stochastic inventory models with fixed costs: Optimality of (s, S) and (s, S)-type policies-Continuous-time case. Prod. Oper. Manag. 2023, 32, 131–153. [Google Scholar] [CrossRef]
- Rajak, S.; Parthiban, P.; Dhanalakshmi, R. A DEA model for evaluation of efficiency and effectiveness of sustainable transportation systems: A supply chain perspective. Int. J. Logist. Syst. Manag. 2021, 40, 220–241. [Google Scholar]
- Wang, J.R.; Zhu, K.Y.; Peng, J.W.; Zhuo, W.Y. Strategic demand information sharing under partial cross ownership. Int. J. Prod. Res. 2023, 61, 604–631. [Google Scholar] [CrossRef]
- Wang, C.C.; Yang, C.L.; Zhang, T. Order planning with an outsourcing strategy for a make-to-order/make-to-stock production system using particle swarm optimization with a self-adaptive genetic operator. Comput. Ind. Eng. 2023, 182, 109420. [Google Scholar] [CrossRef]
- Zhao, Y.X.; Choi, T.M.; Cheng, T.C.E.; Wang, S.Y. Supply option contracts with spot market and demand information updates. Eur. J. Oper. Res. 2018, 266, 1062–1071. [Google Scholar] [CrossRef]
- Bickel, P.; Doksum, K. Mathematical Statistics; Holden Day Publisher: San Francisco, CA, USA, 1977. [Google Scholar]







| Literatures | Wholesale Price | Production Mode | Capacity Restriction | Demand Information Updates |
|---|---|---|---|---|
| [31] | Variable | Regular | No | Yes |
| [10] | Variable | Two | No | Yes |
| [28,29] | Constant | Regular | Yes | Yes |
| [14,16] | Constant | Regular | No | Yes |
| [41] | Variable | Two | No | No |
| [36] | Variable | Regular | Yes | Yes |
| [45] | Constant | Two | No | No |
| [52] | Variable | Regular | Yes | Yes |
| [9] | Variable | Emergency | Yes | Yes |
| [3] | Variable | Regular | No | Yes |
| [37] | Constant | Regular | Yes | Yes |
| [33] | Constant | Two | No | Yes |
| [50] | Constant | Two | No | No |
| [47] | Variable | Regular | No | No |
| This paper | Variable | Two | Yes | Yes |
| Notation | Explanation |
|---|---|
| Early demand information | |
| New demand signal | |
| Realized demand signal | |
| Updated demand information | |
| Retail price | |
| Capacity cost | |
| Production cost in the regular mode | |
| Production cost in the emergency mode | |
| Salvage value of unused capacity | |
| Salvage value of unused product | |
| Salvage value of unsold product | |
| Wholesale price in an advance order | |
| Wholesale price in a delay order | |
| Advance order quantity in strategy , where , 2, 3 (decision variable) | |
| Delay order quantity in strategy , where , 2, 3 (decision variable) | |
| Capacity quantity in strategy , where , 2, 3 (decision variable) | |
| Regular production quantity in strategy , where , 2, 3 (decision variable) | |
| Emergency production quantity in strategy , where , 2, 3 (decision variable) | |
| Expected profit of the retailer in strategy , where , 2, 3 | |
| Expected profit of the supplier in strategy , where , 2, 3 | |
| , | Probability density and distribution function of |
| , | Probability density and distribution function of |
| , | Probability density and distribution function of when |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, J.; Zhao, Y.; Dai, L. Equilibrium Strategy of Production and Order in a Two-Echelon Supply Chain with Demand Information Updates and Capacity Restriction. Mathematics 2023, 11, 4767. https://doi.org/10.3390/math11234767
Peng J, Zhao Y, Dai L. Equilibrium Strategy of Production and Order in a Two-Echelon Supply Chain with Demand Information Updates and Capacity Restriction. Mathematics. 2023; 11(23):4767. https://doi.org/10.3390/math11234767
Chicago/Turabian StylePeng, Jiawu, Yue Zhao, and Lili Dai. 2023. "Equilibrium Strategy of Production and Order in a Two-Echelon Supply Chain with Demand Information Updates and Capacity Restriction" Mathematics 11, no. 23: 4767. https://doi.org/10.3390/math11234767
APA StylePeng, J., Zhao, Y., & Dai, L. (2023). Equilibrium Strategy of Production and Order in a Two-Echelon Supply Chain with Demand Information Updates and Capacity Restriction. Mathematics, 11(23), 4767. https://doi.org/10.3390/math11234767






