Application of Fractional Differential Model in Image Enhancement of Strong Reflection Surface
Abstract
1. Introduction
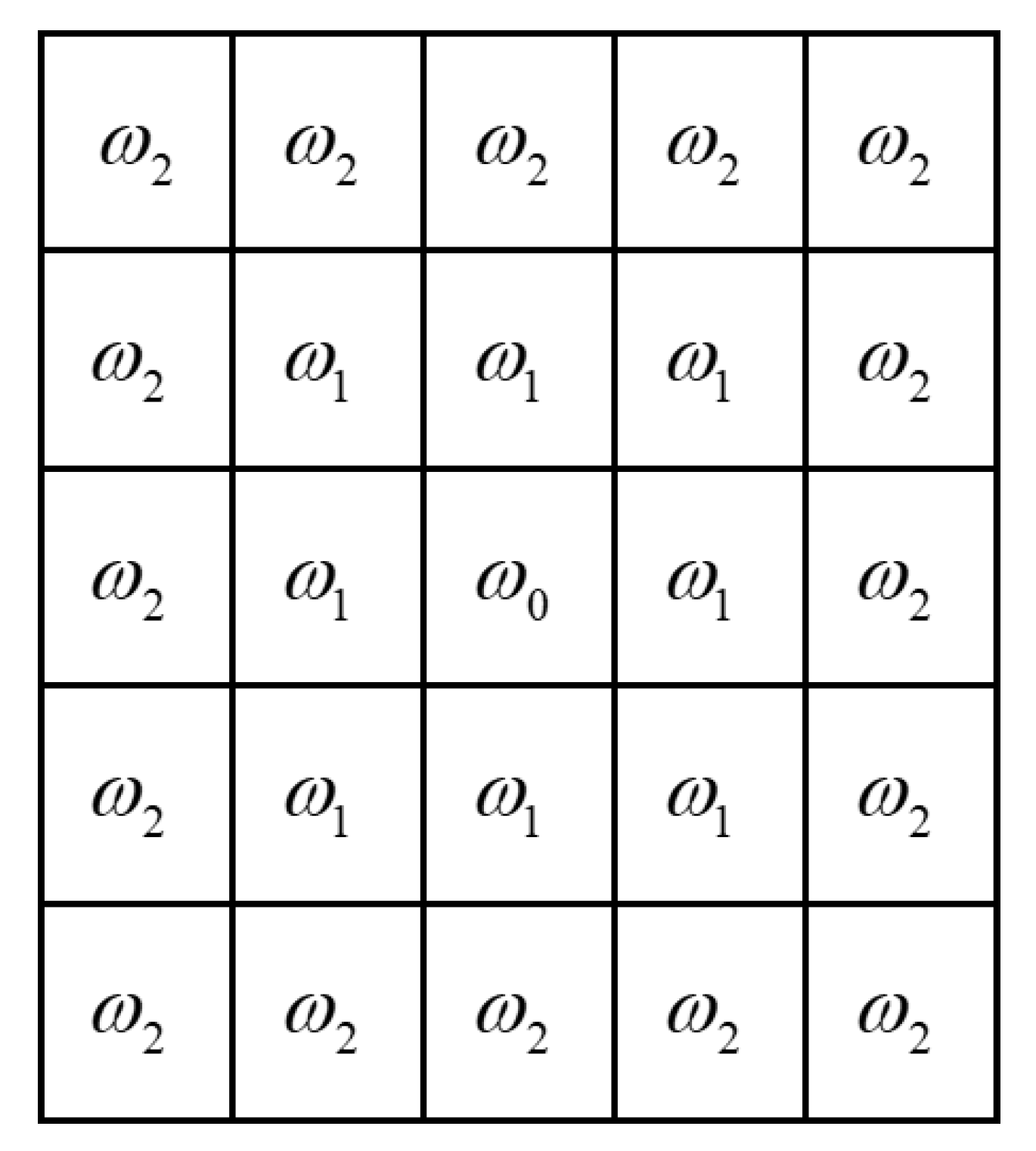
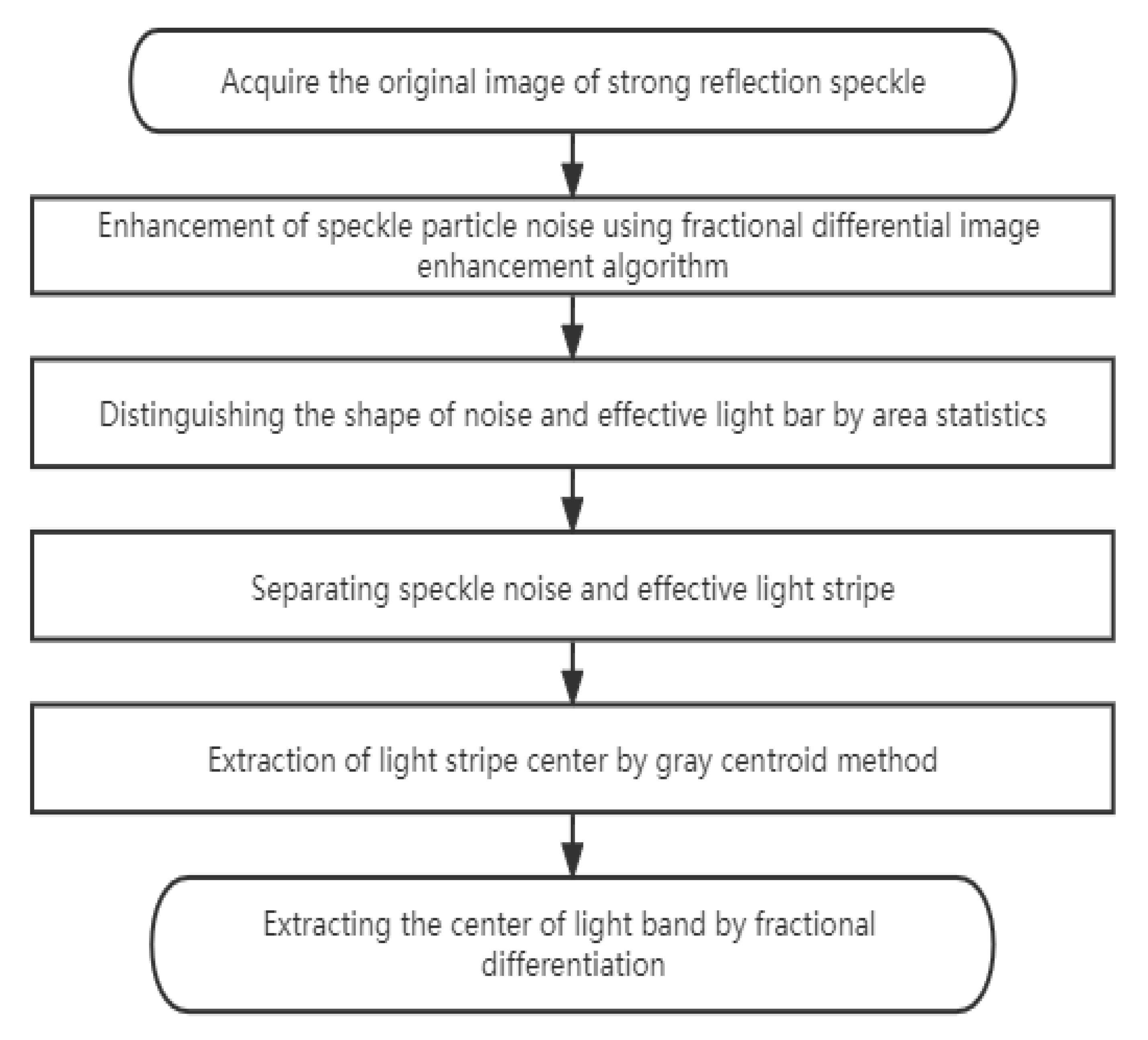
2. Fractional Differential Image Enhancement Model
3. Fractional Differential Image Enhancement Model and Performance Evaluation
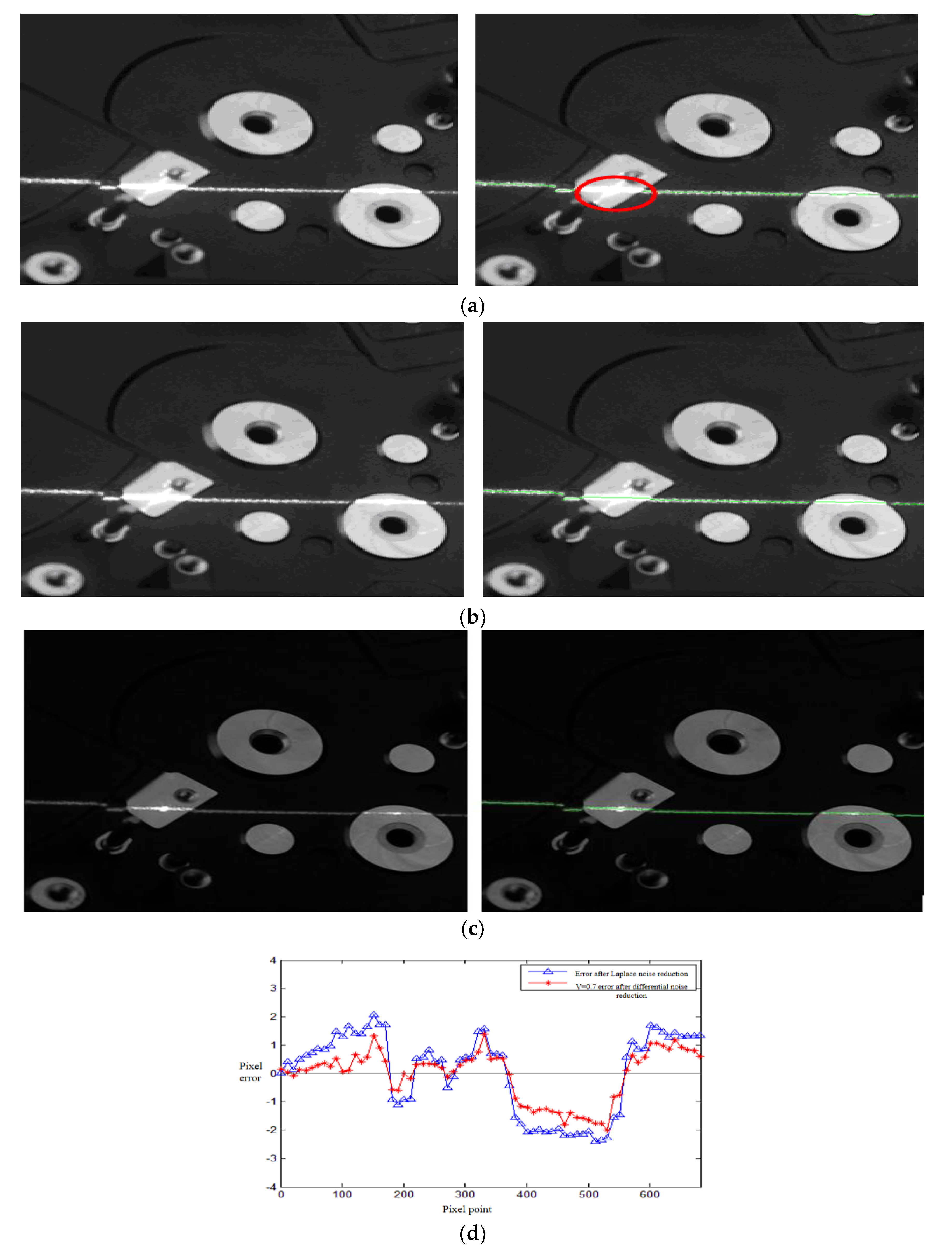
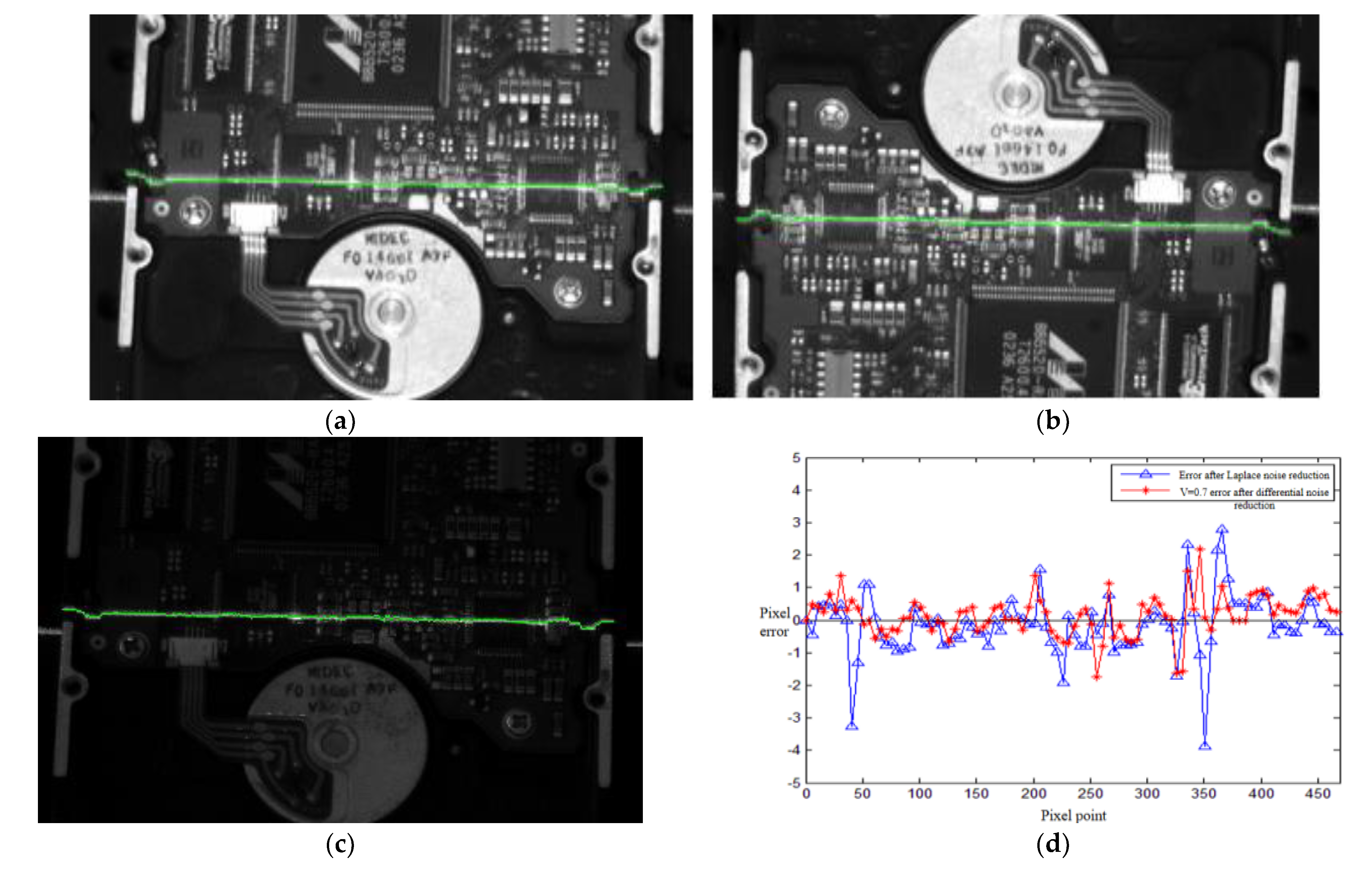
4. Experiment and Results of Structured Light Vision Measurement
5. Comparative Experiment
6. Image Quality Assessment
7. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gao, C.; Zhou, J.; Zhang, W. Fractional Directional Differentiation and Its Application for Multiscale Texture Enhancement. Math. Probl. Eng. 2012, 2012, 325785. [Google Scholar] [CrossRef]
- Chen, Y.; Li, Y.; Guo, H.; Hu, Y.; Luo, L.; Yin, X.; Gu, J.; Toumoulin, C. CT Metal Artifact Reduction Method Based on Improved Image Segmentation and Sinogram In-Painting. Math. Probl. Eng. 2012, 2012, 786281. [Google Scholar] [CrossRef]
- Fellah, M.; Fellah, Z.E.A.; Depollier, C. Transient wave propagation in inhomogeneous porous materials: Application of frac-tional derivatives. Signal Process. 2006, 86, 2658–2667. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Mortensen, J.; Wheatcraft, S.W. Fractional vectorcal culusfor fractional advection-dispersion. Physicaa 2006, 367, 181–190. [Google Scholar] [CrossRef]
- Kamal, S.; Sharma, R.K.; Dinh, T.N.; Ms, H.; Bandyopadhyay, B. Sliding mode control of uncertain fractional-order systems: A reaching phase free approach. Asian J. Control 2021, 23, 199–208. [Google Scholar] [CrossRef]
- Asadi, M.; Farnam, A.; Nazifi, H.; Roozbehani, S.; Crevecoeur, G. Robust Stability Analysis of Unstable Second Order Plus Time-Delay (SOPTD) Plant by Fractional-Order Proportional Integral (FOPI) Controllers. Mathematics 2022, 10, 567. [Google Scholar] [CrossRef]
- Machado, J.T.; Jesus, I.S.; Galhano, A.; Cunha, J.B. Fractional order electromagnetic. Signal Process. 2006, 86, 2637–2644. [Google Scholar] [CrossRef]
- Davis, G.B.; Kohandel, M.; Sivaloganathan, S.; Tenti, G. The constitutive properties of the brain paraenchyma Part2. Fractional derivative approach. Med. Eng. Phys. 2006, 28, 455–459. [Google Scholar] [CrossRef]
- Sierociukd, D.; Dzieliński, A. Fractional Kalman Filteral gorithm forstates, parameters and order offractional system estimation. Int. J. Appl. Math. Comput. Sci. 2006, 16, 129–140. [Google Scholar]
- Castleman, K.R. Digital Image Processing, 2nd ed.; Prentice Hall: London, UK, 2002. [Google Scholar]
- Didas, S.; Burgeth, B.; Imiya, A.; Weickert, J. Regularity and Scalespace Properties of Fractional High Order Linear Filtering. In International Conference on Scale-Space Theories in Computer Vision; Springer: Berlin/Heidelberg, Germany, 2005; Volume 34, pp. 13–25. [Google Scholar]
- Land, E.H.; McCann, J.J. Lightness and Retinex theory. J. Opt. Soc. Amercia 1971, 61, 1–11. [Google Scholar] [CrossRef]
- Jobson, D.J.; Rahman, Z.U.; Woodell, G.A. Properties and performance of a center/surround Retinex. IEEE Trans. Image Process. 1997, 6, 451–462. [Google Scholar] [CrossRef]
- Andrivanov, N.; Belyanchikov, A.; Vasiliev, K.; Dementiev, V. Restoration of Spatially Inhomogeneous Images Based on Doubly Stochastic Filters. In Proceedings of the 2022 VIII International Conference on Information Technology and Nano-technology (ITNT), Samara, Russia, 23–27 May 2022; pp. 1–4. [Google Scholar] [CrossRef]
- Jobson, D.J.; Rahman, Z.U.; Woodell, G.A. A multiscale Retinex for bridging the gap between color images and the human observation of scenes. IEEE Trans. Image Process. 1997, 6, 965–976. [Google Scholar] [CrossRef]
- Zhuang, Z.; Lei, N.; Joseph Raj, A.N.; Qiu, S. Application of fractal theory and fuzzy enhancement in ultrasound image se-mentation. Med. Biol. Eng. Comput. 2019, 57, 623–632. [Google Scholar] [CrossRef] [PubMed]
- Cao, T.; Wang, W. Depth image enchancement and detection on NSCT and fractional differential. Wirel. Pers. Commun. 2018, 130, 1025–1035. [Google Scholar] [CrossRef]
- Ding, Q.; Abba, O.A.; Jahanshahi, H.; Alassafi, M.O.; Huang, W.H. Dynamical Investigation, Electronic circuit realization and emulation of a fractional-order chaotict hree-echelon supply chain system. Mathematics 2022, 10, 625. [Google Scholar] [CrossRef]
- Wei, Z.; Zhang, B.; Jiang, Y. Analysis and modeling of fractional-order buck converter based on Riemann-Liouville derivative. IEEE Access 2019, 7, 162768–162777. [Google Scholar] [CrossRef]
- Pu, Y.F.; Siarry, P.; Zhou, J.L.; Zhang, N. A fractional partial differential equation based multiscale denoising model for tex-tureimage. Math. Methods Appl. Sci. 2014, 37, 1784–1796. [Google Scholar] [CrossRef]
- Chen, D.L.; Chen, Y.Q.; Xue, D.Y. Fractional-order total vari-ation image denoising based on proximity algorithm. Appl. Math. Comput. 2015, 25, 537–545. [Google Scholar]
- Mathieu, B.; Melchior, P.; Oustaloup, A.; Ceyral, C. Fractional differentiation for edge detection. Signal Process. 2003, 83, 2421–2432. [Google Scholar] [CrossRef]
- Liu, N.; Liu, G.; Sun, H. Real-Time detection on SPAD value of potato plant using an In-Field spectral Imaging sensor system. Sensors 2020, 20, 3430. [Google Scholar] [CrossRef]
- Zhang, Y.; Guo, F.; Zhao, G.; Liu, Q.; Zhang, X. A comprehensive review of medical image enhancement technologies. Comput. Aided Draft. Des. Manuf. 2012, 22, 1–11. [Google Scholar] [CrossRef]
- Guo, X.; Li, Y.; Ling, H. LIME: Low-Light Image Enhancement via Illumination Map Estimation. IEEE Trans. Image Process. 2017, 26, 982–993. [Google Scholar] [CrossRef] [PubMed]
- Wu, X.; Sun, Y.; Kimura, A.; Kashino, K. Reflectance-Oriented Probabilistic Equalization for Image Enhancement. In Proceedings of the ICASSP 2021—2021 IEEE International Conference on Acoustics, Speech and Signal Processing (ICASSP), Toronto, ON, Canada, 6–11 June 2021; pp. 1835–1839. [Google Scholar] [CrossRef]
- Deng, G.; Galetto, F.; Al–nasrawi, M.; Waheed, W. A Guided Edge-Aware Smoothing-Sharpening Filter Based on Patch In-terpolation Model and Generalized Gamma Distribution. IEEE Open J. Signal Process. 2021, 2, 119–135. [Google Scholar] [CrossRef]
- Cao, G.; Huang, L.; Tian, H.; Huang, X.; Wang, Y.; Zhi, R. Contrast enhancement of brightness-distorted images by improved adaptive gamma correction. Comput. Electr. Eng. 2018, 66, 569–582. [Google Scholar] [CrossRef]


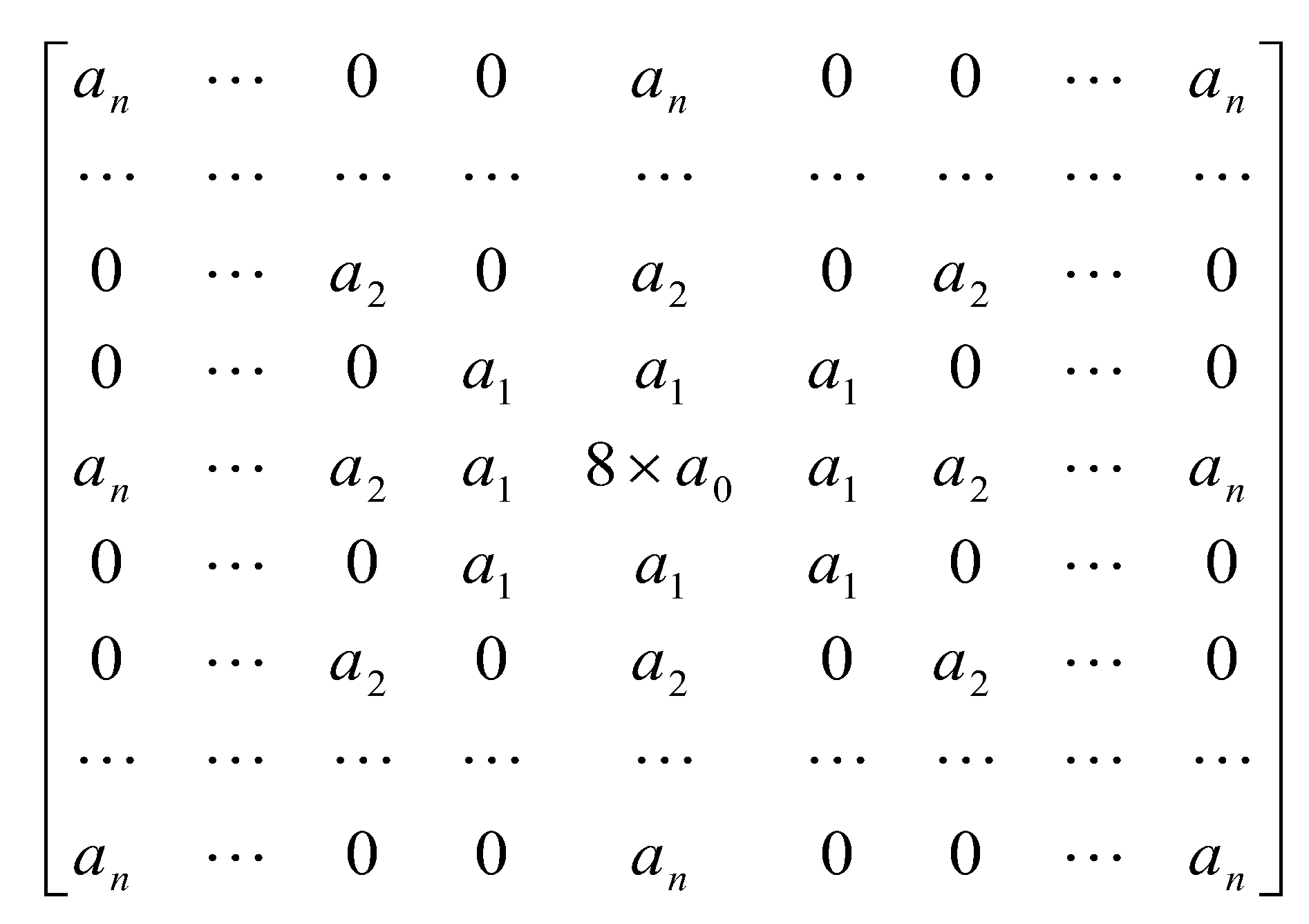
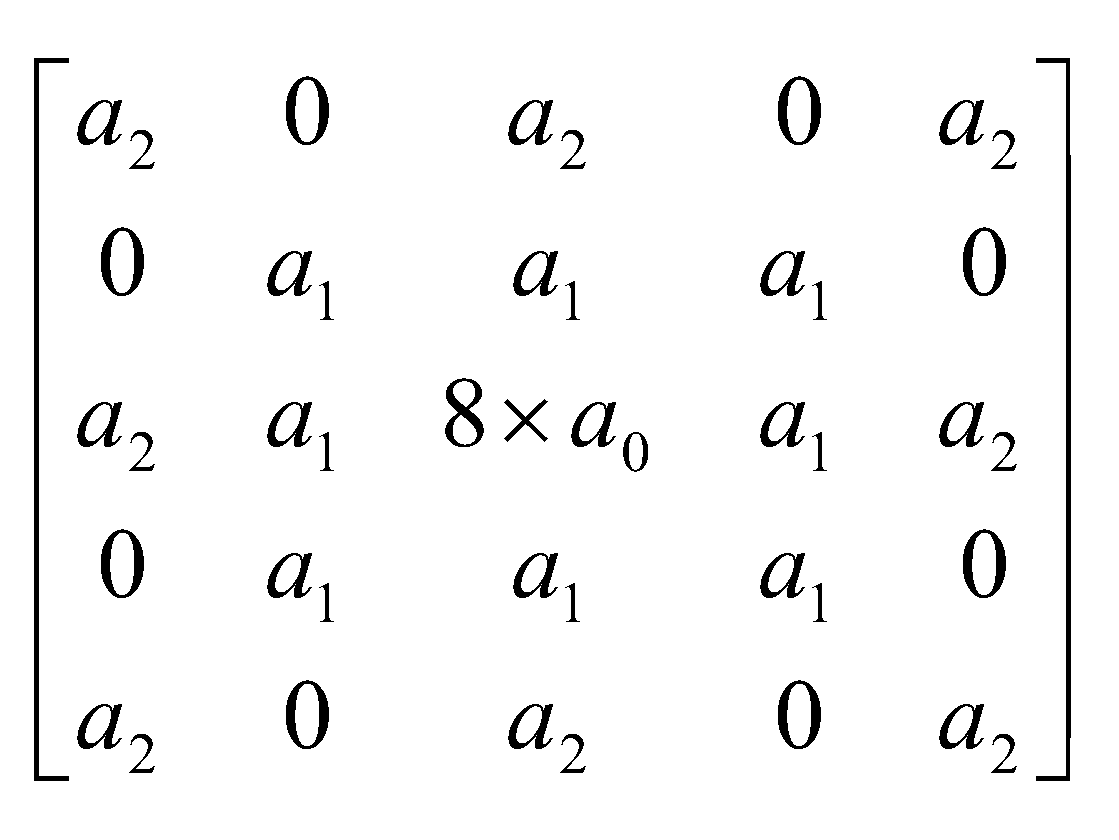

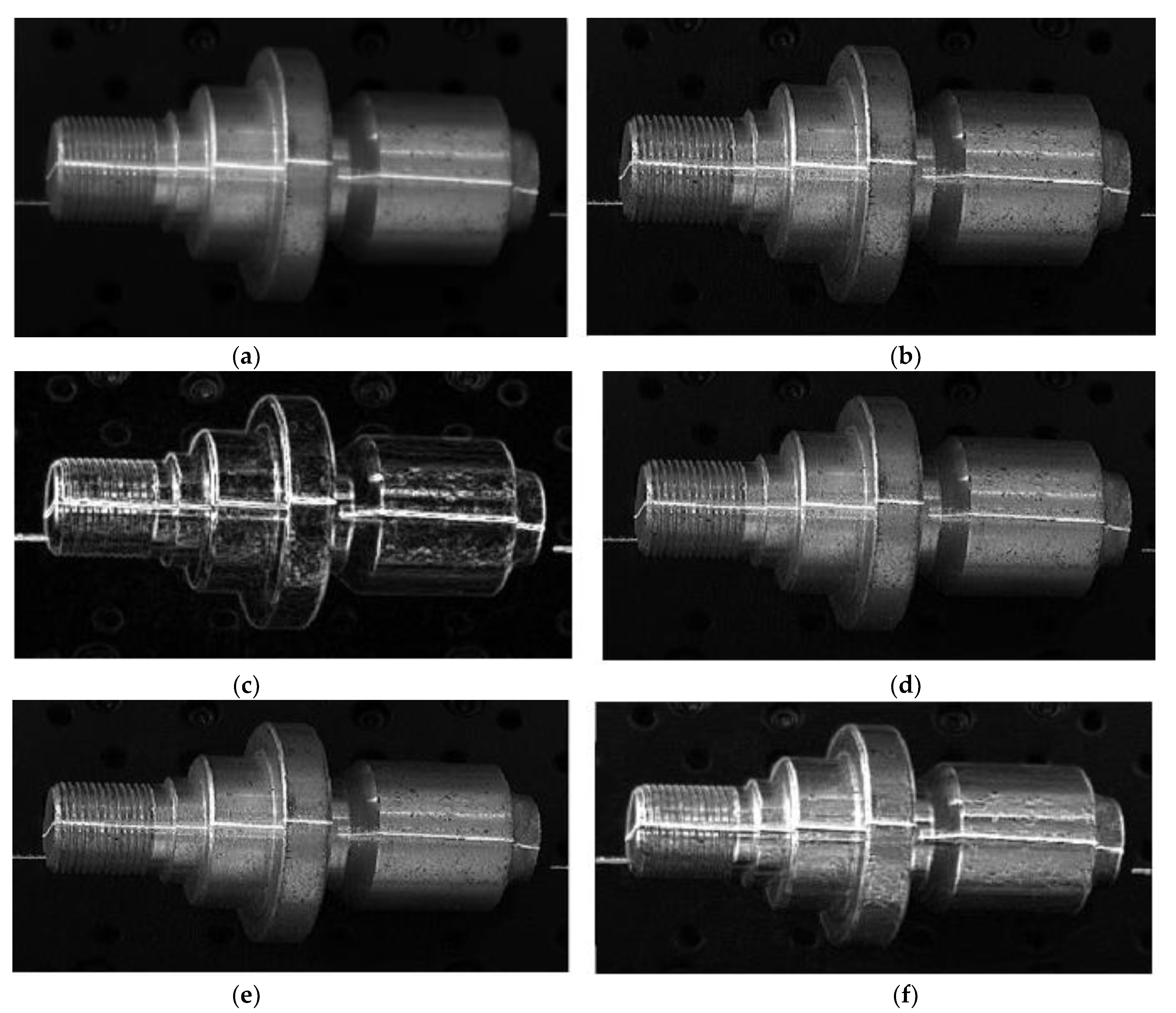
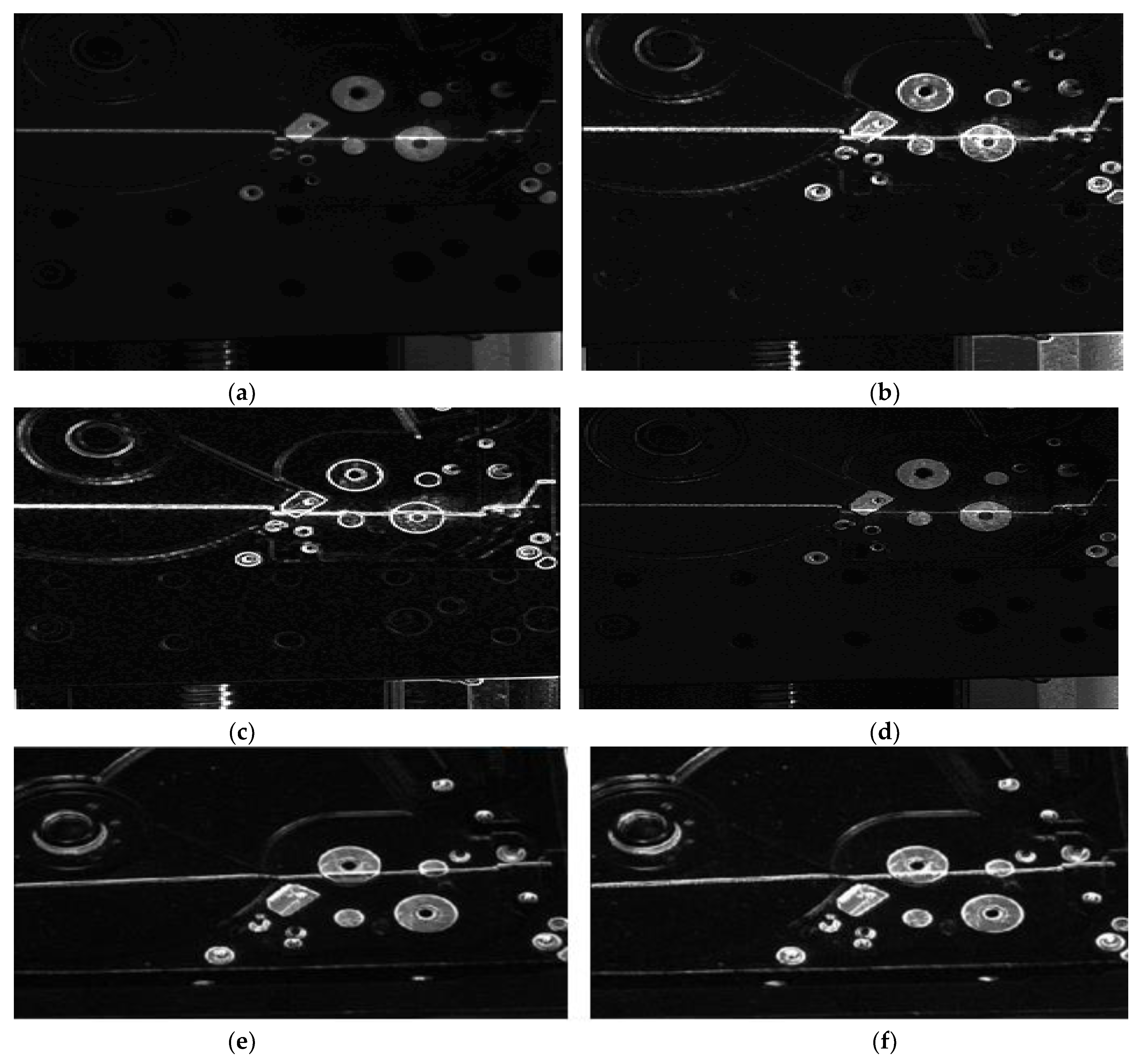


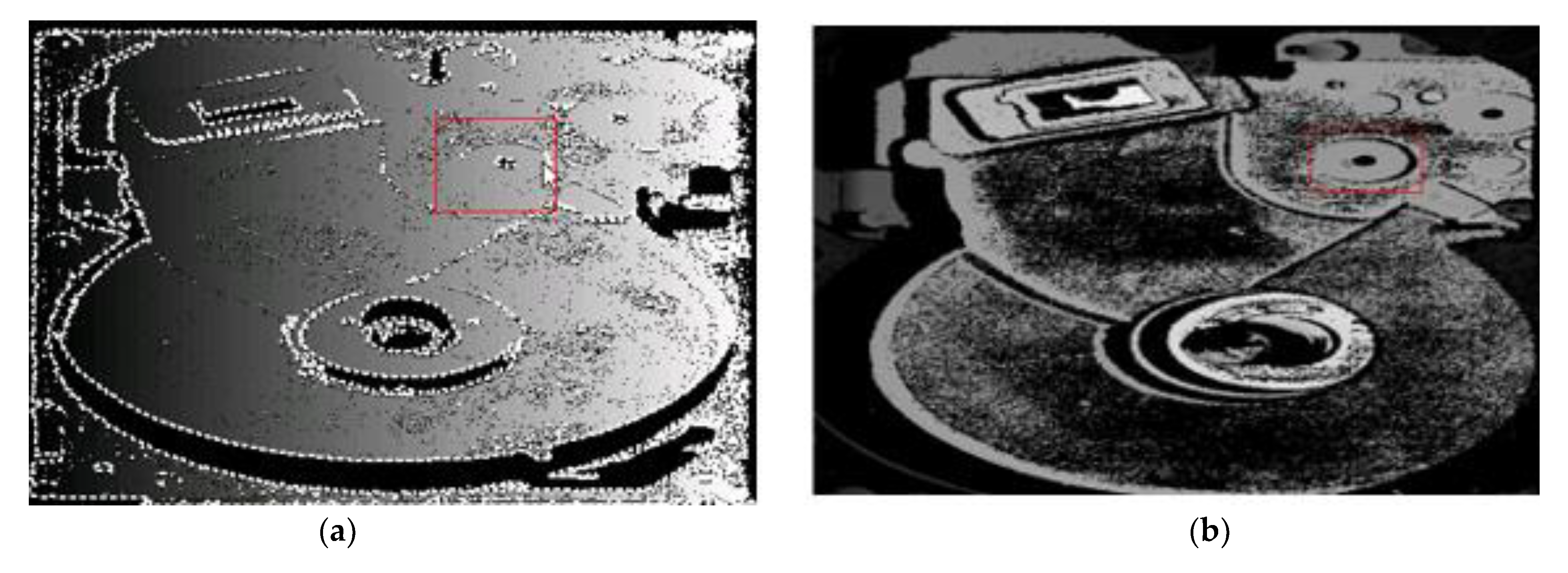
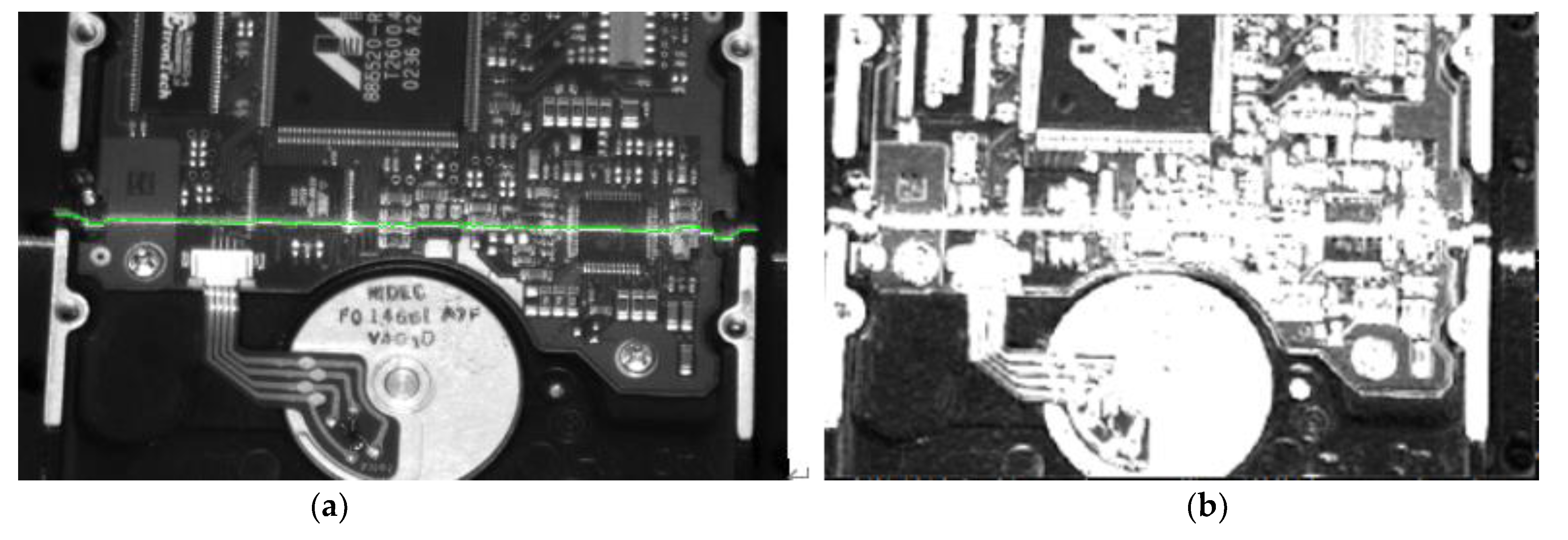
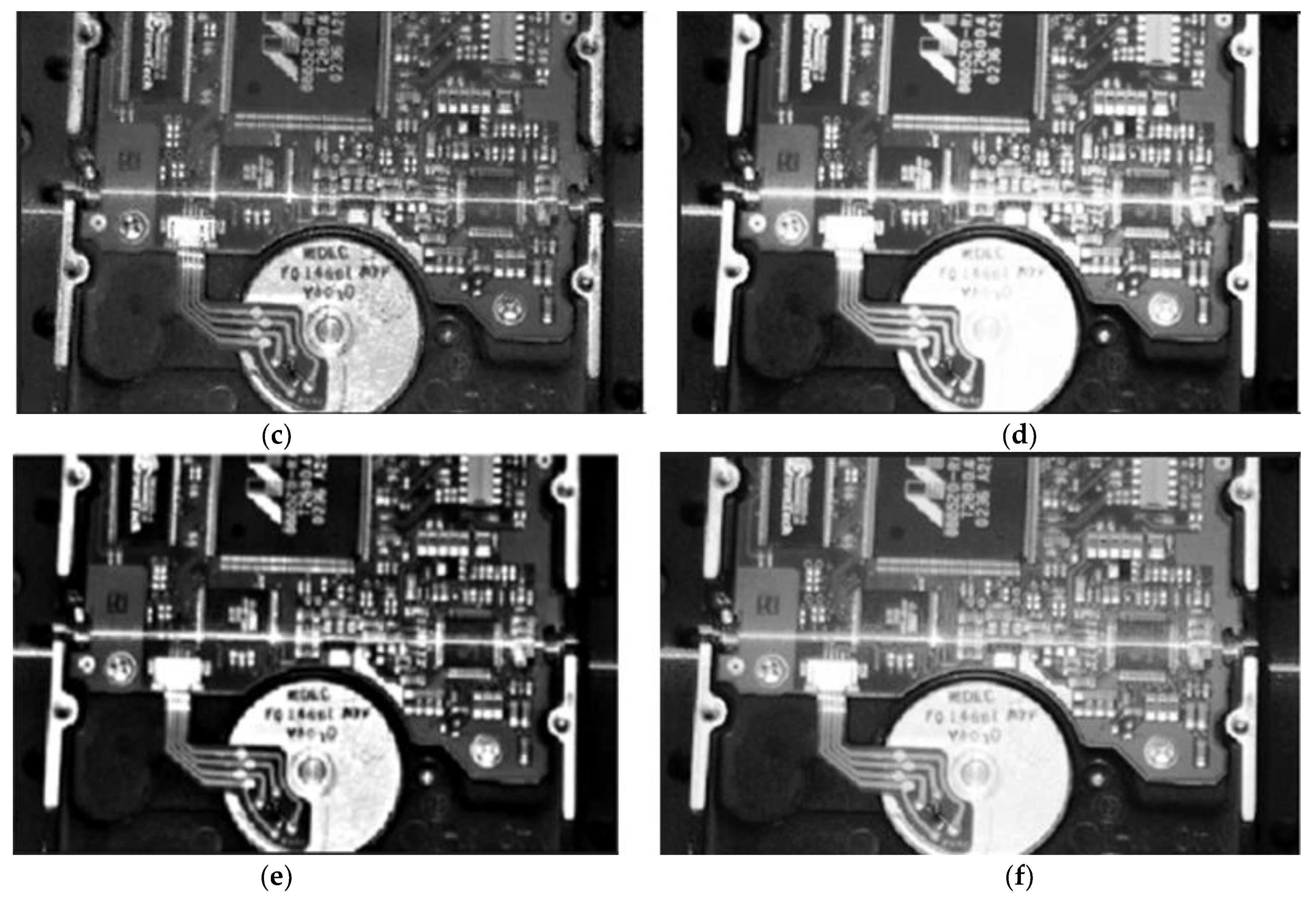
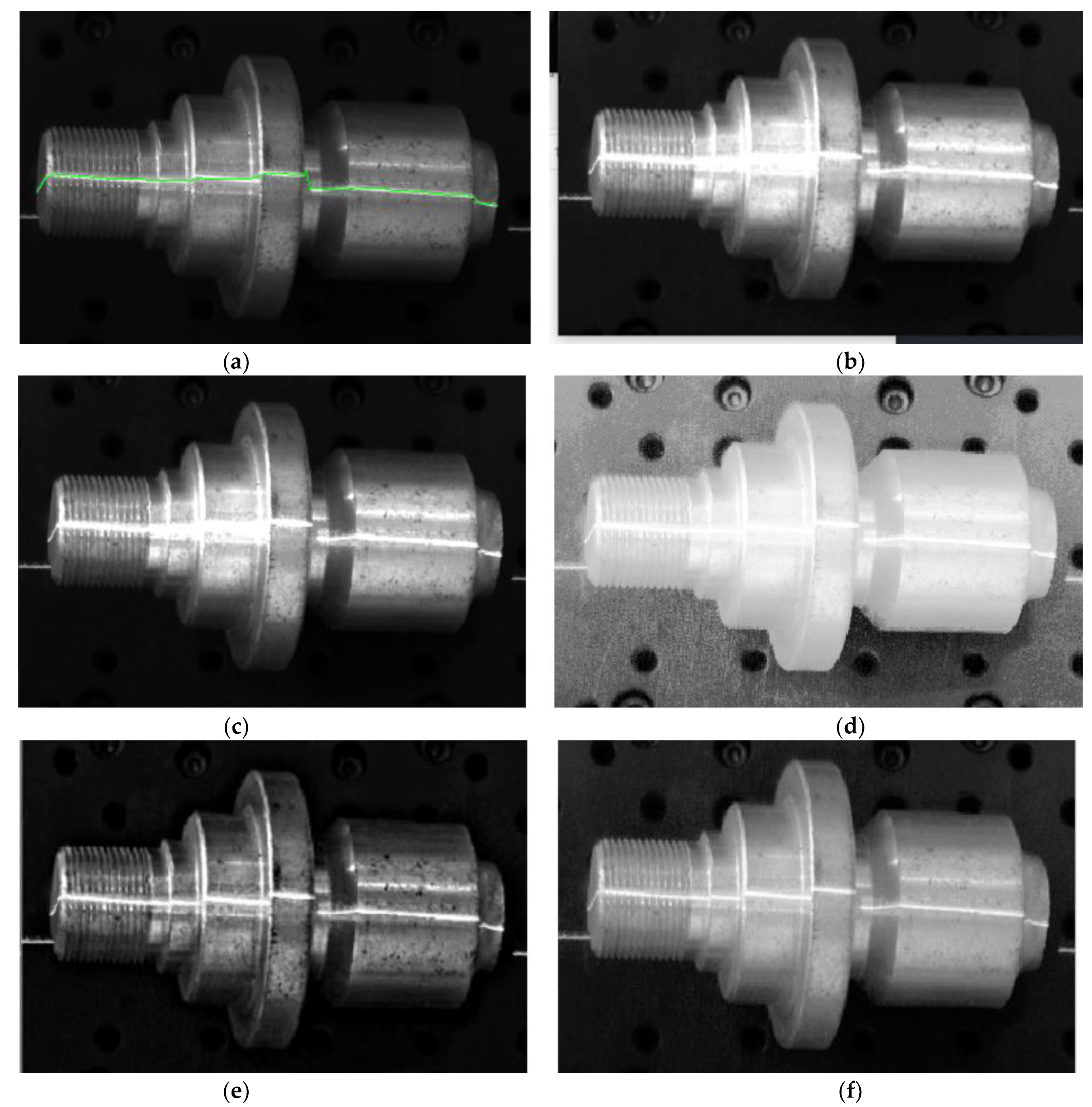
| Information Entropy | Gradient | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Image | Laplacian | Sobel | Differential | Laplacian | Sobel | Differential | ||||
| V = 0.5 | V = 0.7 | V = 0.8 | V = 0.5 | V = 0.7 | V = 0.8 | |||||
| Pcb board | 10.541 | 10.992 | 11.102 | 11.144 | 10.863 | 28.091 | 29.584 | 30.173 | 31.712 | 27.954 |
| Roller | 6.801 | 7.117 | 7.311 | 8.028 | 7.053 | 15.652 | 17.463 | 18.251 | 20.344 | 17.882 |
| Hard disk base plate | 6.154 | 7.206 | 7.494 | 7.779 | 7.196 | 14.396 | 15.461 | 17.512 | 18.197 | 16.996 |
| Image | NIQE Value |
|---|---|
| Steger method | 23.755 |
| Differential enhancement | 17.624 |
| Dark background dark ligh+ differential enhancement | 15.403 |
| Reference [24] algorithm | 25.288 |
| Reference [25] algorithm | 18.624 |
| Reference [26] algorithm | 20.638 |
| Reference [27] algorithm | 17.953 |
| Reference [28] algorithm | 19.978 |
| Image | NIQE Value |
|---|---|
| Steger method | 16.294 |
| Differential enhancement | 15.852 |
| Dark background dark ligh+ differential enhancement | 14.474 |
| Reference [24] algorithm | 19.040 |
| Reference [25] algorithm | 18.745 |
| Reference [26] algorithm | 28.174 |
| Reference [27] algorithm | 16.967 |
| Reference [28] algorithm | 19.638 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ruiyin, T.; Bo, L. Application of Fractional Differential Model in Image Enhancement of Strong Reflection Surface. Mathematics 2023, 11, 444. https://doi.org/10.3390/math11020444
Ruiyin T, Bo L. Application of Fractional Differential Model in Image Enhancement of Strong Reflection Surface. Mathematics. 2023; 11(2):444. https://doi.org/10.3390/math11020444
Chicago/Turabian StyleRuiyin, Tang, and Liu Bo. 2023. "Application of Fractional Differential Model in Image Enhancement of Strong Reflection Surface" Mathematics 11, no. 2: 444. https://doi.org/10.3390/math11020444
APA StyleRuiyin, T., & Bo, L. (2023). Application of Fractional Differential Model in Image Enhancement of Strong Reflection Surface. Mathematics, 11(2), 444. https://doi.org/10.3390/math11020444




