Exploring Limit Cycle Bifurcations in the Presence of a Generalized Heteroclinic Loop
Abstract
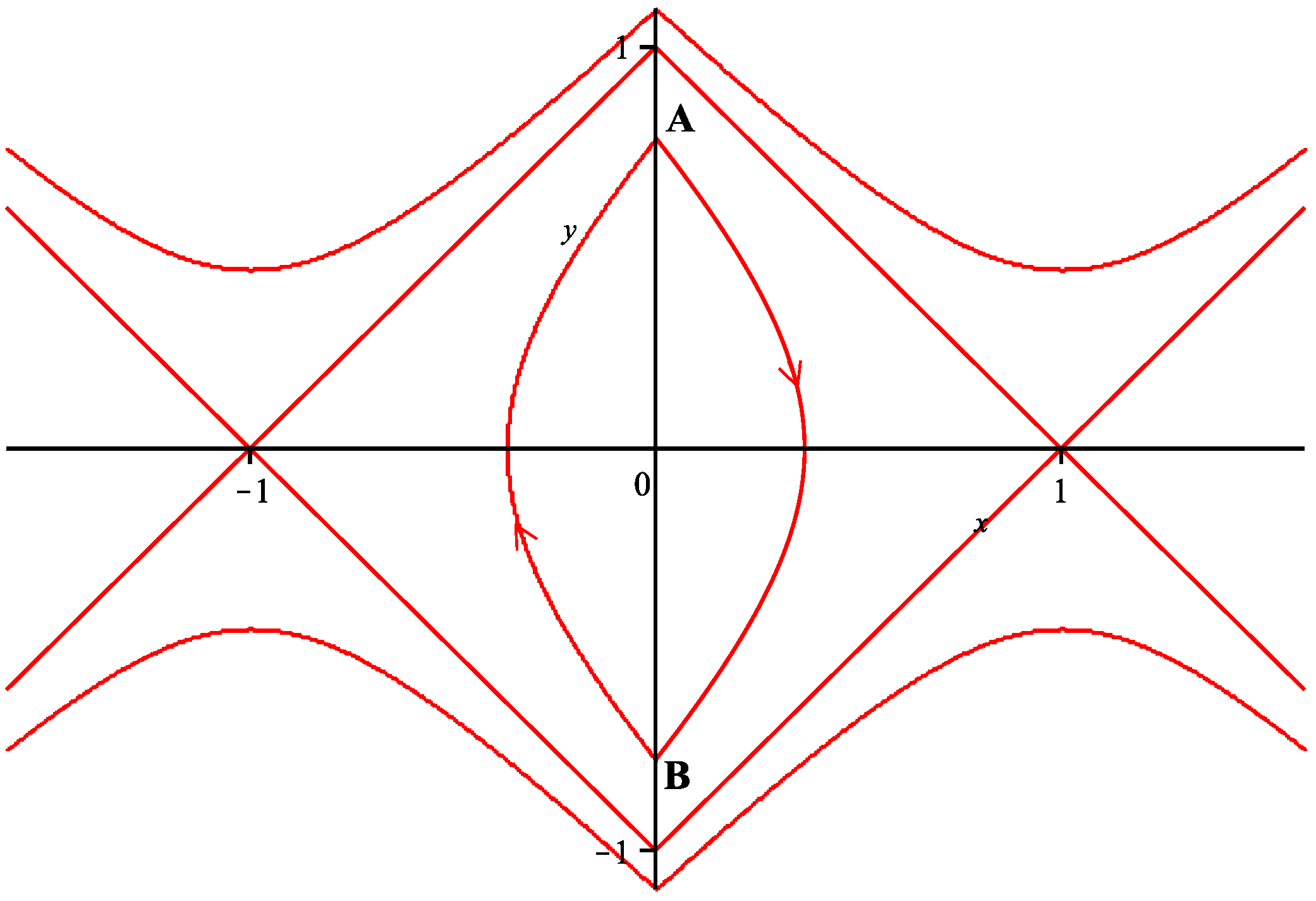
:1. Introductory Notes
2. The Algebraic Structure of the FOMF
3. The Asymptotic Expansion of
Proof of Theorem 1
4. Concluding Summaries
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Di Bernardo Laurea, M.; Champneys, A.R.; Budd, C.J.; Kowalczyk, P. Piecewise-Smooth Dynamical Systems. In Theory and Applications; Springer: London, UK, 2008. [Google Scholar]
- Teixeira, M. Perturbation Theory for Non-Smooth Systems. In Encyclopedia of Complexity and Systems Science; Springer: New York, NY, USA, 2009; Volume 22. [Google Scholar]
- Yang, J.; Zhao, L. Bounding the number of limit cycles of discontinuous differential systems by using Picard-Fuchs equations. J. Differ. Equ. 2018, 264, 5734–5757. [Google Scholar] [CrossRef]
- Yang, J.; Zhao, L. Limit cycle bifurcations for piecewise smooth Hamiltonian systems with a generalized eye-figure loop. Int. J. Bif. Chaos 2016, 26, 1650204. [Google Scholar] [CrossRef]
- Yang, J.; Zhao, L. Bifurcation of limit cycles of a piecewise smooth Hamiltonian system. Qual. Theo. Dyn. Sys. 2022, 21, 142. [Google Scholar] [CrossRef]
- Chen, J.; Han, M. Bifurcation of limit cycles by perturbing a piecewise linear Hamiltonian system. Qual. Theory Dyn. Syst. 2022, 21, 34. [Google Scholar] [CrossRef]
- Llibre, J.; Mereu, A.; Novaes, D. Averaging theory for discontinuous piecewise differential systems. J. Diff. Equ. 2015, 258, 4007–4032. [Google Scholar] [CrossRef]
- Ramirez, O.; Alves, A. Bifurcation of limit cycles by perturbing piecewise non-Hamiltonian systems with nonlinear switching manifold. Nonl. Anal. Real World Appl. 2021, 57, 103188. [Google Scholar] [CrossRef]
- Xiong, Y.; Hu, J. A class of reversible quadratic systems with piecewise polynomial perturbations. Appl. Math. Comput. 2019, 362, 124527. [Google Scholar] [CrossRef]
- Sui, S.; Yang, J.; Zhao, L. On the number of limit cycles for generic Lotka-Volterra system and Bogdanov-Takens system under perturbations of piecewise smooth polynomials. Nonl. Anal. Real World Appl. 2019, 49, 137–158. [Google Scholar] [CrossRef]
- Yang, J.; Zhang, E.; Liu, M. Limit cycle bifurcations of a piecewise smooth Hamiltonian system with a generalized heteroclinic loop through a cusp. Comm. Pure Appl. Anal. 2017, 16, 2321–2336. [Google Scholar] [CrossRef]
- Han, M.; Liu, S. Further studies on limit cycle bifurcations for piecewise smooth near-Hamiltonian systems with multiple parameters. J. Appl. Anal. Comput. 2020, 10, 816–829. [Google Scholar]
- Yang, J.; Zhang, E.; Liu, M. Bifurcation analysis and chaos control in a modified finance system with delayed feedback. Int. J. Bifur. Chaos 2016, 26, 1650105. [Google Scholar] [CrossRef]
- Han, M.; Sun, H.; Balanov, Z. Upper estimates for the number of periodic solutions to multi-dimensional systems. J. Diff. Equ. 2019, 266, 8281–8293. [Google Scholar] [CrossRef]
- Archid, A.; Bentbib, A.H. Global symplectic Lanczos method with application to matrix exponential approximation. J. Math. Model. 2022, 10, 143–160. [Google Scholar]
- Gao, Q.; Zhang, E.; Jin, N. The ultimate ruin probability of a dependent delayed-claim risk model perturbed by diffusion with constant force of interest. Bull. Korean Math. Soc. 2015, 52, 895–906. [Google Scholar] [CrossRef]
- Yang, P.; Yu, J. Melnikov analysis in a cubic system with a multiple line of critical points. Appl. Math. Lett. 2023, 145, 108787. [Google Scholar] [CrossRef]
- Zhang, E.; Li, H.; Huang, J. A simple calculation of the first order Melnikov function for a non-smooth Hamilton system. J. Appl. Math. Comput. 2023, 7, 142–146. [Google Scholar] [CrossRef]
- Liu, X.; Han, M. Bifurcation of limit cycles by perturbing piecewise Hamiltonian systems. Int. J. Bifur. Chaos Appl. Sci. Eng. 2010, 20, 1379–1390. [Google Scholar] [CrossRef]
- Han, M.; Sheng, L. Bifurcation of limit cycles in piecewise smooth systems via Melnikov function. J. Appl. Anal. Comput. 2015, 5, 809–815. [Google Scholar]
- Liang, F.; Han, M. Limit cycles near generalized homoclinic and double homoclinic loops in piecewise smooth systems. Chaos Sol. Frac. 2012, 45, 454–464. [Google Scholar] [CrossRef]
- Liang, F.; Han, M.; Romanovski, V. Bifurcation of limit cycles by perturbing a piecewise linear Hamiltonian system with a homoclinic loop. Nonl. Anal. 2012, 75, 4355–4374. [Google Scholar]
- Liang, F.; Han, M. On the number of limit cycles in small perturbations of a piecewise linear Hamiltonian system with a heteroclinic loop. Chin. Ann. Math. 2016, 37, 267–280. [Google Scholar] [CrossRef]
- Li, S.B.; Ma, W.S.; Zhang, W.; Hao, Y.X. Melnikov method for a three-zonal planar hybrid piecewise-smooth system and application. Int. J. Bif. Chaos 2016, 26, 1650014. [Google Scholar] [CrossRef]
- Xie, S.; Han, M.; Zhao, X. Bifurcation of periodic orbits of a three-dimensional piecewise smooth system. Qual. Theory Dyn. Syst. 2019, 18, 1077–1112. [Google Scholar] [CrossRef]
- Pi, D. Periodic orbits for double regularization of piecewise smooth systems with a switching manifold of codimension two. Disc. Cont. Dyn. Sys. B 2022, 27, 1055–1073. [Google Scholar] [CrossRef]
- Li, J.; Guo, Z.; Zhu, S.; Gao, T. Bifurcation of periodic orbits and its application for high-dimensional piecewise smooth near integrable systems with two switching manifolds. Commun. Nonl. Sci. Numer. Simul. 2023, 116, 106840. [Google Scholar] [CrossRef]
- Liu, S.; Hana, M.; Li, J. Bifurcation methods of periodic orbits for piecewise smooth systems. J. Diff. Equ. 2021, 275, 204–233. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, E.; Shateyi, S. Exploring Limit Cycle Bifurcations in the Presence of a Generalized Heteroclinic Loop. Mathematics 2023, 11, 3944. https://doi.org/10.3390/math11183944
Zhang E, Shateyi S. Exploring Limit Cycle Bifurcations in the Presence of a Generalized Heteroclinic Loop. Mathematics. 2023; 11(18):3944. https://doi.org/10.3390/math11183944
Chicago/Turabian StyleZhang, Erli, and Stanford Shateyi. 2023. "Exploring Limit Cycle Bifurcations in the Presence of a Generalized Heteroclinic Loop" Mathematics 11, no. 18: 3944. https://doi.org/10.3390/math11183944
APA StyleZhang, E., & Shateyi, S. (2023). Exploring Limit Cycle Bifurcations in the Presence of a Generalized Heteroclinic Loop. Mathematics, 11(18), 3944. https://doi.org/10.3390/math11183944





