Fractal Continuum Mapping Applied to Timoshenko Beams

Abstract

In this work, a generalization of the Timoshenko beam theory is introduced, which is based on fractal continuum calculus. The mapping of the bending problem onto a non-differentiable self-similar beam into a corresponding problem for a fractal continuum is derived using local fractional differential operators. Consequently, the functions defined in the fractal continua beam are differentiable in the ordinary calculus sense. Therefore, the non-conventional local derivatives defined in the fractal continua beam can be expressed in terms of the ordinary derivatives, which are solved theoretically and numerically. Lastly, examples of classical beams with different boundary conditions are shown in order to check some details of the physical phenomenon under study.

1. Introduction

Fractional operators have become indispensable tools in the analysis of physical and technological problems. The success of this approach is due to the fact that they present a better description of the phenomenon under study and allow solving functions that are not differentiable in the ordinary calculus sense.

To model the scale invariance of complex systems in the real world as a continuum body, two popular alternatives are often applied: fractional calculus [1,2,3,4,5,6], which uses a standard measure on the length scale where ordinary calculus is no longer valid, and fractal continuum calculus ${\mathcal{F}}^{\alpha }$-CC [7,8,9,10,11], which uses non-standard measures like the Hausdorff measure [12,13,14,15] and incorporates fractional dimensions and fractal properties to characterize a fractal set.

Accordingly, significant efforts have been made by researchers and engineers to describe physical phenomena on fractal domains. Specifically, they have focused on stresses and strains in beams with complex geometries using ordinary [16,17,18], fractional [19,20,21,22,23], and fractal continuum calculus [24,25,26,27].

In this regard, Carpinteri’s beam is a mathematical model consisting of the Cartesian product of the Cantor set and the Sierpinski carpet that was suggested by Carpinteri et al. [28] to idealize inhomogeneous structures [29,30,31]. It is a classical example of the product measure framework suggested in [10,32] as a methodology for analysis in the fractal continuum calculus sense.

The product measure was developed to model anisotropic fractal media [33], which are characterized by different Hausdorff dimensions $0<{\alpha }_k\le 1$ in the fractional directions ${\xi }_k\in E^3$ (in some cases, ${\alpha }_1={\alpha }_2={\alpha }_3$ as in the Cantor dusts [34]), transforming them into a parallelepiped with lengths ${\xi }_1,{\xi }_2,{\xi }_3$. A modified product measure was presented by Balankin [35], which is a generalized version of the previous fractal continuum model. This new approach includes a particular fractional norm, metric, and measure, as well as vector differential calculus and a proper Laplacian. Therefore, it can be effectively applied to path-connected fractals [36]. Consequently, $0<{\alpha }_k\le d_{min}$, where $d_{min}$ is the Hausdorff dimension of the shorter path on the fractal, and ${\alpha }_k=\mathfrak{D}-d_A$, where $\mathfrak{D}$ is the Hausdorff dimension of mass, while $d_A$ denotes the Hausdorff dimension of the cross-section area of the fractal. It should be noted that the fractal distances in the fractal continuum can be written in terms of Cartesian coordinates $x_k\in E^3$. Another modification of the product measure was introduced by Tarasov [37], whereas in [38], this framework was updated.

Many practical applications in the real world have been addressed using the original and modified versions of the product measure, for example, fluid flow [39,40] seismology [41], wave propagation [10,42], viscoelasticity [43], fracture mechanics [24,44], and electric fields [37]. Consequently, in [34], the concept of a fractal continuum beam was introduced to address structural problems in self-similar beams, referred to as Balankin’s beams.

In this work, a new fractal formulation of the Timoshenko beam theory within the ${\mathcal{F}}^{\alpha }$-CC framework is proposed. The efficiency and accuracy of the proposed equations are demonstrated through graphs depicting the structural response of both Carpinteri’s and Balankin’s beams along their lengths.

This paper is organized as follows. We present the mathematical foundations of ${\mathcal{F}}^{\alpha }$-CC in Section 2. In Section 3, the fractal Timoshenko beam theory is explained. In Section 4, the proposed formulation is applied to beams with various geometries and fractal topologies, and the structural details are discussed. The main conclusions are summarized in Section 5.

2. Terminology and Notations

In this section, first, the ${\mathcal{F}}^{\alpha }$-CC framework is summarized, and then Carpinteri’s and Balankin’s beams are reviewed.

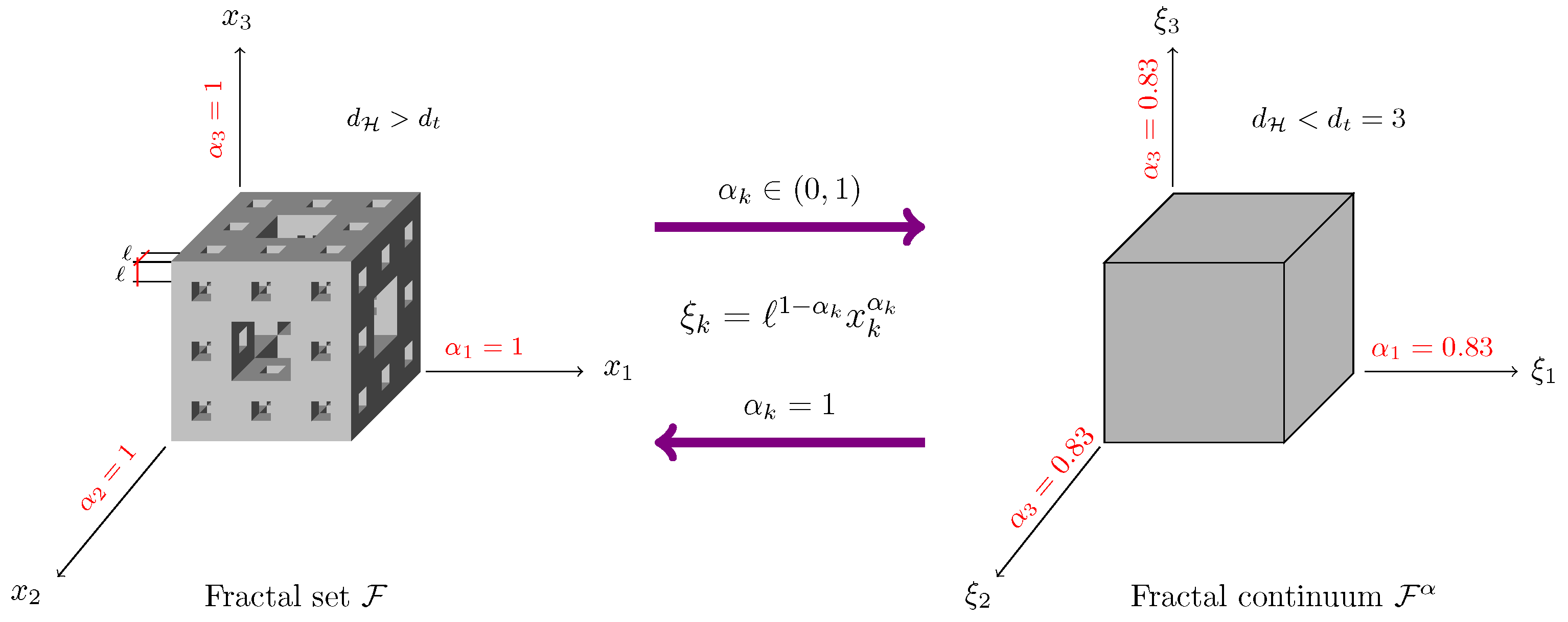
2.1. Fractal Continuum Calculus, ${\mathcal{F}}^{\alpha }$-CC

The present technique involves creating a homogenized continuum model using a fractal metric mapping from a fractal domain $\mathcal{F}$, constructed in Euclidean space with Cartesian coordinates $x_k\in \mathcal{F}\subset E^3$, to a fractal continuum domain ${\mathcal{F}}^{\alpha }$ in fractional coordinates ${\xi }_k\in {\mathcal{F}}^{\alpha }\subset E^3$ (see Figure 1).

There are two types of fractals that can be mapped from $\mathcal{F}$ to ${\mathcal{F}}^{\alpha }$. The first type occurs when $\mathcal{F}$ is totally disconnected, which is constructed as a Cartesian product. This implies that ${\mathcal{F}}^{\alpha }$ becomes a parallelepiped with lengths ${\xi }_1,{\xi }_2,{\xi }_3$, density $\rho =\rho ({\xi }_1,{\xi }_2,{\xi }_3)$, and mass $m=m({\xi }_1,{\xi }_2,{\xi }_3)\sim {\xi }_1^{{\alpha }_1}{\xi }_2^{{\alpha }_2}{\xi }_3^{{\alpha }_3}$ [10,38], whose Hausdorff dimension is given by $\mathfrak{D}={\alpha }_1+{\alpha }_2+{\alpha }_3$, where $0<{\alpha }_k<1$ is the Hausdorff dimension in each fractional spatial coordinate embedded in Euclidean space ${\xi }_k\in E^3$. The difficulties with implementing the Hausdorff dimension for numerical applications motivate the development of empirical estimates such as box-counting [13], packing [12], Hall-Wood [45], and two-scale transform [46], which are frequently used. On the other hand, the second type of fractal occurs when $\mathcal{F}$ is a path-connected fractal, which means that its chemical dimension is equal to its Hausdorff dimension $d_{ch}=\mathfrak{D}<3$, and the number of its independent coordinates is <3. In contrast, the infinitesimal volume element in ${\mathcal{F}}^{\alpha }$-CC is given by $d{V}_{\mathfrak{D}}=d{\xi }_k\left(x_k\right)d{A}^{\left(k\right)}=c_1^{\left(k\right)}\left(x_k\right)c_2^{\left(k\right)}d{x}_{k}d{A}_2^{\left(k\right)}=c_3\left(x_k\right)d{V}_3=c_{3}d{x}_{1}d{x}_{2}d{x}_3$, where $d{A}^{\left(k\right)}d{x}_i·d{x}_j$ and $d{A}^{\left(k\right)}$ are the infinitesimal area elements on the intersection between ${\mathcal{F}}^{\alpha }$ and a two-dimensional plane normal to the k-axis and ${\mathcal{F}}^{\alpha }\in E^3$, respectively. $c_2^{\left(k\right)}\left(x_{j\ne k}\right)$ is the density of admissible states in the plane of this intersection, and $c_3=c_1^{\left(k\right)}{c}_2^{\left(k\right)}$.

Consequently, the measure in ${\mathcal{F}}^{\alpha }$ is defined by the following relations: $\int d{V}_3{c}_3\sim {\ell }^{3-\mathfrak{D}}{L}^{\mathfrak{D}}$, $d{A}_2^{\left(k\right)}{c}_2^{\left(k\right)}\sim L^{d{A}^{\left(k\right)}}$, and $\int d{\xi }_k=\int d{x}_k{c}_1^{\left(k\right)}\sim {\ell }^{1-{\alpha }_k}{L}^{{\alpha }_k}$. Furthermore, ${\mathcal{F}}^{\alpha }$-CC has the following local fractional differential operators [34,44,47]:

-

The fractional norm is given by $\Vert N\Vert ={\left[{\sum }_k^3{\xi }_k^{2\gamma }\right]}^{1/2\gamma }$, where $\gamma =d_{ch}/3\le 1$, and the mapping of the fractional coordinates in the fractal continuum ${\xi }_k\in {\mathcal{F}}^{\alpha }$ to the integer coordinates in the embedding Euclidean space $x_k\in E^3$ is defined as

$$\begin{array}{c}\hfill {\xi }_k={\ell }^{1-{\alpha }_k}{x}_k^{{\alpha }_k}.\end{array}$$

(1)

-

The distance between two points $A,B\in {\mathfrak{F}}^{\gamma }$ is given as $\Delta (A,B)={\left[{\sum }_k^3{\Delta }_k^{2\gamma }\right]}^{1/2\gamma }$, where ${\Delta }_k=\Vert {\xi }_{ak}-{\xi }_{bk}\Vert$.

-

The fractal gradient operator is ${\nabla }^{\mathfrak{D}}={\mathbf{e}}_k{\nabla }_k^{\mathfrak{D}}$, where ${\nabla }_k^{\mathfrak{D}}={\left(c_1^{\left(k\right)}\right)}^{-1}{\nabla }_k$ and $c_1^{\left(k\right)}={\alpha }_k{\ell }^{1-{\alpha }_k}{x}_k^{{\alpha }_k-1}$.

-

The divergence operator is ${\nabla }^{\mathfrak{D}}·\overrightarrow{F}$.

-

The Laplacian is ${\Delta }^{\alpha }={\nabla }^{\alpha }·({\nabla }^{\alpha }·\overrightarrow{F})={\sum }_k^3{\left(c_1^{\left(k\right)}\right)}^{-2}\left[{\nabla }_k^2+\frac{\gamma -{\alpha }_k}{x_k}{\nabla }_k\right]$.

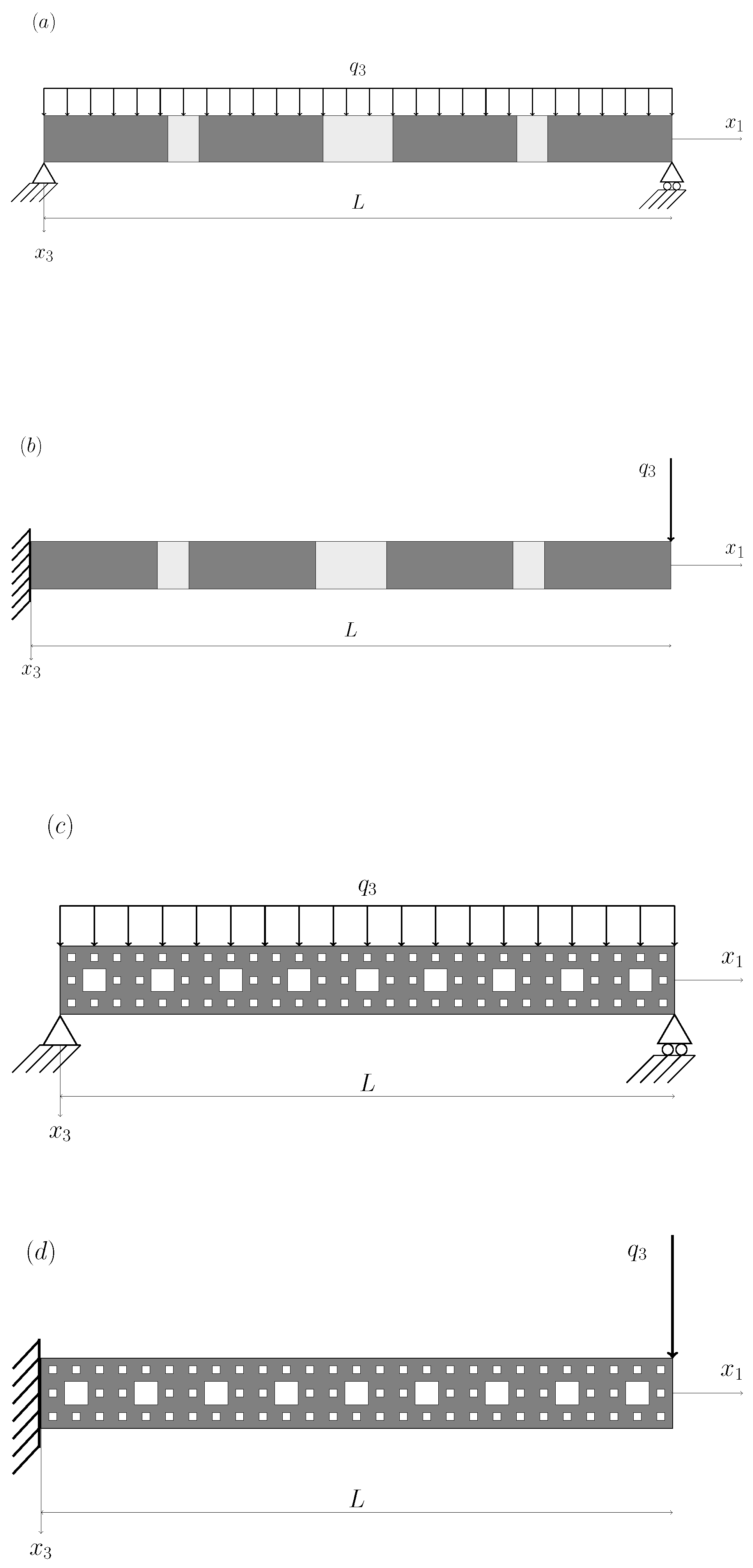
2.2. Fractal Beams

In this manuscript, two kinds of fractal beams are considered, whose geometries and fractal topologies are characterized by their Hausdorff, chemical, and elastic backbone ($d_{eb}$) dimensions. For the beams studied here, $\mathfrak{D}=d_{ch}=d_{eb}$: (i) Carpinteri’s beams, which are fractal sets constructed as Cartesian products [28], and (ii) Balankin’s beams [34], which are self-similar fractals with a path-connected structure, including the Menger sponge type and its modifications.

2.2.1. Carpinteri’s Beam

Carpinteri’s beam, ${\mathfrak{C}}_B^{\psi }={\mathcal{C}}_{x_1}^{\psi }\times {\mathcal{S}}_{x_2{x}_3}^{\psi }$, is the Cartesian product of the Cantor set ${\mathcal{C}}^{\psi }$ and the Sierpinski carpet ${\mathcal{S}}^{\psi }$. The cross-sectional shape of this beam is the Sierpinski carpet, which is relatively smaller than its length defined by the Cantor set. It is embedded in Euclidean space, and it can be denoted using spatial coordinates, $x_k\in E^d$ (see Figure 2a,b). The Cantor middle-$\psi$ set can be built by iteratively removing open middle-$\psi$ segments from the remaining segments of the previous iteration, starting from the unit interval $[0,1]$. The Sierpinski carpet is obtained through an iterative process applied to the unit square ${[0,1]}^2$. This square is divided into $\psi \times \psi$ sub-squares of equal size, and the interior of ${\beta }^2$ sub-squares are deleted through multiple iterations. The Hausdorff dimensions of these fractals are defined by [48]

$$\begin{array}{c}\hfill \alpha =d·\frac{\log \left[N\right(\psi )-\beta ]}{\log N\left(\psi \right)},\end{array}$$

(2)

where d is the dimension of the embedding Euclidean space, $N\left(\psi \right)$ is the number of boxes covering the fractal mass, $\beta$ is the number of deleted boxes of the fractal mass, and $d=1,2$, defines the Hausdorff dimension of the Cantor middle-$\psi$ set and the classical Sierpinski carpet, respectively. Note that $\alpha$ in the above equation can also be computed using the two-scale transform approach [46] with results identical to those of box-counting.

It is easy to see that the Hausdorff dimension of the cross-sectional area of the beam is equal to the Hausdorff dimension of the Sierpinski carpet, denoted as $d_A=\mathfrak{D}\left({\mathcal{S}}^{\psi }\right)={\alpha }_2+{\alpha }_3$. On the other hand, the Hausdorff dimension of Carpinteri’s beam is given by $\mathfrak{D}\left({\mathfrak{C}}_B^{\psi }\right)=\mathfrak{D}\left({\mathcal{C}}^{\psi }\right)+\mathfrak{D}\left({\mathcal{S}}^{\psi }\right)={\alpha }_1+{\alpha }_2+{\alpha }_3$, where $0<{\alpha }_k<1$ is the Hausdorff dimension along the fractional axes ${\xi }_k$ to which Carpinteri’s beam can be mapped.

2.2.2. Balankin’s Beam

Balankin’s beam ${\mathfrak{B}}_B^{\psi }$, is part of the i-th iteration of the Menger sponge and is built using n sub-cubes of smaller iterations along the $x_1$-axis, as depicted in Figure 2c,d. Here, all iterations $i=1,2,3\dots n$ share the same value of $\mathfrak{D}$ but possess different length scales of self-similarity ${\ell }^{1-\alpha }{L}^{\alpha }$. So, the Hausdorff dimension of Balankin’s beam is equal to that of the Menger sponge, and its cross-sectional area forms the Sierpinski carpet within the $x_2{x}_3$-plane. Additionally, the Hausdorff dimensions of their fractional axes are defined as ${\alpha }_k=\mathfrak{D}\left({\mathfrak{B}}_B^{\psi }\right)-\mathfrak{D}\left({\mathcal{S}}^{\psi }\right)$.

Note that the Menger sponge is a 3D version of the Cantor set, which can be constructed by taking the unit cube ${[0,1]}^3\in E^3$ and dividing it into $\psi \times \psi \times \psi$ cubes of equal size. Then, the interiors of the $\beta$ cubes are removed. In each of the remaining cubes, the same operation is repeated ad infinitum. Therefore, the value of $\mathfrak{D}$ for the Menger sponge can be computed using Equation (2) when $d=3$.

3. Fractal Timoshenko Beam Equations

Timoshenko’s bending theory for a fractal continuum map is explained in this section, which is based on the principle of virtual work.

Timoshenko Beam Equations in ${\mathcal{F}}^{\alpha }$-CC

The governing equations in the regime of elastostatics with the assumptions that the material is linearly elastic and a small fractional deformation holds in the concept of fractal elasticity [7] are as follows: the momentum conservation equation ${\nabla }_k^{{\alpha }_k}{\sigma }_{ij}+f_{bi}=0$, where ${\sigma }_{ij}$ is the stress tensor, $f_{bi}$ are the body forces, and ${\nabla }_j^{{\alpha }_k}={\partial }^{{\alpha }_k}/\partial x_j^{{\alpha }_k},j,k=1,2,3$; the strain tensor is defined in fractal continuum dimensions as ${\epsilon }_{ij}^{\alpha }=2^{-1}\left[{\nabla }_j^{\alpha }{v}_i+{\nabla }_i^{\alpha }{v}_j\right]$; the constitutive relationship between stress and strain for a linear elastic isotropic domain is defined by ${\sigma }_{ij}=\mu ({\nabla }_j^{\alpha }{v}_i+{\nabla }_i^{\alpha }{v}_j)+\lambda {\nabla }^{\alpha }{\upsilon }_k{\delta }_{ij}$, where the term in parentheses is the deformation tensor ${\epsilon }_{ij}^{\alpha }$, and $\lambda$ and $\mu$ are the Lamé parameters of the fractal continuum. The components of the fractal displacement vectors ${\upsilon }_k\in {\mathcal{F}}^{\alpha }$, expressed in Cartesian coordinates, are given by

$$\begin{array}{c}\hfill {\upsilon }_k\left({\xi }_k\right)={\alpha }_k{\left(\frac{x_k}{\ell }\right)}^{{\alpha }_k-1}{u}_k\left(x_k\right).\end{array}$$

(3)

On the other hand, the displacement field of the Timoshenko beam in the fractal continuum space framework is given by ${\upsilon }_1\left({\xi }_1,{\xi }_3\right)={\xi }_3\theta \left({\xi }_1\right)$, ${\upsilon }_2\left({\xi }_1,{\xi }_3\right)=0$ and $\left({\xi }_1,{\xi }_3\right)={\upsilon }_3\left({\xi }_1\right)$. Here, ${\upsilon }_3$ is the fractal transversal displacement of the point $({\xi }_1,0)$ on the mid-plane, where ${\xi }_3=0$ and $\theta$ denotes the rotation of the cross-section (see Figure 1). Consequently, the strain–displacement relation is defined by

$$\begin{array}{c}\hfill {\epsilon }_{11}=\frac{\partial {\upsilon }_1}{\partial {\xi }_1}={\xi }_3\frac{d\theta }{d{\xi }_1},\end{array}$$

(4)

and

$$\begin{array}{c}\hfill {\gamma }_{13}=\frac{\partial {\upsilon }_1}{\partial {\xi }_3}+\frac{\partial {\upsilon }_3}{\partial {\xi }_1}=\theta +\frac{d{\upsilon }_3}{d{\xi }_1}\end{array}$$

(5)

The virtual strain energy $\delta U$ includes the virtual energy associated with the shearing strain

$$\begin{array}{c}\hfill \begin{array}{ccc}\delta U\hfill & =\hfill & {\int }_0^L{\int }_A\left({\sigma }_{11}\delta {\epsilon }_{11}+{\sigma }_{13}\delta {\gamma }_{13}\right)dAd{\xi }_1\hfill \\ & =\hfill & {\int }_0^L{\int }_A\left[{\sigma }_{11}{\xi }_3\frac{d\delta \theta }{d{\xi }_1}+{\sigma }_{13}\left(\delta \theta +\frac{d\delta {\upsilon }_3}{d{\xi }_1}\right)\right]dAd{\xi }_1\hfill \\ & =\hfill & {\int }_0^L\left[M_{11}\frac{d\delta \theta }{d{\xi }_1}+Q_{{\xi }_1}\left(\delta \theta +\frac{d\delta {\upsilon }_3}{d{\xi }_1}\right)\right]d{\xi }_1,\hfill \end{array}\end{array}$$

(6)

where ${\sigma }_{11}$ is the normal stress, ${\sigma }_{13}$ is the transverse shear stress, $M_{11}={\int }_A{\xi }_3{\sigma }_{11}$ is the bending moment, and $Q_1={\int }_A{\sigma }_{13}$ is the shear force.

Assuming that the transverse load $q\left({\xi }_1\right)$ acts at the centroidal axis of the Timoshenko beam, the virtual potential energy of the transverse load q is given by

$$\begin{array}{c}\hfill \delta V=-{\int }_0^{L}q\left({\xi }_1\right)\delta {\upsilon }_{3}d{\xi }_1.\end{array}$$

(7)

By substituting the expressions for $\delta U$ and $\delta V$ into $\delta W=\delta U+\delta V$, and performing integration by parts to remove differentiation from $\delta {\epsilon }_3$ and $\delta \theta$, we obtain

$$\begin{array}{c}\hfill \begin{array}{c}0={\int }_0^L\left[M_{11}\frac{d\delta \theta }{d{\xi }_1}+Q_{{\xi }_1}\left(\delta \theta +\frac{d\delta {\upsilon }_3}{d{\xi }_1}\right)-\delta {\upsilon }_{3}q\right]d{\xi }_1\hfill \\ ={\int }_0^L\left[\left(-\frac{d{M}_{11}}{d{\xi }_1}+Q_{{\xi }_1}\right)\delta \theta +\left(-\frac{d{Q}_{{\xi }_1}}{d{\xi }_1}-q\right)\delta {\upsilon }_3\right]d{\xi }_1+{\left[M_{11}\delta \theta +Q_{{\xi }_1}\delta {\upsilon }_3\right]}_0^L.\hfill \end{array}.\end{array}$$

(8)

By setting the coefficients of $\delta {\upsilon }_3$ and $\delta \theta$ in $1<{\xi }_1<L$ to zero, the following equilibrium equations are obtained

$$\begin{array}{c}\hfill -\frac{d{M}_{11}}{d{\xi }_1}+Q_1=0,-\frac{d{Q}_1}{d{\xi }_1}=q.\end{array}$$

(9)

The constitutive relations imply that ${\sigma }_{11}=E_1{\epsilon }_{11}=E_1{\xi }_3(d^2{\upsilon }_3/d{\xi }_1^2)$ and ${\sigma }_{13}=G_{13}{\gamma }_{13}$.

The bending moment and shear force can be expressed in terms of the generalized displacement (${\upsilon }_3,\theta$)

$$\begin{array}{c}\hfill M_{11}={\int }_A{\xi }_3{\sigma }_{11}dA=E_1{I}_{22}\frac{d\theta }{d{\xi }_1},\end{array}$$

(10)

$$\begin{array}{c}\hfill Q_1=K_s{\int }_A{\sigma }_{13}dA=k{G}_{13}A\left(\theta +\frac{d{\upsilon }_3}{d{\xi }_1}\right),\end{array}$$

(11)

where $I_{22}={\int }_A{\xi }_3^{2}dA$ is the second moment of the area about the ${\xi }_2$-axis, and k is the shear correction factor that has been introduced to compensate for the error caused by assuming a constant transverse shear stress distribution throughout the beam’s depth.

By substituting $M_{11}$ and $Q_1$ from Equations (9) and (11) into (8), we obtain the system of the partial differential equations for the Timoshenko beam theory in fractional coordinates ${\xi }_i\in {\mathfrak{F}}^{\gamma }$:

$$\begin{array}{c}\hfill \begin{array}{c}\frac{d{\upsilon }_3}{d{\xi }_1}=\frac{EI}{GAk}\left(\frac{d^2\theta }{d{\xi }_1^2}\right)-\theta \hfill \\ \frac{d^2{\upsilon }_3}{d{\xi }_1^2}=\frac{q}{GAk}-\frac{d\theta }{d{\xi }_1},\hfill \end{array}.\end{array}$$

(12)

for $0<{\xi }_1<L$. It is evident that if the transverse shear strain is zero in Equation (5), Equation (12) simplifies to the Euler–Bernoulli beam equation.

The above equation can be related to the Cartesian coordinates $x_i\in E^n$ using Equations (1) and (3):

$$\begin{array}{c}\hfill \begin{array}{c}{\ell }^{{\alpha }_1-1}\frac{d{u}_3}{d{x}_1^{{\alpha }_1}}=\frac{EI}{GAk}\left({\ell }^{2{\alpha }_1-2}\frac{d^2\theta }{d{x}_1^{2{\alpha }_1}}\right)-\theta \hfill \\ {\ell }^{2{\alpha }_1-2}\frac{d^2{u}_3}{d{x}_1^{2{\alpha }_1}}=\frac{q}{GAk}-\left({\ell }^{{\alpha }_1-1}\frac{d\theta }{d{x}_1^{{\alpha }_1}}\right).\hfill \end{array}\end{array}$$

(13)

Equation (13) is the generalized version of the Timoshenko beam equation, obtained by applying ${\mathcal{F}}^{\alpha }$-CC. The standard version is a particular case of the fractal Timoshenko beam equation, occurring when ${\alpha }_1=1$.

4. Transversal Displacement on Fractal Beams

This section is devoted to applying the proposed fractal model to Carpinteri’s and Balankin’s fractal beams with different fractal geometries determined by $\psi$ in order to show the structural behavior.

Consider two different fractal beams: one of them follows a Carpinteri-like configuration, as presented in Figure 2a,b, whereas the other beam follows a Balankin-type configuration, as depicted in Figure 2c,d. The characteristics of these beams are shown in

Table 1. Hausdorff dimensions of the considered beams.

Beam	Dimension	$\mathit{\psi }=0$	$\mathit{\psi }=1/11$	$\mathit{\psi }=1/7$	$\mathit{\psi }=3/15$
	$\mathfrak{D}$	3	$\frac{\log 1200}{\log 11}$	$\frac{\log 288}{\log 7}$	$\frac{\log 2592}{\log 15}$
Carpinteri’s	${\alpha }_1$	1	$\frac{\log 10}{\log 11}$	$\frac{\log 6}{\log 7}$	$\frac{\log 12}{\log 15}$
	${\alpha }_2={\alpha }_3$	1	$\frac{\log 120}{\log 121}$	$\frac{\log 48}{\log 49}$	$\frac{\log 216}{\log 225}$
	$\mathfrak{D}$	3	$\frac{\log 1300}{\log 11}$	$\frac{\log 324}{\log 7}$	$\frac{\log 2745}{\log 15}$
Balankin’s	$d_{\mathcal{A}}$	2	$\frac{\log 120}{\log 11}$	$\frac{\log 48}{\log 7}$	$\frac{\log 216}{\log 15}$
	${\alpha }_i$	1	$\frac{\log (65/6)}{\log 11}$	$\frac{\log (27/4)}{\log 7}$	$\frac{\log (2745/216)}{\log 15}$

, where $\psi$ represents the proportion of mass that has been removed from Carpinteri’s and Balankin’s beams. Specifically, when $\psi =0$, both fractal beams possess $d_{\mathcal{H}}=3$, indicating that they are Euclidean solid beams.

Also, each beam possesses the following mechanical and geometric properties: $L=2.7$ m, $h=b=0.3$ m, $E=200\times {10}^9$ N/m², $q_3=100$ N/m (uniformly distributed load), $q_3=100$ N (concentrated load), and $I=675\times {10}^{-6}$ m⁴. The Hausdorff dimension of the cross-sectional area of both Carpinteri’s and Balankin’s beams is the same (Hausdorff dimension of the Sierpinski carpet).

Equation (12) is applied for two different boundary conditions:

I

Simply supported fractal beam

$$\begin{array}{c}\hfill {\upsilon }_3\left(0\right)=M\left(0\right)={\upsilon }_3\left(L\right)=M\left(L\right)=0,\end{array}$$

(14)

which leads to

$$\begin{array}{c}\hfill {\upsilon }_3\left({\xi }_1\right)=\frac{q_3{\xi }_1^4}{24EI}-\frac{q_{3}L{\xi }_1^3}{12EI}+\frac{q_3{L}^3{\xi }_1}{24EI}-\frac{q_3{\xi }_1^2}{2kGA}+\frac{q_{3}L{\xi }_1}{2kGA}.\end{array}$$

(15)

II

Cantilever fractal beam

$$\begin{array}{c}\hfill {\upsilon }_3\left(0\right)=\theta \left(0\right)=M\left(L\right)=Q\left(L\right)=0,\end{array}$$

(16)

therefore

$$\begin{array}{c}\hfill {\upsilon }_3\left({\xi }_1\right)=\frac{q_3{L}^3}{6EI}\left[3{\left(\frac{{\xi }_1}{L}\right)}^2-{\left(\frac{{\xi }_1}{L}\right)}^3\right]+\frac{q_{3}L}{kGA}\left(\frac{{\xi }_1}{L}\right).\end{array}$$

(17)

The above transversal displacements can be expressed in Cartesian coordinates as follows:

$$\begin{array}{c}\hfill {\upsilon }_3\left({\xi }_1\right)=\frac{q_3{\ell }^{4-4{\alpha }_1}}{24EI}\left(x_1^{4{\alpha }_1}-2L^{{\alpha }_1}{x}_1^{3{\alpha }_1}+L^{3{\alpha }_1}{x}_1^{{\alpha }_1}\right)-\frac{q_3{\ell }^{2-2{\alpha }_1}}{2kGA}\left(x_1^{2{\alpha }_1}-L^{{\alpha }_1}{x}_1^{{\alpha }_1}\right).\end{array}$$

(18)

for a simply supported beam. Meanwhile, for a Cantilever beam, it is

$$\begin{array}{c}\hfill {\upsilon }_3\left({\xi }_1\right)=\frac{q_3{\ell }^{3-3{\alpha }_1}{L}^{3{\alpha }_1}}{6EI}\left[3{\left(\frac{x_1^{{\alpha }_1}}{L^{{\alpha }_1}}\right)}^2-{\left(\frac{x_1^{{\alpha }_1}}{L^{{\alpha }_1}}\right)}^3\right]+\frac{q_3{\ell }^{1-{\alpha }_1}{L}^{{\alpha }_1}}{kGA}\left(\frac{x_1^{{\alpha }_1}}{L^{{\alpha }_1}}\right).\end{array}$$

(19)

By applying Mathematica software and using the fractal parameters given in Table 1 for both boundary conditions in Carpinteri’s beams, the total response is obtained and is plotted in Figure 3a,b, whereas in Figure 4c,d, the structural behavior of Balankin’s beams is presented.

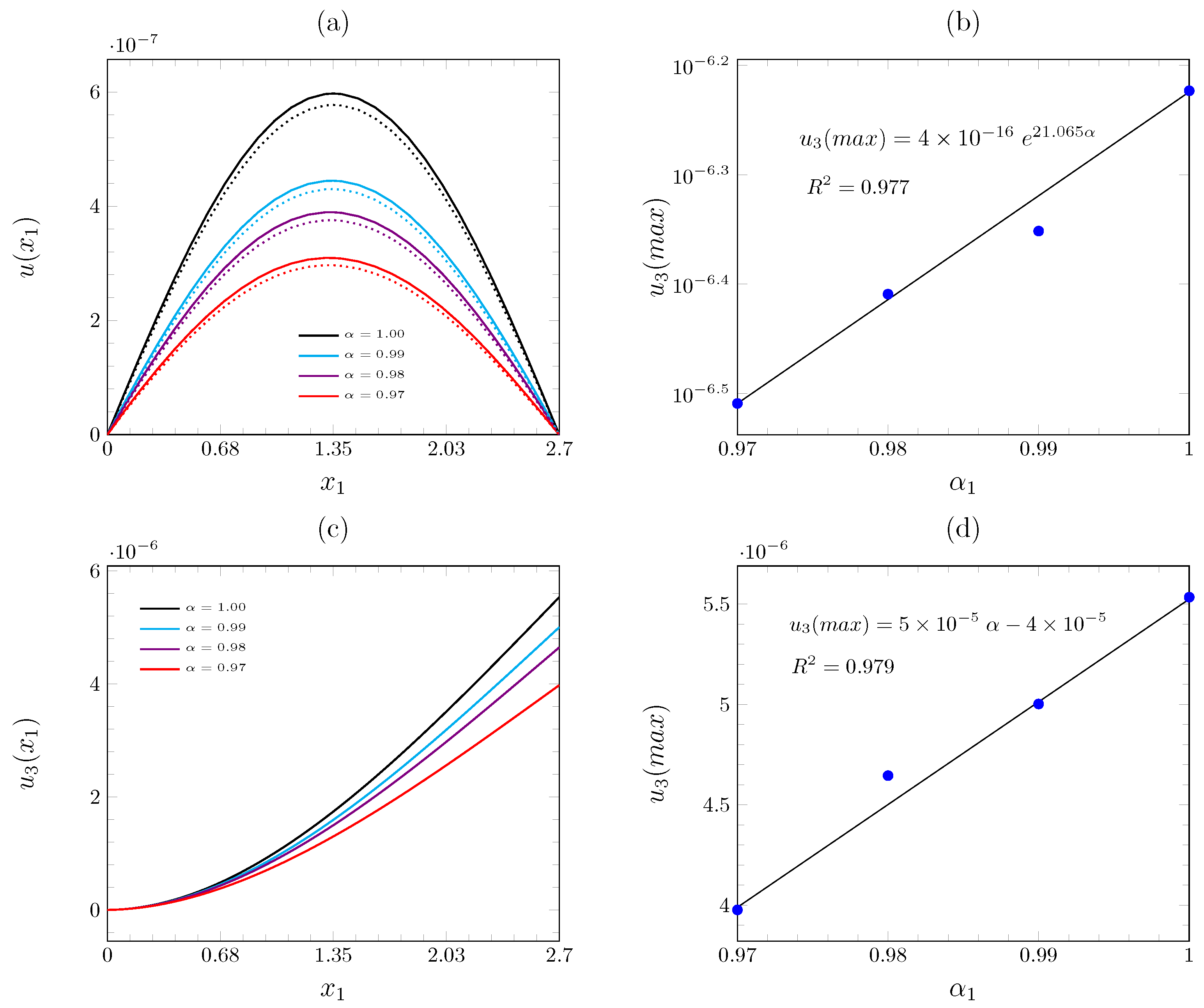
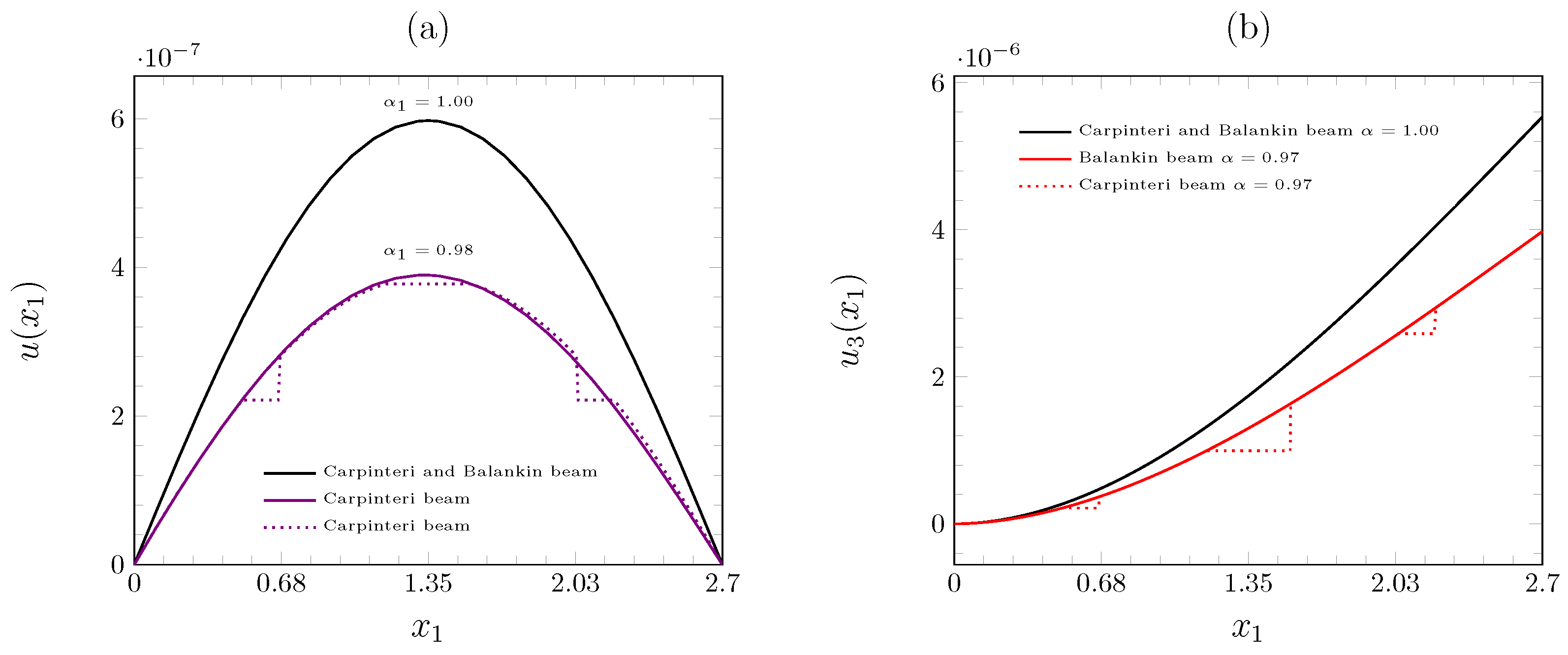
Discussion of Results

The mechanical behavior of Carpinteri’s and Balankin’s beams has been analyzed from the perspective of the Timoshenko beam theory and for different Hausdorff dimensions: ${\alpha }_1=0.97,0.98,0.99,$ and $1.00$. The geometries of the beams are given in Table 1.

The deflection curve has been calculated for two cases: the simply supported beam subjected to a uniformly distributed load and the cantilever beam subjected to a concentrated load applied at its free end, as illustrated in Figure 2. In the case of Carpinteri’s beam, the deflection curves for the second iteration $\psi =1/7$ are shown in Figure 3a, where it can be observed that under the same loading conditions as in a simply supported beam, the deflection increases with the value of ${\alpha }_1$. For the value of ${\alpha }_1=1$, the maximum deflection is obtained, whereas for ${\alpha }_1=0.97$, the minimum deflection curve is obtained. We can say that the stiffness of the bending increases as the value of ${\alpha }_1$ decreases.

Similarly, in the case of the cantilever beam in Figure 3b, it can be seen that the flexural stiffness of the beam increases as the value of ${\alpha }_1$ increases for the same load conditions. In addition, a stepped deformation behavior in the form of a devil’s staircase can be observed in this type of beam, assuming that the beam has no deflection in each step.

Figure 4a shows the deflection for the simply supported condition of Balankin’s beam subjected to a uniformly distributed load for various Hausdorff dimensions: ${\alpha }_1=0.97,0.98,0.99$, and $1.00$. It can be observed that the maximum deflection is obtained with values of ${\alpha }_1=1.00$, corresponding to a classical Timoshenko beam. In the same figure, the deflection curve of the Euler–Bernoulli beam is shown with dotted lines. It can be seen that the Euler–Bernoulli beam has a shorter deflection compared to the Timoshenko beam. Figure 4b shows the trend of the maximum deflection value in relation to the Hausdorff dimension ${\alpha }_1$. The maximum deflection exhibits an exponential relationship with ${\alpha }_1$.

Figure 4c shows Balankin’s cantilever beam. As in the previous case, the beam exhibits a larger deflection for higher values of ${\alpha }_1$, with the curve showing the largest deflection when ${\alpha }_1=1$. For the cantilever beam, it has been found that there is a linear relationship between the maximum deflection and the Hausdorff dimension ${\alpha }_1$.

Finally, comparisons between the standard and fractal cases are shown in Figure 5, for ${\alpha }_1=1$ and ${\alpha }_1<1$.

5. Conclusions

In this work, a specific set of differential operators in the fractal continuum framework was used to describe the structural response of complex scale-invariant beams. These beams are impossible to model using conventional vector differential calculus due to the irregular functions defined on fractal domains, which are non-differentiable in the conventional sense. So, a generalization of the Timoshenko beam theory was conducted, transitioning from ordinary to fractal calculus.

The proposed formulation was applied to fractal beams with two different boundary conditions to validate it and describe the effects of fractal parameters in beams with complex domains. From this study, the following conclusions and outlooks can be drawn:

i

The stiffness of the self-similar beam is governed by the parameters ${\alpha }_k,\ell$, $d_{\mathcal{A}}$, ${\gamma }_{13}$, and the iteration number of the pre-fractal.

ii

The flexural rigidity $EI$ is affected by the geometry and fractal topology of the beam, such that when $d_{\mathcal{A}}$ increases, ${\alpha }_k$ decreases. However, Carpinteri’s beam is stiffer than Balankin’s beam, as shown in Figure 4a,c.

iii

The fractal mass of each iteration and the boundary conditions of beams, as well as the length scales of similarity, are linked with the scale effect of fractal beams.

iv

Using the generalized Timoshenko beam formulation is possible to obtain the exact solutions of a complex scale-invariant beam through the parameter $\alpha =\mathfrak{D}-d_{\mathcal{A}}$, which includes its topology and fractal geometry.

v

The introduced solution coincides with the classical Timoshenko beam theory when ${\alpha }_k=1$.

vi

A devil’s staircase-like structural response is presented in Carpinteri’s beams.

vii

In future work, we will extend this formulation to model self-similar column buckling. It is also possible to understand the mechanical behavior of other fractal beams, for example, Koch’s curve-like beam suggested in [49,50,51].