A New Result Concerning Nonlocal Controllability of Hilfer Fractional Stochastic Differential Equations via almost Sectorial Operators
Abstract
1. Introduction
2. Preliminaries
- 1.
- Suppose , then is a Banach space with .
- 2.
- Assume that , then , A is compact and the embedding is also compact.
- 3.
- For all , exists such that
- (i)
- ;
- (ii)
- , for all and let be a constant,
- (a)
- , for all
- (b)
- , where the constant ;
- (c)
- The range of , is contained in . Particularly, for all withand hence there exists a constant such that
- (d)
- Suppose that , then
- (e)
- , and .
- 1.
- are strongly continuous, for .
- 2.
- If are bounded linear operators on Z, for any fixed , then we obtainwhere, .
- (i)
- is precompact if and only if ;
- (ii)
- where and denotes the closure and convex hull of , respectively;
- (iii)
- If then ;
- (iv)
- such that ;
- (v)
- ;
- (vi)
- , when Z be a real Banach space;
- (vii)
- If the operator is Lipschitz-continuous with constant , then we know for all bounded subset , where is a Banach space and t represents the Hausdorff in .
- 1.
- is continuous on ,
- 2.
- .
3. Controllability
- (H1)
- Let A be the almost sectorial operator of the analytic semigroup in Z such that where is a constant.
- (H2)
- The function satisfies:
- (a)
- The Caratheodory condition: is strongly measurable for all , and is continuous for a.e. ;
- (b)
- There is a constant and and non-decreasing continuous function such that , where g satisfies ;
- (c)
- There is a constant and such that, for all bounded subsets for a.e. .
- (H3)
- (a)
- The linear operator is bounded, denoted by , and it has an inverse operator , which take the values in , and there are two positive values and such that
- (b)
- There is a constant and such that, for all bounded sets .
- (H4)
- The function is a continuous, compact operator, and there exists such that .
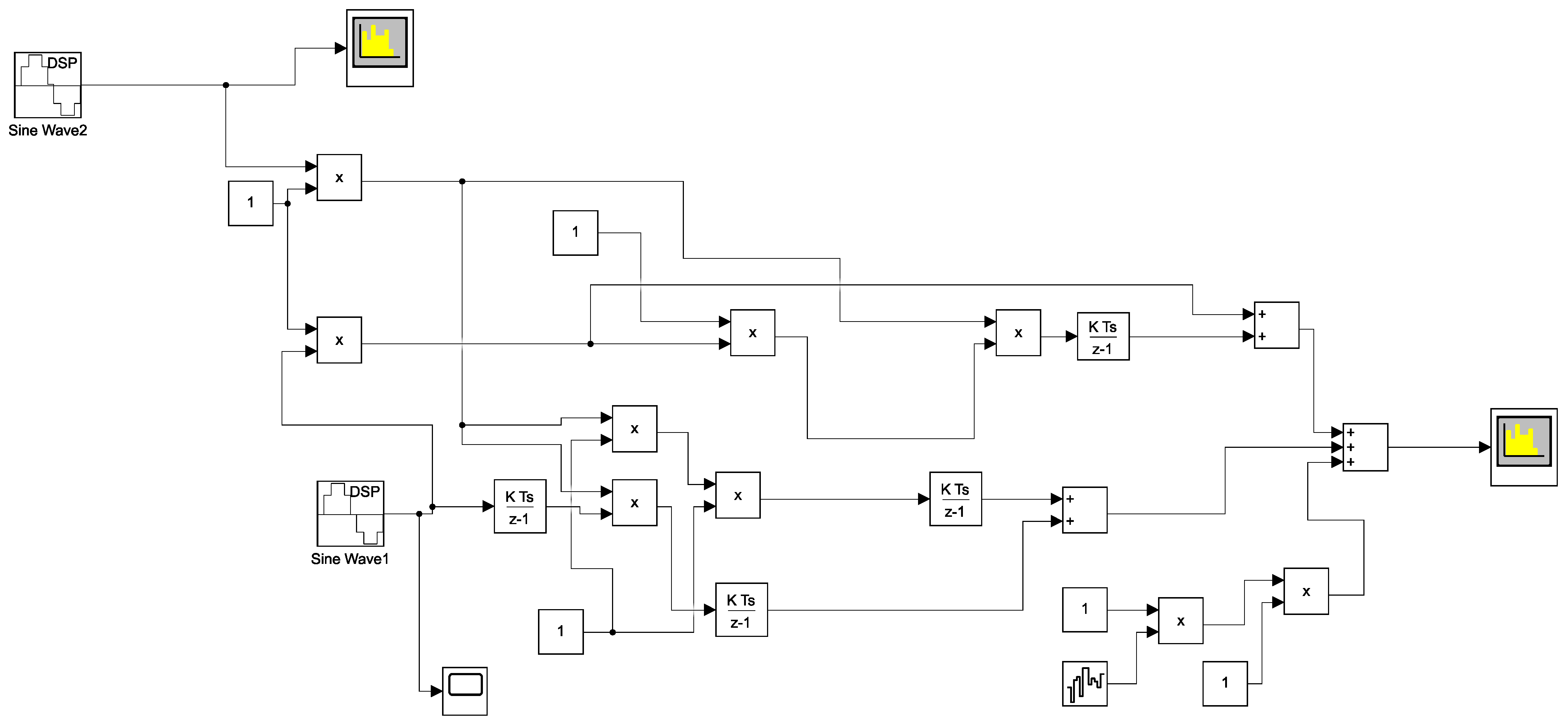
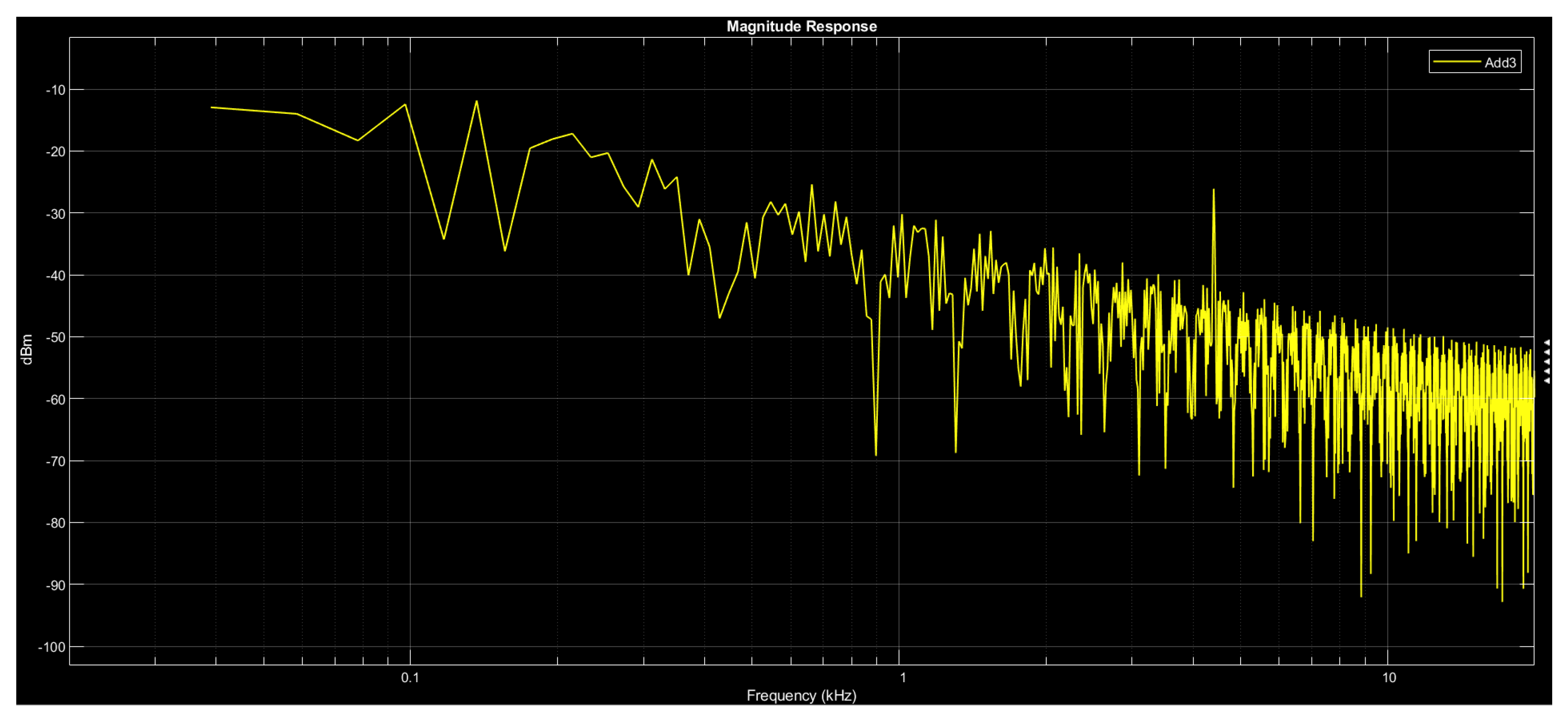
4. Example
4.1. Example-I
4.2. Example-II
- 1.
- Product modulator 1 receives the input , and G produces .
- 2.
- Product modulator 2 receives the input , and B produces .
- 3.
- Product modulator 3, receives the input , and at time produces .
- 4.
- The integrator performs the integral ofover the period s.
- (i)
- Inputs are combined and multiplied with an output of the integrator over .
- (ii)
- Inputs are combined and multiplied with an output of the integrator over .
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ahmad, B.; Alsaedi, A.; Ntouyas, S.K.; Tariboon, J. Hadamard-Type Fractional Differential Equations, Inclusions and Inequalities; Springer International Publishing AG: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Diethelm, K. The Analysis of Fractional Differential Equations: An Application-Oriented Exposition Using Differential Operators of Caputo Type; Lecture Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Lakshmikantham, V.; Vatsala, A.S. Basic theory of fractional differential equations. Nonlinear Anal. Theory Methods Appl. 2008, 69, 2677–2682. [Google Scholar] [CrossRef]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Differential Equations; John Wiley: New York, NY, USA, 1993. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Zhou, Y. Basic Theory of Fractional Differential Equations; World Scientific: Singapore, 2014. [Google Scholar]
- Zhou, Y. Fractional Evolution Equations and Inclusions: Analysis and Control; Elsevier: New York, NY, USA, 2015. [Google Scholar]
- Agarwal, R.P.; Lakshmikanthan, V.; Nieto, J.J. On the concept of solution for fractional differential equations with uncertainty. Nonlinear Anal. Theory Methods Appl. 2010, 72, 2859–2862. [Google Scholar] [CrossRef]
- Dineshkumar, C.; Udhayakumar, R.; Vijayakumar, V.; Nisar, K.S. Results on approximate controllability of neutral integro-differential stochastic system with state-dependent delay. Numer. Methods Partial Differ. Equ. 2020. [Google Scholar] [CrossRef]
- Khaminsou, B.; Thaiprayoon, C.; Sudsutad, W.; Jose, S.A. Qualitative analysis of a proportional Caputo fractional Pantograph differential equation with mixed nonlocal conditions. Nonlinear Funct. Anal. Appl. 2021, 26, 197–223. [Google Scholar]
- Sivasankar, S.; Udhayakumar, R. New Outcomes Regarding the Existence of Hilfer Fractional Stochastic Differential Systems via Almost Sectorial Operators. Fractal Fract. 2022, 6, 522. [Google Scholar] [CrossRef]
- Williams, W.K.; Vijayakumar, V.; Udhayakumar, R.; Nisar, K.S. A new study on existence and uniqueness of nonlocal fractional delay differential systems of order 1 < r < 2 in Banach spaces. Numer. Methods Partial Differ. Equ. 2020, 37, 949–961. [Google Scholar]
- Serrano, E.Z.; Munoz-Pacheco, J.M.; Serrano, F.E.; Sánchez-Gaspariano, L.A.; Volos, C. Experimental verification of the multi-scroll chaotic attractors synchronization in PWL arbitrary-order systems using direct coupling and passivity-based control. Integr. VLSI J. 2021, 81, 56–70. [Google Scholar] [CrossRef]
- Ding, Y.; Liu, X.; Chen, P.; Luo, X.; Luo, Y. Fractional-Order Impedance Control for Robot Manipulator. Fractal Fract. 2022, 6, 684. [Google Scholar] [CrossRef]
- Abdelhadi, M.; Alhazmi, S.; Al-Omari, S. On a Class of Partial Differential Equations and Their Solution via Local Fractional Integrals and Derivatives. Fractal Fract. 2022, 6, 210. [Google Scholar] [CrossRef]
- Hasan, S.; Harrouche, N.; Al-Omari, S.K.Q.; Al-Smadi, M.; Momani, S.; Cattani, C. Hilbert solution of fuzzy fractional boundary value problems. Appl. Math. Comput. 2022, 41, 1–22. [Google Scholar]
- Edwan, R.; Al-Omari, S.; Al-Smadi, M.; Momani, S.; Fulga, A. A new formulation of finite-difference and finite volume methods for solving a space-fractional convection-diffusion model with less error estimates. Adv. Differ. Equ. 2021, 2021, 1–19. [Google Scholar] [CrossRef]
- Momani, S.; Djeddi, N.; Al-Smadi, M.; Al-Omari, S. Numerical investigation for Caputo-Fabrizio fractional Riccati and Bernoulli equations using iterative reproducing kernel method. Appl. Numer. Math. 2021, 170, 418–434. [Google Scholar] [CrossRef]
- Al-Smadi, M.; Djeddi, N.; Momani, S.; Al-Omari, S.; Araci, S. An attractive numerical algorithm for solving nonlinear Caputo-Fabrizio fractional Abel differential equation in a Hilbert space. Adv. Differ. Equ. 2021, 271, 1–19. [Google Scholar] [CrossRef]
- Wang, J.R.; Zhou, Y. Complete controllability of fractional evolution systems. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 4346–4355. [Google Scholar] [CrossRef]
- Wang, J.R.; Fin, Z.; Zhou, Y. Nonlocal controllability of semilinear dynamic systems with fractional derivative in Banach spaces. J. Optim. Theory Appl. 2012, 154, 292–302. [Google Scholar] [CrossRef]
- Ji, S.; Li, G.; Wang, M. Controllability of impulsive differential systems with nonlocal conditions. Appl. Math. Comput. 2011, 217, 6981–6989. [Google Scholar] [CrossRef]
- Balachandran, K.; Sakthivel, R. Controllability of integro-differential systems in Banach spaces. Appl. Math. Comput. 2001, 118, 63–71. [Google Scholar]
- Wang, R.N.; Chen, D.H.; Xiao, T.J. Abstract fractional Cauchy problems with almost sectorial operators. J. Differ. Equ. 2012, 252, 202–235. [Google Scholar] [CrossRef]
- Mohan Raja, M.; Vijayakumar, V.; Udhayakumar, R. Results on existence and controllability of fractional integro-differential system of order 1 < r < 2 via measure of noncompactness. Chaos Solitons Fractals. 2020, 139, 110299. [Google Scholar]
- Evans, L.C. An Introduction to Stochastic Differential Equations; University of California: Berkeley, CA, USA, 2013. [Google Scholar]
- Mao, X. Stochastic Differential Equations and Applications; Horwood: Chichester, UK, 1997. [Google Scholar]
- Sivasankar, S.; Udhayakumar, R. A note on approximate controllability of second-order neutral stochastic delay integro-differential evolution inclusions with impulses. Math. Methods Appl. Sci. 2022, 45, 6650–6676. [Google Scholar] [CrossRef]
- Li, F. Mild solutions for abstract differential equations with almost sectorial operators and infinite delay. Adv. Differ. Equ. 2013, 327, 1–11. [Google Scholar] [CrossRef][Green Version]
- Pazy, A. Semigroups of Linear Operators and Applications to Partial Differential Equations. Applied Mathematical Sciences; Springer: New York, NY, USA, 1983. [Google Scholar]
- Periago, f.; Straub, B. A functional calculus for almost sectorial operators and applications to abstract evolution equations. J. Evol. Equ. 2002, 2, 41–68. [Google Scholar] [CrossRef]
- Sivasankar, S.; Udhayakumar, R. Hilfer Fractional Neutral Stochastic Volterra Integro-Differential Inclusions via Almost Sectorial Operators. Mathematics 2022, 10, 2074. [Google Scholar] [CrossRef]
- Zhang, L.; Zhou, Y. Fractional Cauchy problems with almost sectorial operators. Appl. Math. Comput. 2014, 257, 145–157. [Google Scholar] [CrossRef]
- Hilfer, R. Application of Fractional Calculus in Physics; World Scientific: Singapore, 2000. [Google Scholar]
- Dineshkumar, C.; Udhayakumar, R. New results concerning to approximate controllability of Hilfer fractional neutral stochastic delay integro-differential system. Numer. Methods Partial Differ. Equ. 2020, 37, 1072–1090. [Google Scholar] [CrossRef]
- Gu, H.; Trujillo, J.J. Existence of integral solution for evolution equation with Hilfer fractional derivative. Appl. Math. Comput. 2015, 257, 344–354. [Google Scholar]
- Varun Bose, C.S.; Udhayakumar, R. Existence of Mild Solutions for Hilfer Fractional Neutral Integro-Differential Inclusions via Almost Sectorial Operators. Fractal Fract. 2022, 6, 532. [Google Scholar] [CrossRef]
- Kavitha, K.; Vijayakumar, V.; Udhayakumar, R. Results on controllability of Hilfer fractional neutral differential equations with infinite delay via measure of noncompactness. Chaos Solitons Fractals. 2020, 139, 110035. [Google Scholar] [CrossRef]
- Varun Bose, C.S.; Udhayakumar, R. A note on the existence of Hilfer fractional differential inclusions with almost sectorial operators. Math. Methods Appl. Sci. 2022, 45, 2530–2541. [Google Scholar] [CrossRef]
- Yang, M.; Wang, Q. Existence of mild solutions for a class of Hilfer fractional evolution equations with nonlocal conditions. Fract. Calc. Appl. Anal. 2017, 20, 679–705. [Google Scholar] [CrossRef]
- Bedi, P.; Kumar, A.; Abdeljawad, T.; Khan, Z.A.; Khan, A. Existence and approximate controllability of Hilfer fractional evolution equations with almost sectorial operators. Adv. Differ. Equ. 2020, 615, 1–15. [Google Scholar] [CrossRef]
- Jaiswal, A. Bahuguna, D. Hilfer fractional differantial equations with almost sectorial operators. Differ. Equ. Dyn. Syst. 2020. [Google Scholar] [CrossRef]
- Karthikeyan, K.; Debbouche, A.; Torres, D.F.M. Analysis of Hilfer fractional integro-differential equations with almost sectorial operators. Fractal Fract. 2021, 5, 22. [Google Scholar] [CrossRef]
- Singh, V. Controllability of Hilfer fractional differential systems with non-dense domain. Numer. Funct. Anal. Optim. 2019, 40, 1572–1592. [Google Scholar] [CrossRef]
- Sivasankar, S.; Udhayakumar, R.; Subramanian, V.; AlNemer, G.; Elshenhab, A.M. Existence of Hilfer Fractional Stochastic Differential Equations with Nonlocal Conditions and Delay via Almost Sectorial Operators. Mathematics 2022, 10, 4392. [Google Scholar] [CrossRef]
- Atraoui, M.; Bouaouid, M. On the existence of mild solutions for nonlocal differential equations of the second order with conformable fractional derivative. Adv. Differ. Equ. 2021, 447, 1–11. [Google Scholar] [CrossRef]
- Bouaouid, M.; Hannabou, M.; Hilal, K. Nonlocal conformable fractional differential equations with a measure of noncompactness in Banach spaces. J. Math. 2020, 2020, 5615080. [Google Scholar] [CrossRef]
- Zhou, M.; Li, C.; Zhou, Y. Existence of mild solutions for Hilfer fractional differential evolution equations with almost sectorial operators. Axioms 2022, 11, 144. [Google Scholar] [CrossRef]
- Don, H.; Jainzhong, W.; Robert, G. The Lebesgue Integral; Real Analysis with an Introduction to Wavelets and Application; Academic Press: Cambridge, MA, USA, 2005. [Google Scholar]
- Broner, N. The effects of low frequency noise on people-A review. J. Sound Vib. 1978, 58, 483–500. [Google Scholar] [CrossRef]
- Agnew, D.C.; Hodgkinson, K. Designing Compact Causal Digital Filters for Low-Frequency Strainmeter Data. Bull. Seismol. Soc. Am. 2007, 97, 91–99. [Google Scholar] [CrossRef]
- Chandra, A.; Chattopadhyay, S. Design of hardware efficient FIR filter: A review of the state of the art approaches. Eng. Sci. Technol. Int. J. 2016, 19, 212–226. [Google Scholar] [CrossRef]
- Vijayakumar, V.; Udhayakumar, R. Results on approximate controllability for non-densely defined Hilfer fractional differential system with infinite delay. Chaos Solitons Fractals. 2020, 139, 110019. [Google Scholar] [CrossRef]
- Zahoor, S.; Naseem, S. Design and implementation of an efficient FIR digital filter. Cogent. Eng. 2017, 4, 1323373. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sivasankar, S.; Udhayakumar, R.; Hari Kishor, M.; Alhazmi, S.E.; Al-Omari, S. A New Result Concerning Nonlocal Controllability of Hilfer Fractional Stochastic Differential Equations via almost Sectorial Operators. Mathematics 2023, 11, 159. https://doi.org/10.3390/math11010159
Sivasankar S, Udhayakumar R, Hari Kishor M, Alhazmi SE, Al-Omari S. A New Result Concerning Nonlocal Controllability of Hilfer Fractional Stochastic Differential Equations via almost Sectorial Operators. Mathematics. 2023; 11(1):159. https://doi.org/10.3390/math11010159
Chicago/Turabian StyleSivasankar, Sivajiganesan, Ramalingam Udhayakumar, Muchenedi Hari Kishor, Sharifah E. Alhazmi, and Shrideh Al-Omari. 2023. "A New Result Concerning Nonlocal Controllability of Hilfer Fractional Stochastic Differential Equations via almost Sectorial Operators" Mathematics 11, no. 1: 159. https://doi.org/10.3390/math11010159
APA StyleSivasankar, S., Udhayakumar, R., Hari Kishor, M., Alhazmi, S. E., & Al-Omari, S. (2023). A New Result Concerning Nonlocal Controllability of Hilfer Fractional Stochastic Differential Equations via almost Sectorial Operators. Mathematics, 11(1), 159. https://doi.org/10.3390/math11010159







