Influence of Context on Greatest Common Divisor Problem Solving: A Qualitative Study
Abstract
1. Introduction
2. Theoretical Framework
3. Objectives
- (O1)
- Study competence in solving verbal problems in different situations of divisibility involving the concept of g.c.d.
- (O2)
- Analyze whether some contexts present greater difficulties than others do.
- (O3)
- Find different erroneous resolution patterns and inquire about their origin.
4. Methodology
- (1)
- Problems with solutions obtained directly through the calculation of the g.c.d. or the g.c.d. and a division operation.
- (2)
- Problems in five different contexts: length, area, volume, discrete sets, and decontextualized numbers.
- GCDDQ problem: problem whose data refer to discrete sets and in which the g.c.d. must be obtained for its resolution, and division must be performed.
- GCDDL problem: problem whose data refer to continuous sets measuring length (meters) and in which the g.c.d. must be obtained for its resolution and division must be performed.
- GCDDC problem: problem whose data refer to continuous sets measuring volume (liters) and in which the g.c.d. must be obtained for its resolution and division must be performed.
- GCDDA problem: problem whose data refer to area and in which the g.c.d. must be obtained for its resolution and division must be performed.
- GCDQ problem: problem whose data refer to discrete sets and in which only the g.c.d. must be obtained for its resolution.
- GCDL problem: problem whose data refer to continuous sets measuring length (meters) and in which only the g.c.d. must be obtained for its resolution.
- GCDC problem: problem whose data refer to continuous sets measuring capacity (liters) and in which only the g.c.d. must be obtained for its resolution.
- GCDA problem: problem whose data refer to areas and in which only the g.c.d. must be obtained for its resolution.
- GCDD problem: problem whose data refer to discrete decontextualized quantities and in which only the g.c.d. should be obtained for its resolution.
5. Preliminary Analysis
6. Qualitative Analysis: Case Study
6.1. Case of Context: Areas
“You want to parcel out a field, 36 m long and 45 m wide, in square plots as large as possible, how long will these plots measure?”
- Student C:
- Buff, this problem is like the one the other day, buff, I don’t know… (referring to the similar problem they did in the written test)
- Student X:
- Okay, but let’s see what you ask for…
- Student C:
- I don’t know how to do it, ask what the plots will be like
- Student X:
- Let’s see…
- Interviewer:
- Why do you find this problem difficult?
- Student C:
- It is asking what the plots measure.
- Interviewer:
- Ok, can you describe the problem in your own words?
- Student C:
- We have a piece of land and…
- Student X:
- We want to divide it into large squares
- Interviewer:
- Ok, what is the data?
- Student C:
- The land is 36 and 45 m (write the data on the board)
- Interviewer:
- Are the data of the same type?
- Student X:
- Uh… yeah… it’s meters.
- Interviewer:
- What is required?
- Student C:
- Ehh… what the plots measure.
- Interviewer:
- Is the unknown the same type as the data?
- Student C:
- Well, ask for the area, I think…
- Student X:
- Good or the side of the plot.
- Student C:
- Yes, now.
- Interviewer:
- Is it the same type?
- Student C:
- It will be meters too.
- Interviewer:
- Draw the terrain (Student X draws a square on the board).
- Interviewer:
- Now put in a possible plot (Student X draws a small square in the upper right corner of the plot of land).
- Interviewer:
- What is required?
- Student C:
- This… (pointing to the small square).
- Interviewer:
- So what is asked is the side of the plot, right?
- Student C:
- Oh sure, okay.
- Interviewer:
- So, can you explain in your words what you have to do?
- Student C:
- Yes, we have to calculate the side of the plots, knowing that the total land is that (indicating the drawing).
- Interviewer:
- How are the data and the unknown related?
- Student C:
- Everything is meters.
- Student X:
- The plots are smaller.
- Interviewer:
- So is there a concept that relates the data and the unknown?
- Student C:
- What operation could we do?
- Interviewer:
- Do you think that the g.c.d. of the two quantities could be the solution?
- Student C:
- Ehh… no.
- Interviewer:
- Why?
- Student C:
- Well, the 18 m are here and the others are below (indicating the vertical and horizontal lines of the drawing of the terrain on the board).
- Interviewer:
- And that, what does it mean?
- Student C:
- Well, there cannot be common divisors.
- Interviewer:
- Why do you think they have no common divisors?
- Student C:
- They are not the same, they are in different places…
- Interviewer:
- So if it were 2 strings…
- Student C:
- So yeah
- Interviewer:
- And you, what do you think?—referring to Student X-
- Student X:
- The same, if it were two strings we could do the g.c.d.
6.2. Case of l.c.m.
“Marc has 18 stickers for soccer players and 30 for basketball players. If you want to put them in envelopes with the same number of stickers without mixing the soccer players with the basketball players and getting the greatest number of players per envelope, how many envelopes do you have to prepare?”
- Student G:
- Let’s see, fifteen… And of the others, twenty-four.
- Student L:
- Same envelopes.
- Student G:
- And they ask us how many envelopes.
- Student L:
- Yes.
- Student G:
- As we distribute the cards, we draw… it seems to me that the greatest common divisor, but…
- Student L:
- Uh… okay.
- Student L:
- Get 6 stickers.
- Student G:
- But you ask us the number of envelopes, right?
- Student L:
- Ah… and then what?
- Student G:
- Buff, then it will be the l.c.m.
- Student L:
- It could be
- Interviewer:
- Do you think the result is in line with what was requested?
- Student L:
- Yes, that’s 90 envelopes.
- Interviewer:
- If you read the statement again, knowing the resulting envelopes, is it possible?
- Student G:
- Let’s see if we have 18 for soccer and 30 for basketball and we put them in 90 envelopes… oh!… It can’t be.
- Student L:
- They have to be less envelopes, of course.
- Student G:
- Buff… well I don’t know
6.3. Case of g.c.d.
“Marc has 18 stickers for soccer players and 30 for basketball players. If you want to put them in envelopes with the same number of stickers without mixing the soccer players with the basketball players and that there is the greatest number of players per envelope, how many envelopes do you have to prepare? “
- Student M:
- I put the data, okay?
- Student S:
- Ok.
- Student S:
- That’s it, there is no more data.
- Student M:
- We have to find out the envelopes…
- Student S:
- So we have to divide, find the greatest common divisor, right?
- Student M:
- Well, but… yes, okay.
- Student S:
- Get 6 envelopes.
- Student M:
- Good.
- Interviewer:
- Just one question, is the result envelopes or stickers?
- Student S:
- They ask us for the envelopes.
- Student M:
- Of course… you have to distribute in envelopes, therefore divide. It is a divisor, … the g.c.d. are the envelopes.
7. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Godino, J.; Batanero, C.; Font, V. Matemáticas y su Didáctica Para Maestros; Universidad de Granada: Granada, Spain, 2003. [Google Scholar]
- Ausubel, D. Teoría del Aprendizaje Significativo. 1983. Available online: https://www.academia.edu/10435788/teoría_teoria_del_aprendizaje_significativo (accessed on 23 August 2020).
- Chamorro, M.D.C. Didáctica de las Matemáticas Para Educación Infantil; Pearson Educación: Madrid, Spain, 2005. [Google Scholar]
- Castro, E. Didáctica de la Matemática en la Educación Primaria; Síntesis, S.A.: Madrid, Spain, 2001. [Google Scholar]
- Rayas, J. El Reconocimiento de las Ideas Previas Como Condición Necesaria Para Mejorar las Posibilidades de los Alumnos en los Procesos Educativos en Ciencias Naturales. Revista Xictli de la Unidad UPD. n. 94. 2004. Available online: http://189.208.102.74/u094/revista/54/02.html (accessed on 23 August 2020).
- Pantano, L.V. Enseñando adición y sustracción a partir del método para el aprendizaje natural de las matemáticas y la granja de Don Juan. Góndola Enseñanza Aprendiz. Cienc. 2014, 9, 60–78. [Google Scholar] [CrossRef][Green Version]
- Arias-Rueda, J.H.; Arias-Rueda, C.A.; Burgos-Hernández, C.A. Procesos aplicados por los estudiantes en la resolución de problemas matemáticos: Caso de estudio sobre la función cuadrática. Góndola Enseñanza Y Aprendiz. Cienc. 2020, 15, 129–147. [Google Scholar] [CrossRef]
- Blanco, M.; Bermejo, V. Dificultades de aprendizaje en matemáticas. In Cómo Enseñar Matemáticas para Aprender Mejor; Editorial CCS: Madrid, Spain, 2004; pp. 215–238. [Google Scholar]
- Carrillo, B. Dificultades en el Aprendizaje Matemático. Innovación Exp. Educ. 2009, 16, 1–10. Available online: https://archivos.csif.es/archivos/andalucia/ensenanza/revistas/csicsif/revista/pdf/Numero_16/BEATRIZ_CARRILLO_2.pdf (accessed on 3 March 2022).
- Zazkis, R.; Campbell, S. Divisibility and multiplicative structure of natural numbers: Preservice teacher’s understanding. J. Res. Math. Educ. 1996, 27, 540–563. [Google Scholar] [CrossRef]
- Ordoñez, C. La Construcción de la Noción de División y Divisibilidad de Números Naturales, Mediada por Justificaciones, en Alumnos de Tercer Grado de Nivel de Primaria. Educación Matemática Master’s Thesis, Pontificia Universidad Católica del Perú, Lima, Perú, 2014. [Google Scholar]
- Vallejo, E. Análisis y Propuesta en Torno a las Justificaciones en la Enseñanza de la Divisibilidad en el Primer Grado de Secundaria. Educación Matemática Master’s Theisis, Pontificia Universidad Católica del Perú, Lima, Perú, 2012. [Google Scholar]
- Noblet, K. Preservice elementary teachers’ understanding of greatest common factor story problems. In Proceedings of the 16th Annual Conference on Research in Undergraduate Mathematics Education, Denver, CO, USA, 21–23 February 2013; Sigma: Denver, CO, USA, 2013; pp. 219–225. [Google Scholar]
- Gutiérrez-Gutiérrez, A.; Gómez, P.; Rico, L. Conocimiento matemático sobre números y operaciones de los estudiantes de Magisterio. Educ. XX1 2016, 19, 135–158. [Google Scholar] [CrossRef][Green Version]
- Martínez, S.; González-Calero, J.A.; Sotos, M.A. La influencia del enunciado en la relación de problemas de m.c.d. y m.c.m. de estudiantes para maestro. In Investigación en Educación Matemática XIX; Fernández, C., Molina, M., Planas, N., Eds.; SEIEM: Alicante, Spain, 2015; pp. 343–350. [Google Scholar]
- González-Calero, J.A.; Martínez, S.; Sotos, M.A. La tendencia a restar en la resolución de problemas de g.c.d. en alumnos de primaria. In Investigación en Educación Matemática XX; Macías, J.A., Jiménez, A., González, J.L., Sánchez, M.T., Hernández, P., Fernández, C., Ruiz, F.J., Fernández, T., Berciano, A., Eds.; SEIEM: Málaga, Spain, 2016; p. 295. [Google Scholar]
- Dias, A. Using lattice models to determine greatest common factor and least common multiple. Int. J. Math. Teach. Learn. 2005, 730–738. Available online: https://www.cimt.org.uk/journal/dias.pdf (accessed on 3 March 2022).
- Zazkis, R.; Gadowsky, K. Attending to transparent features of opaque representations of natural numbers. In NCTM 2001 Yearbook: The Roles of Representation in School Mathematics; Cuoco, A., Ed.; NCTM: Reston, VA, USA, 2001; pp. 41–52. [Google Scholar]
- Zazkis, R.; Liljedahl, P. Understanding primes: The role of representation. J. Res. Math. Educ. 2004, 35, 164–186. [Google Scholar] [CrossRef][Green Version]
- Uribe, L.C. Estrategias cualitativas de investigación en Educación Matemática. In Proceedings of the XV Conferencia Interamericana de Educación Matemática, Medellín, Colombia, 5–10 May 2019. [Google Scholar]
- Bodí, S. Análisis de la Comprensión de Divisibilidad en el Conjunto de los Números Naturales. Ph.D. Thesis, Universitat d’Alacant, Alicante, Spain, 2006. [Google Scholar]
- Dwi Hastuti, I.; Listiawan, T.; Komariah, A.; Dabirnia, M. Fourth-grade primary school students’ misconception on greatest common factor and least common multiple. Educ. Res. Int. 2021, 2021, 6581653. [Google Scholar]
- Li, H.C.; Tsai, T.L. The effects of a problem-based learning intervention on primary students’ performance on greatest common factor and least common multiple and on their attitudes towards mathematics. Int. J. Innov. Learn. 2022, 31, 51–69. [Google Scholar] [CrossRef]
- Nortes Martinez-Artero, R.; Nortes Checa, A. Los problemas de m.c.d. y m.c.m. en futuros maestros. In Investigaciones Sobre la Formación Docente en el Siglo XXI; Maquilón Sánchez, J.J., Izquierdo Rus, T., Belmonte Almagro, M.L., Alfageme González, M.B., Eds.; Ediciones de la Universidad de Murcia: Murcia, Spain, 2018; pp. 91–104. [Google Scholar]
- Puri, R.I.I. Developing learning trajectory using traditional games in supporting students learning greatest common divisor in Indonesian primary school. In Proceedings of the 12th ICME, Soul, Korea, 8–15 July 2012; Volume 1, p. 7721. [Google Scholar]
- Macnab, D.S.; Cummine, J.A. La Enseñanza de las Matemáticas de 11 a 16: Un Enfoque Centrado en la Dificultad; Aprendizaje Visor: Madrid, Spain, 1992. [Google Scholar]
- Alba, M.Á.B.; Mellado, F.J.C.; Compaña, M.T.S.; Palacián, M.A. Pensamiento matemático avanzado y scratch: El caso del máximo común divisor. Pensam. Matemático 2017, 7, 43–64. [Google Scholar]
- Baeza Alba, M.Á.; Arnal Palacián, M.; Claros Mellado, F.J.; Sánchez Compaña, M.T. Comprensión del máximo común divisor: Propuesta didáctica con scratch. Uno Rev. Didáctica Matemáticas 2018, 80, 43–47. [Google Scholar]
- Bell, A.; Fischbein, E.; Greer, B. Choice of operation in verbal arithmetic problems: The effects of number size, problem structure and context. Educ. Stud. Math. 1984, 15, 129–147. [Google Scholar] [CrossRef]
- Puig, L.; Cerdán, F. Problemas Aritméticos Escolares; Síntesis: Madrid, Spain, 1988. [Google Scholar]

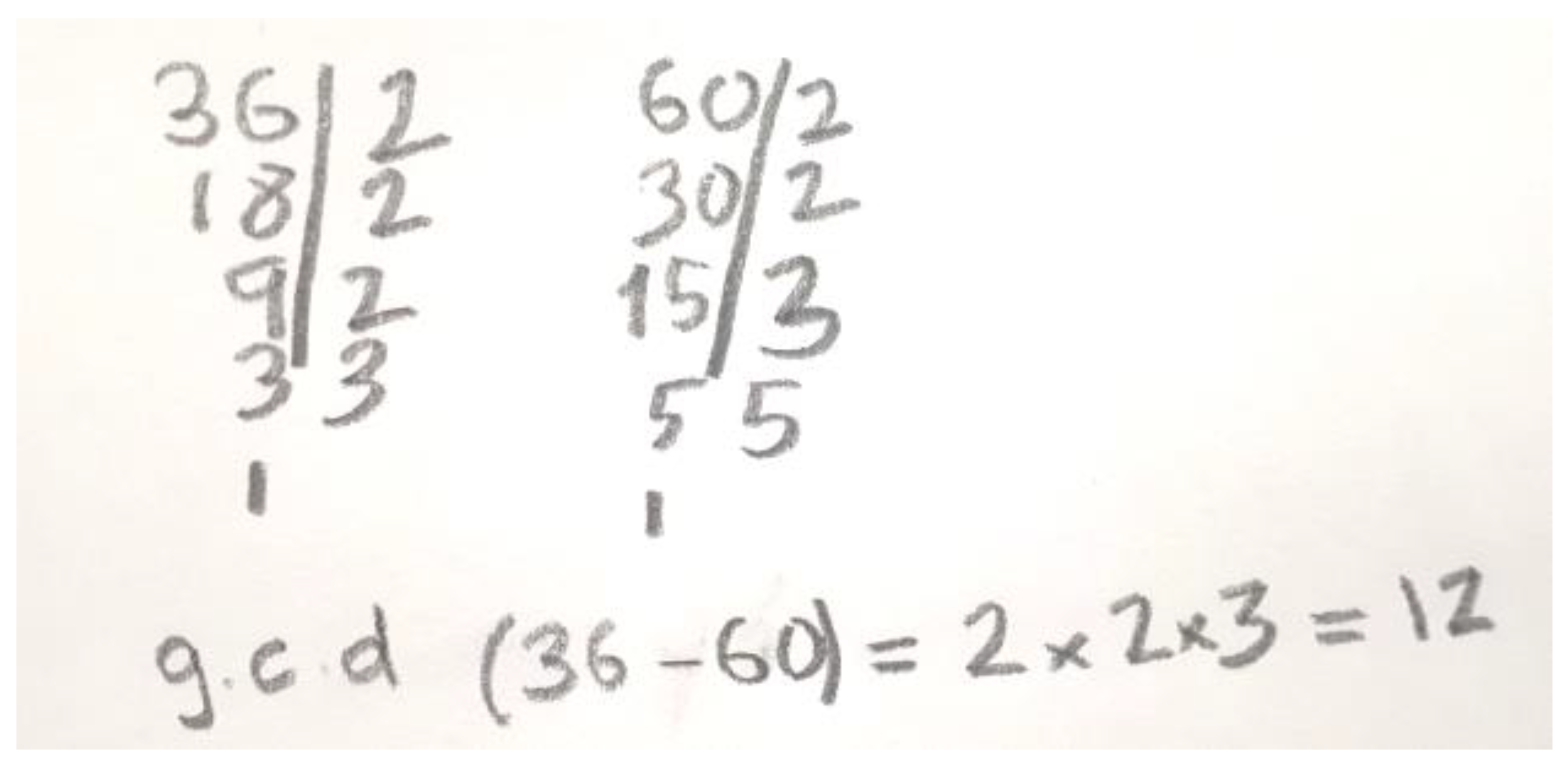
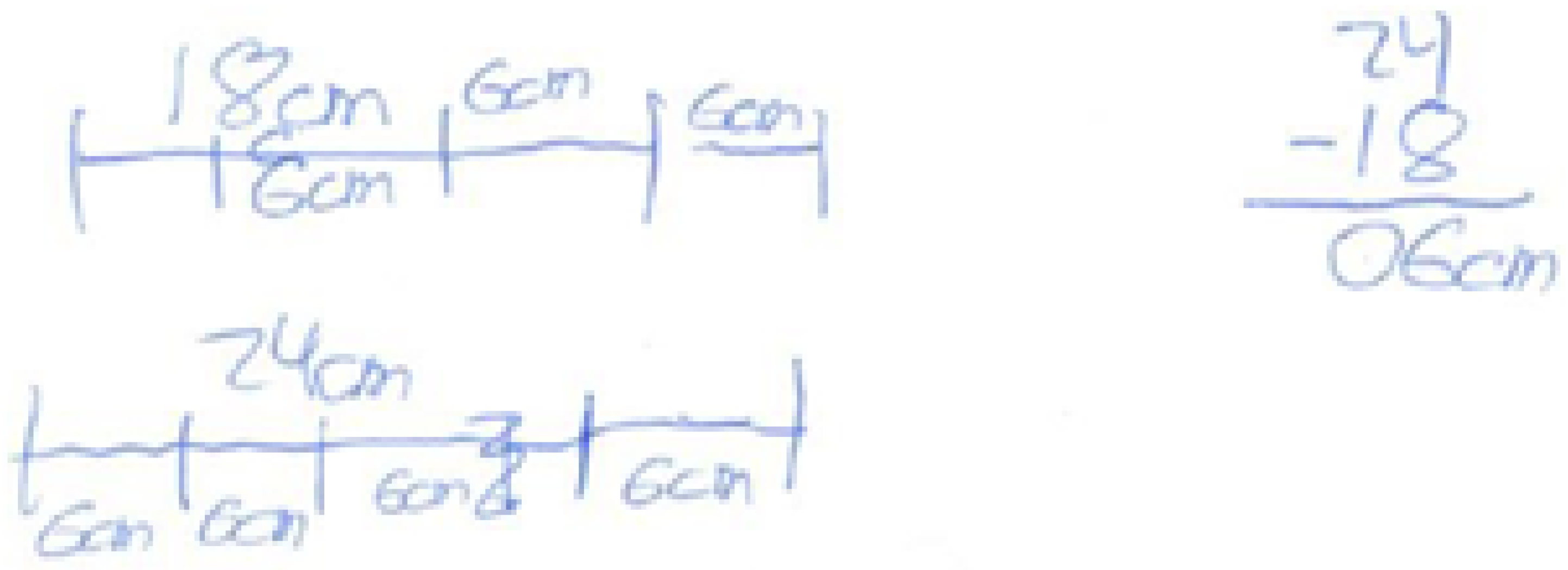
| Key | Operation | Context | Statement |
|---|---|---|---|
| GCDDQ | GCD + div | DISCRETE SETS | Carla has 36 strawberry candies and 60 chocolate candies. She wants to separate them in identical boxes with the largest number of candies without mixing flavors. How many boxes does she need? |
| GCDDL | GCD + div | LENGTH | For one of the camp tests, a scout group needs to prepare equal length ribbons as long as possible from two strings, one of 15 cm and another of 24 cm, how many ribbons can they get without leftover strings? |
| GCDDC | GCD + div | VOLUME | Calculate how many containers of maximum volume are needed to be able to measure exactly a 12-L and a 40-L container. |
| GCDDA | GCD + div | AREA | A carpenter wants to cut a wooden board that measures 18 cm long and 75 cm wide, into squares as large as possible. How many squares does he get? |
| GCDQ | GCD | DISCRETE SETS | Carla has 18 strawberry candies and 30 chocolate candies. If Carla wants to put them in boxes with the same number of chocolates without mixing flavors, how many candies can you put maximum in each box? |
| GCDL | GCD | LENGTH | A group of scouts needs to prepare ties for the camp trial. If you have two strings, one 18 cm and the other 24 cm, what is the largest possible size that you can cut the ties from both strings, so that they are all identical? |
| GCDC | GCD | VOLUME | Calculate the maximum volume that a container must have in order to measure exactly the contents of two containers of 12 and 40 L. |
| GCDA | GCD | AREA | A carpenter wants to cut a wooden board that measures 18 cm long and 75 cm wide, into squares as large as possible. What is the squares? |
| GCDD | GCD | Decontextualized | Find the largest number that can exactly divide both 54 and 30. |
| Type of Problem | Total Correct |
|---|---|
| GCDDQ | 43.7% |
| GCDDL | 40.8% |
| GCDDC | 35.2% |
| GCDDA | 26.8% |
| GCDQ | 67.6% |
| GCDL | 69.0% |
| GCDC | 56.3% |
| GCDA | 31.0% |
| GCDD | 70.4% |
| All | 19.7% |
| Type of Problem | Total Incorrect | l.c.m. | g.c.d. | Others | Blank |
|---|---|---|---|---|---|
| GCDDQ | 56.3% | 19.7% | 21.1% | 11.3% | 4.2% |
| GCDDL | 59.2% | 15.5% | 31.0% | 9.9% | 2.8% |
| GCDDC | 64.8% | 22.5% | 32.4% | 8.5% | 1.4% |
| GCDDA | 73.2% | 21.1% | 21.1% | 11.3% | 19.7% |
| GCDQ | 32.4% | 12.7% | - | 19.7% | 0.0% |
| GCDL | 31.0% | 9.9% | - | 21.1% | 0.0% |
| GCDC | 43.7% | 15.5% | - | 18.3% | 9.9% |
| GCDA | 69.0% | 19.7% | - | 14.1% | 35.2% |
| GCDD | 29.6% | 8.5% | - | 21.1% | 0.0% |
| Average | 51.0% | 16.1% | 26.4% | 15.0% | 8.1% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martinez, S.; Valverde, J.C. Influence of Context on Greatest Common Divisor Problem Solving: A Qualitative Study. Mathematics 2022, 10, 1325. https://doi.org/10.3390/math10081325
Martinez S, Valverde JC. Influence of Context on Greatest Common Divisor Problem Solving: A Qualitative Study. Mathematics. 2022; 10(8):1325. https://doi.org/10.3390/math10081325
Chicago/Turabian StyleMartinez, Silvia, and Jose C. Valverde. 2022. "Influence of Context on Greatest Common Divisor Problem Solving: A Qualitative Study" Mathematics 10, no. 8: 1325. https://doi.org/10.3390/math10081325
APA StyleMartinez, S., & Valverde, J. C. (2022). Influence of Context on Greatest Common Divisor Problem Solving: A Qualitative Study. Mathematics, 10(8), 1325. https://doi.org/10.3390/math10081325






