Stochastic Bifurcations and Excitement in the ZS-Model of a Thermochemical Reaction
Abstract
:1. Introduction
2. Deterministic Model
3. Stochastic Model
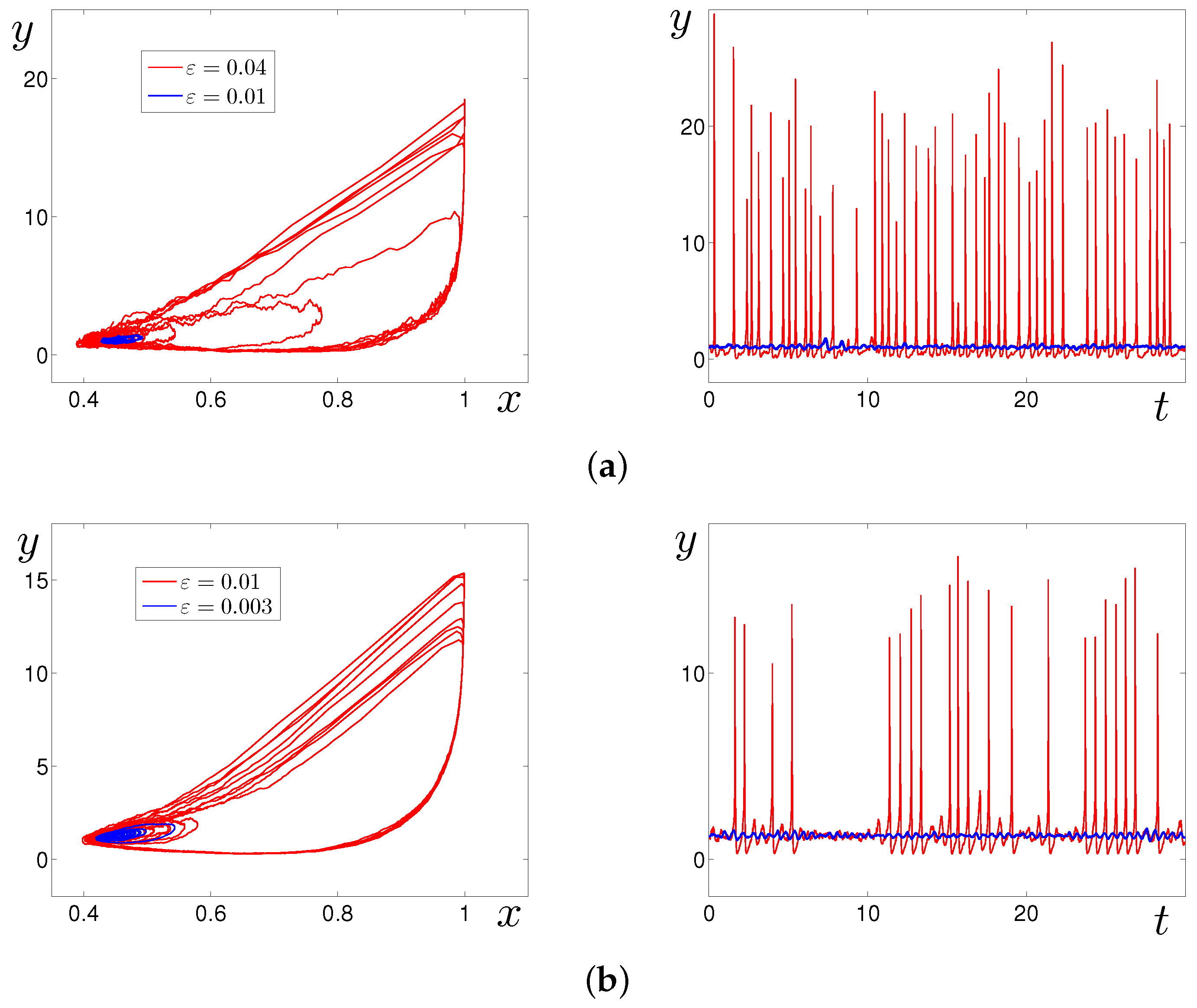
3.1. Stochastic Excitability
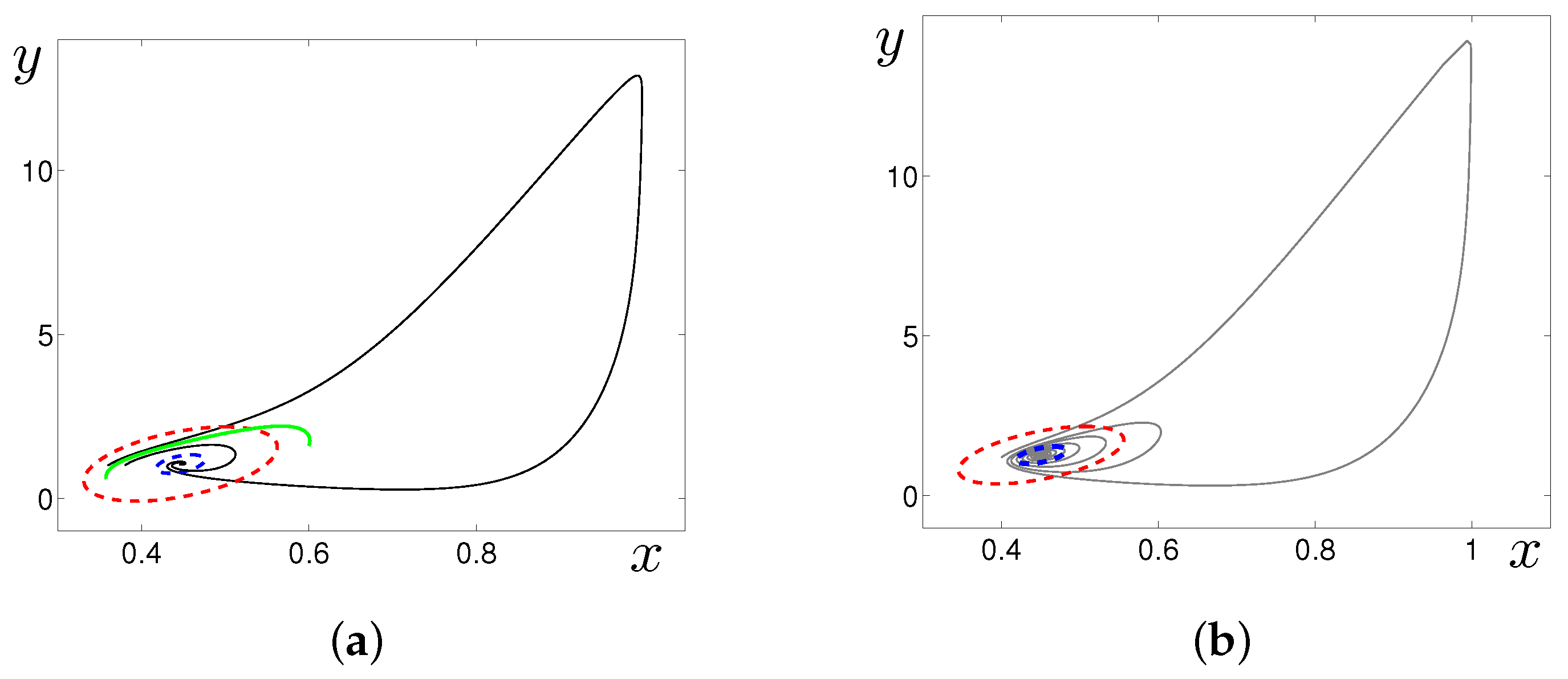
3.2. Stochastic Sensitivity and Confidence Domains
3.3. Stochastic P-Bifurcations
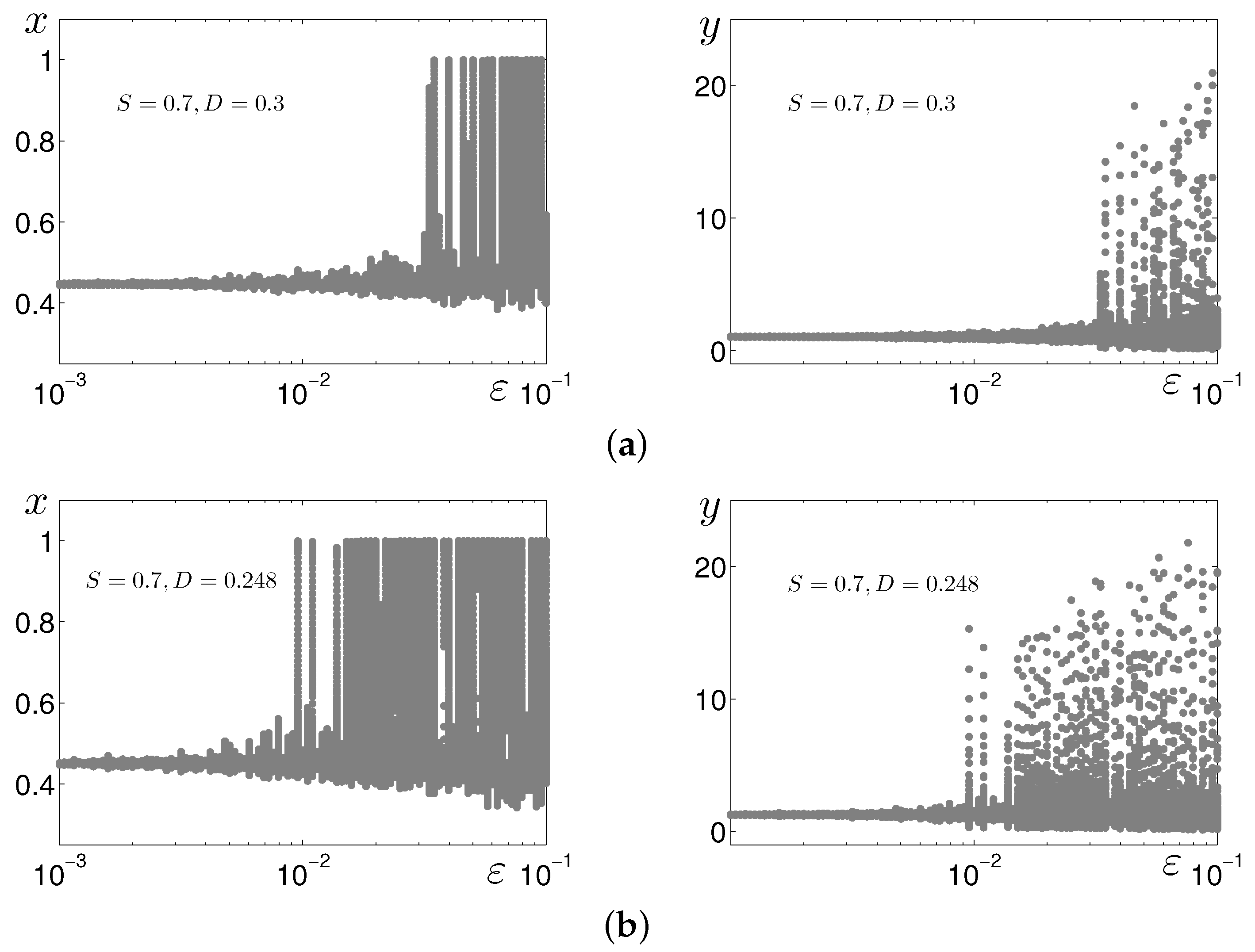
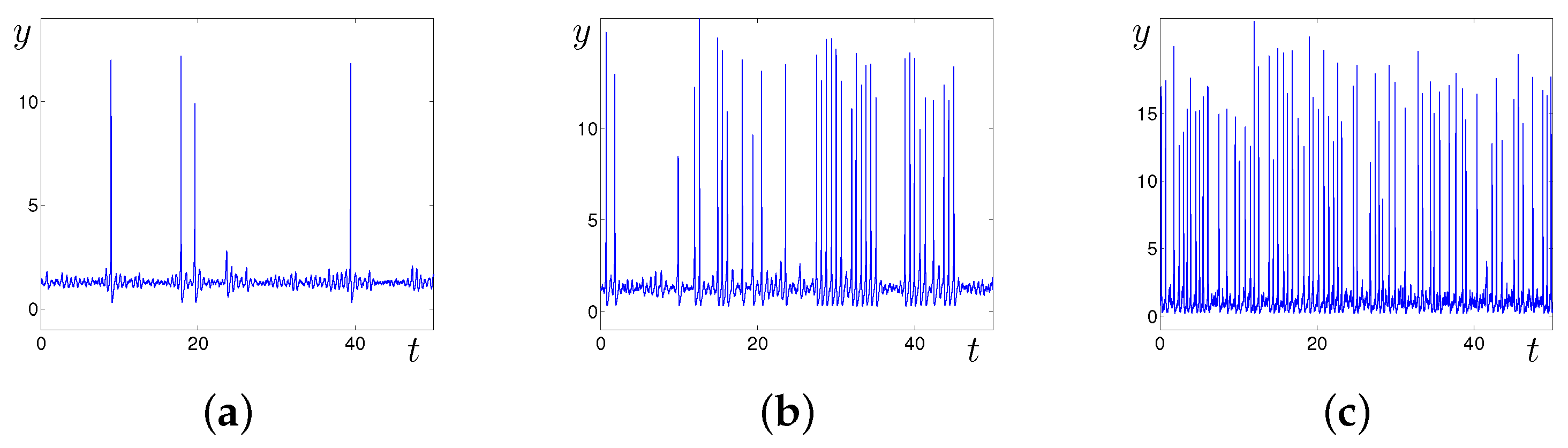
3.4. Frequency Characteristics of Stochastic Oscillations
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Uppal, A.; Ray, W.H.; Poore, A.B. The classification of the dynamic behavior of continuous stirred tank reactors—Influence of reactor resident time. Chem. Eng. Sci. 1976, 31, 205–214. [Google Scholar] [CrossRef]
- Sheplev, V.S.; Treskov, S.A.; Volokitin, E.P. Dynamics of a stirred tank reactor with first-order reaction. Chem. Eng. Sci. 1998, 53, 3719–3728. [Google Scholar] [CrossRef]
- Kawczyński, A.L.; Nowakowski, B. Master equation simulations of a model of a thermochemical system. Phys. Rev. E 2003, 68, 036218. [Google Scholar] [CrossRef] [PubMed]
- Semenov, N.N. Theories of combustion processes. Z. Phys. Chem. 1928, 48, 571–582. [Google Scholar]
- Zeldovich, J.B.; Zysin, J.A. On the theory of heat stress. Exothermic reaction in the jet. Zhurnal Tekhnicheskoi Fiz. 1941, 11, 501–508. [Google Scholar]
- Frank-Kamenetskii, D.A. Diffusion and Heat Exchange in Chemical Kinetics; Princeton University Press: Princeton, NJ, USA, 1955. [Google Scholar]
- Vaganov, D.A.; Samoilenko, N.G.; Abramov, V.G. Periodic regimes of continuous stirred tank reactors. Chem. Eng. Sci. 1978, 33, 1131–1140. [Google Scholar] [CrossRef]
- Bykov, V.I.; Serafimov, L.A.; Tsybenova, S.B. Emergency starting regimes of a continuous stirred tank reactor. Theor. Found. Chem. Eng. 2015, 49, 361–369. [Google Scholar] [CrossRef]
- Bykov, V.I.; Tsybenova, S.B. Parametric analysis of the simplest model of the theory of thermal explosion–the Zel’dovich–Semenov model. Combust. Explos. Shock Waves 2001, 37, 523–534. [Google Scholar] [CrossRef]
- Bykov, V.I.; Tsybenova, S.B.; Yablonsky, G. Chemical Complexity via Simple Models; De Gruyter: Berlin, Germany, 2018. [Google Scholar]
- Arnold, L. Random Dynamical Systems; Springer: Berlin, Germany, 1998; p. 600. [Google Scholar]
- Bashkirtseva, I.; Ryazanova, T.; Ryashko, L. Stochastic bifurcations caused by multiplicative noise in systems with hard excitement of auto-oscillations. Phys. Rev. E 2015, 92, 042908. [Google Scholar] [CrossRef]
- Simpson, S.H.; Arita, Y.; Dholakia, K.; Zemánek, P. Stochastic Hopf bifurcations in vacuum optical tweezers. Phys. Rev. A 2021, 104, 043518. [Google Scholar] [CrossRef]
- Horsthemke, W.; Lefever, R. Noise-Induced Transitions; Springer: Berlin, Germany, 1984; p. 338. [Google Scholar]
- Bashkirtseva, I.; Chen, G.; Ryashko, L. Analysis of noise-induced transitions from regular to chaotic oscillations in the Chen system. Chaos 2012, 22, 033104. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Coti Zelati, M.; Hairer, M. A noise-induced transition in the Lorenz system. Commun. Math. Phys. 2021, 383, 2243–2274. [Google Scholar] [CrossRef]
- Anishchenko, V.S.; Astakhov, V.V.; Neiman, A.B.; Vadivasova, T.E.; Schimansky-Geier, L. Nonlinear Dynamics of Chaotic and Stochastic Systems. Tutorial and Modern Development; Springer: Berlin/Heidelberg, Germany, 2007; p. 535. [Google Scholar]
- McDonnell, M.D.; Stocks, N.G.; Pearce, C.E.M.; Abbott, D. Stochastic Resonance: From Suprathreshold Stochastic Resonance to Stochastic Signal Quantization; Cambridge University Press: Cambridge, UK, 2008; p. 446. [Google Scholar]
- Pikovsky, A.S.; Kurths, J. Coherence resonance in a noise-driven excitable system. Phys. Rev. Lett. 1997, 78, 775–778. [Google Scholar] [CrossRef] [Green Version]
- Bashkirtseva, I.; Ryashko, L. How additive noise generates a phantom attractor in a model with cubic nonlinearity. Phys. Lett. A 2016, 380, 3359–3365. [Google Scholar] [CrossRef]
- Ryashko, L.; Alexandrov, D.; Bashkirtseva, I. Analysis of stochastic generation and shifts of phantom attractors in a climate–vegetation dynamical model. Mathematics 2021, 9, 1329. [Google Scholar] [CrossRef]
- Lindner, B.; Garcia-Ojalvo, J.; Neiman, A.; Schimansky-Geier, L. Effects of noise in excitable systems. Phys. Rep. 2004, 392, 321–424. [Google Scholar] [CrossRef]
- Orcioni, S.; Paffi, A.; Apollonio, F.; Liberti, M. Revealing spectrum features of stochastic neuron spike trains. Mathematics 2020, 8, 1011. [Google Scholar] [CrossRef]
- Lima Dias Pinto, I.; Copelli, M. Oscillations and collective excitability in a model of stochastic neurons under excitatory and inhibitory coupling. Phys. Rev. E 2019, 100, 062416. [Google Scholar] [CrossRef]
- Gao, J.B.; Hwang, S.K.; Liu, J.M. When can noise induce chaos? Phys. Rev. Lett. 1999, 82, 1132–1135. [Google Scholar] [CrossRef] [Green Version]
- Lai, Y.C.; Tel, T. Transient Chaos. Complex Dynamics on Finite Time Scales; Springer: New York, NY, USA, 2011; p. 502. [Google Scholar]
- Bashkirtseva, I.; Ryashko, L.; Slepukhina, E. Noise-induced oscillation bistability and transition to chaos in FitzHugh-Nagumo model. Fluct. Noise Lett. 2014, 13, 1450004. [Google Scholar] [CrossRef]
- Freidlin, M.I.; Wentzell, A.D. Random Perturbations of Dynamical Systems; Springer: New York, NY, USA; Berlin, Germany, 1984. [Google Scholar]
- Gardiner, C.W. Handbook of Stochastic Methods for Physics, Chemistry and the Natural Sciences; Springer: Berlin, Germany, 1983. [Google Scholar]
- Dembo, M.; Zeitouni, O. Large Deviations Techniques and Applications; Jones and Bartlett Publishers: Boston, MA, USA, 1995; p. 396. [Google Scholar]
- Bashkirtseva, I.; Ryashko, L. Sensitivity analysis of stochastically forced Lorenz model cycles under period doubling bifurcations. Dyn. Syst. Appl. 2002, 11, 293–310. [Google Scholar]
- Bashkirtseva, I.; Chen, G.; Ryashko, L. Analysis of stochastic cycles in the Chen system. Int. J. Bifurc. Chaos 2010, 20, 1439–1450. [Google Scholar] [CrossRef]
- Danylenko, V.; Skurativskyi, S. Stationary and periodic regimes in relaxing media with fluctuations. Eur. Phys. J. B 2014, 87, 218. [Google Scholar] [CrossRef]
- Sun, Y.; Hong, L.; Jiang, J. Stochastic sensitivity analysis of nonautonomous nonlinear systems subjected to Poisson white noise. Chaos Solitons Fractals 2017, 104, 508–515. [Google Scholar] [CrossRef]
- Xu, C.; Yuan, S.; Zhang, T. Confidence domain in the stochastic competition chemostat model with feedback control. Appl. Math. J. Chin. Univ. 2018, 33, 379–389. [Google Scholar] [CrossRef]
- Xu, C.; Yuan, S.; Zhang, T. Sensitivity analysis and feedback control of noise-induced extinction for competition chemostat model with mutualism. Phys. A Stat. Mech. Its Appl. 2018, 505, 891–902. [Google Scholar] [CrossRef]
- Das, P.; Mukherjee, S.; Das, P.; Banerjee, S. Characterizing chaos and multifractality in noise-assisted tumor-immune interplay. Nonlinear Dyn. 2020, 101, 675–685. [Google Scholar] [CrossRef]
- Alexandrov, D.V.; Bashkirtseva, I.A.; Crucifix, M.; Ryashko, L.B. Nonlinear climate dynamics: From deterministic behaviour to stochastic excitability and chaos. Phys. Rep. 2021, 902, 1–60. [Google Scholar] [CrossRef]
- Mil’shtein, G.; Ryashko, L. A first approximation of the quasipotential in problems of the stability of systems with random non-degenerate perturbations. J. Appl. Math. Mech. 1995, 59, 47–56. [Google Scholar] [CrossRef]
- García-Vellisca, M.A.; Pisarchik, A.N.; Jaimes-Reátegui, R. Experimental evidence of deterministic coherence resonance in coupled chaotic systems with frequency mismatch. Phys. Rev. E 2016, 94, 012218. [Google Scholar] [CrossRef]
- Jaimes-Reátegui, R.; García-López, J.; Gallegos, A.; Huerta Cuellar, G.; Chholak, P.; Pisarchik, A. Deterministic coherence and anti-coherence resonances in networks of chaotic oscillators with frequency mismatch. Chaos Solitons Fractals 2021, 152, 111424. [Google Scholar] [CrossRef]
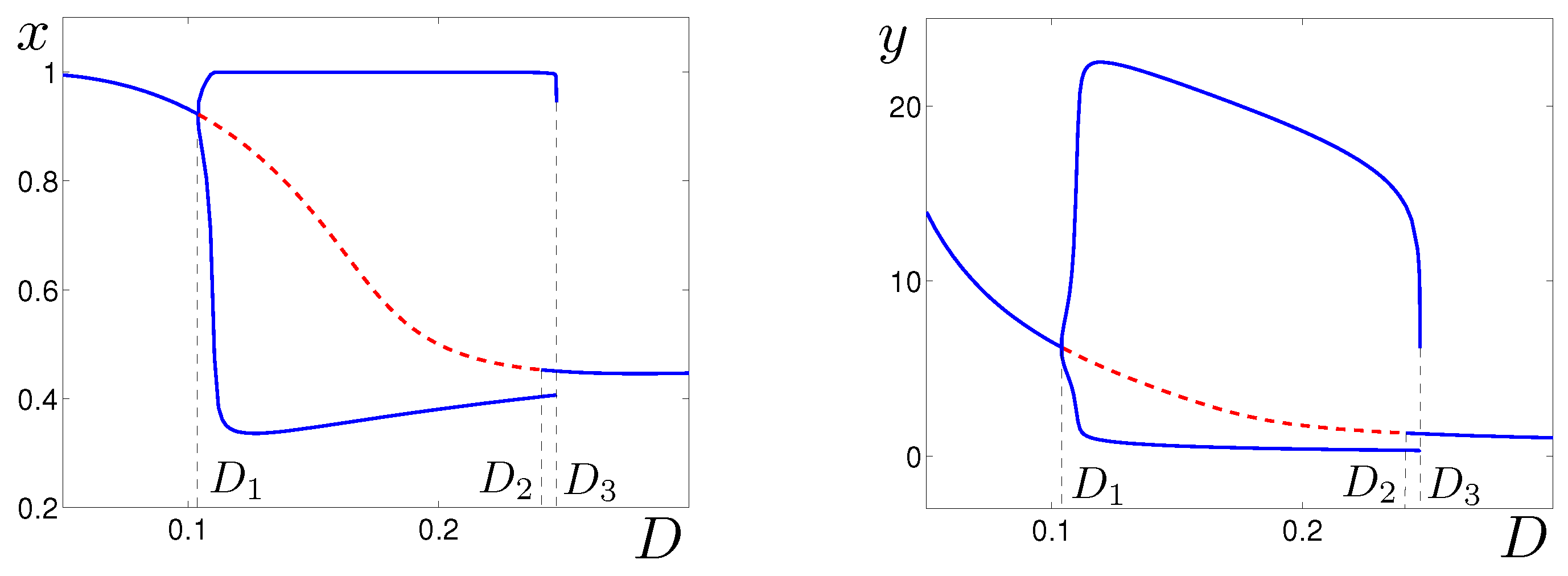
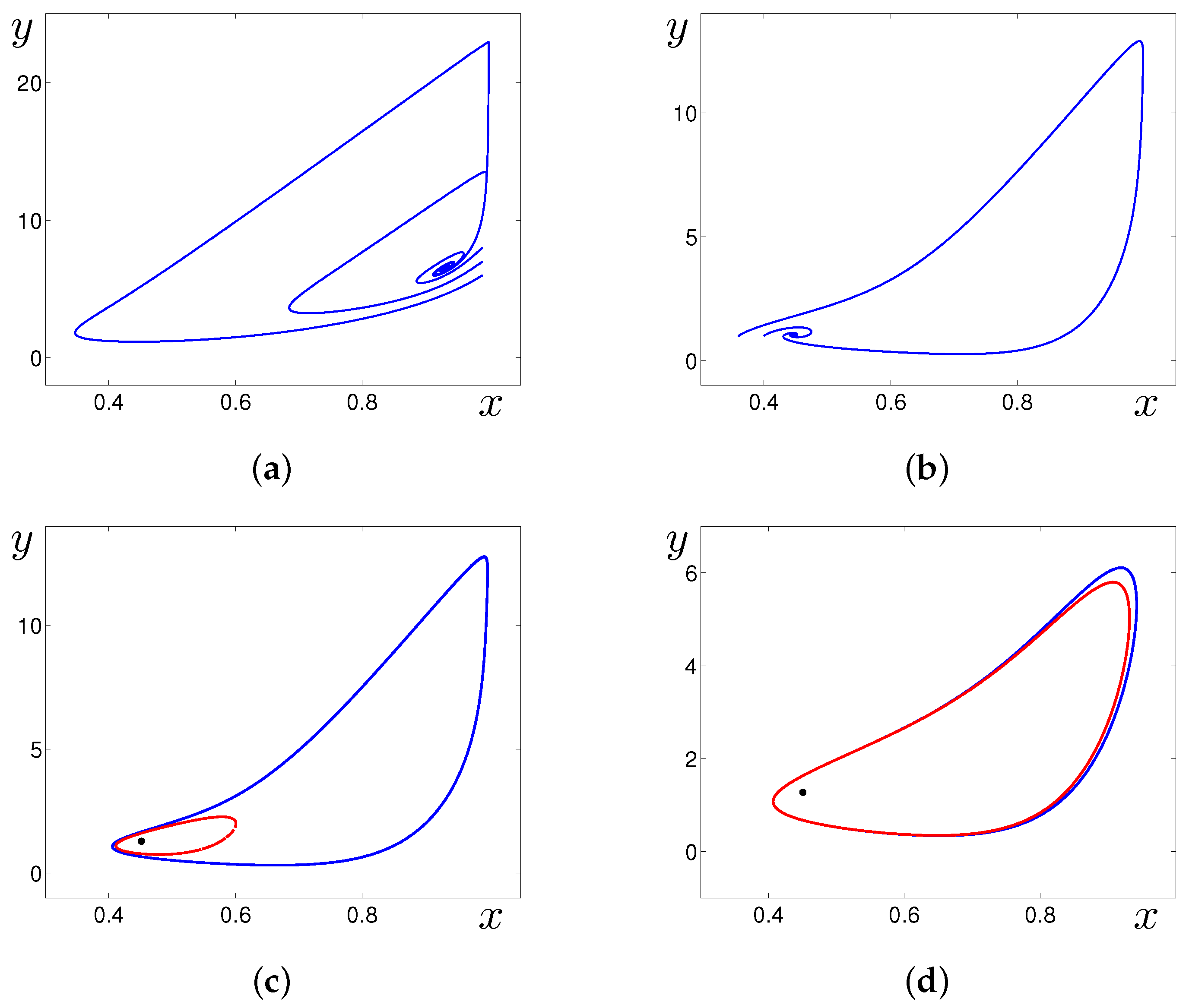
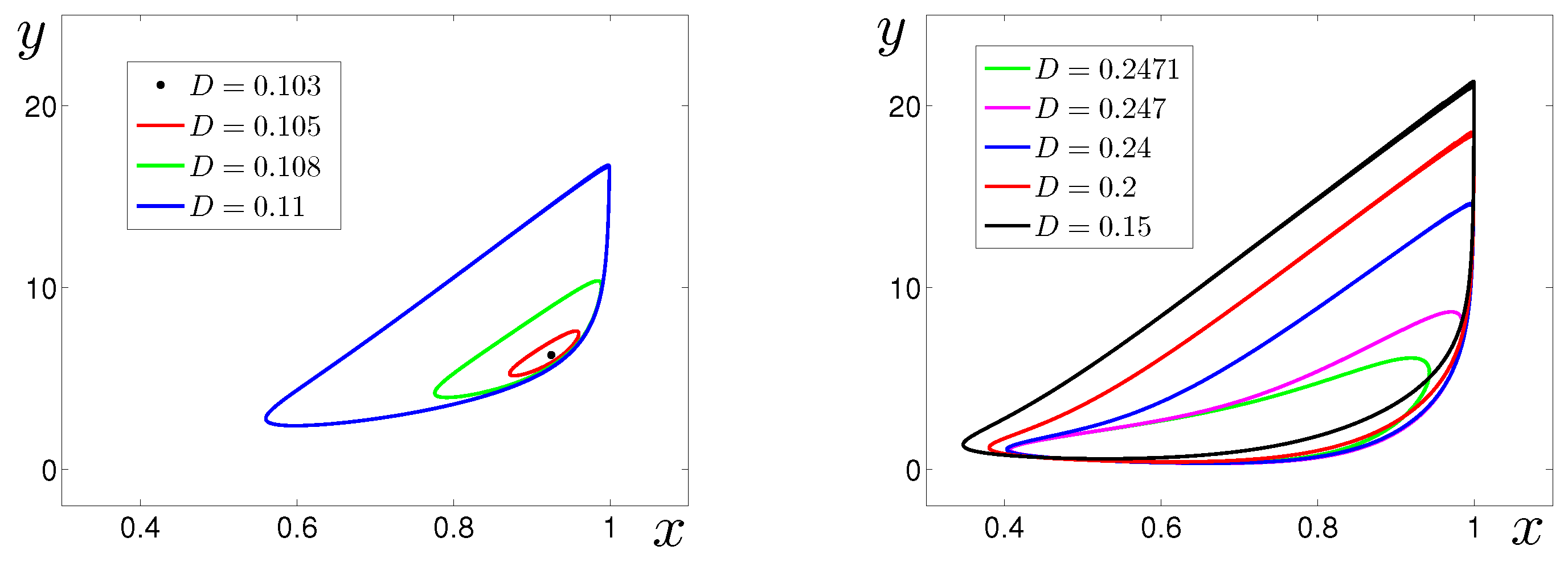


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ryashko, L.; Bashkirtseva, I. Stochastic Bifurcations and Excitement in the ZS-Model of a Thermochemical Reaction. Mathematics 2022, 10, 960. https://doi.org/10.3390/math10060960
Ryashko L, Bashkirtseva I. Stochastic Bifurcations and Excitement in the ZS-Model of a Thermochemical Reaction. Mathematics. 2022; 10(6):960. https://doi.org/10.3390/math10060960
Chicago/Turabian StyleRyashko, Lev, and Irina Bashkirtseva. 2022. "Stochastic Bifurcations and Excitement in the ZS-Model of a Thermochemical Reaction" Mathematics 10, no. 6: 960. https://doi.org/10.3390/math10060960
APA StyleRyashko, L., & Bashkirtseva, I. (2022). Stochastic Bifurcations and Excitement in the ZS-Model of a Thermochemical Reaction. Mathematics, 10(6), 960. https://doi.org/10.3390/math10060960






