Optimal Constant-Stress Accelerated Life Test Plans for One-Shot Devices with Components Having Exponential Lifetimes under Gamma Frailty Models
Abstract
:1. Introduction
2. Model Description
3. Optimal CSALT Plans
3.1. Asymptotic Variance
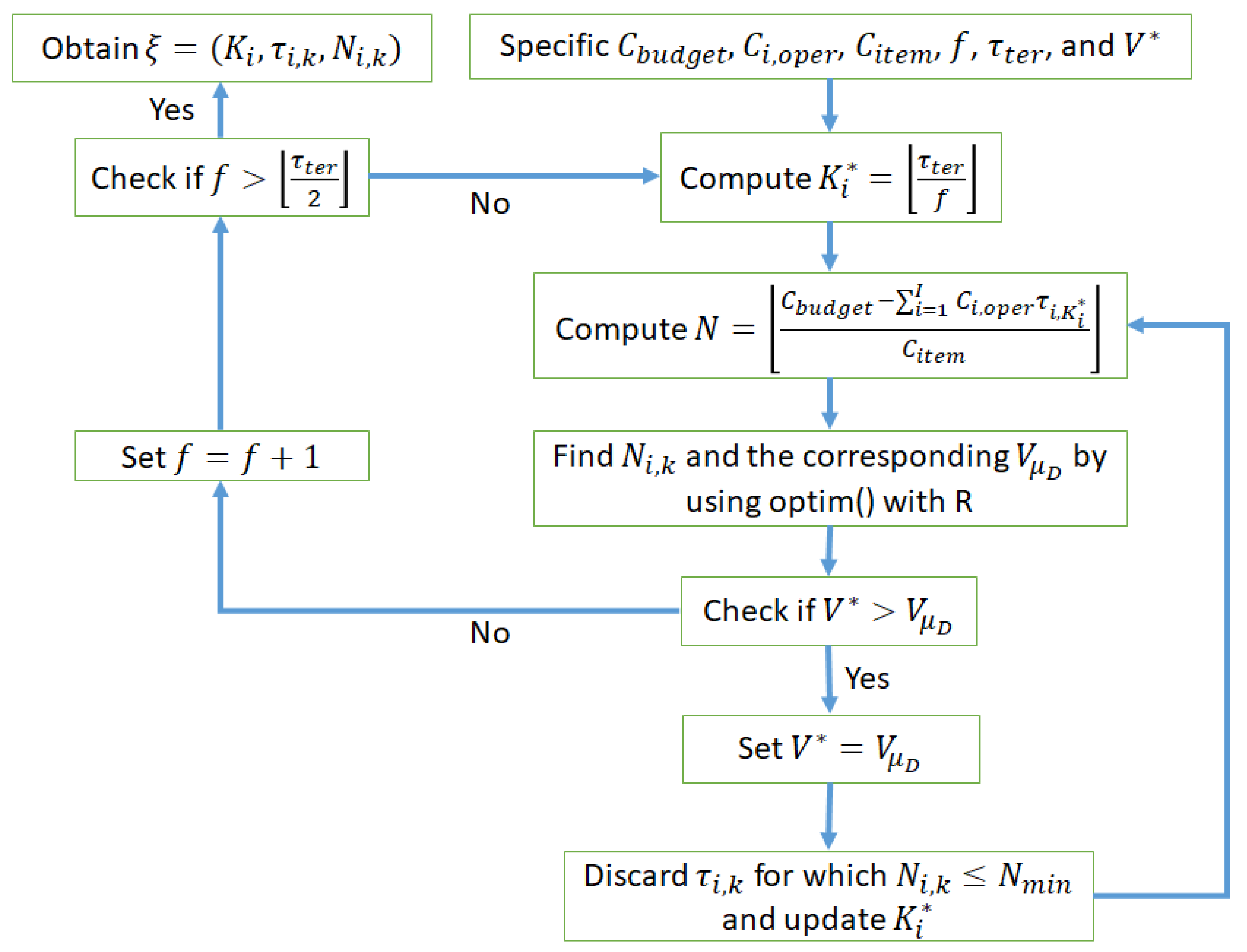
3.2. Procedure of Obtaining the Optimal CSALT Plan
4. Simulation Study
5. Eye Data from Diabetic Retinopathy Study
6. Concluding Remarks
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| ALT | accelerated life test |
| CSALT | constant-stress ALT |
| MLEs | maximum likelihood estimates |
| probability density function | |
| MSE | mean square error |
References
- Morris, M.D. A sequential experimental design for estimating a scale parameter from quantal life testing data. Technometrics 1991, 29, 173–181. [Google Scholar] [CrossRef]
- Zhang, X.P.; Shang, J.Z.; Chen, X.; Zhang, C.H.; Wang, Y.S. Statistical inference of accelerated life testing with dependent competing failures based on copula theory. IEEE Trans. Reliab. 2014, 63, 764–780. [Google Scholar] [CrossRef]
- Zheng, J.; Li, Y.; Wang, J.; Shiju, E.; Li, X. Accelerated thermal aging of grease-based magnetorheological fluids and their lifetime prediction. Mater. Res. Express 2018, 5, 085702. [Google Scholar] [CrossRef]
- Balakrishnan, N.; Ling, M.H.; So, H.Y. Accelerated Life Testing of One-Shot Devices: Data Collection and Analysis; John Wiley & Sons: Hoboken, NJ, USA, 2021. [Google Scholar]
- Balakrishnan, N.; Castilla, E.; Martín, N.; Pardo, L. Robust inference for one-shot device testing data under Weibull lifetime model. IEEE Trans. Reliab. 2019, 69, 937–953. [Google Scholar] [CrossRef]
- Balakrishnan, N.; Castilla, E.; Martín, N.; Pardo, L. Robust inference for one-shot device testing data under exponential lifetime model with multiple stresses. Qual. Reliab. Eng. Int. 2020, 36, 1916–1930. [Google Scholar] [CrossRef]
- Ling, M.H.; Hu, X.W. Optimal design of simple step-stress accelerated life tests for one-shot devices under Weibull distributions. Reliab. Eng. Syst. Saf. 2020, 193, 106630. [Google Scholar] [CrossRef]
- Balakrishnan, N.; Castilla, E.; Martín, N.; Pardo, L. Divergence-based robust inference under proportional hazards model for one-shot device life-test. IEEE Trans. Reliab. 2021, 70, 1355–1367. [Google Scholar] [CrossRef]
- Balakrishnan, N.; Castilla, E.; Ling, M.H. Optimal designs of constant-stress accelerated life-tests for one-shot devices with model misspecification analysis. Qual. Reliab. Eng. Int. 2021, in press. [CrossRef]
- Balakrishnan, N.; Castilla, E. EM-based likelihood inference for one-shot device test data under log-normal lifetimes and the optimal design of a CSALT plan. Qual. Reliab. Eng. Int. 2021, in press. [CrossRef]
- Zhu, X.; Liu, K.; He, M.; Balakrishnan, N. Reliability estimation for one-shot devices under cyclic accelerated life-testing. Reliab. Eng. Syst. Safety 2021, 212, 107595. [Google Scholar] [CrossRef]
- Zhu, X.; Balakrishnan, N. One-shot device test data analysis using non-parametric and semi-parametric inferential methods and applications. Reliab. Eng. Syst. Saf. 2022, in press. [CrossRef]
- Wang, Y.; Pham, H. Modeling the dependent competing risks with multiple degradation processes and random shock using time-varying copulas. IEEE Trans. Reliab. 2012, 61, 13–22. [Google Scholar] [CrossRef]
- Pan, Z.; Balakrishnan, N.; Sun, Q.; Zhou, J. Bivariate degradation analysis of products based on Wiener processes and copulas. J. Stat. Comput. Simul. 2013, 83, 1316–1329. [Google Scholar] [CrossRef]
- Peng, W.; Li, Y.F.; Yang, Y.J.; Zhu, S.P.; Huang, H.Z. Bivariate analysis of incomplete degradation observations based on inverse Gaussian processes and copulas. IEEE Trans. Reliab. 2016, 65, 624–639. [Google Scholar] [CrossRef]
- Wang, Y.C.; Emura, T.; Fan, T.H.; Lo, S.M.; Wilke, R.A. Likelihood-based inference for a frailty-copula model based on competing risks failure time data. Qual. Reliab. Eng. Int. 2020, 36, 1622–1638. [Google Scholar] [CrossRef]
- Liu, X. Planning of accelerated life tests with dependent failure modes based on a gamma frailty model. Technometrics 2012, 54, 398–409. [Google Scholar] [CrossRef]
- Ling, M.H.; Ng, H.K.T.; Chan, P.S.; Balakrishnan, N. Autopsy data analysis for a series system with active redundancy under a load-sharing model. IEEE Trans. Reliab. 2016, 65, 957–968. [Google Scholar] [CrossRef]
- Asha, G.; Raja, A.V.; Ravishanker, N. Reliability modelling incorporating load share and frailty. Appl. Stoch. Model. Bus. Ind. 2018, 34, 206–223. [Google Scholar] [CrossRef]
- Li, Y.; Coolen, F.P.; Zhu, C.; Tan, J. Reliability assessment of the hydraulic system of wind turbines based on load-sharing using survival signature. Renew. Energy 2020, 153, 766–776. [Google Scholar] [CrossRef]
- Meeker, W.Q.; Hahn, G.J. A comparison of accelerated test plans to estimate the survival probability at a design stress. Technometrics 1978, 20, 245–247. [Google Scholar] [CrossRef]
- Meeker, W.Q. A comparison of accelerated life test plans for Weibull and lognormal distributions and type I censoring. Technometrics 1984, 26, 157–171. [Google Scholar] [CrossRef]
- Escobar, L.A.; Meeker, W.Q. Planning accelerated life tests with two or more experimental factors. Technometrics 1995, 37, 411–427. [Google Scholar] [CrossRef]
- Han, D.; Ng, H.K.T. Comparison between constant-stress and step-stress accelerated life tests under time constraint. Nav. Res. Logist. 2013, 60, 541–556. [Google Scholar] [CrossRef]
- Wu, S.J.; Huang, S.R. Planning two or more level constant-stress accelerated life tests with competing risks. Reliab. Eng. Syst. Saf. 2017, 158, 1–8. [Google Scholar] [CrossRef]
- Han, D. Optimal design of a simple step-stress accelerated life test under progressive type I censoring with nonuniform durations for exponential lifetimes. Qual. Reliab. Eng. Int. 2019, 35, 1297–1312. [Google Scholar] [CrossRef]
- Fang, G.; Pan, R.; Stufken, J. Optimal setting of test conditions and allocation of test units for accelerated degradation tests with two stress variables. IEEE Trans. Reliab. 2020, 70, 1096–1111. [Google Scholar] [CrossRef]
- Han, D. On the existence of the optimal step-stress accelerated life tests under progressive Type-I censoring. IEEE Trans. Reliab. 2019, 69, 903–915. [Google Scholar] [CrossRef]
- Balakrishnan, N.; So, H.Y.; Ling, M.H. EM algorithm for one-shot device testing with competing risks under exponential distribution. Reliab. Eng. Syst. Saf. 2015, 137, 129–140. [Google Scholar] [CrossRef]
- Balakrishnan, N.; So, H.Y.; Ling, M.H. EM algorithm for one-shot device testing with competing risks under Weibull distribution. IEEE Trans. Reliab. 2016, 65, 973–991. [Google Scholar] [CrossRef]
- Balakrishnan, N.; Ling, M.H. Expectation maximization algorithm for one shot device accelerated life testing with Weibull lifetimes, and variable parameters over stress. IEEE Trans. Reliab. 2013, 62, 537–551. [Google Scholar] [CrossRef]
- Ling, M.H.; Chan, P.S.; Ng, H.K.T.; Balakrishnan, N. Copula models for one-shot device testing data with correlated failure modes. Commun. Stat.-Theory Methods 2021, 50, 3875–3888. [Google Scholar] [CrossRef]
- Ling, M.H.; Balakrishnan, N.; Yu, C.; So, H.Y. Inference for One-Shot Devices with Dependent k-Out-of-M Structured Components under Gamma Frailty. Mathematics 2021, 9, 3032. [Google Scholar] [CrossRef]
- Wang, W.; Kececioglu, D.B. Fitting the Weibull log-linear model to accelerated life-test data. IEEE Trans. Reliab. 2000, 49, 217–223. [Google Scholar] [CrossRef]
- Nayak, T.K. Multivariate Lomax distribution: Properties and usefulness in reliability theory. J. Appl. Probab. 1987, 24, 170–177. [Google Scholar] [CrossRef]
- Johnson, N.L.; Kotz, S.; Balakrishnan, N. Continuous Univariate Distributions—Volume 1, 2nd ed.; John Wiley & Sons: New York, NY, USA, 1994. [Google Scholar]
- Sakai, S.I.; Yoshida, H.; Hiratsuka, J.; Vandecasteele, C.; Kohlmeyer, R.; Rotter, V.S.; Yano, J. An international comparative study of end-of-life vehicle (ELV) recycling systems. J. Mater. Cycles Waste Manag. 2014, 16, 1–20. [Google Scholar] [CrossRef] [Green Version]
- Sahu, S.K.; Dey, D.K. A comparison of frailty and other models for bivariate survival data. Lifetime Data Anal. 2000, 6, 207–228. [Google Scholar] [CrossRef]
- Emura, T.; Sofeu, C.L.; Rondeau, V. Conditional copula models for correlated survival endpoints: Individual patient data meta-analysis of randomized controlled trials. Stat. Methods Med Res. 2021, 30, 2634–2650. [Google Scholar] [CrossRef]
| Setting | Optimal CSALT | Total Cost | ||||||
|---|---|---|---|---|---|---|---|---|
| V | MSE | |||||||
| 0.3 | 500K | 60 | (1,0,1) | (60, 0, 24) | (325, 0, 119) | 499.2K | 0.015 | 0.017 |
| 0.2 | 500K | 60 | (1,0,1) | (60, 0, 24) | (329, 0, 115) | 499.2K | 0.011 | 0.011 |
| 0.1 | 500K | 60 | (1,0,1) | (60, 0, 24) | (332, 0, 112) | 499.2K | 0.008 | 0.008 |
| 0.01 | 500K | 60 | (1,0,1) | (60, 0, 24) | (335, 0, 109) | 499.2K | 0.006 | 0.006 |
| 0.3 | 500K | 30 | (1,0,1) | (30, 0, 30) | (277, 0, 169) | 499.6K | 0.023 | 0.025 |
| 0.2 | 500K | 30 | (1,0,1) | (30, 0, 30) | (297, 0, 149) | 499.6K | 0.017 | 0.017 |
| 0.1 | 500K | 30 | (1,0,1) | (30, 0, 30) | (313, 0, 133) | 499.6K | 0.013 | 0.013 |
| 0.01 | 500K | 30 | (1,0,1) | (30, 0, 30) | (325, 0, 121) | 499.6K | 0.010 | 0.010 |
| 0.3 | 200K | 60 | (1,0,1) | (60, 0, 24) | (125, 0, 47) | 200K | 0.039 | 0.039 |
| 0.2 | 200K | 60 | (1,0,1) | (60, 0, 24) | (127, 0, 45) | 200K | 0.028 | 0.029 |
| 0.1 | 200K | 60 | (1,0,1) | (60, 0, 20) | (128, 0, 44) | 199.2K | 0.021 | 0.022 |
| 0.01 | 200K | 60 | (1,0,1) | (60, 0, 24) | (129, 0, 43) | 200K | 0.016 | 0.015 |
| 0.3 | 200K | 30 | (1,0,1) | (30, 0, 30) | (107, 0, 66) | 199.3K | 0.060 | 0.060 |
| 0.2 | 200K | 30 | (1,0,1) | (30, 0, 30) | (115, 0, 58) | 199.3K | 0.045 | 0.047 |
| 0.1 | 200K | 30 | (1,0,1) | (30, 0, 30) | (121, 0, 52) | 199.3K | 0.034 | 0.034 |
| 0.01 | 200K | 30 | (1,0,1) | (30, 0, 30) | (126, 0, 47) | 199.3K | 0.027 | 0.024 |
| 0.3 | 100K | 60 | (1,0,1) | (60, 0, 24) | (59, 0, 22) | 99.9K | 0.083 | 0.078 |
| 0.2 | 100K | 60 | (1,0,1) | (60, 0, 24) | (60, 0, 21) | 99.9K | 0.059 | 0.066 |
| 0.1 | 100K | 60 | (1,0,1) | (60, 0, 20) | (60, 0, 21) | 99.1K | 0.044 | 0.052 |
| 0.01 | 100K | 60 | (1,0,1) | (60, 0, 24) | (61, 0, 20) | 99.9K | 0.033 | 0.037 |
| 0.3 | 100K | 30 | (1,0,1) | (30, 0, 24) | (52, 0, 31) | 99.1K | 0.127 | 0.113 |
| 0.2 | 100K | 30 | (1,0,1) | (30, 0, 30) | (54, 0, 28) | 99.2K | 0.094 | 0.089 |
| 0.1 | 100K | 30 | (1,0,1) | (30, 0, 30) | (57, 0, 25) | 99.2K | 0.072 | 0.074 |
| 0.01 | 100K | 30 | (1,0,1) | (30, 0, 30) | (59, 0, 23) | 99.2K | 0.057 | 0.055 |
| Setting | Optimal CSALT | Revenue | |||||||
|---|---|---|---|---|---|---|---|---|---|
| 0.3 | 500K | 60 | (1,0,1) | (60, 0, 24) | (325, 0, 119) | 29,995 | 30,114 | 1216 | 1279 |
| 0.2 | 500K | 60 | (1,0,1) | (60, 0, 24) | (329, 0, 115) | 30,873 | 30,937 | 1197 | 1173 |
| 0.1 | 500K | 60 | (1,0,1) | (60, 0, 24) | (332, 0, 112) | 31,544 | 31,504 | 1172 | 1112 |
| 0.01 | 500K | 60 | (1,0,1) | (60, 0, 24) | (335, 0, 109) | 32,493 | 32,462 | 1146 | 1176 |
| 0.3 | 500K | 30 | (1,0,1) | (30, 0, 30) | (277, 0, 169) | 28,817 | 28,854 | 1287 | 1297 |
| 0.2 | 500K | 30 | (1,0,1) | (30, 0, 30) | (297, 0, 149) | 29,815 | 29,777 | 1284 | 1271 |
| 0.1 | 500K | 30 | (1,0,1) | (30, 0, 30) | (313, 0, 133) | 30,863 | 30,801 | 1277 | 1265 |
| 0.01 | 500K | 30 | (1,0,1) | (30, 0, 30) | (325, 0, 121) | 31,848 | 31,846 | 1266 | 1251 |
| 0.3 | 200K | 60 | (1,0,1) | (60, 0, 24) | (125, 0, 47) | 11,618 | 11,641 | 757 | 728 |
| 0.2 | 200K | 60 | (1,0,1) | (60, 0, 24) | (127, 0, 45) | 11,958 | 11,918 | 745 | 755 |
| 0.1 | 200K | 60 | (1,0,1) | (60, 0, 20) | (128, 0, 44) | 12,601 | 12,560 | 734 | 715 |
| 0.01 | 200K | 60 | (1,0,1) | (60, 0, 24) | (129, 0, 43) | 12,583 | 12,565 | 713 | 714 |
| 0.3 | 200K | 30 | (1,0,1) | (30, 0, 30) | (107, 0, 66) | 11,173 | 11,176 | 801 | 834 |
| 0.2 | 200K | 30 | (1,0,1) | (30, 0, 30) | (115, 0, 58) | 11,563 | 11,586 | 799 | 799 |
| 0.1 | 200K | 30 | (1,0,1) | (30, 0, 30) | (121, 0, 52) | 11,965 | 11,986 | 795 | 807 |
| 0.01 | 200K | 30 | (1,0,1) | (30, 0, 30) | (126, 0, 47) | 12,352 | 12,393 | 789 | 809 |
| 0.3 | 100K | 60 | (1,0,1) | (60, 0, 24) | (59, 0, 22) | 5472 | 5471 | 519 | 539 |
| 0.2 | 100K | 60 | (1,0,1) | (60, 0, 24) | (60, 0, 21) | 5632 | 5629 | 511 | 510 |
| 0.1 | 100K | 60 | (1,0,1) | (60, 0, 20) | (60, 0, 21) | 5935 | 5936 | 504 | 514 |
| 0.01 | 100K | 60 | (1,0,1) | (60, 0, 24) | (61, 0, 20) | 5927 | 5915 | 490 | 507 |
| 0.3 | 100K | 30 | (1,0,1) | (30, 0, 24) | (52, 0, 31) | 5615 | 5573 | 560 | 572 |
| 0.2 | 100K | 30 | (1,0,1) | (30, 0, 30) | (54, 0, 28) | 5474 | 5500 | 550 | 551 |
| 0.1 | 100K | 30 | (1,0,1) | (30, 0, 30) | (57, 0, 25) | 5665 | 5657 | 547 | 534 |
| 0.01 | 100K | 30 | (1,0,1) | (30, 0, 30) | (59, 0, 23) | 5840 | 5870 | 542 | 559 |
| Juvenile | Adult | |||
|---|---|---|---|---|
| Mean age | 10.21 | 35.30 | ||
| Treated eye | Untreated eye | Treated eye | Untreated eye | |
| Mean time to blindness | 36.48 | 33.33 | 42.16 | 30.85 |
| Patient | (months) | K |
|---|---|---|
| Juvenile | 60 | 80 |
| Adult | 60 | 120 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ling, M.-H. Optimal Constant-Stress Accelerated Life Test Plans for One-Shot Devices with Components Having Exponential Lifetimes under Gamma Frailty Models. Mathematics 2022, 10, 840. https://doi.org/10.3390/math10050840
Ling M-H. Optimal Constant-Stress Accelerated Life Test Plans for One-Shot Devices with Components Having Exponential Lifetimes under Gamma Frailty Models. Mathematics. 2022; 10(5):840. https://doi.org/10.3390/math10050840
Chicago/Turabian StyleLing, Man-Ho. 2022. "Optimal Constant-Stress Accelerated Life Test Plans for One-Shot Devices with Components Having Exponential Lifetimes under Gamma Frailty Models" Mathematics 10, no. 5: 840. https://doi.org/10.3390/math10050840
APA StyleLing, M.-H. (2022). Optimal Constant-Stress Accelerated Life Test Plans for One-Shot Devices with Components Having Exponential Lifetimes under Gamma Frailty Models. Mathematics, 10(5), 840. https://doi.org/10.3390/math10050840






