A Combined Experimental-Numerical Investigation of the Thermal Efficiency of the Vessel in Domestic Induction Systems
Abstract
:1. Introduction
2. Materials & Methods
2.1. Experimental Set-Up
2.2. Model Description
2.2.1. Heat Transfer Model
2.2.2. Finite Element Model
2.2.3. Finite Element Method Discretization
2.3. Design of the Experiment
3. Results & Discussion
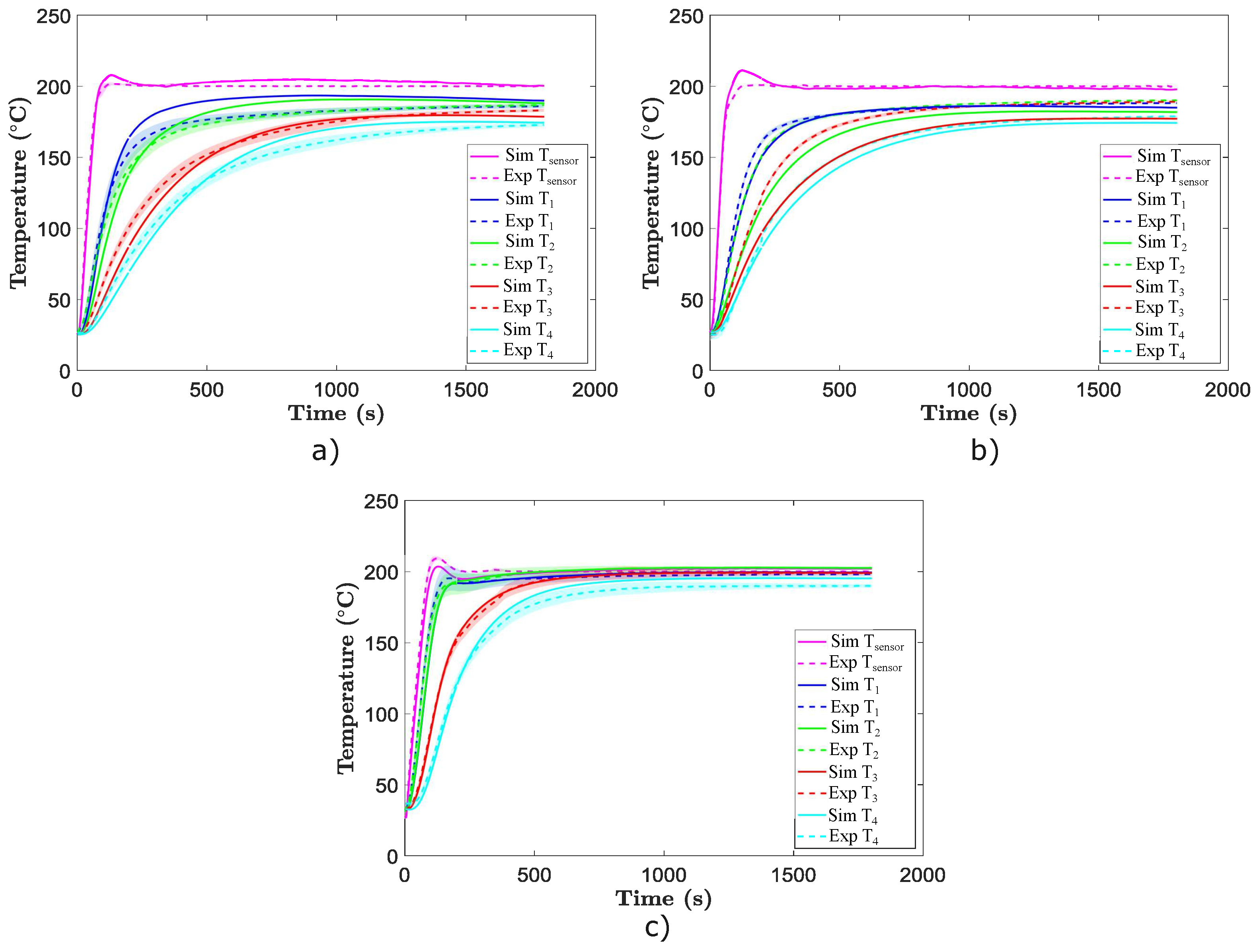
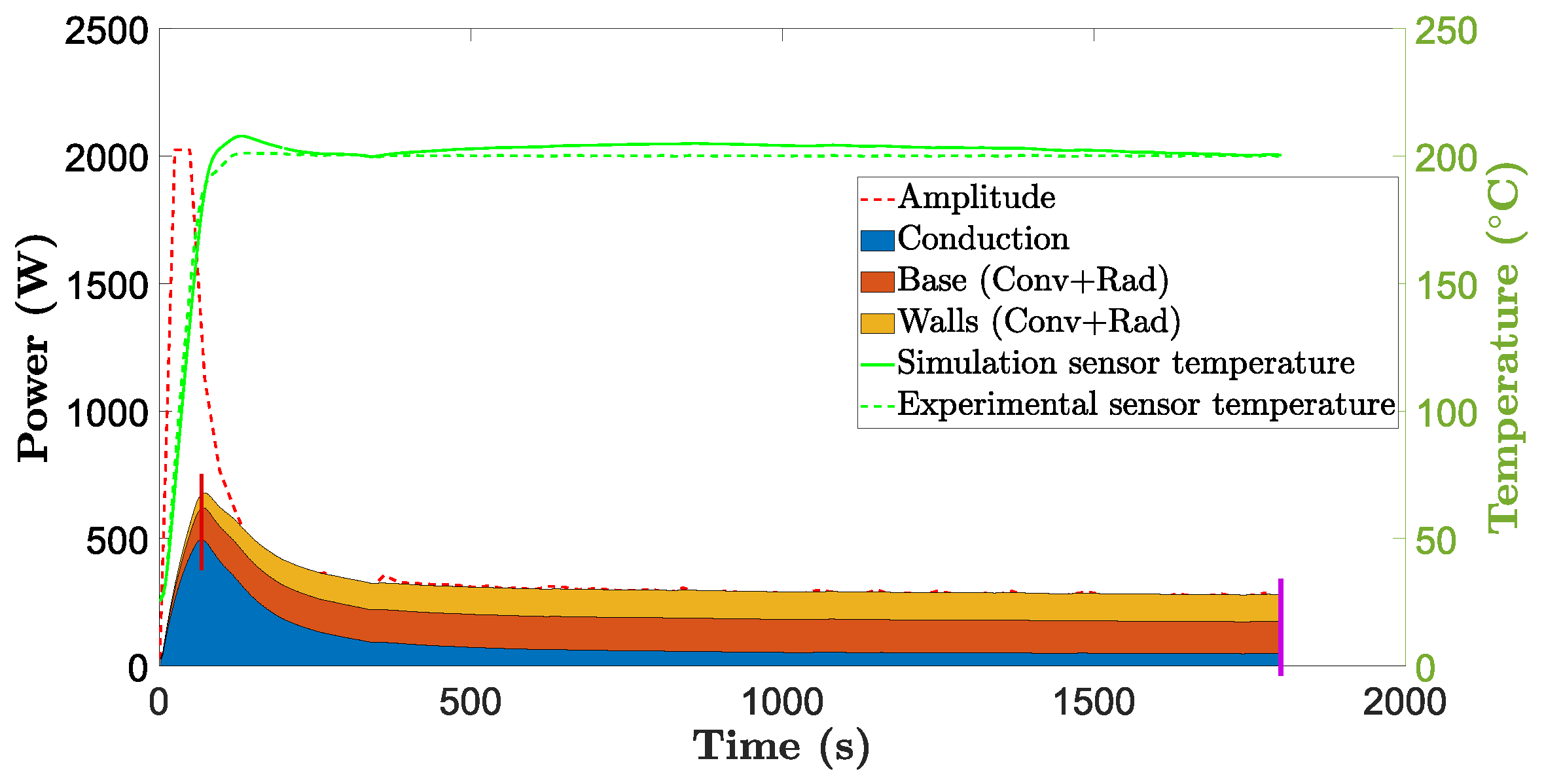
3.1. Experimental-Numerical Calibration of the Heat Loss Coefficients
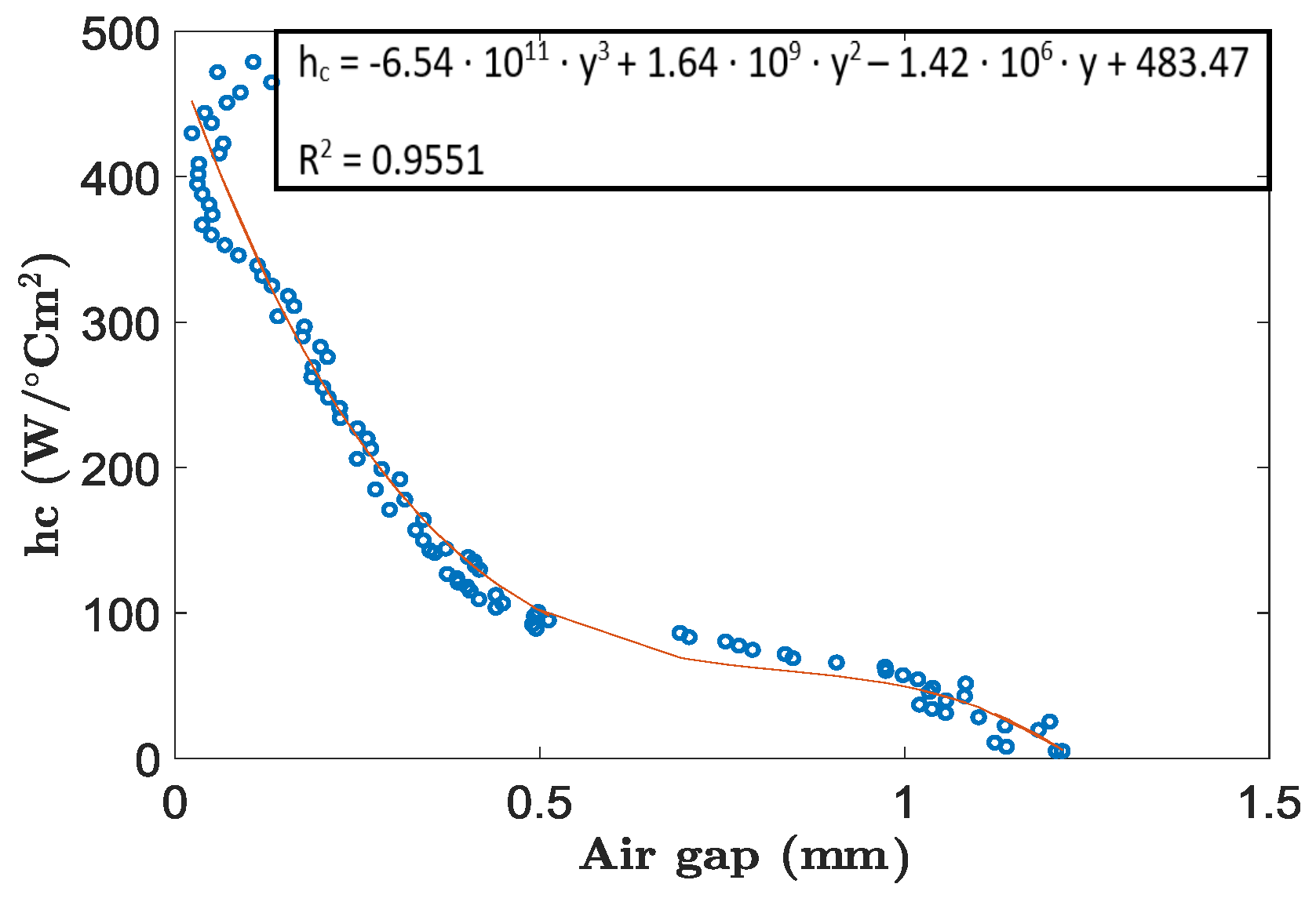
3.1.1. Model I: Modelling the Layer of Air between the Vessel and the Pan
3.1.2. Model II: Fitting of the Nonlinear Thermal Conductance along the Radius
3.1.3. Comparative between Model I and Model II
3.1.4. Heat Flux Analysis
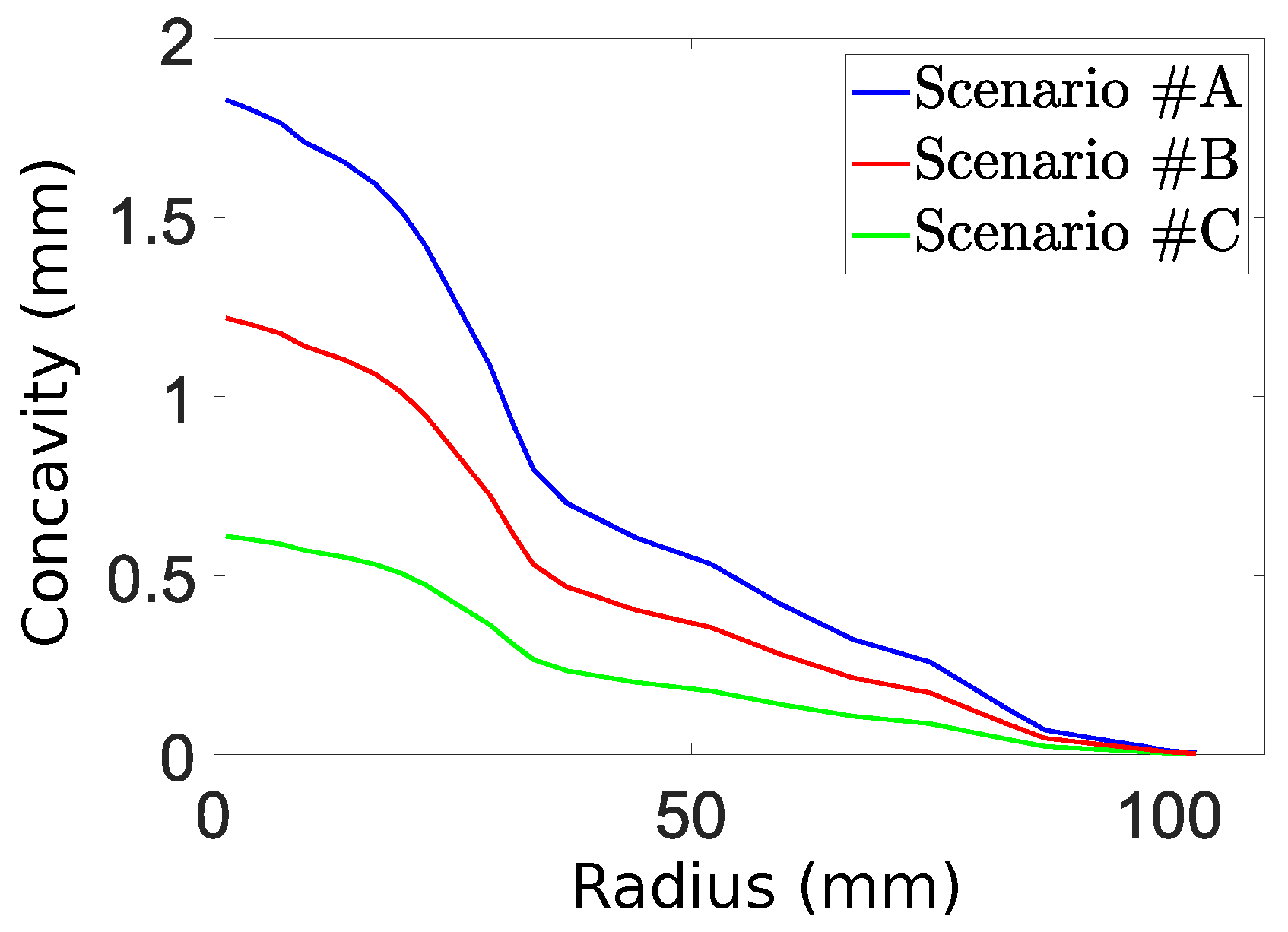
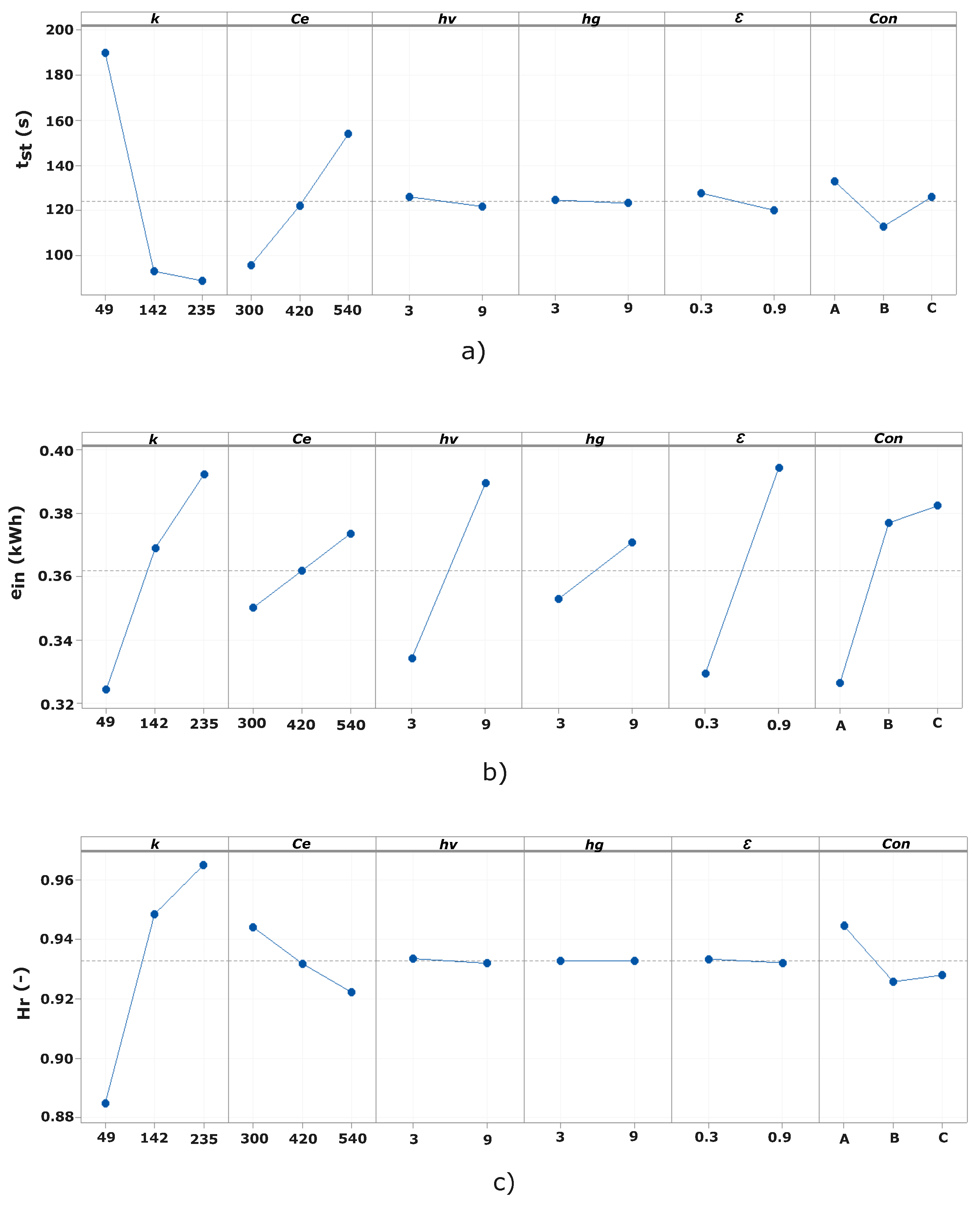
3.2. Design of Experiments (DoE)
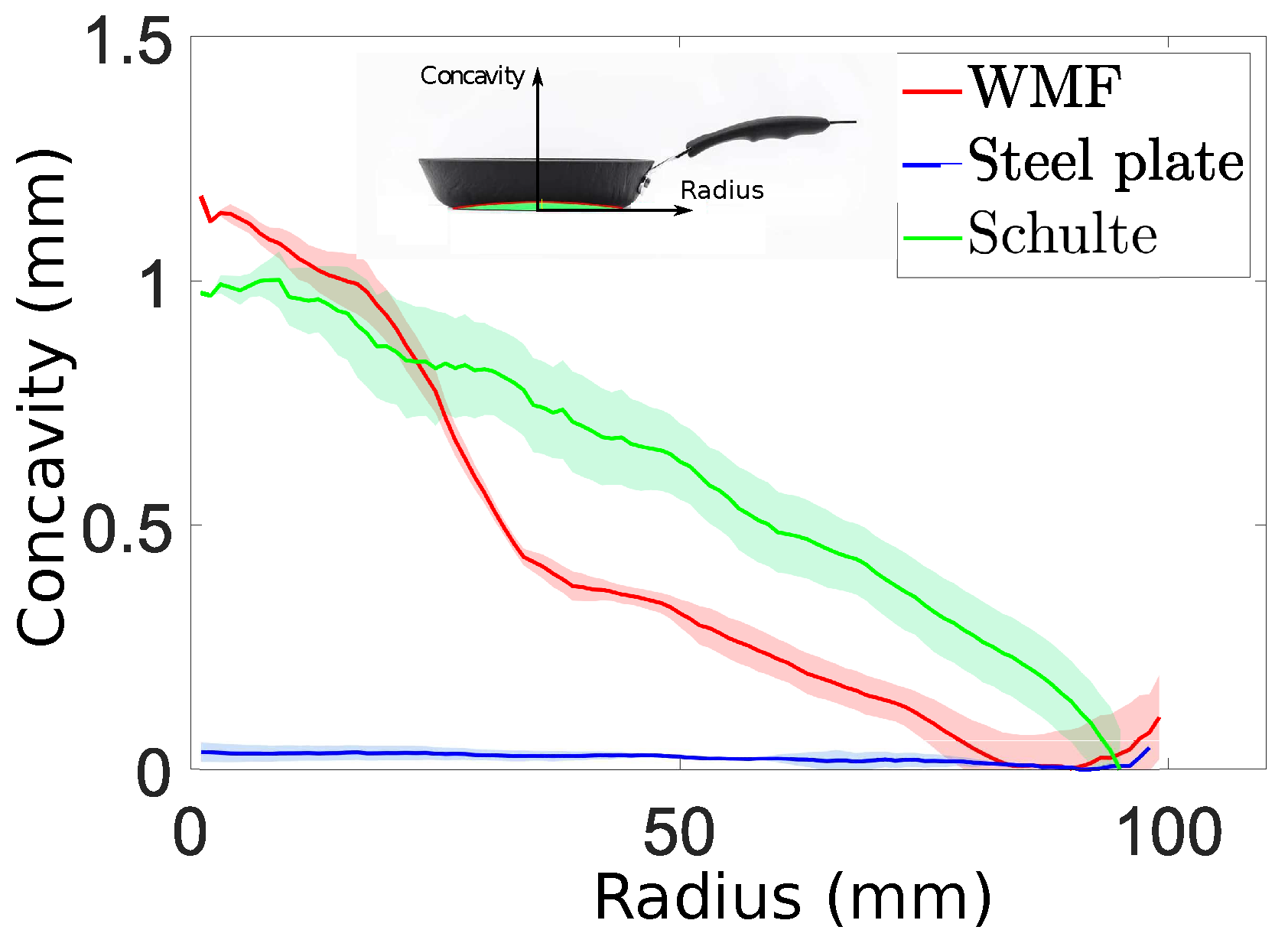
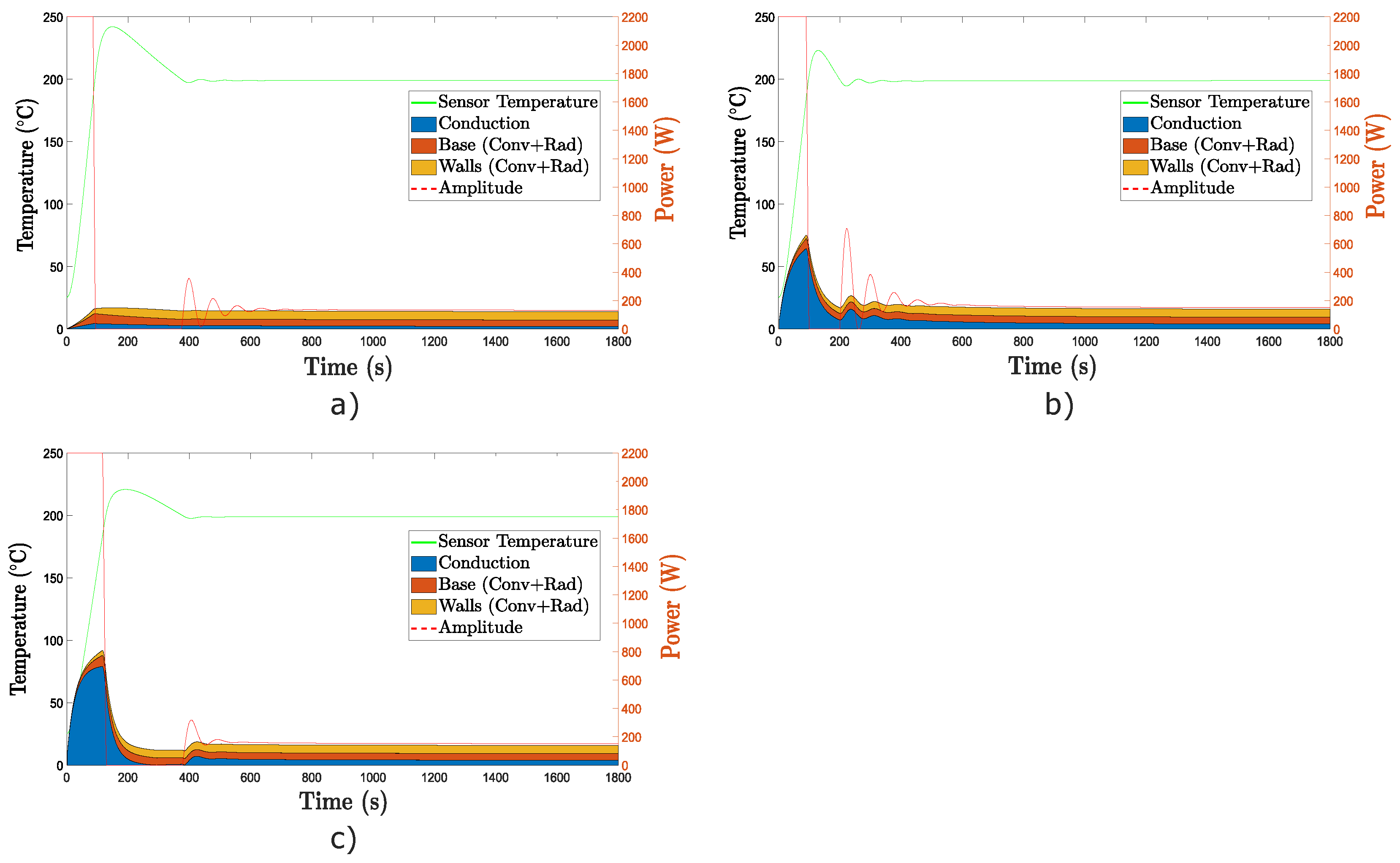
3.3. Influence of the Vessel Concavity
4. Conclusions
- The conductivity is the governing factor of the effect of the vessel in the cooking. When the vessel conductivity is high, it achieves the steady state and a better temperature homogeneity sooner. In contrast, the supplied energy is considerably higher. Thus, manufacturers should reach a compromise between being more energy effective and achieving a homogeneous temperature in the cooking surface in the shortest possible time.
- A more concave cooking vessel is more efficient, as the air layer between the pan base and the glass acts as insulation, reducing the heat losses of the vessel to the glass. The air gap should not be sufficiently large, as the magnetic field could lose efficiency.
- More than 80% of the heat losses during the transient state are due to the heat losses from the vessel to the glass.
- The main heat losses in the steady state are due to the convection and radiation.
- Both numerical approaches (Model I, including a layer of air and Model II, adding a variable thermal contact conductance) lead to similar results with MAEs lower than 5 K for the temperatures in the vessel and the glass. Model II adds the complexity of calibrating the variable thermal contact conductance.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Acero, J.; Burdio, J.; Barragan, L.; Navarro, D.; Alonso, R.; Ramon, J.; Monterde, F.; Hernandez, P.; Llorente, S.; Garde, I. Domestic Induction Appliances. IEEE Ind. Appl. Mag. 2010, 16, 39–47. [Google Scholar] [CrossRef]
- Borg, S.; Kelly, N. The effect of appliance energy efficiency improvements on domestic electric loads in European households. Energy Build. 2011, 43, 2240–2250. [Google Scholar] [CrossRef]
- Yohanis, Y.G. Domestic energy use and householders’ energy behaviour. Energy Policy 2012, 41, 654–665. [Google Scholar] [CrossRef]
- Villacís, S.; Martínez, J.; Riofrío, A.; Carrión, D.; Orozco, M.; Vaca, D. Energy Efficiency Analysis of Different Materials for Cookware Commonly Used in Induction Cookers. Energy Procedia 2015, 75, 925–930. [Google Scholar] [CrossRef] [Green Version]
- Cadavid, F.J.; Cadavid, Y.; Amell, A.A.; Arrieta, A.E.; Echavarría, J.D. Numerical and experimental methodology to measure the thermal efficiency of pots on electrical stoves. Energy 2014, 73, 258–263. [Google Scholar] [CrossRef]
- Hannani, S.; Hessari, E.; Fardadi, M.; Jeddi, M. Mathematical modeling of cooking pots’ thermal efficiency using a combined experimental and neural network method. Energy 2006, 31, 2969–2985. [Google Scholar] [CrossRef]
- Sedighi, M.; Dardashti, B. Finite element analysis of heat transfer in multi-layer cooking pots with emphasis on layer number. Int. J. Automot. Mech. Eng. 2015, 11, 2253–2261. [Google Scholar] [CrossRef]
- Ayata, T.; Çavuşoğlu, A.; Arcaklioğlu, E. Predictions of temperature distributions on layered metal plates using artificial neural networks. Energy Convers. Manag. 2006, 47, 2361–2370. [Google Scholar] [CrossRef]
- Karunanithy, C.; Shafer, K. Heat transfer characteristics and cooking efficiency of different sauce pans on various cooktops. Appl. Therm. Eng. 2016, 93, 1202–1215. [Google Scholar] [CrossRef]
- Cabeza-Gil, I.; Calvo, B.; Grasa, J.; Franco, C.; Llorente, S.; Martínez, M. Thermal analysis of a cooking pan with a power control induction system. Appl. Therm. Eng. 2020, 180, 115789. [Google Scholar] [CrossRef]
- Sanz-Serrano, F.; Sagues, C.; Llorente, S. Inverse modeling of pan heating in domestic cookers. Appl. Therm. Eng. 2016, 92, 137–148. [Google Scholar] [CrossRef] [Green Version]
- Ogata, K. Modern Control Engineering; Prentice Hall: Hoboken, NJ, USA, 2010. [Google Scholar]
- Hewitt, G.F.; Shires, G.L.; Bott, T.R. Process Heat Transfer; BHB: USA, 1994. Available online: https://www.begellhouse.com/ebook_platform/monograph/book/7daba87f6fb65d65.html (accessed on 10 February 2022).
- Incropera, F.P.; DeWitt, D.P. Fundamentals of Heat and Mass Transfer, 8th ed.; John Wiley & Sons, Inc.: New York, NY, USA, 2017. [Google Scholar]
- Acero, J.; Carretero, C.; Lope, I.; Alonso, R.; Burdio, J.M. FEA-Based Model of Elliptic Coils of Rectangular Cross Section. IEEE Trans. Magn. 2014, 50, 1–7. [Google Scholar] [CrossRef]
- Lope, I.; Acero, J.; Carretero, C. Analysis and Optimization of the Efficiency of Induction Heating Applications with Litz-Wire Planar and Solenoidal Coils. IEEE Trans. Power Electron. 2016, 31, 5089–5101. [Google Scholar] [CrossRef] [Green Version]
- Weissman, S.A.; Anderson, N.G. Design of experiments (DoE) and process optimization. A review of recent publications. Org. Process. Res. Dev. 2015, 19, 1605–1633. [Google Scholar] [CrossRef]
- Seli, H.; Ismail, A.I.M.; Rachman, E.; Ahmad, Z.A. Mechanical evaluation and thermal modelling of friction welding of mild steel and aluminium. J. Mater. Process. Technol. 2010, 210, 1209–1216. [Google Scholar] [CrossRef]
- Paesa, D.; Llorente, S.; Sagues, C.; Aldana, O. Adaptive Observers Applied to Pan Temperature Control of Induction Hobs. IEEE Trans. Ind. Appl. 2009, 45, 1116–1125. [Google Scholar] [CrossRef]
- Lawless, Z.D.; Hobbs, M.L.; Kaneshige, M.J. Thermal conductivity of energetic materials. J. Energ. Mater. 2020, 38, 214–239. [Google Scholar] [CrossRef]
- Newborough, M.; Probert, S.; Newman, M. Thermal performances of induction, halogen and conventional electric catering hobs. Appl. Energy 1990, 37, 37–71. [Google Scholar] [CrossRef]



| Temperature (K) | Density (kg/m) | Conductivity (W/mK) | Specific Heat (kJ/kgK) | Expansion Coefficient (-) |
|---|---|---|---|---|
| 300 | 1.16 | 0.026 | 1.007 | 0.0037 |
| 350 | 0.99 | 0.030 | 1.009 | |
| 400 | 0.87 | 0.034 | 1.014 | |
| 450 | 0.77 | 0.037 | 1.021 | |
| 500 | 0.69 | 0.041 | 1.030 | |
| 550 | 0.63 | 0.044 | 1.040 | |
| 600 | 0.58 | 0.047 | 1.051 |
| Process Parameters | Low Level | Intermediate Level | Maximum Level |
|---|---|---|---|
| k (W/mK) | 49 | 142 | 235 |
| (J/kgK) | 300 | 420 | 540 |
| Scenario #A | Scenario #B | Scenario #C | |
| (W/mK) | 3 | - | 9 |
| (W/mK) | 3 | - | 9 |
| 0.3 | - | 0.9 |
| Model I | Model II | ||||
|---|---|---|---|---|---|
| WMF | Schulte | WMF | Schulte | Steel Plate | |
| (W/mK) | 4 | 6 | 5.5 | 7 | 8 |
| (W/mK) | 4 | 4 | 5.5 | 5.5 | 4 |
| (W/mK) | - | - | (r) | (r) | (r) |
| (W/mK) | 3000 | 3000 | - | - | - |
| (W/mK) | 3000 | 3000 | - | - | - |
| 0.95 | 0.87 | 0.95 | 0.87 | 0.4 | |
| WMF | 2.63 | 4.63 | 5.36 | 3.33 | 6.75 |
| Schulte | 1.33 | 2.93 | 4.94 | 13.27 | 3.31 |
| WMF | 3.09 | 9.45 | 6.69 | 3.77 | 5.65 |
| Schulte | 1.8 | 2.48 | 12.17 | 15.01 | 4.48 |
| Steel plate | 2.76 | 4.55 | 1.7 | 0.89 | 5.39 |
| Maximum Level | End of the Cooking | |||||
|---|---|---|---|---|---|---|
| WMF | Schulte | Steel Plate | WMF | Schulte | Steel Plate | |
| Conduction (W) | 496.7 | 122.4 | 546.6 | 49.6 | 14.9 | 46.8 |
| Convection (W) | 104.9 | 65.1 | 53.5 | 153.1 | 111.0 | 65.1 |
| Radiation (W) | 77.8 | 71.2 | 32.76 | 77.8 | 71.2 | 32.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bonet-Sánchez, B.; Cabeza-Gil, I.; Calvo, B.; Grasa, J.; Franco, C.; Llorente, S.; Martínez, M.A. A Combined Experimental-Numerical Investigation of the Thermal Efficiency of the Vessel in Domestic Induction Systems. Mathematics 2022, 10, 802. https://doi.org/10.3390/math10050802
Bonet-Sánchez B, Cabeza-Gil I, Calvo B, Grasa J, Franco C, Llorente S, Martínez MA. A Combined Experimental-Numerical Investigation of the Thermal Efficiency of the Vessel in Domestic Induction Systems. Mathematics. 2022; 10(5):802. https://doi.org/10.3390/math10050802
Chicago/Turabian StyleBonet-Sánchez, Belén, Iulen Cabeza-Gil, Begoña Calvo, Jorge Grasa, Carlos Franco, Sergio Llorente, and Miguel A. Martínez. 2022. "A Combined Experimental-Numerical Investigation of the Thermal Efficiency of the Vessel in Domestic Induction Systems" Mathematics 10, no. 5: 802. https://doi.org/10.3390/math10050802
APA StyleBonet-Sánchez, B., Cabeza-Gil, I., Calvo, B., Grasa, J., Franco, C., Llorente, S., & Martínez, M. A. (2022). A Combined Experimental-Numerical Investigation of the Thermal Efficiency of the Vessel in Domestic Induction Systems. Mathematics, 10(5), 802. https://doi.org/10.3390/math10050802






