Critical Percolation on Temporal High-Speed Railway Networks
Abstract
1. Introduction
2. Materials and Methods
2.1. Static HSR Network
2.2. Temporal HSR Network
3. Results
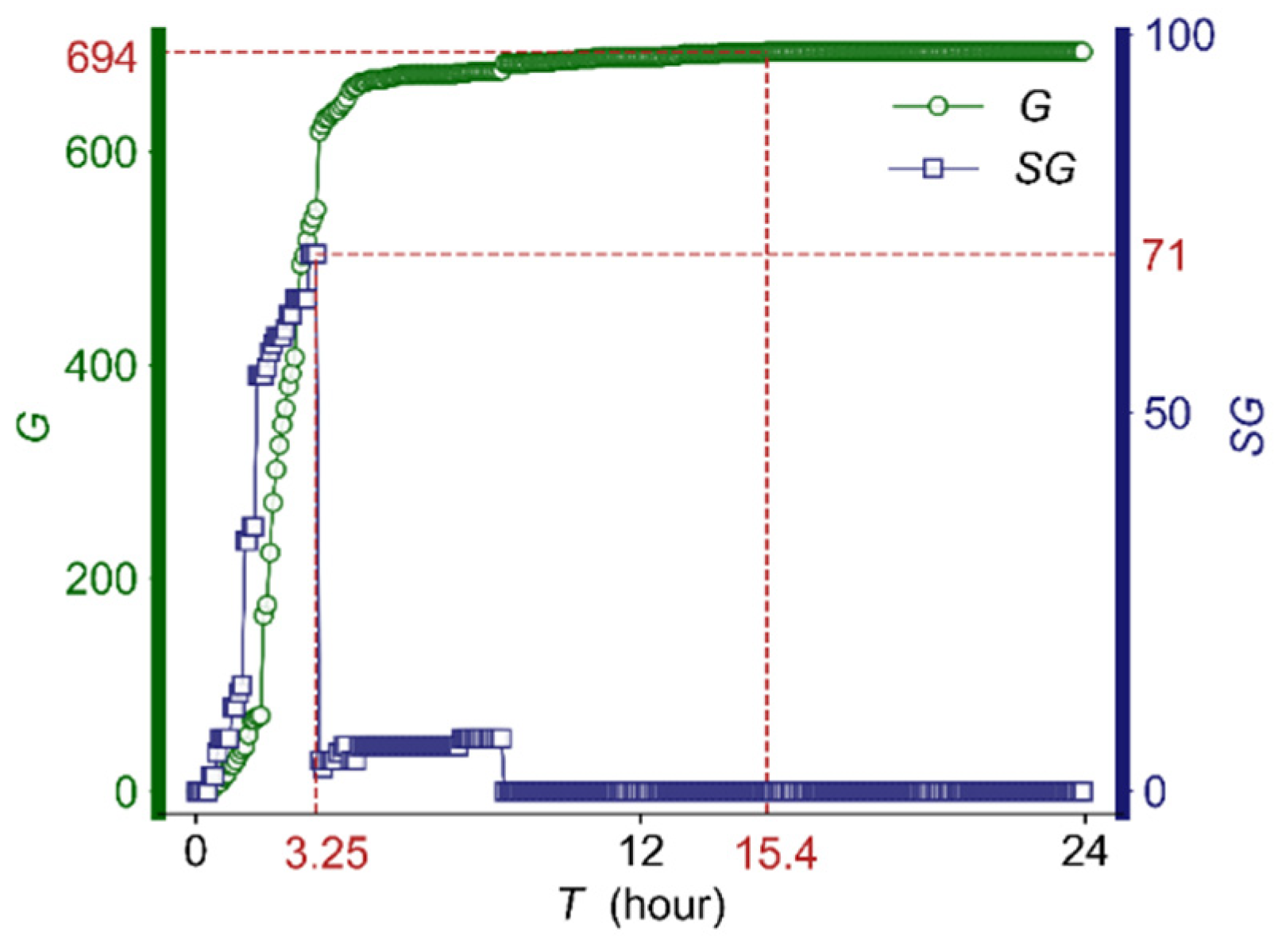
3.1. Percolation Transition in Temporal HSR Networks
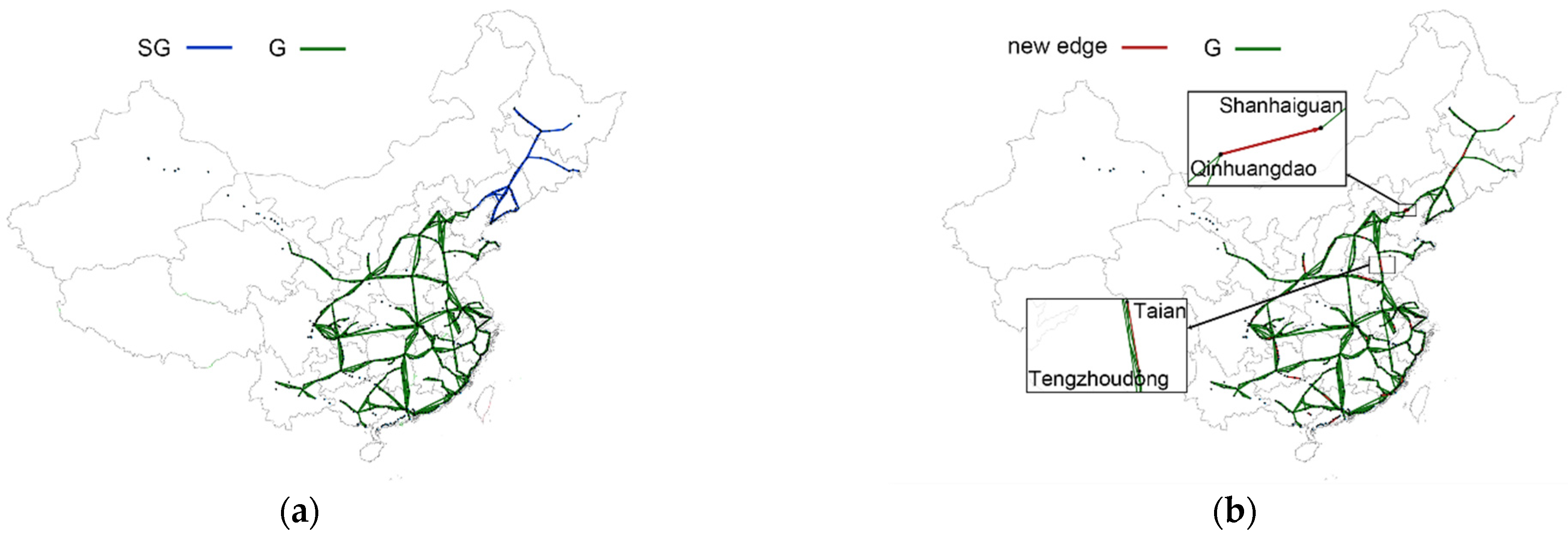
3.2. Critical Edges during Percolation Transition
3.3. Comparison of Percolation Processes for Temporal and Static HSR Networks
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, W.D.; Hilmola, O.-P.; Wu, J.H. Chinese High-speed Railway: Efficiency Comparison and the Future. Promet-Traffic Transp. 2019, 31, 693–702. [Google Scholar] [CrossRef]
- Zhang, J.H.; Wang, M. Transportation functionality vulnerability of urban rail transit networks based on movingblock: The case of Nanjing metro. Phys. A 2019, 535, 122367. [Google Scholar] [CrossRef]
- Li, Q.R.; Zhang, Z.; Li, K.; Chen, L.; Wei, Z.L.; Zhang, J.C. Evolutionary dynamics of traveling behavior in social networks. Phys. A 2020, 545, 123664. [Google Scholar] [CrossRef]
- Liu, W.C.; Cao, Y.H.; Wu, W.; Guo, J.Y. Spatial impact analysis of trans-Yangtze highway fixed links: A case study of the Yangtze River Delta, China. J. Transp. Geogr. 2020, 88, 102822. [Google Scholar] [CrossRef]
- Cao, W.W.; Feng, X.N.; Zhang, H. The structural and spatial properties of the high-speed railway network in China: A complex network perspective. J. Rail Transp. Plan. Manag. 2018, 9, 46–56. [Google Scholar] [CrossRef]
- Wei, S.; Jiao, J.J.; Wang, L.; Xu, J.G. Evolving Characteristics of High-Speed Railway Network Structure in Yangtze River Delta, China: The Perspective of Passenger Flows. Appl. Spat. Anal. Policy 2020, 13, 925–943. [Google Scholar] [CrossRef]
- Wang, W.; Cai, K.Q.; Du, W.B.; Wu, X.; Tong, L.; Zhu, X.; Cao, X.B. Analysis of the Chinese railway system as a complex network. Chaos Solitons Fractals 2020, 130, 109408. [Google Scholar] [CrossRef]
- Xie, F.J.; Ma, M.D.; Ren, C.P. Research on multilayer network structure characteristics from a higher-order model: The case of a Chinese high-speed railway system. Phys. A 2022, 586, 126473. [Google Scholar] [CrossRef]
- Zhang, H.; Cui, H.D.; Wang, W.; Song, W.B. Properties of Chinese railway network: Multilayer structures based on timetable data. Phys. A 2020, 560, 125184. [Google Scholar] [CrossRef]
- Wang, Z.J.; Jia, L.M.; Ma, X.P.; Sun, X.H.; Tang, Q.X.; Qin, S.N. Accessibility-oriented performance evaluation of high-speed railways using a three-layer network model. Reliab. Eng. Syst. Saf. 2022, 222, 108411. [Google Scholar] [CrossRef]
- Li, T.; Rong, L.L. Impacts of service feature on vulnerability analysis of high-speed rail network. Transp. Policy 2012, 110, 283. [Google Scholar] [CrossRef]
- Meng, Z.J.; Jia, L.M.; Xiang, W.L. Complex network model for railway timetable stability optimization. IET Intell. Transp. Syst. 2018, 12, 1369–1377. [Google Scholar] [CrossRef]
- Holme, P.; Saramäki, J. Temporal networks. Phys. Rep. 2012, 519, 97–125. [Google Scholar] [CrossRef]
- Anwar, M.S.; Ghosh, D. Intralayer and interlayer synchronization in multiplex network with higher-order interactions. Chaos 2022, 32, 033125. [Google Scholar] [CrossRef]
- Ma, H.K.; Yang, C.D. Exponential Synchronization of Hyperbolic Complex Spatio-Temporal Networks with Multi-Weights. Mathematics 2022, 10, 2451. [Google Scholar] [CrossRef]
- DiBrita, N.S.; Eledlebi, K.; Hildmann, H.; Culley, L.; Isakovic, A.F. Temporal Graphs and Temporal Network Characteristics for Bio-Inspired Networks during Optimization. Appl. Sci. 2022, 12, 1315. [Google Scholar] [CrossRef]
- Pan, R.K.; Saramaki, J. Path lengths, correlations, and centrality in temporal networks. Phys. Rev. E 2011, 84, 061605. [Google Scholar] [CrossRef]
- Mou, J.H.; Liu, C.C.; Chen, S.R.; Hang, G.; Lv, X. Temporal Characteristics of the Chinese Aviation Network and their Effects on the Spread of Infectious Diseases. Sci. Rep. 2017, 7, 1275. [Google Scholar] [CrossRef][Green Version]
- Li, M.; Liu, R.R.; Lv, L.Y.; Hu, M.B.; Xu, S.Q.; Zhang, Y.C. Percolation on complex networks: Theory and application. Phys. Rep. 2021, 907, 1–68. [Google Scholar] [CrossRef]
- Wang, F.L.; Li, D.Q.; Xu, X.Y.; Wu, R.Q.; Havlin, S. Percolation properties in a traffic model. Europhys. Lett. 2015, 112, 38001. [Google Scholar] [CrossRef]
- Kim, J.-H.; Kim, S.-J.; Goh, K.-I. Critical behaviors of high-degree adaptive and collective-influence percolation. Chaos 2020, 30, 073131. [Google Scholar] [CrossRef] [PubMed]
- Gao, J.X.; Liu, X.M.; Li, D.Q.; Havlin, S. Recent Progress on the Resilience of Complex Networks. Energies 2015, 8, 12187–12210. [Google Scholar] [CrossRef]
- Malekan, A.; Saber, S.; Saberi, A.A. Exact finite-size scaling for the random-matrix representation of bond percolation on square lattice. Chaos 2022, 32, 023112. [Google Scholar] [CrossRef] [PubMed]
- Li, D.Q.; Fu, B.W.; Wang, Y.P.; Lu, G.Q.; Berezin, Y.; Stanley, H.E.; Havlin, S. Percolation transition in dynamical traffic network with evolving critical bottlenecks. Proc. Natl. Acad. Sci. USA 2015, 112, 669–672. [Google Scholar] [CrossRef] [PubMed]
- Hamedmoghadam, H.; Jalili, M.; Vu, H.L.; Stone, L. Percolation of heterogeneous flows uncovers the bottlenecks of infrastructure networks. Nat. Commun. 2021, 12, 1254. [Google Scholar] [CrossRef] [PubMed]
- Zeng, G.W.; Li, D.Q.; Guo, S.M.; Gao, L.; Gao, Z.Y.; Stanley, H.E.; Havlin, S. Switch between critical percolation modes in city traffic dynamics. Proc. Natl. Acad. Sci. USA 2019, 116, 23–28. [Google Scholar] [CrossRef]
- Liu, S.Y.; Li, Z.F.; Zhong, J.L. Percolation transition in temporal airport network. Chin. J. Aeronaut. 2020, 33, 219–226. [Google Scholar] [CrossRef]
- Ru, W.; Tan, J.X.; Xin, W.; Wang, D.J.; Xu, C. Geographic coarse graining analysis of the railway network of China. Phys. A 2008, 387, 5639–5646. [Google Scholar]
- Zhang, J.H.; Hu, F.N.; Wang, S.L.; Dai, Y.; Wang, Y.X. Structural vulnerability and intervention of high speed railway networks. Phys. A 2016, 462, 743–751. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Zhou, L.S.; Guo, B.; Chen, X.; Zhou, H.X. An efficient hybrid approach for scheduling the train timetable for the longer distance high-Speed railway. Sustainability 2021, 13, 2538. [Google Scholar] [CrossRef]
- Benson, A.R.; Gleich, D.F.; Leskovec, J. Higher-order organization of complex networks. Science 2016, 353, 163–166. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.J.; Le, M.L. High-order community detection in the air transport industry: A comparative analysis among 10 major international airlines. Appl. Sci. 2021, 11, 9378. [Google Scholar] [CrossRef]
- Sun, H.L.; Bianconi, G. Higher-order percolation processes on multiplex hypergraphs. Phys. Rev. E 2021, 104, 034306. [Google Scholar] [CrossRef] [PubMed]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Yu, S.; Zhang, C.; Zhang, P.; Wang, Y.; Gao, L. Critical Percolation on Temporal High-Speed Railway Networks. Mathematics 2022, 10, 4695. https://doi.org/10.3390/math10244695
Liu Y, Yu S, Zhang C, Zhang P, Wang Y, Gao L. Critical Percolation on Temporal High-Speed Railway Networks. Mathematics. 2022; 10(24):4695. https://doi.org/10.3390/math10244695
Chicago/Turabian StyleLiu, Yi, Senbin Yu, Chaoyang Zhang, Peiran Zhang, Yang Wang, and Liang Gao. 2022. "Critical Percolation on Temporal High-Speed Railway Networks" Mathematics 10, no. 24: 4695. https://doi.org/10.3390/math10244695
APA StyleLiu, Y., Yu, S., Zhang, C., Zhang, P., Wang, Y., & Gao, L. (2022). Critical Percolation on Temporal High-Speed Railway Networks. Mathematics, 10(24), 4695. https://doi.org/10.3390/math10244695






