Arnold’s Piecewise Linear Filtrations, Analogues of Stanley–Reisner Rings and Simplicial Newton Polyhedra
Abstract
1. Introduction
1.1. Prehistory
1.2. Fulfilling the Wishes of V.I. Arnold Dated 1975
1.3. Associated Graded Rings of Newton Filtered Rings and Stanley–Reisner Face Rings
1.4. Directions for Further Work
1.4.1. Direct Generalizations of the Results of This Paper to the Case of Conventional Polynomials and Formal Series
1.4.2. Approximation of a Non-Simplicial Newton Polytope by a Simplicial One
1.4.3. Explicit Construction of a Monomial Basis for Factor Algebra of Laurent Polynomials Modulo Ideal Generated by Generic Polynomials with the Same Non-Simplicial Newton Polytope
1.4.4. Development of a Module for Computer Algebra Systems That Generates the Basis Built in This Article, Having Received as Input a Simplicial Super-Convenient Polytope and Its Shelling
1.4.5. Preparation of Student Workshops on Acquaintance with Computer Systems of Convex Geometry and Commutative Algebra Based on the Material of This Article
1.4.6. An Interesting Unsolved Problem of Explicitly Constructing a Monomial Basis for the Jacobian Ideal of a Formal Series in d Variables with a Given Simplicial Newton Polytope
1.5. Definitions and Notations That Do Not Use Newtons Polytope Shelling
- —the dimension of the Newton polytope and the ambient vector space;
- —field of characteristic 0;
- In this article, only rings endowed with the structure of a -algebras will be considered, so we will consider the terms -algebra, algebra, ring as synonyms;
- —the vector space over of d-tuples of the form , equipped with coordinate-wise operations of addition and multiplication by a real number, equipped with the usual topology. We fix coordinates on this space and call them y-coordinates. It will be convenient for us, depending on the context, to call the elements of the vector space points or vectors. We will call a point integer if all of its y-coordinates are integers;
- —nonnegative volume (measure) in , normalized by the condition: volume of a unit cube in y-coordinates is equal to 1;
- Let X and Y be two sets in . We call the set defined by the formulathe Minkowski sum of sets X and Y, The Minkowski sum of a finite number of sets is defined in a similar way.If Y is a one-point set consisting of one element y, then, to simplify the notation, we will write instead of just ;
- We will consider various subsemigroups of the group , both with a neutral element and without it. An example of a subsemigroup without a neutral element is the set of d-tuples of integers in which the first components are positive and the rest are non-negative;
- Let be a subsemigroup of . We denote by the semigroup algebra of functions on G with values in that take nonzero values only at a finite number of points. Multiplication in this algebra is the convolution of functions. In particular, we will denote by the group algebra of the group over the field . It is convenient to speak of this algebra as the algebra of Laurent polynomials in variables over the field with operations of addition and multiplication of polynomials;
- facet—any highest-dimensional face on the boundary of any convex polytope. The facets of a d-dimensional polytope have dimension and are closed sets in ;
- A convex polytope is called integer if all its vertices have integer y-coordinates and it is called simplicial if all its facets are simplices;
- A polytope in is called super-convenient if it is convex and contains some neighborhood of the origin. A super-convenient polytope has dimension d, and its boundary is covered with facets of dimension . It is obvious that each ray emanating from the origin intersects the boundary of the super-convenient polytope at exactly one point. The super-convenience property of a polytope is not preserved under translations;
- Let be a super-convenient simplicial polytope in with integer vertices. This polytope will be fixed until the end of this article. denotes the boundary of the polytope .Let
- N be a number of facets of ;
- L be a number of vertices of ;
- As a rule, in this article, we will denote:- By a facet, involved in a current reasoning;- By facets of the shelling, defined in Section 2.1;- By v, vertices participating in a current reasoning.
- For any facet of a superconvenient polytope , the vertex-vectors of this facet are linearly independent. Denote by the rational simplicial cone with generators , that is, the set of all possible linear combinations of the vectors with non-negative real coefficients. It follows from the superconvenience of the polytope that each cone is proper, that is, it does not contain any one-dimensional vector subspace ;
- On each simplicial cone , where is a facet of , we introduce a coordinate system for working with objects belonging to this cone. Since the facet’s vertex-vectors form a basis in , there exist linear functions on satisfying the conditions (the Kronecker delta). These functions will be called c-coordinates on the cone . For a point , we put ;
- Let us call the set of all possible linear combinations of vertices with non-negative integer coefficients the vertex semigroup of an facet . This set coincides with the set of points of the cone with non-negative integer c-coordinates, so this semigroup is isomorphic to the free commutative additive semigroup of rows of length d composed of non-negative integers;
- Let us call the set of points of the cone with integer y-coordinates the complete semigroup of an facet . Since the generators of the vertex semigroup have integer y-coordinates, all elements of the vertex semigroup have integer y-coordinates, that is, ;
- We say that a point is a shift of a point by an element . Obviously, for the shifts of any point by and do not coincide. The orbit of a point under the action of the vertex semigroup is the set of all shifts of this point: . The complete semigroup is invariant under shifts by elements of the vertex semigroup, i.e., the orbit of any point under the action of the vertex semigroup lies in ;
- The number of points in a finite set X will be denoted by .
1.6. -Algebra of Laurent Polynomials
1.7. Algorithm for Constructing a Monomial Basis of the Factor Algebra
Shelling Extension of Super-Convenient Newton Polytope
2. Shelling of Super-Convinient Simplicial Polytope and Objects Defined by That Shelling
2.1. Definition of a Shelling
- -
- Elements of preceding the facet:
- -
- Elements of preceding the facet:Each face of dimension of any convex d-dimensional polytope belongs to exactly two facets of this polytope. Therefore, any -dimensional face belongs to the boundary of exactly one facet of the polytope other than . This second facet is a member of sequence (8) or a member of sequence (9). In the first case, the face belongs to the intersection (6), in the second case, the face belongs to the intersection (7).
2.2. Shelling Defines a Covering and Partitioning of the Boundary of a Convex Simplicial Polytope
2.3. Covers and Partitions of the Sets and Defined by the Shelling of a Super-Convinient Simplicial Newtons Polytope
- is the union of all closed rays starting from the origin and passing through some point on . The set is closed in and, due to the convexity of the face , is a commutative semigroup with respect to the addition operation with a neutral element ;
- is a semigroup with neutral element 0, consisting of all integer points of the cone . This semigroup is called the complete semigroup of . The semigroup -algebra is called the complete algebra of the face ;
- is a subsemigroup with a neutral element in generated by all vertices of facet . The semigroup is freely generated by elements . We call this semigroup vertex semigroup of facet . The -algebra will be called the vertex algebra of facet . This -algebra is isomorphic to the algebra of polynomials in d independent variables. Since the semigroup is a subsemigroup of , for any facet , the algebra is a module over the vertex algebra . We verify below that this module is both finitely generated and freely generated.
2.4. Semi-Open Parallelepipeds and Sets of Their Integer Points as Fundamental Domains for Action of Vertex Semigroups on -Cones and -Semigroups
- -
- is a closed orthant of points with non-negative c-coordinates;
- -
- Δ is the set of points for which the sum of all c-coordinates is equal to 1 and all c-coordinates are non-negative;
- -
- A cone with vertex at the origin and base equal to facet Δ is the set of points whose sum of all c-coordinates is less than or equal to 1 and all c-coordinates are non-negative;
- -
- For any vertex v of the facet Δ, one of the c-coordinates is 1, and the other c-coordinates are 0.
- -
- The full semigroup is nothing else than the set of points of the cone with integer y-coordinates;
- -
- The vertex semigroup is nothing else than the set of points of the cone with non-negative integer c-coordinates. Since the generators of vertex semigroups have integer y-coordinates, all elements of the vertex semigroup have integer y-coordinates, that is, .
2.4.1. Fundamental Domains for Actions of the Vertex Semigroup on the Sets and
2.4.2. Fundamental Domains for Actions of the Vertex Semigroup of Any Facet on the -Cone and the -Semigroup of This Facet
- -
- The set of points of the -cone is closed with respect to the addition operation, which means that its subset of points with integer y-coordinates is also closed with respect to addition;
- -
- Adding any vector with non-negative c-coordinates to a point satisfying the inequalities (35) does not violate these inequalities, so the semigroup acts on the -cone . Finally, shifting an integer point in by a vector with non-negative integer y-coordinates from the set gives a point with integer coordinates, so acts on .
- (ii)
- ;
- (iii)
- The number of integer points in a semi-open parallelepiped is equal to its volume:
- (b)
- For any point p of any of the sets the sum of the c-coordinates of the point p does not exceed d;
- (c)
- Only one of the sets , namely, the set , contains a point whose sum of c-coordinates equals d and such a point is unique;
- (d)
- Newtonian degree of any point of the setdoes not exceed , this set contains exactly one element of Newtonian degree 0 and exactly one element of maximum Newtonian degree .
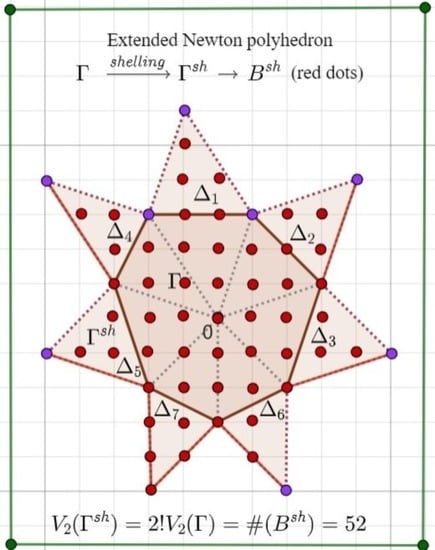
2.5. Shelling Extensions of the Super-Convenient Newton Polytope
- (i)
- (ii)
- (iii)
- The number of integer points in any shelling extension of the polytope Γ does not depend on the shelling and is equal to , i.e.,
3. Combinatorial Problem Whose Solution Leads to Construction of Monomial Basises for Quotient Algebras
3.1. Newtonian Degree
Defining a Convex Super-Convenient Polytope by a Non-Negative Piecewise Linear Function
- (i)
- for any ;
- (ii)
- .
- (i)
- for any ;
- (ii)
- ;
- (iii)
- ;
- (iv)
- .
- (a)
- ;
- (b)
- , where is some facet of Γ if and only if ;
- (c)
- For any and any
- (d)
- (c) becomes an equality if and only if there exists a facet such that any of points belongs to ;
- (e)
- For any , any , and any
3.2. Newtonian Generating Functions of Various Subsets of
- (b)
- If the set is the union of a finite or countable number of pairwise disjoint subsetsthen
- (a)
- (b)
- (c)
- (d)
3.3. Graded Vector Spaces and Their Poincaré Series
3.4. Calculation of the Newtonian Generating Function by Two-Stage Partitioning of the Group into Disjoint Subsets
3.4.1. Stage 1. Partitioning the Group into -Semigroups
3.4.2. Stage 2. Partitioning the Set of Integer Points of the -Cone into Orbits of the Action of the Semigroup of Vertices
3.5. The Dehn–Sommerville Property of the Newtonian Generating Function
- -
- Cones ;
- -
- Fundamental domains ;
- -
- Finite sets of integer points ; and
- -
- Polynomials .
- -
- Cones ;
- -
- Fundamental domains ;
- -
- Finite sets of integer points ; and
- -
- Polynomials .
4. Standard Information about Filtered and Graded Objects and Their Poincaré Series
4.1. Elementwise Comparison of Poincaré Series of Graded Algebras
4.2. Graded Cohen–Macaulay Rings
- (i)
- the ideal generated by all elements of the sequence does not coincide with R;
- (ii)
- the element is not a zero divisor in R, the element is not a zero divisor in the quotient algebra , the element is not a zero divisor in the quotient algebra ,…, and, finally, the element is not a zero divisor in the quotient algebra .
- (a)
- The following conditions are equivalent:
- (i)
- is finite;
- (ii)
- Sequence is regular;
- (iii)
- The -algebra R is a free module with a finite number of homogeneous generators over its subalgebra ;
- (iv)
- The Poincaré series of the -algebra , is given by the formula
- (b)
- If the conditions of part a) are satisfied, then:
- -
- The formal series H(R/I,t) is actually a polynomial with non-negative coefficients;
- -
- The sum of the coefficients of this polynomial is equal to the codimension of the ideal I, and is also equal to the number of generators of the free module defined in (iii);
- -
- Any homogeneous basis of the quotient algebra can be taken as the set of generators of the free module from (iii);
- -
- The cosets of any minimal set of generators from (iii) form a basis of the vector space .
5. Newton Filtration on Algebra of Laurent Polynomial and Associated Graded Algebra
5.1. Newton’s Filtration on
5.1.1. Newtoninian Degrees of Monomials
5.1.2. Definition of Newton’s Filtration
5.2. Associated Graded Ring of the Arnold–Newton Filtered Ring of Laurent Polynomials
5.2.1. Monomials Multiplication Rule in the Associated Graded Ring
- (ii)
- A similar rule is valid for the product of an arbitrary number of monomials:
5.2.2. The Subring of Vertices of the Graded Ring of the Super-Convenient Convex Integer Simplicial Polytope
5.3. The Vertex Ring Is Nothing Else Than the Stanley–Reisner Ring of the Simplicial Complex of Facets of the Convex Integer Simplicial Polytope
6. “Shelling” of the Set and “Shelling” of the Rings
- -
- A sequence of subsemigroups of the group belonging to the cones ;
- -
- A sequence of finite sets of the group belonging to the semigroups .
- -
- -
- , in particular, ;
- -
- for ;
- -
- The subsemigroup is invariant under the action of the vertex semigroup on the semigroup ;
- -
- For any i, and for , ;
- -
- The set is the fundamental domain for action of the vertex semigroup on the subsemigroup , that is, shifts of the finite set by all possible elements of the vertex semigroup form a partition of the set ;
- -
- The cardinality of is equal to the volume of the parallelepiped defined in the Section 2.4.2.
6.1. “Shelling” of the Ring
- (ii)
- Similarly, we define homoconicality and heteroconicality for several points in :integer points in will be called homoconical if there is at least one number such that , …. Points will be called heteroconical if they are not homoconical ;
- (iii)
- Define the operation “” with a rule similar to the rule (72):
- (iv)
- Let us extend the operation “”, allowing us to use as its arguments not only monomials of the form , but also and assuming in this case, the result of the operation is equal to .
- (ii)
- Extension by linearity of this operation on the entire vector space defines -algebra structure on ;
- (iii)
- The product of an arbitrary number of monomials in the -algebra is calculated according to the rule
6.2. Ring’s “Shelling” Properties
- (1)
- Points simultaneously belong to one of the cones. In this case, according to the Definition 18,and equality (80) holds for .
- (2)
- The points do not simultaneously lie in any of the cones. In this case, according to Definition 18,and and again equality (80) holds for . It remains to analyze the last case.
- (3)
- The points do not simultaneously lie in any of the cones, i.e., , but both lie in the cone , that is, . Let us prove that in this case , and hence and the equality (80) holds for .
7. Cohen–Macaulayness of Algebra
7.1. Non-Degeneracy of Sequence Homogeneous Elements of the Ring Having Supports in . “Shelling” of Such a Sequence
- (b)
- Subalgebras and of are identical.
7.2. Formulation of the Main Technical Result of This Article
- (A)
- For any , the equivalence classes of monomials with exponents running through the set generate as a module over ;
- (B)
- The sequence is regular and hence, is a Cohen–Macaulay ring;
- (C)
- The equivalence classes of monomials with exponents running through the setform a basis of , hence, the codimension of the ideal I is equal to .
7.3. Derivation of Conclusions (B) and (C) of Theorem 4 from Conclusion (A) of This Theorem
- (1)
- The equivalence classes of these monomials form a basis of the quotient algebra and the codimension of I is equal to ;
- (2)
- These monomials freely generate as a module over X;
- (3)
- The sequence of elements of algebra is regular.
7.4. Proof of Theorem 4 (A)
8. Main Theorem
8.1. Verbose Formulation of the Main Theorem
- Super-convenient convex integer simplicial polytope ;
- The number defined by this polytope;
- Field of characteristic 0;
- An exhaustive increasing Newton -filtration of -algebra ; this -algebra will be denoted for brevity by A:
- Natural number M defined by the condition that the monomials corresponding to the vertices of Γ belong to ;
- An exhaustive increasing -filtration of free A-module formed by tuples of d elements of the algebra A, defined by the relations
- The -graded algebra associated with the Newton filtration, denoted for brevity by ;
- -grading of the free module formed by tuples of d elements of the algebra , defined by the relations
- Definition of the Newtonian degree of an element , well defined since Newtons filtering is exhaustive:
- Definition of leading_term (aka boundary_term) of a nonzero element of the filtered algebra, this leading_term is denoted by , belongs to , where , and is defined by the formula
- Shelling of polytope Γ;
- Finite set constructed from the super-convenient simplicial polytope Γ and its shellingcontaining all points of Newtonian degree less M and not containing points of Newtonian degree higher than ;
- The set of monomials in the filtered algebra consisting of the elements
- The set of monomials in the graded algebra , consisting of the elements
- Finite-dimensional vector space of d-tuples of Laurent polynomials whose supports belong to the polytope Γ;
- Finite-dimensional vector space of d-tuples of Laurent polynomials whose supports belong to the boundary of the polytope Γ;
- Linear mappingLeading_term: (aka Boundary_term) of finite-dimensional vector spaces, which deletes from each component of the tuple of Laurent polynomials the monomials corresponding to the interior points of the polytope Γ, leaving only the terms of the Newtonian degree M corresponding to monomials with exponents lying on the boundary of the polytope Γ.
8.1.1. Proof of Conclusion (Main.a)
8.1.2. Outline of the Proof of (Main.b)
- -
- A sufficient condition for the strictness of mapping filtered modules, formulated in Proposition 38 below;
- -
- A known theorem on the vanishing of the homology of dimension 1 of the Koszul complex of a regular sequence of homogeneous elements of positive degrees of a finitely generated graded algebra over a field of characteristic 0, see, for example, Theorem 06 in [26]
8.1.3. Proof of Assertion (Main.c)
8.1.4. Proof of Assertion (Main.d)
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Arnol’d, V.I. Normal forms of functions in neighbourhoods of degenerate critical points. Russ. Math. Surv. 1974, 29, 10–50. [Google Scholar] [CrossRef]
- Kushnirenko, A.G. Newton polyhedron and Milnor numbers. Funct. Anal. Its Appl. 1975, 9, 71–72. [Google Scholar] [CrossRef]
- Kushnirenko, A.G. Newton polytopes and the Bezout theorem. Funct. Anal. Its Appl. 1976, 10, 233–235. [Google Scholar] [CrossRef]
- Kouchnirenko, A.G. Polyèdres de Newton et nombres de Milnor. Invent. Math. 1976, 32, 1–32. [Google Scholar] [CrossRef]
- Hochster, M. Rings Invariants of Tori, Cohen-Macaulay Rings Generated by Monomials, and Polytopes. Ann. Math. Second. Ser. 1972, 96, 318–337. [Google Scholar] [CrossRef]
- Hochster, M. Cohen-Macaulay Varieties, Geometric Complexes, and Combinatorics. Available online: http://www.math.lsa.umich.edu/~hochster/comb2.pdf (accessed on 22 August 2022).
- Merle, M. Les anneaux Coniques Sont de Cohen-Macaulay, d’après A. G. Kouchnirenko. Séminaire sur les Singularités des Surfaces (1976–1977): 1–7. Available online: http://eudml.org/doc/114151 (accessed on 22 August 2022).
- Elashvili, A.G.; Jibladze, M.A.; Vinberg, E.B. Moduli algebras of some non-semiquasihomogeneous singularities. Funct. Anal. Its Appl. 2017, 51, 86–97. [Google Scholar] [CrossRef]
- Kind, B.; Kleinschmidt, P. Schälbare Cohen–Macaulay Komplexe und ihre Parametrisierung. Math. Z. 1979, 167, 173–179. [Google Scholar] [CrossRef]
- Stanley, R.P. A Glimpse of Combinatorial Commutative Algebra. Available online: www-math.mit.edu/~rstan/algcomb/chapter13.pdf (accessed on 22 August 2022).
- Richard, P. Stanley, Combinatorics and Commutative Algebra; Birkhäuser: Basel, Switzerland, 1983. [Google Scholar]
- Bruggesser, H.; Mani, P. Shellable decompositions of cells and spheres. Math. Scand. 1971, 29, 197–205. [Google Scholar] [CrossRef]
- Gallier, J. Notes on Convex Sets, Polytopes, Polyhedra, Combinatorial Topology, Voronoi Diagrams and Delaunay Triangulations. arXiv 2008, arXiv:0805.0292. [Google Scholar]
- Arnold, V.I. Arnold’s Problems; PHASIS: Moscow, Russia; Springer: Berlin, Germany, 2004. [Google Scholar]
- Brzostowski, S.; Krasi´nski, T.; Walewska, J. Arnold’s problem on monotonicity of the Newton number for surface singularities. J. Math. Soc. Jpn. 2019, 71, 1257–1268. [Google Scholar] [CrossRef]
- Selyanin, F. A non-negative analogue of the Kouchnirenko formula. arXiv 2020, arXiv:2006.11795. [Google Scholar]
- Barvinok, A. Lattice Points, Polyhedra, and Compexity. Lecture 3, Theorem 1, and Theorem 2, in Geometric Combinatorics; IAS/ParkCity Mathematics Series v. 13; Miller, E., Reiner, V., Sturmfels, B., Eds.; American Mathematical Society: Providence, RI, USA, 2007; Available online: http://www.math.lsa.umich.edu/~barvinok/lectures.pdf (accessed on 22 August 2022)ISSN 1079-5634.
- Günter, M. Ziegler, Lectures on Polytopes (in Graduate Texts in Mathematics, Vol 152); Springer: New York, NY, USA, 1995. [Google Scholar]
- Douai, A.; Sabbah, C. Gauss—Manin systems, Brieskorn lattices and Frobenius structures I. Ann. Inst. Fourier 2003, 53, 1055–1116. [Google Scholar] [CrossRef][Green Version]
- Douai, A. A note on the Newton spectrum of a polynomial. arXiv 2018, arXiv:1810.03901. [Google Scholar]
- Douai, A. Ehrhart polynomials of polytopes and spectrum at infinity of Laurent polynomials. J. Algebr. Comb. 2021, 54, 719–732. [Google Scholar] [CrossRef]
- Stanley, R.P. Combinatorics and Commutative Algebra, 2nd ed.; Progress in Mathematics, 41; Birkhäuser Boston, Inc.: Boston, MA, USA, 1996; ISBN 0-8176-3836-9. [Google Scholar]
- Atiyah, M.F.; Macdonald, I.G. Introduction to Commutative Algebra; Addison-Wesley: Reading, MA, USA, 1969. [Google Scholar]
- Hochster, M. Cohen-Macoley Rings. Available online: http://www.math.lsa.umich.edu/~hochster/615W14/CM.pdf (accessed on 22 August 2022).
- Bourbaki, N. Commutative Algebra; Springer: New York, NY, USA, 1985; Chapters 1–7. [Google Scholar]
- Ogus, A. Commutative Algebra. Available online: https://math.berkeley.edu/~ogus/Math_250B-2016/Notes/koszul.pdf (accessed on 22 August 2022).
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kushnirenko, A. Arnold’s Piecewise Linear Filtrations, Analogues of Stanley–Reisner Rings and Simplicial Newton Polyhedra. Mathematics 2022, 10, 4445. https://doi.org/10.3390/math10234445
Kushnirenko A. Arnold’s Piecewise Linear Filtrations, Analogues of Stanley–Reisner Rings and Simplicial Newton Polyhedra. Mathematics. 2022; 10(23):4445. https://doi.org/10.3390/math10234445
Chicago/Turabian StyleKushnirenko, Anatoly. 2022. "Arnold’s Piecewise Linear Filtrations, Analogues of Stanley–Reisner Rings and Simplicial Newton Polyhedra" Mathematics 10, no. 23: 4445. https://doi.org/10.3390/math10234445
APA StyleKushnirenko, A. (2022). Arnold’s Piecewise Linear Filtrations, Analogues of Stanley–Reisner Rings and Simplicial Newton Polyhedra. Mathematics, 10(23), 4445. https://doi.org/10.3390/math10234445