Significance of Multi-Hybrid Morphology Nanoparticles on the Dynamics of Water Fluid Subject to Thermal and Viscous Joule Performance
Abstract
1. Introduction
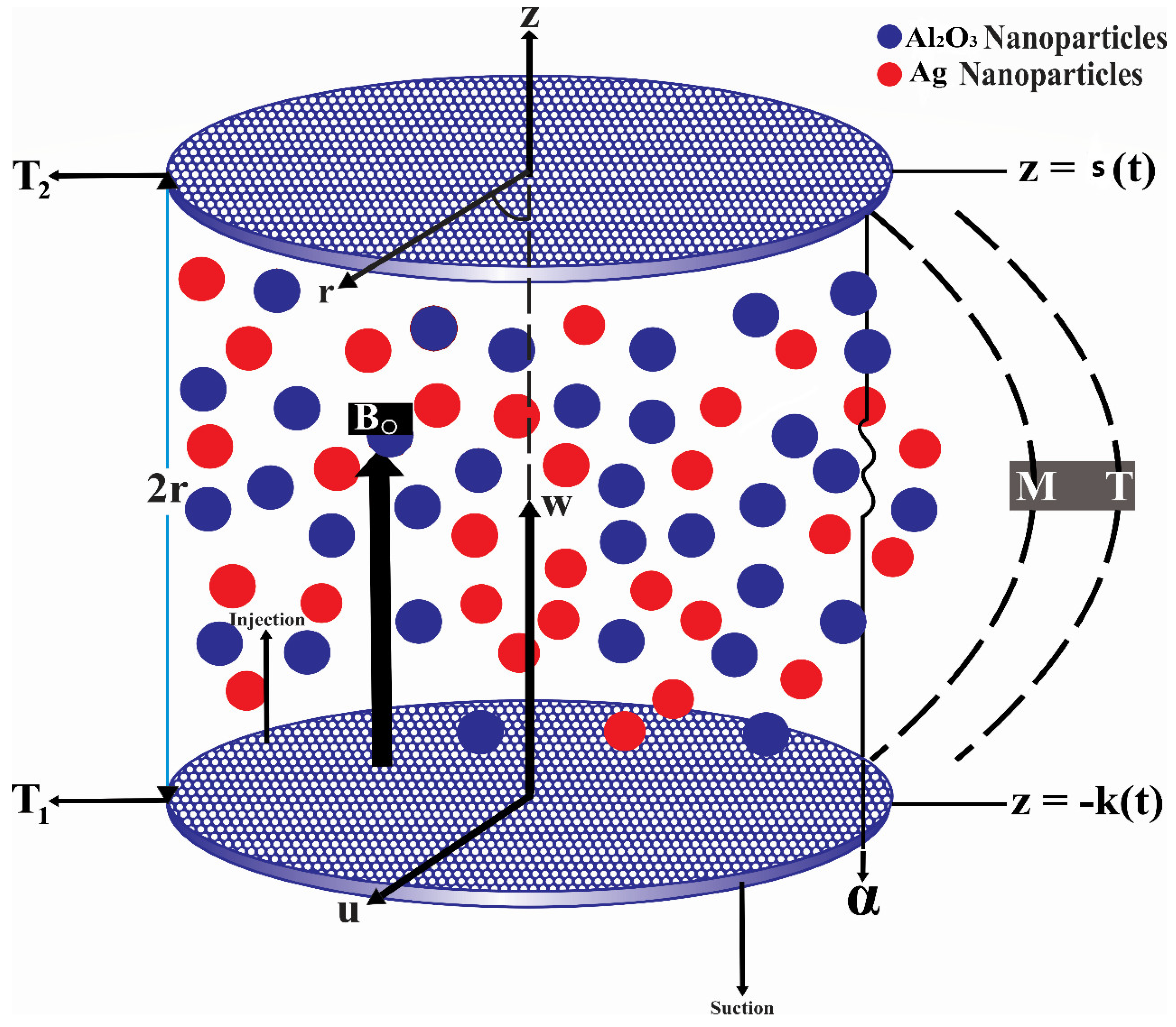
2. Mathematical Formulation
2.1. Practical and Engineering Interests
2.2. Skin Friction Coefficients
2.3. Nusselt Numbers
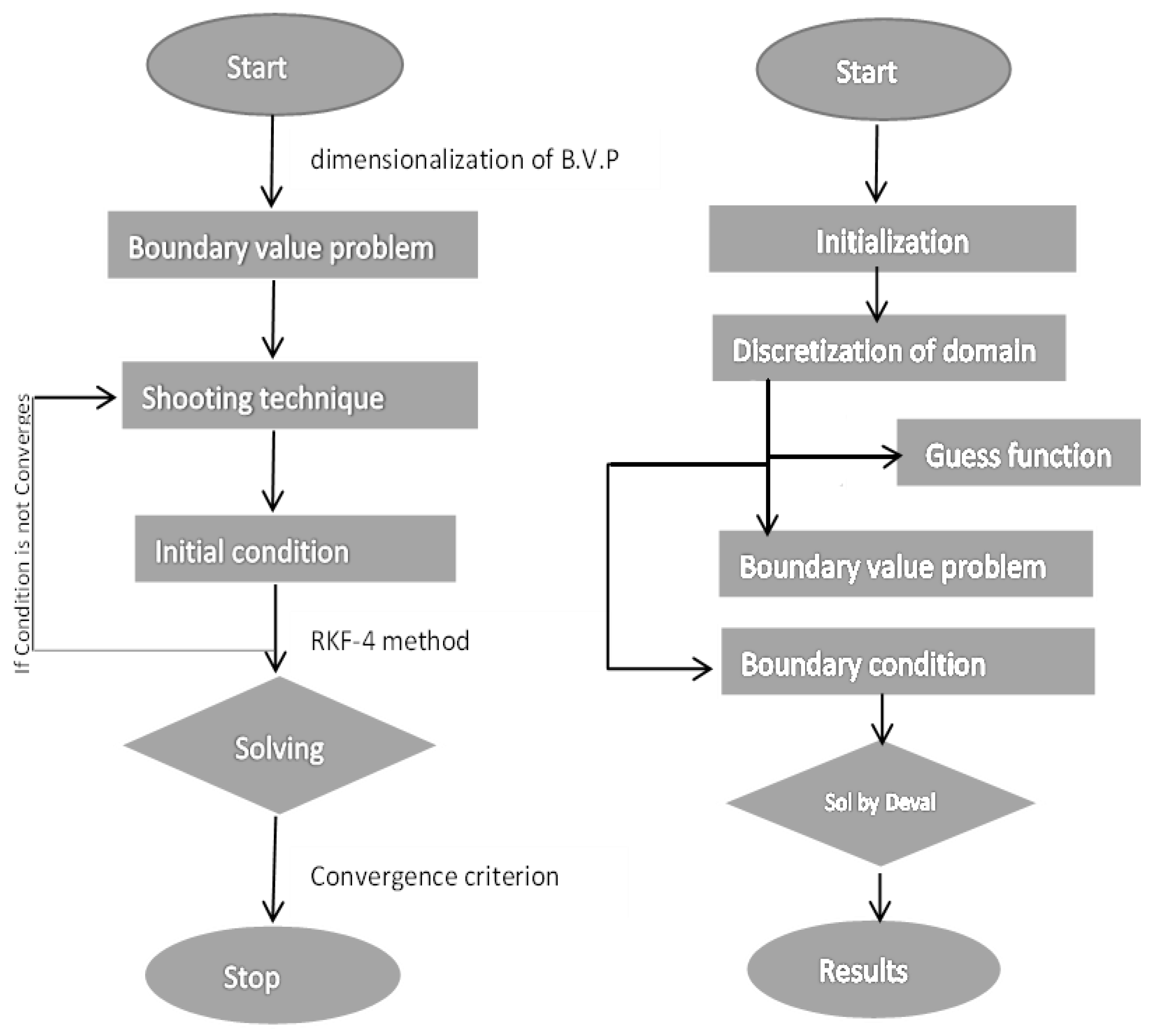
2.4. Numerical Solution
2.5. Solution of the Problem
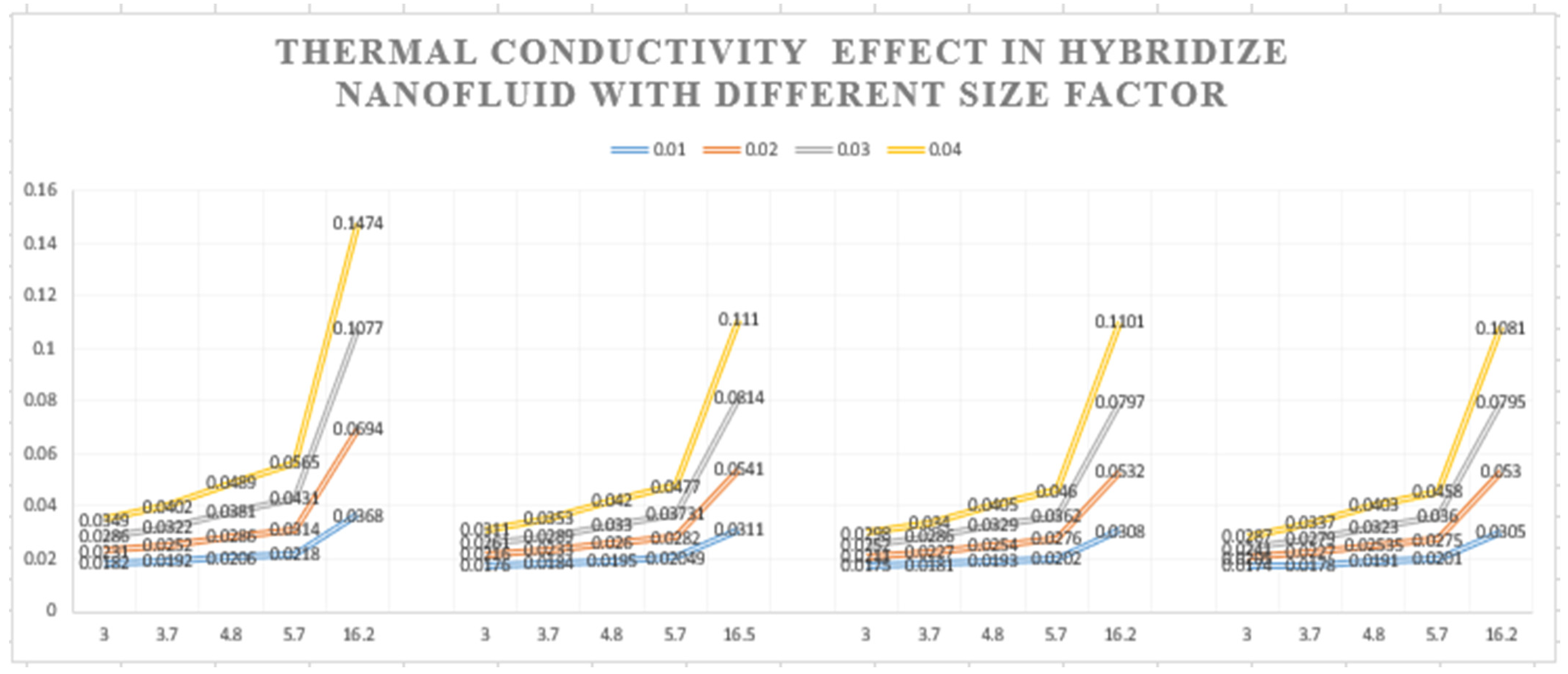
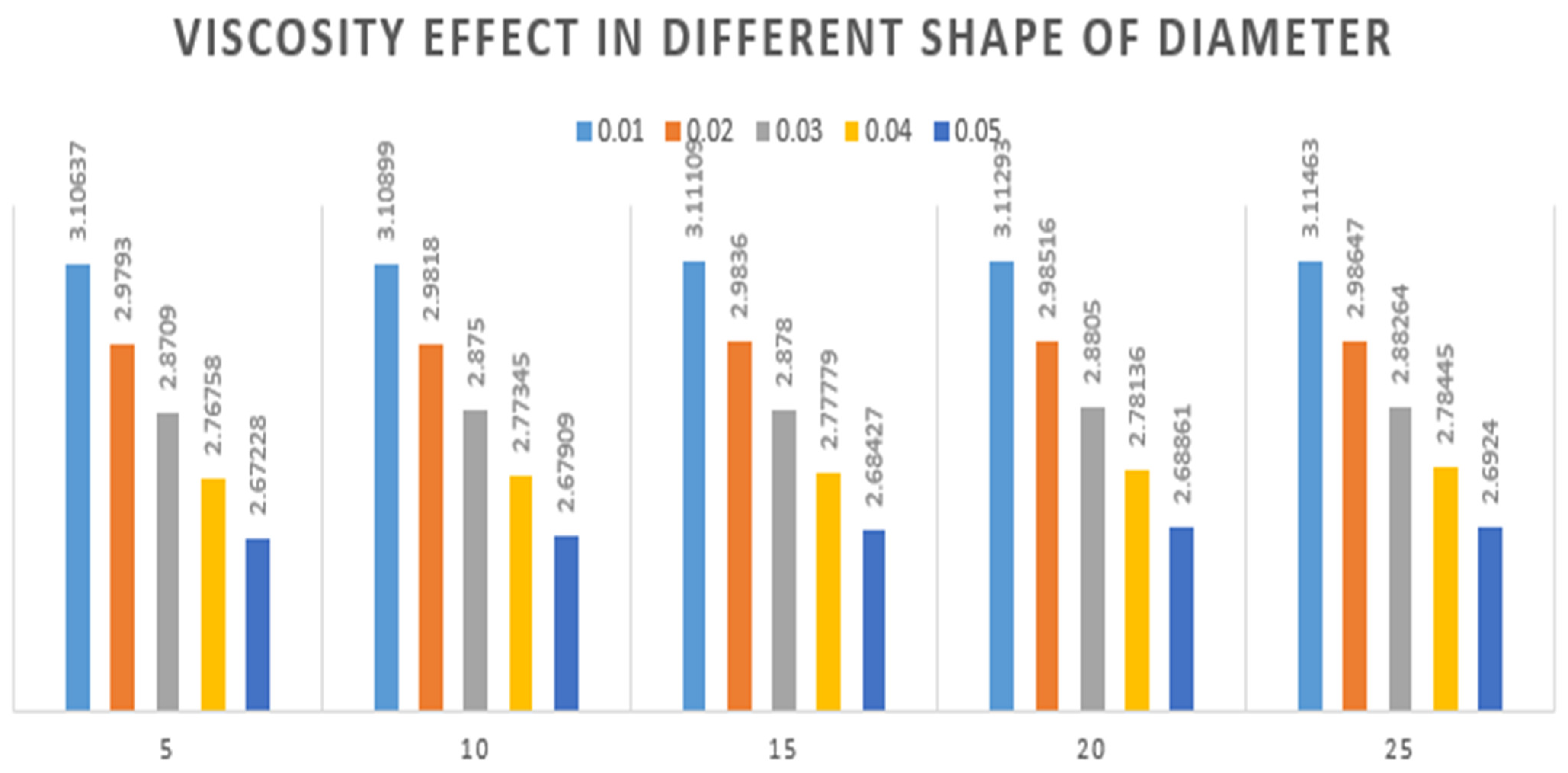
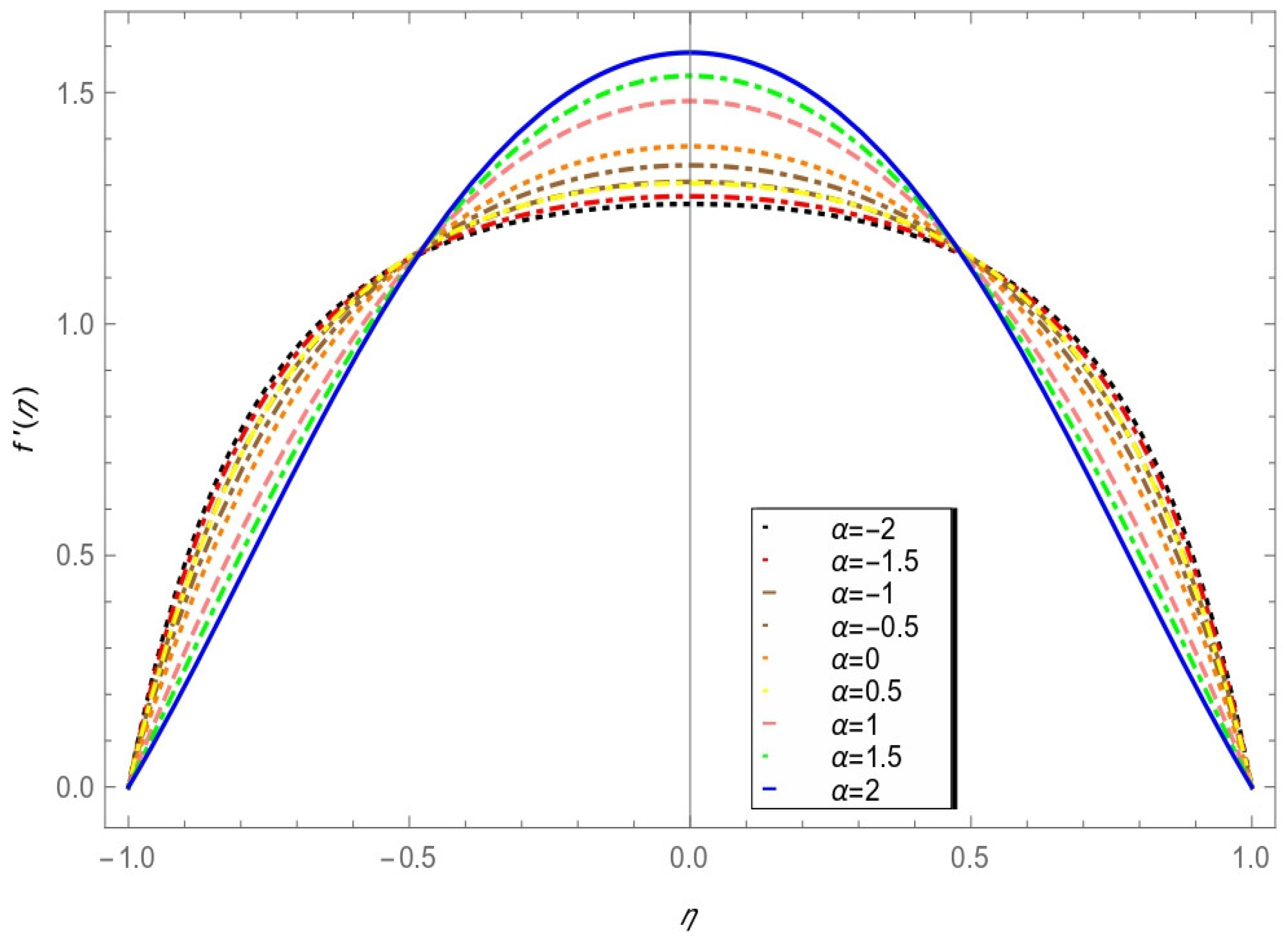
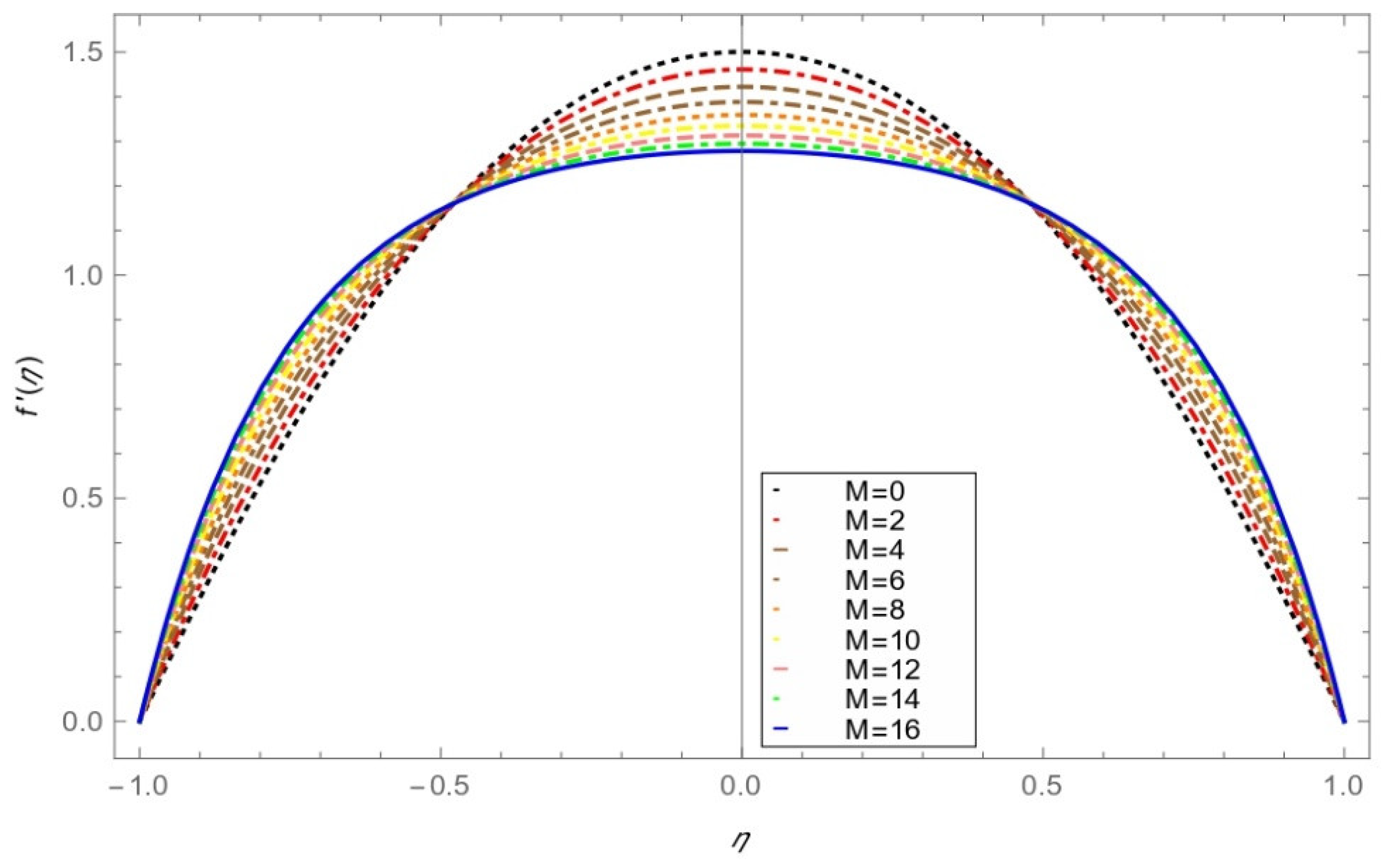
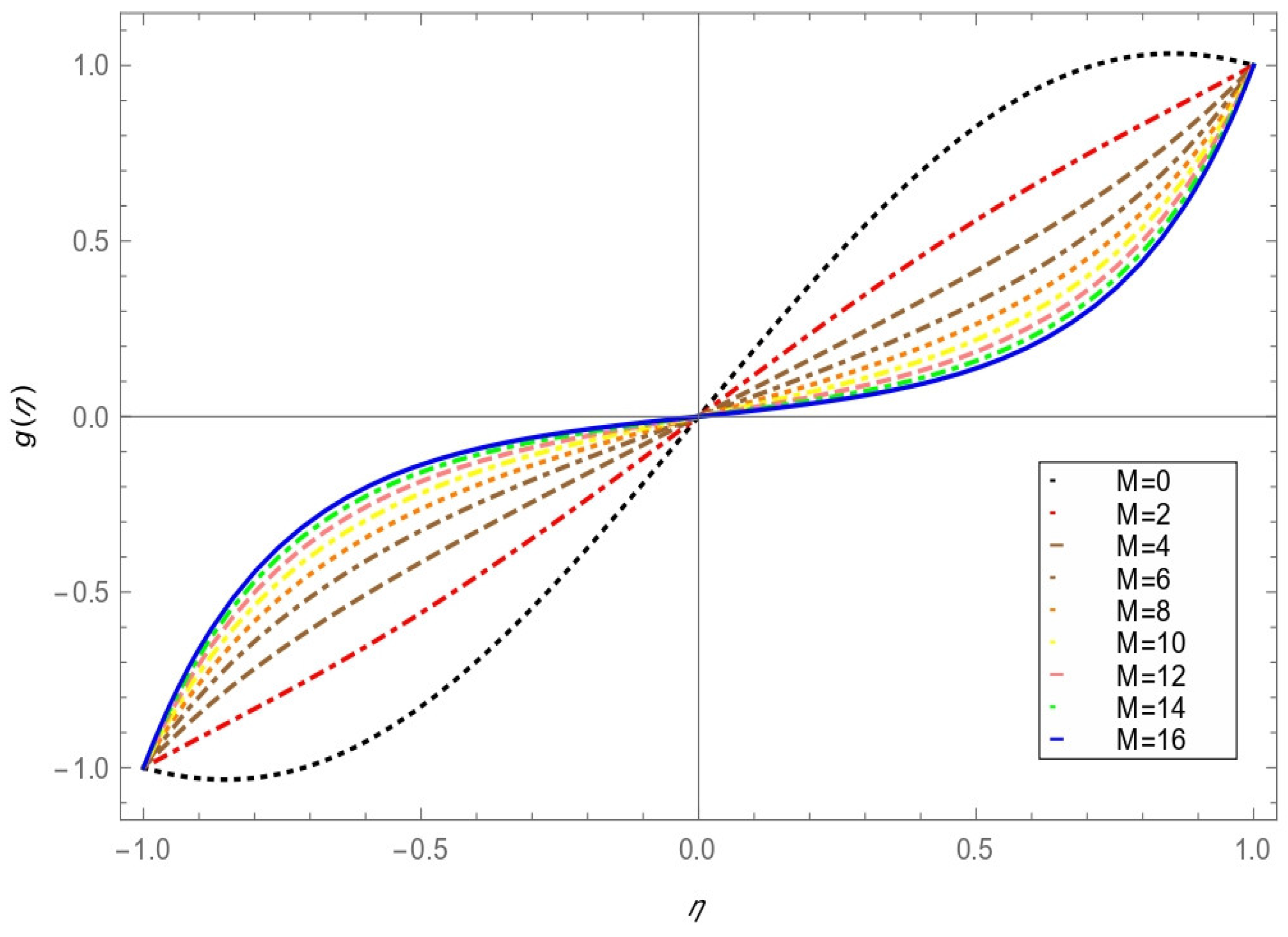
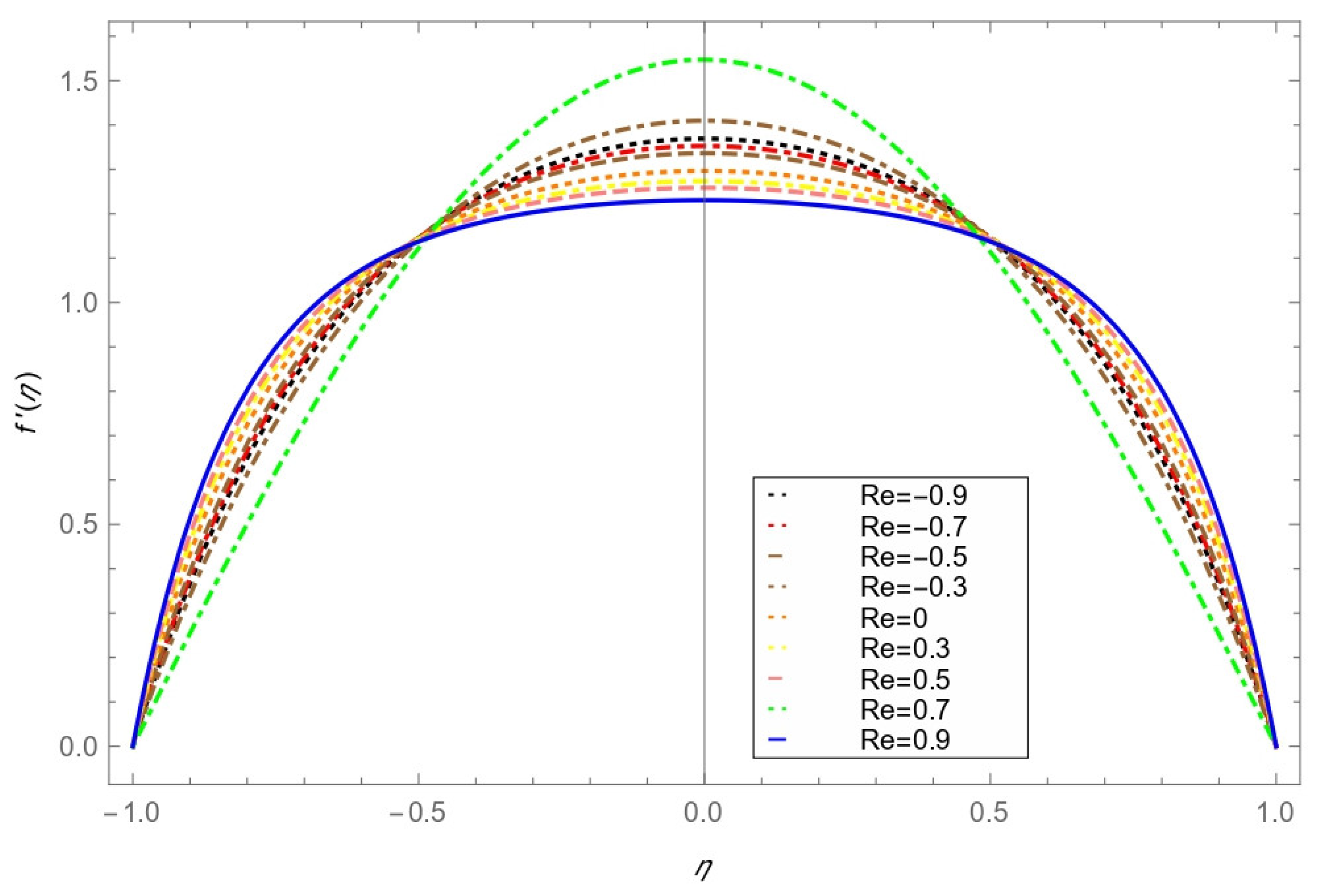
3. Results and Discussion
4. Conclusions
- Laminar nanoparticles 16.2 within the volume fraction of 4 percent in HNFD1 have the maximum thermal conductivity and viscosity intensity, as compared to other shapes spherical, brick, cylindrical, and platelet nanoparticles, respectively.
- The flow rate of heat transfer is enhanced on both porous disks by increasing the expansion of the value ratio and permeable Reynolds number .
- Enhance the values of permeability Reynolds number , Forchheimer number , and magnetic parameter , the momentum boundary layer increases in the upper and lower porous surface.
- Shear stress and tensional stress flow rates are slowed down if the porosity parameter, as well as the diameters of the nanoparticles, , and , are larger than zero.
- Heat transfer rates are raised on both porous disks by increasing the values of Prandtl number and Reynolds number .
- Eckert number parameter values are raised and the flow of heat transfer rates in both porous disks is reduced.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Strength of magnetic field [T] | |
| Dimensionless form radial velocity | |
| Dimensionless form tangential velocity | |
| Prandtl number (m2 s−1) | |
| Skin friction coefficient | |
| Dimensionless form temperature | |
| Fixed pressure at a specific heat capacity (J/(kg K) | |
| Nusselt number | |
| Dynamic viscosity (kg m−1 s−1) | |
| Eckert number | |
| r z | Cylindrical coordinates system |
| Dimensionless parameter | |
| Porosity parameter | |
| M | Magnetic field (wb/m2) |
| Forchheimer number | |
| (u, v, w) | Velocity components [gr or m/s] |
| Re | Reynolds number |
| α | Expansion ratio |
| and | Diameter of 1st and 2nd nanoparticles |
| Electrical conductivity [()/kg] | |
| Kinematic viscosity [/s] | |
| Thermal diffusivity of hybrid nanofluid [/s] | |
| Density [kg/ | |
| Volumetric heat capacity [J/(m3 K)] | |
| 1st nanoparticle volume fraction | |
| 2nd nanoparticle volume fraction | |
| Subscripts | |
| () | Base fluid (NFs) nanofluids |
| (HNFDs) | Hybrid nanofluids |
| HNFD1 | |
| HNFD2 | |
| HNFD3 | oil |
| HNFD4 | Ag-TiO2/H2O |
| HNFD5 | Ag-TiO2/ |
| HNFD6 | Ag-TiO2/ oil |
| HNFD7 | Cu-Al2O3/H2O |
| HNFD8 | Cu-Al2O3/ |
| HNFD9 | Cu-Al2O3/ oil |
| HNFD10 | Cu-TiO2/H2O |
| HNFD11 | Cu-TiO2/ |
| HNFD12 | Cu-TiO2/ oil |
| NP | Nanoparticles |
| MHD | Magnetohydrodynamics |
| PDE | Partial differential equation |
References
- Ahmed, N.; Adnan; Khan, U.; Mohyud-Din, S.T. Influence of shape factor on flow of magneto-nanofluid squeezed between parallel disks. Alex. Eng. J. 2018, 57, 1893–1903. [Google Scholar] [CrossRef]
- Abbas, W.; Magdy, M.M. Heat and Mass Transfer Analysis of Nanofluid Flow Based on, and over a Moving Rotating Plate and Impact of Various Nanoparticle Shapes. Math. Probl. Eng. 2020, 2020, 9606382. [Google Scholar] [CrossRef]
- Motlagh, S.Y.; Soltanipour, H. Natural convection of Al2O3-water nanofluid in an inclined cavity using Buongiorno’s two-phase model. Int. J. Therm. Sci. 2017, 111, 310–320. [Google Scholar] [CrossRef]
- Acharya, N.; Bag, R.; Kundu, P.K. Influence of Hall current on radiative nanofluid flow over a spinning disk: A hybrid approach. Phys. E Low-Dimens. Syst. Nanostruct. 2019, 111, 103–112. [Google Scholar] [CrossRef]
- Akbar, N.S.; Raza, M.; Ellahi, R. Influence of induced magnetic field and heat flux with the suspension of carbon nanotubes for the peristaltic flow in a permeable channel. J. Magn. Magn. Mater. 2015, 381, 405–415. [Google Scholar] [CrossRef]
- Dinarvand, S.; Nademi Rostami, M. An innovative mass-based model of aqueous zinc oxide–gold hybrid nanofluid for von Kármán’s swirling flow. J. Therm. Anal. Calorim. 2019, 138, 845–855. [Google Scholar] [CrossRef]
- Ramesh, G.K. Three different hybrid nanometrial performances on rotating disk: A non-Darcy model. Appl. Nanosci. 2018, 9, 179–187. [Google Scholar] [CrossRef]
- Xu, H. Modelling unsteady mixed convection of a nanofluid suspended with multiple kinds of nanoparticles between two rotating disks by generalized hybrid model. Int. Commun. Heat Mass Transf. 2019, 108, 104275. [Google Scholar] [CrossRef]
- Abdelmalek, Z.; Qureshi, M.Z.A.; Bilal, S.; Raza, Q.; Sherif, E.-S.M. A case study on morphological aspects of distinct magnetized 3D hybrid nanoparticles on fluid flow between two orthogonal rotating disks: An application of thermal energy systems. Case Stud. Therm. Eng. 2020, 23, 100744. [Google Scholar] [CrossRef]
- Dinarvand, S.; Rashidi, M.M.; Shahmohamadi, H. Analytic approximate solution of three-dimensional Navier-Stokes equations of flow between two stretchable disks. Numer. Methods Partial Differ. Equ. 2010, 26, 1594–1607. [Google Scholar] [CrossRef]
- Acharya, N.; Maity, S.; Kundu, P.K. Framing the hydrothermal features of magnetized TiO2–CoFe2O4 water-based steady hybrid nanofluid flow over a radiative revolving disk. Multidiscip. Model. Mater. Struct. 2019, 16, 765–790. [Google Scholar] [CrossRef]
- Yin, C.; Zheng, L.; Zhang, C.; Zhang, X. Flow and heat transfer of nanofluids over a rotating disk with uniform stretching rate in the radial direction. Propuls. Power Res. 2017, 6, 25–30. [Google Scholar] [CrossRef]
- Lou, Q.; Ali, B.; Rehman, S.U.; Habib, D.; Abdal, S.; Shah, N.A.; Chung, J.D. Micropolar dusty fluid: Coriolis force effects on dynamics of MHD rotating fluid when lorentz force is significant. Mathematics 2022, 10, 2630. [Google Scholar] [CrossRef]
- Ashraf, M.Z.; Rehman, S.U.; Farid, S.; Hussein, A.K.; Ali, B.; Shah, N.A.; Weera, W. Insight into significance of bioconvection on MHD tangent hyperbolic nanofluid flow of irregular thickness across a slender elastic surface. Mathematics 2022, 10, 2592. [Google Scholar] [CrossRef]
- Fallah, B.; Dinarvand, S.; Eftekhari Yazdi, M.; Rostami, M.N.; Pop, I. MHD flow and heat transfer of SiC-TiO2/DO hybrid nanofluid due to a permeable spinning disk by a novel algorithm. J. Appl. Comput. Mech. 2019, 5, 976–988. [Google Scholar]
- Hatami, M.; Sheikholeslami, M.; Ganji, D.D. RETRACTED: Laminar flow and heat transfer of nanofluid between contracting and rotating disks by least square method. Powder Technol. 2014, 253, 769–779. [Google Scholar] [CrossRef]
- Mustafa, M.; Khan, J.A.; Hayat, T.; Alsaedi, A. On Bödewadt flow and heat transfer of nanofluids over a stretching stationary disk. J. Mol. Liq. 2015, 211, 119–125. [Google Scholar] [CrossRef]
- Dogonchi, A.; Chamkha, A.J.; Seyyedi, S.M.; Ganji, D. Radiative nanofluid flow and heat transfer between parallel disks with penetrable and stretchable walls considering Cattaneo-Christov heat flux model. Heat Transfer Asian Res. 2018, 47, 735–753. [Google Scholar] [CrossRef]
- Sajjan, K.; Shah, N.A.; Ahammad, N.A.; Raju, C.S.K.; Kumar, M.D.; Weera, W. Nonlinear Boussinesq and Rosseland approximations on 3D flow in an interruption of Ternary nanoparticles with various shapes of densities and conductivity properties. AIMS Math. 2022, 7, 18416–18449. [Google Scholar] [CrossRef]
- Hasnain, J.; Abid, N. Numerical investigation for thermal growth in water and engine oil-based ternary nanofluid using three different shaped nanoparticles over a linear and nonlinear stretching sheet. Numer. Heat Transfer Part A Appl. 2022, 1–12. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Wahid, N.S.; Arifin, N.M.; Pop, I. Insight into three-dimensional flow of three different dynamics of nanofluids subject to thermal radiation: The case of water–cobalt ferrite, water–manganese–zinc ferrite, and water–magnetite. Heat Transfer 2022, 51, 4434–4450. [Google Scholar] [CrossRef]
- Koriko, O.K.; Adegbie, K.S.; Animasaun, I.L.; Olotu, M.A. Numerical solutions of the partial differential equations for investigating the significance of partial slip due to lateral velocity and viscous dissipation: The case of blood-gold Carreau nanofluid and dusty fluid. Numer. Methods Part. Differ. Equ. 2021, 7, 1–15. [Google Scholar] [CrossRef]
- Shah, N.A.; Wakif, A.; El-Zahar, E.R.; Ahmad, S.; Yook, S.-J. Numerical simulation of a thermally enhanced EMHD flow of a heterogeneous micropolar mixture comprising (60%)-ethylene glycol (EG), (40%)-water (W), and copper oxide nanomaterials (CuO). Case Stud. Therm. Eng. 2022, 35, 102046. [Google Scholar] [CrossRef]
- Rout, B.R.; Parida, S.K.; Panda, S. MHD Heat and Mass Transfer of Chemical Reaction Fluid Flow over a Moving Vertical Plate in Presence of Heat Source with Convective Surface Boundary Condition. Int. J. Chem. Eng. 2013, 2013, 296834. [Google Scholar] [CrossRef]
- Hayat, T.; Qayyum, A.; Alsaedi, A. Effects of heat and mass transfer in flow along a vertical stretching cylinder with slip conditions. Eur. Phys. J. Plus 2014, 129, 63. [Google Scholar] [CrossRef]
- Reddy, J.R.; Sugunamma, V.; Sandeep, N.; Sulochana, C. Influence of chemical reaction, radiation and rotation on MHD nanofluid flow past a permeable flat plate in porous medium. J. Niger. Math. Soc. 2016, 35, 48–65. [Google Scholar] [CrossRef]
- Hayat, T.; Rashid, M.; Imtiaz, M.; Alsaedi, A. Magnetohydrodynamic (MHD) flow of Cu-water nanofluid due to a rotating disk with partial slip. AIP Adv. 2015, 5, 067169. [Google Scholar] [CrossRef]
- Vajravelu, K.; Prasad, K.V.; Ng, C.-O.; Vaidya, H. MHD squeeze flow and heat transfer of a nanofluid between parallel disks with variable fluid properties and transpiration. Int. J. Mech. Mater. Eng. 2017, 12, 9. [Google Scholar] [CrossRef]
- Das, K.; Jana, S.; Acharya, N. Slip effects on squeezing flow of nanofluid between two parallel disks. Int. J. Appl. Mech. Eng. 2016, 21, 5–20. [Google Scholar] [CrossRef]
- Mohyud-Din, S.T.; Khan, S.I.; Bin-Mohsin, B. Velocity and temperature slip effects on squeezing flow of nanofluid between parallel disks in the presence of mixed convection. Neural Comput. Appl. 2016, 28, 169–182. [Google Scholar] [CrossRef]
- Qayyum, S.; Imtiaz, M.; Alsaedi, A.; Hayat, T. Analysis of radiation in a suspension of nanoparticles and gyrotactic microorganism for rotating disk of variable thickness. Chin. J. Phys. 2018, 56, 2404–2423. [Google Scholar] [CrossRef]
- Aziz, A.; Alsaedi, A.; Muhammad, T.; Hayat, T. Numerical study for heat generation/absorption in flow of nanofluid by a rotating disk. Results Phys. 2018, 8, 785–792. [Google Scholar] [CrossRef]
- Elnaqeeb, T.; Shah, N.A.; Mirza, I.A. Natural convection flows of carbon nanotubes nanofluids with Prabhakar-like thermal transport. Math. Meth. Appl. Sci. 2020, 1–14. [Google Scholar] [CrossRef]
- Uddin, I.; Akhtar, R.; Khan, M.A.R.; Zhiyu, Z.; Islam, S.; Shoaib, M.; Raja, M.A.Z. Numerical treatment for fluidic system of activation energy with non-linear mixed convective and radiative flow of magneto nanomaterials with Navier’s velocity slip. AIP Adv. 2019, 9, 055210. [Google Scholar] [CrossRef]
- Khan, M.I.; Hayat, T.; Alsaedi, A. A modified homogeneous-heterogeneous reactions for MHD stagnation flow with viscous dissipation and Joule heating. Int. J. Heat Mass Transf. 2017, 113, 310–317. [Google Scholar] [CrossRef]
- Khan, N.; A Sajid, M.; Mahmood, T. Heat transfer analysis for magnetohydrodynamics axisymmetric flow between stretching disks in the presence of viscous dissipation and Joule heating. AIP Adv. 2015, 5, 057115. [Google Scholar] [CrossRef]
- Imtiaz, M.; Hayat, T.; Alsaedi, A.; Asghar, S. Slip flow by a variable thickness rotating disk subject to magnetohydrodynamics. Results Phys. 2017, 7, 503–509. [Google Scholar] [CrossRef]
- Doh, D.; Muthtamilselvan, M. Thermophoretic particle deposition on magnetohydrodynamic flow of micropolar fluid due to a rotating disk. Int. J. Mech. Sci. 2017, 130, 350–359. [Google Scholar] [CrossRef]
- Shah, Z.; Dawar, A.; Kumam, P.; Khan, W.; Islam, S. Impact of Nonlinear Thermal Radiation on MHD Nanofluid Thin Film Flow over a Horizontally Rotating Disk. Appl. Sci. 2019, 9, 1533. [Google Scholar] [CrossRef]
- Rasool, G.; Shah, N.A.; El-Zahar, E.R.; Wakif, A. Numerical investigation of EMHD nanofluid flows over a convectively heated riga pattern positioned horizontally in a Darcy-Forchheimer porous medium: Application of passive control strategy and generalized transfer laws. Waves Random Complex Media 2022, 1–20. [Google Scholar] [CrossRef]
- Rostami, M.N.; Dinarvand, S.; Pop, I. Dual solutions for mixed convective stagnation-point flow of an aqueous silica–alumina hybrid nanofluid. Chin. J. Phys. 2018, 56, 2465–2478. [Google Scholar] [CrossRef]
- Ghadikolaei, S.; Hosseinzadeh, K.; Hatami, M.; Ganji, D. MHD boundary layer analysis for micropolar dusty fluid containing Hybrid nanoparticles (Cu-Al2O3) over a porous medium. J. Mol. Liq. 2018, 268, 813–823. [Google Scholar] [CrossRef]
- Qureshi, M.Z.A.; Bilal, S.; Malik, M.Y.; Raza, Q.; Sherif, E.-S.M.; Li, Y.-M. Dispersion of metallic/ceramic matrix nanocomposite material through porous surfaces in magnetized hybrid nanofluids flow with shape and size effects. Sci. Rep. 2021, 11, 12271. [Google Scholar] [CrossRef] [PubMed]
- Raza, Q.; Qureshi, M.Z.A.; Khan, B.A.; Hussein, A.K.; Ali, B.; Shah, N.A.; Chung, J.D. Insight into Dynamic of Mono and Hybrid Nanofluids Subject to Binary Chemical Reaction, Activation Energy, and Magnetic Field through the Porous Surfaces. Mathematics 2022, 10, 3013. [Google Scholar] [CrossRef]
- Raza, Q.; Qureshi, M.Z.A.; Ali, B.; Hussein, A.K.; Khan, B.A.; Shah, N.A.; Weera, W. Morphology of Hybrid MHD Nanofluid Flow through Orthogonal Coaxial Porous Disks. Mathematics 2022, 10, 3280. [Google Scholar] [CrossRef]
- Qureshi, M.Z.; Raza, Q.; Ramzan, A.; Faisal, M.; Ali, B.; Shah, N.A.; Weera, W. Activation Energy Performance through Magnetized Hybrid Fe3O4–PP Nanofluids Flow with Impact of the Cluster Interfacial Nanolayer. Mathematics 2022, 10, 3277. [Google Scholar] [CrossRef]
- Habib, D.; Salamat, N.; Ahsan, M.; Abdal, S.; Siddique, I.; Ali, B. Significance of bioconvection and mass transpiration for MHD micropolar Maxwell nanofluid flow over an extending sheet. Waves Random Complex Media 2022, 1–15. [Google Scholar] [CrossRef]
- Ali, K.; Akbar, M.Z.; Iqbal, M.F.; Ashraf, M. Numerical simulation of heat and mass transfer in unsteady nanofluid between two orthogonally moving porous coaxial disks. AIP Adv. 2014, 4, 107113. [Google Scholar] [CrossRef]
- Ali, K.; Iqbal, M.F.; Akbar, Z.; Ashraf, M. Numerical simulation of unsteady water-based nanofluid flow and heat transfer between two orthogonally moving porous coaxial disks. J. Theor. Appl. Mech. 2014, 52, 1033–1046. [Google Scholar] [CrossRef][Green Version]
- Majdalani, J.; Zhou, C.; Dawson, C.A. Two-dimensional viscous flow between slowly expanding or contracting walls with weak permeability. J. Biomech. 2002, 35, 1399–1403. [Google Scholar] [CrossRef]
- Saba, F.; Ahmed, N.; Khan, U.; Waheed, A.; Rafiq, M.; Mohyud-Din, S.T. Thermophysical Analysis of Water Based (Cu–Al2O3) Hybrid Nanofluid in an Asymmetric Channel with Dilating/Squeezing Walls Considering Different Shapes of Nanoparticles. Appl. Sci. 2018, 8, 1549. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D.D. Nanofluid flow and heat transfer between parallel plates considering Brownian motion using DTM. Comput. Methods Appl. Mech. Eng. 2014, 283, 651–663. [Google Scholar] [CrossRef]
- Hussain, M.F.; Akbar, M.Z. Numerical Analysis of Nanofluids with Convective Heat Transfer through Porous Disks. Casp. J. Comput. Math. Eng. 2017, 2, 5–26. [Google Scholar]
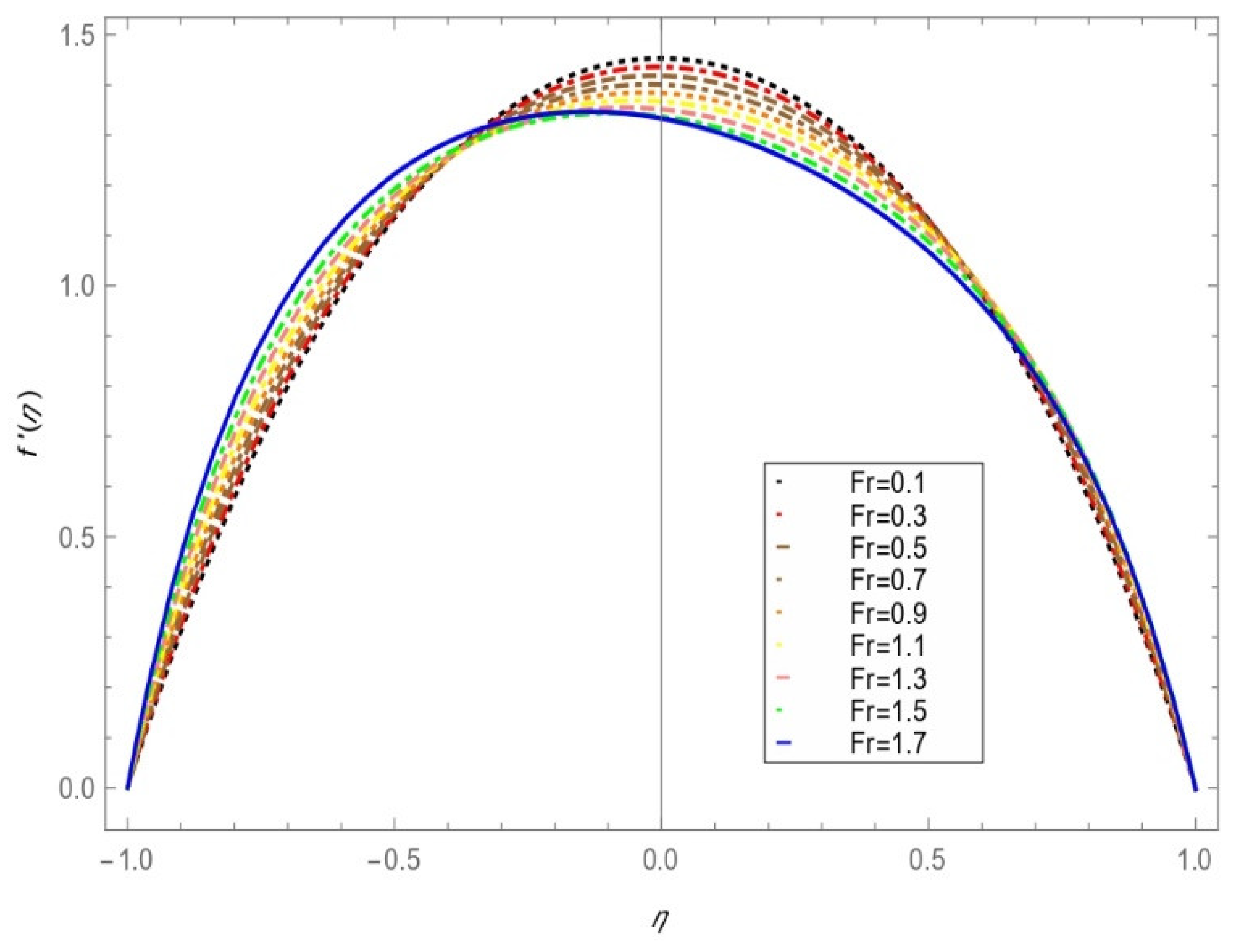
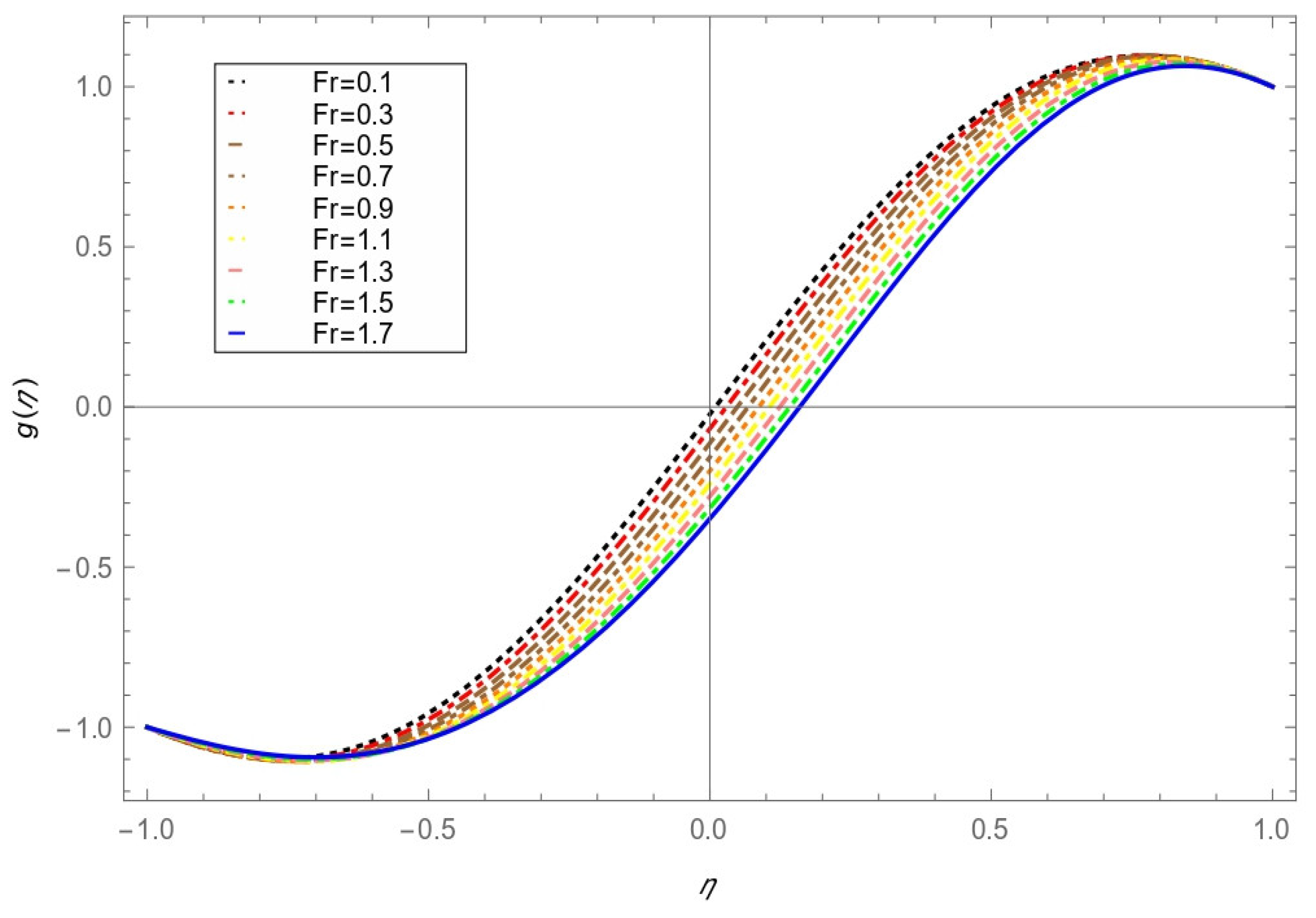
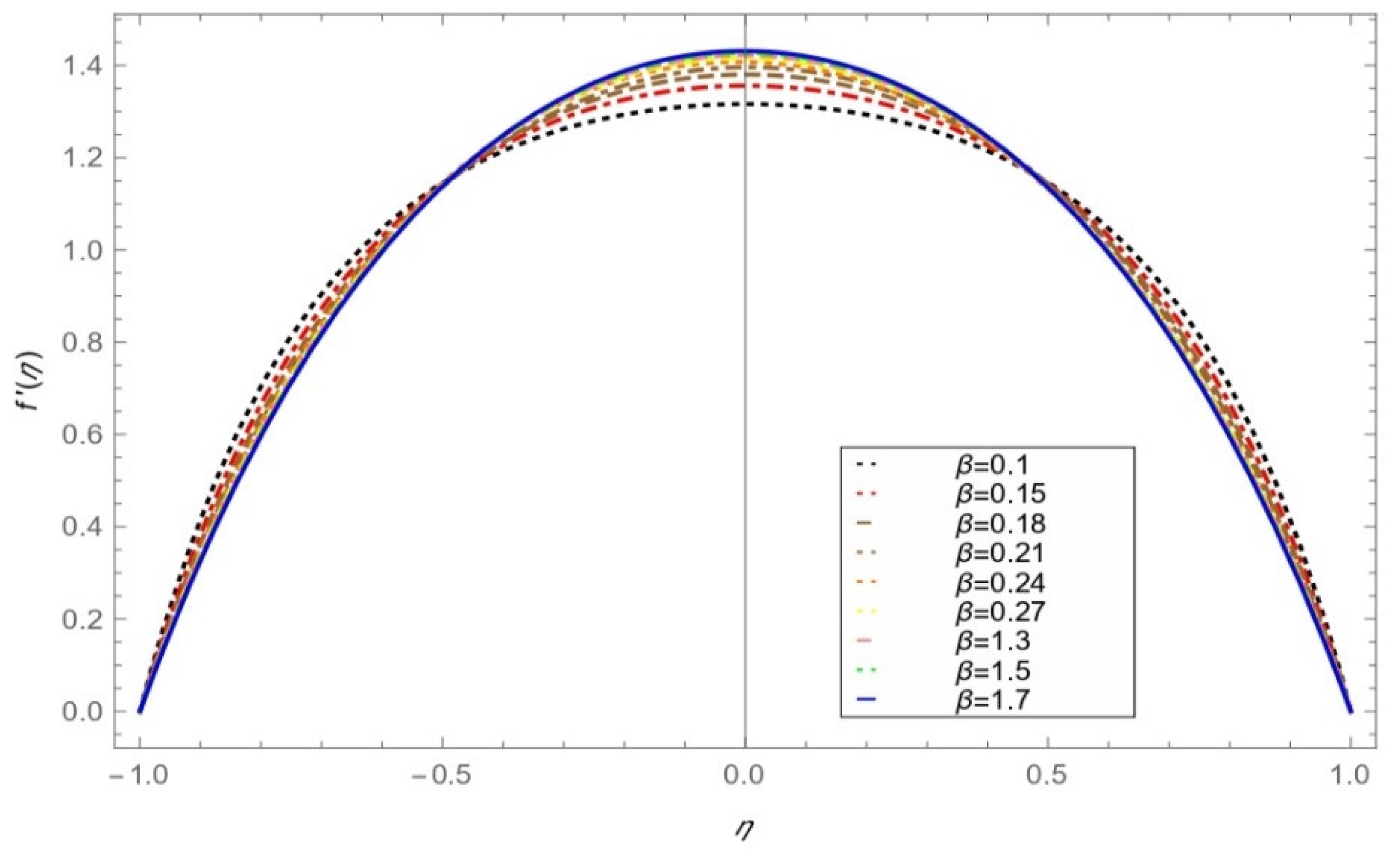
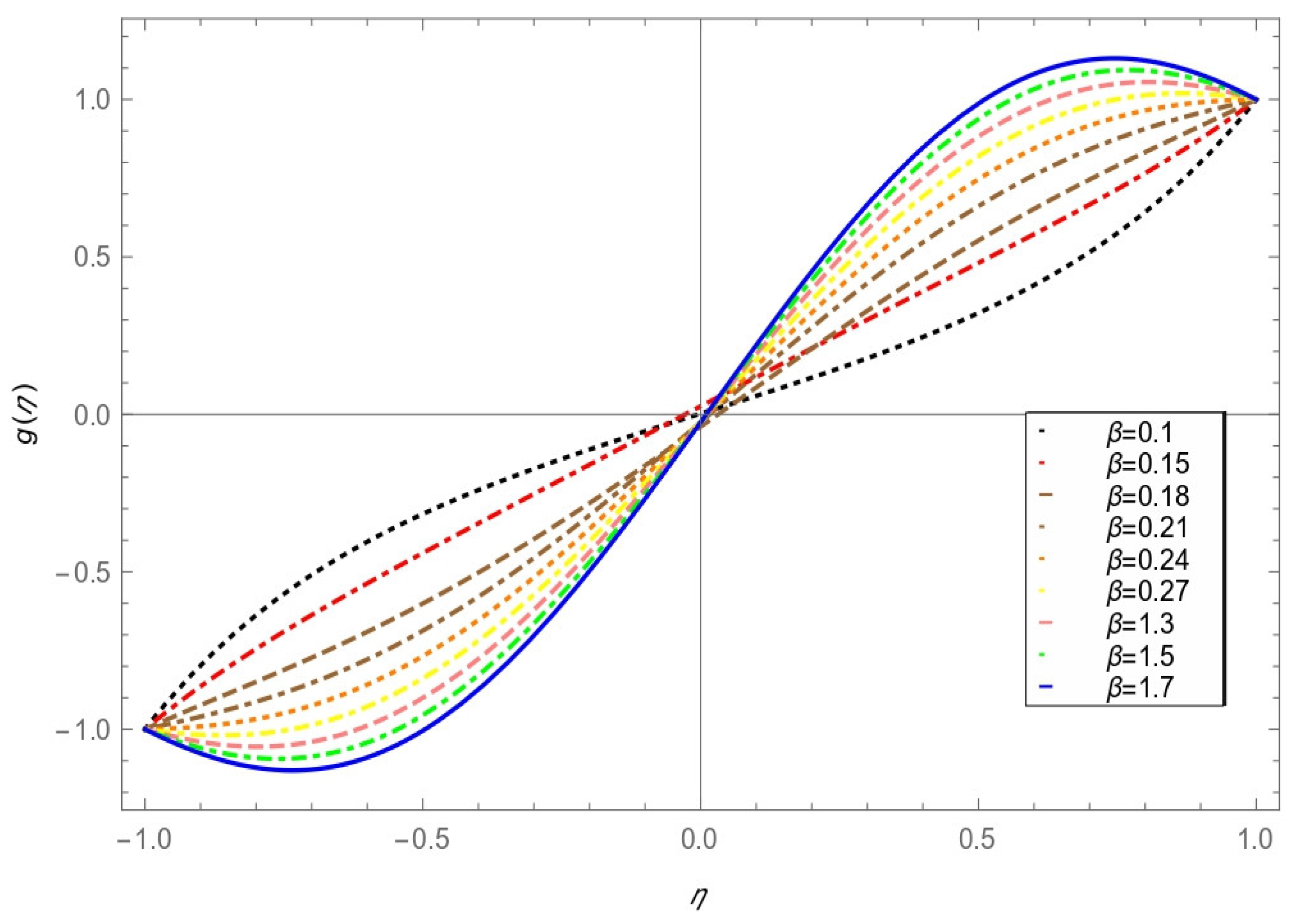


| Properties | (HNFDs) |
|---|---|
| Density () | |
| Viscosity () | +)) |
| ) | |
| Thermal Conductivity (K) | = where = |
| Nanoparticles and Base Fluid | |||
|---|---|---|---|
| H2O | 997.1 | 4179 | 0.613 |
| Ethylene glycol | 1115 | 2430 | 0.253 |
| Engine oil | 884 | 1910 | 0.144 |
| Ag | 10,500 | 235.0 | 429 |
| Cu | 8933 | 385 | 401 |
| 3970 | 765 | 40 | |
| 4250 | 686.2 | 8.9538 |
| (a) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Ethylene glycol | ||||||||||
| 1% | 0.0362 | |||||||||
| 2% | ||||||||||
| 3% | ||||||||||
| 4% | ||||||||||
| Ethylene glycol | ||||||||||
| 1% | ||||||||||
| 2% | ||||||||||
| 3% | ||||||||||
| 4% | ||||||||||
| Engine oil | Engine oil | |||||||||
| 1% | ||||||||||
| 2% | ||||||||||
| 3% | ||||||||||
| 4% | ||||||||||
| (b) | ||||||||||
| Ethylene glycol | ||||||||||
| 1% | ||||||||||
| 2% | ||||||||||
| 3% | ||||||||||
| 4% | ||||||||||
| Ethylene glycol | ||||||||||
| 1% | ||||||||||
| 2% | ||||||||||
| 3% | ||||||||||
| 4% | ||||||||||
| Engine oil | Engine oil | |||||||||
| 1% | ||||||||||
| 2% | ||||||||||
| 3% | 0 | |||||||||
| 4% | ||||||||||
| −0.5 | 1 | 0.3 | 1 | 0.1 | 0.1 | 0.01 | 0.01 | 0.5 | ||
| −1 | ||||||||||
| −1.5 | ||||||||||
| 3 | ||||||||||
| 6 | ||||||||||
| 9 | ||||||||||
| 0.6 | ||||||||||
| 0.9 | ||||||||||
| 1.2 | ||||||||||
| 2 | ||||||||||
| 4 | ||||||||||
| 6 | ||||||||||
| 0.2 | ||||||||||
| 0.3 | ||||||||||
| 0.4 | ||||||||||
| 0.2 | ||||||||||
| 0.3 | 0 | |||||||||
| 0.4 | ||||||||||
| 0.05 | 9 | |||||||||
| 0.1 | ||||||||||
| 0.15 | ||||||||||
| 0.05 | 9 | |||||||||
| 0.1 | ||||||||||
| 0.15 | ||||||||||
| 1 | ||||||||||
| 1.5 | ||||||||||
| 2 |
| 2 | 1.9051 | 0.2206 |
| 4 | 2.5239 | 0.0906 |
| 6 | 2.9857 | 0.0437 |
| 8 | 3.369 | 0.0232 |
| 10 | 3.7172 | 0.0129 |
| −1.5 | 1 | 1 | |
| −1 | |||
| 1 | |||
| 1.5 | |||
| −1 | −2 | ||
| −1 | |||
| 1 | |||
| 2 | |||
| −1 | 2 | ||
| 4 | |||
| 6 | |||
| 8 |
| Hussain and Akbar [53] | Present Results | Hussain and Akbar [53] | Present Results | |
|---|---|---|---|---|
| Ec | α < 0 | α < 0 | α > 0 | α > 0 |
| 0 | 1.2461 | 1.2462 | 1.5675 | 1.5676 |
| 0.3 | 4.7629 | 4.7630 | 5.99363 | 5.99364 |
| 0.5 | 7.9383 | 7.9384 | 9.98939 | 9.98940 |
| 0.8 | 12.701 | 12.702 | 15.9834 | 15.9835 |
| 1.1 | 17.464 | 17.465 | 21.9767 | 21.9768 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alanazi, M.M.; Hendi, A.A.; Raza, Q.; Qureshi, M.Z.A.; Hira, F.S.; Ali, B.; Shah, N.A.; Chung, J.D. Significance of Multi-Hybrid Morphology Nanoparticles on the Dynamics of Water Fluid Subject to Thermal and Viscous Joule Performance. Mathematics 2022, 10, 4259. https://doi.org/10.3390/math10224259
Alanazi MM, Hendi AA, Raza Q, Qureshi MZA, Hira FS, Ali B, Shah NA, Chung JD. Significance of Multi-Hybrid Morphology Nanoparticles on the Dynamics of Water Fluid Subject to Thermal and Viscous Joule Performance. Mathematics. 2022; 10(22):4259. https://doi.org/10.3390/math10224259
Chicago/Turabian StyleAlanazi, Meznah M., Awatif A. Hendi, Qadeer Raza, M. Zubair Akbar Qureshi, Fatima Shafiq Hira, Bagh Ali, Nehad Ali Shah, and Jae Dong Chung. 2022. "Significance of Multi-Hybrid Morphology Nanoparticles on the Dynamics of Water Fluid Subject to Thermal and Viscous Joule Performance" Mathematics 10, no. 22: 4259. https://doi.org/10.3390/math10224259
APA StyleAlanazi, M. M., Hendi, A. A., Raza, Q., Qureshi, M. Z. A., Hira, F. S., Ali, B., Shah, N. A., & Chung, J. D. (2022). Significance of Multi-Hybrid Morphology Nanoparticles on the Dynamics of Water Fluid Subject to Thermal and Viscous Joule Performance. Mathematics, 10(22), 4259. https://doi.org/10.3390/math10224259






