On the Global Behaviour of Solutions for a Delayed Viscoelastic-Type Petrovesky Wave Equation with p-Laplacian Operator and Logarithmic Source
Abstract
:1. Introduction
2. Preliminaries
- (H1)
- The relaxation function is a twice-differentiable and bounded function satisfying andThere exist constants depending on such thatwhenever .
- (H2)
- The exponent p satisfies for and for .
- (H3)
- Assume that l satisfies whenever and whenever .
3. Global Existence and Energy Decay
- (i)
- , ;
- (ii)
- is increasing in the interval , decreasing in the interval and takes its maximum at where ;
- (iii)
- for , for and .
3.1. Global Existence for Low Initial Energy
3.2. General Decay of Global Solution
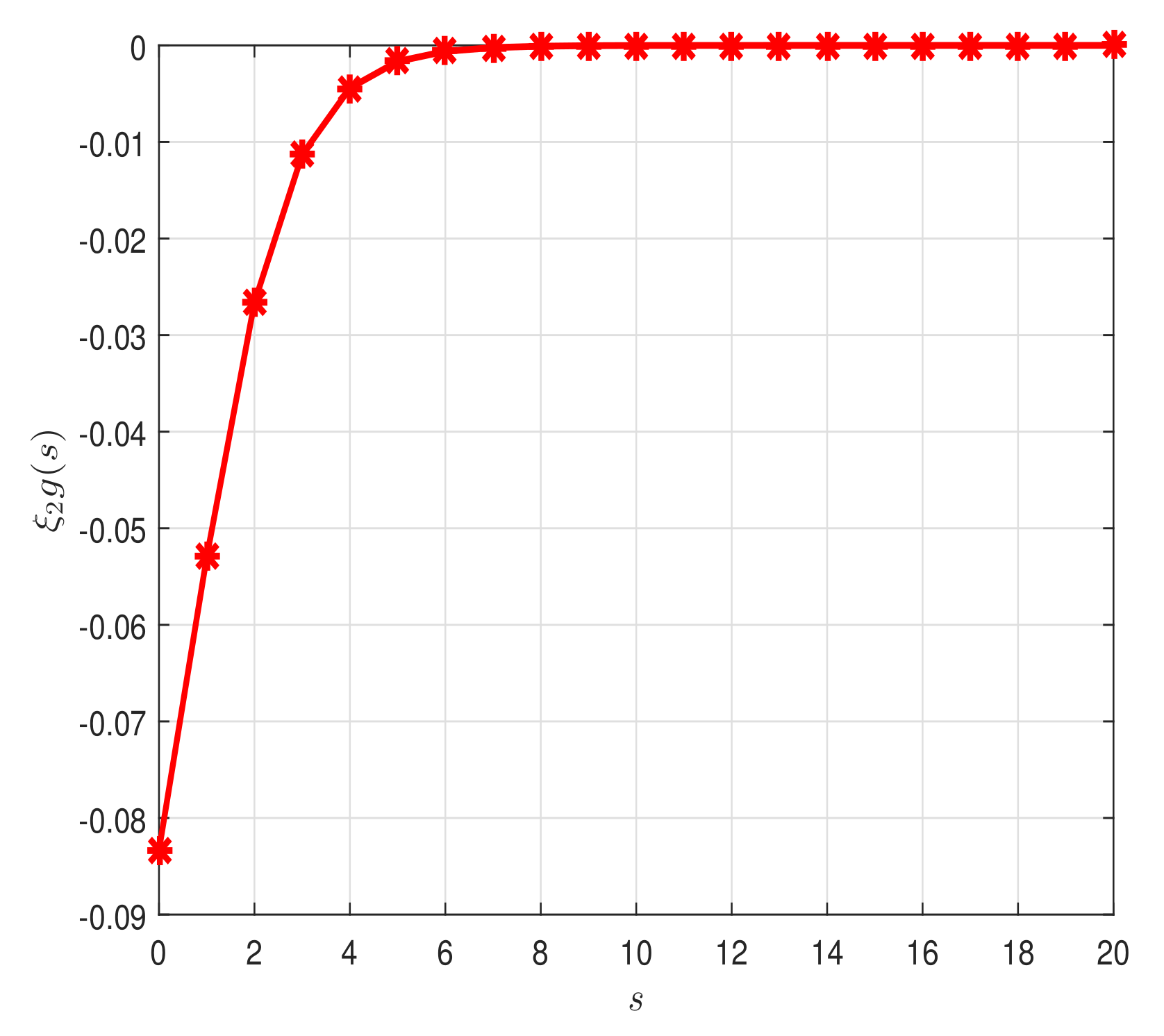
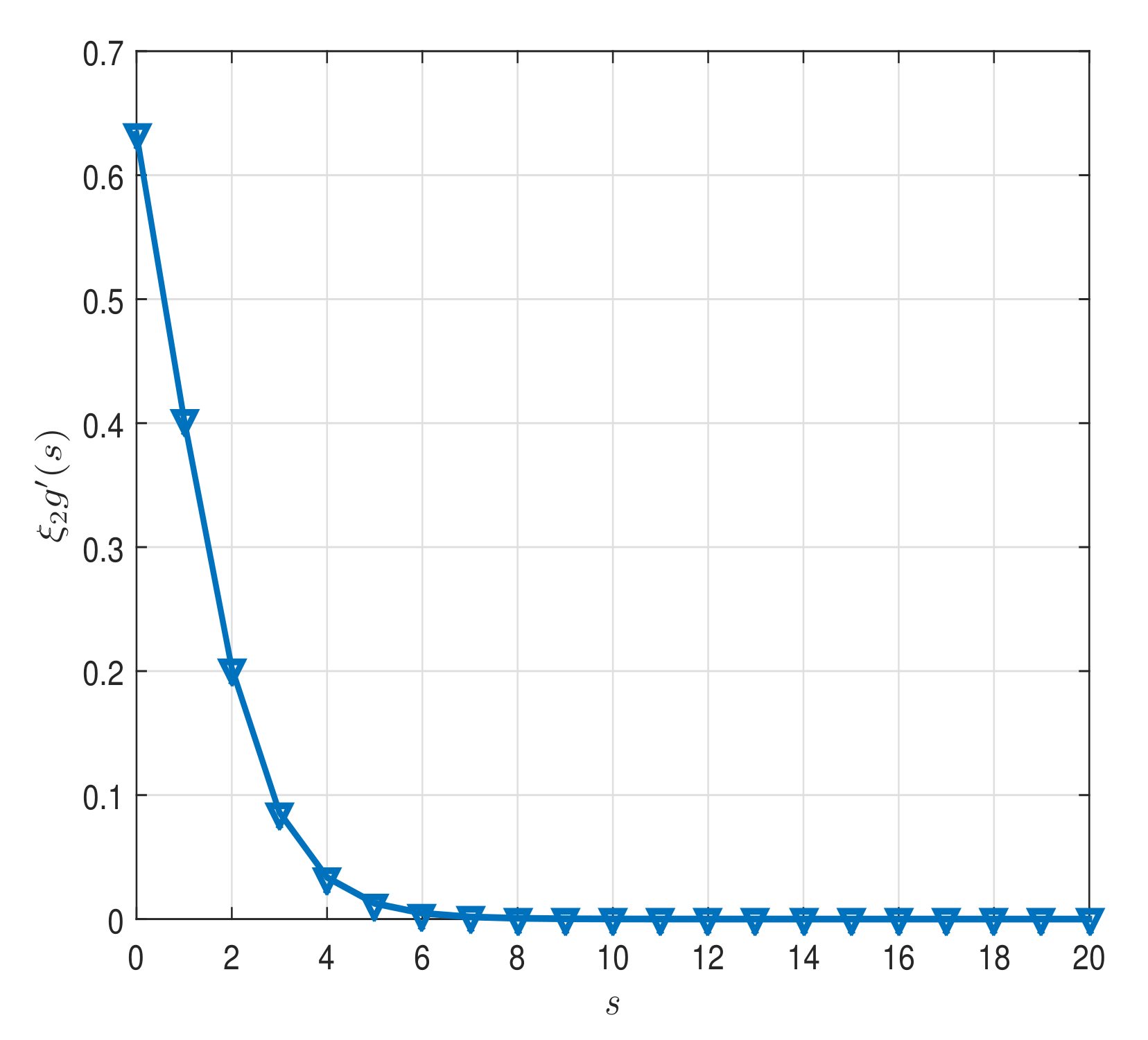
4. An Example and Numerical Study
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Barrow, J.D.; Parsons, P. Inflationary models with logarithmic potentials. Phys. Rev. D 1995, 52, 5576. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Górka, P. Logarithmic Klein–Gordon equation. Acta Phys. Pol. 2009, 40, 3477–3482. [Google Scholar]
- Zhao, K. Global stability of a novel nonlinear diffusion online game addiction model with unsustainable control. AIMS Math. 2022, 7, 20752–20766. [Google Scholar] [CrossRef]
- Sattinger, D.H. On global solution of nonlinear hyperbolic equations. Arch. Ration. Mech. Anal. 1968, 30, 148–172. [Google Scholar] [CrossRef]
- Messaoudi, S.A. Blow up and global existence in a nonlinear viscoelastic wave equation. Math. Nachrichten 2003, 260, 58–66. [Google Scholar] [CrossRef]
- Gazzola, F.; Squassina, M. Global solutions and finite time blow up for damped semilinear wave equations. Ann. Inst. H. Poincaré Anal. Non Linéaire 2006, 23, 185–207. [Google Scholar] [CrossRef]
- Wang, Y.J. A suffiecient condition for finite time blow up of the nonlinear Klein-Gordon equations with arbitrarily positive initial energy. Proc. Am. Math. Soc. 2008, 136, 3477–3482. [Google Scholar] [CrossRef]
- Wu, Y.; Xue, X. Uniform decay rate estimates for a class of quasilinear hyperbolic equations with nonlinear damping and source terms. Appl. Anal. 2013, 92, 1169–1178. [Google Scholar] [CrossRef]
- Pişkin, E.; Boulaaras, S.; Irkil, N. Qualitative analysis of solutions for the p-Laplacian hyperbolic equation with logarithmic nonlinearity. Math. Methods Appl. Sci. 2021, 44, 4654–4672. [Google Scholar] [CrossRef]
- Ma, L.; Fang, Z.B. Eenrgy decay estimates and infinite blow-up phenomena for a strongly damped semilinear wave equation with logarithmic nonlinear source. Math. Methods Appl. Sci. 2018, 41, 2639–2653. [Google Scholar] [CrossRef]
- Di, H.; Shang, Y.; Song, Z. Initial boundary value problems for a class of strongly damped semilinear wave equations with logarithmic nonlinearity. Nonlinear Anal. Real World Appl. 2020, 51, 102968. [Google Scholar] [CrossRef]
- Ha, T.G.; Park, S.H. Blow-up phenomena for a viscoelastic wave equation with strong damping and logarithmic nonlinearity. Adv. Differ. Equ. 2020, 2020, 1–17. [Google Scholar] [CrossRef]
- Menglan, L. The Lifespan of Solutions for a Viscoelastic Wave Equation with a Strong Damping and Logarithmic Nonlinearity; Evolution Equations & Control Theory: Paris, France, 2021. [Google Scholar]
- Zang, A.; Fu, Y. Interpolation inequalities for derivatives in variable exponent Lebesgue–Sobolev spaces. Nonlinear Anal. 2008, 269, 3629–3636. [Google Scholar] [CrossRef]
- Adams, R.A. Sobolev Spaces. In Pure and Applied Mathematics; Academic Press: Cambridge, MA, USA, 1975; p. 65. [Google Scholar]
- Boulaaras, S. Existence of positive solutions for a new class of Kirchhoff parabolic systems. Rocky Mt. J. Math. 2020, 50, 445–454. [Google Scholar] [CrossRef]
- Nakao, M. Asymptotic stability of the bounded or almost periodic solution of the wave equation with nonlinear dissipation term. J. Math. Anal. Appl. 1977, 58, 336–343. [Google Scholar] [CrossRef] [Green Version]
- Datko, R.; Lagnese, J.; Polis, M.P. An example on the effect of time delays in boundary feedback stabilization of wave equations. SIAM J. Control Optim. 1986, 24, 152–156. [Google Scholar] [CrossRef]
- Kafini, M.; Messaoudi, S. Local existence and blow up of solutions to a logarithmic non linear wave equation with delay. Appl. Anal. 2019, 99, 530–547. [Google Scholar]
- Simon, J. Compact sets in the space Lp(O, T, B). Ann. Mat. Pura Ed. Appl. 1986, 146, 65–96. [Google Scholar] [CrossRef]
- Lions, J.L. Quelques Méthodes de Résolution des Problémes aux Limites non Linéaires; Dunod: Paris, France, 1969. [Google Scholar]
- Cao, Y.; Liu, C. Initial boundary value problem for a mixed pseudo-parabolic p-Laplacian type equation with logarithmic nonlinearity. Electron. J. Differ. Equ. 2018, 2018, 1–19. [Google Scholar]
- Nakao, M. A difference inequality and its application to nonlinear evolution equations. J. Math. Soc. Jpn. 1978, 30, 747–762. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Belhadji, B.; Alzabut, J.; Samei, M.E.; Fatima, N. On the Global Behaviour of Solutions for a Delayed Viscoelastic-Type Petrovesky Wave Equation with p-Laplacian Operator and Logarithmic Source. Mathematics 2022, 10, 4194. https://doi.org/10.3390/math10224194
Belhadji B, Alzabut J, Samei ME, Fatima N. On the Global Behaviour of Solutions for a Delayed Viscoelastic-Type Petrovesky Wave Equation with p-Laplacian Operator and Logarithmic Source. Mathematics. 2022; 10(22):4194. https://doi.org/10.3390/math10224194
Chicago/Turabian StyleBelhadji, Bochra, Jehad Alzabut, Mohammad Esmael Samei, and Nahid Fatima. 2022. "On the Global Behaviour of Solutions for a Delayed Viscoelastic-Type Petrovesky Wave Equation with p-Laplacian Operator and Logarithmic Source" Mathematics 10, no. 22: 4194. https://doi.org/10.3390/math10224194
APA StyleBelhadji, B., Alzabut, J., Samei, M. E., & Fatima, N. (2022). On the Global Behaviour of Solutions for a Delayed Viscoelastic-Type Petrovesky Wave Equation with p-Laplacian Operator and Logarithmic Source. Mathematics, 10(22), 4194. https://doi.org/10.3390/math10224194








