Boundary Coupling for Consensus of Nonlinear Leaderless Stochastic Multi-Agent Systems Based on PDE-ODEs
Abstract
1. Introduction
2. Consensus of PDE-ODEs Based MASs under the Collocated Boundary Measurement Form
3. Consensus of PDE-ODEs Based MASs under the Distributed Boundary Measurement Form
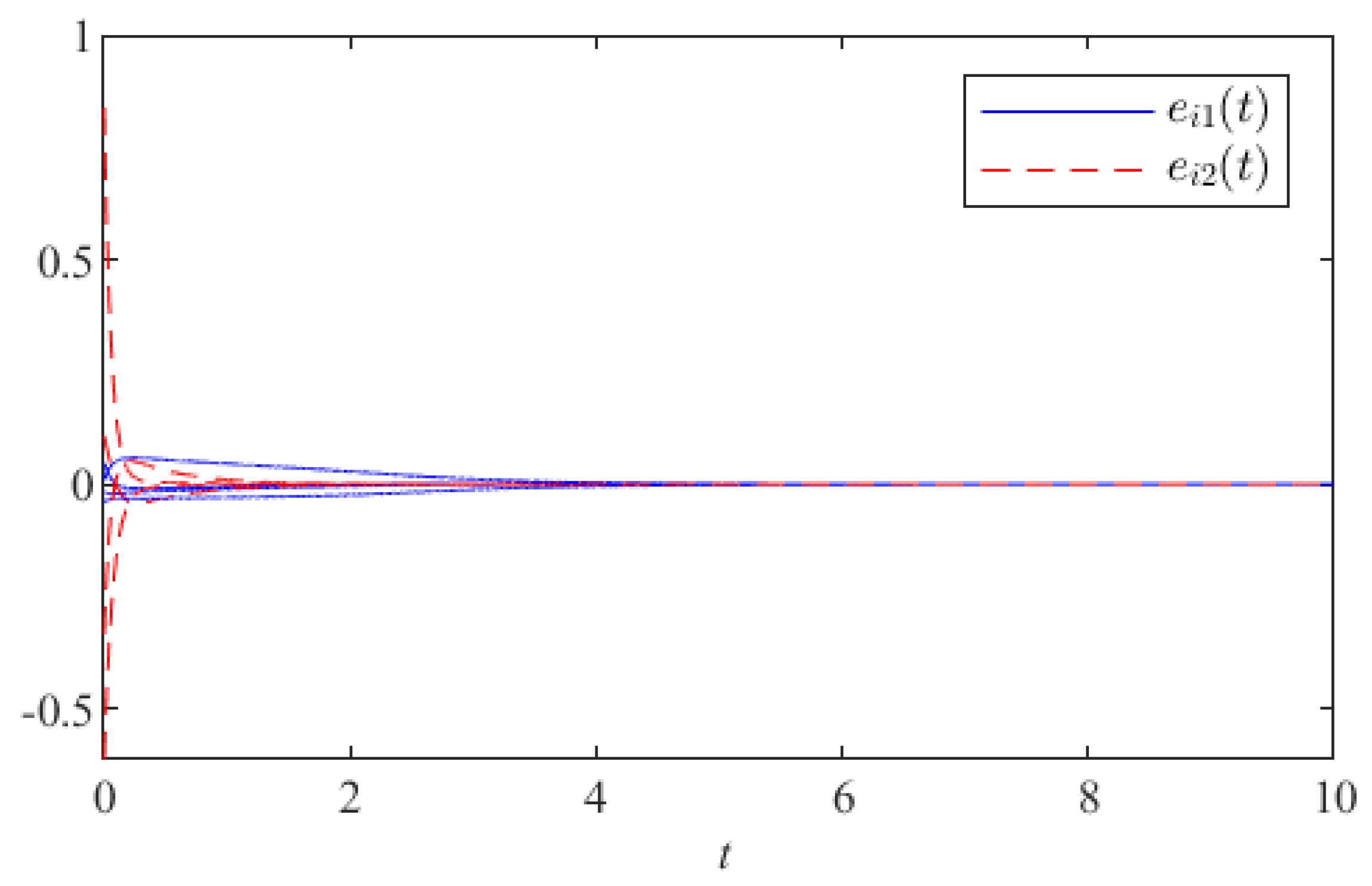
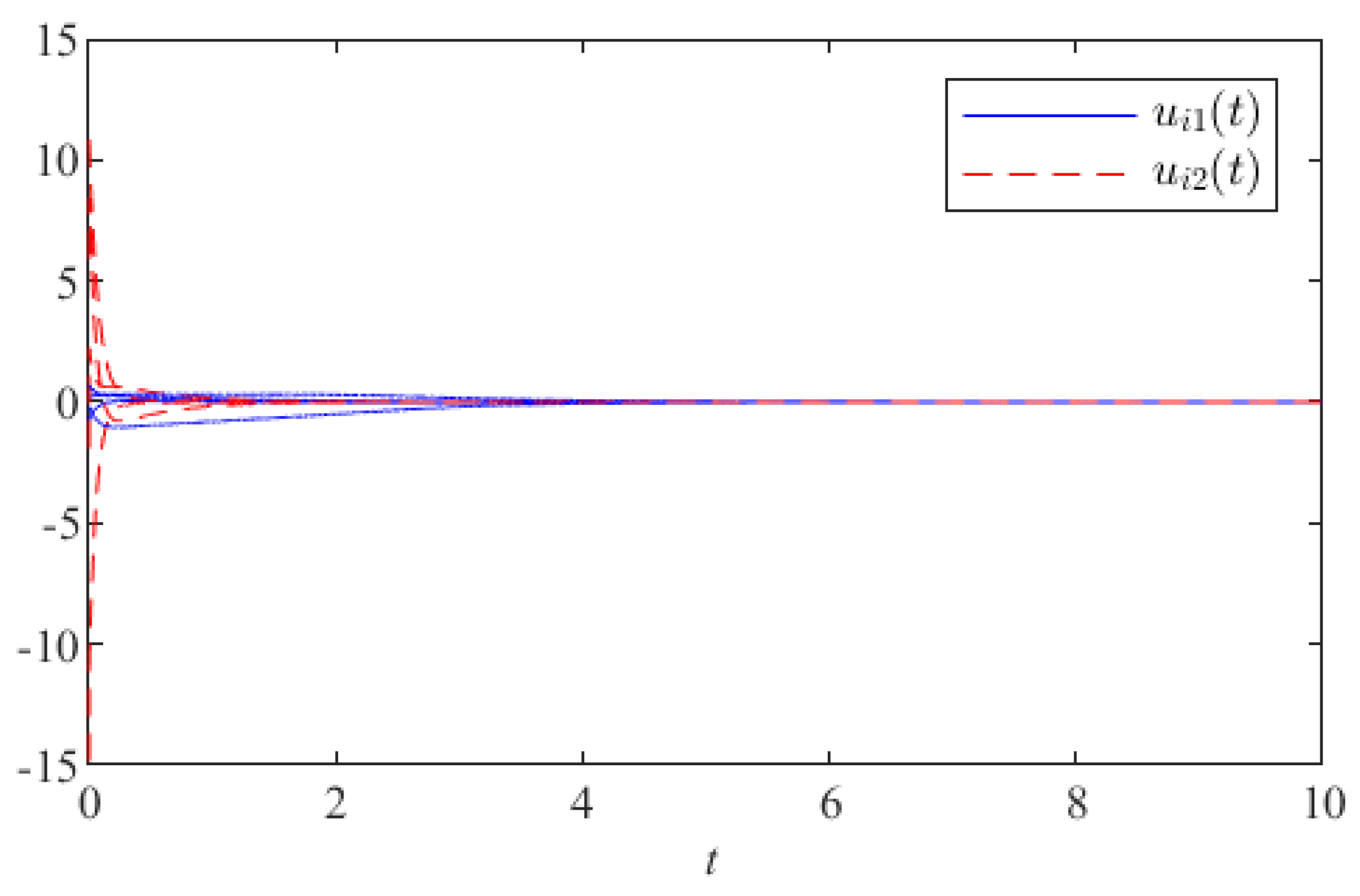
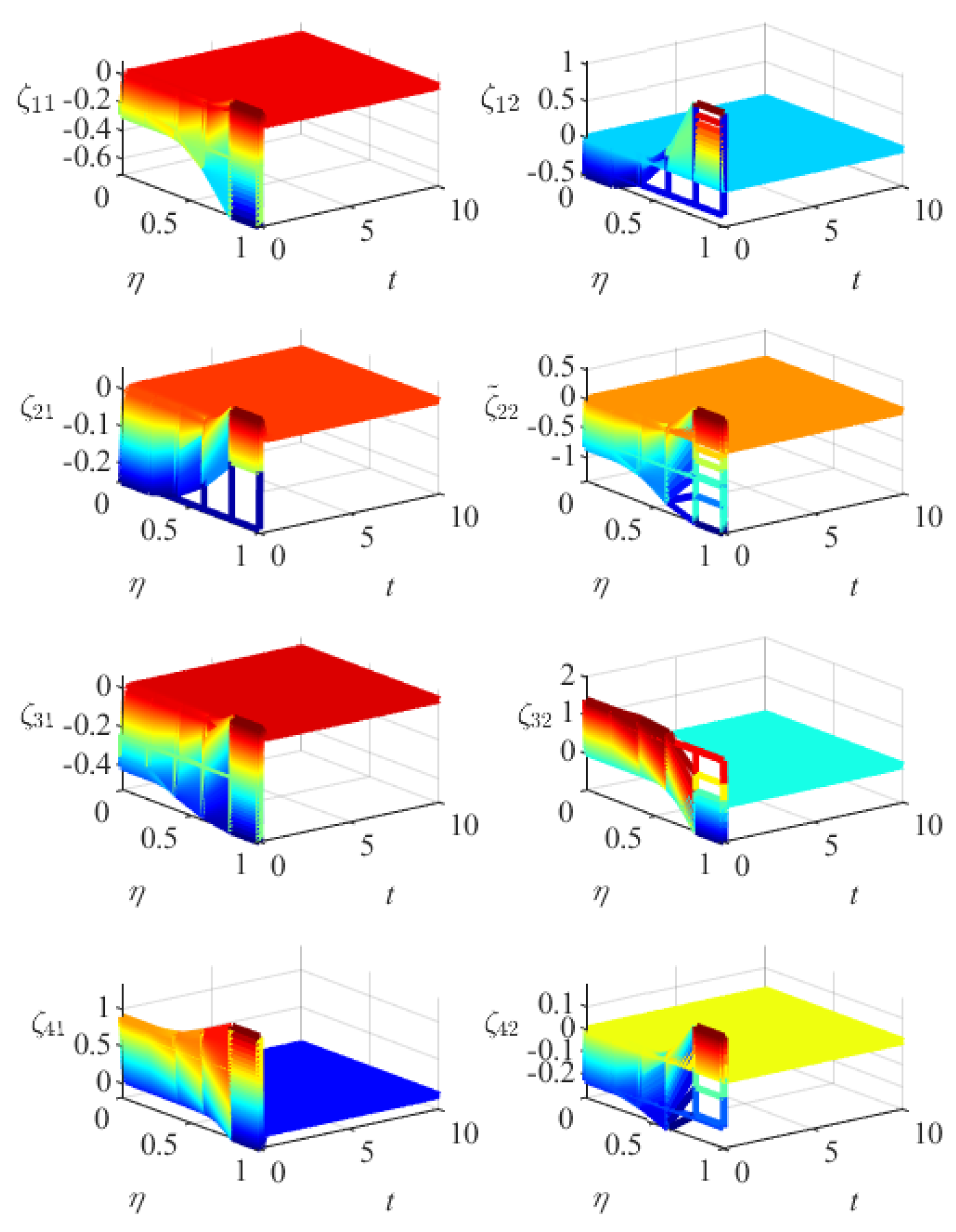
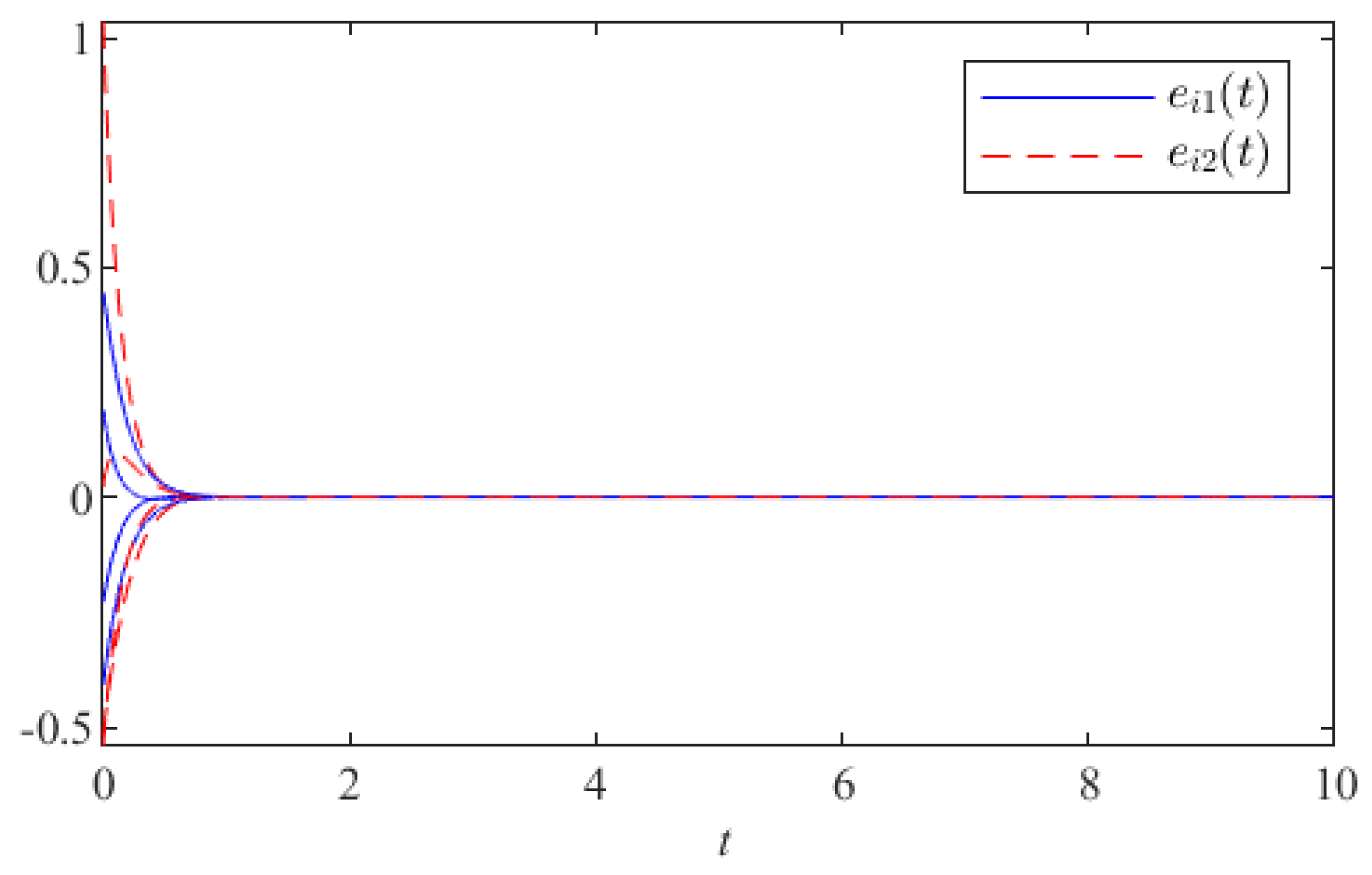
4. Simulation Examples
5. Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lu, M.; Wu, J.; Zhan, X.; Han, T.; Yan, H. Consensus of second-order hetero-geneous multi-agent systems with and without input saturation. ISA Trans. 2022, 126, 14–20. [Google Scholar] [CrossRef] [PubMed]
- Cao, X.; Zhang, C.; Zhao, D.; Sun, B.; Li, Y. Event-triggered consensus control of continuous-time stochastic multi-agent systems. Automatica 2022, 137, 110022. [Google Scholar] [CrossRef]
- Dong, G.; Li, H.; Ma, H.; Lu, R. Finite-time consensus tracking neural network FTC of multi-agent systems. IEEE Trans. Neural Netw. Learn. Syst. 2020, 32, 653–662. [Google Scholar] [CrossRef]
- Li, X.; Tang, Y.; Karimi, H. Consensus of multi-agent systems via fully dis-tributed event-triggered control. Automatica 2020, 116, 108898. [Google Scholar] [CrossRef]
- Ruch, C.; Gächter, J.; Hakenberg, J.; Frazzoli, E. The+ 1 method: Model-free adaptive repositioning policies for robotic multi-agent systems. IEEE Trans. Netw. Sci. Eng. 2020, 7, 3171–3184. [Google Scholar] [CrossRef]
- Wang, X.; Weiming, L.; Li, S. A modular optimal formation control scheme of multi-agent systems with application to multiple mobile robots. IEEE Trans. Ind. Electron. 2021, 69, 9331–9341. [Google Scholar] [CrossRef]
- Yang, Y.; Liu, F.; Yang, H.; Li, Y.; Liu, Y. Distributed finite-time integral sliding-mode control for multi-agent systems with multiple disturbances based on nonlinear disturbance observers. J. Syst. Sci. Complex. 2021, 34, 995–1013. [Google Scholar] [CrossRef]
- Liu, T.; Jiang, Z. Distributed control of multi-agent systems with pulse-width-modulated controllers. Automatica 2020, 119, 109020. [Google Scholar] [CrossRef]
- Li, R.; Yang, G. Distributed optimization for a class of uncertain MIMO non-linear multi-agent systems with arbitrary relative degree. Inf. Sci. 2020, 506, 58–77. [Google Scholar] [CrossRef]
- Sigler, T.; Martinus, K.; Iacopini, I.; Derudder, B.; Loginova, J. The struc-tural architecture of international industry networks in the global economy. PLoS ONE 2021, 16, e0255450. [Google Scholar] [CrossRef]
- Gao, C.; Wang, Z.; He, X.; Dong, H. Encryption–decryption-based consensus control for multi-agent systems: Handling actuator faults. Automatica 2021, 134, 109908. [Google Scholar] [CrossRef]
- Zhou, H.; Liu, Z.; Chu, D.; Li, W. Sampled-data synchronization of complex network based on periodic self-triggered intermittent control and its application to image encryption. Neural Netw. 2022, 152, 419–433. [Google Scholar] [CrossRef] [PubMed]
- Qian, Y.; Liu, L.; Feng, G. Output consensus of heterogeneous linear multi-agent systems with adaptive event-triggered control. IEEE Trans. Autom. Control 2018, 64, 2606–2613. [Google Scholar] [CrossRef]
- Yang, R.; Zhang, H.; Feng, G.; Yan, H.; Wang, Z. Robust cooperative output regulation of multi-agent systems via adaptive event-triggered control. Automatica 2019, 102, 129–136. [Google Scholar] [CrossRef]
- Zhao, C.; Liu, X.; Zhong, S.; Shi, K.; Liao, D.; Zhong, Q. Secure consensus of multi-agent systems with redundant signal and communication interference via distributed dynamic event-triggered control. ISA Trans. 2021, 112, 89–98. [Google Scholar] [CrossRef] [PubMed]
- He, W.; Gao, X.; Zhong, W.; Qian, F. Secure impulsive synchronization control of multi-agent systems under deception attacks. Inf. Sci. 2018, 459, 354–368. [Google Scholar] [CrossRef]
- Yang, C.; Cao, J.; Huang, T.; Zhang, J.; Qiu, J. Guaranteed cost boundary control for cluster synchronization of complex spatio-temporal dynamical networks with community structure. Sci. China Inf. Sci. 2018, 61, 1–13. [Google Scholar] [CrossRef]
- Luo, S.; Ye, D. Cluster Consensus Control of Linear Multiagent Systems under Directed Topology with General Partition. IEEE Trans. Autom. Control 2021, 67, 1929–1936. [Google Scholar] [CrossRef]
- Yang, C.; Yang, Y.; Yang, C.; Zhu, J.; Zhang, A.; Qiu, J. Adaptive control for synchronization of semi-linear complex spatio-temporal networks with time-invariant coupling delay and time-variant coupling delay. Int. J. Adapt. Control Signal Process. 2022, 36, 2640–2659. [Google Scholar] [CrossRef]
- Fu, D.; Zhao, X.; Yuan, H. Nonsingular terminal sliding mode control based on adaptive time delay estimation for permanent magnet linear synchronous motor. Int. J. Control Autom. Syst. 2022, 20, 24–34. [Google Scholar] [CrossRef]
- Xin, Y.; Cheng, Z. Adaptive Synchronization for Delayed Chaotic Memristor-Based Neural Networks. IEEE Trans. Neural Netw. Learn. Syst. 2021. [Google Scholar] [CrossRef]
- Yuan, M.; Zhai, J. Adaptive event-triggered output feedback control for a class of switched stochastic nonlinear systems with unknown output gain. Int. J. Control Autom. Syst. 2022, 20, 109–116. [Google Scholar] [CrossRef]
- Wang, J.; Jiang, H.; Hu, C.; Ma, T. Exponential passivity of discrete-time switched neural networks with transmission delays via an event-triggered sliding mode control. Neural Netw. 2021, 143, 271–282. [Google Scholar] [CrossRef]
- Wang, Z.; Zhu, Y.; Xue, H.; Liang, H. Neural networks-based adaptive event-triggered consensus control for a class of multi-agent systems with communication faults. Neurocomputing 2022, 470, 99–108. [Google Scholar] [CrossRef]
- Xu, J.; Huang, J. An Overview of Recent Advances in the Event-Triggered Consensus of Multi-Agent Systems with Actuator Saturations. Mathematics 2022, 10, 3879. [Google Scholar] [CrossRef]
- Botmart, T.; Weera, W.; Hongsri, A.; Yotha, N.; Niamsup, P. Dissipative pinning sampled-data control for function projective synchronization of neural networks with hybrid cou-plings and time-varying delays. IEEE Access 2022, 10, 58467–58666. [Google Scholar] [CrossRef]
- Yang, X.; Song, Q.; Cao, J.; Lu, J. Synchronization of coupled Mar-kovian reaction–diffusion neural networks with proportional delays via quantized control. IEEE Trans. Neural Netw. Learn. Syst. 2018, 30, 951–958. [Google Scholar] [CrossRef]
- Wang, Y.; Cao, J.; Lu, B.; Cheng, Z. Global asymptotic consensus of multi-agent internet congestion control system. Neurocomputing 2021, 446, 50–64. [Google Scholar] [CrossRef]
- Zou, W.; Shi, P.; Xiang, Z.; Shi, Y. Consensus Tracking Control of Switched Stochastic Nonlinear Multiagent Systems via Event-Triggered Strategy. IEEE Trans. Neural Netw. Learn. Syst. 2020, 31, 1036–1045. [Google Scholar] [CrossRef]
- Cao, Y.; Li, B.; Wen, S.; Huang, T. Consensus tracking of stochastic mul-ti-agent system with actuator faults and switching topologies. Inf. Sci. 2022, 607, 921–930. [Google Scholar]
- Yang, C.; Yang, C.; Hu, C.; Qiu, J.; Cao, J. Two boundary coupling approaches for synchronization of stochastic reaction-diffusion neural networks based on semi-linear PIDEs. J. Frankl. Inst. 2022. [Google Scholar] [CrossRef]
- Yang, C.; Huang, T.; Zhang, A.; Qiu, J.; Cao, J.; Alsaadi, F. Output consensus of multiagent systems based on PDEs with input constraint: A boundary control approach. IEEE Trans. Syst. Man Cybern. Syst. 2018, 51, 370–377. [Google Scholar] [CrossRef]
- Wang, Z.; Sun, H.; Zhang, H.; Liu, X. Bounded consensus control for stochastic multi-agent systems with additive noises. Neurocomputing 2020, 408, 72–79. [Google Scholar] [CrossRef]
- Qi, Q.; Yang, X.; Xu, Z.; Zhang, M.; Huang, T. Novel LKF Method on H∞ Syn-chronization of Switched Time-Delay Systems. IEEE Trans. Cybern. 2022. [Google Scholar] [CrossRef]
- Zou, W.; Guo, J.; Ahn, C.; Xiang, Z. Sampled-Data Consensus Protocols for a Class of Second-Order Switched Nonlinear Multiagent Systems. IEEE Trans. Cybern. 2022. [Google Scholar] [CrossRef]
- Li, S.; Ahn, C.; Guo, J.; Xiang, Z. Global output feedback sampled-data stabilization of a class of switched nonlinear systems in the p-normal form. IEEE Trans. Syst. Man Cybern. Syst. 2019, 51, 1075–1084. [Google Scholar] [CrossRef]
- Li, S.; Ahn, C.; Xiang, Z. Command-Filter-Based Adaptive Fuzzy Finite-Time Control for Switched Nonlinear Systems Using State-Dependent Switching Method. IEEE Trans. Fuzzy Syst. 2021, 29, 833–845. [Google Scholar] [CrossRef]
- Yang, X.; Li, X.; Lu, J.; Cheng, Z. Synchronization of time-delayed complex networks with switching topology via hybrid actuator fault and impulsive effects control. IEEE Trans. Cybern. 2019, 50, 4043–4052. [Google Scholar] [CrossRef]
- Wu, K.; Liu, X.; Shi, P.; Lim, C. Boundary control of linear stochastic reac-tion-diffusion systems. Int. J. Robust Nonlinear Control 2019, 29, 268–282. [Google Scholar] [CrossRef]
- Ni, X.; Yi, K.; Jiang, Y.; Zhang, A.; Yang, C. Consensus control of leaderless and leader-following coupled PDE-ODEs modeled multi-agent systems. Mathematics 2022, 10, 201. [Google Scholar] [CrossRef]
- Seuret, A.; Gouaisbaut, F. Jensen’s and Wirtinger’s inequalities for time-delay systems. IFAC Proc. Vol. 2013, 46, 343–348. [Google Scholar] [CrossRef]
- Qin, J.; Gao, H.; Zheng, W. Exponential synchronization of complex net-works of linear systems and nonlinear oscillators: A unified analysis. IEEE Trans. Neural Netw. Learn. Syst. 2014, 26, 510–521. [Google Scholar] [CrossRef] [PubMed]
- Cont, R.; Fournie, D. A functional extension of the Ito formula. Comptes Rendus Math. 2010, 348, 57–61. [Google Scholar] [CrossRef]
- Lei, Y.; Liu, X.; Xie, C. Stabilization of an ODE-PDE cascaded system by boundary control. J. Frankl. Inst. 2020, 357, 9248–9267. [Google Scholar] [CrossRef]
- Liu, J. Sliding mode control to stabilization of an ODE-Schrödinger cascade sys-tems subject to boundary control matched disturbance. J. Syst. Sci. Complex. 2018, 31, 1146–1163. [Google Scholar] [CrossRef]
- Gong, W.; Mateos, M.; Singler, J.; Zhang, Y. Analysis and approximations of Dirichlet boundary control of Stokes flows in the energy space. SIAM J. Numer. Anal. 2022, 60, 450–474. [Google Scholar] [CrossRef]
- Yang, C.; He, H.; Huang, T.; Zhang, A.; Qiu, J.; Cao, J.; Li, X. Consensus for non-linear multi-agent systems modelled by PDEs based on spatial boundary communication. IET Control Theory Appl. 2017, 11, 3196–3200. [Google Scholar] [CrossRef]
- Chentouf, B.; Mansouri, S. On the exponential stabilization of a flexible structure with dynamic delayed boundary conditions via one boundary control only. J. Frankl. Inst. 2021, 358, 934–962. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, C.; Wang, J.; Miao, S.; Zhao, B.; Jian, M.; Yang, C. Boundary Coupling for Consensus of Nonlinear Leaderless Stochastic Multi-Agent Systems Based on PDE-ODEs. Mathematics 2022, 10, 4111. https://doi.org/10.3390/math10214111
Yang C, Wang J, Miao S, Zhao B, Jian M, Yang C. Boundary Coupling for Consensus of Nonlinear Leaderless Stochastic Multi-Agent Systems Based on PDE-ODEs. Mathematics. 2022; 10(21):4111. https://doi.org/10.3390/math10214111
Chicago/Turabian StyleYang, Chuanhai, Jin Wang, Shengfa Miao, Bin Zhao, Muwei Jian, and Chengdong Yang. 2022. "Boundary Coupling for Consensus of Nonlinear Leaderless Stochastic Multi-Agent Systems Based on PDE-ODEs" Mathematics 10, no. 21: 4111. https://doi.org/10.3390/math10214111
APA StyleYang, C., Wang, J., Miao, S., Zhao, B., Jian, M., & Yang, C. (2022). Boundary Coupling for Consensus of Nonlinear Leaderless Stochastic Multi-Agent Systems Based on PDE-ODEs. Mathematics, 10(21), 4111. https://doi.org/10.3390/math10214111






