Effects of the Nonlocal Thermoelastic Model in a Thermoelastic Nanoscale Material
Abstract
:1. Introduction
2. Basic Equations
3. Initial and Boundary Conditions
4. Laplace Transforms
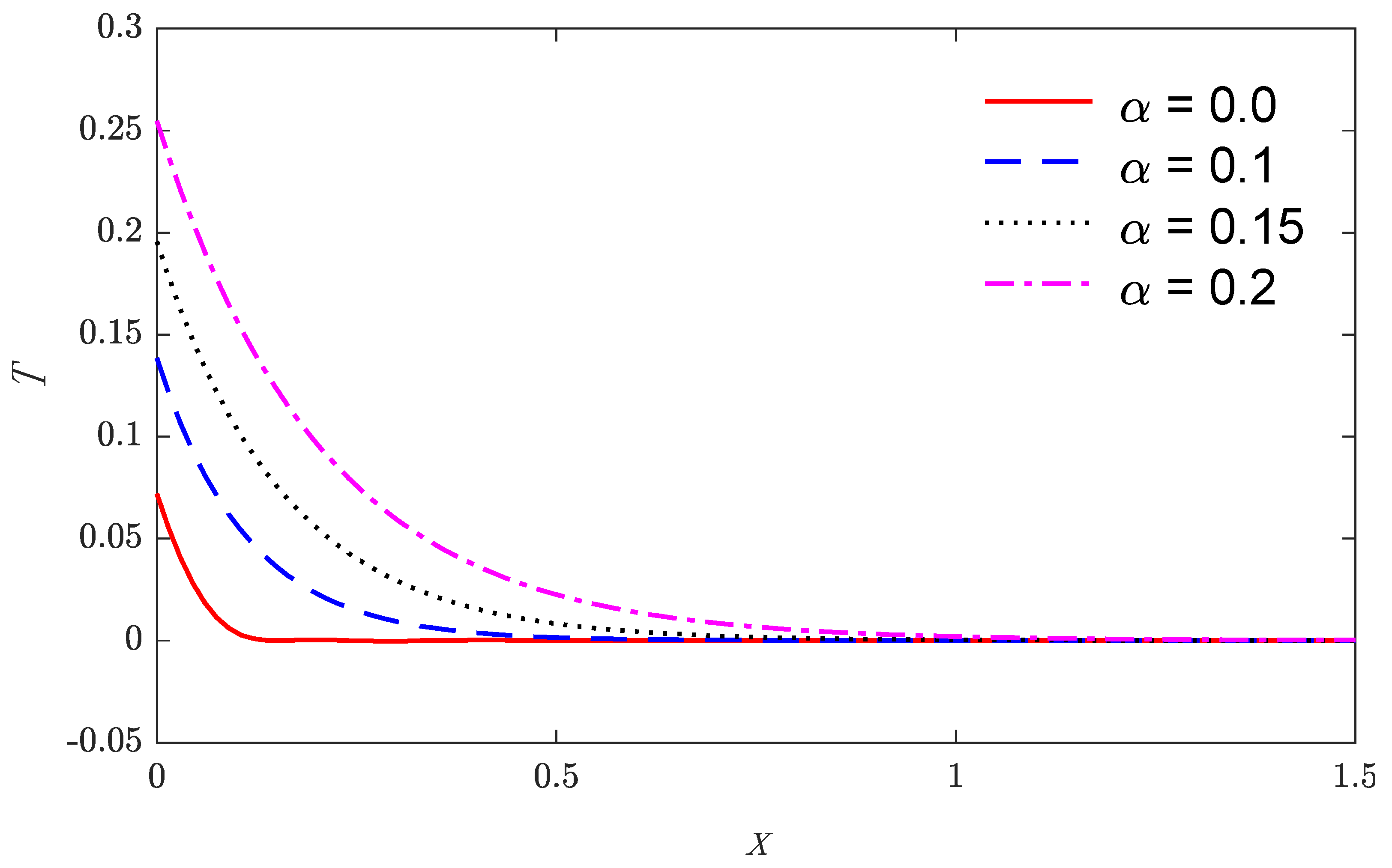
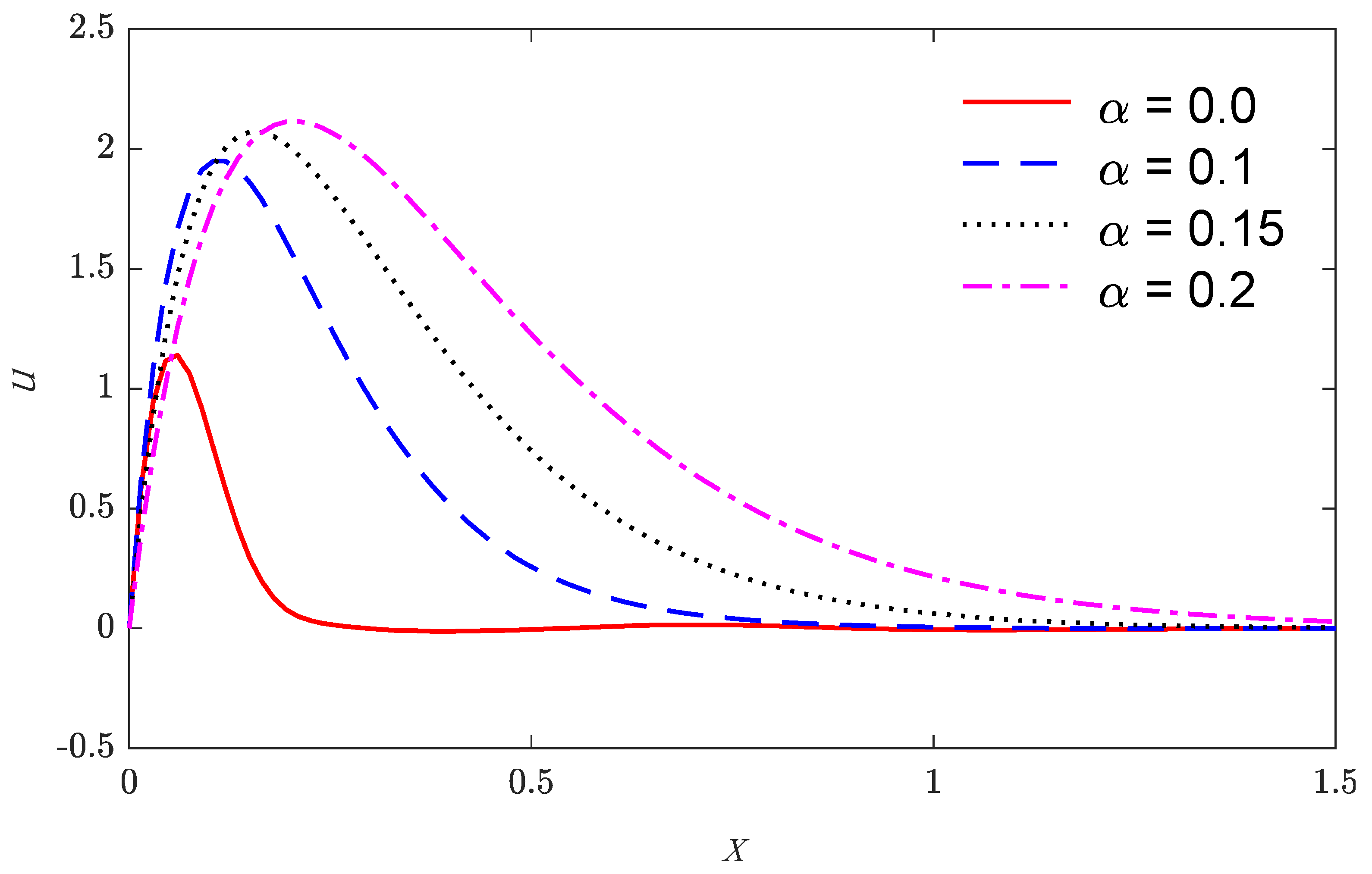
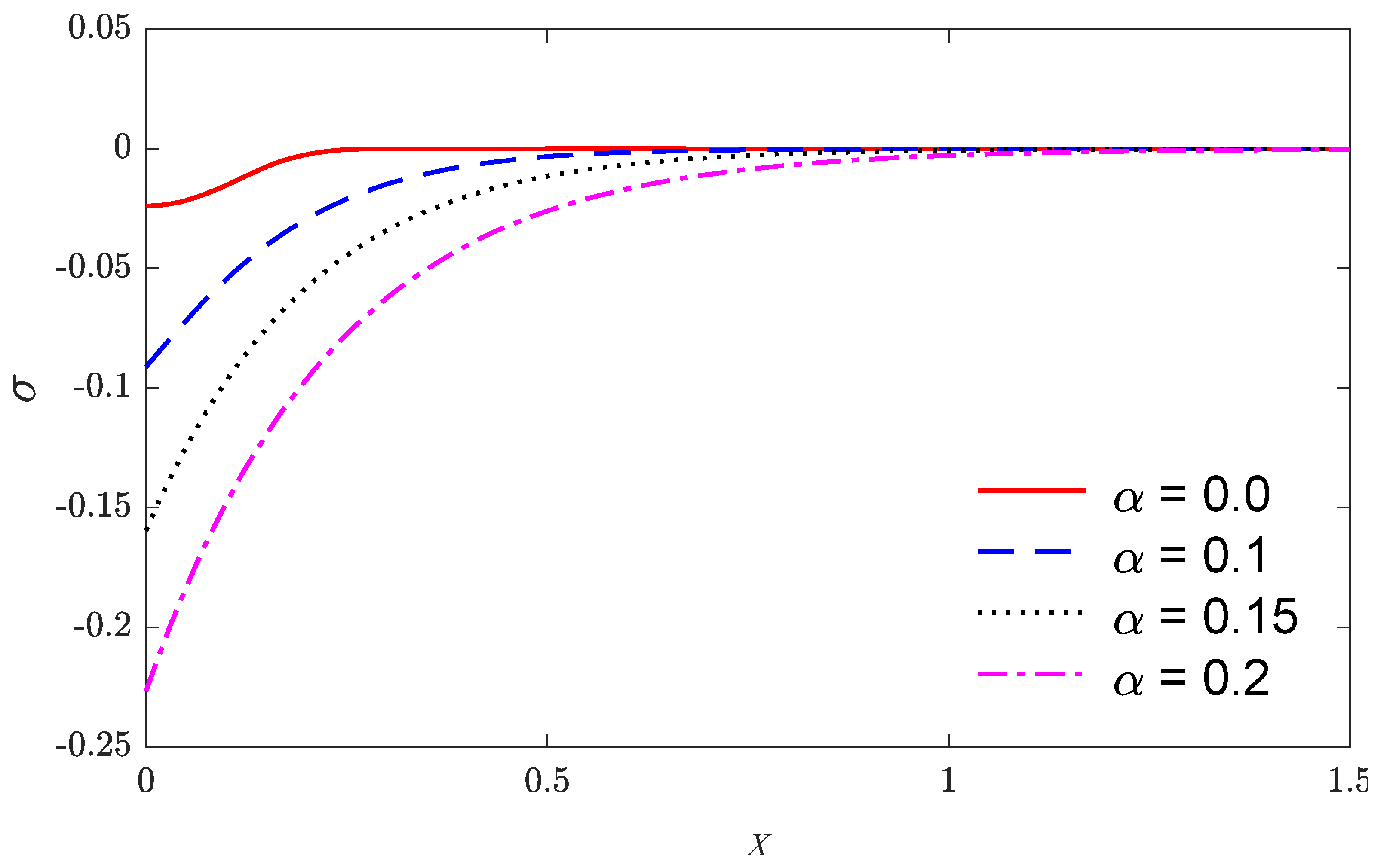
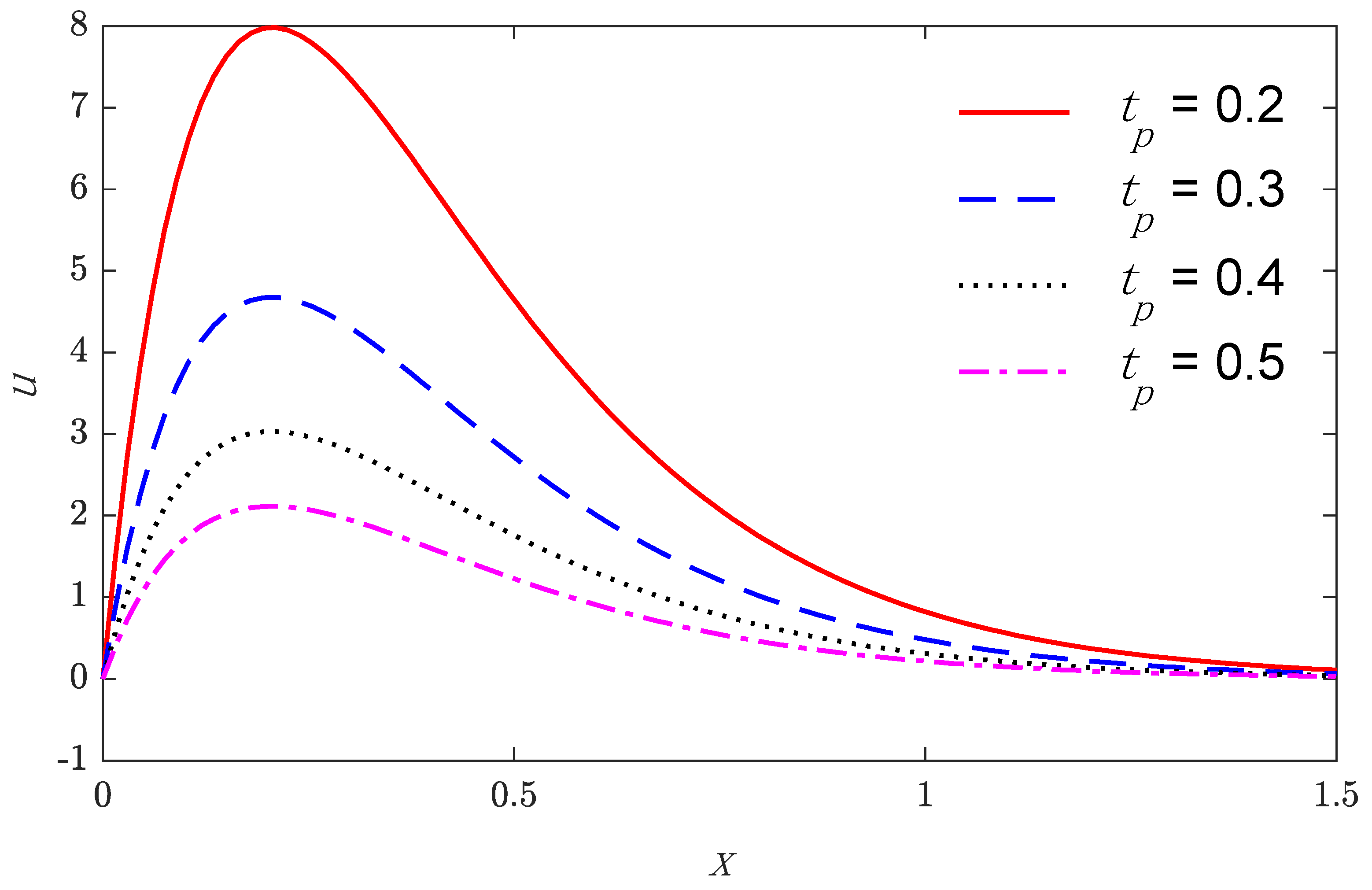
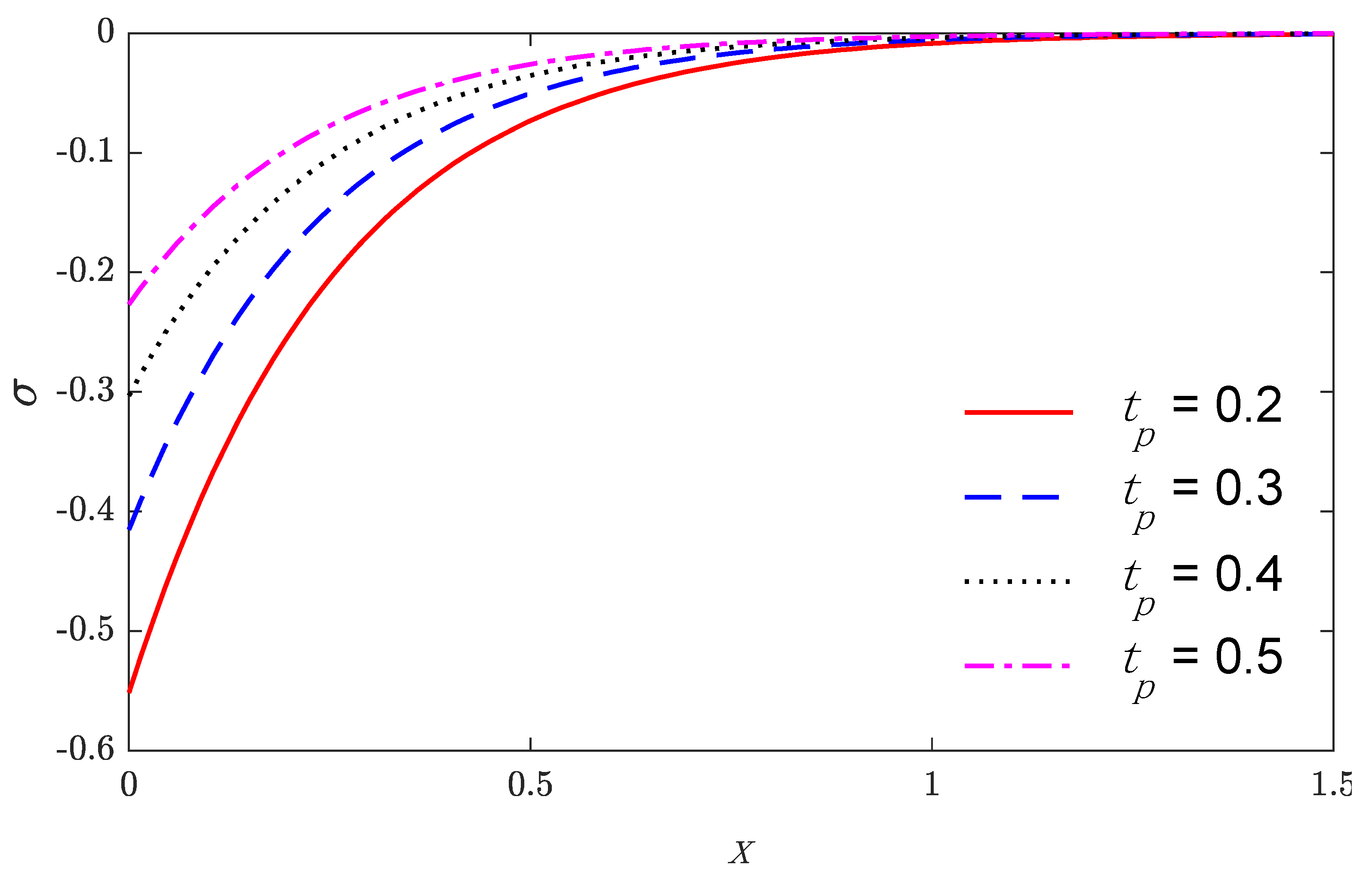
5. Results and Discussions
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lord, H.W.; Shulman, Y. A generalized dynamical theory of thermoelasticity. J. Mech. Phys. Solids 1967, 15, 299–309. [Google Scholar] [CrossRef]
- Green, A.; Lindsay, K. Thermoelasticity. J. Elast. 1972, 2, 1–7. [Google Scholar] [CrossRef]
- Green, A.E.; Naghdi, P.M. A re-examination of the basic postulates of thermomechanics. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1991, 432, 171–194. [Google Scholar]
- Green, A.; Naghdi, P. On undamped heat waves in an elastic solid. J. Therm. Stresses 1992, 15, 253–264. [Google Scholar] [CrossRef]
- Green, A.; Naghdi, P. Thermoelasticity without energy dissipation. J. Elast. 1993, 31, 189–208. [Google Scholar] [CrossRef]
- Guo, J.-G.; Zhao, Y.-P. The size-dependent elastic properties of nanofilms with surface effects. J. Appl. Phys. 2005, 98, 074306. [Google Scholar] [CrossRef] [Green Version]
- Eringen, A.C. Nonlocal polar elastic continua. Int. J. Eng. Sci. 1972, 10, 1–16. [Google Scholar] [CrossRef]
- Eringen, A.C. Theory of nonlocal thermoelasticity. Int. J. Eng. Sci. 1974, 12, 1063–1077. [Google Scholar] [CrossRef]
- Eringen, A.C. Memory-dependent nonlocal electromagnetic elastic solids and superconductivity. J. Math. Phys. 1991, 32, 787–796. [Google Scholar] [CrossRef]
- Eringen, A.C.; Wegner, J. Nonlocal continuum field theories. Appl. Mech. Rev. 2003, 56, B20–B22. [Google Scholar] [CrossRef]
- Povstenko, Y.Z. The nonlocal theory of elasticity and its applications to the description of defects in solid bodies. J. Math. Sci. 1999, 97, 3840–3845. [Google Scholar] [CrossRef]
- Marin, M.I.; Agarwal, R.P.; Abbas, I.A. Effect of intrinsic rotations, microstructural expansion and contractions in initial boundary value problem of thermoelastic bodies. Bound. Value Probl. 2014, 2014, 129. [Google Scholar] [CrossRef] [Green Version]
- Abbas, I.A.; Kumar, R.; Reen, L.S. Response of thermal source in transversely isotropic thermoelastic materials without energy dissipation and with two temperatures. Can. J. Phys. 2014, 92, 1305–1311. [Google Scholar] [CrossRef]
- Marin, M. A domain of influence theorem for microstretch elastic materials. Nonlinear Anal. Real World Appl. 2010, 11, 3446–3452. [Google Scholar] [CrossRef]
- Sarkar, N.; De, S.; Sarkar, N. Waves in nonlocal thermoelastic solids of type II. J. Therm. Stresses 2019, 42, 1153–1170. [Google Scholar] [CrossRef]
- Zenkour, A.M.; Kutbi, M.A. Thermoelastic interactions in a hollow cylinder due to a continuous heat source without energy dissipation. Mater. Res. Express 2020, 7, 035702. [Google Scholar] [CrossRef]
- Marin, M. A partition of energy in thermoelasticity of microstretch bodies. Nonlinear Anal. Real World Appl. 2010, 11, 2436–2447. [Google Scholar] [CrossRef]
- Lata, P.; Kaur, I. Thermomechanical interactions in transversely isotropic magneto thermoelastic solid with two temperatures and without energy dissipation. Steel Compos. Struct. 2019, 32, 779–793. [Google Scholar] [CrossRef]
- Youssef, H.M. Theory of two-temperature thermoelasticity without energy dissipation. J. Therm. Stresses 2011, 34, 138–146. [Google Scholar] [CrossRef]
- Zenkour, A.M. Nonlocal thermoelasticity theory without energy dissipation for nano-machined beam resonators subjected to various boundary conditions. Microsyst. Technol. 2017, 23, 55–65. [Google Scholar] [CrossRef]
- Mondal, S.; Sarkar, N.; Sarkar, N. Waves in dual-phase-lag thermoelastic materials with voids based on Eringen’s nonlocal elasticity. J. Therm. Stresses 2019, 42, 1035–1050. [Google Scholar] [CrossRef]
- Sarkar, N.; Tomar, S.K. Plane waves in nonlocal thermoelastic solid with voids. J. Therm. Stresses 2019, 42, 580–606. [Google Scholar] [CrossRef]
- Sarkar, N. Thermoelastic responses of a nonlocal elastic rod due to nonlocal heat conduction. ZAMM Z. Angew. Math. Mech. 2020, 100, e201900252. [Google Scholar] [CrossRef]
- Ansari, R.; Gholami, R. Nonlocal free vibration in the pre- and post-buckled states of magneto-electro-thermo elastic rectangular nanoplates with various edge conditions. Smart Mater. Struct. 2016, 25, 095033. [Google Scholar] [CrossRef]
- Mahinzare, M.; Mohammadi, K.; Ghadiri, M.; Rajabpour, A. Size-dependent effects on critical flow velocity of a SWCNT conveying viscous fluid based on nonlocal strain gradient cylindrical shell model. Microfluid. Nanofluidics 2017, 21, 123. [Google Scholar] [CrossRef]
- Bachher, M.; Sarkar, N. Nonlocal theory of thermoelastic materials with voids and fractional derivative heat transfer. Waves Random Complex Media 2019, 29, 595–613. [Google Scholar] [CrossRef]
- Bayones, F.S.; Mondal, S.; Abo-Dahab, S.M.; Kilany, A.A. Effect of moving heat source on a magneto-thermoelastic rod in the context of Eringen’s nonlocal theory under three-phase lag with a memory dependent derivative. Mech. Based Des. Struct. Mach. 2021. [Google Scholar] [CrossRef]
- Zhou, H.; Li, P. Nonlocal dual-phase-lagging thermoelastic damping in rectangular and circular micro/nanoplate resonators. Appl. Math. Model. 2021, 95, 667–687. [Google Scholar] [CrossRef]
- Singh, B.; Bijarnia, R. Nonlocal effects on propagation of waves in a generalized thermoelastic solid half space. Struct. Eng. Mech. 2021, 77, 473–479. [Google Scholar] [CrossRef]
- Lata, P.; Singh, S. Stoneley wave propagation in nonlocal isotropic magneto-thermoelastic solid with multi-dual-phase lag heat transfer. Steel Compos. Struct. 2021, 38, 141–150. [Google Scholar] [CrossRef]
- Kaur, I.; Lata, P.; Singh, K. Study of transversely isotropic nonlocal thermoelastic thin nano-beam resonators with multi-dual-phase-lag theory. Arch. Appl. Mech. 2021, 91, 317–341. [Google Scholar] [CrossRef]
- Biswas, S. Rayleigh waves in porous nonlocal orthotropic thermoelastic layer lying over porous nonlocal orthotropic thermoelastic half space. Waves Random Complex Media 2021. [Google Scholar] [CrossRef]
- Palani, G.; Abbas, I. Free convection MHD flow with thermal radiation from an impulsively started vertical plate. Nonlinear Anal. Model. Control 2009, 14, 73–84. [Google Scholar] [CrossRef] [Green Version]
- Abbas, I.A.; Marin, M. Analytical Solutions of a Two-Dimensional Generalized Thermoelastic Diffusions Problem Due to Laser Pulse. Iran. J. Sci. Technol. Trans. Mech. Eng. 2018, 42, 57–71. [Google Scholar] [CrossRef]
- Saeed, T.; Abbas, I.; Marin, M. A GL Model on Thermo-Elastic Interaction in a Poroelastic Material Using Finite Element Method. Symmetry 2020, 12, 488. [Google Scholar] [CrossRef] [Green Version]
- Hobiny, A.; Abbas, I.A. Analytical solutions of photo-thermo-elastic waves in a non-homogenous semiconducting material. Results Phys. 2018, 10, 385–390. [Google Scholar] [CrossRef]
- Abo-Dahab, S.M.; Abbas, I.A. LS model on thermal shock problem of generalized magneto-thermoelasticity for an infinitely long annular cylinder with variable thermal conductivity. Appl. Math. Model. 2011, 35, 3759–3768. [Google Scholar] [CrossRef]
- Marin, M.; Othman, M.I.A.; Seadawy, A.R.; Carstea, C. A domain of influence in the Moore–Gibson–Thompson theory of dipolar bodies. J. Taibah Univ. Sci. 2020, 14, 653–660. [Google Scholar] [CrossRef]
- Hobiny, A.; Alzahrani, F.; Abbas, I.; Marin, M. The effect of fractional time derivative of bioheat model in skin tissue induced to laser irradiation. Symmetry 2020, 12, 602. [Google Scholar] [CrossRef]
- Eringen, A.C. Plane waves in nonlocal micropolar elasticity. Int. J. Eng. Sci. 1984, 22, 1113–1121. [Google Scholar] [CrossRef]
- Zenkour, A.M.; Abbas, I.A. Electro-magneto-thermo-elastic response of infinite functionally graded cylinders without energy dissipation. J. Magn. Magn. Mater. 2015, 395, 123–129. [Google Scholar] [CrossRef]
- Zenkour, A.M.; Abouelregal, A.E. Effect of temperature dependency on constrained orthotropic unbounded body with a cylindrical cavity due to pulse heat flux. J. Therm. Sci. Technol. 2015, 10. [Google Scholar] [CrossRef] [Green Version]
- Das, N.C.; Lahiri, A.; Giri, R.R. Eigenvalue approach to generalized thermoelasticity. Indian J. Pure Appl. Math. 1997, 28, 1573–1594. [Google Scholar]
- Alzahrani, F.S.; Abbas, I.A. Fractional order gl model on thermoelastic interaction in porous media due to pulse heat flux. Geomach. Eng. 2020, 23, 217–225. [Google Scholar] [CrossRef]
- Hobiny, A.D.; Abbas, I.A. Fractional order thermoelastic wave assessment in a two-dimension medium with voids. Geomach. Eng. 2020, 21, 85–93. [Google Scholar] [CrossRef]
- Stehfest, H. Algorithm 368: Numerical inversion of Laplace transforms [D5]. Commun. ACM 1970, 13, 47–49. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saeed, T.; Abbas, I. Effects of the Nonlocal Thermoelastic Model in a Thermoelastic Nanoscale Material. Mathematics 2022, 10, 284. https://doi.org/10.3390/math10020284
Saeed T, Abbas I. Effects of the Nonlocal Thermoelastic Model in a Thermoelastic Nanoscale Material. Mathematics. 2022; 10(2):284. https://doi.org/10.3390/math10020284
Chicago/Turabian StyleSaeed, Tareq, and Ibrahim Abbas. 2022. "Effects of the Nonlocal Thermoelastic Model in a Thermoelastic Nanoscale Material" Mathematics 10, no. 2: 284. https://doi.org/10.3390/math10020284
APA StyleSaeed, T., & Abbas, I. (2022). Effects of the Nonlocal Thermoelastic Model in a Thermoelastic Nanoscale Material. Mathematics, 10(2), 284. https://doi.org/10.3390/math10020284





