Multiagent Control of Airplane Wing Stability with “Feathers” under the Flexural Torsional Flutter
Abstract
1. Introduction
2. Related Work
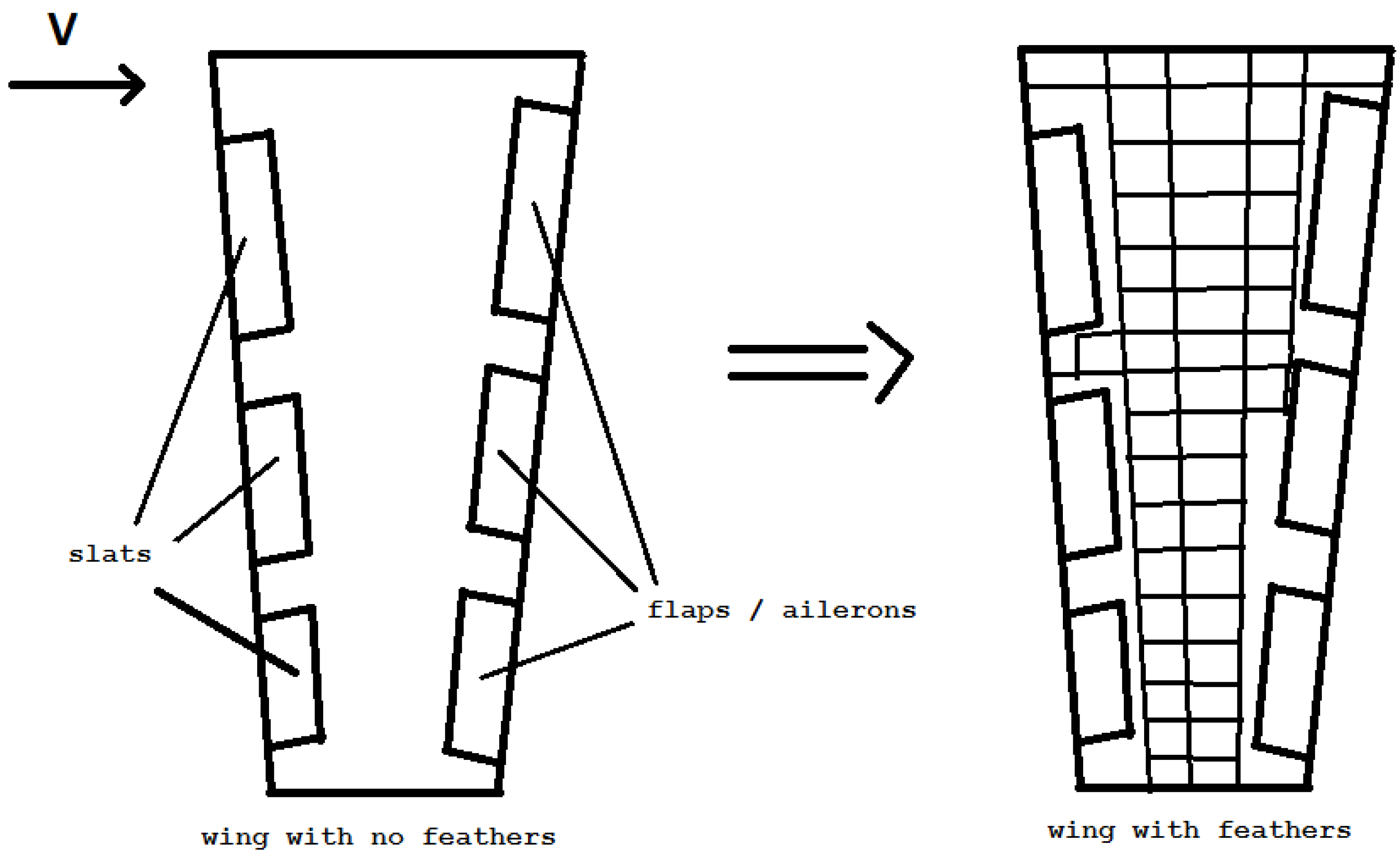
3. Dynamics Equations of Wing with “Feathers”
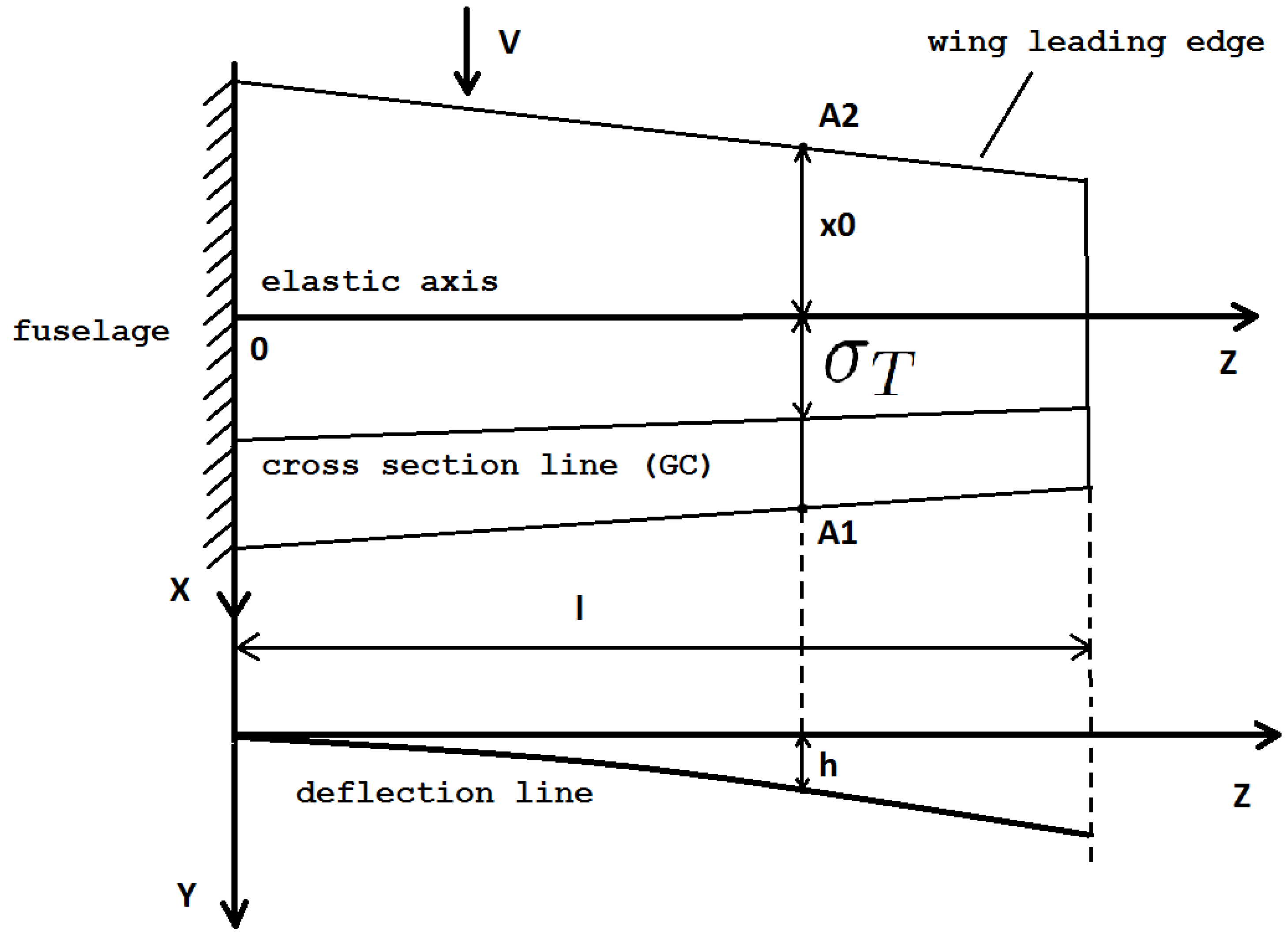
- b is the wing section chord;
- h is the transverse deflection of the section ;
- is the angle of attack in the section of the undeformed wing;
- is the wing twist angle, which is considered positive if it increases the angle of attack in the section;
- are the wing stiffness in bending and torsion, respectively;
- and are the linear force and moment relative to the stiffness axis acting on the wing.
- m is the linear mass of the wing;
- is the linear mass moment of inertia of the wing relative to its stiffness axis;
- and are the linear aerodynamic force of the wing and the linear moment of the aerodynamic force relative to the stiffness axis, due to wing vibrations.
- and are the linear aerodynamic force and moment relative to the stiffness axis respectively, arising due to wing oscillations in the neutral position of the “feathers”;
- and are the linear aerodynamic force and moment created by changing the orientation of the “feathers”.
- ; is the wing lift coefficient;
- is considered constant along the span;
- is the instant value of the angle of attack when the wing moves;
- is the value of the angle of attack at which ;
- is the air density.
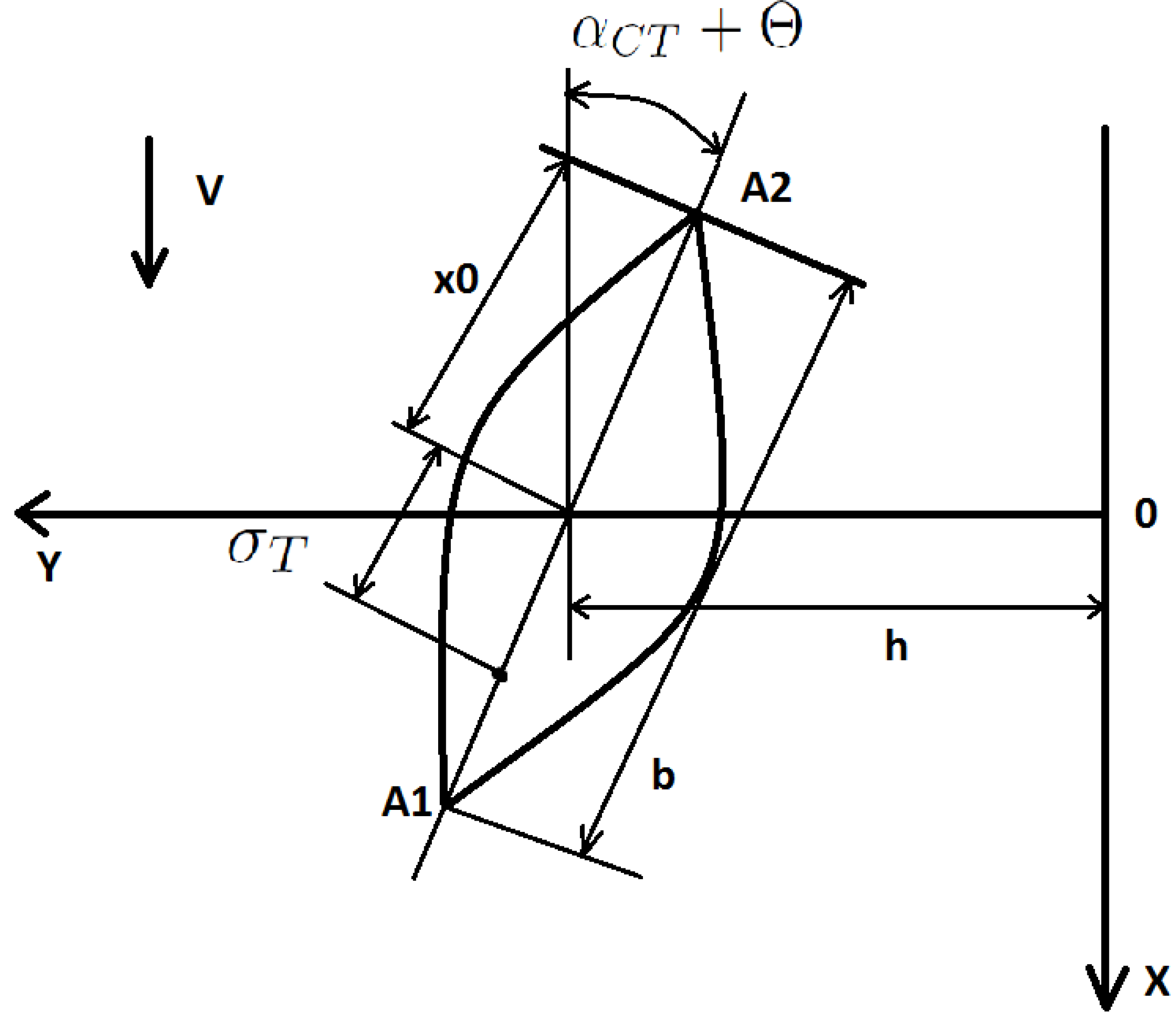
- E and are SC’s of the wing section in the static position and during vibrations, respectively; and is the deflection of the wing;
- X-axis corresponds to the main stream speed;
- Y-axis is perpendicular to X-axis and to the axis of rigidity of the undeformed wing;
- are wing profiles (considered to be sufficiently thin) in a static position (before vibrations);
- are wing profiles during oscillations;
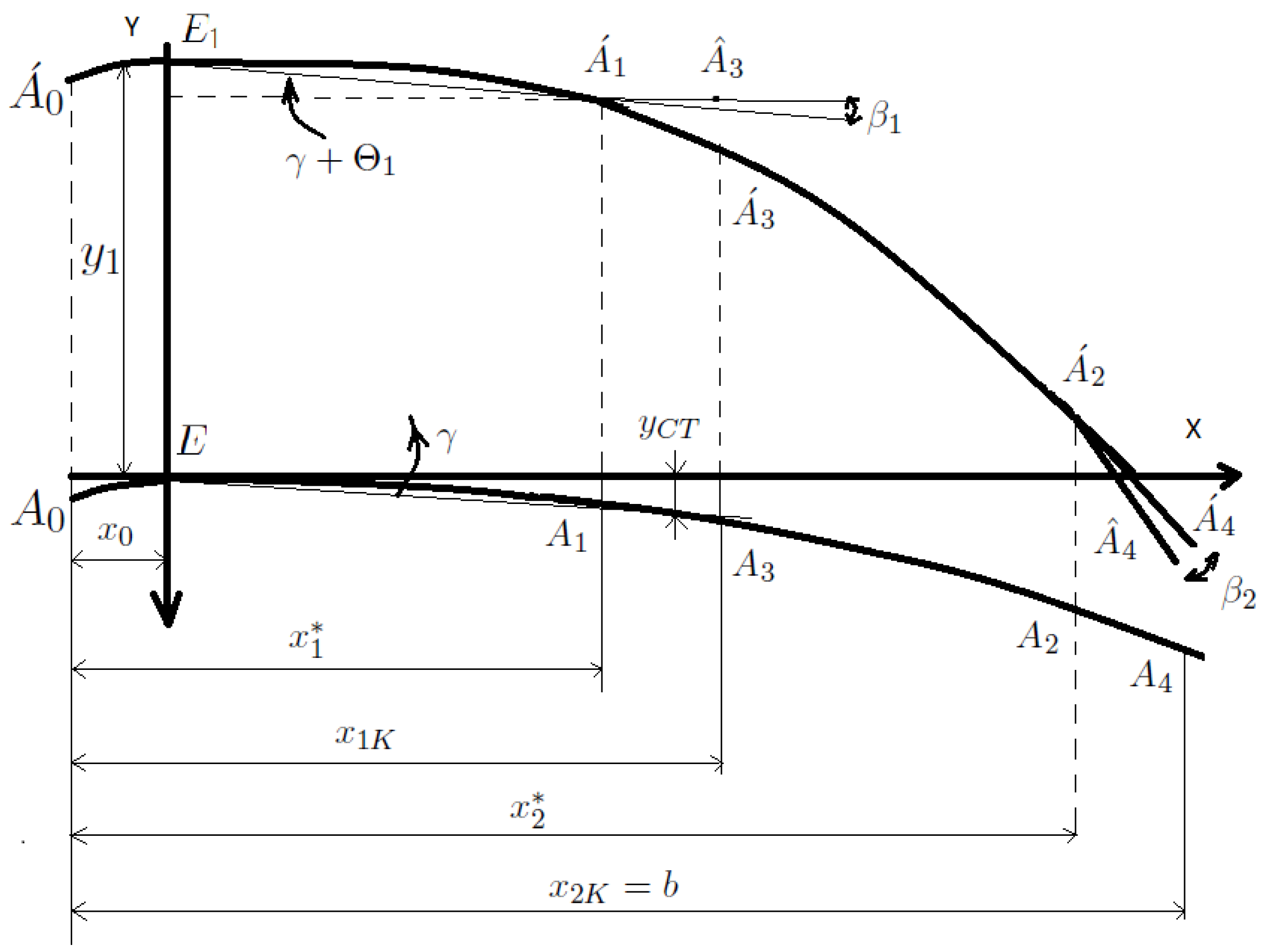
- are the front and back edges of the “feather” 1 in the neutral position on the corresponding profiles;
- are the front and back edges of the “feather” 2 (analog of the aileron) in the neutral position on relevant profiles;
- and are the trailing edges of the “feathers” 1 and 2, respectively, after their deviations.
- and are the distances from the leading and trailing edges of the “feather” 1 to the leading edge of the wing;
- and are similar parameters for the “feather” 2;
- is the angle of twisting of the wing near the point ;
- is the angle of deviation of the “feather” 1 from the neutral position;
- is the angle of deviation of the “feather” 2 from the neutral position;
4. Control Synthesis with the Speed-Gradient Method
5. Multiagent Control
- are coordinates of the point to which the i-th “feather” is attached;
- and are deflection and angle of twisting of the wing at the location of the i-th “feather” (deviations from the curve (2));
- is the subset of “feathers” exchanging information with the i-th “feather”;
- is a non-negative weighting coefficient denoting the significance of information passing from the i-th “feather” to the j-th. Here, we assume that and ; here if the i-th and j-th “feathers” are not connected;
- is the adjacency matrix of the network.
5.1. Non-Multiagent Control Synthesis
5.2. Multiagent Control Synthesis
6. Simulation
- time step ;
- number of time instants ;
- airspeed ;
- air density .
- linear mass of the wing ;
- wing section chord ;
- wing (half-span) length ;
- from (5) the derivative of the wing lift coefficient with respect to , ;
- wing stiffness in bending ;
- wing stiffness in torsion ;
- distance between stiffness centers and gravity centers in the wing cross section assumed to be constant and equal ;
- wing cross-section is assumed to be elliptical with height ;
- linear mass moment of inertia of the wing relative to its stiffness axis
- number of “feathers” ;
- “feather” coordinates in Z axis ;
- “feather” coordinates in X axis according to formulas (9) ;
- the coordinates of “feathers” trailing edges in X axis equal ;
- the coordinates of “feathers” joint in X axis ;
- the distance from the leading edge of the wing to the SC section .
7. Conclusions and Outlook
- a mathematical model of the bending-torsional vibrations of an airplane wing with controlled “feathers” on its surface was constructed;
- three different conditions of the control problem corresponding to distinct subject functions were considered;
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Byushgens, G.S. Aerodinamika i Dinamika Poleta Magistralnykh Samoletov [Aerodynamics and Flight Dynamics of Long Haul Aircraft]; Izdatel’skii otdel TsAGI–Avia-Izdatel’stvo KNR: Moscow-Pekin, Russian, 1995; 774p. [Google Scholar]
- Wright, J.R.; Jonathan, E.C. Introduction to Aircraft Aeroelasticity and Loads; John Wiley & Sons: Hoboken, NJ, USA, 2008; Volume 20. [Google Scholar]
- Fung, Y.C. An Introduction to the Theory of Aeroelasticity; Courier Dover Publications: Mineola, NY, USA, 2008. [Google Scholar]
- Livne, E. Aircraft active flutter suppression: State of the art and technology maturation needs. J. Aircr. 2018, 55, 410–450. [Google Scholar] [CrossRef]
- Bisplinghoff, R.L.; Ashley, H.; Halfman, R.L. Aeroelasticity; Addison-Wesley: Reading, MA, USA, 1955; 527p. [Google Scholar]
- Ashley, H. Flutter suppression within reach. Aerosp. Am. 1988, 26, 14–16. [Google Scholar]
- Bendiksen, O.O. Energy approach to flutter suppression and aeroelastic control. J. Guid. Control Dyn. 2001, 24, 176–184. [Google Scholar] [CrossRef]
- Freymann, R. New simplified ways to understand the interaction between aircraft structure and active control systems. In Proceedings of the 17th Fluid Dynamics, Plasma Dynamics, and Lasers Conference, Snowmass, CO, USA, 25–27 June 1984; p. 1868. [Google Scholar]
- Wykes, J. Structural dynamic stability augmentation and gust alleviation of flexible aircraft. In Proceedings of the 5th Annual Meeting and Technical Display, Philadelphia, PA, USA, 21–24 October 1968; p. 1067. [Google Scholar]
- Preumont, A. Vibration Control of Active Structures—An Introduction, 2nd ed.; Chapter 13; Kluwer Academic: Norwell, MA, USA, 2002; pp. 101–103. [Google Scholar]
- Livne, E. Integrated aeroservoelastic optimization: Status and progress. J. Aircr. 1999, 36, 122–145. [Google Scholar] [CrossRef]
- Vepa, R. Active flutter suppression by feedback compensation of transport lags. J. Guid. Control Dyn. 2007, 30, 879–882. [Google Scholar] [CrossRef]
- Leylek, E.A.; Costello, M. Use of compliant hinges to tailor flight dynamics of unmanned aircraft. J. Aircr. 2015, 52, 1692–1706. [Google Scholar] [CrossRef][Green Version]
- Bollay, W. Aerodynamic stability and automatic control: The fourteenth wright brothers lecture. J. Aeronaut. Sci. 1951, 18, 569–617. [Google Scholar] [CrossRef]
- Roskam, J. Airplane Flight Dynamics and Automatic Flight Controls, Parts I and II; DARcorporation: Lawrence, KS, USA, 2013. [Google Scholar]
- Schmidt, D.K. Modern Flight Dynamics; McGraw–Hill: New York, NY, USA, 2012. [Google Scholar]
- Wykes, J.H.; Lawrence, R.E. Aerothermoelasticity: Its impact on stability and control of winged aerospace vehicles. J. Aircr. 1965, 2, 517–526. [Google Scholar] [CrossRef]
- Nicolai, L.; Hunten, K.; Zink, P.S.; Flick, P. System benefits of active flutter suppression for a sensorcraft-type vehicle. In Proceedings of the 13th AIAA/ISSMO Multidisciplinary Analysis Optimization Conference, Fort Worth, TX, USA, 13–15 September 2010; p. 9349. [Google Scholar]
- Huang, R.; Hu, H.Y.; Zhao, Y.H. Single-input/single-output adaptive flutter suppression of a three-dimensional aeroelastic system. J. Guid. Control Dyn. 2012, 35, 659–665. [Google Scholar] [CrossRef]
- Lee, K.W.; Singh, S.N. Multi-input noncertainty-equivalent adaptive control of an aeroelastic system. J. Guid. Control Dyn. 2010, 33, 1451–1460. [Google Scholar] [CrossRef]
- Zeng, J.; Wang, J.; de Callafon, R.; Brenner, M. Suppression of the aeroelastic/aeroservoelastic interaction using adaptive feedback control instead of notching filters. In Proceedings of the AIAA Atmospheric Flight Mechanics Conference, Portland, Oregon, 8–11 August 2011; p. 6459. [Google Scholar]
- Nguyen, N.T.; Swei, S.-M. Adaptive linear quadratic gaussian optimal control modification for flutter suppression of adaptive wing. In Proceedings of the AIAA Infotech @ Aerospace, Kissimmee, FL, USA, 5–9 January 2015; p. 0118. [Google Scholar]
- Lee, K.W.; Singh, S.N. Adaptive control of multi-input aeroelastic system with constrained inputs. J. Guid. Control Dyn. 2015, 38, 2337–2350. [Google Scholar] [CrossRef]
- Danowsky, B.P.; Thompson, P.M.; Lee, D.C.; Brenner, M. Modal Isolation and damping for adaptive aeroservoelastic suppression. In Proceedings of the AIAA Atmospheric Flight Mechanics (AFM) Conference, Boston, MA, USA, 19–22 August 2013; p. 4743. [Google Scholar]
- Nissim, E. Controller reduction using normal coordinates of reconstruction error matrix and component cost analysis method. J. Guid. Control Dyn. 1997, 20, 826–828. [Google Scholar] [CrossRef]
- Balakrishnan, A.V. Aeroelasticity, the Continuum Theory; Springer: New York, NY, USA, 2012. [Google Scholar]
- Platanitis, G.; Strganac, T.W. Control of a nonlinear wing section using leading- and trailing-edge surfaces. J. Guid. Control Dyn. 2004, 27, 52–58. [Google Scholar] [CrossRef]
- Xiang, J.; Yan, Y.; Li, D. Recent advance in nonlinear aeroelastic analysis and control of the aircraft. Chin. J. Aeronaut. 2014, 27, 12–22. [Google Scholar] [CrossRef]
- Mannarino, A.; Dowell, E.H.; Mantegazza, P. An adaptive controller for nonlinear flutter suppression and free-play compensation. J. Vib. Control 2015, 23, 2269–2290. [Google Scholar] [CrossRef]
- Tantaroudas, N.D.; Da Ronch, A.; Gai, G.; Badcock, K.J. An adaptive aeroelastic control approach using non linear reduced order models. In Proceedings of the 14th AIAA Aviation Technology, Integration, and Operations Conference, Atlanta, GA, USA, 16–20 June 2014; p. 2590. [Google Scholar]
- Singh, K.V.; McDonough, L.A.; Kolonay, R.; Cooper, J. Receptance based active aeroelastic control using multiple control surfaces. J. Aircr. 2014, 51, 335–342. [Google Scholar] [CrossRef]
- Amelina, N.; Granichin, O.; Kornivetc, A. Local Voting Protocol in decentralized load balancing problem with switched topology, noise, and delays. In Proceedings of the 52nd IEEE Conference on Decision and Control, Firenze, Italy, 10–13 December 2013; pp. 4613–4618. [Google Scholar]
- Amelina, N.; Granichin, O.; Granichina, O.; Ivanskiy, Y.; Jiang, Y. Optimal step-size of a local voting protocol for differentiated consensuses achievement in a stochastic network with priorities. In Proceedings of the 2015 European Control Conference, Linz, Austria, 15–17 July 2015; pp. 628–633. [Google Scholar]
- Amelin, K.; Granichin, O.; Sergeenko, A.; Volkovich, Z.V. Emergent intelligence via self-organization in group of robotics devices. Mathematics. 2021, 9, 1314. [Google Scholar] [CrossRef]
- Weiss, G. (Ed.) Multiagent Systems: A Modern Approach to Distributed Artificial Intelligence; MIT Press: Cambridge, MA, USA, 1999. [Google Scholar]
- Olfati-Saber, R.; Fax, J.A.; Murray, R.M. Consensus and cooperation in networked multi-agent systems. Proc. IEEE 2007, 95, 215–233. [Google Scholar] [CrossRef]
- Aleksandrov, A.; Fradkov, A.; Semenov, A. Delayed and switched control of formations on a line segment: Delays and switches do not matter. IEEE Trans. Autom. Control 2020, 65, 794–800. [Google Scholar] [CrossRef]
- Parsegov, S.; Polyakov, A.; Shcherbakov, P. Nonlinear fixed time control protocol for uniform allocation of agents on a segment. In Proceedings of the 51st IEEE Conference on Decision and Control (CDC), Maui, HI, USA, 10–13 December 2012; pp. 7732–7737. [Google Scholar]
- Shang, Y. Resilient consensus in multi-agent systems with state constraints. Automatica 2020, 122, 109288. [Google Scholar] [CrossRef]
- Granichin, O.; Khantuleva, T.; Granichina, O. Local voting protocol for the adaptation of airplane’s “feathers” in a turbulence flow. In Proceedings of the 2017 American Control Conference (ACC), Seattle, WA, USA, 24–26 May 2017; pp. 5684–5689. [Google Scholar]
- Granichin, O.N.; Khantuleva, T.A. Adapting wing elements (feathers) of an airplane in a turbulent flow with a multiagent protocol. Autom Remote Control 2017, 78, 1867–1882. [Google Scholar] [CrossRef]
- Amelin, K.; Amelina, N.; Granichin, O.; Granichina, O.; Ivanskiy, Y. Synchronization of multi-agent system of “feathers” on the surface of the wing in a turbulent airflow. In Proceedings of the 2019 IEEE Conference on Control Technology and Applications (CCTA), Hong Kong, China, 19–21 August 2019; pp. 355–359. [Google Scholar]
- Proskurnikov, A.; Granichin, O. Evolution of clusters in large-scale dynamical networks. Cybern. Phys. 2018, 7, 102–129. [Google Scholar] [CrossRef]
- Granichin, O.; Uzhva, D.; Volkovich, Z. Cluster flows and multiagent technology. Mathematics 2021, 9, 22. [Google Scholar] [CrossRef]
- Granichin, O.; Uzhva, D. Cluster control of complex cyber-physical systems. Cybern. Phys. 2021, 10, 191–200. [Google Scholar]
- Grossman, E.P. A Course in Vibrations in Parts of Aircraft; Oborongiz: Moscow, Russia, 1940; 312p. (In Russian) [Google Scholar]
- Fradkov, A.L. Cybernetical Physics: From Control of Chaos to Quantum Control; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Fradkov, A.L. Speed-Gradient scheme and its application in adaptive control problems. Autom. Remote Control 1979, 40, 1333–1342. [Google Scholar]
- Shang, Y.; Ye, Y. Fixed-time group tracking control with unknown inherent nonlinear dynamics. IEEE Access 2017, 5, 12833–12842. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shalymov, D.; Granichin, O.; Ivanskiy, Y.; Volkovich, Z. Multiagent Control of Airplane Wing Stability with “Feathers” under the Flexural Torsional Flutter. Mathematics 2022, 10, 236. https://doi.org/10.3390/math10020236
Shalymov D, Granichin O, Ivanskiy Y, Volkovich Z. Multiagent Control of Airplane Wing Stability with “Feathers” under the Flexural Torsional Flutter. Mathematics. 2022; 10(2):236. https://doi.org/10.3390/math10020236
Chicago/Turabian StyleShalymov, Dmitry, Oleg Granichin, Yury Ivanskiy, and Zeev Volkovich. 2022. "Multiagent Control of Airplane Wing Stability with “Feathers” under the Flexural Torsional Flutter" Mathematics 10, no. 2: 236. https://doi.org/10.3390/math10020236
APA StyleShalymov, D., Granichin, O., Ivanskiy, Y., & Volkovich, Z. (2022). Multiagent Control of Airplane Wing Stability with “Feathers” under the Flexural Torsional Flutter. Mathematics, 10(2), 236. https://doi.org/10.3390/math10020236






