Global Stabilization of Nonlinear Finite Dimensional System with Dynamic Controller Governed by 1 − d Heat Equation with Neumann Interconnection
Abstract
1. Introduction
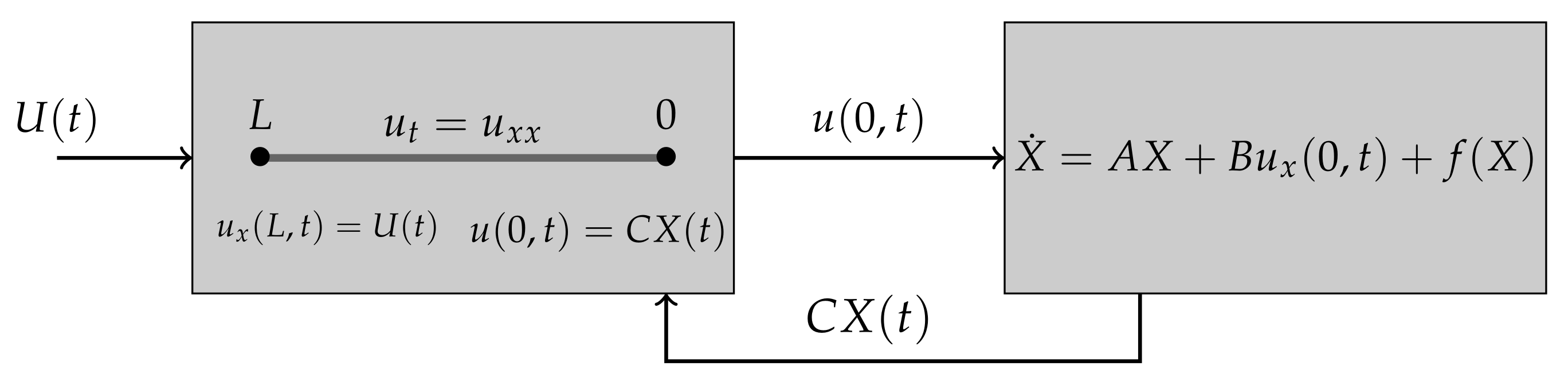
2. Problem Setting and Controller Design
2.1. Problem Formulation
2.2. Backstepping Transformations
3. Analysis of the Closed-Loop
3.1. Well-Posedness
3.2. Exponential Stability
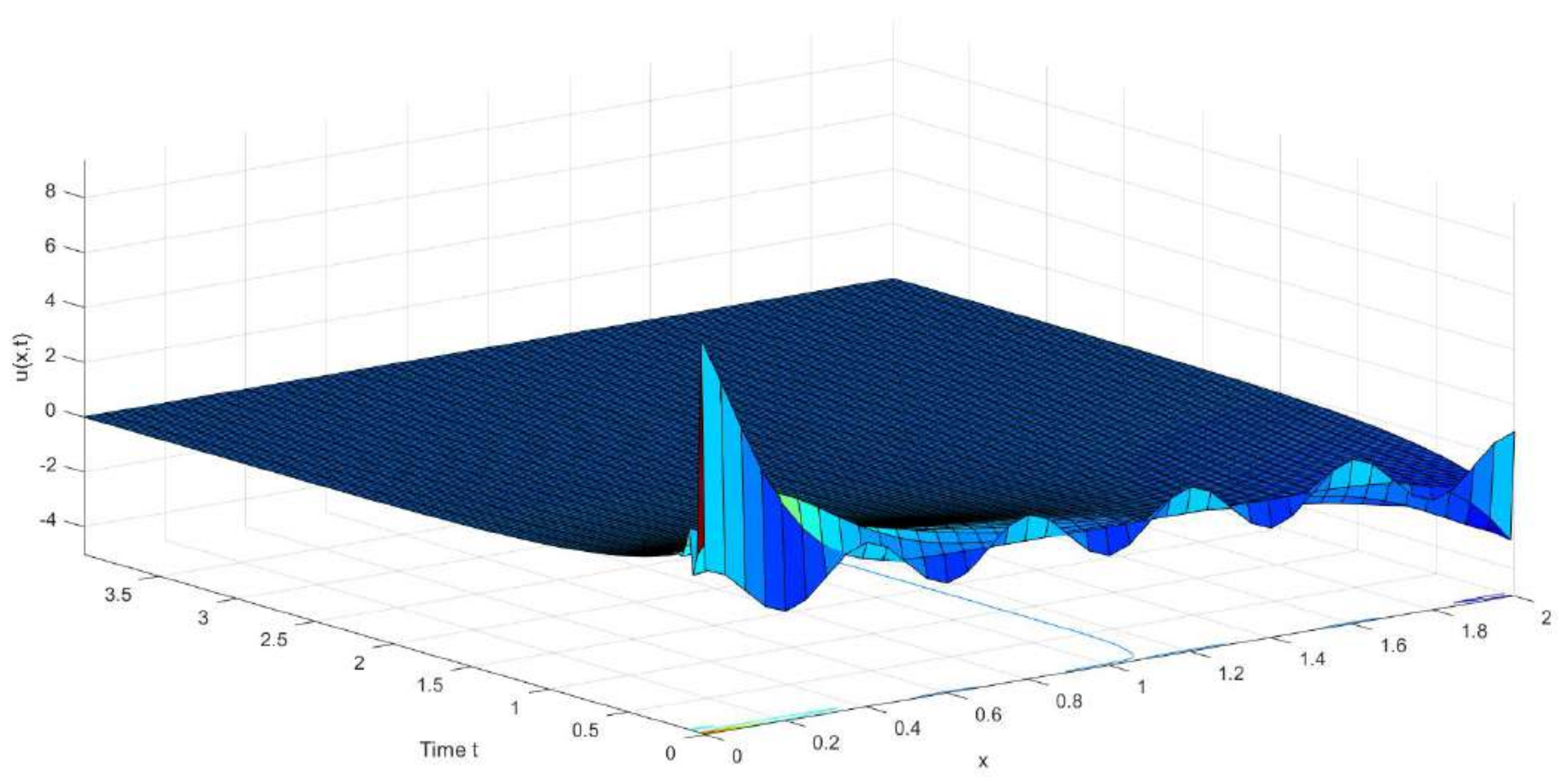
4. Numerical Example
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Zhao, A.; Xie, C. Stabilization of coupled linear plant and reaction—Diffusion process. J. Frankl. Inst. 2014, 351, 857–877. [Google Scholar] [CrossRef]
- Benabdallah, A. Stabilization of a class of nonlinear uncertain ordinary differential equation by parabolic partial differential equation controller. Int. J. Robust Nonlinear Control 2020, 30, 3023–3038. [Google Scholar] [CrossRef]
- Zhen, Z.; Si, Y.; Xie, C. Indirect control to stabilize reaction-diffusion equation. Int. J. Control 2021, 94, 3091–3098. [Google Scholar] [CrossRef]
- Benabdallah, A.; Dlala, M. Rapid exponential stabilization by boundary state feedback for a class of coupled nonlinear ODE and 1 − d heat diffusion equation. Discret. Contin. Dyn. 2021. [Google Scholar] [CrossRef]
- Goatin, P. The Aw-Rascle vehicular traffic flow model with phase transitions. Math. Comput. Model. 2006, 44, 287–303. [Google Scholar] [CrossRef]
- Tang, Y.; Prieur, C.; Girard, A. Stability analysis of a singularly perturbed coupled ODE-PDE system. In Proceedings of the 54 th IEEE Conference on Decision and Control, Osaka, Japan, 15–18 December 2015; pp. 4591–4596. [Google Scholar]
- J Daafouz, J.; Tucsnak, M.; Valein, J. Nonlinear control of a coupled PDE-ODE system modeling a switched power converter with a transmission line. Syst. Control Lett. 2014, 70, 92–99. [Google Scholar] [CrossRef]
- Saldivar, B.; Mondié, S.; Avila Vilchis, J.C. The Control of Drilling Vibrations: A Coupled PDE-ODE Modeling Approach. Int. J. Appl. Math. Comput. Sci. 2016, 26, 335–349. [Google Scholar] [CrossRef]
- Tang, S.; Xie, C. State and output feedback boundary control for a coupled PDE-ODE system. Syst. Control Lett. 2011, 60, 540–545. [Google Scholar] [CrossRef]
- Tang, S.; Xie, C. Stabilization for a coupled PDE-ODE control system. J. Frankl. Inst. 2011, 348, 2142–2155. [Google Scholar] [CrossRef]
- Krstic, M. Compensating a String PDE in the Actuation or Sensing Path of an Unstable ODE. IEEE Trans. Autom. Control 2009, 54, 1362–1368. [Google Scholar] [CrossRef]
- Susto, G.A.; Krstic, M. Control of PDE-ODE cascades with Neumann interconnections. J. Frankl. Inst. 2010, 347, 284–314. [Google Scholar] [CrossRef]
- Boundary stabilization of a coupled wave-ODE system with internal anti-damping. Int. J. Control 2012, 85, 1683–1693. [CrossRef]
- Wu, H.N.; Wang, J.W. Observer design and output feedback stabilization for nonlinear multivariable systems with diffusion PDE-governed sensor dynamics. Nonlinear Dyn. 2013, 72, 615–628. [Google Scholar] [CrossRef]
- Ahmed-Ali, T.; Giri, F.; Krstic, M.; Lamnabhi-Lagarrigue, F. Observer design for a class of nonlinear ODE-PDE cascade systems. Syst. Control Lett. 2015, 83, 19–27. [Google Scholar] [CrossRef]
- Cai, X.; Liao, L.; Zhang, J.; Zhang, W. Observer design for a class of nonlinear system in cascade with counter-convecting transport dynamics. Kybernetika 2016, 52, 76–88. [Google Scholar] [CrossRef][Green Version]
- Bekiaris-Liberis, N.; Krstic, M. Compensation of Wave Actuator Dynamics for Nonlinear Systems. IEEE Trans. Autom. Control 2014, 59, 1555–1570. [Google Scholar] [CrossRef]
- Diagne, M.; Bekiaris-Liberis, N.; Otto, A.; Krstic, M. Control of Transport PDE/Nonlinear ODE Cascades with State-Dependent Propagation Speed. In Proceedings of the IEEE 55th Conference on Decision and Control, Las Vegas, NV, USA, 12–14 December 2016; pp. 3125–3130. [Google Scholar]
- Hasana, A.; Aamoa, O.M.; Krstic, M. Boundary observer design for hyperbolic PDE-ODE cascade systems. Automatica 2016, 68, 75–86. [Google Scholar] [CrossRef]
- Cai, X.; Krstic, M. Nonlinear stabilization through wave PDE dynamics with a moving uncontrolled boundary. Automatica 2016, 68, 27–38. [Google Scholar] [CrossRef]
- Zhao, X.; Weiss, G. Controllability and Observability of a Well-posed System Coupled with a Finite-dimensional System. IEEE Trans. Autom. Control 2011, 56, 1–12. [Google Scholar] [CrossRef]
- Jankovic, M. Recursive predictor design for state and output feedback controllers for linear time delay systems. Automatica 2010, 46, 510–517. [Google Scholar] [CrossRef]
- Bekiaris-Liberis, N.; Krstic, M. Compensation of state-dependent input delay for nonlinear systems. IEEE Trans. Autom. Control 2013, 58, 275–289. [Google Scholar] [CrossRef]
- Krstic, M. Compensating actuator and sensor dynamics governed by diffusion PDEs. Syst. Control Lett. 2009, 58, 372–377. [Google Scholar] [CrossRef]
- Bekiaris-Liberis, N.; Krstic, M. Compensating the distributed effect of diffusion and counter-convection in multi-input and multi-output LTI systems. IEEE Trans. Autom. Control 2011, 56, 637–642. [Google Scholar] [CrossRef]
- Ren, B.; Wang, J.M.; Krstic, M. Stabilization of an ODE-Schrodinger Cascade. Syst. Control Lett. 2013, 62, 503–510. [Google Scholar] [CrossRef]
- Bekiaris-Liberis, N.; Krstic, M. Compensating the distributed effect of a wave PDE in the actuation or sensing path of MIMO LTI Systems. Syst. Control Lett. 2010, 59, 713–719. [Google Scholar] [CrossRef]
- Chang, Y.; Sun, T.; Zhang, X.; Chen, X. Output Feedback Stabilization for a Class of Cascade Nonlinear ODE-PDE Systems. Int. J. Control. Autom. Syst. 2021, 19, 2519–2528. [Google Scholar] [CrossRef]
- Mazenc, F.; Praly, L. Global stabilization by output feedback: Examples and counter examples. Syst. Control Lett. 1994, 23, 119–125. [Google Scholar] [CrossRef]
- Liu, X.; Xie, C. Control law in analytic expression of a system coupled by reaction—Diffusion equation. Syst. Control Lett. 2020, 137, 104643. [Google Scholar] [CrossRef]
- Andrieua, V.; Praly, L. A unifying point of view on output feedback designs for global asymptotic stabilization. Automatica 2009, 45, 1789–1798. [Google Scholar] [CrossRef]
- Gohbergue, I.; Krein, M.G. Introduction to the Theory of Linear Nonselfadjoint Operators; American Mathematical Society: Providence, RI, USA, 1969. [Google Scholar]
- Pazy, A. Semigroups of Linear Operators and Applications to Partial Differential Equations; Springer: New York, NY, USA, 1992. [Google Scholar]
- Adams, R.A.; Fournier, J.J.F. Sobolev Spaces, 2nd ed.; Elsevier/Academic Press: Amsterdam, The Netherlands, 2003. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dlala, M.; Benabdallah, A. Global Stabilization of Nonlinear Finite Dimensional System with Dynamic Controller Governed by 1 − d Heat Equation with Neumann Interconnection. Mathematics 2022, 10, 227. https://doi.org/10.3390/math10020227
Dlala M, Benabdallah A. Global Stabilization of Nonlinear Finite Dimensional System with Dynamic Controller Governed by 1 − d Heat Equation with Neumann Interconnection. Mathematics. 2022; 10(2):227. https://doi.org/10.3390/math10020227
Chicago/Turabian StyleDlala, Mohsen, and Abdallah Benabdallah. 2022. "Global Stabilization of Nonlinear Finite Dimensional System with Dynamic Controller Governed by 1 − d Heat Equation with Neumann Interconnection" Mathematics 10, no. 2: 227. https://doi.org/10.3390/math10020227
APA StyleDlala, M., & Benabdallah, A. (2022). Global Stabilization of Nonlinear Finite Dimensional System with Dynamic Controller Governed by 1 − d Heat Equation with Neumann Interconnection. Mathematics, 10(2), 227. https://doi.org/10.3390/math10020227