Effects of Media Coverage on Global Stability Analysis and Optimal Control of an Age-Structured Epidemic Model with Multi-Staged Progression
Abstract
:1. Introduction
2. Model Formulation
3. Qualitative Analysis of SEIAM Model
3.1. Basic Reproduction Number and Endemic Equilibrium
3.2. Nonnegativity and Uniform Persistence of Solution
3.3. Stability Analysis of Endemic Equilibrium
- (i)
- when , it follows from and that
- (ii)
- when , it follows from (42) that
- (iii)
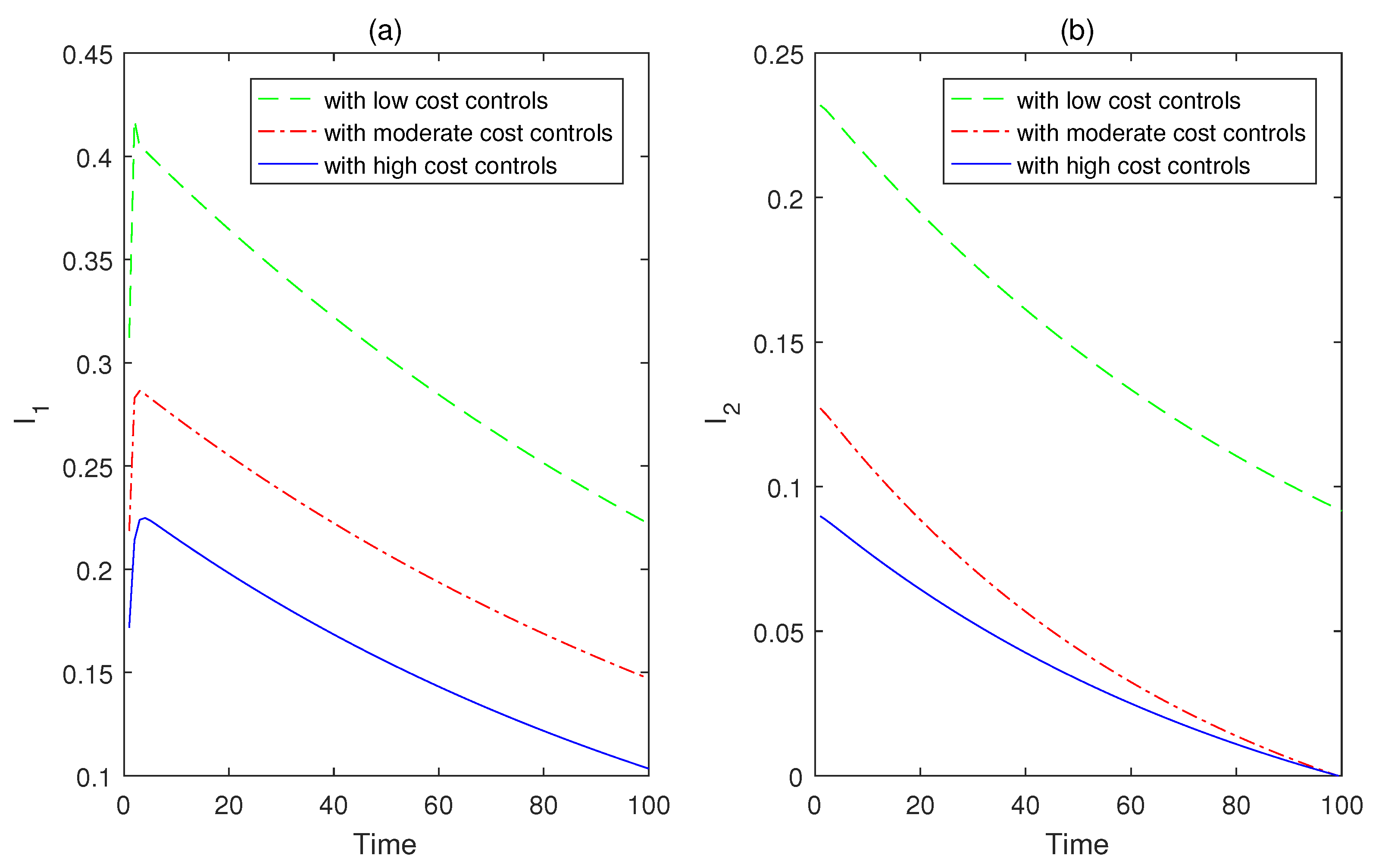
4. Optimal Control Strategy
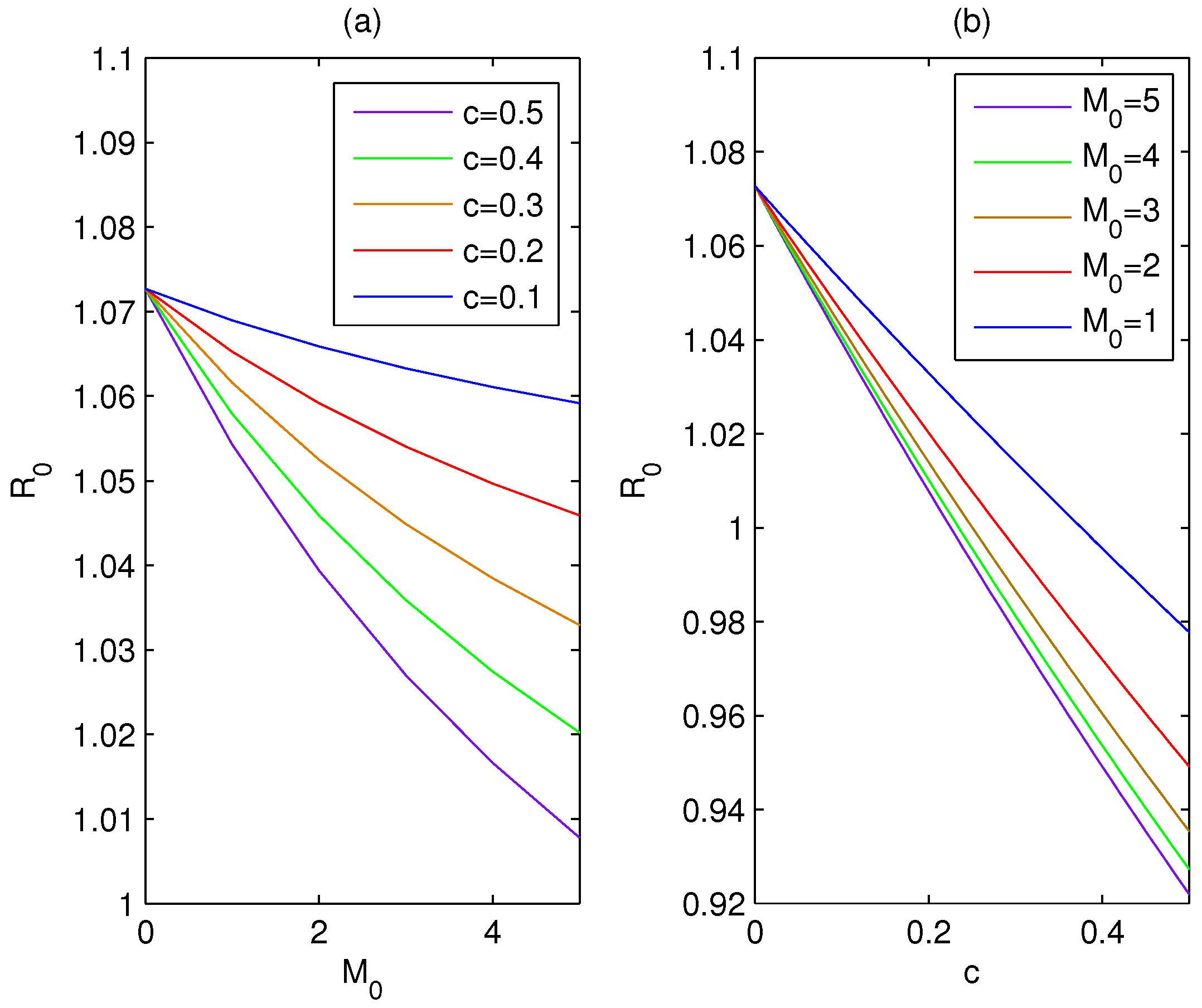
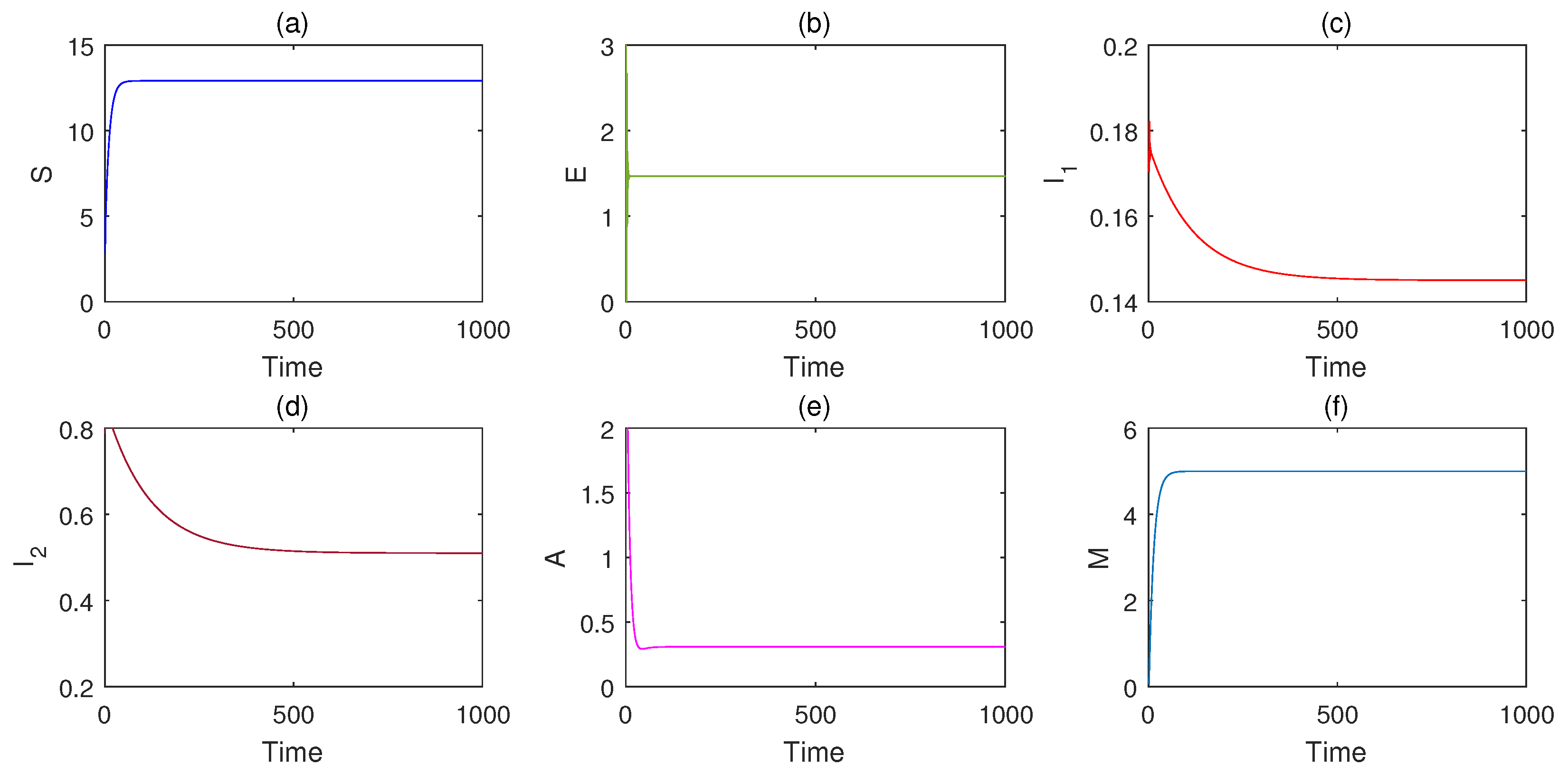
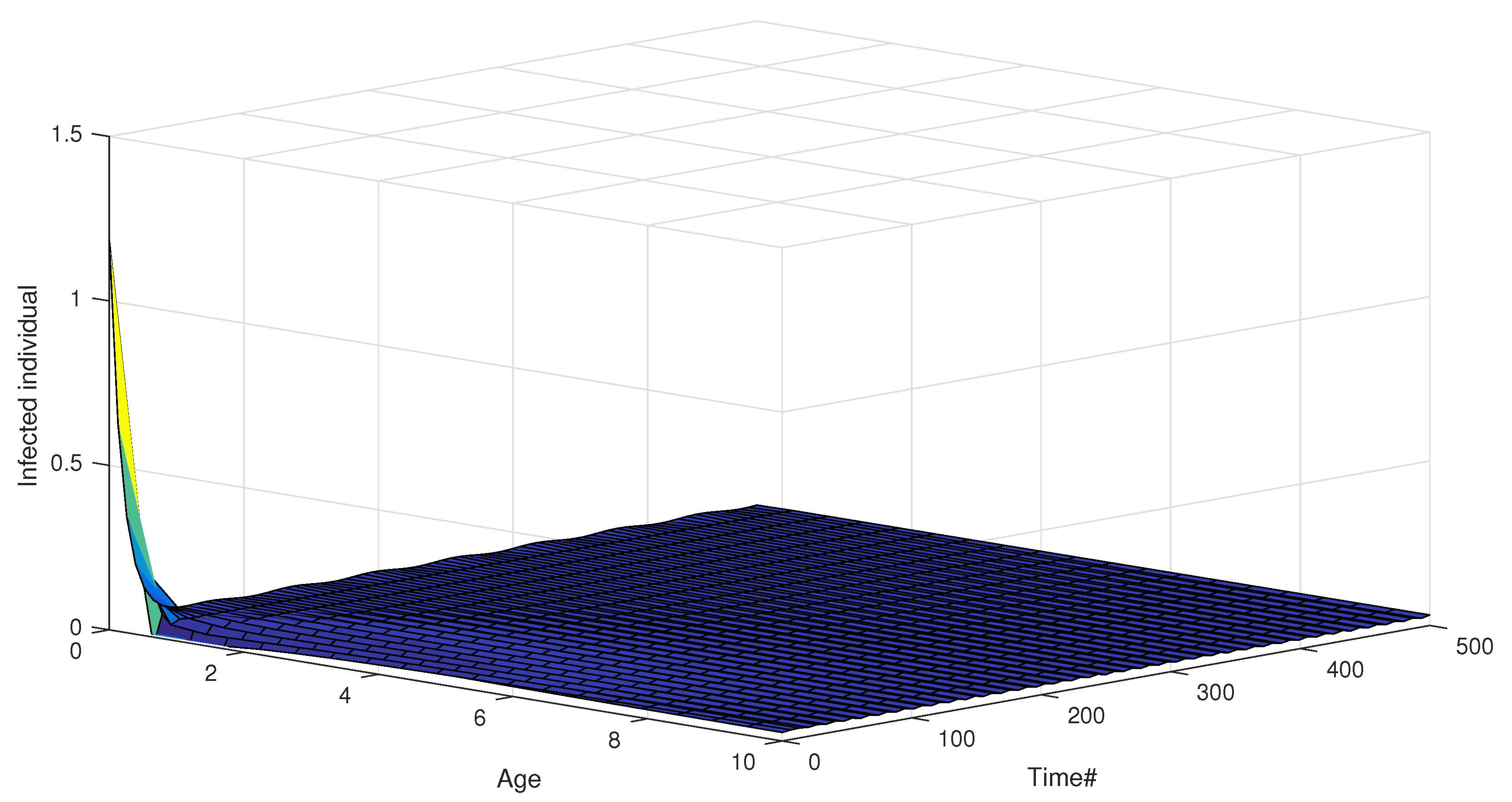
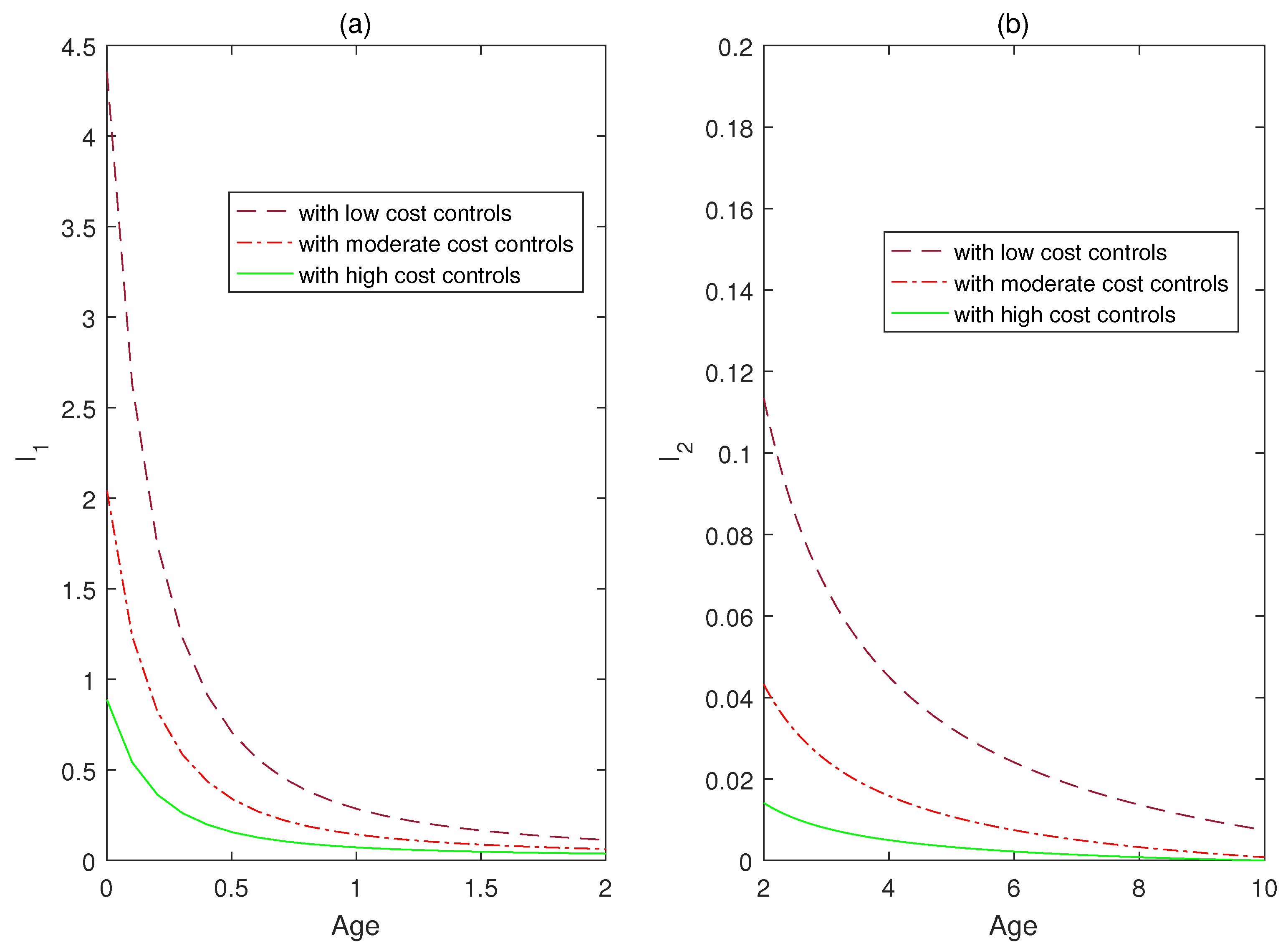
5. Numerical Simulation
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hyman, J.M.; Li, J.; Stanley, E.A. The differential infectivity and staged progression models for the transmission of HIV. Math. Biosci. 1999, 155, 77–109. [Google Scholar] [CrossRef]
- Guo, H.; Li, M. Global dynamics of a staged progression model for infectious diseases. Math. Biosci. Eng. 2006, 3, 513–525. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Xu, D.; Feng, Z. Analysis of a model with multiple infectious stages and arbitrarily distributed stage durations. Math. Model. Nat. Phenom. 2008, 3, 180–193. [Google Scholar] [CrossRef] [Green Version]
- Guo, H.; Li, M. Global dynamics of a staged progression model with amelioration for infectious diseases. J. Biol. Dyn. 2008, 2, 154–168. [Google Scholar] [CrossRef] [PubMed]
- Yuan, Z.; Zou, X. Global threshold property in an epidemic model for disease with latency spreading in a heterogeneous host population. Nonlinear Anal. Real World Appl. 2010, 11, 3479–3490. [Google Scholar] [CrossRef]
- Guo, H.; Li, M.; Shuai, Z. Global dynamics of a general class of multistage models for infectious disseises. SIAM J. Appl. Math. 2012, 72, 261–270. [Google Scholar] [CrossRef]
- Wang, J.L.; Liu, S.Q. The stability analysis of a general viral infection model with distributed delays and multi-staged infected progression. Commun. Nonlinear Sci. Numer. Simul. 2015, 20, 263–272. [Google Scholar] [CrossRef]
- Magal, P.; Clusky, C.C.M.; Webb, G. Lyapunov functional and global asymptotic stability for an infection age model. Appl. Anal. 2010, 89, 1109–1140. [Google Scholar] [CrossRef]
- Cluskey, C.C.M. Global stability for an SEI epidemiological model with continuous age structure in the exposed and infectious classes. Math. Biosci. Eng. 2012, 9, 819–841. [Google Scholar]
- Huang, G.; Liu, X.; Takeuchi, Y. Lyapunov functions and global stability for age structured HIV infection model. SIAM J. Appl. Math. 2012, 72, 25–38. [Google Scholar] [CrossRef]
- Shen, M.; Xiao, Y.; Rong, L. Global stability of an infection-age structured HIV-1 model linking within-host and between-host dynamics. Math. Biosci. 2015, 263, 37–50. [Google Scholar] [CrossRef]
- Cluskey, C.C.M. Global stability for an SEI model of infectious disease with age structure and immigration of infected. Math. Biosci. Eng. 2016, 13, 381–400. [Google Scholar]
- Feng, Z.L.; Huang, W.Z.; Chavez, C.C. Global behavior of a multi-group SIS epidemic model with age structure. J. Differ. Equ. 2005, 218, 292–324. [Google Scholar] [CrossRef] [Green Version]
- Kuniya, T. Global stability analysis with a discretization approach for an age-structured multi-group SIR epidemic model. Nonlinear Anal. Real World Appl. 2011, 12, 2640–2655. [Google Scholar] [CrossRef]
- Shen, M.; Xiao, Y. Global stability of a multi-group SVEIR epidemiological model with the vaccination age and infection age. Acta Appl. Math. 2016, 144, 137–157. [Google Scholar] [CrossRef]
- Liu, Z.J.; Hu, J.; Wang, L.W. Modelling and analysis of global resurgence of mumps: A multi-group epidemic model with asymptomatic infection, general vaccinated and exposed distributions. Nonlinear Anal. Real World Appl. 2017, 37, 137–161. [Google Scholar] [CrossRef]
- Cherif, A.; Dyson, J.; Maini, P.K.; Gupta, S. An age-structured multi-strain epidemic model for antigenically diverse infectious diseases: A multi-locus framework. Nonlinear Anal. Real World Appl. 2017, 34, 275–315. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.X.; Guo, H.B. Global analysis of age-structured multi-stage epidemic models for infectious diseases. Appl. Math. Comput. 2018, 337, 214–233. [Google Scholar] [CrossRef]
- Zhang, S.X.; Guo, H.B.; Smith, R. Dynamical analysis for a hepatitis B transmission model with immigration and infection age. Math. Biosci. Eng. 2018, 15, 1291–1313. [Google Scholar] [CrossRef] [Green Version]
- Baiya, V.P.; Tripathi, J.P.; Kakkar, V.; Wang, J.S.; Sun, G.Q. Global dynamics of a multi-group SEIR epidemic model with infection age. Chin. Ann. Math. Ser. B 2021, 42, 833–860. [Google Scholar] [CrossRef]
- Luo, Y.T.; Tang, S.T.; Teng, Z.D.; Zhang, L. Global dynamics in a reaction diffusion multi-group SIR epidemic model with nonlinear incidence. Nonlinear Anal. Real World Appl. 2019, 50, 365–385. [Google Scholar] [CrossRef]
- Liu, R.; Wu, J.; Zhu, H. Media/psychological impact on multiple outbreaks of emerging infectious diseases. Comput. Math. Methods Med. 2007, 8, 153–164. [Google Scholar] [CrossRef] [Green Version]
- Cui, J.; Tao, X.; Zhu, H. An SIS infection model incorporating media coverage. Rocky Mt. J. Math. 2008, 38, 1323–1334. [Google Scholar] [CrossRef]
- Funk, S.; Gilad, E.; Jansen, V.A.A. Endemic disease, awareness and local behavioural response. J. Theor. Biol. 2010, 264, 501–509. [Google Scholar] [CrossRef] [Green Version]
- Nyabadza, F.; Chiyaka, C.; Mukandavire, Z.; Musekwa, S.D.H. Analysis of an HIV/AIDS model with public health information campaigns and individual withdrawal. J. Biol. Syst. 2010, 18, 357–375. [Google Scholar] [CrossRef]
- Misra, A.K.; Sharma, A.; Shukla, J.B. Modeling and analysis of effects of awareness programs by media on the spread of infectious diseases. Math. Comput. Model. 2011, 53, 1221–1228. [Google Scholar] [CrossRef]
- Kim, M.; Yoo, B.K. Cost-effectiveness analysis of a television campaign to promote seasonal influenza vaccination among the elderly. Value Health 2015, 18, 622–630. [Google Scholar] [CrossRef] [Green Version]
- Agaba, G.O.; Kyrychko, Y.N.; Blyuss, K.B. Mathematical model for the impact of awareness on the dynamics of infectious disease. Math. Biosci. 2017, 286, 22–30. [Google Scholar] [CrossRef] [Green Version]
- Xiao, Y.; Tang, S.; Wu, J. Media impact switching surface during an infectious disease outbreak. Sci. Rep. 2015, 5, 1–9. [Google Scholar] [CrossRef]
- Pharaon, J.; Bauch, C.T. The influence of social behaviour on competition between virulent pathogen strains. J. Theor. Biol. 2018, 455, 47–53. [Google Scholar] [CrossRef] [Green Version]
- Misra, A.K.; Rai, R.K.; Takeuchi, Y. Modelling the control of infectious diseases: Effects of TV and social media advertisements. Math. Biosci. Eng. 2018, 15, 1315–1343. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yang, A.J.; Song, B.J.; Yuan, S.L. Noise-induced transmitions in a non-smooth SIS epidemic model with media alert. Math. Biosci. Eng. 2021, 18, 745–763. [Google Scholar] [CrossRef] [PubMed]
- Shim, E. Optimal strategies of social distancing and vaccination against seasonal influenza. Math. Biosci. Eng. 2013, 10, 1615–1634. [Google Scholar] [CrossRef] [PubMed]
- Kumar, A.; Srivastava, P.K.; Takeuchi, Y. Modeling the role of information and limited optimal treatment on disease prevalence. J. Theor. Biol. 2017, 414, 103–119. [Google Scholar] [CrossRef]
- Yu, T.H.; Cao, D.Q.; Liu, S.Q. Epidemic model with group mixing: Stability and optimal control based on limited vaccination resources. Commun. Nonlinear Sci. Numer. Simul. 2018, 61, 54–70. [Google Scholar] [CrossRef]
- Zhang, H.T.; Yang, Z.W.; Pawelek, K.A.; Liu, S.Q. Optimal control strategies for a two group epidemic model with vaccination resource constraints. Appl. Math. Comput. 2020, 371, 124956. [Google Scholar] [CrossRef]
- Yang, J.Y.; Modnak, C.; Wang, J. Dynamical analysis and optimal control simulation for an age-structured cholera transmission model. J. Frankl. Inst. 2019, 356, 8438–8467. [Google Scholar] [CrossRef]
- Kumar, A.; Srivastava, P.K.; Dong, Y.P.; Takeuchi, Y. Optimal control of infectious disease: Information-induced vaccination and limited treatment. Phys. A Stat. Mech. Appl. 2020, 542, 123196. [Google Scholar] [CrossRef]
- Gashirai, T.B.; Musekwa-Hove, S.D.; Lolika, P.O.; Mushayabasa, S. Global stability and optimal control analysis of a foot and mouth disease model with vaccine failure and environmental transmission. Chaos Solitons Fractals 2020, 132, 109568. [Google Scholar] [CrossRef]
- Khatun, M.S.; Biswas, M.H.A. Optimal control strateties for preventing hepatitis B infection and reducing chronic liver cirrhosis incidence. Infect. Dis. Model. 2020, 5, 91–110. [Google Scholar]
- Wang, L.W.; Liu, Z.J.; Zhang, X.A. Global dynamics for an age-structured epidemic model with media impact and incomplete vaccination. Nonlinear Anal. Real World Appl. 2016, 32, 136–158. [Google Scholar] [CrossRef]
- Wang, L.W.; Liu, Z.J.; Xu, D.S.; Zhang, X.A. Global dynamics and optimal control of an influenza model with vaccination, media coverage and treatment. Int. J. Biomath. 2017, 10, 1750068. [Google Scholar] [CrossRef]
- Webb, G.F. Theory of Nonlinear Age-Dependent Population Dynamics; Marcel Dekker: New York, NY, USA, 1985; pp. 101–134. [Google Scholar]
- Thieme, H.R. Semiflows generated by Lipschitz perturbation of non-densely defined operators. Differ. Integral Equ. 1990, 3, 1035–1066. [Google Scholar]
- Hale, J. Theory of Functional Differential Equations; Springer: New York, NY, USA, 1977; pp. 25–76. [Google Scholar]
- Zhao, X.Q. Dynamical Systems in Population Biology; Springer: New York, NY, USA, 2003; pp. 112–149. [Google Scholar]
- Zhang, S.; Xu, X. A mathematical model for hepatitis B with infection-age structure. Discret. Contin. Dyn. Syst. Ser. B 2016, 21, 1329–1346. [Google Scholar] [CrossRef] [Green Version]
- Brezis, H. Functional Analysis, Sobolev Spaces and Partial Differential Equations; Springer: London, UK, 2011; pp. 36–89. [Google Scholar]
- Inaba, H. A semigroup approach to the strong ergodic theorem of the multistate stable population process. Popul. Stud. 1988, 1, 49–77. [Google Scholar] [CrossRef] [PubMed]
- Hale, J.; Waltman, P. Persistence in infinite dimensional systems. SIAM J. Appl. Math. 1989, 20, 388–395. [Google Scholar] [CrossRef]
- DelValle, S.Y.; Hyman, J.M.; Chitnis, N. Mathematical models of contact patterns between age groups for predicting the spread of infectious diseases. Math. Biosci. Eng. 2013, 10, 1475–1497. [Google Scholar]
- Fister, K.R.; Gaff, H.; Lenhart, S.; Numfor, E.; Schaefer, E.; Wang, J. Optimal Control of Vaccination in An Age-Structured Cholera Model, Mathematical and Statistical Modeling for Emerging and Re-Emerging Infectious Diseases; Springer: New York, NY, USA, 2016; pp. 221–248. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.; Chen, P.; Jia, Q.; Cheung, L. Effects of Media Coverage on Global Stability Analysis and Optimal Control of an Age-Structured Epidemic Model with Multi-Staged Progression. Mathematics 2022, 10, 2712. https://doi.org/10.3390/math10152712
Liu C, Chen P, Jia Q, Cheung L. Effects of Media Coverage on Global Stability Analysis and Optimal Control of an Age-Structured Epidemic Model with Multi-Staged Progression. Mathematics. 2022; 10(15):2712. https://doi.org/10.3390/math10152712
Chicago/Turabian StyleLiu, Chao, Peng Chen, Qiyu Jia, and Lora Cheung. 2022. "Effects of Media Coverage on Global Stability Analysis and Optimal Control of an Age-Structured Epidemic Model with Multi-Staged Progression" Mathematics 10, no. 15: 2712. https://doi.org/10.3390/math10152712
APA StyleLiu, C., Chen, P., Jia, Q., & Cheung, L. (2022). Effects of Media Coverage on Global Stability Analysis and Optimal Control of an Age-Structured Epidemic Model with Multi-Staged Progression. Mathematics, 10(15), 2712. https://doi.org/10.3390/math10152712





