Local Lagrange Exponential Stability Analysis of Quaternion-Valued Neural Networks with Time Delays
Abstract
:1. Introduction
2. Problem Formulation and Some Preliminaries
3. Existence and Stability of Multiple Equilibrium Points
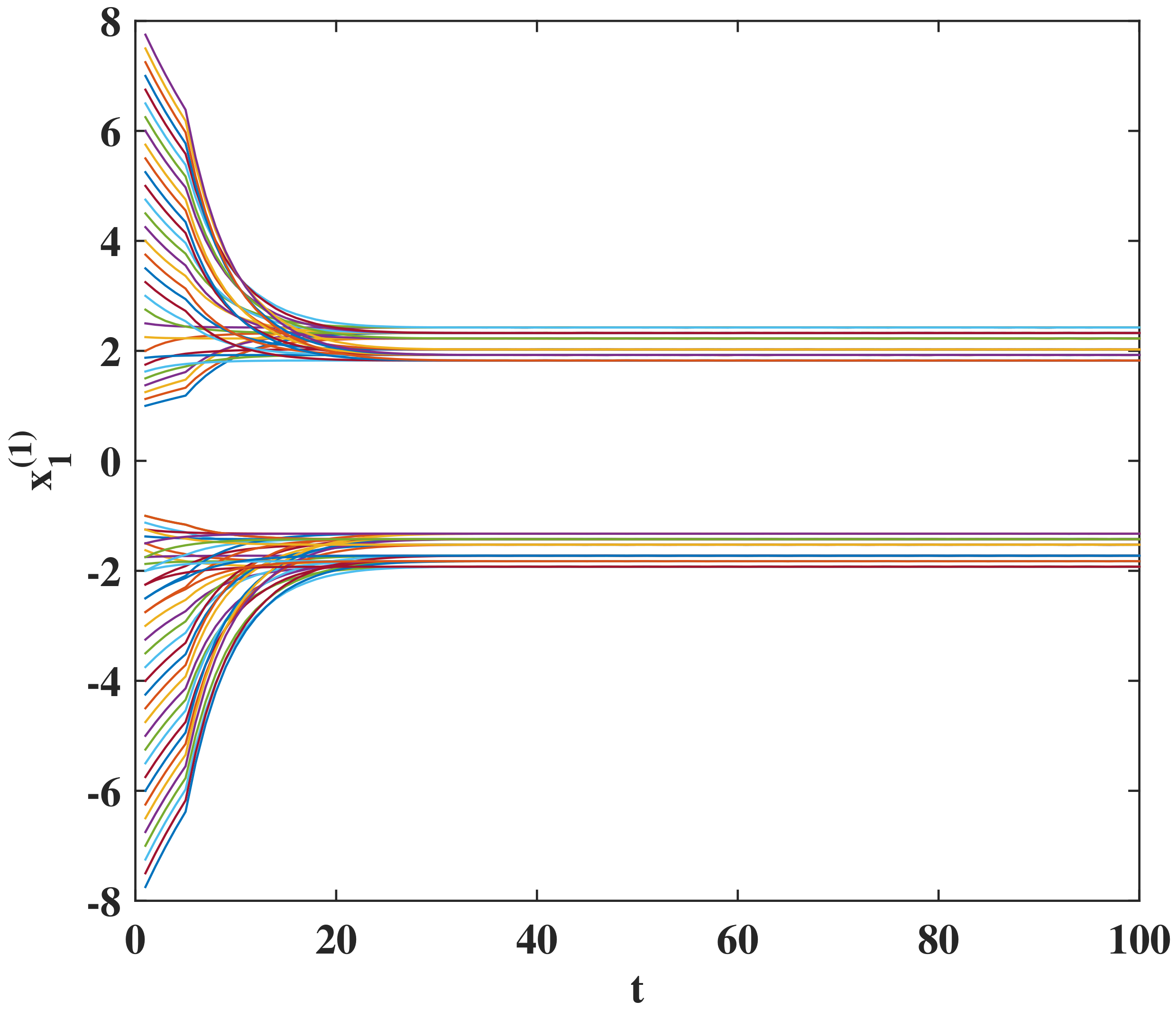
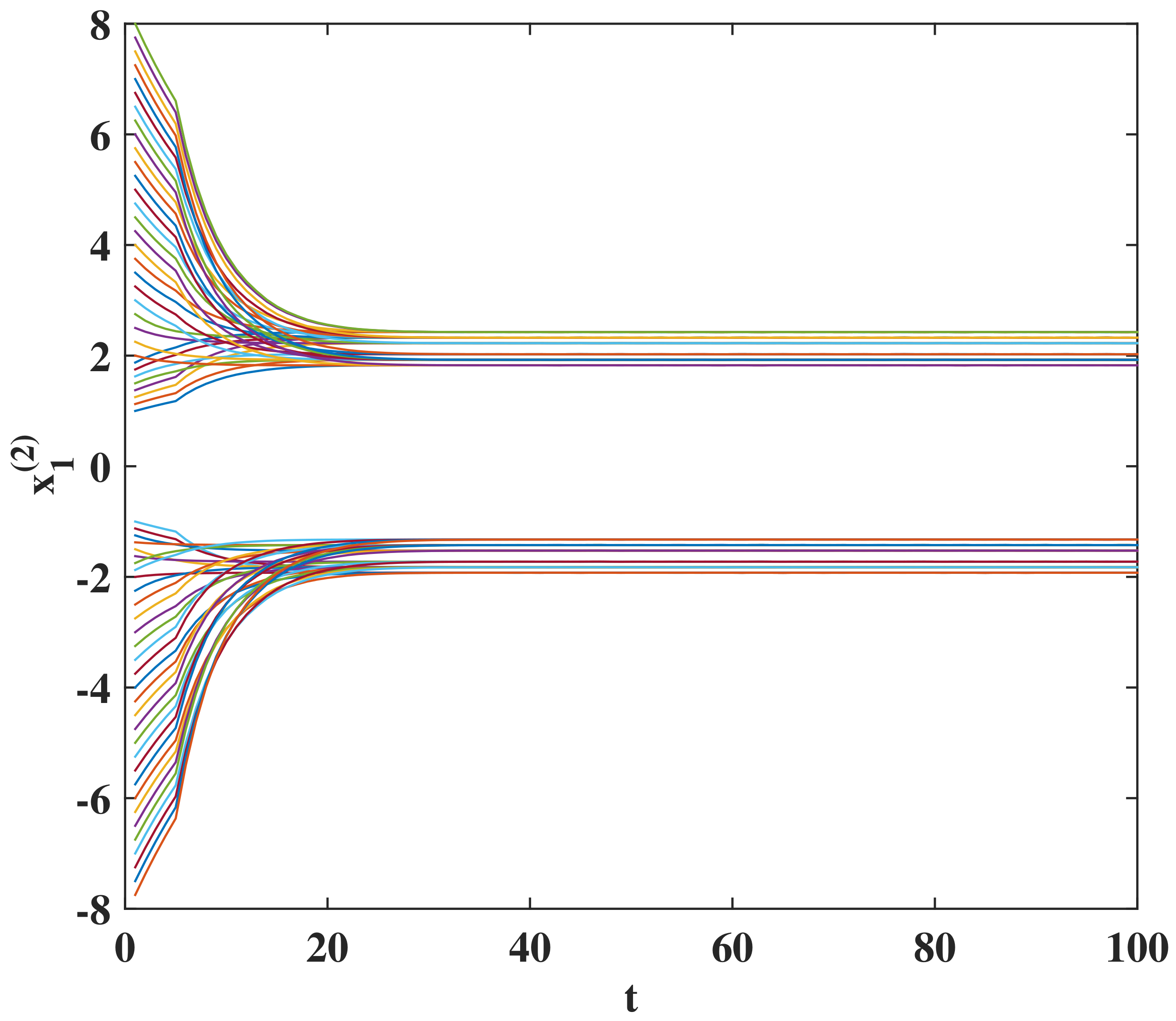
4. Simulation Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zheng, Y.; Sheng, W.; Sun, X.; Chen, S. Airline passenger profiling based on fuzzy deep machine learning. IEEE Trans. Neural Netw. Learn. Syst. 2017, 28, 2911–2923. [Google Scholar] [CrossRef] [PubMed]
- Zheng, Y.; Chen, S.; Xue, Y.; Xue, J. A Pythagorean-Type Fuzzy Deep Denoising Autoencoder for Industrial Accident Early Warning. IEEE Trans. Fuzzy Syst. 2017, 25, 1561–1575. [Google Scholar] [CrossRef]
- Zheng, Y.; Zhou, X.; Sheng, W.; Xue, Y.; Chen, S. Generative adversarial network based telecom fraud detection at the receiving bank. Neural Netw. 2018, 102, 78–86. [Google Scholar] [CrossRef] [PubMed]
- Cao, J.; Wang, J. Global asymptotic stability of a general class of recurrent neural networks with time-varying delays. IEEE Trans. Circuits Syst. I 2003, 50, 34–44. [Google Scholar]
- Cao, J.; Wang, J. Global asymptotic stability of recurrent neural networks with multiple time-varying delays. IEEE Trans. Neural Netw. 2008, 19, 855–873. [Google Scholar]
- Zhang, H.; Liu, Z.; Huang, G.; Liu, Z.; Wang, Z. Novel weighting-delay-based stability criteria for recurrent neural networks with time-varying delay. IEEE Trans. Neural Netw. 2010, 21, 91–106. [Google Scholar] [CrossRef]
- Wu, A.; Zeng, Z. Exponential stabilization of memristive neural networks with time delays. IEEE Trans. Neural Netw. Learn. Syst. 2012, 12, 1919–1929. [Google Scholar]
- Wu, A.; Zeng, Z. Dynamic behaviors of memristor-based recurrent neural networks with time-varying delays. Neural Netw. 2012, 36, 1–10. [Google Scholar] [CrossRef]
- Wu, A.; Zeng, Z. Lagrange stability of memristive neural networks with discrete and distributed delays. IEEE Trans. Neural Netw. Learn. Syst. 2014, 25, 690–703. [Google Scholar] [CrossRef]
- Wen, S.; Huang, T.; Yu, X.; Chen, M.; Zeng, Z. Aperiodic sampled-data sliding-mode control of fuzzy systems with communication delays via the event-triggered method. IEEE Trans. Fuzzy Syst. 2016, 24, 1048–1057. [Google Scholar] [CrossRef]
- Lakshmanan, S.; Prakash, M.; Lim, C.P.; Rakkiyappan, R.; Balasubramaniam, P.; Nahavandi, S. Synchronization of an inertial neural network with time-varying delays and its application to secure communication. IEEE Trans. Neural Netw. Learn. Syst. 2018, 29, 195–207. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Guo, R.; Liu, X.; Lin, C. Lagrange Exponential Stability of Complex-Valued BAM Neural Networks with Time-Varying Delays. IEEE Trans. Syst. 2020, 50, 3072–3085. [Google Scholar] [CrossRef]
- Liu, P.; Zeng, Z.; Wang, J. Multistability of recurrent neural networks with nonmonotonic activation functions and mixed time delays. IEEE Trans. Syst. 2016, 46, 512–523. [Google Scholar] [CrossRef]
- Song, Q.; Chen, X. Multistability Analysis of Quaternion-Valued Neural Networks With Time Delays. IEEE Trans. Neural Netw. Learn. Syst. 2018, 29, 5430–5440. [Google Scholar] [CrossRef] [PubMed]
- Zhang, F.; Zeng, Z. Multistability and Stabilization of Fractional-Order Competitive Neural Networks With Unbounded Time-Varying Delays. IEEE Trans. Neural Netw. Learn. Syst. 2021, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Zhang, F.; Huang, T.; Feng, D.; Zeng, Z. Multistability and robustness of complex-valued neural networks with delays and input perturbation. Neurocomputing 2021, 447, 319–328. [Google Scholar] [CrossRef]
- Zhang, F.; Huang, T.; Feng, D.; Zeng, Z. Multistability of delayed fractional-order competitive neural networks. Neural Netw. 2021, 140, 325–335. [Google Scholar] [CrossRef]
- Cheng, C.Y.; Lin, K.H.; Shih, C.W. Multistability and convergence in delayed neural networks. Phys. D Nonlin. Phenom. 2007, 225, 61–74. [Google Scholar] [CrossRef]
- Zeng, Z.; Huang, T.; Zheng, W. Multistability of recurrent neural networks with time-varying delays and the piecewise linear activation function. IEEE Trans. Neural Netw. 2010, 21, 1371–1377. [Google Scholar] [CrossRef]
- Wang, L.L.; Chen, T.P. Multistability of neural networks with Mexican-hat-type activation functions. IEEE Trans. Neural Netw. Learn. Syst. 2012, 23, 1816–1826. [Google Scholar] [CrossRef]
- Huang, Y.; Zhang, H.; Wang, Z. Multistability and multiperiodicity of delayed bidirectional associative memory neural networks with discontinuous activation functions. Appl. Math. Comput. 2012, 219, 899–910. [Google Scholar] [CrossRef]
- Huang, Y.; Zhang, H.; Wang, Z. Dynamical stability analysis of multiple equilibrium points in time-varying delayed recurrent neural networks with discontinuous activation functions. Neurocomputing 2012, 91, 21–28. [Google Scholar] [CrossRef]
- Huang, Y.; Zhang, H.; Wang, Z. Multistability of complex-valued recurrent neural networks with real-imaginary-type activation functions. Appl. Math. Comput. 2014, 229, 187–200. [Google Scholar] [CrossRef]
- Zhang, F.; Zeng, Z. Multiple ψ-Type Stability of Cohen-Grossberg Neural Networks With Both Time-Varying Discrete Delays and Distributed Delays. IEEE Trans. Neural Netw. Learn. Syst. 2019, 30, 566–579. [Google Scholar] [CrossRef]
- Cai, Z.; Huang, L.; Zhang, L. Finite-time synchronization of master-slave neural networks with time-delays and discontinuous activations. Appl. Math. Model. 2017, 47, 208–226. [Google Scholar] [CrossRef]
- Wang, S.; Zhang, Z.; Lin, C.; Chen, J. Fixed-time synchronization for complex-valued BAM neural networks with time-varying delays via pinning control and adaptive pinning control. Chaos Solitons Fractals 2021, 153, 111583. [Google Scholar] [CrossRef]
- Wei, X.; Zhang, Z.; Lin, C.; Chen, J. Synchronization and anti-synchronization for complex-valued inertial neural networks with time-varying delays. Appl. Math. Comput. 2021, 403, 126194. [Google Scholar] [CrossRef]
- Wei, R.; Cao, J.; Huang, C. Lagrange exponential stability of quaternion-valued memristive neural networks with time delays. Math. Methods Appl. Sci. 2020, 43, 7269–7291. [Google Scholar] [CrossRef]
- Xiao, J.; Cao, J.; Zhong, S.; Wen, S. Novel methods to finite-time Mittag-Leffler synchronization problem of fractional-order quaternion-valued neural networks. Inf. Sci. 2020, 526, 221–244. [Google Scholar] [CrossRef]
- Wu, Z. Multiple asymptotic stability of fractional-order quaternion-valued neural networks with time-varying delays. Neurocomputing 2021, 448, 301–312. [Google Scholar] [CrossRef]
- Udhayakumar, K.; Rakkiyappan, R.; Liu, X.; Cao, J. Mutiple psi-type stability of fractional-order quaternion-valued neural networks. Appl. Math. Comput. 2021, 401, 126092. [Google Scholar]
- Gopalsamy, K. Stability and Oscillations in Delay Differential Equations of Population Dynamics; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1992. [Google Scholar]
- Cao, J.; Wang, J. Absolute exponential stability of recurrent neural networks with Lipschitz-continuous activation functions and time delays. Neural Netw. 2004, 17, 379–390. [Google Scholar] [CrossRef]
- Liao, X.; Luo, Q.; Zeng, Z.; Guo, Y. Global exponential stability in Lagrange sense for recurrent neural networks with time delays. Nonlinear Anal. Real World Appl. 2008, 9, 1535–1557. [Google Scholar] [CrossRef]
- Aladdin, A.M.; Rashid, T.A. A New Lagrangian Problem Crossover: A Systematic Review and Meta-Analysis of Crossover Standards. arXiv 2022, arXiv:2204.10890. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dong, W.; Huang, Y.; Chen, T.; Fan, X.; Long, H. Local Lagrange Exponential Stability Analysis of Quaternion-Valued Neural Networks with Time Delays. Mathematics 2022, 10, 2157. https://doi.org/10.3390/math10132157
Dong W, Huang Y, Chen T, Fan X, Long H. Local Lagrange Exponential Stability Analysis of Quaternion-Valued Neural Networks with Time Delays. Mathematics. 2022; 10(13):2157. https://doi.org/10.3390/math10132157
Chicago/Turabian StyleDong, Wenjun, Yujiao Huang, Tingan Chen, Xinggang Fan, and Haixia Long. 2022. "Local Lagrange Exponential Stability Analysis of Quaternion-Valued Neural Networks with Time Delays" Mathematics 10, no. 13: 2157. https://doi.org/10.3390/math10132157
APA StyleDong, W., Huang, Y., Chen, T., Fan, X., & Long, H. (2022). Local Lagrange Exponential Stability Analysis of Quaternion-Valued Neural Networks with Time Delays. Mathematics, 10(13), 2157. https://doi.org/10.3390/math10132157





